Entire positive $k$-convex solutions to $k$-Hessian type equations and systems

1.   Introduction

The control problems of nonlinear systems have received a great deal of attention, and a considerable amount of literatures have been published ^[1,2,3,4,5]. A two-layer neural networks (NNs) based robust control for nonlinear induction motors is proposed in ^[1]. In ^[2] the unknown nonlinear items in the dynamic model of robotic manipulators are identified by NNs. Dynamic properties and optimal stabilization issues of fractional-order (FO) self-sustained electromechanical seismograph system is investigated in ^[3]. A neural adaptive control scheme is raised for a class of uncertain multi-input/multi-output nonlinear systems ^[4] and only one learning parameter is updated in the parameter identification. And coupled FO chaotic electromechanical devices are studied in ^[5]. Specifically, an adaptive dynamic programming policy is proposed to address the zero-sum differential game issue in the optimal neural feedback controller. Obviously, approximation-based adaptive backstepping control has been widely utilized in designing controllers for various nonlinear systems ^[6,7,8]. However, traditional approximation-based adaptive backstepping technique faces two crucial issues that hinder its application. The first issue is so-called "EOC" arising from the derivations of virtual control input ^[7]. The second one is a large computational burden caused by high precision approximation requirements ^[8].

For the backstepping technique, the design of the control law relies on intermediate state variables as virtual control signals. The controller of each subsystem requires the virtual control signal and its derivative. The lower-order derivatives of the virtual control signals are likely simple in theory, but the higher-order derivatives in higher-order systems are quite complex, which is called the "EOC" problem. For handling the "EOC" problem, a first-order filter was used to calculate the virtual control signal derivatives in each recursive step ^[9]. This technique is the so-called dynamic surface control (DSC). The utilization of the first-order filter overcomes the EOC problem, but its own characteristics lead to the derivative errors of virtual control signal, which will certainly affect the tracking performance of the system. Based on this, a modified scheme of the DSC method named command filter based control (CFC) method was proposed in ^[10]. On the one hand, the EOC problem is avoided by substituting a first-order filter with a second-order one to obtain the derivatives of the virtual control signal. On the other hand, the filter errors are compensated by a constructed error compensation mechanism for obtaining better tracking performance of the system. Moreover, constructing an effective error compensation mechanism needs to be further studied.

For the approximation-based control, the NNs or fuzzy logic systems (FLSs) are utilized to approximate unknown functions and external disturbances for ensuring better tracking performance ^[11,12], which is also a kind of learning control ^[13]. In ^[11] a NNs-based approximator is utilized to solve the unmodeled dynamics of the system. FLSs are employed to identify unknown functions existing in systems ^[12]. Iterative learning control schemes are designed to suppress the vibrations in bending and twisting of the flexible micro aerial vehicle ^[13]. The approximation accuracy improves with the increase of the number of neural network nodes or fuzzy rules in general, but it also significantly increases the number of estimated parameters. Therefore, the burdens of computation required for online learning will become very heavy. For decreasing the computational burden of approximation-based control, a tuning-function is inserted in the controller of strict-feedback systems ^[14,15], in which the number of parameter to be updated is the same as the number of unknown parameters. Recently, a kind of one-parameter estimation approach is proposed in ^[16,17], which needs only one parameter to be updated online and can significantly reduce the computational burden. Nevertheless, the mentioned control schemes do not take issues of input constraints, state constraints and prescribed performance into account. Therefore, for applying to a broader range of control problems with security, reliability and performance consideration in reality, further research is needed.

In the real world, many physical constraints are generated with security and reliability consideration, such as the output of MEMS resonator ^[18], the state constraint of aircraft engine ^[19] and the input constraint of magnetic-field electromechanical transducer ^[20]. Obviously, severe security matters, performance degradation, and other troubles can be caused without considering these constraints. For the issue of input constraint, a considerable amount of literatures have been published about it ^[21,22,23]. A non-smooth and piecewise input constraint model is described in ^[21]. Furthermore, the model in ^[22] is a smooth but piecewise function. The input constraint nonlinearity is tackled by asymmetric smooth input constraint model in ^[23]. For the issues of output constraint and state constraint, Barrier Lyapunov Function (BLF) is seen as an effective tool, and a significant number of typical works have been published including symmetric BLF ^[24,25] and asymmetric BLF ^[26,27]. However, the aforementioned BLF-based controllers have the following three drawbacks: 1) Discontinuous actions exist when constructing asymmetric BLF deals with asymmetric constraints; 2) Output/state constraints are achieved indirectly through error constraint, which leads to a more conservative initial output and state; 3) It is not allowed to handle both constrained and unconstrained systems without changing the controller structure. Although the integral BLF (IBLF)-based approach is possible to tackle output/state constraints directly ^[28], it can only overcome the disadvantages 1) and 2). By constructing a novel state transformation nonlinear function in ^[29,30], all those shortcomings can be overcome simultaneously. However, in practical applications, ensuring system security and reliability is the foundation, and achieving high performance control of the system is surely the ultimate goal. To be the best of our knowledge, no relevant results have been reported which can overcome the all above drawbacks and ensure safety reliability and high performance of systems simultaneously.

In this paper, with consideration of security, reliability and high performance, a LAFN-PPC of strict-feedback systems considering asymmetric full-state and input constraints is raised. In the controller design procedure, the constrained system is transformed into an unconstrained system using one-to-one nonlinear state transformation technique. One-to-one nonlinear error transformation technique is used to guarantee the prescribed performance. Furthermore, the unknown control direction and the input constraint nonlinearity are resolved by Nussbaum gain technique simultaneously. By introducing command filter and an error compensation mechanism, the constructed scheme can not only overcomes the so-called "EOC" problem, but also reduces filter errors. Moreover, the maximum values of the norm of optimal weight vector in FWNN is constructed as a virtual parameter, and the only one virtual parameter is estimated instead of the optimal weight vectors (OWVs). Regardless of the order of the system and the dimension of the system parameters, only one parameter is required to be updated online, which significantly reduces the computational burdens. The major contributions comparing with the existing ones are listed as:

1) In order to ensure the controlled systems with higher security, faster response speed and lower tracking error simultaneously, we combine a simple state transformation function with an error transformation function. All states and tracking error are always in symmetric or asymmetric prescribed bounds. Compared with the BLF-based methods ^{[24,25,26,27]}, the LAFN-PPC can overcome all the three drawbacks, because we utilize the state transformation function instead of BLF, by which the constrained system is converted to an unconstrained system. In contrast to state transformation based methods ^[29,30], the tracking error is always remained within the prescribed performance bound by using an error transformation function.

2) By using command filtering to get the virtual control signal derivatives, the "EOC" problem of traditional backstepping method is overcome, the filter error caused by command filter is compensated by the carefully constructed error compensation mechanism. Compared with ^[1,2,3,4,5], the method we take only requires the reference signal and its first derivative, which greatly reduces the amount of calculation and meets many practical engineering requirements.

3) To significantly improve the computational efficiency of FWNN-based approximator and to replace estimating the OWVs in each step of backstepping, we construct the maximum value of the norm of OWVs in the FWNN as a virtual parameter. Only one virtual parameter needs to be estimated in the FWNN-based approximator, with this one-parameter estimation-based approach, the number of parameters updated online is independent of the order of the system and the dimension of OWVs, and the computational burden is significantly reduced, while the computational efficiency is significantly improved.

2.   System formulation and preliminaries

2.1.   System descriptions

The considered strict-feedback systems with input constraint nonlinearity are given as

where ${\overline x }_{i} = {\left[{x}_{1}, \dots, {x}_{i}\right]}^{T}\in {R}^{i}$, $i = 1, \ldots, n$ and $v \in R$ are the states and the system input. $y \in R$ denotes the system output. $x = {\left[{x}_{1}, \dots, {x}_{n}\right]}^{T}\in {R}^{n}$ are the whole states of the system, ${f_i}\left({{{\bar x}_i}, {\ell _i}} \right)$, $i = 1, \dots, n$ are unknown smooth functions, Specifically, ${f_i}\left({{{\bar x}_i}, {\ell _i}} \right)$ denote system uncertainties and external disturbances, ${\ell}_{i}$ are the unknown constant parameters inseparable from ${f_i}\left({{{\bar x}_i}, {\ell _i}} \right)$. $g$ denotes the unknown control gain. Here, $u$ is the actual control signal which is subjected to the input constraint nonlinearity $C\left(v\right):R\to R$. And the input constraint nonlinearity will be given later. In this paper, ${x}_{i}\left(t\right)$ are constrained in the open sets $\left(-{\underline \kappa }_{i}, {\overline \kappa }_{i}\right)$, i.e., ${x}_{i}\left(t\right)\in {{\mathit{\Omega}} }_{i}$.

As is known that input saturation of actuator is a common problem. The input saturation nonlinearity can seriously affect the safety and performance of the system. How to cope with the saturation nonlinearity has become an urgent and challenging research issue. In this paper, we take the input constraint nonlinearity into account. Mostly, the input constraint nonlinearity ^[21] can be expressed as

where ${u}^{+}$ and ${u}^{-}$ are the upper/lower bounds of $u\left(t\right)$.

To simplify the design of the control, we can define $\Delta \left(v\right) = u-{c}_{v}v$, where ${c}_{v}$ is a positive constant. We can rewrite the input constraint model as:

Based on the above strict-feedback systems, the control object of this paper is to design a LAFN-PPC for system (2.1) to realize the following purposes:

(a) All signals of the system are in the sense of uniformly ultimate boundedness.

(b) The input signal and full state variables can be strictly restricted in asymmetric upper and lower bounds.

(c) The output signal can track the reference signal very well. And the output tracking error can be strictly restricted in upper and lower bounds.

2.2.   FWNN

The FWNN ^[20] has strong power in function approximation, which consists of a series of fuzzy IF-THEN rules as:

If ${{\rm Z}_1}$ is ${\rm M}_1^j$ and … ${{\rm Z}_n}$ is ${\rm M}_n^j$, then ${\widehat{f}}_{s}$ is ${\omega }_{j}$, $j = 1, \dots {N}_{s}$, where ${\rm M}_i^j$, $i = 1, \dots, {n}_{s}$, $j = 1, \dots, {N}_{s}$, is $jth$ member function for $ith$ input, ${n_s}$ and ${N_s}$ represent the number of inputs and rulers (fuzzy logical system), respectively.

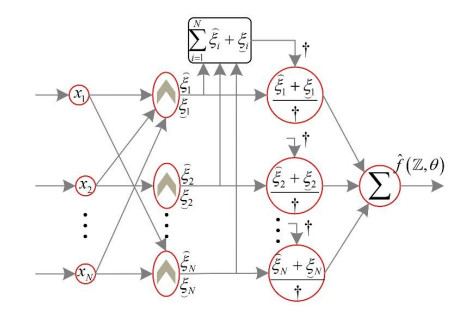
The FWNN shown in Figure 1 consists of five lawyers, including an input layer, a fuzzification layer, a membership layer, a rule layer, and an output layer. The firing degrees of rulers are defined as

where $i = 1, \ldots, N$, $j = 1, \ldots, n$, $n$ and $N$ denote the number of inputs and rulers (neural network system). $c_i^j$ and $\omega _i^j$ represent the center and width of member function.

The firing degrees are defined as

The FWNN can be described as

where $f\left(\theta, \mathbb{Z}\right)$ is a continuous function which is bounded in closed compact set $\mathbb{\mho }\to {R}^{n}$, $\mathbb{Z} = \left[{Z}_{1}, {Z}_{2}, \cdots, {Z}_{N}\right]\in \mho \subset {R}^{n}$ is the input vector, $n$ is the input dimension of neural network. $\theta = {\left[{{\theta _1}, {\theta _2}, \cdots {\theta _N}} \right]^T} \in {R^N}$ denotes the weight vector, and $N > 1$ is the node number of neuron. and $\xi \left(\mathbb{Z}\right) = {\left[{\xi }_{1}\left(\mathbb{Z}\right), {\xi }_{2}\left(\mathbb{Z}\right), \cdots, {\xi }_{N}\left(\mathbb{Z}\right)\right]}^{T}\in {R}^{N}$ indicates the basic function vector. $\varepsilon$ is the estimation error. And there is a positive constant ${\varepsilon _{Mi}}$ which satisfies $\left| {{\varepsilon _i}} \right| \leqslant {\varepsilon _{Mi}}$, $i = 1, \ldots, n$.

Lemma 1 ^[20]. Continuous function $f\left(\mathbb{Z}\right)$ is defined on a compact set $\mathbb{\mho }$. And for $\varepsilon \left(\mathbb{Z}\right) > 0$, there is a FWNN satisfying

The optimal parameter $\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{\theta }$ is equal to $\underset{\theta \in {\mho }_{\theta }}{argmin}\left[\underset{\mathbb{Z}\in \mathbb{\mho }}{sup}\left|f\left(\mathbb{Z}\right)-\widehat{f}\left(\mathbb{Z}, \theta \right)\right|\right]$, where ${\mho }_{\theta }$ is a compact set, and $\tilde \theta = \overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{\theta } - \theta$, where $\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{\theta }$ represents the estimation of $\theta$.

2.3.   State transformation

For resolving physical constraints generated with security and reliability consideration, the following state transformation function ^[29,30] is introduced to achieve asymmetric constraints, symmetric constraints and no constraints on states simultaneously in a unified form:

where ${{\underline{\kappa }}_{i}}$ and ${\bar \kappa _i}$ are positive constants, the initial state ${x_i}\left(0 \right) \in {{{\Omega}} _i}$, $i = 1, 2, \ldots, n$. It is obvious that if the states ${x_i}\left(t \right) \to - {{\underline{\kappa }}_{i}}$ or ${x_i}\left(t \right) \to {\bar \kappa _i}$, the transformed states ${s_i}\left(t \right) \to \pm \infty$. Therefore, the state transformation function can constrain ${x_i}\left(t \right)$ within the open sets $\left({ - {{\underline{\kappa }}_{i}}, {{\bar \kappa }_i}} \right)$, i.e., ${x_i}\left(t \right) \in {{{\Omega}} _i}$.

Remark 1. If asymmetric constraints need to be addressed, i.e., let ${{\underline{\kappa }}_{i}} \ne {\bar \kappa _i}$, $i = 1, 2, \ldots, n$. Else symmetric constraints need to be tackled, i.e., let ${\kappa _i} = {{\underline{\kappa }}_{i}} = {\bar \kappa _i}$. Then (2.5) becomes

If no constraints need to be handled, let ${\kappa _i} \to + \infty$. It is clear that ${s_i}\left(t \right) \to {x_i}\left(t \right)$, i.e.,

The above state transformation function can solve the control problems with asymmetric, symmetric and no state constraints in a unified form. Furthermore, it can handle above three kinds of control problems without changing adaptive laws. Figure 2 shows that the state transformation function can constrain ${x_i}\left(t \right)$ within the open set $\left({ - {{\underline{\kappa }}_{i}}, {{\bar \kappa }_i}} \right)$ (For the symmetric one: ${{\underline{\kappa }}_{i}} = {\bar \kappa _i} = 4$; For the asymmetric one: ${{\underline{\kappa }}_{i}} = 4$, ${\bar \kappa _i} = 6$).

The state transformation function can be rewritten as

where

Remark 2. ${\eta _i}$ are also bounded in the sets ${{{\Omega}} _{\eta i}}$, i.e., $0 < {\eta _i} \leqslant {\bar \eta _i}$, and ${\bar \eta _i} = \frac{{{{\left({{{\underline{\kappa }}_{i}} + {{\bar \kappa }_i}} \right)}^2}}}{{4{{\underline{\kappa }}_{i}}{{\bar \kappa }_i}}}$, $i = 1, 2, \ldots, n$.

Proof. See Appendix A. Taking the time derivative of the state transformation function (2.5):

where

The constrained system is converted to an unconstrained system as

2.4.   Error transformation

For achieving the prescribed performance and guaranteeing the transformed output tracking error to converge within the prescribed performance bounds. Firstly, we define transformed output tracking error, virtual control errors and the command filters ^[10] as:

where ${z_1}$ is the transformed output tracking error, ${e_i}$ are intermediate tracking errors, ${\alpha _{ic}}$ are the outputs of the command filters, and the virtual control${\alpha _i}$ are the inputs of the command filters. $\omega$ and $\varpi \in \left({0, 1} \right)$ are positive design parameters. The initial values satisfy ${\alpha _{jc}}\left(0 \right) = {\alpha _{j - 1}}\left(0 \right)$ and ${\alpha _{jc, j}}\left(0 \right) = 0$ for $j = 2, 3, \ldots, n$. ${\alpha _{1c}}$ and its time derivative are calculated as:

where ${y_r}$ is the reference signal.

To ensure the transformed output tracking error ${z_1}$ strictly converges in the prescribed performance region during the whole time, we define

where the design parameters $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{k}$ and $\bar k$ are positive constants, and

is the prescribed performance function, $\mu \left(0 \right) = {\mu _0}$, $0 < {\mu _\infty } < {\mu _0}$. The parameter $\hbar$ is also a positive constant.

Remark 3. It is obvious that the transformed output tracking error is the output tracking error of unconstrained system and is restricted in the prescribed domain, i.e. ${z_1}\left(t \right) \in \left({ - \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{k} \mu \left(t \right), \bar k\mu \left(t \right)} \right)$. However, our control objective is that the output tracking error of original constrained system ${e_r} = {x_1} - {y_r}$ is restricted in a prescribed domain. The output tracking error is bounded in a set

Proof. See Appendix B. The error transformation can be defined as:

where ${\mathbb{Z}}_{1}\left(t\right)$ is the transformed error and $\Upsilon \left({\mathbb{Z}}_{1}\right)$ is defined as:

Remark 4. It is obvious that $\Upsilon \left(t \right)$ is strictly constrained in symmetric or asymmetric domain $\left({ - \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{k}, \bar k} \right)$ showed in Figure 3 (For the symmetric one: $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{k} = \bar k = 0.5$. For the asymmetric one: $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{k} = 0.5$, $\bar k = 0.7$). It means that the error transformation function can deal with the issues considering symmetric or asymmetric exponential constraint simultaneously.

According to (2.15) and (2.16), one can obtain:

and

where

We finally define the transformed tracking error as

3.   Controller design

In order to reduce the errors caused by the command filters, we introduce compensation signals ${\varsigma _i}$, and the compensation errors can be defined as ${v_i} = {e_i} - {\varsigma _i}$, $i = 1, \ldots, n$.

FWNN is used to approximate the system uncertainties and external disturbances ${f}_{i}$:

For facilitating adaptive law design, we define

where ${\bar \theta _i}$ are unknown virtual parameters with ${\bar \theta _i} < \theta$. ${\theta _i}$ is the ideal constant weight of $ith$ NN and ${\varepsilon _{Mi}}$ is the upper bound of approximation error ${\varepsilon _i}$.

Assumption 1. The reference trajectory ${y_r}$ is continuous, and satisfies

${\Xi _r}$ is a known compact set

${B_r}$ is a known positive constant. Furthermore, $- {\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{\kappa } _1} < {y_r} < {\bar \kappa _1}$ holds.

Assumption 2. There are some constants $\bar \Delta$, $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{g}$ and $\bar g$ which satisfied $\Delta \left(v \right) \leqslant \bar \Delta$ and $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{g} \leqslant \left| g \right| \leqslant \bar g$.

For solving the unknown gain and input constraint of systems simultaneously, Nussbaum function is introduced ^[10]. Obviously, a Nussbaum-type function $N\left(\chi \right)$ needs to hold following the properties:

In general, $\ln \left({\chi + 1} \right)\cos \sqrt {\ln \left({\chi + 1} \right)}$, ${\chi ^2}\cos \left(\chi \right)$ and ${e^{{\chi ^2}}}\cos \left({{{\pi \chi }/ 2}} \right)$ are commonly used Nussbaum-type function, and $N\left(\chi \right) = {\chi ^2}\cos \left(\chi \right)$ is employed in this paper.

Lemma 2 ^[10]. Let $V\left(\cdot \right)$ and $\chi \left(\cdot \right)$ be smooth functions defined on $\left[{0, {t_f}} \right)$ with $V\left(t \right) \geqslant 0$. $\forall t \in \left[{0, {t_f}} \right)$, and $N\left(\cdot \right)$ is a smooth Nussbaum-type function. If the following inequality holds:

where ${g_f}$ is a non-zero parameter and ${c_0}$ is an appropriate constant, then $V\left(t \right)$, $\chi \left(t\right)$ and $\int_0^t {\left({gN\left(\chi \right) + 1} \right)\dot \chi d\tau }$ must be bounded on $\left[{0, {t_f}} \right)$.

Step 1. Taking the time derivative of ${e_1}$ based on (2.17)–(2.19), it has:

The error compensation signal is constructed as

Choosing a Lyapunov function and taking its time derivative, one can obtain

where the compensation error ${v_1} = {e_1} - {\varsigma _1}$.

Using a FWNN to approximate the unknown item ${f_1}$, one can obtain:

By the Young's inequality, it has

Therefore, we have

with

Hence, it has

By substituting compensation signal ${\dot \varsigma _1}$ from (3.6) into (3.13), it has

Then, the virtual control law ${\alpha _1}$ is designed as the following

Substituting the virtual control ${\alpha _1}$ into (3.14) results in

The adaptive law is given as

By integrating (3.16) and (3.17), we have

Note that

One has

Step 2. Taking the time derivative of ${e_2}$, it has:

The error compensation signal is constructed as

Choosing a Lyapunov function and taking its time derivative, one can obtain

where the compensation error ${v_2} = {e_2} - {\varsigma _2}$.

By integrating compensation signal ${\dot{\varsigma }}_{2}$ and (3.23), it has

Using a FWNN to approximate the unknown item ${f_2}$, one can obtain:

By the Young's inequality, it has

Therefore, we have

with

Hence, it has

Then, the virtual control law ${\alpha _2}$ is designed as the following

Substituting the virtual control ${\alpha _2}$ into (3.30) results in

Step i. Taking the time derivative of ${e_i}$, it has:

The error compensation signal is constructed as

Choosing a Lyapunov function and taking its time derivative, one can obtain

where the compensation error ${v_i} = {e_i} - {\varsigma _i}$.

By integrating compensation signal ${\dot{\varsigma }}_{i}$ and (3.35), it has

Using a FWNN to approximate the unknown item ${f_i}$, one can obtain:

By the Young's inequality, it has

Therefore, we have

with

Hence, it has

Then, the virtual control law ${\alpha _i}$ is designed as the following

Substituting the virtual control ${\alpha _i}$ into (3.42) results in

Step n. Taking the time derivative of ${e_n}$, it has:

The error compensation signal is constructed as

Choosing a Lyapunov function and taking its time derivative, one can obtain

where the compensation error ${v_n} = {e_n} - {\varsigma _n}$.

By integrating compensation signal ${\dot{\varsigma }}_{n}$ and (3.47), it has

Using a FWNN to approximate the unknown item ${f_n}$, one can obtain:

By the Young's inequality, it has

Therefore, we have

with

Hence, it has

Then, the control input $v$ is designed as the following

Substituting the virtual control $v$ into (3.54) results in

Up to now, the whole construction of LAFN-PPC is completed.

4.   Stability analysis

For any given positive constant $p$, consider a closed set

Theorem 1. For the strict-feedback systems (2.1) with full-state and input constraints under Assumptions 1 and 2, the controllers (3.15), (3.31), (3.43) and (3.55)–(3.57), adaptive law (3.17) and compensation signal (3.6), (3.22), (3.34) and (3.46) are constructed. If initial conditions satisfy ${\Theta _i}$, $i = 1, 2, \ldots, n$, ${x_i}(0) \in \left({ - {{\underline{\kappa }}_{i}}, {{\bar \kappa }_i}} \right)$, $i = 1, 2, \ldots, n$, and ${y_r}(0) \in \left({ - {{\underline{\kappa }}_{1}}, {{\bar \kappa }_1}} \right)$, then the proposed control scheme ensures the achievement of objectives (a)–(c).

Proof. The Lyapunov function choosing as

From (3.58), we have

To facilitate analysis, the above inequality can be written as

where

By computing the integration of the above differentiation inequality at the interval $\left[{0, t} \right)$, one can obtain

From Lemma 2, we can get that $V$, $\chi$ and ${\int }_{0}^{t}\left(g{c}_{v}N\left(\chi \right)+1\right)\dot{\chi }d\tau$ are bounded at the interval $\left[{0, t} \right)$. And the result holds even if $t \to \infty$. Furthermore, let $\mathbb{C}$ be the upper bound of ${e}^{-{\rm A}t}{\int }_{0}^{t}\left(g{c}_{v}N\left(\chi \right)+1\right)\dot{\chi }{e}^{{\rm A}\tau }d\tau$. The following inequality will holds

We denote ${F_\infty }$ as the set of all bounded functions. According to the above analysis, we can get $V \leqslant p$. Therefore, we can obtain that ${v_1}$, ${v_2}$, $\cdots$, ${v_n}$, $\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{\theta }$, ${\varsigma _1}$, ${\varsigma _2}$, $\cdots$, ${\varsigma _n}$ are bounded. Furthermore, ${e_i}$ are also bounded due to ${v_i} = {e_i} - {\varsigma _i}$. As ${e}_{1} = {\mathbb{Z}}_{1}\left(t\right)$ and ${e_1} \in {F_\infty }$ it has ${\mathbb{Z}}_{1}\left(t\right)\in {F}_{\infty }$. It implies ${z_1}\left(t \right), \rho \in {F_\infty }$ and ${s_1} = {z_1} + {\alpha _{1c}} \in {F_\infty }$. Noting (2.9), we obtain ${\rho _1} \in {F_\infty }$. From (2.18), it yields $\rho, {\mu }_{1}\left(t\right), {\dot{\mu }}_{1}\left(t\right)\in {F}_{\infty }$. With the help of (3.15) and (2.12), we have ${\alpha _1} \in {F_\infty }$ and ${\alpha }_{2c}, {\dot{\alpha }}_{2c}\in {F}_{\infty }$. Since ${s_2} = {v_2} + {\alpha _{2c}} + {\varsigma _2}$, we get ${s_2} \in {F_\infty }$. Noting (2.9), we obtain ${\rho _2} \in {F_\infty }$. From (3.31) and (2.12), we get ${\alpha _2} \in {F_\infty }$ and ${\alpha }_{3c}, {\dot{\alpha }}_{3c}\in {F}_{\infty }$. Similarly, we can easily get

From (2.8), it yields ${x}_{1}, {x}_{2}, \cdots {, x}_{n}\in {F}_{\infty }$. This finishes the proof.

Remark 5. In the proposed low-cost adaptive neural prescribed performance control (LAFN-PPC) scheme, we can obtain a satisfactory performance by reasonably adjusting ${k_i}$, $\gamma$, $\sigma$, ${\bar \kappa _i}$, ${{\underline{\kappa }}_{i}}$, $\bar k$, $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{k}$, ${\mu _0}$, ${\mu _\infty }$ and $\hbar$, where $i = 1, 2, \ldots, n$. The larger ${k_i}$ and smaller$\gamma$, $\sigma$ can improve the convergence speed and tracking accuracy of the controller. But too large ${k_i}$ and small $\gamma$, $\sigma$ values can result in large control input, which may be far beyond physical limitations of actuator. The adaptive parameters $\gamma$ and $\sigma$ play the part of regulators between controller and control output. To avoid the large control input, the values of the parameters ${k_i}$, $\gamma$, $\sigma$, $i = 1, 2, \ldots, n$ are limited to a certain interval. Meanwhile, ${\bar \kappa _i}$ and ${{\underline{\kappa }}_{i}}$ are used to constrain the states ${x_i}\left(t \right)$ within the open sets $\left({ - {{\underline{\kappa }}_{i}}, {{\bar \kappa }_i}} \right)$, which can be selected according to the actual application requirements. From this it can be concluded that the time varying parameter

The parameters $\bar k$, $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{k}$, ${\mu _0}$, ${\mu _\infty }$ and $\hbar$ are designed to constrain the transformed output tracking error within the open set $\left({ - \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{k} \mu \left(t \right), \bar k\mu \left(t \right)} \right)$, where

${\mu _0}$, $\hbar$ and ${\mu _\infty }$ determine the initial error, the error convergence rate and the steady-state error of the transformed output tracking error bound, which can be selected according to the performance requirements. $\bar k$ and $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{k}$ are always within $\left({0, 1} \right]$ and can deal with the issues considering symmetric or asymmetric transformed output tracking error constraint.

5.   Simulations

In order to prove the effectiveness and feasibility of our control scheme, this section provides comparison simulation cases. Meanwhile, the control schemes based on works in ^[29,30] are compared with our suggested control scheme.

A rigid manipulator system ^[30] is given as

where $\theta$, $\dot{\theta }$ and $\ddot{\theta }$ are the position, velocity and acceleration of the link, respectively. $M$, ${g_v}$ and $L$ denote the link mass, gravity constant and the distance from the joint to the mass center of the link, respectively. ${T_E}$ indicates the unknown external load. $u$ is the actual control torque which is subjected to the unknown input constraint nonlinearity $H\left(v \right)$, and $v$ is system control input signal.

To facilitate the controller design, we transfer the system (5.1) with new variables. Let ${x_1} = \theta$, ${x}_{2} = \dot{\theta }$. Then the dynamic model of system (5.1) can be expressed as follows:

To simplify the expression, the dynamic model is rewritten as

where

In the simulation, we set ${f_1}\left({{x_1}} \right) = 0$, ${f_2}\left({{x_1}, {x_2}} \right) = {f_L}$ and $g = {G_L}$. $M = 0.35Kg,$ ${g}_{v}$ = 9.8 m/s², $L = 1.47m$ and ${T_E} = 0.1\sin t$. The tracking signal of following cases are set as ${y_r} = 0.5\sin t$.

Case 1. The initial system states and output are $x\left(0 \right) = \left[{0.1, 0} \right]$. And $\overset{\lower0.5em\hbox{$\smash{\scriptscriptstyle\frown}$}}{\theta } (0) = {\alpha _{jc}}(0) = {\alpha _{jc, j}}(0) = 0$, ${\varsigma _1}(0) = {\varsigma _2}(0) = 0$. The proposed controller, adaptive laws and error compensation schemes are constructed as

where the parameters are chosen as ${k_1} = 50$, ${k_2} = 15$, $\gamma = 10$, $\sigma = 0.7$, $\omega = 300$, $\varpi = 0.95$. The parameters of FWNN are chosen as: The nodes of neural network is 7, the center $c_1^j$ is distributed in the field of $\left[{- 3, 3} \right]$, and its width $\omega _1^j = 1$. The parameters of full-state constraints, input constraint and prescribed performance constraint are set as: ${\bar \kappa _1} = 1.5$, ${\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{\kappa } _1} = 1.5$, ${\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{\kappa } _2} = 3$, ${\bar \kappa _2} = 4$, ${u^ + } = 30$, ${u^ - } = - 30$, ${\mu _0} = 0.2$, ${\mu _\infty } = 0.02$, $\hbar = 1$, $\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{k} = 1$, $\bar k = 1$.

Case 2. Base on the case 1, we further study the output tracking errors when give different prescribed performance. ${e_{r1}}$ for ${\mu _\infty } = 0.02$, ${e_{r2}}$ for ${\mu _\infty } = 0.016$, ${e_{r3}}$ for ${\mu _\infty } = 0.012$, ${e_{r4}}$ for ${\mu _\infty } = 0.008$, ${e_{r5}}$ for ${\mu _\infty } = 0.004$, ${e_{r6}}$ for ${\mu _\infty } = 0.002$.

Case 3. Based on the case 2, we set ${\mu _\infty } = 0.004$ and ${y_d} = {y_r}$, two comparative simulation from ^[29,30] are carried out to further show the advantage of our control scheme, which is still based on the rigid manipulator system (5.3). The controller, adaptive laws and first-order filter of work in ^[29] are given as

where ${z_1} = {x_1} - {y_d}$, ${z_2} = {x_2} - {\alpha _{2f}}$, $\theta \left(0 \right) = 0$, ${\alpha _{2f}}\left(0 \right) = 0$, ${c_1} = 40$, ${c_2} = 80$, ${\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{F} _1} = 1.5$, ${\bar F_1} = 1.5$, ${\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{F} _2} = 3$, ${\bar F_2} = 4$, $\gamma = 15$, $\sigma = 0.5$, $\varphi = 5$ and $\varepsilon = 0.03$. Detailed controller design and parameter meaning is found in ^[29].

The controller, adaptive laws and first-order filter of works in ^[30]. are given as

where ${z_1} = {x_1} - {y_d}$, ${z_2} = {x_2} - {\psi _2}$, ${\zeta _2}\left(0 \right) = 0$, ${\psi _2}\left(0 \right) = 0$, ${k_1} = 50$, ${k_2} = 30$, ${\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{k} _1} = 1.5$, ${\bar k_1} = 1.5$, ${\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{k} _2} = 3$, ${\bar k_2} = 4$, $\Gamma = 15$ and $\sigma = 0.5$.$\phi \left(Z \right)$ is the activation function of neural network, $Z$ is the input of neural network. The parameters of RBF are chosen as: The nodes of neural network is 7, the center ${\mu _1}$ is distributed in the field of $\left[{- 0.3, 0.3} \right]$, and its width ${\sigma _1} = 0.1$. Detailed controller design and parameter meaning is found in ^[30].

Figures 4–7 reflect the main results of our control scheme. Figure 4 shows that output $y$ of rigid manipulator system can track desired trajectory well without violating the output constraint. Figure 5 reveals the output tracking error ${e_r}$ is in the region boundary all the times. The actual controls $u$is showed in Figure 6. And the state ${x_2}$ is illustrated in Figure 7. From Figure 8, the proposed control scheme tracks the desired signal well with different prescribed performance, and the boundedness of prescribed performance is not violated.

Figures 9–11 present the comparative results of tracking trajectories, output tracking errors and input signals. We can easily find that the results of LAFN-PPC is better than controllers in ^[29,30]. Hence, for strict-feedback systems with purpose of high-precision tracking performance, full-state constraints and input constraint, we can conclude that our suggested control scheme has a potential to control them.

6.   Conclusions

The LAFN-PPC is newly constructed for strict-feedback systems with prescribed output performance, full-state constraints and input constraint. The newly constructed command filter based adaptive control scheme with an error compensation mechanism can not only overcome the so-called "EOC" problem, but also reduce filter errors. By introducing a one-to-one nonlinear state transformation function, the full-state constraints are resolved. The prescribed performance can be guaranteed by using the one-to-one nonlinear error transformation function. The unknown control direction and the input constraint nonlinearity are resolved by introducing the Nussbaum function simultaneously. Moreover, the large computational cost is solved by introducing a virtual parameter of adaptive laws. Only one parameter needs to be updated online. Future works can focus on command-filter based optimal control of nonstrict-feedback systems considering performance constraint and address how to minimize resources consumption while ensuring performance.

Appendix A

Proof. According to the state transformation function (2.8), we have

${x_i}$ are always constrained in the open sets $\left({ - {{\underline{\kappa }}_{i}}, {{\bar \kappa }_i}} \right)$, i.e., ${x_i}\left(t \right) \in {{{\Omega}} _i}$. Therefore, we can easily obtain that ${\eta _i} > 0$ and ${\eta _i}$take the maximal value at the points $x_i^0 = {{\left({{{\bar \kappa }_i} - {{\underline{\kappa }}_{i}}} \right)} /2}$ according to the properties of the quadratic function. It is obvious that $x_i^0$ is in the interval $\left({ - {{\underline{\kappa }}_{i}}, {{\bar \kappa }_i}} \right)$. Thus, the maximal value of ${\eta _i}$ is

This finishes the proof.

Appendix B

Proof. According to (2.5), (2.11), (2.13) and (2.14), we have

${x_1}$ and ${y_r}$ are constrained in the open sets $\left({ - {{\underline{\kappa }}_{i}}, {{\bar \kappa }_i}} \right)$. Thus, $0 < {\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{\kappa } _1} + {y_r} < {\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{\kappa } _1} + {\bar \kappa _1}$, $0 < {\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{\kappa } _1} + {x_1} < {\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{\kappa } _1} + {\bar \kappa _1}$, $0 < {\bar \kappa _1} - {x_1} < {\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{\kappa } _1} + {\bar \kappa _1}$ and $0 < {\bar \kappa _1} - {y_r} < {\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{\kappa } _1} + {\bar \kappa _1}$. One obtains

The transformed output tracking error ${z_1} = {s_1} - {\alpha _{1c}}$ strictly converges in the prescribed performance region $- \underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{k} \mu \left(t \right) < {z_1}\left(t \right) < \bar k\mu \left(t \right)$. Hence, it has

This finishes the proof.

Acknowledgments

The authors would like to appreciate all the editors and reviewers for improving the quality of this article. This work was supported by the National Key Research and Development Program of China (2018YFB1304800) and Key Research and Development Program of Guangdong Province (2020B090926002).

Conflict of interest

All authors declare no conflicts of interest in this paper.