Flux functions
We consider two scalar conservation laws with non-local flux functions, describing traffic flow on roads with rough conditions. In the first model, the velocity of the car depends on an averaged downstream density, while in the second model one considers an averaged downstream velocity. The road condition is piecewise constant with a jump at
Citation: Wen Shen. Traveling waves for conservation laws with nonlocal flux for traffic flow on rough roads[J]. Networks and Heterogeneous Media, 2019, 14(4): 709-732. doi: 10.3934/nhm.2019028
| [1] | Wen Shen . Traveling waves for conservation laws with nonlocal flux for traffic flow on rough roads. Networks and Heterogeneous Media, 2019, 14(4): 709-732. doi: 10.3934/nhm.2019028 |
| [2] | Wen Shen . Traveling wave profiles for a Follow-the-Leader model for traffic flow with rough road condition. Networks and Heterogeneous Media, 2018, 13(3): 449-478. doi: 10.3934/nhm.2018020 |
| [3] | Dong Li, Tong Li . Shock formation in a traffic flow model with Arrhenius look-ahead dynamics. Networks and Heterogeneous Media, 2011, 6(4): 681-694. doi: 10.3934/nhm.2011.6.681 |
| [4] | Tong Li . Qualitative analysis of some PDE models of traffic flow. Networks and Heterogeneous Media, 2013, 8(3): 773-781. doi: 10.3934/nhm.2013.8.773 |
| [5] | Mauro Garavello, Roberto Natalini, Benedetto Piccoli, Andrea Terracina . Conservation laws with discontinuous flux. Networks and Heterogeneous Media, 2007, 2(1): 159-179. doi: 10.3934/nhm.2007.2.159 |
| [6] | Maya Briani, Emiliano Cristiani . An easy-to-use algorithm for simulating traffic flow on networks: Theoretical study. Networks and Heterogeneous Media, 2014, 9(3): 519-552. doi: 10.3934/nhm.2014.9.519 |
| [7] | Raimund Bürger, Harold Deivi Contreras, Luis Miguel Villada . A Hilliges-Weidlich-type scheme for a one-dimensional scalar conservation law with nonlocal flux. Networks and Heterogeneous Media, 2023, 18(2): 664-693. doi: 10.3934/nhm.2023029 |
| [8] | Abraham Sylla . Influence of a slow moving vehicle on traffic: Well-posedness and approximation for a mildly nonlocal model. Networks and Heterogeneous Media, 2021, 16(2): 221-256. doi: 10.3934/nhm.2021005 |
| [9] | Maria Laura Delle Monache, Paola Goatin . Stability estimates for scalar conservation laws with moving flux constraints. Networks and Heterogeneous Media, 2017, 12(2): 245-258. doi: 10.3934/nhm.2017010 |
| [10] | Paola Goatin, Chiara Daini, Maria Laura Delle Monache, Antonella Ferrara . Interacting moving bottlenecks in traffic flow. Networks and Heterogeneous Media, 2023, 18(2): 930-945. doi: 10.3934/nhm.2023040 |
We consider two scalar conservation laws with non-local flux functions, describing traffic flow on roads with rough conditions. In the first model, the velocity of the car depends on an averaged downstream density, while in the second model one considers an averaged downstream velocity. The road condition is piecewise constant with a jump at
We consider two scalar conservation laws with nonlocal flux, describing traffic flow with varying road condition. To be specific, we consider the integro-differential equations
| $ (M1)ρt+[ρ(t,x)κ(x)⋅v(∫x+hxρ(t,y)w(y−x)dy)]x=0, $ | (1.1) |
and
| $ (M2)ρt+[ρ(t,x)⋅∫x+hxκ(y)v(ρ(t,y))w(y−x)dy]x=0. $ | (1.2) |
In both equations,
| $ v(0)=1,v(1)=0,andv′(ρ)<0,v″(ρ)≤0∀ρ∈[0,1]. $ | (1.3) |
For example, the classical Lighthill-Whitham model [17] uses
The function
The models (M1) and (M2) can be formally derived as the continuum limit of particle models where the speed of each car follows certain rules. For model (M1), the speed of the car at
| $ \kappa(x) \cdot v\left(\int_x^{x+h} \rho(t, y) w(y-x) \, dy\right), $ |
where a weighted average of car density on an interval of length
| $ \int_x^{x+h} \kappa(y) v( \rho(t, y)) w(y-x) \; dy, $ |
where the weighted average is taken over
The weight
| $ ∫h0w(x)dx=1,w(x)=0∀x≥h,w′(x)<0∀x∈(0,h). $ | (1.4) |
Here, the assumption
Formally, as
| $ ρt+f(κ(x),ρ)x=0,wheref(κ,ρ)=κ(x)⋅ρv(ρ). $ | (1.5) |
Unfortunately rigorous proofs of such convergences have not been established yet in the literature, not even for the case where
Non-local conservation laws has gained growing interests in recent years. In the simpler case where
In this paper we are interested in the traveling wave profiles for (M1) and (M2). We remark that, when the road condition is uniform, say
| $ κ(x)={κ−,x<0,κ+,x>0. $ | (1.6) |
The main objective of this paper is to study the stationary wave profiles of (M1) and (M2), crossing the jump in
Unfortunately, for (M1) and (M2), due to the discontinuity in the coefficient
Traveling wave profiles for a local follow-the-leader model for traffic flow was studied in [20] for homogeneous road conditions, and in [19] with rough road condition. We also mention that, for the non-local models for slow erosion of granular flow, traveling waves and their local stability were studied in [16].
The rest of the paper is organized as follows. In Section 2 we consider model (M1) and analyze two cases with
We seek a stationary wave profile
| $ A(Q;x) \;\dot = \; \int_x^{x+h} Q(y) w(y-x) \; dy = \int_0^h Q(x+s) w(s) \; ds. $ |
Note that, since
Since
| $ Q(x)κ(x)⋅v(A(Q;x))≡ˉf=constant, $ | (2.1) |
where
| $ \bar f = \lim\limits_{x\to\pm\infty} Q(x) \kappa(x) \cdot v(A(Q;x)) . $ |
In the case when
| $ limx→−∞Q(x)=ρ−,limx→+∞Q(x)=ρ+,limx→±∞Q′(x)=0, $ | (2.2) |
the following constraint on
| $ ˉf=f(κ−,ρ−)=f(κ+,ρ+). $ | (2.3) |
Differentiating (2.1) in
| $ Q′(x)κ(x)v(A(Q;x))=−Q(x)[δ0(x)(κ+−κ−)v(A(Q;x))+k(x)v′(A(Q;x))A(Q;x)x]. $ | (2.4) |
Here,
Note that
| $ Q(0−)κ−=Q(0+)κ+. $ | (2.5) |
Thus, the jump in
| $ Q(x)v(A(Q;x))={ˉf/κ−,(x<0)ˉf/κ+,(x>0)andQ(0−)κ−=Q(0+)κ+. $ | (2.6) |
The simpler case where
Under the assumption (1.3), there exists a unique stagnation point
| $ fρ(κ,ˆρ)=0∀κ,fρ(κ,ρ)>0forρ<ˆρ,fρ(κ,ρ)<0forρ>ˆρ. $ | (2.7) |
Let
| $ W(x)⋅v(∫x+hxW(y)w(y−x)dy)≡ˉfˉκ=1ˉκlimx→±∞f(ˉκ,W(x)). $ | (2.8) |
Next Lemma was proved in [18] (Lemma 3.1).
Lemma 2.1 (Asymptotic limits). Assume that
| $ \lim\limits_{x\to-\infty} W(x) = \rho^-, \qquad \lim\limits_{x\to +\infty} W(x) = \rho^+. $ |
Let
i) As
ii) As
The following existence and uniqueness result of the profile is proved in Theorem 3.2 of [18].
Theorem 2.2. Let
| $ limx→±∞W(x)=ρ±,wheref(ˉκ,ρ−)=f(ˉκ,ρ+),ρ−<ˆρ<ρ+. $ | (2.9) |
There exist solutions
In the remaining of this section we let
| $ \lim\limits_{x\to\infty}W(x) = \rho^+, \quad \lim\limits_{x\to-\infty} W(x) = \rho^-_*, $ |
where
| $ 0 < \rho^-_* < \hat\rho < \rho^+ < 1, \qquad f(\kappa^+, \rho^-_* ) = f(\kappa^+, \rho^+). $ |
By Lemma 2.1, we see that the asymptote
When
For notational convenience, we introduce the functions:
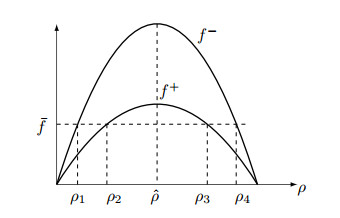
| $ f−(ρ)˙=f(κ−,ρ)=κ−ρv(ρ),f+(ρ)˙=f(κ+,ρ)=κ+ρv(ρ). $ | (2.10) |
Under the assumptions (1.3), both
The values
| $ ρ1<ρ2≤ˆρ≤ρ3<ρ4,f−(ρ1)=f−(ρ4)=ˉf=f+(ρ2)=f+(ρ3). $ | (2.11) |
We have 4 sub cases:
We first observe that the cases with
● For Case A1, we have
● For Case A2, we have
● for Case A3, we have
● For Case A4, we have
For the rest of the paper we only consider the nontrivial case with
Theorem 2.3 (Existence of profiles).
Assume
| $ limx→−∞Q(x)=ρ−,limx→∞Q(x)=ρ+. $ | (2.12) |
The profiles
Proof. The proof takes several steps.
Step 1. Since
Step 2. At
| $ 0 < Q(0-) = \frac{\kappa^+}{\kappa^-} Q(0+) < Q(0+)\le \rho^+ < 1. $ |
Furthermore, recalling the definition of
| $ \rho_2 < \hat \rho\quad \mbox{and}\quad \kappa^+ \rho_2 v(\rho_2) = \kappa^- \rho^- v(\rho^-). $ |
Then it holds
| $ Q(0-) > \frac{\kappa^+}{\kappa^-} \rho_2 = \frac{ \kappa^- \rho^- v(\rho^-)}{\kappa^- v(\rho_2)} = \frac{ v(\rho^-)}{ v(\rho_2)} \rho^- > \rho^-. $ |
Step 3. With this
| $ xi=iΔx,Qi≈Q(xi),i=Z−,andQ0=Q(0−). $ | (2.13) |
On
| $ Q^{\Delta x}(x) = Q_{i-1} \frac{x_i-x}{{\Delta x}} + Q_{i}\frac{x-x_{i-1}}{{\Delta x}} , \qquad \mbox{for}\quad x\in[x_{i-1}, x_i], \quad i = \mathbb{Z}^-. $ |
The discrete values
| $ G(Qi−1)=0whereG(Qi−1)˙=Qi−1v(A(QΔx;xi−1))−ˉf/κ−, $ | (2.14) |
assuming that
| $ Qkv(A(QΔx;xk))=ˉf/κ−,∀k≥i. $ | (2.15) |
Step 4. We now verify that (2.14) has a unique solution. We compute:
| $ G(Qi)=Qi[v(A(QΔx;xi−1))−v(A(QΔx;xi))]>0,G(0)=−ˉf/κ−<0. $ |
Furthermore, for
| $ G′(Qi−1)=v(A(QΔx;xi−1))+Qi−1∂v(A(QΔx;xi))∂Qi−1=v(A(QΔx;xi−1))+Qi−1v′(A(QΔx;xi))∂A(QΔx;xi)∂Qi−1=v(A(QΔx;xi−1))+Qi−1v′(A(QΔx;xi))∫xixi−1xi−yΔxw(y−xi−1)dy. $ |
Since
| $ \int_{x_{i-1}}^{x_i} \frac{x_i-y}{{\Delta x}} w(y-x_{i-1})\; dy = \int_{0}^{{\Delta x}} \frac{{\Delta x}-s}{{\Delta x}} w(s)\; ds \le \frac12 w(0) \cdot {\Delta x} = \mathcal{O}(1) \cdot {\Delta x}, $ |
we conclude that
| $ G'(Q_{i-1}) > 0. $ |
Thus, (2.14) has a unique solution
By induction we conclude that
Step 5. It remains to verify the limit as
| $ \bar f = \lim\limits_{x\to-\infty} \kappa(x) Q(x) v(A(Q;x)) = \kappa^- \rho^- v(\rho^-). $ |
We must also have
Several sample profiles are plotted in Figure 2. They are generated by numerical simulation, solving (2.14) by Newton iterations. All profiles are bounded and monotone, continuous except an upward jump at
Corollary 1 (Ordering of the profiles). Consider the setting of Theorem 2.3, and let
| $ \mathit{\mbox{either}} \quad \Big\{ Q_1(x) > Q_2(x) \quad \forall x \not = 0 \Big\} \quad\mathit{\mbox{or}}\quad \Big\{ Q_1(x) < Q_2(x) \quad \forall x\not = 0 \Big\}. $ |
Proof. First we observe that on
We continue by contradiction, and assume that there exists a point
| $ Q1(y)=Q2(y),andQ1(x)>Q2(x)∀x>y. $ | (2.16) |
Then, by equation (2.1) we have
| $ Q_1(y) \kappa^- v(A(Q_1;y)) = \bar f = Q_2(y) \kappa^- v(A(Q_2;y)), $ |
which implies
| $ v(A(Q_1;y)) = v(A(Q_2;y)), \qquad \mbox{i.e.} \quad A(Q_1;y) = A(Q_2;y) $ |
a contradiction to (2.16). We conclude that the graphs of
Stability. We now show the local stability of these profiles, such that they are local attractors for solutions of the Cauchy problems for (M1) with suitable initial data. When
However, if
| $ ρ(t,0−)κ−=ρ(t,0+)κ+,∀t>0. $ | (2.17) |
We have the following definition.
Definition 2.4. We say that a function
We remark that, a general existence theorem for the Cauchy problem of (M1) with discontinuous coefficient
Let
| $ D˙={(x,q):Q♭(x)≤q≤Q♯(x)}. $ | (2.18) |
By Corollary 1, all these profiles are ordered and they do not intersect with each other. One can parametrize each profile by its trace
| $ Φ(t,x)˙=˜Q(0+),where ˜Q(⋅) is a profile s.t. ˜Q(x)=ρ(t,x). $ | (2.19) |
We have the following stability Theorem.
Theorem 2.5 (Stability of the profiles).
Consider (M1) with
| $ (x,ˉρ(x))∈D,∀x,andκ−ˉρ(0−)=κ+ˉρ(0+). $ | (2.20) |
Let
| $ (x,ˉρ(t,x))∈D,∀x≠0,∀t≥0. $ | (2.21) |
Furthermore, let
| $ limt→∞[maxx{Φ(t,x)}−minx{Φ(t,x)}]=0. $ | (2.22) |
Proof. We first observe that, since the initial data is smooth except at
● If
| $ Φ(t,y)≥Φ(t,x)∀x,Φ(t,y)>Φ(t,x)∀x>y, $ | (2.23) |
then
● Symmetrically, if
| $ Φ(t,y)≤Φ(t,x)∀x,Φ(t,y)<Φ(t,x)∀x>y, $ | (2.24) |
then
This claim would imply both (2.21) and (2.22).
We provide a proof for the case of a maximum value point, while the minimum value point can be treated in a completely similar way. Fix a time
(1). Consider
| $ Q(y) = \rho(t, y), \quad Q'(y) = \rho_x(t, y), \quad \mbox{and}\quad Q(x) > \rho(t, x) \quad \forall x > y. $ |
Since
| $ A(Q;y)>A(ρ;t,y)andv(A(Q;y))<v(A(ρ;t,y)). $ | (2.25) |
We also have
| $ A(Q;y)x=Q(y+h)w(h)−Q(y)w(0)−∫h0Q(y+s)w′(s)ds,A(ρ;t,y)x=ρ(t,y+h)w(h)−ρ(t,y)w(0)−∫h0ρ(t,y+s)w′(s)ds. $ |
Using
| $ A(Q;y)x−A(ρ;t,y)x>0. $ | (2.26) |
We now compute:
| $ (κ+)−1ρt(t,y)=−[ρ(t,y)v(A(ρ;t,y))]x=[Q(y)v(A(Q;y))]x−[ρ(t,y)v(A(ρ;t,y))]x=Q′(y)[v(A(Q;y))−v(A(ρ;t,y))]+Q(y)[v′(A(Q;y))−v′(A(ρ;t,y))]A(Q;y)x+Q(y)v′(A(ρ;t,y))[A(Q;y)x−A(ρ;t,y)x]<0. $ |
Here the last inequality holds thanks to (2.25)-(2.26).
(2). Consider
| $ Q(0-) = \rho(t, 0-), \qquad Q(0+) = \rho(t, 0+), $ |
Since the delay differential equation has one-sided delay, then the derivative
| $ Q(0-) = \rho(t, 0-), \qquad Q'(0-) \le \rho_x(t, 0-), \qquad Q(x) > \rho(t, x) \; \forall x > 0. $ |
We have similar estimates as those in (2.25)-(2.26), i.e.,
| $ {A(Q;0−)>A(ρ;t,0−),v(A(Q;0−))<v(A(ρ;t,0−)),A(Q;0−)x>A(ρ;t,0−)x. $ | (2.27) |
We now compute, by using (2.27)
| $ (κ−)−1ρt(t,0−)=[Q(0−)v(A(Q;0−))]x−[ρ(t,0−)v(A(ρ;t,0−))]x=Q′(0−)[v(A(Q;0−))−v(A(ρ;t,0−))]+v(A(ρ;t,0−)[Q′(0−)−ρx(t,0−)]+Q(0−)[v′(A(Q;0−))−v′(A(ρ;t,0−))]A(Q;0−)x+Q(0−)v′(A(ρ;t,0−))[A(Q;0−)x−A(ρ;t,0−)x]<0. $ |
(3). If
| $ Q(y) = \rho(t, y), \quad Q'(y) = \rho_x(t, y), \quad Q(x) > \rho(t, x) \quad \forall x > y. $ |
The rest of the computation remains the same as in the previous steps.
We perform a numerical simulation with Riemann initial data
Since
Theorem 2.6 (Existence of a unique profile).
Let
| $ Q(x)\equiv \rho^+ \quad (x > 0), \qquad \mathit{\mbox{and}} \quad \lim\limits_{x\to-\infty} Q(x) = \rho^-. $ |
See Figure 4 for a sample profile.
Since
Since
A numerical simulation with Riemann initial data is performed, and results are plotted in Figure 6. We observe that oscillations are formed around
Since both
Fix
| $ \rho_1 < \rho_2 \le \hat\rho\le \rho_3 < \rho_4, \qquad f^+(\rho_1) = f^+(\rho_4) = \bar f = f^-(\rho_2) = f^-(\rho_3). $ |
Similar to Case A, there are 4 sub-cases:
B1. We have
B2. We have
B3. We have
B4. We have
Note that since
| $ Q(0+)≤κ−κ+. $ | (2.28) |
As previously, we let
Theorem 2.7. Given
| $ \rho^- < \hat\rho < \rho^+, \qquad f^-(\rho^-) = f^+(\rho^+) > 0, $ |
there exist infinitely many solutions
| $ \lim\limits_{x\to-\infty} Q(x) = \rho^-, \quad \lim\limits_{x\to+\infty} Q(x) = \rho^+. $ |
All profiles are monotone increasing on
Proof. The proof takes several steps.
(1) On
In conclusion, on
(2) Furthermore, we consider only the profiles where
(3) Utilizing the same analysis as in Step 3-4 of Theorem 2.3, we have that, if a profile
(4) We now construct a lower envelope
We claim that, on
| $ Q(0-) = \frac{\kappa^+Q(0+)}{\kappa^-} = \frac{\kappa^+\rho_1}{\kappa^-} = \frac{\kappa^- \rho^- v(\rho^-)}{v(\rho_1) \kappa^- } = \frac{ \rho^- v(\rho^-)}{v(\rho_1) } < \rho^-. $ |
Then, by (2.4), we have
| $ (Q^\flat)'(0-) v(A(Q^\flat;0)) = -Q(0-) v'(A(Q^\flat;0)) A(Q^\flat;0-)_x < 0, $ |
where the last inequality holds thanks to
To show that
| $ Q^\flat(y) \kappa^- v(A(Q^\flat;y)) = \bar f = \kappa^- \rho^- v(\rho^-) $ |
thus
| $ v(A(Q^\flat;y)) = v(\rho^-) \quad \rightarrow \quad A(Q^\flat;y) = \rho^-, $ |
a contradiction which proofs our claim.
Finally, by the same argument as in Step 5 in the proof of Theorem 2.3 one concludes that the profile
(5) By the ordering of the profiles, any profile
Indeed, by (2.1), on
| $ Q'(x) = Q(x) \frac{-v'(A(Q;x))}{v(A(Q;x))} A(Q;x)_x. $ |
Thus,
| $ A(Q;x)_x = \int_0^{h} [Q(x+s) - Q(x)] (-w'(s)) \; ds. $ |
Since
Thus, there exists a sequence of local maxima
| $ y_{k+1} < y_k, \qquad Q(y_k) > \rho^-, \qquad Q(y_{k+1}) < Q(y_k)\qquad \forall k. $ |
The sequence might be finite or infinite. We conclude that there exists an increasing function on
By continuity there exists an upper profile
We conclude that, in between the profiles
Sample profiles for Case B1 are given in Figure 8. By a similar argument as for Case A1, one concludes that these profiles are time asymptotic limits for solutions of the Cauchy problems for (M1). We omit the details of the proof. Result of a numerical simulation is given in Figure 9, with Riemann initial data. We observe that the solution approaches a certain traveling wave profile as
For Case B2, we have
For case B3, we have
Let
| $ P(x)⋅V(P;x)≡ˉf,whereV(P;x)˙=∫x+hxκ(y)v(P(y))w(y−x)dy, $ | (3.1) |
and
| $ \bar f = \lim\limits_{x\to\pm\infty} P(x) \cdot \int_x^{x+h} \kappa(y) v( P(y)) w(y-x) \; dy. $ |
In the case where
We see that
Previous results. Consider the simpler case where
| $ W(x)⋅∫x+hxv(W(y))w(y−x)dy=ˉfˉκ. $ | (3.2) |
By the results in [18], Lemma 2.1 and Theorem 2.2 in Section 2.1 hold for the profile
Below we consider two cases, where Case C is for
Similar to Case A, we let
Theorem 3.1. Let
Proof. A proof very similar to the proof of Theorem 2.3 can be carried out here, with small modifications. Here we provide a new approach, utilizing a contractive Picard operator.
On
| $ U≐{u:R↦[ρ−,ρ+];uis Lipschitz continuous,u(x)=W(x) for x≥0,limx→−∞u(x)=ρ−,u′(x)≥0∀x∈R}. $ | (3.3) |
Let
| $ (mathcalPu)(x)˙=ˉfV(u;x)=ˉf∫x+hxκ(y)v(u(y))w(y−x)dy. $ | (3.4) |
Note that a fixed point for
We claim that
| $ limx→−∞(Pu)(x)=limx→−∞ˉfV(u;x)=ˉflimx→−∞∫x+hxκ(y)v(u(y))w(y−x)dy=ˉfκ−v(ρ−)=ρ−. $ |
Furthermore,
| $ \rho^- \le (\mathcal{P} u)(x) \le \rho^+, \qquad \kappa^+ \rho^- \le V(u;x) \le \kappa^- \rho^+, \qquad \forall x\in\mathbb{R}. $ |
Finally, we estimate the Lipschitz constant for
| $ 0≤−V(u;x)x=κ(x)v(u(x))w(0)−∫x+hxκ(y)v(u(y))(−w′(y−x))dy≤κ(x)v(u(x))w(0)≤κ−w(0), $ |
we have
| $ 0 \le (\mathcal{P} u)'(x) = -\frac{\bar f}{ V^2(u;x)} V(u;x)_x \le \frac{\bar f \kappa^- w(0)}{(\kappa^+ \rho^-)^2}, $ |
proving the claim.
We introduce a norm which we call the
| (3.5) |
We claim that the operator
It remains to establish the contractive property. Indeed, let
| $ \|u_1-u_2\|_\alpha = \delta, \qquad \mbox{i.e.}\quad {|u1(x)−u2(x)|≤δe−αx∀x≤0,|u1(x)−u2(x)|=0∀x≥0. $ |
Then, on
| $ |(Pu1)(x)−(Pu2)(x)|=|ˉfV(u1;x)−ˉfV(u2;x)|=|ˉf⋅(V(u1;x)−V(u2;x))V(u1;x)V(u2;x)|≤ˉf(κ+ρ−)2∫x+hxκ(y)|v(u1(y))−v(u2(y))|w(y−x)dy≤ˉfκ−‖v′‖L∞w(0)(κ+ρ−)2∫x+hxδe−αydy<ˉfκ−‖v′‖L∞(κ+ρ−)2⋅δ⋅1αe−αx≤12δe−αx. $ |
Hence
| $ \left\| (\mathcal{P} u_1)-(\mathcal{P} u_2)\right\|_\alpha \le \sup\limits_{x < 0} \left| (\mathcal{P} u_1)(x)-(\mathcal{P} u_2)(x)\right| \le \frac12 \delta = \frac12 \left\| u_1- u_2\right\|_\alpha, $ |
completing the proof.
We remark that a suitable Picard operator could be defined for the proof of Theorem 2.3 for Case A1.
Sample profiles for case C1 are plotted in Figure 14. We observe that they do not cross each other. The ordering property in Corollary 1 holds for these profile, with a very similar proof.
Stability. In the case when
Definition 3.2 We say that
| $ ρx(t,0+)−ρx(t,0−)=(κ+−κ−)ρ(t,0)v(ρ(t,0))w(0)κ+∫h0v(ρ(t,y))w(y)dy∀t>0. $ | (3.6) |
With a very similar argument as those for Case A1, one can prove that the profiles
Theorem 3.3. Let
We perform a numerical simulation with Riemann initial data, and plot the result in Figure 15. We observe that the solution
For Case C2 we have
For Case C3 with
This is the counter part for Case B, and similarly we have 4 sub-cases which we discuss in detail in the following sub sections.
Theorem 3.4. Let
| $ 0 < \rho^- < \hat\rho < \rho^+ < 1, \qquad f^-(\rho^-) = f^+(\rho^+) . $ |
Then there exist infinitely many solutions for (3.1) which satisfy the asymptotic boundary conditions
| $ \lim\limits_{x\to-\infty} P(x) = \rho^-, \qquad \lim\limits_{x\to+\infty} P(x) = \rho^+. $ |
Proof. The proof follows a similar line of argument as those in the proof of Theorem 2.7. Below we briefly outline the adjustments.
Step 1. On
Step 2. Let
Step 3. Let
| $ V(P;x)_x = -\kappa^- \int_0^h [v(P(x+s)) - v(P(x))] w'(s) \; ds. $ |
Thus, if
Sample profiles are given in Figure 20. Using a similar argument as for Theorem 2.5 for Case A1, these profiles are time asymptotic limits for the solutions of the the Cauchy problem of (1.2). We omit the details of the proof. A numerical simulation is presented in Figure 21, with Riemann data, where we observe this asymptotic behavior as
Case D2, D3 and D4 are the counter part of Case B1, B2 and B3, respectively, with similar results. Here we only present the sample plots for Case D2 in Figure 22, and numerical simulations in Figure 23, Figure 24 and Figure 25 for Case D2, D3 and D4, respectively.
We study traveling wave profiles for two nonlocal PDE models for traffic flow with rough road condition. For all possible cases, we show that for different cases there can exist infinitely many traveling wave profiles, a unique profile, or no profiles at all, depending on the jump in speed limit and the limits
Formally, the non-local PDE models are the macroscopic limits of the corresponding non-local particle models, referred to as the follow-the-leaders (FtLs) model. In the case where
Codes for all the numerical simulations in this paper can be found at:
| 1. | Felisia Angela Chiarello, Giuseppe Maria Coclite, Nonlocal scalar conservation laws with discontinuous flux, 2022, 18, 1556-1801, 380, 10.3934/nhm.2023015 | |
| 2. | Alberto Bressan, Wen Shen, On Traffic Flow with Nonlocal Flux: A Relaxation Representation, 2020, 237, 0003-9527, 1213, 10.1007/s00205-020-01529-z | |
| 3. | F. A. CHIARELLO, J. FRIEDRICH, P. GOATIN, S. GÖTTLICH, O. KOLB, A non-local traffic flow model for 1-to-1 junctions, 2020, 31, 0956-7925, 1029, 10.1017/S095679251900038X | |
| 4. | Alexandre Bayen, Jan Friedrich, Alexander Keimer, Lukas Pflug, Tanya Veeravalli, Modeling Multilane Traffic with Moving Obstacles by Nonlocal Balance Laws, 2022, 21, 1536-0040, 1495, 10.1137/20M1366654 | |
| 5. | Andrea Corli, Luisa Malaguti, 2021, Chapter 8, 978-3-030-61345-7, 167, 10.1007/978-3-030-61346-4_8 | |
| 6. | Alexander Keimer, Lukas Pflug, 2023, 15708659, 10.1016/bs.hna.2022.11.001 | |
| 7. | Kuang Huang, Qiang Du, Asymptotic Compatibility of a Class of Numerical Schemes for a Nonlocal Traffic Flow Model, 2024, 62, 0036-1429, 1119, 10.1137/23M154488X | |
| 8. | Maria Teresa Chiri, Minyan Zhang, On backward Euler approximations for systems of conservation laws, 2024, 31, 1021-9722, 10.1007/s00030-023-00920-5 | |
| 9. | F. A. Chiarello, J. Friedrich, S. Göttlich, A non-local traffic flow model for 1-to-1 junctions with buffer, 2024, 19, 1556-1801, 405, 10.3934/nhm.2024018 | |
| 10. | Veerappa Gowda G. D., Sudarshan Kumar Kenettinkara, Nikhil Manoj, Convergence of a second-order scheme for non-local conservation laws, 2023, 57, 2822-7840, 3439, 10.1051/m2an/2023080 | |
| 11. | F. A. Chiarello, H. D. Contreras, L. M. Villada, Existence of entropy weak solutions for 1D non-local traffic models with space-discontinuous flux, 2023, 141, 0022-0833, 10.1007/s10665-023-10284-5 |
Flux functions
Sample traveling waves for Case A1, with
Numerical simulation for model (M1) with Riemann initial data for Case A1
Typical traveling wave profile for Case A2
Numerical simulation for the PDE model with Riemann initial data for Case A2
Numerical simulation for the PDE model with Riemann initial data for Case A3
Numerical simulation for the PDE model with Riemann initial data for Case A4
Sample traveling waves for Case B1
Numerical simulation for the PDE model with Riemann initial data for Case B1
Sample traveling wave for Case B2
Numerical simulation for the PDE model with Riemann initial data for Case B2
Numerical simulation for the PDE model with Riemann initial data for Case B3
Numerical simulation for the PDE model with Riemann initial data for Case B4
Sample traveling wave for Case C1
Numerical simulation for the PDE model with Riemann initial data for Case C1
Sample traveling wave for Case C2
Numerical simulation for the PDE model with Riemann initial data for Case C2
Numerical simulation for the PDE model with Riemann initial data for Case C3
Numerical simulation for the PDE model with Riemann initial data for Case C4
Sample traveling wave for Case D1
Numerical simulation for the PDE model with Riemann initial data for Case D1
Sample traveling wave for Case D2
Solution of Riemann problem for Case D2
Solution of Riemann problem for Case D3
Solution of Riemann problem for Case D4