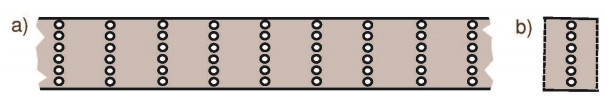
a) The perforated strip
We address a spectral problem for the Dirichlet-Laplace operator in a waveguide $ \Pi^ \varepsilon $. $ \Pi^ \varepsilon$ is obtained from repsilon an unbounded two-dimensional strip $ \Pi $ which is periodically perforated by a family of holes, which are also periodically distributed along a line, the so-called "perforation string". We assume that the two periods are different, namely, $ O(1) $ and $ O( \varepsilon) $ respectively, where $ 0< \varepsilon\ll 1 $. We look at the band-gap structure of the spectrum $ \sigma^ \varepsilon $ as $ \varepsilon\to 0 $. We derive asymptotic formulas for the endpoints of the spectral bands and show that $ \sigma^ \varepsilon $ has a large number of short bands of length $ O( \varepsilon) $ which alternate with wide gaps of width $ O(1) $.
Citation: Sergei A. Nazarov, Rafael Orive-Illera, María-Eugenia Pérez-Martínez. Asymptotic structure of the spectrum in a Dirichlet-strip with double periodic perforations[J]. Networks and Heterogeneous Media, 2019, 14(4): 733-757. doi: 10.3934/nhm.2019029
| [1] | Sergei A. Nazarov, Rafael Orive-Illera, María-Eugenia Pérez-Martínez . Asymptotic structure of the spectrum in a Dirichlet-strip with double periodic perforations. Networks and Heterogeneous Media, 2019, 14(4): 733-757. doi: 10.3934/nhm.2019029 |
| [2] | Valeria Chiado Piat, Sergey S. Nazarov, Andrey Piatnitski . Steklov problems in perforated domains with a coefficient of indefinite sign. Networks and Heterogeneous Media, 2012, 7(1): 151-178. doi: 10.3934/nhm.2012.7.151 |
| [3] | Luis Caffarelli, Antoine Mellet . Random homogenization of fractional obstacle problems. Networks and Heterogeneous Media, 2008, 3(3): 523-554. doi: 10.3934/nhm.2008.3.523 |
| [4] | Günter Leugering, Sergei A. Nazarov, Jari Taskinen . The band-gap structure of the spectrum in a periodic medium of masonry type. Networks and Heterogeneous Media, 2020, 15(4): 555-580. doi: 10.3934/nhm.2020014 |
| [5] | Vivek Tewary . Combined effects of homogenization and singular perturbations: A bloch wave approach. Networks and Heterogeneous Media, 2021, 16(3): 427-458. doi: 10.3934/nhm.2021012 |
| [6] | Chaoqun Huang, Nung Kwan Yip . Singular perturbation and bifurcation of diffuse transition layers in inhomogeneous media, part I. Networks and Heterogeneous Media, 2013, 8(4): 1009-1034. doi: 10.3934/nhm.2013.8.1009 |
| [7] | Martin Heida . Stochastic homogenization on perforated domains III–General estimates for stationary ergodic random connected Lipschitz domains. Networks and Heterogeneous Media, 2023, 18(4): 1410-1433. doi: 10.3934/nhm.2023062 |
| [8] | Patrizia Donato, Florian Gaveau . Homogenization and correctors for the wave equation in non periodic perforated domains. Networks and Heterogeneous Media, 2008, 3(1): 97-124. doi: 10.3934/nhm.2008.3.97 |
| [9] | Carlos Conca, Luis Friz, Jaime H. Ortega . Direct integral decomposition for periodic function spaces and application to Bloch waves. Networks and Heterogeneous Media, 2008, 3(3): 555-566. doi: 10.3934/nhm.2008.3.555 |
| [10] | Oleh Krehel, Toyohiko Aiki, Adrian Muntean . Homogenization of a thermo-diffusion system with Smoluchowski interactions. Networks and Heterogeneous Media, 2014, 9(4): 739-762. doi: 10.3934/nhm.2014.9.739 |
We address a spectral problem for the Dirichlet-Laplace operator in a waveguide $ \Pi^ \varepsilon $. $ \Pi^ \varepsilon$ is obtained from repsilon an unbounded two-dimensional strip $ \Pi $ which is periodically perforated by a family of holes, which are also periodically distributed along a line, the so-called "perforation string". We assume that the two periods are different, namely, $ O(1) $ and $ O( \varepsilon) $ respectively, where $ 0< \varepsilon\ll 1 $. We look at the band-gap structure of the spectrum $ \sigma^ \varepsilon $ as $ \varepsilon\to 0 $. We derive asymptotic formulas for the endpoints of the spectral bands and show that $ \sigma^ \varepsilon $ has a large number of short bands of length $ O( \varepsilon) $ which alternate with wide gaps of width $ O(1) $.
In this paper we consider a spectral problem for the Laplace operator in an unbounded strip

a) The perforated strip
We provide asymptotic formulas for the endpoints of the spectral bands and show that these bands collapse asymptotically at the points of the spectrum of the Dirichlet problem in a rectangle obtained by gluing the lateral sides of the periodicity cell. These formulas show that the spectrum has spectral bands of length
It should be emphasized that waveguides with periodically perturbed boundaries have been the subject of research in the last decade: let us mention e.g. [34], [21], [22], [2] and [3] and the references therein. However the type of singular perturbation that we study in our paper has never been addressed. We consider a waveguide perforated by a periodic perforation string, which implies using a combination of homogenization methods and spectral perturbation theory.
As usual in waveguide theory, we first apply the Gelfand transform (cf. [6], [30], [33], [26], [11] and (11)) to convert the original problem, cf. (7), into a family of spectral problems depending on the Floquet-parameter
The above mentioned homogenization spectral problems have different boundary conditions from those considered in the literature (cf. [5], [14] and [16] for an extensive bibliography). Obtaining convergence for their spectra, correcting terms and precise bounds for discrepancies (cf. (10)), as
Let
| $ Π={x=(x1,x2):x1∈R,x2∈(0,H)} $ | (1) |
be a strip of width
| $ ¯ω=ω∪∂ω⊂ϖ0, $ | (2) |
where
| $ ϖ0=(−1/2,1/2)×(0,H)⊂Π. $ | (3) |
We also introduce the strip
| $ ωε(j,k)={x:ε−1(x1−j,x2−εkH)∈ω}with j∈Z,k∈{0,…,N−1}, $ | (4) |
where
| $ Πε=Π∖⋃j∈ZN−1⋃k=0¯ωε(j,k) $ | (5) |
is made equal to 1 by rescaling, and similarly, the period is made equal to
| $ \varpi^ \varepsilon = \varpi^0 \setminus\bigcup\limits_{k = 0}^{N-1}\overline{ \omega^ \varepsilon(0, k)}, $ |
(see b) in Figure 1). For brevity, we shall denote by
| $ ωε=⋃j∈ZN−1⋃k=0ωε(j,k), $ | (6) |
while
In the domain (5) we consider the Dirichlet spectral problem
| $ {−Δuε(x)=λεuε(x),x∈Πε,uε(x)=0,x∈∂Πε. $ | (7) |
The variational formulation of problem (7) refers to the integral identity
| $ (∇uε,∇v)Πε=λε(uε,v)Πε∀v∈H10(Πε), $ | (8) |
where
Problem (7) gets a positive cutoff value
| $ σε=⋃n∈NBεn, $ | (9) |
where
In Section 2 we address the setting of the Floquet parametric family of problems (13)-(16), obtained by applying the Gelfand transform (11) to the original problem (7). They are homogenization spectral problems in a perforated domain, the periodicity cell
| $ \Lambda_i^ \varepsilon(\eta)\to \Lambda_i^0 \, \quad \mbox{ as }\quad \varepsilon\to 0, \quad \forall \eta \in [-\pi, \pi], \quad i = 1, 2, \cdots. $ |
However, this result does not give information on the spectral gaps.
Using the method of matched asymptotic expansions for the eigenfunctions of the homogenization problems (cf. Section 4) we are led to the unit cell boundary value problem (31)-(33), the so-called local problem, that is, a problem to describe the boundary layer phenomenon. Section 3 is devoted to the study of this stationary problem for the Laplace operator, which is independent of
| $ |Λεi(η)−Λ0i−εΛ1i(η)|≤ciε3/2, $ | (10) |
with some
As a consequence, we deduce that the bands
| $ \left [\Lambda^{0}_{i}+ \varepsilon B^i_- -c_i \varepsilon^{3/2} \, , \, \Lambda^{0}_{i}+ \varepsilon B^i_+ + c_i \varepsilon^{3/2}\right], $ |
of length
Dealing with the precise length of the band, we note that the results rely on the fact that the elements of the antidiagonal of the polarization matrix do not vanish (cf. (70)-(75)), but this is a generic property for many geometries of the unit hole
Summarizing, Section 2 addresses some asymptotics for the spectrum of the Floquet-parameter family of spectral problems; Section 3 considers the unit cell problem; Section 4 deals with the asymptotic expansions; in Section 5.1, we formulate the main asymptotic results of the paper, while the proofs are performed in Section 5.2.
In this section, we deal with the setting of the Floquet-parameter dependent spectral problems and the limit behavior of their spectra, cf. Sections 2.1 and 2.2, respectively.
The Floquet-Bloch-Gelfand transform (FBG-transform, in short)
| $ uε(x)→Uε(x;η)=1√2π∑n∈Ze−inηuε(x1+n,x2), $ | (11) |
see [6] and, e.g., [30], [33], [11], [26] and [4], converts problem (7) into a
| $ ϖε={x∈Πε:|x1|<1/2} $ | (12) |
see Figure 1, b. Note that
| $ −ΔUε(x;η)=Λε(η)Uε(x;η),x∈ϖε, $ | (13) |
| $ Uε(x;η)=0,x∈Γε, $ | (14) |
| $ Uε(1/2,x2;η)=eiηUε(−1/2,x2;η),x2∈(0,H), $ | (15) |
| $ ∂Uε∂x1(12,x2;η)=eiη∂Uε∂x1(−12,x2;η),x2∈(0,H), $ | (16) |
where
The variational formulation of the spectral problem (13)-(16) reads:
| $ (∇Uε,∇V)ϖε=Λε(Uε,V)ϖεV∈H1,ηper(ϖε;Γε), $ | (17) |
where
| $ 0<Λε1(η)≤Λε2(η)≤⋯≤Λεm(η)≤⋯→∞, $ | (18) |
which are repeated according to their multiplicities (see Ch. 10 in [1] and Ch. 13 in [30]). The eigenfunctions are assumed to form an orthonormal basis in
The function
| $ η∈[−π,π]↦Λεm(η) $ | (19) |
is continuous and
| $ Bεm={Λεm(η):η∈[−π,π]} $ | (20) |
are closed, connected and bounded intervals of the real positive axis
A first approach to the asymptotics for eigenpairs of (13)-(16) is given by the following convergence result, that we show adapting standard techniques in homogenization and spectral perturbation theory: see, e.g., Ch. 3 in [27] for a general framework and [14] for its application to spectral problems in perforated domains with different boundary conditions. Let us recall
| $ ωε(0,0),…,ωε(0,N−1)⊂ϖ0. $ | (21) |
Theorem 2.1. Let the spectral problem (13)-(16) and the sequence of eigenvalues (18). Then, for any
| $ Λεm(η)→Λ0m,as ε→0, $ | (22) |
where
| $ 0<Λ01<Λ02≤⋯≤Λ0m≤⋯→∞,as m→∞, $ | (23) |
are the eigenvalues, repeated according to their multiplicities, of the Dirichlet problem
| $ −ΔU0(x)=Λ0U0(x),x∈υ,υ≡(0,1)×(0,H)U0(x)=0,x∈∂υ. $ | (24) |
Proof. First, for each fixed
| $ 0<C≤Λεm(η)≤Cm∀η∈[−π,π]. $ | (25) |
To obtain the lower bound in (25), it suffices to consider (17) for the eigenpair
| $ Λεm(η)=minEεm⊂H1,ηper(ϖε;Γε)maxV∈Eεm,V≠0(∇V,∇V)ϖε(V,V)ϖε, $ |
where the minimum is computed over the set of subspaces
Hence, for each
| $ Λεm(η)→Λ0m(η),Uεm(⋅;η)⇀U0m(⋅;η) in H1(ϖ0)−weak, as ε→0, $ | (26) |
for a certain positive
| $ \Vert U; L^2(\varpi^0\setminus {\overline \omega})\Vert \leq C \Vert \nabla U; L ^2 (\varpi^0\setminus {\overline \omega})\Vert \quad \forall U\in H^1(\varpi^0\setminus {\overline \omega}), \quad U = 0 \mbox{ on } \partial \omega, $ |
and we deduce
| $ \varepsilon^{-1} \Vert U_m^ \varepsilon (\cdot;\eta); L^2( \{\vert x_1\vert \leq { \varepsilon}/{2}\} \cap \varpi^0 ) \Vert^2 \leq C \varepsilon \Vert \nabla U_m^ \varepsilon (\cdot;\eta) ; L^2( \{\vert x_1\vert \leq { \varepsilon}/{2}\} \cap \varpi^0 ) \Vert^2 . $ |
Now, taking limits as
| $ −ΔU0m(x;η)=Λ0m(η)U0m(x;η),x1∈{(−1/2,0)∪(0,1/2)},x2∈(0,H),U0m(x;η)=0 for x2∈{0,H},x1∈(−1/2,1/2) and x1=0,x2∈(0,H),U0m(1/2,x2;η)=eiηU0m(−1/2,x2;η),x2∈(0,H),∂U0m∂x1(1/2,x2;η)=eiη∂U0m∂x1(−1/2,x2;η),x2∈(0,H), $ | (27) |
where the differential equation has been obtained by taking limits in the variational formulation (17) for
Now, from the orthonormality of
In addition, extending by
| $ u0m(x;η)={U0m(x;η),x1∈(0,1/2),eiηU0m(x1−1,x2;η),x1∈(1/2,1), $ | (28) |
we obtain a smooth function in the rectangle
Remark 1. Note that the eigenpairs of (24) can be computed explicitly
| $ Λ0np=π2(n2+p2H2),U0np(x)=2√Hsin(nπx1)sin(pπx2/H),p,n∈N. $ | (29) |
The eigenvalues
In this section, we study the properties of certain solutions of the boundary value problem in the unbounded strip
In order to obtain a corrector for the approach to the eigenpairs of (13)-(16) given by Theorem 2.1, we introduce the stretched coordinates
| $ ξ=(ξ1,ξ2)=ε−1(x1,x2−εkH). $ | (30) |
which transforms each opening of the string
| $ −ΔξW(ξ)=0,ξ∈Ξ, $ | (31) |
with the periodicity conditions
| $ W(ξ1,H)=W(ξ1,0),∂W∂ξ2(ξ1,H)=∂W∂ξ2(ξ1,0),ξ1∈R, $ | (32) |
and the Dirichlet condition on the boundary of the hole
| $ W(ξ)=0,ξ∈∂ω. $ | (33) |
Regarding (31)-(33), it should be noted that, for any
| $ \Delta_x+\Lambda^ \varepsilon = \varepsilon^{-2} (\Delta_\xi + \varepsilon^2 \Lambda^ \varepsilon), $ |
and
| $ w(x2)W(ε−1x), $ | (34) |
for the boundary layer. Here,
It is worth recalling that, according to the general theory of elliptic problems in domains with cylindrical outlets to infinity, cf., e.g., Ch. 5 in [26], problem (31)-(33) has just two solutions with a linear polynomial growth as
| $ ¯ω⊂(−R,R)×(0,H) $ | (35) |
and define the cut-off functions
| $ χ±(y)={1, for ±y>2R,0, for ±y<R, $ | (36) |
where the subindex
Proposition 3.1. There are two normalized solutions of (31)-(33) in the form
| $ W±(ξ)=±χ±(ξ1)ξ1+∑τ=±χτ(ξ1)pτ±+˜W±(ξ),ξ∈Ξ, $ | (37) |
where the remainder
| $ p(Ξ)=(p++(Ξ)p+−(Ξ)p−+(Ξ)p–(Ξ)). $ | (38) |
Proof. The existence of two linearly independent normalized solutions
Let us consider the functions
| $ ˆW±(ξ)=W±(ξ)∓χ±(ξ1)ξ1, $ | (39) |
which, obviously, satisfy (32), (33) and
| $ −ΔξˆW±(ξ)=F±(ξ),ξ∈Ξ, $ | (40) |
with
Let
| $ \|W, \mathcal{H}\| = \|\nabla_y W ;L^2(\Xi)\|. $ |
The variational formulation of (40), (32) and (33) reads: to find
| $ (∇yˆW±,∇yV)Ξ=(F±,V)Ξ∀V∈H. $ | (41) |
Since
In addition, since for each
| $ \widehat W^\tau (\xi) = c^\tau_\pm+ O(e^{-(\pm\xi_1) 2\pi/H}) \mbox{ as } \pm\xi_1\to +\infty , $ |
where the constants
| $ cτ±=limT→∞1H∫H0ˆWτ(±T,ξ2)dξ2=limT→∞1H∫H0(Wτ(±T,ξ2)−τδτ,±T)dξ2. $ | (42) |
Obviously,
In this section, we detect certain properties of the matrix
Proposition 3.2. The matrix
Proof. We represent (37) in the form
| $ W±(ξ)=W±0(ξ)+{±ξ1−R,±ξ1>R,0,±ξ1<R. $ | (43) |
The function
| $ [W_0^\pm]_\pm(\xi_2) = 0, \quad \left[\frac{\partial W_0^\pm}{\partial|\xi_1|}\right]_\pm(\xi_2) = -1, \quad \xi_2\in (0, H), $ |
where
In what follows, we write the equations for
| $ ∫H0Wτ0(±R,ξ2)dξ2=−∫H0Wτ0(±R,ξ2)[∂W±0∂|ξ1|]±(ξ2)dξ2=−(∇ξWτ0,∇ξW±0)Ξ. $ | (44) |
On the other hand, on account of (43) and the definition of
| $ W^\tau_0(\pm R , \xi_2) = W^\tau(\pm R , \xi_2) \quad \mbox { and } \quad \left[\frac{\partial W^\tau}{\partial|\xi_1|}\right]_\pm(\xi_2) = 0. $ |
Consequently, we can write
| $ ∫H0Wτ0(±R,ξ2)dξ2=−∫H0Wτ(±R,ξ2)[∂W±0∂|ξ1|]±(ξ2)dξ2=∫H0(Wτ(±R,ξ2)[∂W±0∂|ξ1|]±(ξ2)−W±0(±R,ξ2)[∂Wτ∂|ξ1|]±(ξ2))dξ2, $ |
and using again the Green formula for
| $ ∫H0Wτ0(±R,ξ2)dξ2=+limT→∞∫H0(Wτ(τT,ξ2)∂W±0∂|ξ1|(τT,ξ2)−W±0(τT,ξ2)∂Wτ∂|ξ1|(τT,ξ2))dξ2=−H(pτ±(Ξ)+δτ,±R). $ | (45) |
Here, we have used the following facts:
| $ W^\pm_0(\xi) = \chi_\pm(\xi_1)\left(p_{\pm\pm}+R\right) + \chi_\mp(\xi_1)p_{\mp\pm}+\widetilde{W}^\pm(\xi). $ |
Considering (44) and (45) we have shown the equality for the Gram matrix
| $ \left(\nabla_\xi W^\tau_0, \nabla_\xi W^\pm_0\right)_\Xi = H\left(p_{\tau\pm}(\Xi) + \delta_{\tau, \pm}R\right), $ |
which gives the symmetry and the positiveness of the matrix
Let us note that our results above apply for Lipschitz domains or even cracks as it was pointed out in Section 2.1. Now, we get the following results in Propositions 3.3 and 3.4 depending on whether
Proposition 3.3. Let
| $ H\left(2p_{+-}-p_{++}-p_{–}\right) > mes_2(\omega). $ |
Proof. We consider the linear combination
| $ W_0(\xi) = W^+(\xi)-W^-(\xi)-\xi_1 = \chi_+(\xi_1)\left(p_{++}-p_{+-}\right)-\chi_-(\xi_1)\left(p_{–}-p_{-+}\right)+\widetilde{W}_0(\xi). $ |
It satisfies
| $ -\Delta_\xi W_0(\xi) = 0, \, \xi\in\Xi, \qquad W_0(\xi) = -\xi_1, \, \xi\in\partial\omega, $ |
with the periodicity conditions in the strip, and
| $ 0<‖∇W0;L2(Ξ)‖2+mes2(ω)=−∫∂ωξ1∂ν(ξ1)dν+∫∂ωW0∂ν(W0(ξ))dν=−∫∂ωξ1∂ν(ξ1+W0(ξ))dν=∫∂ω(∂νξ1(ξ1+W0(ξ))−ξ1∂ν(ξ1+W0(ξ)))dν=−limT→∞∑±±∫H0W0(±T,ξ2)dξ2=−H(p+++p–−p+−−p−+). $ |
Remark 2. observe that for a hole
| $ p++=p–. $ | (46) |
Indeed, this is due to the fact that each one of the two normalized solutions in (37) are related with each other by symmetry. Also, we note that, on account of Proposition 3.2, the symmetry
Proposition 3.4. Let
| $ p+−=p−+>0. $ | (47) |
In addition,
Proof. First, let us note that due to the symmetry
| $ W−(ξ1,ξ2)={−ξ1+W∗(−ξ1,ξ2),ξ1<0,W∗(ξ1,ξ2),ξ1>0. $ | (48) |
where
| $ −ΔξW∗(ξ)=0, for ξ∈Π+,W∗(0,ξ2)=0, for ξ2∈(h,H−h),−∂ξ1W∗(0,ξ2)=1/2, for ξ2∈(0,h)∪(H−h,H). $ | (49) |
Indeed, denoting by
| $ [\widetilde W^* ] (0, \xi_2) = 0, \quad \left[\frac{\partial\widetilde W^* }{\partial \xi_1}\right] (0, \xi_2) = -1, $ |
and hence, the function on the right hand side of (48) is a harmonic function in
Now, considering (49), integrating by parts on
| $ \int\limits_{\Upsilon(0)} W^*(0, \xi_2)d\xi_2 = \lim\limits_{T\to\infty}\int\limits^H_0 W^*(T, \xi_2)d\xi_2 = H p_{-+}(\Xi). $ |
Similarly, from (49), we get
| $ 0 = -\int\limits_{\Pi^+} W^*(\xi)\Delta_\xi W^*(\xi) d\xi = \int\limits_{\Pi^+} |\nabla_\xi W^*(\xi)|^2 d\xi -\frac{1}{2}\int\limits_{\Upsilon(0)} W^*(0, \xi_2)d\xi_2. $ |
Therefore, we deduce
| $ H2p−+(Ξ)=∫Π+|∇ξW∗(ξ)|2dξ>0 $ | (50) |
and from the symmetry of
Also, from the definition (48), we have
From Proposition 3.4, note that when
| $ ¯ω={ξ:δ−2ξ21+(ξ2−H/2)2≤τ2},τ=H/2−h, $ | (51) |
(47) holds true, for a small
In this section we construct asymptotic expansions for the eigenpairs (
Let
| $ Λεm=Λ0m+εΛ1m(η)+⋯. $ | (52) |
To construct asymptotics of the corresponding eigenfunctions
| $ Uεm(x;η)=U0m(x;η)+εU1m(x;η)+⋯ $ | (53) |
as the outer expansion, and
| $ Uεm(x;η)=ε∑±wm±(x2;η)W±(xε)+⋯ $ | (54) |
as the inner expansion near the perforation string, cf. (4) and (21).
Above,
| $ U0m(x;η)={U0m(x),x1∈(0,1/2),e−iηU0m(x1+1,x2),x1∈(−1/2,0), $ | (55) |
First, let us notice that
| $ Uεm(x;η)=0+x1∂U0m∂x1(0,x2)+εU1m(+0,x2;η)+⋯,x1>0,Uεm(x;η)=0+x1e−iη∂U0m∂x1(1,x2)+εU1m(−0,x2;η)+⋯,x1<0, $ | (56) |
where, for second formula (56), we have used (55).
The inner expansion (54) is processed by means of decompositions (37). We have
| $ Uεm(x;η)=εwm+(x2;η)(ξ1+p++)+εwm−(x2;η)p−++⋯,ξ1>0,Uεm(x;η)=εwm−(x2;η)(−ξ1+p–)+εwm+(x2;η)p+−+⋯,ξ1<0. $ | (57) |
Recalling relationship between
| $ wm+(x2;η)=∂U0m∂x1(0,x2),wm−(x2;η)=−e−iη∂U0m∂x1(1,x2), $ | (58) |
and also obtain the equalities
| $ U1m(+0,x2;η)=∑τ=±wmτ(x2;η)pτ+,U1m(−0,x2;η)=∑τ=±wmτ(x2;η)pτ−. $ | (59) |
Formulas (58) define coefficients of the linear combination (54) while formulas (59) are the boundary conditions for the correction term in (53). Moreover, inserting ans
| $ {−ΔxU1m(x;η)−Λ0mU1m(x;η)=Λ1m(η)U0m(x;η),x∈ϖ0,x1≠0,U1m(x1,H;η)=U1m(x1,0;η)=0,x1∈(−1/2,0)∪(0,1/2), $ | (60) |
and the quasi-periodic conditions with
Since
| $ ∫ϖ0Λ1m(η)U0m(x;η)¯U0m(x;η)dx=H∫0U1m(−0,x2;η)¯∂U0m∂x1(−0,x2;η)dx2−H∫0U1m(+0,x2;η)¯∂U0m∂x1(+0,x2;η)dx2. $ |
Thus, by (55) and (59), the only compatibility condition in (60) (recall that
| $ Λ1m(η)=−H∫0¯Bm(x2;η)⋅p(Ξ)Bm(x2;η)dx2 $ | (61) |
where
| $ Bm(x2;η)=(∂U0m∂x1(0,x2),−e−iη∂U0m∂x1(1,x2))T∈C2, $ | (62) |
and it determines uniquely the second term of the ansatz (52). Here and in what follows, the top index
Also, from (53), (54) and (57) the composite expansion approaching
| $ Uεm(x;η)≈U0m(x;η)+εU1m(x;η)+ε∑τ=±wmτ(x2;η)Wτ(xε)−(εwm±(x2;η)(ε−1|x1|+p±±)+εwm∓(x2;η)p∓±),±x1≥0. $ | (63) |
We address the case where
| $ Λεm+l(η)→Λ0m+l as ε→0, for l=0,⋯,κm−1. $ | (64) |
Let
Following Section 4.1, for each
| $ Λεm+l=Λ0m+εΛ1m+l(η)+⋯, $ | (65) |
the outer expansion for
| $ Uεm+l(x;η)=U0m+l(x;η)+εU1m+l(x;η)+⋯, $ | (66) |
and the inner expansion
| $ Uεm(x;η)=ε∑±wm+l±(x2;η)W±(xε)+⋯, $ | (67) |
where the terms
By repeating the reasoning in Section 4.2, we obtain formulas for the above mentioned terms in (65), (66) and (67) by replacing index
| $ -\Delta_x U^{1}_{m+l}(x;\eta)-\Lambda^{0}_{m} U^{1}_{m+l}(x;\eta) = \Lambda^{1}_{m+l}(\eta) U^{0}_{m+l}(x;\eta), \quad x\in \varpi^0, \, x_1\not = 0, $ |
and integrating by parts, we obtain
| $ ∫ϖ0Λ1m+l(η)U0m+l(x;η)¯U0m+l′(x;η)dx=−H∫0(∂U0m+l′∂x1(0,x2),−eiη∂U0m+l′∂x1(1,x2))⋅p(Ξ)×(∂U0m+l∂x1(0,x2),−e−iη∂U0m+l∂x1(1,x2))Tdx2. $ |
Since the eigenfunctions
| $ \int\limits^H_0 \frac{\partial {U^{0}_{m+l' }}}{\partial x_1}(x_1^*, x_2)\frac{\partial U^{0}_{m+l }}{\partial x_1}(x_1^*, x_2)dx_2 = 0, \mbox{ with } x_1^*\in \{0, 1\}, \quad l\not = l', $ |
and, hence, for each
| $ Λ1m+l(η)=−H∫0¯Bm+l(x2;η)⋅p(Ξ)Bm+l(x2;η)dx2, $ | (68) |
where
| $ Bm+l(x2;η)=(∂U0m+l∂x1(0,x2),−e−iη∂U0m+l∂x1(1,x2))T. $ | (69) |
Therefore we have determined completely all the terms in the asymptotic ans
In this section, we justify the results obtained by means of matched asymptotic expasions in Section 4. Since the case in which all the eigenvalues of the Dirichlet problem (24) are simple can be a generic property, we first consider this case, cf. Theorem 5.1 and Corollary 5.1, and then the case in which these eigenvalues have a multiplicity greater than
Theorem 5.1. Let
| $ |Λεm(η)−Λ0m−εΛ1m(η)|≤cmε3/2 $ | (70) |
and there are no other different eigenvalues in the sequence (18) satisfying (70).
Theorem 5.1 shows that
In order to detect the gaps between consecutive spectral bands (20) it is worthy writing formulas
| $ Λ1m(η)=B0(m)+B1(m)cos(η), with B0(m)=H∫0(p++|∂U0m∂x1(0,x2)|2+p–|∂U0m∂x1(1,x2)|2)dx2,B1(m)=2p+−H∫0∂U0m∂x1(0,x2)∂U0m∂x1(1,x2)dx2, $ | (71) |
which are obtained from (61) and (62). Formula (29) demonstrates that
| $ B_0(m) = \left(p_{++}+p_{–}\right)\int\limits^H_0\left|\frac{\partial U^{0}_{m }}{\partial x_1}(0, x_2)\right|^2dx_2, $ |
and that the integral in
Remark 3. Let us consider that the eigenvalue
| $ B_0(m) = 2\left(p_{++}+p_{–}\right)n^2\pi^2, \quad B_1(m) = (-1)^n 4 p_{+-} n^2\pi^2, $ |
and, consequently,
| $ Λ1m(η)=2(p+++p–)n2π2+(−1)n4p+−n2π2cos(η). $ | (72) |
Corollary 5.1. Under the hypothesis of Theorem 5.1, the endpoints
| $ |Bε±(m)−Λ0m−ε(B0(m)±|B1(m)|)|≤cmε3/2. $ | (73) |
Hence, the length of the band
Note that for the holes such that the polarization matrix (38) satisfies
Theorem 5.2. Let
| $ |Λεm+l0(η)−Λ0m−εΛ1m+l(η)|≤cmε3/2. $ | (74) |
In addition, when
Corollary 5.2. Under the hypothesis in Theorem 5.2, the spectral bands
| $ [Λ0m+εmin0≤l≤κm−1η∈[−π,π]Λ1m+l(η)−cmε3/2,Λ0m+εmax0≤l≤κm−1η∈[−π,π]Λ1m+l(η)+cmε3/2]. $ | (75) |
Hence, the length of the the bands
Remark 4. Under the hypothesis of Theorem 5.2, it may happen that, for
Remark 5. Notice that the positive cutoff value
In this section we prove the results of Theorems 5.1 and 5.2 and of their respective corollaries.
Proof of Theorem 5.1. Let us fix
| $ ⟨Tε(η)Uε,Vε⟩=(Uε,Vε)ϖε∀Uε,Vε∈H1,ηper(ϖε;Γε). $ | (76) |
The integral identity (17) for problem (13)-(16) can be rewritten as the abstract equation
| $ T^ \varepsilon(\eta) U^ \varepsilon(\cdot;\eta) = \tau^ \varepsilon(\eta) U^ \varepsilon(\cdot;\eta) \quad \hbox{in } H^{1, \eta}_{per} (\varpi^ \varepsilon;\Gamma^ \varepsilon), $ |
with the new spectral parameter
| $ τε(η)=(1+Λε(η))−1. $ | (77) |
Since
| $ \left\{\tau^{ \varepsilon}_{m}(\eta) = (1 +\Lambda^{ \varepsilon}_{m}(\eta) )^{-1}\right\}_{m\in {\mathbb N }}. $ |
For the point
| $ tεm(η)=(1+Λ0m+εΛ1m(η))−1, $ | (78) |
cf. (52) and (61), we construct a function
| $ ‖Uεm;H1,ηper(ϖε;Γε)‖≥cm, $ | (79) |
| $ ‖Tε(η)Uεm−tεm(η)Uεm;H1,ηper(ϖε;Γε)‖≤Cmε3/2, $ | (80) |
where
| $ \|(T^ \varepsilon(\eta) -t^{ \varepsilon}_{m}(\eta))^{-1}; H^{1, \eta}_{per} (\varpi^ \varepsilon;\Gamma^ \varepsilon)\to H^{1, \eta}_{per} (\varpi^ \varepsilon;\Gamma^ \varepsilon) \|\geq \mathbf{c}_m^{-1} \varepsilon^{-3/2}, $ |
with
| $ \operatorname{dist}(t^{ \varepsilon}_{m}(\eta), \sigma(T^ \varepsilon(\eta)) = \|(T^ \varepsilon(\eta) -t^{ \varepsilon}_{m}(\eta))^{-1}; H^{1, \eta}_{per} (\varpi^ \varepsilon;\Gamma^ \varepsilon)\to H^{1, \eta}_{per} (\varpi^ \varepsilon;\Gamma^ \varepsilon) \|^{-1} $ |
supported by the spectral decomposition of the resolvent (cf., e.g., Section V.5 in [9] and Ch. 6 in [1]), we deduce that the closed segment
| $ [t^{ \varepsilon}_{m}(\eta)- \mathbf{c}_m \varepsilon^{3/2}, t^{ \varepsilon}_{m}(\eta) + \mathbf{c}_m \varepsilon^{3/2}] $ |
contains at least one eigenvalue
| $ |(1+Λεp(η))−1−(1+Λ0m+εΛ1m(η))−1|≤cmε3/2. $ | (81) |
Then, simple algebraic calculations (cf. (81) and (25)) show that, for a
| $ |Λεp(η)−Λ0m−εΛ1m(η)|≤Cmε3/2 $ | (82) |
is satisfied with a constant
To conclude with the proof of Theorem 5.1, there remains to present a function
| $ Vεmout(x;η)=U0m(x;η)+εU1m(x;η), $ | (83) |
where
| $ Vεmin(x;η)=ε∑±wm±(x2;η)W±(ε−1x), $ | (84) |
and
| $ Vεmmat(x;η)=εwm±(x2;η)(ε−1|x1|+p±±)+εwm∓(x2;η)p∓±,±x1>0, $ | (85) |
with
| $ Uεm(x;η)=Xε(x1)Vεmout(x;η)+X(x1)Vεmin(x;η)−Xε(x1)X(x1)Vεmmat(x;η), $ | (86) |
where
| $ Xε(x1)={1, for |x1|>2Rε,0, for |x1|<Rε, and X(x1)={1, for |x1|<1/6,0, for |x1|>1/3. $ | (87) |
Note that (85) takes into account components in both expressions (83) and (84), but the last subtrahend in
First, let us show that
First of all, we recall (83) and (87) to derive
| $ ‖Uεm;H1,ηper(ϖε;Γε)‖≥‖Uεm;L2((1/3,1/2)×(0,H))‖=‖Vεmout;L2((1/3,1/2)×(0,H))‖≥‖U0m;L2((1/3,1/2)×(0,H))‖−ε‖U1m;L2((1/3,1/2)×(0,H))‖≥c>0, $ |
for a small
Using (76) and (78), we have
| $ ‖Tε(η)Uεm−tεm(η)Uεm;H1,ηper(ϖε;Γε)‖=sup|⟨Tε(η)Uεm−tεm(η)Uεm,Wε⟩|=(1+Λ0m+εΛ1m(η))−1sup|(∇Uεm,∇Wε)ϖε−(Λ0m+εΛ1m(η))(Uεm,Wε)ϖε|, $ | (88) |
where the supremum is computed over all
| $ \| \mathcal{W}^{ \varepsilon}; H^{1, \eta}_{per} (\varpi^ \varepsilon;\Gamma^ \varepsilon)\|\leq 1. $ |
Taking into account the Dirichlet conditions on
| $ \int_{ \Pi_T\setminus\overline{\omega} } \vert U \vert^2\, dy \leq C_{T}\int_{ \Pi_T\setminus\overline{\omega} } \vert \nabla_y U \vert^2 dy \quad \forall U \in H^1( \Pi_T\setminus\overline{\omega} ) , \, U = 0 \mbox{ on } \partial \omega, $ |
where
| $ \int_0^\infty \frac{1}{t^2} z(t)^2\, dt\leq 4 \int_0^\infty \left\vert \frac{dz}{dt}(t)\right\vert^2\, dt \quad \forall z\in C^1[0, \infty), \, z(0) = 0. $ |
Then, we have
| $ ‖(ε+|x1|)−1Wε;L2(ϖε)‖≤c‖∇Wε;L2(ϖε)‖≤c. $ | (89) |
Clearly, from (71),
| $ |(∇Uεm,∇Wε)ϖε−(Λ0m+εΛ1m(η))(Uεm,Wε)ϖε|=|(ΔUεm+(Λ0m+εΛ1m(η))Uεm,Wε)ϖε|. $ |
On the basis of (83)-(86) we write
| $ ΔUεm+(Λ0m+εΛ1m(η))Uεm=Xε(ΔU0m+Λ0mU0m+ε(ΔU1m+Λ0mU1m+Λ1mU0m)+ε2Λ1mU1m)+[Δ,Xε](Vεmout−Vεmmat)+X(ΔVεmin−XεΔVεmmat)+[Δ,X](Vεmin−Vεmmat)+(Λ0m+εΛ1m)X(Vεmin−XεVεmmat)=:Sε1+Sε2+Sε3+Sε4+Sε5. $ | (90) |
Here,
Considering
| $ \left|(S^ \varepsilon_1, \mathcal{W}^ \varepsilon)_{\varpi^ \varepsilon}\right|\leq \varepsilon^2 \Lambda^{1}_{m}(\eta) \|U^{1}_{m} ; L^2(\varpi^ \varepsilon)\|\| \mathcal{W}^{ \varepsilon}; L^2(\varpi^ \varepsilon)\|\leq C_m \varepsilon^2. $ |
As regards
| $ |Vεmout(x;η)−Vεmmat(x;η)|≤c(|x1|2+ε|x1|),|∂Vεmout∂x1(x;η)−∂Vεmmat∂x1(x;η)|≤c(|x1|+ε),±x1∈[εR,2εR]. $ |
Above, we have also used the smoothness of the function
| $ |(Sε2,Wε)ϖε|≤‖Sε2;L2(ϖεεR)‖‖Wε;L2(ϖεεR)‖≤cε‖(ε+|x1|)−1Wε;L2(ϖε)‖×(H∫02εR∫εR(1ε2|Vεmout∂x1−Vεmmat∂x1|2+1ε4|Vεmout−Vεmmat|2)d|x1|dx2)12≤c(1ε2ε2+1ε4ε4)12(mes2ϖεεR)12ε‖(ε+|x1|)−1Wε;L2(ϖε)‖≤cε32. $ |
Dealing with
| $ ΔVεmin(x;η)−Xε(x1)ΔVεmmat(x;η)=2∑±∂wm±∂x2(x2;η)∂W±∂ξ2(y)+ε∑±∂2wm±∂x22(x2;η)˜W±(y), $ |
when
| $ |(Sε3,Wε)ϖε|≤c(‖(ε+|x1|)∂W±∂ξ2;L2(ϖε)‖+ε‖(ε+|x1|)˜W±;L2(ϖε)‖)‖1ε+|x1|Wε;L2(ϖε)‖≤c(1/2∫0(ε+t)2e−2δt/εdt)12‖∇Wε;L2(ϖε)‖≤cε32. $ |
Above, obviously, we take the positive constant
| $ \left|(S^ \varepsilon_5, \mathcal{W}^ \varepsilon)_{\varpi^ \varepsilon}\right|\leq c \varepsilon^\frac{3}{2}. $ |
Moreover, the supports of the coefficients
| $ \left|(S^ \varepsilon_4, \mathcal{W}^ \varepsilon)_{\varpi^ \varepsilon}\right|\leq c{e^{-2\delta/(3 \varepsilon) }}. $ |
Revisiting the obtained estimates we find the worst bound
The fact that the constants
| $ −(∂∂y1+iη)(∂∂y1+iη)−∂2∂y22, $ | (91) |
and therefore, performing this change in (60), gives the solution
Proof of Corollary 5.1. Due to the continuity of the function (19), the maximum and minimum of
In order to show (73) for the maximum
| $ \Lambda^{0}_{m}+ \varepsilon B_0(m) + \varepsilon |B_1(m)|-c_m \varepsilon^{3/2} \leq \Lambda_m^{ \varepsilon}(\eta^+) \leq \Lambda^{0}_{m}+ \varepsilon B_0(m) + \varepsilon |B_1(m)| + c_m \varepsilon^{3/2} $ |
and
| $ \Lambda^{0}_{m} + \varepsilon \Lambda^1_m(\eta^+_{ \varepsilon, m}) -c_m \varepsilon^{3/2} \leq\Lambda_m^{ \varepsilon}(\eta^+_{ \varepsilon, m}) \leq \Lambda^{0}_{m}+ \varepsilon \Lambda^1_m(\eta^+_{ \varepsilon, m}) + c_m \varepsilon^{3/2}. $ |
Consequently, from (71), we derive
| $ Λ0m+εB0(m)+ε|B1(m)|−cmε3/2≤Λεm(η+)≤Λεm(η+ε,m)≤Λ0m+εB0(m)+ε|B1(m)|+cmε3/2, $ |
which gives (73) for
We proceed in a similar way for the minimum
| $ \left [\Lambda^{0}_{m}+ \varepsilon B_0(m) - \varepsilon |B_1(m)|-c_m \varepsilon^{3/2} \, , \, \Lambda^{0}_{m}+ \varepsilon B_0(m) + \varepsilon |B_1(m)| + c_m \varepsilon^{3/2}\right] $ |
Therefore, the whole band
Proof of Theorem 5.2. This proof holds exactly the same scheme of Theorem 5.1. Indeed, for each
| $ |Λεp(η)−Λ0m−εΛ1m+l(η)|≤Cm,lε3/2 $ | (92) |
is satisfied for a certain natural
We use a classical argument of contradiction (cf. [15] and [25]). We consider the set of functions
| $ ‖Uεm+l;H1,ηper(ϖε;Γε)‖≥˜cm and |⟨Uεm+l,Uεm+l′⟩|≤˜Cmε1/2, with l≠l′, $ | (93) |
for certain constants
Then, we define
| $ |(1+Λεp(l)(η))−1−(1+Λ0m+εΛ1m+l(η))−1|≤˜cmε3/2, $ | (94) |
for a constant
| $ ‖˜Wεm+l−˜Uεm+l;H1,ηper(ϖε;Γε)‖≤˜Cm, $ | (95) |
where
| $ \left \| \, \widetilde{\mathcal{U }}^ \varepsilon_{m+l} - \mathcal{W}^ \varepsilon_{m+l} ; H^{1, \eta}_{per} (\varpi^ \varepsilon;\Gamma^ \varepsilon) \right \|\leq \widetilde{\mathbf{c }}_m^{-1} \max\limits_{0\leq l\leq \kappa_m-1} \mathcal{C}_{l, m} , $ |
and some straightforward computation (cf., eg., Lemma 1 in Ch. 3 of [27]). Now, from (93) and (95) and straightforward computations we obtain
| $ |⟨˜Wεm+l,˜Wεm+l′⟩|≤5˜Cm with l≠l′, $ | (96) |
and this allows us to assert that set
| $ \sum\limits_{l = 0}^{\kappa_m-1} \alpha_l^ \varepsilon \widetilde{\mathcal{W }}^ \varepsilon_{m+l} = 0. $ |
Let us consider
| $ \langle \widetilde{\mathcal{W }}^ \varepsilon_{m}, \widetilde{\mathcal{W }}^ \varepsilon_{m} \rangle \leq \sum\limits_{l = 1}^{\kappa_m-1} \left\vert \frac{\alpha_l^ \varepsilon}{\alpha_0^ \varepsilon} \right\vert \left\vert \langle \widetilde{\mathcal{W }}^ \varepsilon_{m+l}, \widetilde{\mathcal{W }}^ \varepsilon_{m} \rangle \right \vert \leq (\kappa_m-1) 5\widetilde{\mathcal{C }}_m . $ |
Now, setting
Thus,
| 1. | Delfina Gómez, Sergei A. Nazarov, Rafael Orive-Illera, María-Eugenia Pérez-Martínez, Spectral gaps in a double-periodic perforated Neumann waveguide, 2023, 131, 18758576, 385, 10.3233/ASY-221776 | |
| 2. | D. Gómez, S. A. Nazarov, R. Orive-Illera, M.-E. Pérez-Martínez, Remark on Justification of Asymptotics of Spectra of Cylindrical Waveguides with Periodic Singular Perturbations of Boundary and Coefficients, 2021, 257, 1072-3374, 597, 10.1007/s10958-021-05506-z | |
| 3. | Sergei A. Nazarov, Jari Taskinen, Band-gap structure of the spectrum of the water-wave problem in a shallow canal with a periodic family of deep pools, 2023, 36, 1139-1138, 263, 10.1007/s13163-021-00420-z | |
| 4. | D. Gómez, S. A. Nazarov, R. Orive-Illera, M. -E. Pérez-Martínez, 2022, Chapter 7, 978-3-031-07170-6, 95, 10.1007/978-3-031-07171-3_7 | |
| 5. | Delfina Gómez, Sergei A. Nazarov, Rafael Orive‐Illera, María‐Eugenia Pérez‐Martínez, Asymptotic stability of the spectrum of a parametric family of homogenization problems associated with a perforated waveguide, 2023, 0025-584X, 10.1002/mana.202100589 | |
| 6. | D. Gómez, S. A. Nazarov, M.-E. Pérez-Martínez, Pointwise Fixation along the Edge of a Kirchhoff Plate, 2023, 1072-3374, 10.1007/s10958-023-06862-8 |
a) The perforated strip
The strip