Stochastic homogenization on perforated domains III–General estimates for stationary ergodic random connected Lipschitz domains

1.   Introduction

Cellular ceramic is defined as highly porous solid materials and it has been widely studied, due to their specific properties such as low relative density, low thermal conductivity and high surface specific area, thanks to their porosities, 60 to over 90% of the total volume of solid ^[1,2].These properties are strongly dependent on microstructural of the materials utilized, porosity, shape, distribution and cell size, also the interconnectivity between the cells ^[3,4]. It is believed that such as characteristics are essential in many technological applications, such as thermal insulation, purification and filters for fluids and gases, supports for catalysts, and also in the biomaterials ^[3,5,6].

It was stated that the various methods which give different microstructural features are mainly dependent on the preparation conditions used for cellular ceramic production ^[7]. In this way, several methods of elaboration of porous materials were proposed, including; emulsification of ceramic suspensions with volatile alkanes ^[8], gel casting ^[9,10], and melted paraffin ^[11], in which burnt during the heating process and thus cause pores in the ceramic ^{[12,13,14,15]}. However, these methods are considered as non-reproducible methods, where the samples should undergo dimensional new corrections by machining, which in turn can destroy the ceramic products.

The kaolins as primary clay materials, were obtained from Djebel Debbagh site, Bechar Tabelbala site, Boudouaou site, and processed to produce cellular ceramic bricks using a foaming agent that burnt out during heat treatment and resulting in formation of pores, in which demonstrate the feasibility of producing porous ceramic from these clay materials.

This method facilitates the reproducibility of the manufacture of porous materials having the same porous volume and an only size of the cell. Also, it makes possible to envisage the final density with acceptable precision. Indeed, it is easy to calculate the density by knowing the volume of the casting mold, the content of dry matter, the loss on ignition and the voluminal withdrawal, which the sample undergoes during drying and heating stages.

2.   Materials and methods

2.1.   Raw materials

In this study, three different clays of Algerian origin were used as raw materials for investigating the reproducibility of the method of elaboration of cellular ceramic bricks developed. (i) Clay derived from Djebel Debbagh in Eastern Algeria (Guelma region, Algeria) named DD3. This clay material is rich in alumina (>38 wt%), while other elements oxides such as K₂O, Na₂O, and Fe₂O₃ do not exceed 0.5 wt%. It has been reported that this clay can be an excellent material for manufacturing of firebricks ^[16,17]. Its main minerals are kaolinite, gibbsite which strengthen the alumina content (Al₂O₃) and todorokite (mineral carrying manganese). (ii) The ferruginous clay of Bechar “Tabelbala site”, in Western Algeria, noted KT. Its sedimentary geological formation comprises great quantities of sand and of iron that gives the reddish color to this material. The predominant clay minerals of the Tabelbala kaolin are kaolinite, muscovite and illite. Alumina (Al₂O₃) reaches 25 wt%, the silica exceeds 50 wt%, and the mass percentage of the other elements such as iron compounds is above 4 wt%. According to these characteristics, this clay has medium refractoriness, in which it can be used for the fabrication of thermal insulating bricks. (iii) The argillaceous materials from the central region of Algeria, “Boudouaou site”, noted KB. This clay consists of montmorillonite, illite and chlorite which confer high plasticity to this clay.

Their chemical compositions as shown in Table 1 reveal the significant impurities, which limit its utilization in luxury porcelain and sanitary ware where brightness and whiteness are required. The first kaolin named “DD3”, polluted with manganese oxide (MnO ≥ 1.34%) that gives a blackish coloring to this clay. The second and third named “KT and KB” are ferruginous kaolin Fe₂O₃ (4.30, 5.31%) respectively.

2.2.   Description of method

The elaboration of cellular ceramic bricks was carried out by the following steps:

(1) Preparation of barbotine of clay and chamotte. A deflocculating agent was added to optimise the highest density of barbotine associated with a minimal tenor of water. Chamotte was obtained by the calcination of clay at 900 ℃. The use of chamotte allowed decreasing the large contraction, which undergo the clays during the firing process, in which deformations and damages to the products has been occured. The optimal mixes of clays and chamotte were optimised for avoiding the deformations and guarantee a minimal solidity at dry stage. (2) 15 cm³ of a commercially foamed agent (commercial air entraining agent from Algerian society GRANITEX, the MEDA-AIR EN 934-2, to generate air bubbles in water) was added to obtain the foamed slurry by using a mixing technique of energetic mechanical agitation. (3) The last added solid material was a mineral binder, such as refractory cement or Portland cement. This mineral binder accelerated the drying process of the cast foamed slurry without collapse, and provides solidity to the dry sample. The optimal quantity of cement which can avoid collapse is ~10% in weight based on the weight of solid materials. (4) Pouring the foamed slurry into parallelepiped metallic moulds for drying at room temperature for 24 h, followed by total drying in the oven at 80 ℃. (5) Sintering of the dried specimens was carried out at 1100, 1100 and 900 ℃, respectively for 2 h.

To investigate the reproducibility and the precision of this method, five formulations of foamed slurries were prepared with the same weight of solid materials. The quantity of solid materials (SM) was kept unchanged for all formulations; it consisted of 90 g of clay and chamotte (30g, 60g respectively), and 10 g of mineral binder. To decrease the bulk densities of the cellular specimens, the volume of water (W) was increased, which in turn increased the volume of the foamed slurry. The quantity of total water should be sufficient to form the barbotine and avoid the formation of much light foam. The optimal fluidity of the foamed slurry in which easily filled the molds was obtained with ratios of (SM/W) (solids materials/water) varies from 1.43 to 2.85. These ratios can be varied according to the tenor of argillaceous matter and of its plastic nature; a high tenor of clay decreased the ratio and gave a large viscosity of the foamed slurry, which prevented the filling of the molds. The predicted densities (dpr) were calculated starting from the total weight of solid materials (100 g) without the loss on the ignition of the kaolin (LOI), and divided by the volume of the foamed slurry (VSG) at a sintered state. Total voluminal withdrawal which the specimen undergoes during drying and firing varying from 10 to 15% of the initial volume according to the nature of argillaceous materials as described in Eq 1.

This method of developing cellular ceramic materials has been applied to the three types of clay previously described.

2.3.   Foam production

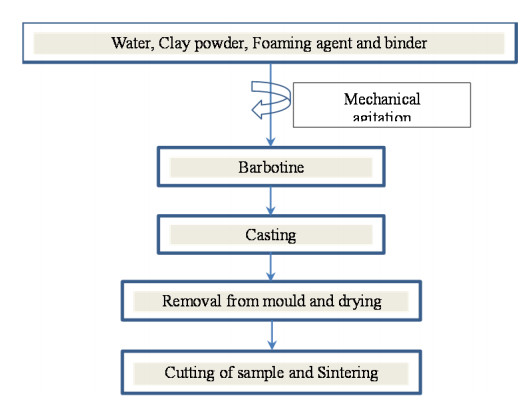
The flowchart in Figure 1 illustrates the process of fabricating the cellular ceramic.

Foam suspensions were prepared separately containing clays DD3, KT and KB. Therefore, three types of cellular ceramics have been made. Each type of clay was thoroughly mixed with water, foaming agent, chamotte and binder, then further homogenized for 15 min with stirring. After casting, all samples were demoulded and left at room temperature. All specimens were initially dried at room temperature for approximately 24 h, then moved in an oven at 80 ℃. The firing process was performed for 2 h at 1100, 1100 and 900 ℃, respectively, using heating rate of 10 ℃·min⁻¹ up to the sintering temperature.

2.4.   Characterization

The experimental bulk density (d_ex) of the cellular refractory specimen was evaluated starting from their weight and their geometrical measurements. The experimental value of the bulk density was compared to the predicted value (d_pr) to evaluate the precision and reproducibility of this method of elaboration of cellular materials. The specific density (d_sp) was determined starting from the weight-volume ratio of water moved of cellular specimen powder (<60 µm), then total porosity (P_t) was estimated starting from the ratio (d_ex/d_sp), according to the following Eq 2.

Thermal analysis is generally used to investigate the thermal behaviours of cellular ceramic specimens, and to determine their thermal linear coefficient of expansion (TEC). Thermogravimetric analysis (TG) and differential thermal analysis (DTA) were performed using the NETZSCH 402 PC dilatometer (URMPE, Boumèrdes) at a heating rate of 5 ℃·min⁻¹ from room temperature to 1400 ℃ under a dry air atmosphere (for all experiments Pt crucibles were used). The appreciation of mechanical resistance at rupture was carried out by testing of flexural strength at three points loading at ambient temperature using a universal testing machine (Zwick/Roell, URMPE, Boumèrdes). Observation of microstructures and cells were conducted using an optical microscope.

3.   Results and discussion

Table 2 displays the variation of the volume of the foamed slurry generated according to the quantity of the added water and the predicted densities, which were calculated by applying Eq 1. The elaboration of cellular materials was carried out within an interval of densities ranging from 1.0 to 0.30 g·cm⁻³. When d < 0.30, the foamed slurry appeared to be lighter and collapsed, however, the cellular has lost its structure when d > 1.0.

3.1.   Density and porosity

Table 3 summarizes the densities, porosities and the precision ratio (d_ex/d_pr) of the cellular specimens elaborated. For all materials, the porosity is varying from 60 to 90% of the total volume. The precision ratio (d_ex/d_pr) demonstrates that the method gives a good prediction of bulk densities of the cellular specimens. However, it can be observed that the experimental densities are lower than the calculated ones, especially, for low densities. This can be explained by the matter of loss that undergoes the samples during machining before the sintering process. Nonetheless, this results show that those values of densities are well comparable, which confirms the possibility of predicting the density of the porous specimen of this method.

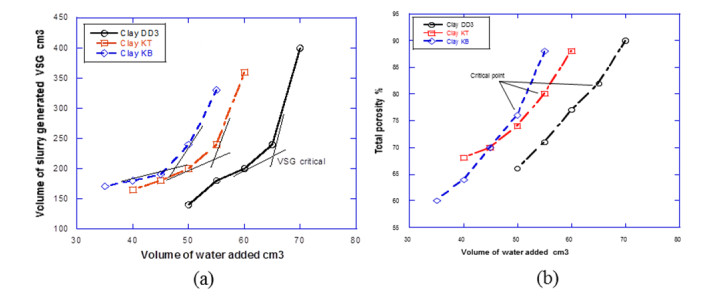
Figure 2 exhibits the evolution of the volume of the foamed slurry and the porosity as a function of the addition of water. However, a critical point clearly appears where these two characteristics change abruptly. The volume of the foamed slurry and porosity increases brutally. The volume of water corresponding to this critical point indicates that this water is completely free of the argillaceous particles, and thus it causes the abrupt increase in the foam formation (Figure 2).

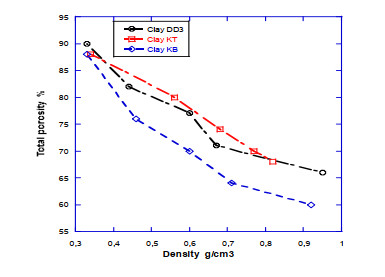
Figure 3 shows the variation of porosity as a function of the density of the produced cellular ceramic. From the same Figure 3, it can be clearly seen that the porosity of the cellular ceramic decreases as the density increased. It has been observed that the quality of the cellular ceramic was strongly influenced by the density of the slurry, as this reflects the degree of porosity. The reduction of the porosity will consequently increase the density of the porous ceramic from 0.3 to 0.9 g·cm⁻³. However, it was found that the density lower than 0.3 g·cm⁻³ is undesirable and making the final product much lighter and collapsed, whereas, for density upper than 1.0 g·cm⁻³ the cellular structure will be affected.

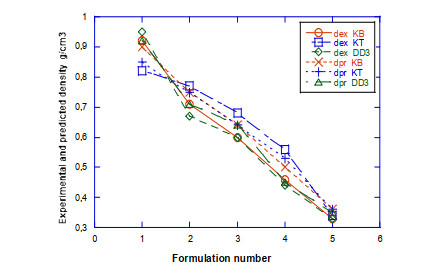
Figure 4 shows the curve of the feasibility of the method used to obtain cellular ceramic. It can be clearly observed that the curves of the predicted density are closer to the experimental ones.

3.2.   Thermo-dilatometer analysis

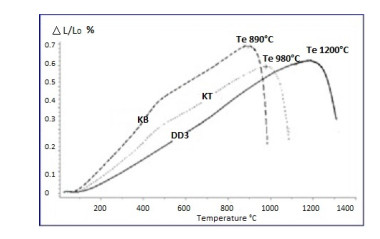
The thermo-dilatometer analysis was conducted on the cellular samples elaborated starting from three clays as shown in Figure 5.

The curve DD3 kaolin shows that this material has fire resistance behaviours, which easily reaches 1000 ℃. At 1100 ℃ a decrease trend can be observed indicating the appearance of a new glassy phase, which limits the use of such cellular material at temperatures beyond 1100 ℃. The average coefficient of expansion for this temperature range is around 6.5 × 10⁻⁶ ℃⁻¹, which is close to that of mullite. So, it can be used in manufacture lightweight refractory bricks,

The curve KT kaolin exhibits a linear expansion up to 980 ℃ and then slightly decreased with the increase of temperature. It is believed that at this temperature a plastic deformation has been occurred on the material. This behaviour can be explained by the appearance of the new glassy phase, which limits the use of this material beyond 980 ℃. Its coefficient of linear expansion is 6.7 × 10⁻⁶ ℃⁻¹, which remains interesting for the needs of thermal shocks.

The curve KB kaolin depicts a bending trend under load from a temperature of 890 ℃, which is believed due to the appearance of the glassy phase. The average coefficient of expansion is 8.5 × 10⁻⁶ ℃⁻¹, which is high when compared with the other coefficients obtained. Besides, this latter has a low resistance to thermal shock, which is believed to be used for low temperature thermal insulation applications.

3.3.   Mechanical properties

3.3.1.   Flexural strength

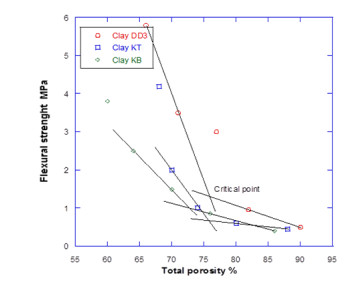
Figure 6 presents the variation of the flexural strength of the cellular ceramic vs the total porosity. It can be seen that the flexural strength decreases from 6.0 to 0.5 MPa with increasing of the total porosity from 60 to 90%. From the same figure, it can be observed that the mechanical resistance sharply decreased when the porosity pointed out at 70 to 80%, which is in agreement with what reported in the literature by Lorna et al. ^[1].

3.4.   Structure of cellular specimens

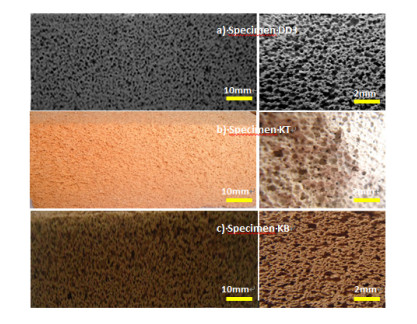
The observation of structure on Figure 7 shows that the cellular specimens have an interconnected cellular structure with a good pore size distribution. The size of the cells ranging from 0.01 to 1 mm. However, Figure 7a represents the cellular materials based on DD3 kaolin, Figure 7b represents the cellular materials based on KT kaolin and Figure 7c represents the materials based on KB kaolin from these photographs, the porosity is very well observed on all the samples. By following the method of elaboration of cellular materials, various porosities can be achieved from 60 to 90% of vacuum at the same ranging cells sizes.

4.   Conclusion

This investigative study shows the performance of using a new method of producing cellular ceramic material based on different kaolin, originally sourced from Algeria. Cellular ceramic materials have been developed with various porosity degrees range from 60 to 90%. The obtained results showed that the size of the cells varies from 0.01 to 1 mm. The dilatometric analysis indicates that a thermal insulation material can be manufactured using the ternary materials used in this study. The final products are believed to be used in different applications including, bricks for construction of ovens walls and other construction where the temperature doesn’t exceed 1100 ℃.

Acknowledgements

The authors are grateful to the faculty of engineer science, university of Boumerdes for financial support that has resulted in this article.

Conflict of interests

The authors have no conflicts of interest to declare.