1.
Introduction
We study the transmission and reflection properties of meta-materials, i.e., of periodic microstructures of a composite material with two components. The interest in meta-materials has immensely grown in the last years as they exhibit astonishing properties such as band gaps or negative refraction; see [23,33,39]. The propagation of electromagnetic waves in such materials is modelled by time-harmonic Maxwell's equations for the electric field E and the magnetic field H:
We use the standard formulation with μ0,ε0>0 the permeability and permittivity of vacuum, μ and ε the corresponding relative parameters, and ω>0 the imposed frequency. While most materials are non-magnetic, i.e., μ=1, the electric permittivity ε covers a wide range. In this paper, we study meta-materials consisting of air (i.e., ε=1) and a (metal) microstructure Ση. The microstructure is assumed to be an η-periodic repetition of scaled copies of some geometry Σ. In the present study, we investigate in detail four different geometries: Σ can be a metal cylinder (in two rotations), a metal plate, or the complement of an air cylinder; see Fig. 2.2 and (2.6)–(2.9) for a detailed definition. For the electric permittivity in the microstructure Ση, we consider two different cases: perfect conductors that are formally obtained by setting ε=∞, and high-contrast materials with ε=ε1η−2, where ε1∈C is some complex number with Im(ε1)>0. In both cases, our study is based on the effective equations for the electric and magnetic field in the limit η→0.
The numerical simulation of electromagnetic wave propagation in such meta-materials is very challenging because of the rapid variations in the electric permittivity. Standard methods require the resolution of the η-scale, which often becomes unfeasible even with today's computational resources. In contrast, we resort to homogenization and multiscale methods to extract macroscopic features and the behaviour of the solution. The effective equations obtained by homogenization can serve as a good motivation and starting point in this process.
Literature. Effective equations for Maxwell's equations in meta-materials are obtained in several different settings. One of the earliest results is contained in [28] where also the case of perfect conductors is treated. In this standard setting (which imposes neither high contrast nor singular geometries), the artificial dielectric coefficients cannot have negative entries. This is different when inclusions with high-contrast media are treated. In [6,7,13], the effect of artificial magnetism (negative eigenvalues of the effective permeability μ) is explained in the framework of Mie-resonance. On the other hand, long wires can lead to unusual effective permittivities [5]. We mention that the wires have to be thin (there volume fraction tends to 0 when the periodicity tends to 0) in order to have nontrivial results.
Artificial magnetism in high-contrast materials appears to be intimately related to band-gap properties for small η: indeed, the key effective magnetism function μeff(ω), independently introduced in [8] appears to be identical to the spectral function β(s) earlier introduced in [44] and then studied further e.g. in [45].
A combination of both structures, bulk inclusions and wires, is used to obtain a negative-index meta-material in [30]. Topological changes in the material in the limit η→0, such as found in split rings, also incite unusual effective behaviour, see [10,31]. Perfect conductors were recently studied as well: split rings in [32] and connected inclusion geometries in [40]. We mention that the geometry in [32] does not include iterated homogenization, a tool that was used in the more theoretical investigation of [35]. Finally, we briefly mention that the Helmholtz equation—as the two-dimensional reduction of Maxwell's equations—is often studied as the first example for unusual effective properties: high-contrast inclusions in [8] or high-contrast layer materials in [11], just to name a few. An overview on this vast topic is provided in [41]. Regarding high contrast problems with nontrivial topologies in the context of elasticity we refer to [29] and [42].
With our results, we identify certain periodic geometries that cannot transmit waves of a certain polarization. Even though this fact can be interpreted in terms of the spectrum of the effective operator, we do not pursue the spectral analysis as, e.g., [14,45].
Concerning the numerical treatment, we focus on the Heterogeneous Multiscale Method (HMM) [21,22]. For the HMM, first analytical results concerning the approximation properties for elliptic problems have been derived in [1,20,37] and then extended to other problems, such as time-harmonic Maxwell's equations [26] and the Helmholtz equation and Maxwell's equations with high-contrast [38,43]. Another related work is the multiscale asymptotic expansion for Maxwell's equations [12]. For further recent contributions to HMM approximations for Maxwell's equations we refer to [17,27]. Sparse tensor product finite elements for multiscale Maxwell-type equations are analyzed in [15] and an adaptive generalized multiscale finite element method is studied in [16].
Main results. We perform an analytical and a numerical study of transmission properties of meta-materials that contain either perfect conductors or high-contrast materials. The main results are the following:
1.) Using the effective equations of [40], we calculate the reflection and transmission coefficients for four microscopic geometries Σ. Few geometrical parameters are sufficient to fully describe the effective coefficients. We show that only certain polarizations can lead to transmission.
2.) For the two geometries that are invariant in the e3-direction, we study the limit behaviour of the electromagnetic fields for high-contrast media. When the electric field is parallel to e3, all fields vanish in the limit. In contrast, when the magnetic field is parallel to e3, transmission cannot be excluded due to resonances.
3.) Extensive numerical experiments for high-contrast media confirm the analytical results. The numerical experiments underline the applicability of the Heterogeneous Multiscale Method to these challenging settings.
Some further remarks on 2.) are in order. The results are related to homogenization results of [7,13,14], but we study more general geometries, since the highly conducting material can be connected. Furthermore, the results are related to [8,11], where connected structures are investigated, but in a two-dimensional formulation. We treat here properties of the three-dimensional solutions. We emphasize that the transmission properties of a high-contrast medium cannot be captured in the framework of perfect conductors, since the latter excludes resonances on the scale of the periodicity (except if three different length-scales are considered as in [32]).
Organization of the paper. The paper is organized as follows: In Section 2, we detail the underlying problem formulations and revisit existing effective equations. In Section 3, we compute the transmission coefficients for perfect conductors and derive effective equations for high-contrast media. In Section 4, we briefly introduce the Heterogeneous Multiscale Method. Finally, in Section 5 we present several numerical experiments concerning the transmission properties of our geometries for high-contrast materials.
2.
Problem formulation and effective equations
This section contains the formulation of the problem, including the description of the four microscopic geometries. We summarize the relevant known homogenization results and apply them to the cases of interest.
2.1. Geometry and material parameters
We study time-harmonic Maxwell's equations with linear material laws. The geometry is periodic with period η>0; solutions depend on this parameter and are therefore indexed with η. On a domain G⊂R3, the problem is to find Eη,Hη:G→C3, such that
subject to appropriate boundary conditions. In the following, we will give details on the geometry G and on the choice of the material parameter εη, the relative permittivity. We are interested in a sequence η=ηi with ηi>0 for every i∈N satisfying ηi→0 as i→∞. We assume that a sequence of solutions (Eη,Hη) is given, in (2.11) below we will additionally assume some boundedness of the sequence. Our interest is to study limits of the sequence.
Note that the system allows to eliminate one unknown. Indeed, if we insert Hη from (2.1a) into (2.1b), we obtain
Alternatively, substituting Eη from (2.1b) into (2.1a), we obtain
Geometry. As sketched in Fig. 2.1, with positive numbers ℓ2,ℓ3>0, the unbounded macroscopic domain is the waveguide domain
With another positive number L>0, the domain is divided into three parts (left, middle, right) as
and
The scatterer Ση is contained in the middle part QM. For the boundary conditions, we consider an incident wave from the right that travels along the x1-axis to the left. We restrict ourselves here to normal incidence. For the analysis, we impose periodic boundary conditions on the lateral boundaries of the domain G. For the numerics, we will modify the boundary conditions slightly: we truncate G in x1-direction to obtain a bounded domain ˜G and consider impedance boundary conditions (with the incident wave as data) on the whole boundary of ˜G. A typical choice is ˜G:=G∩{x1∈(−2L,L)}.
The scatterer Ση is given as an η-periodic structure. We use the periodicity cell Y:=[−12,12]3 and introduce the set Iη of all vectors such that a scaled and shifted copy of Y is contained in QM, Iη:={j∈Z3|η(j+Y)⊂QM}. A set Σ⊂Y specifies the meta-material, which is defined as
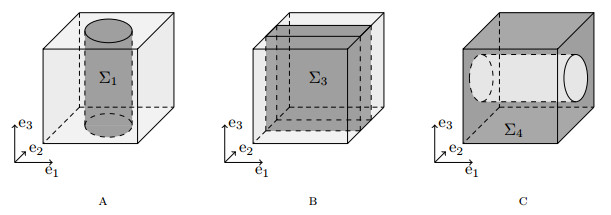
For the microscopic structure Σ we consider the following four examples. The metal cylinder (see Fig. 2.2a) is defined for r∈(0,1/2) as
The set Σ2 is obtained by a rotation which aligns the cylinder with the e1-axis,
To define the metal plate (see Fig. 2.2b), we fix r∈(0,1/2) and set
The fourth geometry is obtained by removing an "air cylinder" from the unit cube (see Fig. 2.2c); for r∈(0,1/2) we set
Material parameters. We recall that all materials are non-magnetic, the relative magnetic permeability is μ≡1. Outside the central region, there is no scatterer; we hence set εη=1 in QL and QR. The middle part QM contains Ση. We set εη=1 in QM∖Ση. It remains to specify the electric permittivity εη in Ση. We consider two different settings.
(PC) In the case of perfect conductors, we set, loosely speaking, εη=+∞ in Ση. More precisely, we require that Eη and Hη satisfy (2.1) in G∖¯Ση and Eη=Hη=0 in Ση. Boundary conditions are induced on ∂Ση: The magnetic field Hη has a vanishing normal component and the electric field Eη has vanishing tangential components on ∂Ση.
(HC) In the case of high-contrast media, we define the permittivity as
where ε1∈C with Re(ε1)>0, Im(ε1)>0. Physically speaking, this means that the scatterer QM consists of periodically disposed metal inclusions Ση embedded in vacuum. The scaling with η2 means that the optical thickness of the inclusions remains constant; see [7].
In both settings and throughout this paper, we consider sequences of solutions (Eη,Hη)η to (2.1). We always assume that, for a bounded subdomain ˜G⊂G, the sequences are bounded in L2(˜G;C3),
Let us remark that the specific geometry of the microstructures Σ1,Σ2, and Σ4 is not important; the cylinders could as well be cuboids.
2.2. Effective equations
Homogenization theory allows to consider the limit η→0. One identifies limiting fields ˆE and ˆH (the latter does not coincide with the weak limit of Hη) and limiting equations for these fields. Using the tool of two-scale convergence, such results have been obtained for perfect conductors as well as for high-contrast materials. We briefly summarize the main findings here; analysis and numerics below are built upon these results.
Perfect conductors (PC). The homogenization analysis for this case has been performed in [40]. Since the parameters of vacuum are used outside the scatterer, the original Maxwell equations describe the limiting fields in QL and QR. In the meta-material QM, however, different equations hold. There holds Eη⇀ˆE and Hη⇀ˆμˆH in L2(G) and the fields ˆE and ˆH solve
The effective coefficients ˆμ and ˆε are determined by cell-problems. For the cell-problems, details on the index sets, and the derivation of system (2.12), we refer to [40]. The index sets NΣ, LΣ, and NY∖¯Σ are subsets of {1,2,3} and can be determined easily from topological properties of Σ. Loosely speaking: An index k is in the set LΣ, if there is a curve (loop) that runs in Σ and connects opposite faces of Y in direction ek. An index k is in NΣ, if there is no loop of that kind. We collect the index sets NΣ, LΣ, and NY∖¯Σ for the geometries Σ1 to Σ4 in Table 2.1.
We will specify equations (2.12c)–(2.12e) for the four chosen geometries in Section 3.1. With the effective equations for the perfect conductors at hand, one can ask for the transmission and reflection coefficients of the meta-material. This is the goal of our analysis in Section 3.1.
High-contrast media (HC). Homogenization results for high-contrast media are essentially restricted to the case of non-connected metal parts, i.e., to geometries that are obtained by Σ which is compactly embedded in Y (it does not touch the boundary of the cube); see, e.g., [6,10,7]. The few exceptions are mentioned below.
For such geometries, the limit equations have again the form of Maxwell's equations,
In QL∪QR, the effective fields coincide with the weak limits of the original fields, and the effective relative coefficients are unit tensors. In the meta-material QM, however, the high-contrast in the definition of the permittivity εη in (2.10) leads to non-trivial limit equations. The effective material parameters ˆε and ˆμ are obtained via cell problems and they can take values that are not to be expected from the choice of the material parameters in the η-problem.
As discussed in Section 2.1, time-harmonic Maxwell's equations can equivalently be written as a single second order PDE for the H-field or the E-field. For the H-field we obtain
Again, the effective material parameters ^ε−1 and ˆμ are defined via solutions of cell problems and we refer to [13,43] for details. We remark that the equivalence of the two formulations (2.13) and (2.14) has been shown in [43]. In particular, the effective permeability ˆμ agrees between both formulations and we have the relation ^ε−1=(ˆε)−1.
The effective equations (2.13) or (2.14) mean that, in the limit η→0, the meta-material QM with high-contrast permittivity εη behaves like a homogeneous material with permittivity ˆε and permeability ˆμ. The occurrence of a permeability ˆμ in the effective equations is striking and this effect is known as artificial magnetism; see [8]. Moreover, ˆμ depends on the frequency ω and it can have a negative real part for certain frequencies. Negative values of the permeability are caused by (Mie) resonances in the inclusions Σ and are studied in detail in [7,43].
As mentioned, a crucial assumption for the homogenization analysis in [7,13] is that Σ is compactly contained in the unit cube. For the four geometries Σ1 to Σ4, this assumption is clearly not met; we therefore ask whether certain components of the effective fields ˆE and ˆH vanish in this case as in the case of perfect conductors. This motivates our analysis in Section 3.2 as well as the numerical experiments in Section 5.
Regarding known results on non-compactly contained inclusions we mention the thin wires in [9] and [30], and the dimensionally reduced analysis of the metal plates Σ3 in [11].
3.
Analysis of the microscopic geometries Σ1 to Σ4
In Section 3.1, we treat the case of perfect conductors and compute the transmission coefficients from the effective equations (2.12). In Section 3.2, we treat the case of high-contrast media and discuss the possibility of nontrivial transmission coefficients.
3.1. Transmission and reflection coefficients for perfect conductors
We compute the transmission and reflection coefficients for four different geometries: metal cylinders, metal plate, and air cylinder. We consider the waveguide G=QL∪ˉQM∪QR of Section 2.1 and impose periodic boundary conditions on the lateral boundary of G. We recall that the four microscopic structures Σ1 to Σ4 are defined in (2.6)–(2.9). Based on the effective equations (2.12) for the perfect conductors, we compute the transmission and reflection coefficients for these geometries.
Results for perfect conductors. Before we discuss the examples in detail, we present an overview of the results. The propagation of the electromagnetic wave in vacuum is described by the time-harmonic Maxwell equations
For the electromagnetic fields, we use the time-convention e−iωt. From (3.1) we deduce that both fields are divergence-free in QL∪QR. We shall assume that the electric field ˆE:G→C3 in QR is the superposition of a normalized incoming wave with normal incidence and a reflected wave:
for x=(x1,x2,x3)∈QR and k∈{2,3}. Here, R∈C is the reflection coefficient and k0=ω√ε0μ0. Note that the electric field ˆE in (3.2) travels along the x1-axis from right to left.
Due to (3.1a), the effective magnetic field ˆH:G→C3 is given by
where l=2 if k=3 and l=3 if k=2 and x∈QR. Equation (3.1b) is satisfied in QR by our choice of k0.
On the other hand, for the transmitted electromagnetic wave in the left domain QL, we make the ansatz
where T∈C is the transmission coefficient. We recall that L>0 is the width of the meta-material QM and {x1=−L} is the interface between left and middle domain. Since the meta-material in QM can lead to reflections, the transmission coefficient T∈C does not necessarily satisfy |T|=1.
Our results are collected in Table 3.1. The table lists transmission coefficients for the four geometries in the case that the incoming magnetic field H is parallel to e3.
In the remainder of this section we compute the transmission coefficient T and the reflection coefficient R for the four microscopic geometries and verify, in particular, the formulas of Table 3.1. Moreover, the effective fields in the meta-material QM are determined.
3.1.1. The metal cylinder Σ1
The metal cylinder Σ1 has a high symmetry, which allows to compute the effective permeability ˆμ. To do so, we define the projection π:Y→R2 onto the first two components, i.e., π(y1,y2,y3):=(y1,y2). Moreover, we set Y2:=π(Y) and Σ21:=π(Σ1).
Choose l∈{1,2} and denote by Hl∈L2(Y;C3) the distributional periodic solution of
with
The normalization of the last equation is defined in [40]; loosely speaking, the left hand side collects values of line integrals of Hl, where the lines are curves in Y∖Σ and connect opposite faces of Y. Problem (3.5) is uniquely solvable by [40,Lemma 3.5]. Given the field Hl=(Hl1,Hl2,Hl3) we define the field hl:Y2→C2 as
Lemma 3.1. Let Hl∈L2(Y;C3) be the solution of (3.5). Then hl∈L2(Y2;C2) of (3.6) is a distributional periodic solution to the two-dimensional problem
Moreover, there exists a potential ψ∈H1♯(Y2;C) such that hl=∇ψ−δ2le1+δ1le2.
Proof. The proof consists of a straightforward calculation.
The decomposition of hl allows to determine the effective permeability ˆμ, which, by [40], is given as
where
Lemma 3.2 (Effective permeability for the metal cylinder). For the microstructure Σ=Σ1 the permeability μeff is given by
Proof. To shorten the notation, we write y′:=(y1,y2)∈Y2. Applying Fubini's theorem and using the decomposition of h1, we find that
where, in the last equality, we exploited that ψ is Y2-periodic and that |Y2|=1. A similar computation shows that (μeff)22=1.
To compute (μeff)12, we note that h11(y′)=∂1ψ(y′). Applying Fubini's theorem, we find
As h22(y′)=∂2ψ(y′), we can proceed as before and find (μeff)21=0.
One readily checks that H3(y):=1Y∖Σ1(y)e3 is the solution of the cell problem (3.5) with ∮H3=e3. The missing entries of the effective permeability matrix μeff can now be computed using the formula for H3 and the definition of μeff in (3.9).
Besides ˆμ, we also need the effective permittivity ˆε. For l∈{1,2,3} we denote by El∈L2(Y;C3) the weak periodic solution to
with
Problem (3.11) is uniquely solvable by [40,Lemma 3.1]. Consequently, the solutions to (3.11) are real vector fields. Indeed, for each index l∈{1,2,3} the vector field Im(El):Y→R3 is a weak solution to (3.11) with ∫YIm(El)=0 and hence Im(El)=0 in Y.
As in [40] we set
where
Lemma 3.3 (Effective permittivity for the metal cylinder). For the microstructure Σ=Σ1, the permittivity εeff is given by
where γ:=(εeff)1,1.
Proof. As shown in Table 2.1, we find that NΣ1={1,2}. From [40,Lemma 3.2] we hence deduce that (εeff)k,3 as well as (εeff)3,k vanish for all k∈{1,2,3}. We claim that the matrix εeff is symmetric. Because of (εeff)k,3=(εeff)3,k=0 we only have to prove that (εeff)1,2=(εeff)2,1. As the solutions E1 and E2 of the cell problem (3.11) are real vector fields, we compute that
To show that (εeff)1,2=(εeff)2,1=0, we consider the map M:Y→Y that is defined by the diagonal matrix diag(−1,1,1). Note that M(Σ1)=Σ1. To shorten the notation, we set E:=E1. Consider the vector field F:Y→R3,
One readily checks that F is a solution to the cell problem (3.11) with ∫YF=−e1. Due to the unique solvability of the cell problem (3.11), we conclude that that F=−E. Similarly, we find that ME2∘M=E2. Thus
Hence (εeff)1,2=(εeff)2,1=0.
We are left to prove (εeff)2,2=(εeff)1,1. To do so, we consider the rotation map R:Y→Y which is defined by the matrix
Then R(Σ1)=Σ1. Moreover, as the cell problem (3.11) is uniquely solvable, we find that RE2∘R=−E1. Thus
This proves the claim.
By Theorem 4.1 of [40], the microstructure Σ1 together with the effective permittivity from (3.14) and permeability from (3.10) implies that the effective equations are
The equations (3.15) do not repeat (2.12a) and (2.12b). Due to (2.12a), the effective electric field is divergence-free. As we assume that ˆE travels along the x1-axis, the first component ˆE1 vanishes. Due to (3.15c) we expect no transmission if the effective electric field is polarized in e3-direction. For nontrivial transmission, we may therefore make the following ansatz for the effective electric field ˆE:G→C3,
Thanks to (2.12b) the magnetic field ˆH is given by
In the meta-material QM we write
and
for x∈QM, where we used equation (2.12a) and (3.10) to determine the magnetic field with α:=|Y∖Σ1|. To compute the value of k0 we use equation (2.12b) and we find that k0=ω√ε0μ0. From (3.15b) we deduce that k1=k0√αγ. In QL we choose (3.4) as the ansatz for ˆE and ˆH, where k=2 and l=3.
Lemma 3.4 (Transmission and reflection coefficients). Given the electric and magnetic fields ˆE and ˆH as described above. Set α:=|Y∖Σ1|, k1=ω√ε0μ0αγ, and p1:=eik1L. The coefficients are then given by
Proof. By (2.12a) the tangential trace of ˆE has no jump across the surfaces {x∈G:x1=0} and {x∈G:x1=−L}. Thus
The effective field ˆH is parallel to e3 and hence, by (3.15b), the third component ˆH3 does not jump across the surfaces {x∈G:x1=0} and {x∈G:x1=−L}. We may therefore conclude that
Here we used that k0=ω√μ0ε0 and k1=k0√αγ. Solving the equations on the left-hand side in (3.16) and (3.17) for R and the other two equations for T, we find that
and
Setting d+:=1+√γ/α and d−:=1−√γ/α, equations (3.18) and (3.19) can be written as
Solving each of the two equations in (3.20) for RM and then equating the two expressions for RM, we obtain
Note that (√α+√γ)2−(√α−√γ)2p21=(α+γ)(1−p21)+2√αγ(1+p21), which yields the formula for TM. From the second equation in (3.20), we deduce that
By (3.18), we have that
To compute the coefficient T we use equation (3.16) and find that
This proves the claim.
3.1.2. The metal cylinder Σ2
Similar to the previous section, we shall determine the transmission and reflection coefficients for a metal cylinder, considering the microstructure Σ2. We define the effective permeability and the effective permittivity ˆμ,ˆε:G→C3 as in (3.8) and (3.12). Following the reasoning of Section 3.1.1, we find that the μeff and εeff are given by
where γ∈C is defined as γ:=∫YE2⋅E2. The effective equations for the microstructure Σ2 are
We may take a similar ansatz for the effective fields as in Section 3.1.1 and obtain the following transmission and reflection coefficients. Note that k1 in Section 3.1.1 has to be replaced by k2:=k0√γ.
Lemma 3.5 (Transmission and reflection coefficients). Within the setting of Section 3.1.1, we set k2=ω√ε0μ0γ and p2:=eik2L. The reflection and transmission coefficients for Σ2 are given by
Note that in the above transmission and reflection coefficients the volume fraction of air α=|Y∖Σ2| does not appear. This is different for the metal cylinder Σ1; see Lemma 3.4.
Proof. Thanks to (2.12a) we know that the tangential components of ˆE do not jump across the surfaces {x∈G:x1=0} and {x∈G:x1=−L}. Hence
The effective field ˆH is parallel to e3 and hence, due to (3.21a), the third component ˆH3 does neither jump across {x∈G:x1=0} nor across {x∈G:x1=−L}. We may therefore conclude that
Here we used that k2=k0√γ=ω√ε0μ0γ.
Solving the equations on the left-hand side in (3.22) and (3.23) for R and the other two for T, we find that
and
Setting c+:=1+√γ and c−:=1−√γ, these two equations can be re-written as
We can solve for TM and obtain
Note that (1+√γ)2−(1−√γ)2p22=(1+γ)(1−p22)+2√γ(1+p22), which proves the formula for TM. By (3.24) we then conclude that
To determine the coefficient R we recall from above that R=TM+RM−1 and hence
As T=p2TM+1/p2RM, we find that
This proves the claim.
We chose the same polarization for the electric and the magnetic field as in Section 3.1.1. By symmetry of the microstructure, we may as well assume that ˆE is parallel to e3 and ˆH is parallel to e2 and obtain the same reflection and transmission coefficients.
3.1.3. The metal plate
We consider the microstructure Σ3; that is, a metal plate which is perpendicular to e2. Following the reasoning in Section 5.2 in [40], we determine the effective equations and obtain:
where α:=|Y∖Σ3|.
The electromagnetic wave is assumed to travel in e1-direction from right to left. Moreover, by (2.12a), the electric field is divergence free. Hence, the first component ˆE1 vanishes. Because of (3.25b) we expect no transmission if the electric field is polarized in e3-direction. We may therefore make the following ansatz for the effective electric field ˆE:G→C3,
Thanks to (2.12b), the magnetic field ˆH is given by
By equation (3.25b), the first and the third component of the effective electric field are trivial; from this and equation (2.12a), we deduce that
and
for x∈QM. The value of k3 can be determined by (3.25a) and we find that k3=k0=ω√ε0μ0. In QL, we choose (3.4) as the ansatz for ˆE and ˆH, where k=2 and l=3.
Lemma 3.6 (Transmission and reflection coefficients). Given the effective fields ˆE and ˆH as described above. Set α:=|Y∖Σ3| and p0:=eiω√ε0μ0L. The reflection and transmission coefficients are given by
Proof. From (2.12a) we deduce that curl ˆE has no singular part and hence the tangential trace of ˆE along the surfaces {x∈G:x1=0} and {x∈G:x1=−L} does not jump. Thus
As ˆH is parallel to e3, we deduce from (3.25a) that ˆH3 does not jump across the surfaces {x∈G:x1=0} and {x∈G:x1=−L}. This implies that
Here we used that k0=k3=ω√ε0μ0. Note that α>0 and hence we find a>0 such that √a=1/α. With this new parameter a, the equations in (3.29) read
Thus the equations in (3.28) and (3.30) have the same structure as the equations in (3.22) and (3.23). We may therefore use the formulas for R,T,RM, and TM derived in Section 3.1.2. Note that
Thus
and
This proves the claim.
3.1.4. The air cylinder
We consider the microstructure Σ4; that is, an air cylinder with symmetry axis parallel to e1 (see Fig. 2.2c). Combining the effective equations (2.12) with the index sets in Table 2.1, we obtain the effective system for this case:
As in the previous sections, we choose the following ansatz for the effective fields ˆE,ˆH:G→C3,
for x∈QR. Equation (2.12b) determines the wave number and we find that k0=ω√ε0μ0. The effective electric field ˆE vanishes in the meta-material QM and hence, by (2.12a) and (3.31b), there is also no effective magnetic field in QM. So ˆE=ˆH=0 in QM. Equation (2.12a) implies that the tangential trace of ˆE does not jump across the surface {x∈G:x1=0}. Thus
As no field is transmitted through the meta-material QM, there is neither an electric nor an magnetic field in QL. In other words, ˆE=ˆH=0 in QL. We have thus shown that
3.2. Vanishing limiting fields in high-contrast media, 2D-analysis
In this section, we perform an analysis of high-contrast media. Of the four geometries Σ1 to Σ4, we study the two e3-invariant geometries: the metal cylinder Σ1 and the metal plate Σ3, compare Fig. 2.2. We analyze the time-harmonic Maxwell equations (2.1) with the high-contrast permittivity εη of (2.10). We recall that the sequence of solutions (Eη,Hη)η is assumed to satisfy the L2(˜G)-bound (2.11). We are interested in the limit behaviour of (Eη,Hη)η as η→0.
When we consider perfect conductors, the effective equations (2.12) imply that some components of Eη or Hη converge weakly to 0 in the meta-material QM. For media with high-contrast, we do not have such a result (we recall that homogenization usually considers compactly contained geometries Σ⊂Y). In this section we ask for Σ1 and Σ3: do the electric fields (Eη)η converge weakly in L2(QM;C3) to 0 as η→0? Is this weak convergence in fact a strong convergence? The same questions are considered for the magnetic fields (Hη)η.
Let us point out that Eη⋅1Ση→0 in L2(QM;C3). Indeed, the L2-estimate (2.11) can be improved to
We mention [10,Section 3.1] as one reference where this observation has also been exploited. The observation is a consequence of an integration by parts formula; it can be applied for the x2-x3-periodic scattering problem in an identical way since integration by parts does not produce boundary terms at periodicity boundaries. Thus
So we have that ‖Eη1Ση‖2L2(˜G)≤η2C which implies that Eη1Ση→0 in L2(QM;C3) as η→0.
We recall that the two geometries of interest are x3-independent. We therefore consider two different cases: In Section 3.2.1, we study electric fields Eη that are parallel to e3. In Section 3.2.2, we study magnetic fields Hη that are parallel to e3. By linearity of the equations, superpositions of these two cases provide the general behaviour of the material.
We will assume that the fields are x3-independent. This is a strong assumption, which can be justified for x3-independent incoming fields with a uniqueness property of solutions. In the rest of this section the fields Eη(x) and Hη(x) depend only on (x1,x2).
Results for high-contrast media. In the E-parallel setting, the electric fields (Eη)η converge strongly to 0 in L2(QM;C3), the magnetic fields converge weakly to 0 in L2(QM;C3). On the other hand, when the magnetic fields Hη are parallel to e3, we can neither expect the electric fields nor the magnetic fields to converge weakly to 0 in L2(QM;C3).
3.2.1. Parallel electric field
We consider here the case of parallel electric fields, i.e., Eη(x):=(0,0,uη(x)) with uη=uη(x1,x2). By abuse of notation, we will consider ˜G also as a domain in R2 and write (x1,x2)∈˜G when (x1,x2,0)∈˜G; similarly for Ση. In this setting, the magnetic field Hη has no third component, Hη(x)=(Hη1(x1,x2),Hη2(x1,x2),0), and Maxwell's equations (2.1) reduce to the two-dimensional system
where we used the two-dimensional orthogonal gradient, ∇⊥u:=(−∂2u,∂1u), as well as the two-dimensional curl, ∇⊥⋅(H1,H2):=−∂2H1+∂1H2. The system (3.34) can equivalently be written as a scalar Helmholtz equation
A solution of this Helmholtz equation provides the fields in the form Eη=(0,0,uη) and Hη=i(ωμ0)−1(∇⊥uη,0).
Lemma 3.7 (Trivial limits for Eη∥e3). For η>0 small, let Ση⊂˜G⊂R2 be a microscopic geometry that is given by Σ1 or Σ3, and let the permittivity εη:˜G→C be defined by (2.10). Let Eη,Hη:˜G→C3 be solutions with Eη(x)=(0,0,uη(x1,x2)) that satisfy the estimate (2.11). Then
Proof. The L2-boundedness of Eη implies the L2-boundedness of uη, and the L2-boundedness of Hη implies the L2-boundedness of ∇uη. Therefore, the sequence (uη)η is bounded in H1(˜G), and we find a subsequence η→0 (not relabeled) and a limit function u∈H1(˜G) such that uη⇀u in H1(˜G) and uη→u in L2(˜G) as η→0.
We write
The left hand side converges strongly to u1QM. The first term on the right hand side of (3.36) is the product of a strongly L2(QM)-convergent sequence and a weakly L2(QM)-convergent sequence: 1QM∖Ση⇀α in L2(QM), where α∈(0,1) is the volume fraction of Y∖Σ. We find that the first term on the right hand side converges in the sense of distributions to αu. The estimate (3.33) provides the strong convergence of the second term on the right hand side of (3.36) to zero. The distributional limit of (3.36) provides
and hence u=0, since α≠1. We have therefore found
which was the claim.
3.2.2. Parallel magnetic field
We now consider a magnetic field that is parallel to e3, Hη(x)=(0,0,uη(x1,x2)), with all quantities being x3-independent. This H-parallel case is the interesting case for homogenization and it has the potential to generate magnetically active materials. It was analyzed e.g. in [6,7,11,24]. In this setting, Maxwell's equations (2.1) reduce to
System (3.38) can equivalently be written as a scalar Helmholtz equation:
In (3.35), the high-contrast coefficient is outside the differential operator, which induces a trivial limit behaviour of solutions. In contrast, (3.39) has the high-contrast coefficient inside the differential operator, which leads to a much richer behaviour of solutions.
The case Σ=Σ3 is the metal plate (see Fig. 2.2b) that was studied in [11]. The result of [11] is the derivation of a limit system with nontrivial solutions. In particular, the weak limit of (uη)η can be non-trivial. Similar results are available for metallic wires Σ=Σ1 (see Fig. 2.2a); the results of [7] imply that also in this case the weak limit of (uη)η can be non-trivial.
We therefore observe that the H-parallel case does not allow to conclude Hη⇀0 in L2(QM;C3). We note that Hη=(0,0,uη)⇀̸0 implies, by boundedness of the magnetic field and equation (3.38a), also Eη⇀̸0.
4.
Finite element based multiscale approximation
In this section, we present numerical multiscale methods that are used to study Maxwell's equations in high-contrast media from a numerical point of view. We introduce the necessary notation for finite element discretisations and briefly discuss the utilized approaches. Based on these methods, numerical experiments illustrating the transmission properties of the microstructures are presented in Section 5.
4.1. Variational problem for the second order formulation
We study time-harmonic Maxwell's equations in their second-order formulation for the magnetic field H (2.3). The macroscopic domain ˜G is assumed to be bounded and we impose impedance boundary conditions on the Lipschitz boundary ∂˜G with the outer unit normal n:
where g∈L2(∂˜G) with g⋅n=0 is given and k0=ω√ε0μ0 is the wavenumber. These boundary conditions can be interpreted as first-order approximation to the Silver-Müller radiation conditions (used for the full space R3); the data g are usually computed from an incident wave. For the material parameters, we choose μ=1 and εη as specified in (2.10). Multiplying with a test function and integrating by parts results in the following variational formulation: Find Hη∈Himp(˜G) such that
for all ψ∈Himp(˜G), where Himp(˜G):={v∈L2(˜G;C3)|curl v∈L2(˜G;C3),vT∈L2(∂˜G)} and vT:=v−(v⋅n)n denotes the tangential component. Existence and uniqueness of the solution to this problem for fixed η is shown, for instance, in [36].
4.2. Traditional finite element discretisation
The standard finite element discretisation of (4.1) is a Galerkin procedure with a finite-dimensional approximation space Vh⊂Himp(˜G) which consists of piecewise polynomial functions on a (tetrahedral) mesh of ˜G. In detail, we denote by Th={Tj|j∈J} a partition of ˜G into tetrahedra. We assume that TH is regular (i.e., no hanging nodes or edges occur), shape regular (i.e., the minimal angle stays bounded under mesh refinement), and that it resolves the partition into the meta-material QM and air ˜G∖¯QM. To allow for such a partition, we implicitly assume ˜G and QM to be Lipschitz polyhedra. Otherwise, boundary approximations have to be used which only makes the following description more technical. We define the local mesh size hj:=diam(Tj) and the global mesh size h:=maxj∈Jhj. As conforming finite element space for Himp(G), we use the lowest order edge elements introduced by Nédélec, i.e.,
It is well known (see [36], for instance), that the finite element method with this test and trial space in (4.1) yields a well-posed discrete solution Hh. Furthermore, the following a priori error estimate holds
For the setting of (4.1) as discussed in this paper, however, two major problems arise. First, due to the discontinuities of the electric permittivity ε−1η the necessary regularity of Hη is not available, see [4,19,18]. Second, even in the case of sufficient regularity the right-hand side of the error estimate experiences a blow-up with ‖Hη‖H1(˜G)+‖curl Hη‖H1(˜G)→∞ for η→0. In other words, a typical solution of (4.1) is subject to (strong) oscillations in η such that its derivative does not remain bounded for the periodicity length tending to zero. As a consequence, the error estimate has a η-dependent right-hand side of the type hη−1. Therefore, convergence of standard finite element discretisations is only to be expected in the asymptotic regime when h≪η, i.e., the mesh has to resolve the oscillations in the PDE coefficients. As discussed in the introduction, this can become prohibitively expensive.
4.3. Heterogeneous multiscale method
As a remedy to these limitations of the standard finite element method, we consider a specific multiscale method. The idea is to extract macroscopic properties of the solution with η-independent complexity or computational effort, respectively. The basic idea directly comes up from the effective equation (2.14): Since this effective equation is independent of η, it can be discretised on a rather coarse mesh Th without the need to resolve the η-scale, i.e., we can have h>η. This results in an approximation of the homogenized solution ˆH, which contains important macroscopic information of Hη. However, for the discretisation of the homogenized equation, the effective material parameters ˆε and ˆμ need to be known, at least at the (macroscopic) quadrature points. This can be achieved by introducing another, again η-independent, mesh ThY={Sl|l∈I} of the unit cube Y with maximal mesh size hY=maxl∈Idiam(Sl). We assume that ThY is regular and shape regular and resolves the partition of Y into Σ and Y∖¯Σ. Furthermore, ThY has to be periodic in the sense that it can be wrapped to a regular triangulation of the torus, i.e., no hanging nodes or edges over the periodic boundary. Note that hY denotes the mesh size of the triangulation of the unit cube. Thus, it is in no way related to η and can be of the same order as h. Based on this mesh, the cell problems occurring in the definition of ˆε and ˆμ can be discretised with standard (Lagrange and Nédélec) finite element spaces. For details we refer to [43]. All in all, we can now compute the homogenized solution ˆH of (2.14) as follows: 1. Compute discrete solutions of the cell problems (see [7] or[43]) using the mesh ThY and the associated standard finite element spaces. 2. Compute the effective parameters ˆε (or ^ε−1) and ˆμ approximatively with the discrete cell problems solutions. 3. Compute the discrete homogenized solution of (2.14) with the approximated effective coefficients and using the mesh Th with the associated finite element space Vh as introduced above.
This (naive) discretisation scheme for the effective equation (2.14) in fact can be interpreted as a specification of the Heterogeneous Mutliscale Method (HMM) in the perfectly periodic case. The Finite Element Heterogeneous Multiscale Method, introduced by E and Enguist [21,22], sets up a macroscopic sesquilinear form to compute the HMM solution Hh, which is an approximation of the homogenized solution ˆH. The macroscopic sesquilinear form is very similar to the effective sesquilinear form associated with the left-hand side of (2.14), but the effective material parameters are not computed a priori. In contrast, local variants of the cell problems are set up on η-scaled cubes Yηj=ηY+xj around macroscopic quadrature points xj. We can still use the mesh ThY of the unit cube Y and transform it to a partition ThY(Yηj) of the scaled unit cell Yηj. Similarly, also the finite element spaces associated with ThY can be transferred to spaces on ThY(Yηj) using a suitable affine mapping. The finescale computations result in so called local reconstructions, which consist of the macroscopic basis functions and the corresponding (discrete) cell problem solutions. Averages (over Yηj) of these local reconstructions then enter the macroscopic sesquilinear form. A detailed definition of the HMM for Maxwell's equations in high-contrast media is presented in [43], where also the connection to analytical homogenization as well as the possibility to treat more general than purely periodic problems are discussed. We only want to emphasize one important feature of the HMM in [43]: Apart from the (macroscopic) approximation Hh, discrete correctors HhY,1, HhY,2, and HhY,3 can be determined from the discrete cell problems (in a second post-processing step). Via these correctors, we can define the zeroth order L2-approximation H0HMM:=Hh+∇yHhY,2(⋅,⋅η)+HhY,3(⋅,⋅η), which corresponds to the first term of an asymptotic expansion and is used to approximate the true solution Hη. We again refer to [43] for details and note that it has been observed in several numerical examples that these correctors are a vital part of the HMM-approximation, see [26,25,38,43]. We close by remarking that in Section 5 below, we extend the described HMM of [43] to general microstructures although the validity of the homogenized models in these cases is not shown so far, see the discussion in Sections 2.2 and 3.2.
5.
Numerical study of transmission properties for high-contrast inclusions
In this section, we numerically study the transmission properties in the case of high-contrast for the three micro-geometries: the metal cylinder, the metal plate, and the air cylinder. Since the aim of this paper is a better understanding of the different microstructures and their effect, we focus on the qualitative behaviour rather than explicit convergence rates. The implementation was done with the module dune-gdt [34] of the DUNE software framework [3,2].
Setting. We consider Maxwell's equations in the second-order formulation for the H-field (4.1) with a high-contrast medium as defined in (2.10). It remains to specify the macroscopic geometry, the boundary data g, the material parameter ε1, and the frequency. We use a slab-like macroscopic geometry similar to Section 2.1, but we truncate G also in the x1-direction to have a finite computational domain ˜G, as described in the previous section. We choose ˜G=(0,1)3 with the meta-material located in QM={x∈G|0.25≤x1≤0.75}. Note that QM is translated in x1-direction compared to Section 2.1, but this does not influence the qualitative results of the analysis. As in Section 2.1, we assume that an incident wave Hinc from the right travels along the x1-axis to the left, i.e., Hinc=exp(−ikx1)p with a normalized polarization vector p⊥e1. This incident wave is used to compute the boundary data g as g=curl Hinc×n−ik0n×(Hinc×n). We choose the inverse permittivity as ε−11=1.0−0.01i and note that ε1 is only slightly dissipative. In all experiments, we choose the same wavenumber k0=12 and the periodicity parameter η=1/8.
As explained in the previous section, we want to use the Heterogeneous Multiscale Method to obtain good approximations with reasonable computational effort. We use the mesh sizes hy=h=√3⋅1/16 and compute the macroscopic HMM-approximation Hh as well as the zeroth order approximation H0HMM, which also utilizes information of the discrete correctors. To demonstrate the validity of the HMM, we use two different reference solutions. First, the homogenized reference solution ˆH is computed as solution to (2.14) on a mesh with size h=√3⋅1/48, where the effective material parameters are calculated approximatively using a discretisation of the unit cube with mesh size hY=√3⋅1/24. Second, the (true) reference solution Hη is computed as direct finite element discretisation of (4.1) on a fine grid with href=√3⋅1/64.
Main results. Before we discuss the examples in detail, we present an overview of the results. The qualitative transmission properties of the meta-material are in good agreement with the theory of Section 3.1, although the numerical examples consider high-contrast media instead of perfect conductors. The predictions and the corresponding numerical examples are summarized in Table 5.1. In contrast to perfect conductors, the high-contrast medium leads to rather high intensities and amplitudes of the H-field inside the inclusions Ση. Depending on the chosen wavenumber, Mie-resonances inside the inclusions can occur for high-contrast media; see Section 3.2 and [7,43]. Our numerical experiments also show that the HMM yields (qualitatively) good approximations, although the validity of the underlying effective models is not proved for the studied geometries.
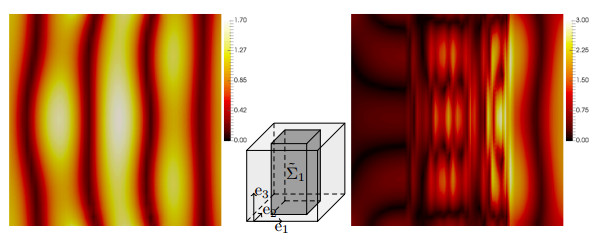
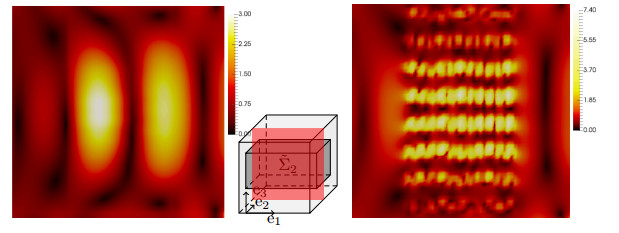
5.1. Metal cuboids ˜Σ1 and ˜Σ2
Instead of metal cylinders with circular base we study metal cuboids with square base, so that we do not have to deal with boundary approximations in our numerical method. We choose ˜Σ1=(0.25,0.75)2×(0,1) and ˜Σ2=(0,1)×(0.25,0.75)2. Note that this choice influences the value of γ, but not the other results of Sections 3.1.1 and 3.1.2. Due to the symmetry of the microstructure, the effective material parameters are diagonal matrices with a11=a22, but with a different value a33; see the analytical computations in Section 3.1.1. Up to numerical errors, we obtain the same structure for the computed approximative effective parameters.
Comparing the homogenized reference solution ˆH for e2- and e3-polarized incoming waves for ˜Σ1 in Fig. 5.1, we observe that the e3-polarized wave is transmitted almost undisturbed through the meta-material. For the e2-polarization, however, the field intensity in QL:={x∈G:x≤0.25} is very low, corresponding to small transmission factors. This matches the analytical predictions of Section 3.1.1, which yields transmission only for e3-parallel H-fields. The same effect is predicted for high-contrast media by the analysis of Section 3.2.1.
The HMM can reproduce the behaviour of the homogenized and of the heterogeneous solution. For the comparison, we only consider the e3-polarized incoming wave in Fig. 5.2 and compare the zeroth order approximation H0HMM (right) to the (true) reference solution Hη (left). Errors are still visible, but the qualitative agreement is good, even for the coarse mesh size of h=hY=√3⋅1/16 chosen for the HMM. In particular, the rather cheaply computable zeroth order approximation H0HMM can capture most of the important features of the true solution, even for inclusions of high-contrast. This clearly underlines the potential of the HMM. Moreover, Fig. 5.2 underlines the specific behaviour of Hη in the inclusions ˜Ση for high-contrast media. As analyzed in Section 3.2.2, Hη cannot be expected to vanish in the inclusions in the limit η→0 due to possible resonances; see [6]. We observe rather high field intensities in the inclusions; see also [43] for a slightly different inclusion geometry.
We also study the rotated metal cuboid ˜Σ2. In correspondence to the analysis of Section 3.1.2, we observe transmission for an e3-polarized incident wave; see Fig. 5.3. Note that the homogenized reference solution looks different to ˜Σ1 because of the rotation of the geometry, which is also reflected in the different structure and values of the reflection and transmission coefficients. The reference solution in Fig. 5.3 (right) shows the high field intensities in the metal cuboids induced by the high-contrast permittivity.
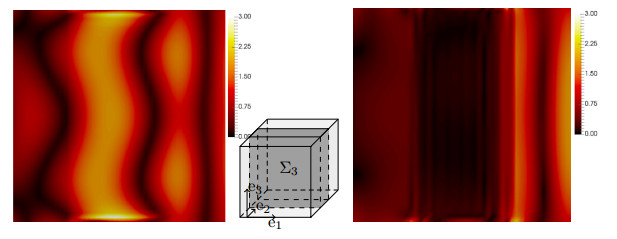
5.2. Metal plate Σ3
As in Section 3.1.3, we choose a metal plate perpendicular to e2 of width 0.5, i.e. Σ3=(0,1)×(0.25,0.75)×(0,1). Discretising the cell problems with mesh size hY=√3⋅1/24, we obtain—up to numerical errors—the effective material parameters as diagonal matrices with
Although we consider high-contrast media, this corresponds astonishingly well to the analytical results for perfect conductors of Section 3.1.3: The structure of the matrices agrees and the non-zero value of ^ε−1=|Y∖¯Σ| is as expected from the theory of perfect conductors. Due to the contributions of the inclusions, the values of μhom are different from the case of perfect conductors.
Section 3.1.3 shows that, for perfect conductors, only an H-field polarized in e3-direction can be transmitted through the meta-material. Our numerical experiments allow a similar observation for high-contrast media in Fig. 5.4: The homogenized reference solution only shows a non-negligible intensity in the domain QL={x∈G|x3≤0.25} left of the scatterer if the incident wave is polarized in e3 direction. Note that we have some reflections from the boundary in Fig. 5.4 since we do not use perfectly matched layers as boundary conditions. The observed transmission properties for high-contrast media are in accordance with the theory in Section 3.2: For an e3-polarized H-field as in the left figure, we cannot expect a (weak) convergence to zero. This corresponds to the observed non-trivial transmission. By contrast, in the right figure, the H-field is e2-polarized and no transmission can be observed. This corresponds to the analysis of Section 3.2.1, which shows that Hη converges to zero, weakly in L2(QM).
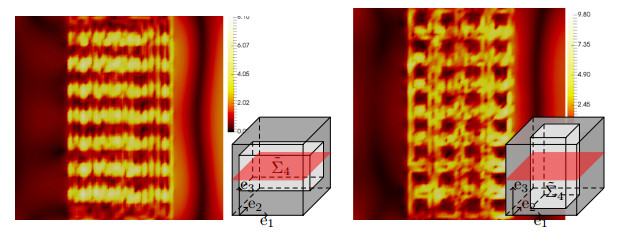
5.3. Air cuboid ˜Σ4
As with the metal cylinder, we equip the air cylinder of Section 3.1.4 with a square base in order to have a geometry-fitting mesh. To be precise, we define the microstructure ˜Σ4=(0,1)3∖((0,1)×(0.25,0.75)2). The effective permittivity ^ε−1 vanishes almost identically for this setting; numerically we obtain only entries of order 10−5 for a discretisation of the corresponding cell problem with mesh size hY=√3⋅1/24. As discussed in Section 3.1.4, no transmission through this meta-material is expected for the high conductors. We observe the same for high-contrast media in Fig. 5.5: The (true) reference solution (almost) vanishes in the left part QL in all situations. Here, we only depict e3-polarized incident waves, once for ˜Σ4 as described and once for the rotated air cuboid with main axis in e3-direction (this is the setting of Section 3.1.1 with interchanged roles of metal and air). Note that inside the microstructure, high intensities and amplitudes of the Hη-field occur due to resonances in the high-contrast medium.
Conclusion
We analyzed the transmission properties of meta-materials consisting of perfect conductors or high-contrast materials. Depending on the geometry of the microstructure, certain entries in the effective material parameters vanish, which induces that also certain components of the solution vanish. This influences the transmission properties of the material. Transmission is possible only for certain polarizations of the incoming wave. For perfect conductors, we derived closed formulas for the reflection and transmission coefficients. Using the Heterogeneous Multiscale Method, the homogenized solution as well as some features of the exact solution can be approximated on rather coarse meshes and, in particular, with a cost that is independent of the periodicity length. Our numerical experiments of three representative geometries with high-contrast materials confirm the theoretical predictions of their transmission properties.









 DownLoad:
DownLoad: