Mixed Erdélyi-Kober and Caputo fractional differential equations with nonlocal non-separated boundary conditions

1.   Introduction

Fractional calculus is an important part of mathematics that considers the operators of arbitrary orders. It also consists of a wide of applications of fractional derivative operators in various fields of sciences; see the monographs by Samko et al. ^[1], Gorenflo and F. Mainardi ^[2], Podlubny ^[3], Hilfer ^[4], and Kilbas et al. ^[5]. Fractional integral operators are the main tools to present some fractional derivative operators, which are used to gather beneficial information about the hereditary and materials involved in the phenomena. In the past years, various fractional derivative operators such as Riemann-Liouville (R-L), Caputo, Erdélyi-Kober, Hadamard, etc. have been considered. The Erdélyi-Kober (E-K) fractional integral operator is a generalization of the R-L fractional integral operator to solve some types of integral equations consist of spatial functions in their kernels. Erdélyi-Kober operators have been used by many authors, in particular, to obtain solutions of the single, dual, and triple integral equations possessing special functions of mathematical physics as their kernels. Anomalous diffusion process derived by the Erdélyi-Kober fractional integral operator has also been studied. For applications of the E-K fractional integral operator in mathematical physics see ^[6], for the transmutation method in ^[7] and in ^[8] for diffusion-wave equation. On the other hand, boundary value problems (BVPs) with different types of boundary conditions have been studied by many authors, for example, with Steiljes-type fractional integral boundary conditions in ^[9], with coupled integral fractional boundary conditions in ^[10], and with fractional integral type boundary conditions in ^[11]. Recently, BVPs of Hilfer fractional differential equations with a variety of boundary conditions have been considered by many authors, for example with nonlocal integro-multi-point boundary conditions in ^[12], for a coupled system with coupled integral fractional boundary conditions in ^[13], and for proportional nonlocal fractional integro-multi-point boundary conditions in ^[14]. In these works, there is a zero initial condition in order to obtain a well-defined solution. In ^[15], by combining the Hilfer and Caputo fractional derivatives, a BVP with non-separated boundary conditions of the form

have been investigated, where ${}^{H}\mathbb{D}^{c, d, \phi}$ and ${}^{C}\mathbb{D}^{\zeta, \phi},$ $0 < c, d, \zeta < 1,$ $\eta = c + d-cd,$ $c+\zeta > 1$ are the $\phi$-Hilfer fractional derivative and $\phi$-Caputo fractional derivative, respectively. Moreover, $\kappa_{1}, \kappa_{2}\in \mathbb{R}$, $\mathbb{I}^{r, \phi}$ is the Riemann-Liouville fractional integral of order $r > 0$, with respect to a function $\phi,$ $f : [0, L] \times \mathbb{R}\times \mathbb{R}\times \mathbb{R} \longrightarrow \mathbb{R}$ is a nonlinear continuous function; $\int_0^L p(s)dw(s)$ is the Riemann-Stieltjes integral and $w:[0, L]\to \mathbb{R}$ is a function of bounded variation.

In the present work, we combine the Caputo and Erdélyi-Kober fractional derivatives to investigate a BVP with non-separated boundary conditions of the form

in which $0 < \alpha, \delta < 1$, $\beta > 0$, $\gamma > -1$ with $\alpha > \beta(1+\gamma)$, $g\in C([0, T], \mathbb{R})$, $a_{i}, b_{i}\in \mathbb{R}, \; i = 1, 2$ and $f_{i}:C([0, T], \mathbb{R})\longrightarrow \mathbb{R}, \; i = 1, 2.$

The tools of fixed point-theory will be applied to obtain the main results. Indeed, Banach's fixed point theory is applied to obtain the uniqueness result, while the Leray-Schauder nonlinear alternative and Krasnosel'skiĭ's fixed-point theorems are applied to obtain the existence results. Moreover, numerical examples are presented to support the theoretical analysis. The method we used to establish our results is standard, but its configuration in the fractional Caputo and Erdélyi-Kober sequential boundary value problem (1.2) is new.

The novelty of the present research lies in the fact that we consider a sequential fractional boundary value problem contains a combination of Caputo and Erdélyi-Kober fractional derivative operators supplemented with non-separated boundary conditions. To the best of our knowledge, this combination of Caputo and Erdélyi-Kober fractional derivatives appears in the literature for the first time.

The next sections of this article have been prepared as follows: Section $2$ begins with some basic definitions and lemmas which will be needed to prove the main results. Besides, a practical lemma is presented to convert the problem (1.2) into a fixed-point problem. Section $3$ presents some existence and uniqueness results of the problem (1.2) via the tools of fixed-point theory. The last section gives some numerical examples to illustrate the obtained results.

2.   Preliminaries

In this section, some definitions and lemmas are presented that are basic to obtain the main results.

Definition 2.1. ^[1,5] The Riemann-Liouville fractional integral operator of order $\zeta > 0$ staring at a point $"a"$, is defined by

In view of the above definition, the Riemann-Liouville and Caputo fractional derivative operators of order $\zeta > 0$ are defined by^[1,5]:

where $\mathbb{D}^{n} = d^n/dt^n$ and $n-1 < \zeta < n$. In case, $a = 0$, we usually use the notations ${^R}\mathbb{I}^{\zeta}(\cdot)$, ${}^{R}\mathbb{D}^{\zeta}(\cdot),$ and ${}^{C}\mathbb{D}^{\zeta}(\cdot)$. In all definitions, we assume the right sides of them are well-defined. The following lemmas state some basic properties of the above definitions.

Lemma 2.2. ^[1,5] Let $n-1\leq \zeta \leq n$, $\varsigma > 0$ be constants, and $t > a$. Then the following properties are satisfied:

$(i)$ $^{R}\mathbb{I}_{a^{+}}^{\zeta}\left(^{R}\mathbb{I}_{a^{+}}^{\varsigma}f\right)(t) = \mathbb{I}_{a^{+}}^{\zeta+ \varsigma}f(t)$;

$(ii)$ $^{R}\mathbb{I}_{a^{+}}^{\zeta} \left(^{C}\mathbb{D}_{a^{+}}^{\zeta}f\right)(t) = f(t)-\sum_{i = 0}^{n-1}\frac{f^{(i)}(a^{+})}{i!}(t-a)^{i}$;

$(iii)$ $^{R}\mathbb{I}_{a^{+}}^{\zeta}(t-a)^{\varsigma-1} = \frac{\Gamma(\varsigma)}{\Gamma(\varsigma+ \zeta)}(t-a)^{\varsigma+ \zeta-1}$;

$(iv)$ If $\varsigma > \zeta+1$ then $^{C}\mathbb{D}_{a^{+}}^{\zeta}(t-a)^{\varsigma-1} = \frac{\Gamma(\varsigma)}{\Gamma(\varsigma-\zeta)} (t-a)^{\varsigma-\zeta-1};$

$(v)$ If $\zeta < \varsigma$ then $^{C}\mathbb{D}_{a^{+}}^{\zeta}\left(^{R}\mathbb{I}_{a^{+}}^{\varsigma}f\right)(t) = ^{R}\mathbb{I}_{a^{+}}^{\varsigma-\zeta}f(t).$

Definition 2.3. ^[1,5] The fractional integral of Erdélyi-Kober type of order $\zeta > 0$ with parameters $\varsigma > 0$ and $\mu\in \mathbb{R}$ of a continuous function $f:(0, \infty)\longrightarrow \mathbb{R}$ is defined by

Note that if $\mu = 0$ and $\varsigma = 1$, then we have ${^E}\mathbb{I}_{1}^{0, \zeta}f(t) = t^{-\zeta}\left({^R}\mathbb{I}^{\zeta}f(t)\right)$.

Based on the above definition, the Erdélyi-Kober fractional derivative ${}^{E}\mathbb{D}_{\varsigma}^{\mu, \zeta}$ of order $\zeta$, with $n-1 < \zeta\leq n$, $\varsigma > 0$, $\mu\in \mathbb{R}$, is defined by ^[1,5] as

Using the relation $\prod_{j = 1}^{n}\left[\mu+j+\frac{1}{\varsigma} t \frac{d}{dt}\right](\cdot) = \left[t^{-\varsigma\mu}\left(\frac{1}{\varsigma t^{\varsigma-1}}\frac{d}{d t}\right)^nt^{\varsigma(n+\mu)}\right](\cdot)$, another form of the Erdélyi-Kober fractional derivative can be presented as

see ^[16,17].

Lemma 2.4. ^[16] Let $n-1 < \zeta < n$, $n\in N$, $\alpha > -\varsigma(\mu+1)$ and $v\in C^{n}_{\alpha}[a, b]$. Then we get

where,

Lemma 2.5. ^[18] Let $\zeta, \varsigma, q > 0$ and $\mu > -(q+\varsigma)/\varsigma$. Then we have

Before we investigate the boundary value problem of sequential differential operators Erdélyi-Kober and Caputo, we would like to understand the behavior of these sequential operators acting on a given function $y(t) = t^m$.

Example 2.6. Let $y(t) = t^m$, $t\geq 0$. Then we have by using the Lemmas 2.2 and 2.5 that

and

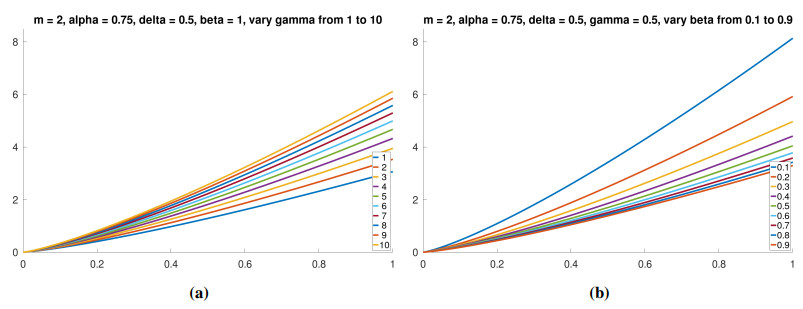
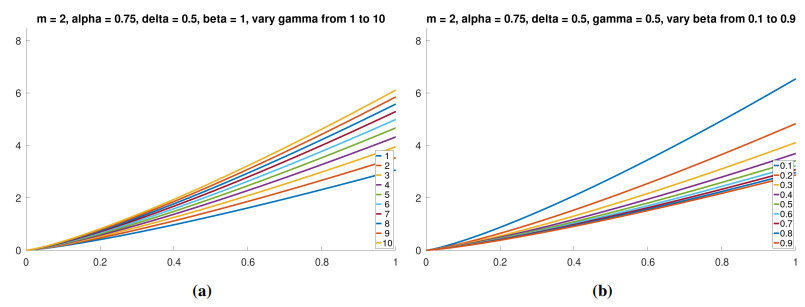
We visualize the solution of example to better understand the behavior of the sequential differential operators Erdélyi-Kober and Caputo. By setting the parameters $m = 2$, $\alpha = 3/4$, $\delta = 1/2$, and the considered domain $[0, 1]$, Figures 1 and 2 depict the graphs of the solutions to Eqs 2.3 and 2.4, respectively. In Figures 1a and 2a, the parameter $\beta$ is fixed at 1, while the parameter $\gamma$ varies from 1 to 10. Conversely, in Figures 1b and 2b, the parameter $\gamma$ is set to 0.5, and the parameter $\beta$ ranges from 0.1 to 0.9. The graphs reveal that the function values slightly change when the sequential order of the operators is altered, but their overall trends remain consistent.

The following lemma is the basic tool to transform the problem (1.1) into an integral equation.

Lemma 2.7. Let $0 < \alpha, \delta < 1$ be the orders, $\beta > 0$, $\gamma > -1$ with $\alpha > \beta(1+\gamma),$ be the Erdély-Kober parameters, $g_1\in C([0, T], \mathbb{R}),$ $a_{i}, b_{i} \in \mathbb{R}$ and two functionals $f_{i} :C([0, T], \mathbb{R})\longrightarrow \mathbb{R}, \; i = 1, 2$. Then the Erdélyi-Kober and Caputo fractional linear boundary value problem

is equivalent to the integral equation

where it is assumed that $a_1+a_2\neq0$ and $b_1+b_2\neq0$.

Proof. Taking the Erdélyi-Kober fractional integral ${}^{E}\mathbb{I}_{\beta}^{\gamma, \delta}$ on both sides of the equation in (2.5) and using Lemma 2.4 we obtain

Now, by taking the fractional integral ${}^{R}\mathbb{I}^{\alpha}$ on both sides of (2.7) and using Lemma 2.2, we have

To obtain fractional derivative in boundary condition, due to Lemma 2.2 and the Caputo derivative of constant is zero, we get

By combining the boundary conditions $a_{1}v(0)+ a_2v(T) = f_{1}(v)$ and $b_{1} {}^{C}\mathbb{D}^{\alpha-\beta(1+ \gamma)}v(0) + b_2 {}^{C}\mathbb{D}^{\alpha-\beta(1+ \gamma)}v(T) = f_2(v)$ with $(2.8)$ and $(2.9)$, we obtain a system of two unknown constants $c_0$ and $c_1$ as

By solving the above system for finding $c_0$ and $c_1$, we obtain

Replacing the values of constants $c_0$ and $c_{1}$ in (2.8), we obtain the desired unique solution $(2.6)$ of the linear boundary value problem (2.5).

The conversion of the proof can be achieved by applying the Caputo and Erdélyi-Kober fractional derivatives to Eq (2.6), respectively, and also demonstrated that (2.6) satisfies the boundary conditions of problem (2.5). □

3.   Existence and uniqueness results

Let $\mathcal{X} = C([0, T], \mathbb{R})$ be the Banach space of all continuous functions from $[0, T]$ into $\mathbb{R}$ endowed with the norm $\| x \| = \sup \{| x(t)|: t\in [0, T]\}$.

In view of Lemma 2.7, we define an operator $\mathcal{F}:\mathcal{X}\longrightarrow \mathcal{X}$ by

For convenience, we set some positive constants:

In the following theorem, Banach's fixed-point theorem ^[19] is applied to obtain a unique solution of the problem (1.1).

Theorem 3.1. Let $g:[0, T]\times \mathbb{R}\longrightarrow \mathbb{R}$ be a continuous function such that:

$(D_{1})$ There exists a constant $\mathbb{L} > 0$ such that for all $t\in [0, T]$ and $v_{1}, v_{2}\in \mathbb{R}$, we have

$(D_{2})$ There exist two constants $\ell_1, \ell_2 > 0$ such that for all $v_{1}, v_{2}\in \mathbb{R}$, we have

Then, the problem (1.1) has a unique solution on $[0, T],$ provided that

where $Q_i, i = 1, 2, 3$ are defined by (3.2).

Proof. Let $g_0 = \sup\{| g(t, 0)|: t\in [0, T]\},$ $m_1 = |f_1(0)|,$ $m_2 = |f_2(0)|$ be constants obtained from the given function and functionals from (1.1) and $B_{y^{*}} = \{ v\in \mathcal{X}: \| v \|\leq y^{*}\}$ be a ball with $y^{*}\geq \frac{m_1Q_1+m_2Q_2+g_0 Q_3}{1-[\ell_1Q_1+\ell_2 Q_2+ \mathbb{L} Q_3 ]}$. First, we will prove that $\mathcal{F}\mathbb{B}_{y^{*}}\subseteq \mathbb{B}_{y^{*}}.$ Applying $(D_{1}), (D_{2})$, for all $v\in \mathbb{B}_{y^{*}}$, we get relations

Then, we have

Hence $\| (\mathcal{F}v)\|\le y^{*}$ which means that $\mathcal{F}\mathbb{B}_{y^{*}}\subseteq \mathbb{B}_{y^{*}}$.

In the next step, we will prove that the operator $\mathcal{F}$ is a contraction. For $v_{1}, v_{2}\in \mathbb{B}_{y^{*}}$, and $t\in [0, T],$ we get

which leads to $\| \mathcal{F}v_{1} - \mathcal{F}v_{2}\|\leq [\ell_1Q_1+\ell_2 Q_2+ \mathbb{L} Q_3 ]\| v_{1} - v_{2}\|$. As $\ell_1Q_1+\ell_2 Q_2+ \mathbb{L} Q_3 < 1$, $\mathcal{F}$ is a contraction. Hence Banach's contraction mapping principle implies that $\mathcal{F}$ has a unique fixed point, which is a solution of the problem (1.1). The proof is completed. □

The next existence result is based on Krasnosel'skiĭ's fixed-point theorem ^[20].

Theorem 3.2. Assume that $g:[0, T]\times \mathbb{R} \longrightarrow \mathbb{R}$ is a continuous function satisfying the condition $(D_{1})$ and such that:

$(D_{3})$ $| g(t, v(t))|\leq \phi(t), \; \; \; \forall\; (t, v)\in [0, T]\times \mathbb{R}$ and $\phi\in C([0, T], \mathbb{R})$.

Moreover, we suppose that $f_i$, $i = 1, 2$ are functionals satisfying $(D_2)$, and there exist $M_i > 0, i = 1, 2$ such that

$(D_{4})$

Then the problem (1.1) has at least one solution on $[0, T],$ provided that

where

Proof. Define the operators $\mathcal{F}_{1}, \mathcal{F}_{2}:\mathcal{X}\longrightarrow \mathcal{X}$ by

Let $\sup_{t\in[0, T]}\phi(t) = \| \phi \|$ and $\delta\geq M_1Q_1+M_2Q_2+\|\phi\|Q_3$, where $Q_i, i = 1, 2, 3$ are defined by (3.2). We consider $\mathbb{B}_{\delta} = \{v\in \mathcal{X}: \| v \|\leq \delta\}$. For all $v\in \mathbb{B}_{\delta}$, as in the proof of Theorem 3.1, we obtain:

Thus, $\|\mathcal{F}_{1}v + \mathcal{F}_{2}u\|\le \delta,$ which means that $\mathcal{F}_{1}v + \mathcal{F}_{2}u\in \mathbb{B}_{\delta}.$ On the other hand, using $(D_{1}), (D_2)$, we obtain:

and consequently $\| \mathcal{F}_{1}v_{1}-\mathcal{F}_{1}v_{2}\|\le [\ell_1Q_1+\ell_2 Q_2+ \mathbb{L} Q_3' ]\| v_{1} - v_{2}\|,$ which by (3.3) implies that $\mathcal{F}_{1}$ is a contraction.

By the continuity of $g$ the operator $\mathcal{F}_{2}$ is continuous. Besides, for all $v\in \mathbb{B}_{\delta}$, we obtain:

and therefore $\mathcal{F}_{2}$ is uniformly bounded.

Next, the compactness property of the operator $\mathcal{F}_{2}$ is proved. For all $t_{1}, t_{2}\in [0, T]$ with $t_{1} < t_{2}$ we have:

which tends to zero as $t_{2}-t_{1}\rightarrow 0$ independently of $v$. Consequently, Arzelá-Ascoli theorem implies that $F_{2}$ is compact on $\mathbb{B}_{\delta},$ and Krasnosel'skiĭ's fixed-point theorem implies that the problem (1.1) has at least one solution on $[0, T]$. □

Remark 3.3. If we interchange the roles of $\mathcal{F}_1$ and $\mathcal{F}_2,$ we have another existence result, where the condition (3.3) is replaced by the following:

The final result is based on Leray-Schauder's nonlinear alternative ^[21].

Theorem 3.4. Assume that $(D_4)$ holds. In addition, we suppose that:

$(D_{5})$ There exist a continuous nondecreasing function $\omega:[0, \infty)\longrightarrow (0, \infty)$ and a continuous function $q:[0, T]\longrightarrow(0, \infty)$ such that:

$(D_{6})$ There exists a constant $M > 0$ such that

where $Q_i, i = 1, 2, 3$ are defined by (3.2).

Then, the boundary value problem (1.1) has at least one solution on $[0, T]$.

Proof. First, we will show that the operator $\mathcal{F}$ maps bounded sets into bounded sets in $\mathcal{X}$. Assume that $\mathbb{B}_{y} = \{v\in \mathcal{X}: \| v \|\leq y\}$ be a bounded set in $\mathcal{X}$. Then for $t\in [0, T]$ we have

and hence $\| \mathcal{F}v\|\le M_1Q_1+M_2Q_2+\|q\|\omega(y)Q_3.$

Next, we will show that the operator $\mathcal{F}$ maps bounded sets into equicontinuous sets of $\mathcal{X}$. Let $t_{1}, t_{2}\in [0, T]$ with $t_{1} < t_{2}$ and $v\in B_{y}$. In Theorem 3.2, we have proved that the operator $\mathcal{F}_2$ is equicontinuous. Now we will prove that the operator $\mathcal{F}_1$ is also equicontinuous. For all $t_{1}, t_{2}, \in [0, T]$ with $t_{1} < t_{2}$ we have:

which tends to zero as $t_{2}-t_{1}\rightarrow 0$ independently of $v$. By Arzelá-Ascoli theorem, $\mathcal{F}_2$ is equicontinuous. Therefore, the operator $\mathcal{F}$ is equicontinuous.

Finally, we indicate that there exists an open set $Z\subseteq \mathcal{X}$ with $v = \theta \mathcal{F}v$ for $\theta\in (0, 1)$ and $v\in \partial Z$. Let $v$ be a solution. Then, similar to the proof in the first step, for $t\in [0, T],$ we obtain:

which leads to

Due to $(D_{6})$ there exists $M > 0$ such that $\| v \|\neq M$. Put

Obviously, the operator $F:\overline{Z}\longrightarrow C([0, T], R)$ is continuous and completely continuous. In view of the choice of $Z$, there is no $v\in \partial Z$ such that $v = \theta \mathcal{F}v$ for $\theta\in (0, 1)$. Consequently, using the nonlinear alternative of Leray-Schauder type, we deduce that $\mathcal{F}$ has a fixed point $v\in Z$ which is a solution of the boundary value problem (1.1). □

4.   Examples

In this section, we will demonstrate some applications of our results to ensure that, by varying the nonlinear functions and functionals, solutions exist for mixed Erdelýi-Kober and Caputo fractional differential equations with nonlocal, non-separated boundary conditions on a given interval. Let us consider the following boundary value problem:

From this given situation, the constants can be set as follows: $\alpha = 3/4$, $\beta = 1/4$, $\gamma = -1/2$, $\delta = 1/2$, $T = 5/3$, $a_1 = 7/99$, $a_2 = 5/88$, $b_1 = 3/77$, $b_2 = 1/66$, $\alpha-\beta(1+\gamma) = 5/8$. Using these constant values, we can determine that $Q_1\approx7.841584158$, $Q_2\approx41.00177341$ and $Q_3\approx5.335618454$.

$(i)$ Assume the nonlinear unbounded function $g:[0, 5/3]\times\mathbb{R}$ and functionals $f_1, f_2:\mathcal{X}\to\mathbb{R}$ are given by

From these, we can conclude that the conditions $(D_1)$ and $(D_2)$ are fulfilled with the constants $\mathbb{L} = 1/16$, $\ell_1 = 1/24$ and $\ell_2 = 1/125$ leading to the inequality

The benefit of Theorem 3.1 is that it can be applied to show that the mixed Erdelýi-Kober and Caputo fractional differential equations with nonlocal, non-separated boundary conditions (4.1)-(4.2) have a unique solution on the interval $[0, 5/3]$.

$(ii)$ Now, we suppose that the given bounded function $g$ and functionals $f_1, f_2$ are defined as

Here we see that $g$ and $f_i$, $i = 1, 2$, satisfy conditions $(D_1)$ and $(D_2)$ in Theorem 3.1 with $\ell_1 = 1/27$, $\ell_2 = 1/84$ and $\mathbb{L} = 1/18$. Then we can check that

which contradicts a sufficient inequality in Theorem 3.1. An alternative approach, we can check the boundedness as

and $|f_1(v)|\leq1/27$, $|f_2(v)|\leq \pi/168$. In addition, we have

where $Q_3'\approx2.506725983$. The conclusion of Theorem 3.2 implies that the boundary value problem (4.1)–(4.3) has at least one solution on the interval $[0, 5/3]$.

$(iii)$ If the expression of $g$ and $f_i$, $i = 1, 2$, in (4.1) is shown as

then we cannot apply the method based on the Lipschitz condition. Hence, we will check their boundedness by

and $|f_1(v)|\leq1/12$, $|f_2(v)|\leq 1/28$ computing $\|q\| = 1/17$. Choosing $M_1 = 1/12$, $M_2 = 1/28$, there exists a constant $M\in (2.24266923, 45.54935379)$ satisfying condition $(D_6)$. Using Theorem 3.4, we can conclude that the problem (4.1) with (4.4) has at least one solution on the interval $[0, 5/3]$.

$(iv)$ Let $g$ and $f_1, f_2$ be defined by

By applying Lemma 2.7, we can also obtain the solution to the linear problem (4.1) with (4.5). In addition, we have examined the behavior of the solution for this example and visualized the graph to provide clear evidence of the existence of a solution to mixed Erdelýi-Kober and Caputo fractional differential equations. The domain for $t$ is considered from $0$ to $5/3$, and parameter $m$ is varied from $1$ to $10$. The resulting graph is presented in Figure 3. The graph demonstrates how the parameter $m$ affects the solution $v(t)$. In the initial range of $t$, as $m$ increases, the solution $v$ grows gradually. However, from around $t = 1$ onward, the behavior shifts: with increasing $m$, the solution begins to rise more rapidly.

5.   Conclusions

In this paper, we investigated a sequential fractional boundary value problem consisting by of a combination of Erdelýi-Kober and Caputo fractional differential operators and subjected to nonlocal non-separated boundary conditions. Existence and uniqueness results were established by using fixed-point theory. The existence of a unique solution was proved with the help of Banach's fixed-point theorem, while the existence result was established via Krasnosel'skiĭ's fixed-point theorem and Leray-Schauder nonlinear alternative. The obtained results were well illustrated by the constructed numerical examples.

The results are new and contribute significantly to this new research subject. For future work, we plan to apply this new method to study other kinds of boundary value problems with nonzero initial conditions as well as coupled systems of fractional differential equations containing a combination of Caputo and Erdélyi-Kober fractional derivative operators.

Author contributions

Ayub Samadi: Conceptualization, methodology, writing–original draft preparation. Chaiyod Kamthorncharoen: Conceptualization, methodology, writing–original draft preparation. Sotiris K. Ntouyas: Conceptualization, methodology, writing–review suggestions and editing, supervision. Jessada Tariboon: Conceptualization, methodology, writing–review and editing, funding acquisition. All authors have read and agreed to the published version of the manuscript.

Acknowledgments

This research was funded by King Mongkut's University of Technology North Bangkok. Contract no. KMUTNB-67-KNOW-30.

Conflict of interest

The authors declare no conflicts of interest.