Comparison of two modified analytical approaches for the systems of time fractional partial differential equations

1.   Introduction

Fractional calculus is considered to be the main research area nowadays due to its numerous applications in applied sciences. These applications have attracted the attention of researchers. The advanced applications in the field of applied science and technology include the nonlinear oscillation fractional order earth quack model, fractional order airfoil model ^[1], fractional-order fluid dynamic traffic model ^[2], financial model with fractional order ^[3], financial models chaos theory ^[4], fractional order Zener model ^[5], fractional Zener model of signal processing phenomena ^[6], Poisson-NerstPlanck diffusion model of fractional order ^[7], electrodynamics ^[8], fractional model of cancer chemotherapy ^[9], optics ^[10], fractional model for the dynamics of hepatitis B virus ^[11], fractional model for tuberculosis ^[12], fractional-order pine wilt disease model ^[13] and various others ^{[14,15,16,17]}.

Whenever the applications attract attention, on the other side, the solution of fractional order physical models also gains the attention of researchers. The solution has become an important issue for researchers ^[18,19,20]. Various analytical and numerical techniques have been introduced by researchers, such as the homotopy perturbation technique ^[21], Laplace Adomian decomposition method ^[22], Laplace homotopy perturbation transform technique ^[23], Crank-Nicholson finite difference method ^[24], p-homotopy analysis method ^[25], novel operational matrix-based method ^[26], Harr wavelet collection approach ^[27], natural transform decomposition method ^[28], dynamical system method ^[29], new approximate analytical method ^[30], new modified decomposition method ^[31], new analytical technique ^[32], approximate analytical method ^[33], kernal Method ^[34], fully Petrov-Galerkin spectral method ^[35] and various others ^[36,37,38].

In the connection of these numerical and analytical methods, we have also used two different analytical approaches and tested for the solution of the non-linear time-fractional system of partial differential equations (PDEs). These approaches are the new approximate analytical approach (NAAA) and Mohand variation iteration transform approach (MVITA). The NAAA is a decomposition procedure that uses Caputo-Riemann operators of derivatives and integration, respectively. Using the combined property of both operators provides a series form solution with fractional order. The obtained fractional-order series-form solution rapidly converges to the exact solution by approaching integer order. The MVITA is a variational iteration strategy that use a new integral transform (Mohand transform). It provides the series form solution without using any decomposition, He's polynomial or discretization. The series form solution provides an exact form solution for the problems by using a specific value of summation, i.e., an integer value instead of fractional order.

We have tested both of the approaches by solving the following systems of time-fractional differential equations ^[39]

where $\vartheta, \tau \in\mathbb{R}, \eth\in (0, 1]$ and

where $\vartheta, \tau \in\mathbb{R}$ and $\eth\in (0, 1]$; we can verify the obtained results with the exact solution and for different fractional orders. We also compared the results of the NAAA and MVITA numerically and by plotting.

The rest of the article is organized as follows. Some related basic definitions and results are elaborated in Section 2. In Section 3, we explained the generalized procedure of the NAAA; in Section 4, we presented the generalized procedure of the MVITA. In Section 5, we tested some systems of PDEs based on the NAAA and MVITA. Finally, we concluded the present research results in Section 6.

2.   Preliminaries and basic concepts

In this section, we have defined some relative elementary concepts of the research work.

Definition 2.1. ^[40] The Riemann-Liouville fractional partial integral $\wr_\tau^{\eth},$ where $\eth\in\mathbb{N}$ and $\eth \geq0$, is defined as

where $\eth$ is denotes the gamma function.

Let $\eth, \beta \in\mathbb{R}\setminus\mathbb{N}$ and $\eth, \beta > 0, \alpha > -1$ then, for the function $\zeta(\vartheta, \tau)$, the operator $\wr_\tau^{\eth}$ has the following properties ^[40]

(ⅰ) $\wr_\tau^{\eth}\zeta(\vartheta, \tau) \wr_\tau^{\beta}\zeta(\vartheta, \tau) = \wr_\tau^{\eth+\beta}\zeta(\vartheta, \tau);$

(ⅱ) $\wr_\tau^{\eth}\zeta(\vartheta, \tau) \wr_\tau^{\beta}\zeta(\vartheta, \tau) = \wr_\tau^{\beta}\zeta(\vartheta, \tau)\wr_\tau^{\eth}\zeta(\vartheta, \tau);$

(ⅲ) $\wr_\tau^{\eth}\tau^{\alpha} = \frac{\Gamma(\alpha+1)}{\Gamma(\eth+\alpha+1)}t^{\eth+\alpha}.$

Definition 2.2. ^[40] The Caputo operator of the fractional partial derivative is defined by

Theorem 2.1. ^[41] Let $\eth, \tau \in\mathbb{R}, \tau > 0$ and $m-1 < \vartheta < m\in\mathbb{N};$ then,

and

The Mohand transform, which is represented by $M(\cdot)$ for a function $\zeta(\tau)$, is defined as

The Mohand transform of a function $\zeta(\tau)$ is $R(\upsilon)$; then, $\zeta(\tau)$ is called the inverse of $R(\upsilon)$, which is expressed as

where $M^{-1}$ is the inverse Mohand operator.

The Mohand transform for $n$th derivatives is defined by ^[41]

3.   Generalized procedure for implementation of NAAA

In this section, we extend the NAAA implementation for the analytical treatment of the general equation of non-linear fractional order PDEs defined as ^[30]:

with the initial sources

where $£$ is the linear operator, $\aleph$ is the non-linear operator and $\delta(\vartheta, \gamma, \tau)$ is a source function.

The computational work will use the following basic concepts.

Lemma 3.1. ^[30] For $\zeta(\vartheta, \gamma, \tau) = \sum_{k = 0}^{\infty}\lambda^k \zeta_k(\vartheta, \gamma, \tau),$ the linearity of $£\zeta(\vartheta, \gamma, \tau)$ is given the following result:

Theorem 3.1. ^[30] If the non-linear term with the $\eta$ parameter $\zeta_k(\vartheta, \gamma, \tau) = \sum_{0}^{\infty}\lambda^k \zeta_k(\vartheta, \gamma, \tau)$, then the nonlinear operator $\aleph\zeta(\vartheta, \gamma, \tau)$ satisfies the following property:

Definition 3.1. ^[30] The non-linear term $\aleph\left(\zeta_k(\vartheta, \gamma, \tau)\right)$ is uniquely expressed in summation form as follows:

The following results verify the existence and uniqueness of the NAAM solution.

Theorem 3.2. Let $\delta(\vartheta, \gamma, \tau)$ and $\zeta(\vartheta, \gamma, \tau)$ be defined for $n-1 < \eth < n$ in (3.1); the wave model with variable coefficients (3.1) yields the unique solution as

where $£_\tau^{-\eth}(\zeta_{(k-1)})$ and $\Im_{(k-1)\tau}^{-\eth}$ represent the Riemmen fractional order ($\eth$) integration with the parameter $\tau$.

Proof. The solution $\zeta(\vartheta, \gamma, \tau)$ of the wave model is achieved by using the expansion as

Similarly, the solution is further summarized by the following procedure

To evaluate the solution of the wave model (3.1), we compute as

with the initial conditions

by using the Riemann integral operator (2.1), with respect to $\tau$, and by applying the basic property, we have

Now, by substituting the initial conditions and (3.5) in (3.8), we get

with using Lemma 3.1 and Definition 3.1, (3.11) becomes

By equating the identical power of $(\lambda)$, the iterative scheme becomes

and

for $k = 2, 3, \ldots.$

4.   Generalized procedure for implementation of MVITA

Consider the general equation defined by (3.1) with the initial condition

Now, by applying the Mohand transform to (3.1), we get

Using the iteration property of transformation, we obtain

And, by using the iterative technique with the Lagrange multiplier $(-\lambda(\upsilon))$, we have

where $\lambda(\upsilon) = - \frac{1}{\upsilon^\eth}.$ Putting (4.3) in (4.2), we get

By applying the inverse Mohand transform to (4.4), we obtain

The initial condition becomes

The recursive scheme becomes

The approximate solution is obtained through the recursive scheme defined by (4.5).

5.   NAAA and MVITA testing analysis

In this section, we test the NAAA approach for the solution of the time fractional time system of PDEs ^[29].

Example 5.1. Consider a non-linear time fractional time system of PDEs in the form of

where $\vartheta, \tau\in\mathbb{R}$ and $\eth\in (0, 1]$ and we have the following conditions:

the exact solution for integer order is

5.1.   Testing Problem 1 by using the NAAA

For the solution of (1.1), we assume the final procedure described by (3.10) and the final recursive scheme becomes

and

for $k = 2, 3, \ldots.$ Consequently, we obtain

and by using

we get the second approximation as

Now, we apply the general recursive scheme as follows:

The NAAM becomes

by substituting the values of (5.5), (5.7) and (5.9) in (5.10), we obtain

For the special case $\gamma = 1$, the NAAA solution in series form becomes

this series form solution directly converges to the exact solution of the problem:

5.2.   Testing Problem 1 by using the MVITA

Consider the same non-linear system of time-fractional PDEs defined by (1.1) with the initial conditions

By using the general recursive scheme (4.5), we get

So, the initial condition becomes

Now, for different values of $n$ $(n = 0, 1, 2, \ldots)$, we have

By simplifying with the initial condition, we get

Consequently, we get

The series form solution, we get

Thus, the solution becomes

For a specific integer value $\eth = 1$, the MVITA solution becomes

which is the exact solution of the problem.

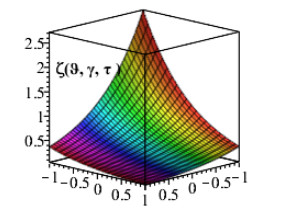
In Figure 1, the approximate solution of $\zeta(\vartheta, \gamma, \tau)$ is obtained by using the NAAA for Example 5.1. Figure 2, the approximate solution of $\zeta(\vartheta, \gamma, \tau)$ is obtained by using the MVITA for Example 5.1. Figure 3, the approximate solution of $\xi(\vartheta, \gamma, \tau)$ is obtained by using the NAAA for Example 5.1. And Figure 4, the approximate solution of $\xi(\vartheta, \gamma, \tau)$ is obtained by using the MVITA for Example 5.1.

Example 5.2. Consider a non-linear time-fractional system of PDEs in the form of

where $\vartheta, \tau \in\mathbb{R}$ and $\eth\in (0, 1]$ with the conditions

the exact solution for integer order is

5.3.   Testing Problem 2 by using the NAAA

For the solution of (5.16), we assume the final procedure described by (3.10), and the final recursive scheme becomes

and

where $\aleph_{1} = -\zeta_\vartheta \xi_\vartheta, \aleph_{2} = -\zeta_\gamma\xi_\gamma, \aleph_{3} = -\xi_\vartheta \Im_\vartheta, \aleph_{4} = \xi_\gamma \Im_\gamma, \aleph_{5} = -\Im_\vartheta\zeta_\vartheta\; and\; \aleph_{6} = -\Im_\gamma\zeta_\gamma$ are the non-linear terms of the given problem.

Consequently, we can obtain the approximated terms as

Now, by using

we obtain the second approximation as

The other approximated terms by obtained by using

For different values of $k$, we get

The NAAM becomes

By substituting the values of (5.20), (5.22) and (5.24) in (5.25), we obtain

For the special case $\gamma = 1$, the NAAA solution in series form becomes

this series form solution directly converges to the exact solution of the problem:

5.4.   Testing Problem 2 by using the MVITA

Consider the same non-linear system of time-fractional PDEs defined by (5.16) with the initial conditions

By using the general recursive scheme (4.5), we get

So, the initial guess becomes

Now, for different values of $n$ $(n = 0, 1, 2, \ldots)$, we have

By simplifying with the initial conditions, we get

Consequently, we get

similarly, we get

Thus the solution becomes

For a specific integer value $\eth = 1$, the MVITA solution becomes

which is the exact solution of Example 5.2.

In Figure 5, the approximate solution of $\zeta(\vartheta, \gamma, \tau)$ is obtained by using the NAAA for Example 5.2. Figure 6, the approximate solution of $\xi(\vartheta, \gamma, \tau)$ is obtained by using the NAAA for Example 5.2. Figure 7, the approximate solution of $\Im(\vartheta, \gamma, \tau)$ is obtained by using the NAAA for Example 5.2. Figure 8, the approximate solution of $\zeta(\vartheta, \gamma, \tau)$ is obtained by using the MVITA for Example 5.2. Figure 9, the approximate solution of $\xi(\vartheta, \gamma, \tau)$ is obtained by using the MVITA for Example 5.2. Figure 10, the approximate solution of $\Im(\vartheta, \gamma, \tau)$ is obtained by using the MVITA for Example 5.2.

6.   Conclusions

The current article has introduced two analytical approaches, the NAAA and MVITA, to solve PDEs. Their validity and applicability were checked by solving time-fractional systems of PDEs. For Example 5.1, the approximations defined by (5.11) and (5.15) have shown that the MVITA has rapid convergence to the exact solution for the problem for integer order; this was also demonstrated by plotting. Similarly, for Example 5.2, the approximated results represented by (5.26) and (5.28) have shown that the MVITA has a series-form solution which quickly converges to the exact solution for the problem; this was checked by plotting. Overall, it has been demonstrated that the MVITA has less computational work and rapid convergence without decomposition, He's polynomial or discretization, while, in the case of the NAAA, the non-linearity is decomposed by an Adomian decomposition procedure, and it has a slow rate of convergence for non-linear problems. The MVITA can easily be extended to high order non-linear physical models.

Acknowledgments

The authors extend their appreciation to the Deanship of Scientific Research at King Khalid University for funding this work through Large Groups (R.G.P2./41/43).

Conflict of interest

The authors declare no conflicts of interest.