Figure 1.
Evolution of the kernel density estimation of digital economy efficiency.
In this paper, assume that H is a Hopf algebra and A/B is an H-Galois extension. Firstly, by introducing the concept of an H-stable tilting complex T∙ over B, we show that T∙⊗BA is a tilting complex over A and a derived equivalence between two H-module algebras can be extended to smash product algebras under some conditions. Then we observe that 0→EndDb(B)(T∙)→EndDb(A)(T∙⊗BA) is an H-Galois Frobenius extension if A/B is an H-Galois Frobenius extension. Finally, for any perfect recollement of derived categories of H-module algebras, we apply the above results to construct a perfect recollement of derived categories of their smash product algebras and generalize it to n-recollements.
Citation: Jinlei Dong, Fang Li, Longgang Sun. Derived equivalence, recollements under H-Galois extensions[J]. AIMS Mathematics, 2023, 8(2): 3210-3225. doi: 10.3934/math.2023165
| [1] | Qingsheng Zhu, Changwen Xie, Jia-Bao Liu . On the impact of the digital economy on urban resilience based on a spatial Durbin model. AIMS Mathematics, 2023, 8(5): 12239-12256. doi: 10.3934/math.2023617 |
| [2] | Zimei Huang, Zhenghui Li . The impact of digital economy on the financial risk ripple effect: evidence from China. AIMS Mathematics, 2024, 9(4): 8920-8939. doi: 10.3934/math.2024435 |
| [3] | Gaoxiang Yang, Xiaoyu Li . Bifurcation phenomena in a single-species reaction-diffusion model with spatiotemporal delay. AIMS Mathematics, 2021, 6(7): 6687-6698. doi: 10.3934/math.2021392 |
| [4] | Lishan Fang . Smooth digital terrain modeling in irregular domains using finite element thin plate splines and adaptive refinement. AIMS Mathematics, 2024, 9(11): 30015-30042. doi: 10.3934/math.20241450 |
| [5] | Xiangsong Ye, Longju Zhou, Zhiyong Wang . Spatial convergence characteristics of low carbon economy and economic growth quality: based on Guangdong urban data. AIMS Mathematics, 2024, 9(5): 11660-11695. doi: 10.3934/math.2024572 |
| [6] | Yong Tao . Generalized Pareto distribution and income inequality: an extension of Gibrat's law. AIMS Mathematics, 2024, 9(6): 15060-15075. doi: 10.3934/math.2024730 |
| [7] | Shuo Xu, Chunrui Zhang . Spatiotemporal patterns induced by cross-diffusion on vegetation model. AIMS Mathematics, 2022, 7(8): 14076-14098. doi: 10.3934/math.2022776 |
| [8] | Akbar Moradi, Alireza Amirteimoori, Sohrab Kordrostami, Mohsen Vaez-Ghasemi . Closest reference point on the strong efficient frontier in data envelopment analysis. AIMS Mathematics, 2020, 5(2): 811-827. doi: 10.3934/math.2020055 |
| [9] | Anna Sun, Ranchao Wu, Mengxin Chen . Turing-Hopf bifurcation analysis in a diffusive Gierer-Meinhardt model. AIMS Mathematics, 2021, 6(2): 1920-1942. doi: 10.3934/math.2021117 |
| [10] | Pedro J. Gutiérrez-Diez, Jorge Alves-Antunes . Stock market uncertainty determination with news headlines: A digital twin approach. AIMS Mathematics, 2024, 9(1): 1683-1717. doi: 10.3934/math.2024083 |
In this paper, assume that H is a Hopf algebra and A/B is an H-Galois extension. Firstly, by introducing the concept of an H-stable tilting complex T∙ over B, we show that T∙⊗BA is a tilting complex over A and a derived equivalence between two H-module algebras can be extended to smash product algebras under some conditions. Then we observe that 0→EndDb(B)(T∙)→EndDb(A)(T∙⊗BA) is an H-Galois Frobenius extension if A/B is an H-Galois Frobenius extension. Finally, for any perfect recollement of derived categories of H-module algebras, we apply the above results to construct a perfect recollement of derived categories of their smash product algebras and generalize it to n-recollements.
Abbreviation:
ICT: Information and communication technology; TFP: Total Factor Productivity
Despite the substantial scale of China's digital economy, its development quality may not always be as positive as anticipated, which can be attributed to certain resource allocation challenges. One significant challenge is the uneven distribution of digital resources among provinces in China. It leads to an over-concentration of benefits in economically thriving areas and perpetuates the digital divide. An analysis by DataReportal reveals significant disparities in internet penetration rate across provinces, ranging from 38% in Tibet to 93% in Beijing. Moreover, the quality and speed of internet access also vary across regions, determined by factors such as the availability and affordability of broadband and mobile networks.1 These disparities make it challenging for less-developed regions to fully participate in the digital economy and compete against large platforms. Consequently, the development gap between regions deepens, potentially squeezing their profit margins, excluding them from the market and leading to a negative cycle of underdevelopment. Another significant challenge is the improper utilization of digital and financial resources. Different departments compete to develop and create a plethora of digital products and digital services that share common functions. Some investors may prioritize projects that appear impressive on the surface but fail to align with actual needs or consider long-term sustainability. Some may focus on infrastructure construction without adequate attention to maintenance. Some may excessively prioritize infrastructure and platform construction without giving sufficient attention to the application. A survey conducted by The State Information Center in 2019 revealed that less than 5% of big data investment projects in Fujian, Chongqing, Zhejiang and Guangdong were dedicated to applications.2
1 The information comes from the website, https://datareportal.com/reports/digital-2022-china.
2 For the latest news about digital economy development in China, once can refer to the official website of The State Information Center at http://www.sic.gov.cn/index.htm. This website serves as a reputable source of information, providing comprehensive and authoritative reports on the advancements and initiatives in China's digital economy.
The majority of studies in the field of evaluating the digital economy utilize scale measurement and comprehensive evaluation approaches. When it comes to scale measurement, two main categories can be identified based on the methods employed in the research. One commonly used method is the value-added approach. This method defines the scope of the digital economy according to the digital sectors that produce or use digital technology or ICT as their main inputs or outputs. It then calculates the value added by these sectors using national economic accounting data [1,2,3]. The Digital Economy Satellite Account (DESA) is another method. This method constructs a satellite account for the digital economy based on the existing national accounts framework. It identifies the digital transactions and activities that are embedded in various industries and adjusts their output and value added accordingly [4,5,6,7,8,9]. Clearly, both the value-added approach and DESA are effective measurements of the digital economy. However, challenges still exist in these measurement approaches [5,7,8,10]. For the value-added approach, the deeply integrated nature of the digital economy within the real economy poses challenges to traditional statistical calibers. In the case of DESA, it is considered an ideal tool for observing Digital Industrialization and Industrial Digitalization, but currently, research on DESA is in its early stages and several issues remain to be resolved. These include determining the value of free digital content, addressing classification issues and ensuring data availability and quality. In the literature on evaluating the digital economy, comprehensive evaluation is another dominant stream. There is extensive literature providing an overview of the general condition of the digital economy by employing the index calculation method in different countries [11,12,13,14,15,16,17,18] or regions in a country [10,19,20,21,22,23] due to its simplicity, data availability and ease of comparison. The basic steps of index calculation are:
(ⅰ) Selecting indicators that are relevant, reliable and representative of the digital economy.
(ⅱ) Standardizing the indicators to eliminate the differences in units, scales and directions of the indicators.
(ⅲ) Assigning weights to the indicators to reflect their relative importance in the digital economy.
(ⅳ) Aggregating the standardizing and weighted indicators to obtain a composite index that summarizes the overall performance of the digital economy. There are different methods for standardizing, weighting and aggregating the indicators.
The majority of studies tend to focus on surface-level metrics of digital economy while overlooking the deeper aspects related to development quality, specifically input-output efficiency. They primarily focus on assessing the performance of the digital economy in terms of size, growth and variations among different countries or regions. While these metrics provide valuable insights, it is the efficient utilization of resources and the effectiveness of converting inputs into outputs that truly signify the quality of development. They reflect how effectively resources, including labor and capital are being utilized within the digital economy. They capture the ability to maximize output while minimizing input, highlighting the potential for productivity gains and sustainable growth. Therefore, to ensure a more comprehensive evaluation, there is a need to expand existing research by incorporating measurement of input-output efficiency. By incorporating it into the assessment, policymakers and researchers can gain a more holistic understanding of the digital economy's overall performance, facilitating informed decision-making aimed at enhancing its effectiveness and promoting sustainable development. To address this gap, this research assesses the digital economy efficiency using the Data Envelopment Analysis (DEA) method. However, most literature uses two approaches to measure the efficiency [1,24,25,26,27,28]. The first method is the DEA method for calculating the efficiency, which can only be compared at a given point in time because the method employs a different efficient frontier for each period. The second method is the DEA-Malmquist Index, which allows for the comparison of efficiency across different periods for the same DMU. To overcome these limitations, this paper calculates the global efficiency that allows for exploration of efficiency variations among different regions and periods.
This study makes several marginal contributions to the assessment of digital economy development. First, this paper provides a metrics of development quality within the digital economy, exploring diverse aspects beyond surface-level metrics like the overall development level of the digital economy. It places a particular emphasis on the input-output efficiency, offering unique insights into the nature of digital economy development. Second, the digital economy efficiency measured in this paper can be directly compared across different periods and regions, effectively overcoming the limitations associated with traditional measurement methods. Third, this paper provides a detailed portrayal of the characteristics of digital economy efficiency. Specifically, it explores the regional variations and temporal changes in efficiency.
The remainder of the paper is organized as follows: Section 2 provides a theoretical analysis of this topic and introduces the model and data used in this study, establishing a foundation for the subsequent empirical analysis. Sections 3–5 reveal the spatiotemporal characteristics of digital economy efficiency, shedding light on efficiency variations across different regions and time periods. Finally, Section 6 summarizes the findings of the study and discusses their implications.
Digital economy efficiency refers to the ability to optimize resource utilization and achieve optimal outcomes. This concept relies on the utilization of the frontier production function to explore the potential for reaching the maximum production possibility boundary. The proximity to this frontier is directly correlated with the effectiveness of the digital economy; the closer an entity operates to this boundary, the greater its efficiency. However, attaining optimal digital economy efficiency can be a formidable task, often hampered by inherent ineffectiveness within the system. Several factors contribute to this efficiency, including technological efficiency, technological progress and scale effects. The measurement of technological efficiency is based on the comparison of the actual output with the frontier output, which indicates how well the inputs are used given the current state of technology. The smaller the distance between these outputs, the higher the technological efficiency. It can be improved by using existing technologies more effectively and efficiently, such as by adopting best practices, optimizing processes or reducing errors and waste [29,30]. The measurement of technological progress is based on the change of the frontier production function over time, which reflects the improvement in the state of technology. The upward shift of the frontier production function indicates that higher potential output can be achieved from the same set of inputs due to technological progress. The larger the shift, the higher the technological progress. It can be achieved by developing and introducing new technologies that enhance the productivity and quality of outputs, such as by innovating new products, services or platforms, or by discovering new methods, techniques or algorithms [31]. Additionally, scale effects refer to the changes in output resulting from changes in the scale or size of production. Scale effects can affect both the level and the shape of the frontier production function. If there are increasing returns to scale, then output increases more than proportionally with inputs, implying that larger scale production is more efficient. If there are decreasing returns to scale, then output increases less than proportionally with inputs, implying that smaller scale production is more efficient. If there are constant returns to scale, then output increases proportionally with inputs, implying that scale does not affect efficiency.
Efficiency changes in the digital economy are intricately connected to its external circumstances [19]. Throughout different periods, there have been distinct factors driving and challenging the digital economy efficiency. In 2008, the global financial crisis had a significant impact on the digital economy. The reduced demand for goods and services, disrupted supply chains and heightened uncertainty and risk likely influenced the innovation and investment efficiency during this period. In 2013, the digital economy entered a new phase of development highlighted by the emergence of big data and its analysis as prominent topics. With this case, the realization of scale effects, wherein the size of scale of operations played a role in influencing efficiency to a certain extent. Technological advancements enabled the provision of more personalized, customized and optimized products and services, as well as new business models and platforms. These changes likely contributed to its improvements in efficiency. In 2020, the digital economy played a crucial role in responding to the COVID-19 pandemic. It facilitated various functions such as remote work, online learning, telehealth, e-commerce and social distancing. The deeper integration of digital technologies into various aspects of society and economic activities may have led to further enhancements in digital economy efficiency during this time. In conclusion, the efficiency of the digital economy is not static but subject to changes influenced by external circumstances. The 2008 financial crisis, the emergence of big data in 2013 and the role of the digital economy in responding to the COVID-19 pandemic in 2020 are examples of how different factors have shaped the digital economy efficiency over time.
The digital economy efficiency varies among provinces in correlation with regional economic conditions and government policies [19,32,33,34,35]. The economic prosperity of a region creates a favorable environment for the digital economy to thrive as provinces with a strong economy can allocate resources to invest in digital infrastructure, such as high-speed internet connectivity, data centers and smart city initiatives. This enhanced infrastructure supports the seamless flow of digital information and enables businesses to operate efficiently in the digital space. Additionally, higher disposable incomes and greater consumer demand foster a vibrant market for digital goods and services, stimulating innovation and customer satisfaction. Furthermore, regional economic conditions influence the availability of skilled human capital. Provinces with better economic conditions often have more resources to invest in education and training programs, thereby cultivating a skilled workforce that possesses the necessary digital competencies. In addition to regional economic conditions, government policies also play a critical role in shaping the efficiency of the digital economy. Provinces that implement supportive policies and regulations tend to foster a conducive environment for digital innovation and entrepreneurship. This may include offering tax incentives for digital startups, streamlining regulatory processes and promoting investment in digital infrastructure. These policies encourage businesses to adopt digital technologies, stimulate competition and innovation and promote the overall efficiency of the digital economy.
This section introduces the global super-efficiency model used to measure the digital economy efficiency, chosen after comparing various measurement methods. Two widely used techniques for efficiency measurement are Stochastic Frontier Analysis (SFA) and Data Envelopment Analysis (DEA). They both compare observed output with maximum potential output to assess relative production unit efficiency [36]. SFA employs a specific functional form for the production function, such as Cobb-Douglas or Translog. Maximum likelihood estimation is employed to estimate the frontier, which is then determined as the expected value of the best-performing units in the dataset. On the other hand, DEA is a non-parametric method that does not rely on a pre-determined functional form for the production function. It avoids imposing subjectivity and arbitrary restrictions on the technology. To envelope the best performing units in the dataset, it constructs the frontier using linear programming. Efficiency only determines whether a unit is efficient or not. However, super-efficiency is a refined measure that allows for the ranking of efficient units. It is calculated by excluding the unit under evaluation from the reference set when constructing the frontier. Super-efficiency values can exceed one, indicating that the unit is more efficient than the best practice in the dataset. Moreover, this paper adopts the non-orientation approach, which allows for comprehensive analysis without limiting the focus to input or output orientation. Non-oriented models do not adhere to a specific orientation. They aim to improve both inputs and outputs simultaneously instead. Furthermore, utilizing global frontiers offer advantages, enabling comparisons of performance across different regions throughout the sample period, regardless of group affiliation or environmental factors, thus contributing to a comprehensive analysis.
The model for measuring the digital economy efficiency is described as follows:
First, the global benchmark technology is constructed using the following equations:
| Pt(inputt)={(outputt)|inputtcanproduceoutputt}. | (1) |
| PG=P1∪P2∪⋯∪PT. | (2) |
In Eq (1), inputt represents the amount of input for each decision-making unit (DMU), outputt is the output produced by each DMU and Pt(inputt) represents the production possibility set at a specific time t=1,2,…,T. Equation (2) combines all contemporaneous benchmark technologies from the panel data of all inputs and outputs for relative DMUs. This ensures the consistency and comparability of digital economy efficiency across different data points.
Second, the linear programming problem is introduced to measure the digital economy efficiency under the assumption of constant returns to scale. The problem is formulated as follows:
| max1−β1+β{n∑j=1j≠kλjinputij+βinputik≤inputikn∑j=1j≠kλjoutputrj+βoutputrk≤outputrkλ≥0i=1,2,⋯,q;r=1,2,⋯,m;j=1,2,⋯,n | (3) |
where λj is a linear combination coefficient, n represents the number of DMUs and each DMUj has q inputs and m outputs, which can be denoted as inputij(i=1,2,⋯,q) and outputrj(r=1,2,⋯,m). The efficiency score, which is the optimal solution of Eq (3), can be deduced as follows:
To begin, Model (3) can be rephrased in an equivalent form:
| max1−β1+β{n∑j=1j≠kλjinputij+βinputik+s−i=inputikn∑j=1j≠kλjoutputrj+βoutputrk−s+r=outputrkλ≥0i=1,2,⋯,q;r=1,2,⋯,m;j=1,2,⋯,n. | (4) |
The reference benchmark can be understood as
| (input∗,output∗)=(∑nj=1j≠kλjinputij+βinputik+s−i,∑nj=1j≠kλjoutputrj+βoutputrk−s+r). |
The ratio of the input-output ratio of the evaluated DMU to the input-output ratio of the reference benchmark is given by:
| inputkoutputkinput∗output∗=outputkoutput∗inputkinput∗=outputk∑nj=1j≠kλjoutputrj+βoutputrk−s+rinputk∑nj=1j≠kλjinputij+βinputik+s−i=1−β1+β. | (5) |
To measure the digital economy efficiency under the assumption of variable returns to scale, Eq (6) is added to the Eq (3):
| n∑j=1j≠kλj=1. | (6) |
Moreover, the ratio of global super-efficiency between adjacent periods t and t−1 is the value of the Global Malmquist Productivity Index (MI) for period t. It can be represented as:
| MI(t,t−1)=etet−1, | (7) |
where et represents the global super-efficiency at period t and et−1 represents the global super-efficiency at period t−1. The Global Malmquist Productivity Index for period t, denoted as MI(t,t−1), indicates the ratio of efficiency between these two consecutive periods.
To assess the digital economy efficiency, it is necessary to identify variables that reflect its input and output. This study specifically focuses on two input variables: capital investment and labor investment, and one output variable: value added. Capital investment and labor investment represent the resources utilized in the production and delivery of digital products and services, while value added represents the value generated by these products and services. These variables offer a representative perspective of the digital economy as they encompass both the physical and human capital essential to its functioning, as well as the monetary value created by the digital economy. However, measuring input-output indicators of the digital economy presents several challenges due to its deep integration with the real economy, making data acquisition difficult. For instance, some digital products and services are intangible and non-market, such as online content and social media, posing challenges in measuring their value and output. Additionally, some digital products and services, such as sensors and software, are embedded and complementary, making it arduous to isolate their specific contributions from other factors and inputs. Furthermore, dynamic and innovative digital products and services like artificial intelligence and blockchain present difficulties in capturing their quality and impact accurately. To address these challenges, this study opts to focus on an industry that demonstrates a high degree of digitalization and provides a relatively clear boundary for measuring the efficiency.
The industry that aligns with the aforementioned criteria is the information transmission, software and information technology services industry. In China's latest industrial classification, this industry includes telecommunications, broadcasting and satellite transmission services, internet and related services, and software and information technology services. Although there are slight differences between the 2002 and 2011 editions of the classification, with the former including telecommunications and other information transmission services, computer services and software industries, they essentially represent the same industry. The decision to focus on data from this industry stems from several considerations. First of all, this industry serves as an excellent representative of the broader digital economy due to its inclusion of telecommunications, software, cloud computing and online platforms. It encompasses a wide range of digital products and services, making it a microcosm of the digital landscape. This industry operates both as a provider and as an enabler. As a provider, it produces and sells digital products and services, such as telecommunications, software, cloud computing and online platforms. These products and services have high value-added and innovation potential, capable of creating new markets and business models. As an enabler, this industry supports and facilitates other sectors of the economy, such as manufacturing, finance, education and healthcare. These sectors can use the digital products and services provided by this industry to improve their efficiency, quality and competitiveness. Second, the clarity of boundaries within this industry is a significant advantage. Data availability is often more reliable and consistent within a well-defined industry like information technology services. Considering these factors, the selected input-output indicators for measuring digital economy efficiency in this paper are the capital investment and labor investment within this industry as input indicators, along with the value added of this industry as the output indicator.
In this study, the capital input and labor input are measured by the total fixed asset investment of the entire society and the number of urban unit employees in the target industry, respectively. The total output of the digital economy is measured by the industry's added value. The rationale for selecting these indicators to proxy the variables is as follows: The total fixed asset investment of the entire society reflects both the physical and intangible capital invested in the digital economy, such as equipment, software, patents and trademarks. On the other hand, the number of urban unit employees in the target industry reflects the human capital involved in the digital economy. Lastly, the added value of the industry represents the net value created by the digital economy, which is derived from the difference between revenue from sales and the cost of intermediate inputs. To conduct the analysis of the digital economy's efficiency, relevant data from the industry covering the period from 2003 to 2020 is selected based on data availability. The data primarily originates from the National Bureau of Statistics, the statistics yearbooks of each province in China, and the Qianzhan Database. In cases where data is missing, it is primarily filled in using time series models and regression models.
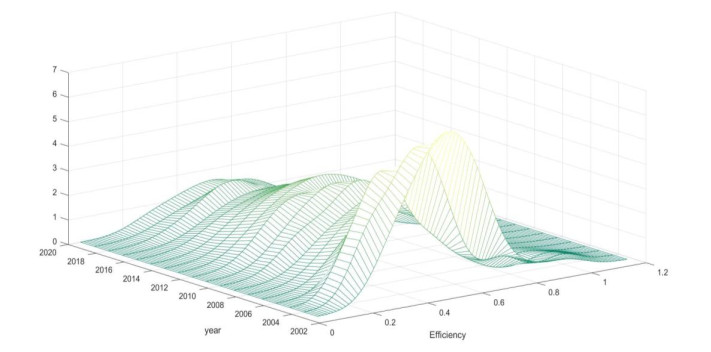
Figure 1 presents a 3D kernel density estimation diagram, which provides valuable insights into the distribution patterns and variations of digital economy efficiency across different spatial and temporal nodes. By incorporating both spatial and temporal elements, this diagram facilitates an understanding of how digital economy efficiency is dispersed among regions and how it evolves over time.
In summary, Figure 1 indicates a relatively stable and consistent level of efficiency within the digital economy, with some minor fluctuations and differentiation across sectors or regions. The peak values, consistently falling within the range of [0.4, 0.6], indicate persistent efficiency. Moreover, a slight leftward shift in the peak is observed during the years 2003–2005, suggesting a temporary decline in efficiency. However, from 2005 to 2020, the peak remains almost unchanged, indicating a period of stability in digital economy efficiency. Furthermore, the height of the peak consistently decreases over time. The most substantial decline occurs between 2003 and 2005, implying a significant alteration in the degree of agglomeration within the digital economy. This reduction during the aforementioned period highlights the dispersion of digital economy efficiency. However, from 2005 to 2020, there is no significant alteration in the height of the peak, suggesting a sustained level of dispersion and a stable degree of agglomeration. Additionally, the width of the wave crest narrows from 2003 to 2005, indicating a decrease in differentiation within the digital economy. This narrower width signifies a convergence of efficiency values during this period. However, after 2005, there is a slight expansion in width. Consequently, this implies a long-term stability in the differentiation of digital economy efficiency. Despite some minor changes in recent years, the overall pattern denotes a consistent level of differentiation, with certain sectors or regions exhibiting distinct levels of efficiency. Finally, the presence of a trailing right tail in the plot corroborates the existence of specific geographic regions that demonstrate exceptional performance in effectively operating within the digital economy.
Figure 2 presents the two-dimensional joint probability density of digital economy efficiency across different time spans. Joint probability density is a statistical measure that quantifies the likelihood of two variables occurring simultaneously. In this case, the variables of interest are the digital economy efficiency in 2016 and its efficiency in subsequent years. The base period was established as 2016 to calculate the joint probability density. Contour line and three-dimensional surface maps were then constructed to depict the joint probability density for 2016 and its corresponding time spans (k = 1, 2, 3, 4), representing 2017, 2018, 2019 and 2020.
Figure 2 reveals a strong stability in the digital economy efficiency across different time spans, with some provinces showing significant growth potential. The contour line maps consistently show the distribution of probability subjects along the positive 45° diagonal, reflecting maintenance of the original efficiency level over time. The slight increase in the position of the peak from 2016 to 2019, followed by a slight decrease in 2020, suggests a temporary deviation from the overall stable trend. However, the efficiency level remains relatively stable within the range of 0.5–0.6 throughout the entire period. Moreover, the peak height of the three-dimensional curved surface remains relatively consistent. The contour line map outlines also exhibit similarities across different time spans, further supporting the notion of stability in the distribution of digital economy efficiency. These findings indicate that regardless of the specific time span, the efficiency level tends to maintain its original level, providing a sense of consistency in the digital economy efficiency. However, the figure also highlights a decrease in the predictability of efficiency levels. The change from a value of 5 to 4 in the innermost ring of the contour line map in 2020 suggests a decline in the joint probability density between the digital economy efficiency and its subsequent time spans. This implies that the efficiency of the digital economy is becoming less stable or predictable over time. Additionally, as the time span increases, the inward convergence of the contour lines and the reduction in the area of the innermost ring indicate a diminishing joint probability density. This signifies a lower probability of simultaneous occurrences of digital economy efficiency and efficiency in subsequent time periods, resulting in reduced accuracy and reliability for future predictions. Therefore, caution should be exercised when making predictions with longer time spans due to the decreased probability density and inherent uncertainty. Despite the overall stability, a few provinces with the potential for significant growth are also identified. When the efficiency level in a province ek falls within the range of 0.4 to 0.6, there is a possibility that the efficiency level in subsequent time periods et+k may exceed 0.8. Although this possibility is relatively small, it suggests the presence of high growth potential in the digital economy efficiency of specific provinces. This indicates that certain provinces have the opportunity to experience substantial advancements in their digital economy efficiency, although it may be a relatively rare occurrence.
The analysis explores the contributions of technological change (TC) and efficiency change (EC) to the overall digital economy efficiency using the Malmquist Index, which is the ratio of global efficiency in the digital economy at two coherent temporal nodes and provides insights into efficiency change over time. The index is further broken into TC and EC components, allowing for a deeper understanding of the specific factors that drive changes in efficiency within the digital economy. The joint probability density is then calculated for representative years (2004, 2008, 2013 and 2020) and used to construct the plots in Figure 3.
Figure 3 displays shifting importance of TC and EC within the digital economy over time with provinces' varying performance. In 2004, the primary peak exhibits structural characteristics with TC>1 and EC<1, indicating that TC plays a pivotal role in driving TFP improvement within the digital economy. However, in 2008, 2013 and 2020, the primary peak shifts to TC<1 and EC>1, implying that the deceleration of technological progress becomes the main hindrance to TFP improvement. Furthermore, in 2013 and 2020, additional minor peaks are observed, situated above the main peak, suggesting that some provinces with lower efficiency change succeed in enhancing TFP through technological progress. The joint probability density of these minor peaks in 2020 is higher when compared to 2013, indicating a higher likelihood of similar occurrence. Additionally, in 2004, the main peak is positioned along the positive 45° diagonal, with the contour line nearly parallel to the y-axis and perpendicular to x=0.9. This positioning signifies that TFP within the digital economy responded to changes in technological progress, while the contribution of technical efficiency to TFP is relatively limited. Moreover, in 2008, the probability subjects are distributed along the positive 45° diagonal, which suggests a relatively balanced contribution between technical progress and technical efficiency towards TFP within the digital economy. The impact of both factors on TFP appears to be approximately symmetrical. However, in 2013 and 2020, there is a notable shift, with probability subjects exhibiting a tendency to be distributed along the opposite 45° diagonal. This indicates a significant change in the relationship between TFP, technical efficiency and technological progress within the digital economy.
To explore the factors influencing efficiency change in the digital economy, EC is further decomposed into two components: pure efficiency change (PEC) and scale effects in efficiency change (SEC). Figure 4 presents plots to visualize the joint distribution of PEC and SEC for representative years (2003, 2008, 2013 and 2020).
The dominant factors influencing technological efficiency change in the digital economy have exhibited variability from 2003 to 2020, with a contrasting pattern observed among certain provinces. In 2004 and 2008, the main peak's position demonstrates structural characteristics with SEC≈1 and PEC≈1, indicating a roughly symmetrical impact of pure technical efficiency and scale effects on technological efficiency changes. However, in 2013 and 2020, the main peak's position exhibits structural characteristics with SEC≈1 and PEC>1, highlighting the significance of pure technological efficiency as the core driver for technological efficiency improvement in the digital economy. In 2013, two smaller peaks emerge, situated above and below the main peak. The upper peak shows structural characteristics with SEC>1 and PEC<1, suggesting that for certain provinces with lower pure technical efficiency, the increase in scale effects plays a crucial role in driving improvement in technological efficiency within the digital economy. Conversely, the lower peak exhibits structural characteristics with SEC<1 and PEC>1, indicating that for some provinces with lower scale efficiency and pure technological efficiency improvement are the main driving force behind the enhancement of technological efficiency in the digital economy. Furthermore, in 2004, the main peak aligns with the positive 45° diagonal, and the contour line is nearly parallel to the y-axis, perpendicular to x=0.9. This indicates that changes in the digital economy's technical efficiency are influenced by variations in scale effects, while the contribution of pure technical efficiency to overall total factor productivity is relatively small. Moreover, in 2008, 2013 and 2020, the subjects' probability distribution shows a noticeable shift towards the reverse 45° diagonal, signaling a significant change in the relationship between technological efficiency, pure technological efficiency and scale efficiency within the digital economy. This highlights the evolving dynamics and interplay of these factors in shaping technological efficiency outcomes.
This section examines the temporal characteristics of digital economy efficiency, specifically focusing on the central tendency and discrete trend of data distribution. Figure 5 presents the average values of digital economy efficiency in China's 31 provinces for each year, enabling the examination of the central tendency of the data over time.
The annual average of digital economy efficiency has exhibited a consistent upward trend, as evidenced by the plotted data. Several significant observations can be noted regarding the trend and characteristics of the digital economy efficiency. Despite a noticeable dip in efficiency in 2012, there has been a general improvement in digital economy efficiency over the years. The global efficiency for the digital economy between 2003 and 2020 falls within the range of 0.1 and 0.6. Analyzing the growth rate, it is observed that the rate remains relatively stable over the years. The slope of the efficiency curve from 2003 to 2012 bears resemblance to that observed from 2013 to 2020. This suggests that the rate of improvement in digital economy efficiency has been consistent over time, without any significant fluctuations. This stability of the growth rate indicates a sustained and gradual enhancement in the annual average of digital economy efficiency in provincial regions.
The decline in digital economy efficiency during 2012-2013 can be attributed to a combination of economic and technological factors. First, the global economic context during that period played a role as it was characterized by economic uncertainties and slowdowns in various parts of the world, exemplified by the European debt crisis. During this period, reduced consumer spending led to decreased demand for digital products and services. Investment constraints made venture capital and private equity firms more cautious, limiting funding for digital startups. A study conducted by the European Commission titled "The 2013 EU Industrial R&D Investment Scoreboard" tracked the research and development (R&D) spending of the top 2000 companies worldwide and confirmed that "the global economic downturn in 2012-2013 had a negative impact on R&D investment, especially in sectors such as ICT, consumer electronics and software, which are more dependent on venture capital and private equity funding".3 Moreover, the rapid increase in data volume during this period, from TB to PB, EB and even ZB levels, could have posed technological challenges. Scaling up the necessary infrastructure to manage such massive volumes of data proved to be a complex and time-consuming process, temporarily affecting the efficiency [37].
3 This report can be downloaded at this website: https://iri.jrc.ec.europa.eu/scoreboard/2013-eu-industrial-rd-scoreboard.
In Figure 6, the analysis shifts to the discrete trend of the digital economy efficiency by examining the standard error value. We calculate the annual standard error value of the digital economy efficiency for each year. These standard error values are then presented in the time series chart (Figure 6) to investigate the discrete trend of the data over time.
The differentiation trend of digital economy efficiency among different provinces in China has been intensifying, as evidenced by the increasing standard deviation over time. The standard deviation, a measure of the dispersion or spread of data points around the average value, reflects the degree of disparity in digital economy efficiency among provinces in China. The current upward trend in standard deviation indicates a widening variation in digital economy efficiency across provinces. Although there is a slight decrease in standard deviation in 2012, the overall trend remains consistently upward, suggesting a continuous increase in the differentiation of digital economy efficiency among provinces with some fluctuations along the way. The range of standard deviation values, ranging from 0.05 to 0.26, signifies the significant variability in digital economy efficiency across provinces. This range indicates a diverse performance among provinces regarding their ability to leverage digital technologies for economic growth. Moreover, the rate of standard deviation increase provides noteworthy insights into the dynamics of this trend. From 2003 to 2012, the rate of standard deviation increase remains relatively stable, suggesting a consistent pace of differentiation in digital economy efficiency among provinces. However, since 2013, there has been a significant acceleration in the rate of standard deviation increase, indicating a more pronounced differentiation trend in recent years and a sharper divide in digital economy efficiency among provinces.
The widening disparities in digital economy efficiency among Chinese provinces reveal a multifaceted challenge, despite the digital economy's reputation for reduced geographical constraints compared to the traditional economy. These disparities result from several critical factors. First, there are significant variations in digital infrastructure and high-speed internet access, granting competitive advantages to provinces with advanced infrastructure, while those lacking these elements face difficulties in keeping pace [38,39]. For example, the number of domain names in 2021, collected by the National Bureau of Statistics, shows a stark contrast, with Beijing having 566.01 million domain names and Tibet having only 1.34 million. Second, the skills and education of the local workforce also play a significant role [40]. Research underscores the role of human capital, particularly skilled information workers, as a driving force behind digital economy development, yet disparities in workforce quality and expertise exist among regions. Data from the National Bureau of Statistics further supports this contention. Lastly, a province's overall economic strength significantly influences digital economy efficiency, as the digital economy is tightly intertwined with the traditional economy and available data resources [40,41]. Regions with higher economic development levels tend to attract more investments and cultivate robust tech ecosystems. In conclusion, addressing the widening gap in digital economy efficiency necessitates a comprehensive approach considering these factors, in order to foster more equitable growth among Chinese provinces.
To illustrate the dynamics of efficiency change over time, Figure 7 is presented. MI represents the ratio of global super-efficiency between adjacent periods, offering key insights into the speed and direction of efficiency evolution. MI is broken into its essential elements, facilitating an understanding of the disparate influences of efficiency change and technological progress on the MI. This detailed perspective enables the identification of the primary drivers of MI variations over time, whether they stem from improvements or declines in efficiency or from shifts in technical progress.
As depicted in Figure 7, the digital economy exhibits volatility while showcasing an overall positive increase in efficiency, primarily driven by advancements in technological progress. It demonstrates the fluctuations of digital economy efficiency and the changes in its decompositions. Examining the improvement rate of digital economy efficiency, it fluctuates between 0.8 and 1.2, with the lowest rate observed in 2013 and the highest rate in 2005. A significant decline in efficiency growth is observed between 2010 and 2012, signifying a notable decrease in the rate of improvement during those years. Conversely, from 2016 to 2020, the decline rate stabilizes, suggesting a relatively consistent performance in digital economy efficiency during that timeframe. It is crucial to note that, despite the presence of decline, the growth rate values consistently remain above 1, except for 2013. This finding indicates an overall positive trend in digital economy efficiency, despite the presence of fluctuations. Moving on to the decomposition analysis of digital economy efficiency changes, the growth rate of technological progress is considered. This rate fluctuates between 0.3 and 1.7, with the lowest value observed in 2013 and the highest in 2005. Notably, a substantial decline is seen in 2012, followed by a rebound in 2013. Throughout the sample period, the rate of change in technological progress consistently remains above 1, indicating an overall upward trend. Similarly, the growth rate of technological efficiency fluctuates between 0.9 and 1.2, with the lowest and highest values occurring in 2004 and 2013, respectively. In the early stages, the growth rate of technological efficiency exhibits more fluctuations, suggesting potential challenges in utilizing technology effectively. However, as the sample period progresses, these fluctuations decrease, and the growth rate stabilizes around 1. This suggests a gradual improvement in the digital economy's ability to leverage digital resources efficiently. Lastly, the growth rate of scale efficiency demonstrates relatively smaller fluctuations within the range of 0.9 to 1.1. This indicates a more consistent performance of scale efficiency compared to technological progress and efficiency. Throughout the sample period, although slight fluctuations are observed, scale efficiency remains relatively stable.
Figure 8 is created for assessing the degree and direction of changes in MI and its decomposition. This time series plot shows the annual standard deviation of MI and its decomposition, providing a visual representation of the difference in change rates over time. By examining the patterns and trends depicted in Figure 8, valuable insights can be obtained regarding the stability, consistency and underlying factors influencing the difference in change rates.
Figure 8 shows a trend of narrowing interprovincial differences in China's digital economy efficiency growth rate from 2003 to 2020, which is primarily driven by the interprovincial difference of technical efficiency growth rate. Throughout this period, the annual standard deviation of China's digital economy efficiency growth rate ranges from 0.1 to 0.45, with the maximum deviation occurring in 2020 and the minimum in 2014. It indicates the presence of disparities in the rates of improvement in digital economy efficiency among provinces, with a notable degree of differentiation. The slope of the standard deviation for the digital economy efficiency improvement rate exhibits the highest value during the 2009–2012 period, with smaller values in other periods. The disparities in efficiency improvement rates among provinces stabilize initially, then fluctuate, and eventually stabilize again. By comparing the initial and final values of the standard deviation, there is a decrease in the degree of differentiation in digital economy efficiency improvement rates among the provinces. The next observation is focused on the line chart depicting the time series of the standard deviation in the decomposition of the growth rate of digital economic efficiency. The standard deviation range for the growth rates is as follows: [0.05, 0.4] for technical efficiency growth rate, [0.05, 0.35] for scale efficiency growth rate and [0.05, 0.15] for technological progress growth rate. The growth rate of technological efficiency is identified as the primary source of differentiation in the growth rate of digital economic efficiency, while the growth rate of scale efficiency influences the differentiation in the growth rate of technical efficiency. The standard deviation of the growth rate of the scale effect experiences frequent changes from 2004 to 2011 but exhibits relatively small and stable slopes in other periods. Technological progress demonstrates minimal slope changes and remains relatively stable. Significant changes and fluctuations in interprovincial differences in the growth rate of technical efficiency are observed during the specific timeframe of 2009 to 2012, while relatively smaller slopes are evident in other periods. These findings indicate significant periodic fluctuations in interprovincial differences in the growth rate of technological efficiency, a gradually decreasing and stable trend in the interprovincial differences in the growth rate of scale efficiency and relatively stable interprovincial differences in the growth rate of technological progress over time.
In this section, charts are used to visually analyze the spatial characteristics of digital economy efficiency in different provinces of China from 2003 to 2020. First, the article offers an overall description of the spatial patterns of digital economy efficiency across the provinces during the specified time period. The average digital economy efficiency of each province is calculated and mapped onto a map shown in Figure 9.
China's digital economy efficiency has room for growth, demonstrating a decreasing trend from east to west. The majority of provinces tend to have average digital economy efficiency levels within the range of 0.2 to 0.4. However, some provinces show lower average levels ranging from 0 to 0.2, while others exhibit higher levels ranging from 0.4 to 0.6. This disparity in digital economy efficiency indicates notable inter-provincial differences. Additionally, the gradual lightening of red hues from east to west further emphasizes that the eastern regions have higher digital economy efficiency, the central regions exhibit intermediate efficiency levels and the western regions tend to have lower efficiency levels. The spatial pattern of digital economy efficiency in China exhibits a strong correlation with the level of economic development. The observed relationship suggests that regions with higher economic development tend to demonstrate greater digital economy efficiency. This connection can be attributed to several factors that influence the adoption and integration of digital technologies, such as advanced infrastructure, robust communication networks and the availability of skilled workforce. In the eastern regions, which have historically experienced more rapid economic growth and urbanization, the higher digital economy efficiency can be attributed to the presence of established technological hubs, strong government support and a concentration of innovative enterprises. Conversely, the western regions, characterized by lower levels of economic development and a relatively dispersed population, face various challenges in embracing digital transformation. These challenges include limited access to digital infrastructure, inadequate investment in technology, and a lack of sufficient human capital.
However, it is crucial to recognize that underdeveloped regions are not necessarily associated with low digital economy efficiency. A noteworthy illustration of this phenomenon can be observed in Hainan and Yunnan provinces with the average level of their digital economy efficiency ranging from 0.4 to 0.6. Their performance serves as compelling evidence that underdeveloped regions can achieve high levels of digital economy efficiency. Several factors contribute to the higher digital economic efficiency in Hainan and Yunnan. One of them is the proactive approach taken by local governments to promote digitalization and technology adoption. For example, Yunan has implemented the "Digital Yunnan" plan, a three-year action plan for promoting the development of new infrastructure and a guideline on fostering the integration of the digital economy and the real economy. Similarly, Hainan has prioritized initiatives to promote digital connectivity and technology innovation. The Hainan Free Trade Port Master Plan and the Hainan Digital Economy Development Plan serve as key guiding frameworks. Additionally, Hainan and Yunnan have capitalized on their distinctive strengths and resources to develop the digital economy. For instance, Hainan's designation as a pilot free trade zone and a special economic zone has attracted significant investments and facilitated cross-border trade, propelling its digital economy growth. According to China Briefing, Hainan has received over $15.4 billion in foreign investment in 2020, an increase of 190% year-on-year. In contrast, Yunnan's unique geographical location has made it an important hub for digital connectivity and trade, creating opportunities for digital innovation and entrepreneurship. As reported by Xinhua New Agency, Yunnan has built over 20 cross-border e-commerce platforms and 11 international data channels, covering 14 countries and regions along the Belt and Road Initiative.
Additionally, this paper investigates the spatial evolution of digital economy efficiency in China's provinces from 2003 to 2020. Due to space limitations, the paper presents a hierarchical map showing the digital economy efficiency data for 31 provinces in China during specific years: 2003, 2008, 2013 and 2020 (Figure 10). These timepoints allow observation and comparison of efficiency changes over time and demonstrate efficiency levels during significant stages of digital economy development.
Figure 10 indicates that a consistent increase in China's digital economy efficiency, while also revealing noticeable variations among provinces. The figure provides a visual representation of three main characteristics related to the evolution of efficiency. When considering numerical classification, China's overall level of digital economic efficiency has improved over time. In the 2001 map, 28 provinces have a digital economic efficiency between 0 and 0.2, while only Tianjin, Zhejiang and Shanghai have an efficiency between 0.2 and 0.4. By 2008, the number of provinces within 0~0.2, 0.2~0.4 and 0.4~0.6 efficiency ranges are 10, 17 and 4, respectively. In 2013, these number shift to 6, 19 and 6 provinces, respectively. In the 2020 map, the respective numbers of provinces fall within the efficiency ranges of 0~0.2, 0.2~0.4 and 0.4~0.6 are 3, 6 and 11, respectively, while 11 provinces have above 0.6 in efficiency. These changes demonstrate the continuous improvement of China's digital economic efficiency, where fewer provinces fall into lower efficiency ranges over time, while more provinces achieve medium to higher efficiency levels. Notably, the 2020 map showcases a significant number of provinces that have reached a higher level of digital economy efficiency. The expansion of the digital economy efficiency range indicates a transition from relative equilibrium to increasing interprovincial differences. The distribution of color blocks on the map reflects significant changes in the spatial pattern of China's digital economy efficiency. In the 2001 map, provinces exhibited consistent color depth, indicating a relatively balanced spatial pattern. However, the 2008 and 2013 maps reveal deeper colors in the eastern region compared to the western region, suggesting higher digital economic efficiency in the east. This pattern continued in the 2020 map, with the eastern region still exhibiting darker colors, but with more variations in shades.
In Section 5.2, this study examines the spatial evolution characteristics of China's digital economy efficiency by analyzing the changes in efficiency from 2003 to 2020. First, an overall description of the spatial characteristics of the digital economy MI in China's 31 provinces during this period is provided. This study calculates the average digital economy MI for each province, creates corresponding data sequences and presents them on maps. Moreover, considering an MI value exceeding 1 as an indication of increased efficiency, the article identifies instances where the MI value of each province surpassed 1, and visualizes them on the map layer (Figure 11).
The digital economy efficiency in China displays regional variations in terms of improvement. The color-coded map in Figure 11 presents two layers of information: the average increase rate of digital economic efficiency and the frequency of digital economic efficiency increases. The figure displays blue color blocks for the MI mean, indicating that the average level of digital economy MI in different provinces of China is equal to or greater than 1. Within this range, 17 provinces fall within the 1~1.1 range, while 14 provinces have MI values exceeding 1.1. This suggests that slightly over half of the provinces tend to have digital economic efficiency fluctuating around the original level, while approximately half of the provinces exhibit improvements at the original level. Examining the number of times the MI value exceeds 1 reveals three categories of colored points on the map, representing different frequency ranges. Provinces falling within the 5~10 range, including Anhui, Heilongjiang and Xinjiang, exhibit a relatively lower frequency of digital economy efficiency increases. This implies that these provinces experience fewer instances of improvements in their digital economy efficiency over the observed time period. On the other hand, a higher growth frequency, ranging from 10 to 15, is observed in 27 provinces, indicating a more pronounced trend of digital economy efficiency improvements. Notably, Beijing stands out as the only province with a growth rate falling between 15 and 20, suggesting a particularly high frequency of efficiency increases in the capital city.
Furthermore, the paper provides a hierarchical map displaying the mean MI data of the digital economy in China's 31 provinces for the years 2003, 2008, 2013 and 2020 (Figure 12). This hierarchical map serves as a supplementary analysis, specifically focusing on the spatial characteristics of digital economy efficiency across different provinces of China.
The spatial distribution pattern has changed over time, but the digital economy growth rate in Chinese provinces has demonstrated stability and convergence. According to Figure 12, the data maps illustrate the digital economy growth rate in different provinces over the years. In 2004, the map displays six color regions ranging from 0.4 to 2.0, with a higher concentration of dark blue regions indicating that most provinces experienced a digital economy growth rate within the range of 1.0 to 1.2. Moving to the 2008 data map, there are four color regions ranging from 0.8 to 1.6, with a notable presence of dark blue and light orange areas, suggesting that the digital economy growth rate of provinces is primarily within the range of 1.0 to 1.4. The 2013 data map reveals four color regions ranging from 0.4 to 1.2, predominantly composed of baby blue and dark green blocks. This implies that the digital economy growth rate of provinces during this period mainly fell within the range of 0.6 to 1.0. Finally, the 2020 data map reveals three color regions ranging from 0.8 to 1.4, with baby blue and dark blue as the predominant colors. This suggests that the level of digital economic growth rate in provinces during this time is mainly within the range of 0.8 to 1.2. Comparing the numerical levels of the digital economy growth rate across these different years, a decreasing trend in the highest level of digital economy growth rate can be observed. This indicates a potential increase in stability of the digital economy growth rate over time. Additionally, examining the range of values and the number of color regions representing the growth rate reveals a narrowing of differences in the digital economy growth rate among provinces.
In this paper, two major problems for evaluating the digital economy are addressed. The first one relates to primarily focusing on the size and overall performance, while neglecting the development quality of digital economy. To overcome this, this paper conducts a measurement of digital economy efficiency. The second gap is on the incomparability of efficiency in different periods and regions, for which the two-step approach is a commonly used method. To address this gap, the global super-efficiency model is employed as an alternative to commonly used two-step approaches. This study's main focus is on focusing on exploring the characteristics of digital economy efficiency in 31 Chinese provinces spanning from 2003 to 2020.
The findings indicate a consistent increasing trend in digital economy efficiency, largely driven by technological progress. This highlights the importance of prioritizing investments in research and development, digital infrastructure and fostering a conducive environment for innovation to enhance the quality and productivity of the digital economy. Strategic investments are warranted to fortify the digital infrastructure, including the expansion of broadband networks and the reinforcement of data centers, ensuring the pervasive access to high-speed internet and data processing capabilities. Simultaneously, the development and formulation of educational programs that emphasize science, technology, engineering and mathematics (STEM) skills are imperative.
Additionally, the study reveals a growing divergence among provinces, accentuating the need for specific interventions by governmental authorities, enterprises and regional development frameworks. The government should prioritize resource allocation for the augmentation of digital infrastructure in provinces that are trailing behind, ensuring widespread accessibility to high-speed internet and essential digital resources, even in remote locales. The implementation of initiatives designed to expedite the exchange of technological expertise and knowledge from provinces with robust digital economies to those encountering challenges is also essential.
Finally, there is a decreasing trend of digital economy efficiency from the east to the west, which is closely related to the specific economic development levels of each province. It's worth noting that not all undeveloped provinces inherently have low digital economy efficiency. Hence, comprehensive assessment of each province is imperative, offering crucial insights into the precise factors influencing the digital economy efficiency. A strategic approach is necessary to strengthen and empower regional development agencies in the western provinces, enabling them to discern and tackle the unique challenges and opportunities in digital economy development. Subsequently, tailored policies can be used to address the specific challenges and opportunities present in each region.
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.
| [1] |
J. Rickard, Morita theory for derived categories, J. London Math. Soc., s2-39 (1989), 436–456. https://doi.org/10.1112/jlms/s2-39.3.436 doi: 10.1112/jlms/s2-39.3.436
|
| [2] |
I. Assem, N. Marmaridis, Tilting modules over split-by-nilpotent extensions, Commun. Algebra, 26 (1998), 1547–1555. https://doi.org/10.1080/00927879808826219 doi: 10.1080/00927879808826219
|
| [3] |
J. Miyachi, Tilting modules over trivial extensions of Artin algebras, Commun. Algebra, 13 (1985), 1319–1326. https://doi.org/10.1080/00927878508823223 doi: 10.1080/00927878508823223
|
| [4] |
J. Miyachi, Extensions of rings and tilting complexes, J. Pure Appl. Algebra, 105 (1995), 183–194. https://doi.org/10.1016/0022-4049(94)00145-6 doi: 10.1016/0022-4049(94)00145-6
|
| [5] |
J. Rickard, Lifting theorems for tilting complexes, J. Algebra, 142 (1991), 383–393. https://doi.org/10.1016/0021-8693(91)90313-W doi: 10.1016/0021-8693(91)90313-W
|
| [6] |
H. Tachikawa, T. Wakamatsu, Extensions of tilting functors and QF-3 algebras, J. Algebra, 103 (1986), 662–676. https://doi.org/10.1016/0021-8693(86)90159-6 doi: 10.1016/0021-8693(86)90159-6
|
| [7] |
J. Rickard, Derived equivalences as derived functors, J. London Math. Soc., s2-43 (1991), 37–48. https://doi.org/10.1112/jlms/s2-43.1.37 doi: 10.1112/jlms/s2-43.1.37
|
| [8] |
A. Marcus, Equivalences induced by graded bimodules, Commun. Algebra, 26 (1998), 713–731. https://doi.org/10.1080/00927879808826159 doi: 10.1080/00927879808826159
|
| [9] |
S. Caenepeel, S. Crivei, A. Marcus, M. Takeuchi, Morita equivalences induced by bimodules over Hopf-Galois extensions, J. Algebra, 314 (2007), 267–302. https://doi.org/10.1016/j.jalgebra.2007.02.033 doi: 10.1016/j.jalgebra.2007.02.033
|
| [10] | P. Deligne, A. A. Beĭlinson, J. Bernstein, Faisceaux pervers, Astérisque, 1983. |
| [11] |
E. Cline, B. Parshall, L. Scott, Finite-dimensional algebras and highest weight categories, J. Reine Angew. Math., 391 (1988), 85–99. https://doi.org/10.1515/crll.1988.391.85 doi: 10.1515/crll.1988.391.85
|
| [12] |
Y. Han, Recollements and Hochschild theory, J. Algebra, 397 (2014), 535–547. https://doi.org/10.1016/j.jalgebra.2013.09.018 doi: 10.1016/j.jalgebra.2013.09.018
|
| [13] | S. Montgomery, Hopf algebras and their actions on rings, In: CBMS regional conference series in mathematics, American Mathematical Society, 1993. https://doi.org/10.1090/cbms/082 |
| [14] | S. König, A. Zimmermann, Derived equivalences for group rings, In: Lecture notes in mathematics, Berlin: Springer-Verlag, 1998. https://doi.org/10.1007/BFb0096366 |
| [15] |
H. Abe, M. Hoshino, Derived equivalences for triangular matrix rings, Algebr. Represent. Theor., 13 (2010), 61–67. https://doi.org/10.1007/s10468-008-9098-1 doi: 10.1007/s10468-008-9098-1
|
| [16] |
M. Barot, H. Lenzing, One-point extensions and derived equivalence, J. Algebra, 264 (2003), 1–5. https://doi.org/10.1016/S0021-8693(03)00124-8 doi: 10.1016/S0021-8693(03)00124-8
|
| [17] |
H. Schneider, Representation theory of Hopf Galois extensions, Israel J. Math., 72 (1990), 196–231. https://doi.org/10.1007/BF02764620 doi: 10.1007/BF02764620
|
| [18] |
E. C. Dade, Group-graded rings and modules, Math. Z., 174 (1980), 241–262. https://doi.org/10.1007/BF01161413 doi: 10.1007/BF01161413
|
| [19] |
F. V. Oystaeyen, Y. Zhang, H-module endomorphism rings, J. Pure Appl. Algebra, 102 (1995), 207–219. https://doi.org/10.1016/0022-4049(94)00076-U doi: 10.1016/0022-4049(94)00076-U
|
| [20] | D. Happel, Triangulated categories in the representation theory of finite-dimensional algebras, Cambridge University Press, 1988. https://doi.org/10.1017/CBO9780511629228 |
| [21] |
Y. Zhang, Hopf Frobenius extensions of algebras, Commun. Algebra, 20 (1992), 1907–1915. https://doi.org/10.1080/00927879208824439 doi: 10.1080/00927879208824439
|
| [22] |
Y. Qin, Y. Han, Reducing homological conjectures by n-recollements, Algebr. Represent. Theor., 19 (2016), 377–395. https://doi.org/10.1007/s10468-015-9578-z doi: 10.1007/s10468-015-9578-z
|
| [23] |
M. van den Bergh, A relation between Hochschild homology and cohomology for Gorenstein rings, Proc. Amer. Math. Soc., 126 (1998), 1345–1348. https://doi.org/10.1090/S0002-9939-98-04210-5 doi: 10.1090/S0002-9939-98-04210-5
|
| 1. | Ding Ding, Zhengxu Cao, Bei Ma, Spatio-temporal evolution and driving mechanism of the dual transformation and coordination between digitalization and greening, 2024, 371, 03014797, 123110, 10.1016/j.jenvman.2024.123110 | |
| 2. | Fengyu Zhao, Ziqing Xu, Xiaowen Xie, Exploring the Role of Digital Economy in Enhanced Green Productivity in China’s Manufacturing Sector: Fresh Evidence for Achieving Sustainable Development Goals, 2024, 16, 2071-1050, 4314, 10.3390/su16104314 | |
| 3. | Jinhong Wang, Yanting Xu, Factors influencing the transition of China's economic growth momentum, 2024, 6, 2689-3010, 220, 10.3934/NAR.2024010 | |
| 4. | Xiang Li, Shujing Yue, Does the Government Digital Attention Improve China's Digital Economy Output Efficiency: Accelerator or Inhibitor, 2024, 03135926, 10.1016/j.eap.2024.12.020 | |
| 5. | Jingkun Zhang, Wang Zhang, Harnessing Digital Technologies for Rural Industrial Integration: A Pathway to Sustainable Growth, 2024, 12, 2079-8954, 564, 10.3390/systems12120564 |
Jinlei Dong, Fang Li, Longgang Sun. Derived equivalence, recollements under H-Galois extensions[J]. AIMS Mathematics, 2023, 8(2): 3210-3225. doi: 10.3934/math.2023165
DownLoad:
