On the mixed solution of reduced biquaternion matrix equation $\sum\limits_{i = 1}^nA_iX_iB_i = E$ with sub-matrix constraints and its application

1.   Introduction

Linear matrix equations play an important role in many fields. Many researchers turn their attention to the solution of real or complex linear matrix equations^[1,2,3,4]. Since W. R. Hamilton proposed quaternion and applied it to various aspects of physics, many models based on quaternion matrix equations have emerged. Meanwhile, with the application of quaternion and quaternion matrix equations in many fields such as the stability theory, cybernetics, quantum mechanics and color images^{[5,6,7,8,9,10,11]}, the related theory research has become more meaningful ^{[12,13,14,15,16,17,18]}. However, the non-commutativeity of quaternion multiplication will make it difficult to implement in many fields.

Reduced biquaternion with product commutability was proposed by Schtte and Wenzel^[19] in 1990, which is represented as

where ${{\bf i}}^2 = {{\bf k}}^2 = -1, \ {{\bf j}}^2 = 1$, ${{\bf i}}{{\bf j}} = {{\bf j}}{{\bf i}} = {{\bf k}}, \ {{\bf j}}{{\bf k}} = {{\bf k}}{{\bf j}} = {{\bf i}}, \ {{\bf k}}{{\bf i}} = {{\bf i}}{{\bf k}} = -{{\bf j}}$ and $a_r, a_i, a_j, a_k\in \mathbb{R}$. A reduced biquaternion matrix $A\in\mathbb{H}_{R}^{m\times n}$ can be expressed as

where $A_r, \ A_i, \ A_j, \ A_k$ are real matrices, $A_1 = A_r+ A_i{{\bf i}}, \ A_2 = A_j + A_k{{\bf i}}$ and the Frobenius norm of $A$ is defined as

As soon as reduced biquaternion was proposed, it was applied in a digital filter. Ueda and Takahashi^[20] proved in 1993 that the first-order digital filter with reduced biquaternion coefficient can realize any real coefficients digital filter less than order four. With the in-depth study of reduced biquaternion, Pei et al.^[21,22] studied Fourier transform, the eigenvalue and the singular value decomposition of reduced biquaternion matrix, respectively, which were used in signal and image processing. In addition, the study of reduced biquaternion matrix equations has been a hot topic in recent years. Yuan et al.^[23] solved the Hermitian solution of the reduced biquaternion equation $(AXB, CXD) = (E, G)$ using the complex representation method, which can transform the problem in reduced biquaternions into complex number fields. Hidayet Hüda Kösal^[24] solved several special least squares solutions of the reduced biquaternion matrix equation $AX = B$ by using the $e_1-e_2$ representation, and successfully applied the least squares pure imaginary solutions to color image restoration. Chen et al.^[25] presented the general solution and necessary and sufficient conditions for the existence of an $\eta$-(anti) Hermitian solution to a constrained Sylvester-type generalized communicative quaternion matrix equation. From above we can see that the study of the matrix equation on reduced biquaternion is a very meaningful work. In this paper, we will study the reduced biquaternion matrix equation with sub-matrix constraints.

The sub-matrix constraint problems were originally from a practical subsystem expansion problem. Thus, researchers have great interest in studying such problems under different sub-matrix constraints. For example, Gong et al.^[26] discussed an anti-symmetric solution of $AXA^T = B$ for $X$ with a leading principal sub-matrix constraint. Zhao et al.^[27] gave some necessary and sufficient conditions for the solvability of the matrix equation $AX = B$ with bisymmetrical central principal sub-matrix constraint. Li et al.^[28] proposed an efficient algorithm to study the symmetric solution of matrix equation $AXB+CYD = E$ with a special sub-matrix constraint. However, as far as we know, the sub-matrix problem for the reduced biquaternion matrix equation

has not been considered yet. In this paper, we will discuss the mixed solution of (1.1) with sub-matrix constraints.

Definition 1.1. If $n-q$ is even, $A = (a_{ij})\in\mathbb{H}_{R}^{n\times n}$, and

then $A_c(q)$ is called a $q$-order central principal matrix of $A$. Clearly, $A$ has only even order central principal matrices when $n$ is even, and odd central principal matrices when $n$ is odd.

Definition 1.2. Suppose $A = (a_{ij})\in\mathbb{H}_R^{n\times n}$.

1) The matrix $A$ is Hermitian if $a_{ij} = \overline{a_{ji}}$, the set of reduced biquaternion Hermition matrices is denoted by $\mathbb{SH}_R^{n\times n}$.

2) The matrix $A$ is Centro-symmetric if $a_{ij} = a_{n-i+1, n-j+1}$, the set of reduced biquaternion centro-symmetric matrices is denoted by $\mathbb{CH}_R^{n\times n}$.

3) The matrix $A$ is Bi-hermitian if $a_{ij} = a_{n-i+1, n-j+1} = \overline{a_{ji}}$, the set of reduced biquaternion Bi-hermitian matrices is denoted by $\mathbb{BH}_R^{n\times n}$.

For a given set of matrices $\left\{X_{t_i}\right\}$, $\left(i = 1, 2, \cdots, n\right)$, where $X_{t_i}\in\mathbb{SH}_R^{t_i\times t_i}$, $i = 1, 2, \cdots, s$; $X_{t_i}\in\mathbb{CH}_R^{t_i\times t_i}$, $i = s+1, s+2, \cdots, m$; $X_{t_i}\in\mathbb{BH}_{R}^{t_i\times t_i}$, $i = m+1, m+2, \cdots, n$. Suppose

Problem 1. Given $A_i\in \mathbb{H}_R^{m\times n}$, $B_i\in \mathbb{H}_R^{n\times q}$, $E\in\mathbb{H}_R^{m\times q}$, and $\left\{X_{t_i}\right\}, \ (i = 1, 2, \cdots, n)$, find a matrix group $\left(X_1, X_2, \cdots, X_n\right)$ satisfying

and denoted the set of such matrix group

where, $X_i\in\theta_1, \ i = 1, 2, \cdots, s$, $X_t\in\theta_2, \ t = s+1, s+2, \cdots, m$, $X_k\in\theta_3, \ k = m+1, m+2, \cdots, n$. Find out $\left(X^Q_1, X^Q_2, \cdots, X^Q_n\right)\in S_Q$ such that

$\left(X^Q_1, X^Q_2, \cdots, X^Q_n\right)$ is called the minimal norm least squares mixed solution of (1.1). If $\min = 0$, $\left(X^Q_1, X^Q_2, \cdots, X^Q_n\right)$ is called the minimal norm mixed solution of (1.1).

Our main tool is the semi-tensor product (STP) of matrices, which is a generalization of conventional matrix product. With the help of the STP of matrices, many meaningful problems have been resolved and scholars have obtained many constructive results^{[29,30,31,32]}. Recently, STP has been applied to the study of the matrix equation^[33,34,35]. However, the limitation of the expanded dimension leads to high computational complexity. This paper aims at providing an improved method based on STP to reduce the computational complexity, as well as extend this method to solve reduced biquaternion matrix equations.

The main contributions of this paper include: (ⅰ) The algebraic expression of isomorphism relation between complex matrix and reduced biquaternion matrix is defined by using STP, which is called $\mathcal{L_C}$-representation of reduced biquaternion matrix. At the same time, the necessary and sufficient conditions for computable algebraic expressions are given by using the structure matrix of reduced biquaternion product; (ⅱ) A new method to reduce the number of variables of unknown reduced biquaternion matrix with special structure is proposed, which is called $\mathcal{GH}$-representation. Relative to the $\mathcal{H}$-representation method, the $\mathcal{GH}$-representation method proposed in this paper is suitable for more special matrix forms. Meanwhile, compared with the method of element simplification in ^[36], the $\mathcal{GH}$-representation method is more systematic.

Notations: $\mathbb{R}/\mathbb{H}_R$ represent the set of real numbers/reduced biquaternions. $\mathbb{R}^{t}$ represents the set of all real column vectors with order $t$. $\mathbb{R}^{m\times n}/\mathbb{H}_R^{m\times n}$ represent the set of all $m\times n$ real matrices/reduced biquaternion matrices, respectively. $A^T$, $A^H$ and $A^\dagger$ represent the transpose, the conjugate transpose and Moore-Penrose (MP) inverse of matrix $A$, respectively. $\left\|\cdot\right\|$ represents the Frobenius norm of a matrix.

The rest of this paper is organized as follows: Section 2 provides the definition and properties of STP on reduced biquaternion. The main results of this paper are contained in Section 3, in which we define $\mathcal{L_C}$-representation of the reduced biquaternion matrix, then the vector operator on reduced biquaternion matrices are proposed. The general expression of least squares mixed solution of Problem 1 and the necessary and sufficient conditions for compatibility are also given in this section. Section 4 provides the corresponding algorithm of Problem 1 and two numerical examples are proposed to illustrate the effectiveness of the algorithm. Section 5 applies the proposed algorithm to color image restoration. Finally, we make some concluding remarks in Section 6.

2.   Basic definitions

In this section we give some necessary preliminaries that will be used throughout this paper, and we introduce some definitions and properties of STP on reduced biquaternion ^[37].

Definition 2.1. Let $A = (a_{ij})\in\mathbb{H}_R^{m\times n}$ and $B = (b _{ij})\in\mathbb{H}_R^{p\times q}$, then, the Kronecker product of $A$ and $B$ is defined to be the following block matrix

Lemma 2.2. Let $A\in\mathbb{H}_R^{m\times n}$, $B\in\mathbb{H}_R^{n\times p}$, $C\in\mathbb{H}_R^{n\times s}$, and $D\in\mathbb{H}_R^{p\times t}$, then

${\bf{1)}}$ $(A\otimes B)\otimes C = A\otimes (B\otimes C)$.

${\bf{2)}}$ $(A\otimes B)(C\otimes D) = AC\otimes BD$.

Definition 2.3. Let $A\in\mathbb{H}_R^{m\times n}$, $B\in\mathbb{H}_R^{p\times q}$ and $t = lcm(n, p)$ be the least common multiple of $n$ and $p$. Then, the left STP of $A$ and $B$, denoted by $A\ltimes B$, is defined as $A\ltimes B = \left(A\otimes I_{t/n}\right)\left(B\otimes I_{t/p}\right).$

Definition 2.4. Let $A\in\mathbb{H}_R^{m\times n}$, $B\in\mathbb{H}_R^{p\times q}$ and $t = lcm(n, p)$ be the least common multiple of $n$ and $p$, then the right STP of $A$ and $B$, denoted by $A\rtimes B$, is defined as $A\rtimes B = \left(I_{t/n}\otimes A\right)\left(I_{t/p}\otimes B\right).$

Example 2.5. Let $A = \begin{pmatrix}2+{{\bf i}}\ & \ -1+{{\bf j}}\ & \ 1+{{\bf k}}\ & \ 2+{{\bf i}}+{{\bf j}}\end{pmatrix}$, $B = \begin{pmatrix}{{\bf i}}\ & \ {{\bf j}}\end{pmatrix}^T$, then

If $n = p$, the STP of matrices reduces to the common matrix product and the STP of matrices retains most of the properties of common matrix product. From Example 2.5, we can obtain one difference between the left STP and the right STP is that the right STP does not satisfy the block product law. This difference makes the left STP more useful. Next, we mainly discuss some properties of the left STP.

Proposition 2.6. Assume the dimensions of the matrices involved in $\bf{(1)}$ and $\bf{(2)}$ meet the dimension requirement such that $\ltimes$ is well defined, then we have

${\bf{(1)}}$ (Distributive Law)

${\bf{(2) }}$ (Associative Law)

Definition 2.7. For $A\in\mathbb{H}_R^{m\times n}$, let $a_t = (a_{1t}, a_{2t}, \cdots, a_{mt})$, $t = 1, 2, \cdots, n$, $a_p = (a_{p1}, a_{p2}, \cdots, a_{pn})$, $p = 1, 2, \cdots, m$, we define

and $V_r(A) = V_c(A^T)$.

Definition 2.8. A swap matrix $W_{[m, n]}$ is an $mn\times mn$ matrix defined as follows: Its rows and columns are labeled by double index $(i, j)$, the columns are arranged by the ordered multi-index $Id(i, j; m, n)$, and the rows are arranged by the order multi-index $Id(j, i; n, m)$. The element at position $[(I, J), (i, j)]$ is

Next, we illustrate the construction of swap matrix through a simple example.

Example 2.9. Let $m = 3, \ n = 2$. The swap matrix $W_{[m, n]}$ can be constructed as follows: Using double index $(i, j)$ to label its columns and rows, the columns of $W_{[m, n]}$ are labeled by $Id(i, j; 3, 2)$, i.e., $(11, 12, 21, 22, 31, 32)$ and the rows of $W_{[m, n]}$ are labeled by $Id(j, i; 2, 3)$, i.e., $(11, 21, 31, 12, 22, 32)$. According to (2.1), we have

The followings are some useful pesudo-commutative properties. Later on, you can see that they are very useful.

Proposition 2.10. Let $A\in\mathbb{H}_R^{m\times n}$, then

${\bf{(1)}}$ $W_{[m, q]}\ltimes A\ltimes W_{[q, n]} = I_q\otimes A,$

${\bf{(2)}}$ $W_{[m, n]}\ltimes V_r(A) = V_c(A)$, $W_{[n, m]}\ltimes V_c(A) = V_r(A)$.

As a kind of cross-dimensional matrix theory with far-reaching significance, the above proposed extended STP not only enriches the reduced biquaternion matrix theory, but also provides a new method for solving reduced biquaternion matrix equation. The two classic conclusions of real matrix equation are stated as follows.

Lemma 2.11. ^[38] The least squares solutions of the linear system of equations $Ax = b$, with $A\in\mathbb{R}^{m\times n}$ and $b\in\mathbb{R}^{m}$, can be represented as

where $y\in\mathbb{R}^n$ is an arbitrary vector. The minimal norm least squares solution of the linear system of equations $Ax = b$ is $A^{\dagger}b$.

Lemma 2.12. ^[38] The linear system of equations $Ax = b$, with $A\in\mathbb{R}^{m\times n}$ and $b\in\mathbb{R}^{m}$, has a solution $x\in\mathbb{R}^n$ if, and only if,

In that case, it has the general solution

where $y\in\mathbb{R}^n$ is an arbitrary vector. The minimal norm solution of the linear system of equations $Ax = b$ is $A^{\dagger}b$.

3.   Main results

3.1.   $\mathcal{L_C}$-representation of reduced biquaternion matrix

Definition 3.1. For $A = A_1+A_2{{\bf j}}\in\mathbb{H}_R^{m\times n}$, $A_s\in\mathbb{C}^{m\times n}(s = 1, 2)$. Denote

Suppose there is a mapping $\varphi(A) = M\ltimes\left(I_2\otimes(E_2\ltimes \overrightarrow{A}) \right)$ of $\mathbb {H}_R^{m\times n}$ into $\mathbb{C}^{2m\times 2n}$, denote $\varphi^c(A) = \varphi(A)\ltimes \delta_2^1$ for $A\in\mathbb{H}_R^{m\times n}$, $B\in\mathbb{H}_R^{n\times p}$. If $\varphi$ satisfies

${\bf{1)}}$ $\varphi(AB) = \varphi(A)\varphi(B)$,

${\bf{2)}}$ $\varphi^c(AB) = \varphi(A)\varphi^c(B)$,

$\varphi$ is called the $\mathcal{L_C}$-representation of the reduced biquaternion matrix.

The computable equivalent conditions of 1) and 2) in Definition 3.1 can be obtained by using the left STP.

Proposition 3.2. Let $A\in\mathbb{H}_R^{m\times n}, \ B\in\mathbb{H}_R^{n\times p}$, then $\varphi$ is the $\mathcal{L_C}$-representation of the reduced biquaternion matrix if

${\bf{1)}}$ $\left(M\otimes I_m\right)\left(I_2\otimes(E_2\ltimes \overrightarrow{AB})\right) = \left(M\otimes I_m\right)\left(M\otimes (E_2\ltimes \overrightarrow{A})\right)\left(I_2\otimes (E_2\ltimes \overrightarrow{B})\right)$,

${\bf{2)}}$ $\left(M\otimes I_m\right)\left(\delta_2^1\otimes(E_2\ltimes \overrightarrow{AB})\right) = \left(M\otimes I_m\right)\left(M\otimes (E_2\ltimes \overrightarrow{A})\right)\left(\delta_2^1\otimes (E_2\ltimes \overrightarrow{B})\right)$.

Proof. The proof is straightforward. Here, we only prove 2). By the $\mathcal{L}_{\mathcal{C}}$-representation of the reduced biquaternion matrix, we know $\varphi^c(AB) = \varphi(A)\varphi^c(B)$ holds if, and only if,

which is equivalent to

Example 3.3. Let $E_2 = \begin{pmatrix}1\ & \ 0\\0\ & \ 1\end{pmatrix}$. It is easy to compute

If $E_2 = \begin{pmatrix}1\ & \ 0\\0\ & \ -1\end{pmatrix}$, we obtain

Moreover, we bring $\varphi^1(A)$ and $\varphi^2(A)$ into Proposition 3.2 for inspection. It can be found that $\varphi^1(A)$ and $\varphi^2(A)$ meet the requirements, so $\varphi^1(A)$ and $\varphi^2(A)$ are all $\mathcal{L_C}$-representation of the reduced biquaternion matrix.

It is not difficult to see that the product of reduced biquaternion matrices is more difficult than that of complex matrices. Therefore, it is very meaningful for us to find the above isomorphic relationship between the reduced biquaternion matrix and the complex matrix to realize the equivalent transformation of the problem. Moveover, the above isomorphism is more general compared with the conclusion ^[23].

3.2.   Vector operator over reduced biquaternion

Using the STP of reduced biquaternion matrices, we can obtain some new properties of vector operators over reduced biquaternions.

Proposition 3.4. Let $A\in\mathbb{H}_R^{m\times n}$, $B\in\mathbb{H}_R^{n\times p}$, then

Proof. It can be seen from Example 2.5 that the left STP can realize the multiplication of block matrices. Here, by means of this property, we realize the proof of (3.1).

(3.1) can be obtained. From Proposition 2.10, we get

After straightforward calculation, it is not difficult to draw the following conclusions.

Proposition 3.5. Let $A\in\mathbb{H}_R^{m\times n}$, $B\in\mathbb{H}_R^{n\times p}$, then

Proposition 3.6. Let $A\in\mathbb{H}_R^{m\times n}$, $X\in\mathbb{H}_R^{n\times n}$, $B\in\mathbb{H}_R^{n\times p}$, then

Proof. Using Propositions 3.4 and 3.5, we get

3.3.   Algebra solutions of Problem 1

Using $\mathcal{L_C}$-representation and the vector operator over reduced biquaternion, we can transform the problem of the reduced biquaternion matrix equation into a system of linear equations in complex number fields. In this subsection, we will discuss Problem 1 using the above methods. According to the special structure of the solution in Problem 1, we propose a systematic method to simplify the calculation.

Definition 3.7. ^[36] Consider a $p$-dimensional real matrix subspace $\mathbb{X}\subset\mathbb{R}^{n\times n}$. Assume $e_1$, $e_2$, $\ldots$, $e_p$ form the bases of $\mathbb {X}$, which means that for any $X\in\mathbb{X}$ we have $X = x_1e_1+x_2e_2+\cdots +x_pe_p$, and define $H = \left[V_c(e_1), V_c(e_2), \ldots, V_c(e_p)\right]$ if we express $\Psi(X) = V_c(X)$ in the form of

Then, $H\widetilde{X}$ is called an $\mathcal{H}$-representation of $\Psi(X)$, and $H$ is called an $\mathcal{H}$-representation matrix of $\Psi(X)$.

Remark 3.8. The main advantage of $\mathcal{H}$-representation is the ability to transform a matrix-valued equation into a standard vector-valued equation with independent coordinates, allowing for the well-known results in the linear system theory to be applied in our study. However, some reduced biquaternion matrices that have special structures cannot be represented by $\mathcal{H}$-representation to achieve the purpose of variable reduction. This is our motivation to give $\mathcal{GH}$-representation.

Definition 3.9. Consider a reduced biquaternion matrix subspace $\mathbb{X}\subset \mathbb{H}_R^{n\times n}$. For each $X = X_1+X_2{{\bf i}}+X_3{{\bf j}}+X_4{{\bf k}}\in\mathbb{X}$, denote $\chi(X) = [X_1\ X_2\ X_3\ X_4]$ if we express

Then, $\mathrm{H}\widehat{X}$ is called a $\mathcal{GH}$-representation of $\Phi(X)$ and $\mathrm{H}$ is called a $\mathcal{GH}$-representation matrix of $\Phi(X)$, where $\mathrm{H} = \begin{bmatrix}H_{X_1}\ & \ O\ & \ O\ & \ O\\ O\ & \ H_{X_2}\ & \ O\ & \ O\\ O\ & \ O\ & \ H_{X_3}\ & \ O\\ O\ & \ O\ & \ O\ & \ H_{X_4}\end{bmatrix}$, $\widehat{X} = \begin{bmatrix}\widetilde{X_1}\\ \widetilde{X_2}\\ \widetilde{X_3}\\ \widetilde{X_4}\end{bmatrix}$, $H_{X_s}$ represents the $\mathcal{H}$-representation matrix of $X_s$, $(s = 1, 2, 3, 4)$.

In this paper we consider the Hermitian matrix, the centro-symmetric matrix and the bisymmetric matrix on reduced biquaternions. From Definition 3.9, we know that the $\mathcal{GH}$-representation matrix can be constructed from some corresponding real matrices, so we are interested in the $\mathcal{H}$-representation of the related real matrices.

We can see from Definition 1.2 that when $X = X_1+X_2{{\bf i}}+X_3{{\bf j}}+X_4{{\bf k}}$ is Hermitian, $X_1$ is symmetric and $X_2, X_3, X_4$ are anti-symmetric. Denote $S_n$ as the set of symmetric matrices and $S_{-n}$ as the set of anti-symmetric matrices. For $\mathbb{X} = S_n$, we select a standard basis throughout this paper as

where $E_{ij} = \left(e_{lk}\right)_{n\times n}$ with $e_{ij} = e_{ji} = 1$ and the other entries being zero. Similarly, for $\mathbb{X} = S_{-n}$, we select a standard basis as

where $\widetilde{E}_{ij} = \left(\widetilde{e}_{lk}\right)_{n\times n}$ with $\widetilde{e}_{ij} = -\widetilde{e}_{ji} = 1$ and the other entries being zero. After the bases are determined above, for $\mathbb{X} = S_n/\mathbb{X} = S_{-n}$ we have

Note that $\Psi(X_{S_n/S_{-n}})$ is a column vector formed by all elements of $X_{S_n}/X_{S_{-n}}$, while $\widetilde{X}_{S_n}$ and $\widetilde{X}_{S_{-n}}$ are column vectors formed by different nonzero elements of $X_{S_n}$ and $X_{S_{-n}}$, respectively. We denote the $\mathcal{H}$-representation matrix corresponding to $\mathbb{X} = S_n$ by $H_n$, and $H_{-n}$ refers to the $\mathcal{H}$-representation matrix corresponding to $\mathbb{X} = S_{-n}$.

Similarly, we use the above ideas to consider two other classes of special matrices. For $\mathbb{X} = \mathcal{C}_s^{n\times n}$, we can select the following standard basis

where $F_{ij} = (f_{lk})_{n\times n}$ with $f_{ij} = f_{n-i+1, n-j+1}$ and the other entries are zero. After the basis is determined, we have:

We denote the $\mathcal{H}$-representation matrix corresponding to $\mathbb{X} = \mathcal{C}_s^{n\times n}$ by $H_{C_s^e}$ and $H_{C_s^o}$.

For the Bi-hermitian matrix, we have the same discussion as the Hermitian matrix. The components of the Bi-hermitian matrix are considered as two kinds of sets. One is the matrix corresponding to the real part, denoted as $\mathbb{BR}_n$, and the other is the matrix corresponding to the imaginary part, denoted as $\mathbb{BI}_n$. For $\mathbb{X} = \mathbb{BR}_n$, we can select a standard basis as

where $B_{ij} = (b_{lk})_{n\times n}$ with $b_{ij} = b_{n-i+1, n-j+1} = b_{ji} = 1$ and the other entries are zero. Based on the above basis, we have

For $\mathbb{X} = \mathbb{BI}_n$, we can select the following standard basis

where $\widetilde{B}_{ij} = (\widetilde{b}_{lk})_{n\times n}$ with $\widetilde{b}_{ij} = \widetilde{b}_{n-i+1, n-j+1} = -\widetilde{b}_{ji} = 1$ and the other entries are zero. Based the above basis, we have

We denote the $\mathcal{H}$-representation matrix corresponding to $\mathbb{X} = \mathbb{BR}_n$ by $H_{BR^e}$/$H_{BR^o}$ and denote the $\mathcal{H}$-representation matrix corresponding to $\mathbb{X} = \mathbb{BI}_n$ by $H_{BI^e}$/$H_{BI^o}$. Based on our earlier discussion, we now turn our attention to Problem 1. The following notation is necessary to derive a solution to Problem 1.

Then, the subspace $\theta_1, \ \theta_2$ and $\theta_3$ can be written as

and Problem 1 is converted into the following problem.

Find a matrix group $\left(\ddot{X}_1, \ddot{X}_2, \cdots, \ddot{X}_n\right)$ such that

where $\ddot{X}_i\in\acute{\theta}_1, \ (i = 1, 2, \cdots, s)$; $\ddot{X}_i\in\acute{\theta}_2, \ (i = s+1, s+2, \cdots, m)$; $\ddot{X}_i\in\acute{\theta}_3, \ (i = m+1, m+2, \cdots, n)$, $\widehat{E} = E-\sum\limits_{i = 1}^nA_i\widehat{X}_iB_i$.

The solution of Problem 1 is expressed as

We firstly state the following notations to Problem 1. For $A\in\mathbb{H}_R^{m\times n}$, $B\in\mathbb{H}_R^{n\times p}$, let

Theorem 3.10. Suppose $A_i\in \mathbb{H}_R^{m\times n}$, $B_i\in \mathbb{H}_R^{n\times p}$, $C\in \mathbb{H}_R^{m\times p}$, then the set $S_Q$ of Problem 1 can be expressed as

where $\ddot{X}_i\in\acute{\theta}_1, \ (i = 1, 2, \cdots, s)$; $\ddot{X}_i\in\acute{\theta}_2, \ (i = s+1, s+2, \cdots, l)$; $\ddot{X}_i\in\acute{\theta}_3, \ (i = l+1, l+2, \cdots, n)$, and $y$ is an arbitrary real vector of appropriate order. Futhermore, the minimal norm least squares constraint mixed solution $\left(\ddot{X}^Q_1, \ddot{X}^Q_2, \cdots \ddot{X}^Q_n\right)\in S_Q$ satisfies

Proof. In order to facilitate our description of the Problem 1, $\varphi$ is designated as $\varphi^1$ in Example 3.3 and

Using the $\mathcal{GH}$-representation matrix of special matrix, we can continue to simplify the above process. As $\ddot{X_i}$ has certain constraints, we only need to remove the constraint part of the bases of $\mathcal{GH}$-representation matrix of $\ddot{X_i}$, $i = 1, 2, \cdots, n$, $t = 1, 2, 3, 4$. Denote

$V_c(\chi(\ddot{X}_i)) = \begin{pmatrix}\widehat{H}_n & 0 & 0 & 0\\ 0 & \widehat{H}_{-n} & 0 & 0\\0 & 0 & \widehat{H}_{-n} & 0 \\0 & 0 & 0 & \widehat{H}_{-n}\end{pmatrix} \begin{pmatrix}\widetilde{\ddot{X}_i^1} \\ \widetilde{\ddot{X}_i^2}\\ \widetilde{\ddot{X}_i^3} \\ \widetilde{\ddot{X}_i^4}\end{pmatrix} = \mathrm{H}_1\widehat{\ddot{X}_i}, \ \ \ i = 1, 2, \cdots, s.$

$V_c(\chi(\ddot{X}_i)) = \begin{pmatrix}\widehat{H}_{C_s^e/C_s^o} & 0 & 0 & 0\\ 0 & \widehat{H}_{C_s^e/C_s^o} & 0 & 0\\0 & 0 & \widehat{H}_{C_s^e/C_s^o} & 0\\0 & 0 & 0 & \widehat{H}_{C_s^e/C_s^o}\end{pmatrix} \begin{pmatrix}\widetilde{\ddot{X}_i^1} \\ \widetilde{\ddot{X}_i^2}\\ \widetilde{\ddot{X}_i^3} \\ \widetilde{\ddot{X}_i^4}\end{pmatrix} = \mathrm{H}_2\widehat{\ddot{X}_i}, \ i = s+1, s+2, \cdots, m.$

$V_c(\chi(\ddot{X}_i)) = \begin{pmatrix}\widehat{H}_{BR^e/BR^o} & 0 & 0 & 0\\ 0 & \widehat{H}_{BI^e/BI^o} & 0 & 0\\0 & 0 & \widehat{H}_{BI^e/BI^o} & 0\\0 & 0 & 0 & \widehat{H}_{BI^e/BI^o} \end{pmatrix} \begin{pmatrix}\widetilde{\ddot{X}_i^1} \\ \widetilde{\ddot{X}_i^2}\\ \widetilde{\ddot{X}_i^3} \\ \widetilde{\ddot{X}_i^4}\end{pmatrix} = \mathrm{H}_3\widehat{\ddot{X}_i}, \ (i = m+1, m+2, \cdots, n$).

Further, we can get

Thus,

if, and only if,

For the real matrix equation

according to Lemma 2.11, its least squares mixed solutions can be represented as

where $y$ is an arbitrary real vector of appropriate order, and the minimal norm least squares mixed solution $\left(X^Q_1, X^Q_2, \cdots, X^Q_n\right)\in S_Q$ of (1.1) satisfies

Therefore, (3.18) and (3.19) can be obtained.

Corollary 3.11. Suppose $A_i\in \mathbb{H}_R^{m\times n}$, $B_i\in \mathbb{H}_R^{n\times p}$, $C\in\mathbb{H}_R^{m\times p}$ $i = 1, 2, \cdots, n$. (1.1) has a solution satisfying (3.16) if, and only if,

The set $S_L$ of the general solution is

where $y$ is an arbitrary real vector of appropriate order and the minimal norm solution $\left(\ddot{X}^L_1, \ddot{X}^L_2, \cdots \ddot{X}^L_n\right)\in S_L$ satisfies

where $y$ is an arbitrary real vector of appropriate order. $U$ and $\begin{pmatrix}\mathrm{Re}(\varphi^c(V_c(\widehat{E})))\\ \mathrm{Im}(\varphi^c(V_c(\widehat{E})))\end{pmatrix}$ are given in Theorem 3.10.

Proof. Since

then

(3.20) holds. Moreover, we can obtain the expression of general solution and the minimal norm mixed solution using Lemma 2.12.

4.   Algorithms and numerical examples

A real vector representation of reduced biquaternion matrices based on STP was proposed^[34], and used it to solve the anti-Hermitain solution of the reduced biquaternion matrix equation $\sum\limits_{i = 1}^nA_iXB_i = C$. In this paper, we take the Hermitian solution of the reduced biquaternion matrix equation $AXB = C$ as an example to illustrate the advantagement of our algorithm.

Example 4.1. For $A_l\in\mathbb{H}_R^{m\times n}$, $B_l\in\mathbb{H}_R^{n\times p}$, $l = 1, 2, 3$, $X_1\in\theta_1$, $X_2\in\theta_2$, $X_3\in\theta_3$, let $m = n = p = 5K$, $t_i = 3K$, $K = 1:10$. Then, we compute

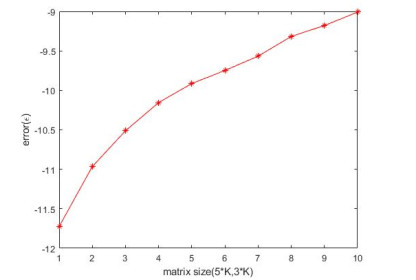
By Algorithm 1, we obtain the calculated solution $[X_1^{*}, X_2^{*}, X_3^{*}]$. Denote the error between the calculated solution and the exact solution as $\varepsilon = log_{10}\left\|[X_1, X_2, X_3]-[X_1^{*}, X_2^{*}, X_3^{*}]\right\|$, and $\varepsilon$ is recorded in Figure 1.

Example 4.2. For $A\in\mathbb{H}_R^{m\times n}$, $B\in\mathbb{H}_R^{n\times p}$, $X\in\mathbb{SH}_R^{n\times n}$, let $m = n = p = 2K$, $K = 1:9$. Then, we compute

For coefficient matrices of the reduced biquaternion equation (4.1) with different orders, we solve the unique solution $X_{\varsigma}$ by the method in this paper and the method in ^[34]. Denote $\xi = log_{10}\left\|X-X_{\varsigma}\right\|$ and note down the $\xi$ and CPU times of two methods, respectively. Detailed results are shown in Figure 2.

Remark 4.3. We make some explanations for the above three examples.

1) It can be seen from Figure 1 that the order of magnitude of $\varepsilon < -9$. Thus, the effectiveness of our algorithm can be tested.

2) As seen from Figure 2, when calculating the Hermitian solution of the reudced biquaternion matrix equation (4.1) by the two methods, the errors between the obtained solution and the exact solution are very small. Compared with the method in ^[34], the method in this paper has an absolute advantage in calculation time. Moreover, with the increasing of $K$, the memory occupied by the method in ^[34] is also relatively large, which is not feasible for calculating the reduced biquaternion matrix equation under large dimension. It can be seen that the effect of our proposed algorithm is very clear.

5.   Application to color image restoration

In the process of image acquisition, it is always affected by external conditions and the surrounding environment, resulting in image quality damage. For example, underwater images are severely affected by the particular physical and chemical characteristics of underwater conditions. It is well known that the first encounters with digital image restoration in the engineering community were in the area of astronomical imaging. With the progress of society, color image restoration technology has been applied in many fields.

Image restoration is the process of removing and minimizing degradations in an observed image. A linear discrete model of image restoration is the matrix-vector equation

where $g$ is an observed image, $f$ is the true or ideal iamge, $n$ is additive noise, and $K$ is a matrix that represents the blurring phenomena. The methods used in image restoration aim to construct an approximation to $f$ given $g$, $K$ and in some cases statistical information about the noise. However, in most cases, the noise $n$ is unknown. We wish to find $f'$ such that

In ^[21], Pei proposed to encode the three channel components of a color image on the three imaginary parts of a pure reduced biquaternion. That is,

where $r(x, y)$, $g(x, y)$ and $b(x, y)$ are the red, green, and blue values of the pixel $(x, y)$, respectively. Thus, a color image with $m$ rows and $n$ columns can be represented by a pure imaginary reduced biquaternion matrix

Since then, reduced biquaternion representation of a color image has attracted great attention. Many researchers applied the reduced biquaternion matrix to study the problems of color image processing^{[24,34,39,40]} due to the ability of reduced biquaternion matrices treating the three color channels holistically without losing color information. The effectiveness of the proposed method was tested by a practical example.

Example 5.1. Two color images are given in Figures 3 and 4. $M = (R, G, B)$ is the image matrix with special structure. $M$ can be represented as the pure imaginary matrix $M = R{{\bf i}}+G{{\bf j}}+B{{\bf k}}$. By operation, we can get $m = (m_r, m_g, m_b)$, where $m_r = vec(R)$, $m_g = vec(G)$ and $m_b = vec(B)$. By using $LEN = 15$, $THETA = 30$ and $PSF = fspecial('motion', LEN, THETA)$, we disturb the image $R$ and get the image $d_R$. Clearly, $K = d_rm_r^{\dagger}$, where $d_r = vec(d_R)$. For convenience, we disturb the images $G, B$ using the same matrix $K$. Thus, $d = (R, G, B)$ becomes an image matrix $d = Km$, that is $d = (d_r, d_b, d_b) = Km = K(m_r, m_g, m_b)$. By computation, we obtain

We denote $\vartheta R$, $\vartheta G$ and $\vartheta B$ as the differences between the computed $R$ and original $R$, computed $G$ and original $G$ and the computed $B$ and original $B$, respectively. All the information is contented in Table 1.

6.   Conclusions

In this paper, with the help of STP, some new properties of the reduced biquaternion vector operator were proposed, and the $\mathcal{L_C}$-representation, a class of algebraic expressions of isomorphism relation between the set of reduced biquaternion matrices and the set of complex matrices, were given. Making use of vector operator $\mathcal{L_C}$-representation and $\mathcal{GH}$-representation of special reduced biquaternion matrices, we solved the mixed solution of reduced biquaternion matrix equation $\sum\limits_{i = 1}^nA_iX_iB_i = E$ with sub-matrix constraints. Both the comparison with other methods, and the effect of application in color image restoration demonstrated the effectiveness of our proposed method.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This work is supported by the National Natural Science Foundation of China (62176112) and the Natural Science Foundation of Shandong Province (ZR2020MA053 and ZR2022MA030) and Discipline with Strong Characteristic of Liaocheng University Intelligent Science and Technology (319462208).

Conflict of interest

All authors declare no conflicts of interest in this paper.