1.
Introduction
In 2008, Bhattacharya et al. [5] and Bell et al. [4] discovered that bipartite chain graphs whose largest eigenvalues within the connected bipartite graph is maximal, and named therein as double nested graphs. After that, many scholars began to study some mathematical properties of chain graphs. Andelic et al. provide that some upper and lower bounds on index of chain graphs [3]. And Alazemi et al. proved that any chain graph has its least positive eigenvalue greater than 12 [2]. Hence Zhang et al. proposed that upper bounds on Laplacian spectral radius of chain graphs [13]. Das et al. studied the energy and Laplacian energy of chain graphs [8]. In this paper, we further study some bounds of energy and Laplacian energy of chain graphs.
We consider finite undirected connected graphs without loops and multiple edges. Let G be a such graph with vertex set V(G)={v1,v2,…,vn} and edge set E(G), where |E(G)|=m. Let di be the degree of the vertex vi for i=1,2,…,n. The minimum vertex degrees of G are denoted by δ(G). Let NG(vi) be the adjacent set of the vertex vi, then di=|NG(vi)|. If G has distinct vertices vi and vj with NG(vi)=NG(vj), then vi and vj are duplicates and (vi,vj) is a duplicate pair.
Let A(G) be the adjacency matrix of G, and rank(G) be the rank of the adjacency matrix A(G). Let λ1≥λ2≥⋯≥λn the eigenvalues of A(G). We denote S(G)={λ1,λ2,…,λn} as the spectrum of G. The energy of graph G is defined as [11]
For its basic properties and application, including various lower and upper bounds, see the [17], the recent paper [1,7,8,11,12,20] and the references cited therein.
The Laplacian matrix of graph G is defined as L(G)=D(G)−A(G), where D(G) is the diagonal matrix of vertex degrees. The matrix L(G) has non-negative eigenvalues μ1≥μ2≥⋯≥μn−1≥μn=0, and the Laplacian spectrum of graph G be denoted by LS(G)={μ1,μ2,…,μn}. The Laplacian energy of G is defined as [10]
It can also be defined as
where σ(1⩽σ⩽n) be the largest positive integer such that μσ≥2mn and Sk(G)=k∑i=1μi.
For its basic properties, including various lower and upper bounds, see [7,8,10,18,19] and the references cited therein. The Laplacian energy found applications not only in theoretical organic chemistry [12,21], but also in image processing [22] and information theory [16].
In the class of bipartite graphs of fixed order and size those having maximal spectral radius of adjacency/Laplacian/signless Laplacian matrix are chain graphs. Thus, they can be significant in modeling some bipartite networks with large spectral radius. Their applications involve ecological networks, in which graphs with nested properties are considered [14] and are used in some applications for economic network modeling.
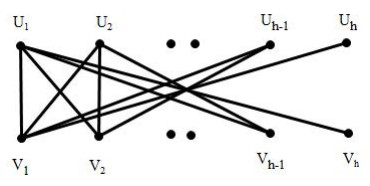
We now introduce the structure of a (connected) chain graph. The vertex set of any chain graph consists of two color classes, which are U and V. Both of them are divided into h non-empty units U1,U2,…,Uh and V1,V2,…,Vh, respectively. All the vertices in Us are joined by edges to all vertices in ⋃h+1−sk=1Vk, for s=1,2,…,h. Therefore, if ui∈Us+1 and uj∈Us, then NG(ui)⊂NG(uj), or if vi∈Vt+1 and vj∈vt, then NG(vi)⊂NG(vj).
If ns=|Us| and ms=|Vs| for s=1,2,…,h, then G is denoted by G(m1,…,mh;n1,…,nh), as shown in Figure 1. And
where
Moreover,
The second smallest Laplacian eigenvalue of a graph is well known as the algebraic connectivity. It has been proved that the second smallest Laplacian eigenvalue μn−1=0 if and only if G is disconnected. The algebraic connectivity is often applied in theoretical chemistry, control theory, combinatorial optimization and other fields [15].
As usual, Kn, Kp,q(p+q=n) and K1,n−1, denote, respectively, the complete graph, the complete bipartite graph and the star on n vertices. For other undefined notations and terminology from graph theory, the readers are referred to [6].
The paper is organized as follows. In Section 2, we list some previously known results. In Section 3, we get some upper and lower bounds on ε(G) of a chain graph G. In Section 4, we establish an upper bound on LE(G) of the chain graphs in terms of vertex cover number. In Section 5, we attain the maximal Laplacian energy of the bicyclic chain graph G by comparing the algebraic connectivity.
2.
Preliminaries
This section lists some known results to be used in this paper.
Lemma 2.1. [8] Let B be a p×p real symmetric matrix and Bk be its leading k×k submatrix. Then for i=1,2,…,k,
where λi(B) is the i-th largest eigenvalue of B.
Lemma 2.2. [9] Let G be a graph with vertices {v1,v2,…,vk}⊆V(G) having same set of adjacent vertices, then G has at least k−1 equal eigenvalues 0.
Lemma 2.3. [18] Let G≆Kn. Then μn−1⩽δ(G).
Lemma 2.4. [10] Let A and B be real symmetric matrices of order n. Then for any 1⩽k⩽n,
where λi(M) denotes the i-th largest eigenvalue of the matrix M.
Lemma 2.5. [1] If G is a connected bipartite graph of rank r, then
Lemma 2.6. [11] If G is a connected bipartite graph of rank r, then
Lemma 2.7. [8] Let G≅G(m1,…,mh;n1,…,nh) be a chain graph of order n. Then
with equation holds if and only if G≅K1,n−1.
Lemma 2.8. [8] Let G be a graph with vertex set V(G)={v1,v2,…,vn}. If G has k−1 duplicate pairs (vi,vi+1), where i=1,2,…,k−1, then G has at least k−1 equal Laplacian eigenvalues and they are all equal to the cardinality of the neighbor set.
3.
Bounds on the energy of chain graphs
Theorem 3.1. Let G≅G(m1,…,mh;n1,…,nh) be a chain graph of order n. Then
with equation holds if and only if G≅Kn1,m1, where n1+m1=n.
Proof. By Lemma 2.2, the eigenvalue 0 with multiplicity h∑i=1(ni+mi−2) of A(G), and the remaining eigenvalues are the eigenvalues of the following matrix,
Let λ1≥λ2≥⋯≥λ2h be the eigenvalues of C. Then
Since G be a bipartite graph, we have λi and −λi are eigenvalues of G. Thus we have
Obviously,
that is,
So
First we assume that h=1. Then G≅Kn1,m1, where n1+m1=n. So S(G)={±√m1n1,0,⋯,0} and ε(G)=2√m1n1=2√m. Hence the equation holds in (3.1).
Next we assume that h≥2. By the definition of chain graph, G(1,1;1,1), that is, P4 is an induced subetaaph of G. By Lemma 2.1, we get λ2(G)≥λ2(P4)>0. Since G is connected, by Perron-Frobenius theorem we have λ1(G)>λ2(G). Hence the inequality 2∑1⩽i<j⩽hλiλj≤h∑i=1(h−1)λ2i is strict. This completes the proof.
Theorem 3.2. Let G≅G(m1,…,mh;n1,…,nh) be a chain graph of order n. Then
Proof. By calculating the matrix C in the proof of Theorem 3.1, we get
Therefore, all the eigenvalues of matrix C are non-zero. Hence r(G)=2h. Using Lemma 2.5, we can get result in (3.2).
4.
Bounds on the Laplacian energy of chain graphs
In this section, we give an upper bound on LE(G) of chain graphs in terms of vertex cover number. Also, the lower bound follows from a known lower bound for Laplacian energy of any graph in terms of rank and energy.
Theorem 4.1. Let G≅G(m1,…,mh;n1,…,nh) be a chain graph of order n, and a1≥b1. Then
with equation holds if and only if G≅K1,n−1.
Proof. Let Γ={v11,v12,…,v1m1,v21,v22,…,v2m2,…,vh1,vh2,…,vhmh} be a vertex cover set of the graph G, where vij is the j-th vertex in Vi. Hence {vi1,vi2,…,vimi}∈Vi. We can assume that Gij are spanning subetaaphs of G such that V(G)=V(Gi1)=V(Gi2)=⋯=V(Gimi), and the edge set of Gij is defined as
Since |NG(vi1)|=|NG(vi2)|=⋯=|NG(vimi)|=ai,
we have
so
By Figure 1,
then we can see easily that
Note that
where Sk(G) is the sum of the k largest Laplacian eigenvalues of graph G.
By Lemma 2.4, we get
So from (1.1), we get
Since G is connected, 1≤σ≤n−1. So it suffices to consider the following two cases.
Case1. 2mn≥b1.
Then we have
Case2. 2mn<b1.
By Lemma 2.3, we get μn−1≤δ(G)≤2mn. Thus it must be 1≤σ≤n−2. Hence
Next we prove that the equality holds.
If G≅K1,n−1, we get b1=m1=1,n1=n−1, and S(G)={0,1n−2,n}. Then
Theorem 4.2. Let G≅G(m1,…,mh;n1,…,nh) be a chain graph of order n. Then
Proof. By Theorem 3.2, we get r(G)=2h. Using Lemmas 2.6 and 2.7, we get result in (4.2).
5.
Laplacian energy of bicyclic chain graphs
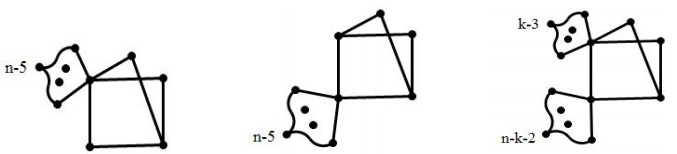
Let G be a connected bicyclic chain graph. We have m=n+1, and h=2 or h=3. If h=2, then G≅G(1,1;3,n−5) or G≅G(1,2;2,n−5). If h=3, then G≅G(1,2,k−3;1,1,n−k−2), where 4≤k≤n−3 (Figure 2). In this section, we will attain the maximal Laplacian energy of all connected bicyclic chain graphs.
Lemma 5.1. Let G be a connected bicyclic chain graph (n≥8).
(1) If G≅G(1,1;3,n−5), then LE(G)=6+2(n−4)(n+1)n−2μn−1.
(2) If G≅G(1,2;2,n−5), then LE(G)=10+2(n−6)(n+1)n−2μn−1.
(3) If G≅G(1,2,k−3;1,1,n−k−2), where 4≤k≤n−3, then LE(G)=10+2(n−6)(n+1)n−2μn−1.
Proof. (1) Let G≅G(1,1;3,n−5). By Lemma 2.8, we conclude that 2,2,1,1,⋯,1⏟n−6 are the Laplacian eigenvalues of G and the remaining Laplacian eigenvalues of G are satisfying the equation f1(x)=0, where f1(x) is the characteristic polynomial of the matrix
that is, f1(x)=x(x3−(4+n)x2+(5n−2)x−3n).
Let h1(x)=x3−(4+n)x2+(5n−2)x−3n. Then we obtain h1(0)=−3n<0, h1(1)=n−5>0, h1(2)=3n−12>0, h1(n−1)=−3<0 and limx→∞h1(x)=∞. Thus the Laplacian eigenvalues of G are μ1,μ2,2,2,1,1,…,1⏟n−6,μn−1,0, where μ1≥n−1, 2≤μ2≤n−1, μn−1<1 and μ1+μ2+μn−1=n+4.
Therefore
(2) Let G≅G(1,2;2,n−5). By Lemma 2.8, we conclude that 3,2,1,1,⋯,1⏟n−6 are the Laplacian eigenvalues of G and the remaining Laplacian eigenvalues of G are satisfying the equation f2(x)=0, where f2(x) is the characteristic polynomial of the matrix
that is, f2(x)=x(x3−(3+n)x2+(5n−8)x−2n).
Let h2(x)=x3−(3+n)x2+(5n−8)x−2n. Then we obtain h2(0)=−2n<0, h2(1)=2n−10>0, h2(3)=4n−24>0, h2(n−2)=−4<0 and limx→∞h2(x)=∞. Thus the Laplacian eigenvalues of G are μ1,μ2,3,2,1,1,…,1⏟n−6,μn−1,0, where μ1≥n−2, 3≤μ2≤n−2, μn−1<1 and μ1+μ2+μn−1=n+3.
Therefore
(3) Let G≅G(1,2,k−3;1,1,n−k−2). When 4≤k≤⌈n2⌉, by Lemma 2.8, we conclude that 2,1,1,⋯,1⏟n−7 are the Laplacian eigenvalues of G and the remaining laplacian eigenvalues of G are satisfying equation f3(x)=0, where f3(x) is the characteristic polynomial of the matrix
that is
Let g(x)=x4−(n+6)x3+(kn+5n−k2+10)x2−(4kn+5n−4k2+12)x+6n. Then we obtain g(0)=6n>0, g(1)=3k2−3kn+5n−7<0, g(2)=4(k−2)(2+k−n)<0, g(k)=−(k−2)(k−3)(2k−n)≥0. Since when n is odd, g(x) is same for k=⌈n2⌉ and k=⌊n2⌋, we take a smaller value k=⌊n2⌋. g(n−k)=(2+k−n)(2k−n)(−n+3+k)≤0 and limx→∞g(x)=∞. Thus the Laplacian eigenvalues of G are μ1,μ2,μ3,2,1,1,⋯,1⏟n−7,μn−1,0, where μ1≥n−k, k≤μ2≤n−k, 2<μ3<k, μn−1<1.
Since n∑i=1μi=2m=2(n+1)=2n+2, we get μ1+μ2+μ3+μn−1=n+6, that is, μ1+μ2+μ3=n+6−μn−1.
Therefore
When ⌈n2⌉<k<n−3, letting k=n−k in the Eq (5.3) we get the same characteristic polynomial, so it is equal to the Laplacian energy when 4≤k≤⌈n2⌉.
When k=n−3, f3(x)=x(x−1)(x−3)(x3−(3+n)x2+(5n−8)x−2n), so it is equal to the Laplacian energy of G(1,2;2,5).
This completes the proof.
Lemma 5.2. Let Gn,k≅G(1,2,k−3;1,1,n−k−2), where 4≤k≤⌈n2⌉. Then μn−1(Gn,k)≥μn−1(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2)), with equation holds if and only if k=⌈n2⌉. In particular, if n is odd, then μn−1(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2))=μn−1(G(1,2,⌈n2⌉−4;1,1,⌊n2⌋−1)).
Proof. If k=⌈n2⌉, then μn−1(Gn,k)=μn−1(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2)). By Lemma 5.1, we obtain that μ1,μ2,μ3,μn−1 are the roots of the equation P(Gn,k,x)=0, where
and μ1≥n−k, k≤μ2≤n−k, 2<μ3<k, μn−1<1.
We need to prove that
Since
we get P(Gn,k+1,x)−P(Gn,k,x)≤0. Hence P(Gn,k+1,x)≤P(Gn,k,x). So when n is odd and k=⌈n2⌉−1, the equation holds.
Thus we have μn−1(Gn,k)>μn−1(Gn,k+1), that is,
Hence μn−1(Gn,k)>μn−1(Gn,⌈n2⌉)=μn−1(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2)).
This completes the proof.
Lemma 5.3. Let G be a bicyclic graph of order n≥8. Then μn−1(G(1,2;2,n−5))>μn−1(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2)).
Proof. When k=3, we get P(Gn,k,x)=f2(x), that is μn−1(Gn,3)=μn−1(G(1,2;2,n−5)).
By Lemma 5.2, we have P(Gn,k+1,x)≤P(Gn,k,x), and P(Gn,4,x)≤P(Gn,3,x) still hold.
By inequation (5.5), we obtain
Hence μn−1(G(1,2;2,n−5))>μn−1(Gn,⌈n2⌉)=μn−1(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2)) for n≥8.
Lemma 5.4. Let G be a bicyclic graph of order n≥8. Then μn−1(G(1,1;3,n−5))−μn−1(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2))>2n.
Proof. For n=8 and n=9, it can be verified by using Maple.
Let n=8, μn−1(G(1,1;3,n−5))=0.8377 and μn−1(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2))=0.5858. Then μn−1(G(1,1;3,n−5))−μn−1(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2))=0.2519>14, so the conclusion is correct.
Let n=9, μn−1(G(1,1;3,n−5))=0.8169 and μn−1(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2))=0.5344. Then μn−1(G(1,1;3,n−5))−μn−1(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2))=0.2825>29, so the conclusion is correct.
Next we prove when n≥10, the inequality holds.
By Lemma 5.3, we get μn−1(G(1,2;2,n−5))≥μn−1(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2)), so we can prove μn−1(G(1,1;3,n−5))−μn−1(G(1,2;2,n−5))>2n. Let α=μn−1(G(1,1;3,n−5)), β=μn−1(G(1,2;2,n−5)). Then it is satisfying
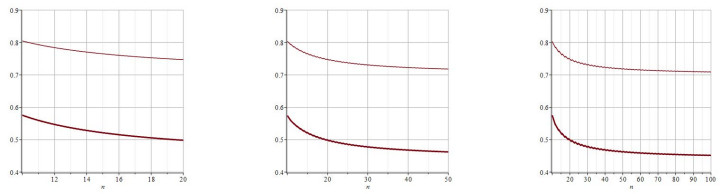
By the implicit function existence theorem and Figure 3, when G≅G(1,1;3,n−5), the relation between the decreases of α and the increase of n, and h1(x) is monotonically increasing on the interval [0,1]. Hence h1(0.81)=−3.713+0.39n>0, h1(0.69)=−2.956−0.26n<0, so 0.69<α<0.81.
Similarly, h2(0.58)=−5.454+0.56n>0, h2(0.43)=−3.915−0.035n<0, so 0.43<β<0.58. Therefore, α−β>0.11>219, that is, when n≥19, hence the conclusion is correct.
When 10≤n≤18, α−β>2n is obvious. The results are shown in Table 1.
So we conclude that when n≥8,
Theorem 5.1. Let G be a connected bicyclic chain graph of order n≥8. Then G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2) attains the maximal Laplacian energy. In particular, when n is odd, LE(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2))=LE(G(1,2,⌈n2⌉−4;1,1,⌊n2⌋−1)).
Proof. By Lemma 5.1, we can attain the maximal Laplacian energy by comparing μn−1 in equations (5.1), (5.2) and (5.4). It is obvious that LE(G(1,2;2,n−5))<LE(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2)). In particular, when n is odd, LE(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2))=LE(G(1,2,⌈n2⌉−4;1,1,⌊n2⌋−1)). So
Hence by Lemma 5.4, LE(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2))−LE(G(1,1;3,n−5))>0, that is, LE(G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2))>LE(G(1,1;3,n−5)). In conclusion, we get G(1,2,⌈n2⌉−3;1,1,⌊n2⌋−2) has the maximal Laplacian energy among all connected bicyclic chain graphs (n≥8).
6.
Conclusions
In this paper, we introduced the definition of chain graph. We obtain some bounds on ε(G) of the chain graphs. Since the rank of the chain graphs is 2h, we can get some bounds on ε(G) and LE(G) of the chain graphs. We present the upper bound on LE(G) of the chain graphs in terms of vertex cover number. In order to attain the maximal Laplacian energy of bicyclic chain graphs, we compare algebraic connectivity of each kind of bicyclic chain graphs. The problem is still open to discuss what chain graphs give the maximal Laplacian energy for given n and whether it is still related to algebraic connectivity.
Acknowledgment
This work was supported by National Nature Science Foundation of China (Grant No. 61774137). The authors express their sincere thanks to the anonymous referee for many valuable comments and suggestions.
Conflict of interest
The authors declare that they have no conflict of interest in this paper.









 DownLoad:
DownLoad: