Central vertex join and central edge join of two graphs

1.   Introduction

In this paper, we consider only simple graphs. Let $G = (V, E)$ be a graph with vertex set $V(G) = \{v_{1}, v_{2}, ..., v_{n}\}$ and edge set $E(G) = \{e_{1}, e_{2}, ..., e_{m}\}$, and let $d_{i}$ be the degree of the vertex $v_{i}, i = 1, 2, ..., n.$ The adjacency matrix $A(G)$ of the graph $G$ is a square matrix of order $n$ whose $(i, j)^{th}$ entry is equal to unity if the vertices $v_{i}$ and $v_{j}$ are adjacent, and is equal to zero otherwise. The Laplacian matrix of $G$, denoted by $L(G)$ is defined as $L(G) = D(G)-A(G)$ and the signless Laplacian matrix of $G$, denoted by $Q(G)$ is defined as $Q(G) = D(G)+A(G)$, where $D(G)$ is the diagonal matrix with vertex degrees. The characteristic polynomial of the $n\times n$ matrix $M$ of $G$ is defined as $f(M, x) = |xI_n-M|$, where $I_n$ is the identity matrix of order $n$. The matrices $A(G), L(G)$ and $Q(G)$ are real and symmetric matrices, its eigenvalues are real. The eigenvalues of $A(G), L(G)$ and $Q(G)$ are denoted by $\lambda_1\geqslant\lambda_2\geqslant...\geqslant\lambda_n, 0 = \mu_1\leq\mu_2\leq...\leq \mu_n$ and $\nu_1\leq\nu_2\leq...\leq\nu_n$ respectively. The collection of all the eigenvalues of $A(G)$ (respectively, $L(G)$, $Q(G)$) together with their multiplicities are called the $A$- spectrum (respectively, $L$-spectrum, $Q$-spectrum) of $G$. Two graphs are said to be $A$-cospectral (respectively, $L$-cospectral, $Q$-cospectral), if they have same $A$-spectrum (respectively, $L$-spectrum, $Q$-spectrum). Otherwise, they are non $A$-cospectral (respectively, non $L$-cospectral, non $Q$-cospectral) graphs.

It is well known that the spectrum of a graph contains a lot of structural information about the graphs, see ^[2,3]. Spectral graph theory plays an important role in theoretical physics and quantum mechanics. Graph spectra plays a vital role in solving various problems in communication networks. Let $\lambda_{1}, \lambda_{2}, ..., \lambda_{t}$ be the distinct eigenvalues of $G$ with multiplicities $m_1, m_2, ..., m_t.$ Then the spectrum of $G$ is denoted by $Spec(G) = \begin{pmatrix} \lambda_1 & \lambda_2 & ... & \lambda_t\\ m_1&m_2 & ...&m_t\\ \end{pmatrix}.$ The incidence matrix of a graph $G$, $I(G)$ is the $n\times m$ matrix whose $(i, j)^{th}$ entry is 1 if $v_i$ is incident to $e_j$ and 0 otherwise. It is known ^[2] that, $I(G)I(G)^T = A(G)+D(G)$ and if $G$ is an $r$-regular graph then $I(G)I(G)^T = A(G)+rI$. The adjacency matrix of the complement of a graph $G$ is $A(\bar{G}) = J_n-I_n-A(G)$, where $J_n$ is an $n\times n$ matrix with all entries are ones. A graph $G$ is called $A$-integral (respectively, $L$-integral, $Q$-integral) if the spectrum of $A(G)$ (respectively, $L(G), Q(G))$ consists only of integers. For an $r$-regular graph it is well known that, $G$ is $A$-integral if and only if it is $L$-integral. If $G$ is an $r$-regular graph then $\lambda_i(G) = r-\mu_i(G), i = 1, 2, ..., n.$ Let $G$ be a connected graph with $n$ vertices. Then the number of spanning trees of $G$ is $t(G) = \frac{\mu_2\cdot\mu_3...\mu_n}{n}$ and the Kirchhoff index of $G$ is defined as $Kf(G) = n \sum\limits_{i = 2}^{n}\frac{1}{\mu_i}$. Let $K_n$, $K_{p, q}$ and $mK_1$ denote the complete graph on $n$ vertices, complete bipartite graph on $p+q$ vertices and completely disconnected graph with $m$ vertices respectively. Throughout, we use $J_n$ is an $n\times n$ matrix with all entries are ones, $J_{s\times t}$ denote the $s\times t$ matrix with all entries equal to one and $I_n$ is the identity matrix of order $n$.

In literature there are many graph operations like, complements, disjoint union, join, cartesian product, direct product, strong product, lexicographic product, corona, edge corona, neighbourhood corona etc. Recently, several variants of corona product of two graphs have been introduced and their spectra are computed. In ^[6], Liu and Lu introduced subdivision-vertex and subdivision-edge neighbourhood corona of two graphs and provided a complete description of their spectra. In ^[5], Lan and Zhou introduced R-vertex corona, R-edge corona, R-vertex neighborhood corona and R-edge neighborhood corona, and studied their spectra. Recently in ^[1], Adiga et al. introduced duplication corona, duplication neighborhood corona and duplication edge corona. In ^[4], Das and Panigrahi computed the spectrum of R-vertex join and R-edge join of two graphs. Motivated by these works, we define two new graph operations based on central graphs.

Definition 1.1 ^[9] Let $G$ be a simple graph with $n$ vertices and $m$ edges. The central graph of $G$, denoted by $C(G)$ is obtained by sub dividing each edge of $G$ exactly once and joining all the non adjacent vertices in $G$.

The number of vertices and edges in $C(G)$ are $m+n$ and $m+\frac{n(n-1)}{2}$ respectively.

The rest of the paper is organized as follows. In Section 2, we present some definitions and lemmas that will be used later. In Section 3, $A$-spectra, $L$-spectra, and $Q$-spectra of $C(G)$ and a formula for the number of spanning trees and Kirchhoff index of $C(G)$ is obtained. In Section 4, we introduce central vertex join and central edge join of two graphs and determine the $A$-spectra, $L$-spectra and $Q$-spectra of $G_1\dot{\vee} G_2$ (respectively $G_1\veebar G_2$) in terms of the corresponding spectra of $G_1$ and $G_2$. Also some new classes of $A$-cospectral, $L$-cospectral and $Q$-cospectral graphs are constructed. In addition to that the number of spanning trees and Kirchhoff index of these join of two graphs are calculated. In Section 5, some new families of integral graphs are given.

2.   Preliminaries

In this section, we give some definitions and results which are useful to prove our main results.

Lemma 2.1. ^[2] Let $U, V, W$ and $X$ be matrices with $U$ invertible. Let

Then $det(S) = det(U)det(X-WU^{-1}V)$ and if $X$ is invertible, then $det(S) = det(X)det(U-VX^{-1}W).$ If $U$ and $W$ are commutes then $det(S) = det(UX-WV)$.

Lemma 2.2. ^[2] Let $G$ be a connected $r$-regular graph on $n$ vertices with adjacency matrix $A$ having $t$ distinct eigenvalues $r = \lambda_1, \lambda_2, ..., \lambda_t$. Then there exists a polynomial

such that $P(A) = J_n, P(r) = n$ and $P(\lambda_i) = 0$ for $\lambda_i\neq r.$

Definition 2.1 ^[4] The $M$-coronal $\chi_M(x)$ of $n\times n$ matrix $M$ is defined as the sum of the entries of the matrix $(xI_n-M)^{-1}$ $($if exists$)$, that is,

Lemma 2.3. ^[8] Let $G$ be an $r$-regular graph on $n$ vertices, then $\chi_A(x) = \frac{n}{x-r}$.

For Laplacian matrix each row sum is zero, so $\chi_L(x) = \frac{n}{x}$.

Lemma 2.4. ^[8] Let $G$ be a bipartite graph $K_{p, q}$ with $p+q = n$, then $\chi_{A(G)}(x) = \frac{nx+2pq}{(x^2-pq)}.$

Lemma 2.5. ^[7] Let $A$ be an $n\times n$ real matrix. Then $det(A+\alpha J_{n}) = det(A)+\alpha J_{n\times 1}^T adj(A)J_{n\times 1}$, where $\alpha$ is a real number and $adj(A)$ is the adjoint of $A$.

Corollary 2.6. ^[7] Let $A$ be an $n\times n$ real matrix. Then

Lemma 2.7. ^[7] For any real numbers $c, d > 0, (cI_n-dJ_{n})^{-1} = \frac{1}{c}I_n+\frac{d}{c(c-nd)}J_{n}.$

3.   Spectra of central graphs

In this section, we compute the adjacency spectrum (respectively, Laplacian spectrum, signless Laplacian spectrum) of central graph of regular graphs.

Theorem 3.1. Let $G$ be an $r$-regular graph on $n$ vertices and $\frac{nr}{2}$ edges. Then the characteristic polynomial of central graph of $G$ is

Proof. Let $I(G)$ be the incidence matrix of $G$ and $m = \frac{nr}{2}$. Then by a proper labeling of vertices, the adjacency matrix of $C(G)$ can be written as

The characteristic polynomial of $C(G)$ is

By Lemma 2.1, we have

By Lemma 2.2, we have

Corollary 3.2. Let $G$ be an $r$-regular graph on $n$ vertices and $\frac{nr}{2}$ edges. Then the spectrum of central graph of $G$ is

i = 2, ..., n.

Corollary 3.3. Let $G$ be an $r$-regular graph on $n$ vertices and $\frac{nr}{2}$ edges. Then the spectrum of central graph of $K_n$ is

Theorem 3.4. Let $G$ be an $r$-regular graph on $n$ vertices and $\frac{nr}{2}$ edges. Then the Laplacian characteristic polynomial of central graph of $G$ is

Proof. Let $I(G)$ be the incidence matrix of $G$ and $m = \frac{nr}{2}$. Then by a proper labeling of vertices, the Laplacian matrix of $C(G)$ can be written as

The Laplacian characteristic polynomial of $C(G)$ is

By Lemmas 2.1 and 2.2, we have

Corollary 3.5. Let $G$ be an $r$-regular graph on $n$ vertices and $\frac{nr}{2}$ edges. Then the Laplacian spectrum of central graph of $G$ is

for i = 2, ..., n.

By Corollary 3.5, we can readily obtain the following result.

Corollary 3.6. Let $G$ be an $r$-regular graph on $n$ vertices and $\frac{nr}{2}$ edges. Then the number of spanning trees of central graph of $G$ is

Corollary 3.7. Let $G$ be an $r$-regular graph on $n$ vertices and $\frac{nr}{2}$ edges. Then the Kirchhoff index of central graph of $G$ is

Theorem 3.8. Let $G$ be an $r$-regular graph on $n$ vertices and $\frac{nr}{2}$ edges. Then the signless Laplacian characteristic polynomial of central graph of $G$ is

Proof. Let $I(G)$ be the incidence matrix of $G$ and $m = \frac{nr}{2}$. Then by a proper labeling of vertices, the signless Laplacian matrix of $C(G)$ can be written as

The characteristic polynomial of $Q(C(G))$ is

By Lemmas 2.1 and 2.2, we have

Corollary 3.9. Let $G$ be an $r$-regular graph on $n$ vertices and $\frac{nr}{2}$ edges. Then the signless Laplacian spectrum of central graph of $G$ is

for i = 2, ..., n.

4.   Spectra of two new joins of graphs

In this section, we define two new joins namely the central vertex join and central edge join of two graphs and compute their spectra. Moreover, we determine the number of spanning trees and Kirchhoff index of central vertex join and central edge join of two regular graphs.

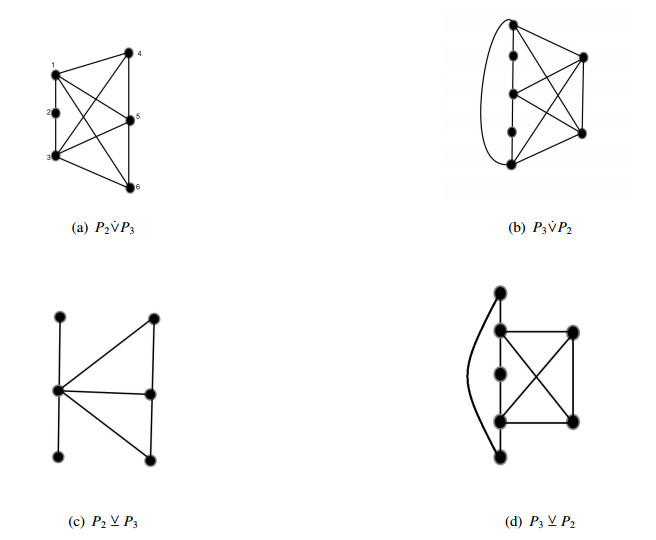
Definition 4.1. Let $G_1$ and $G_2$ be any two graphs on $n_1, n_2$ vertices and $m_1, m_2$ edges respectively. The central vertex join of $G_1$ and $G_2$ is the graph $G_1\dot{\vee} G_2$, is obtained from $C(G_1)$ and $G_2$ by joining each vertex of $G_1$ with every vertex of $G_2$.

Note that the central vertex join $G_1\dot{\vee} G_2$ has $m_1+n_1+n_2$ vertices and $m_1+m_2+n_1n_2+\frac{n_1(n_1-1)}{2}$ edges.

Definition 4.2. Let $G_i$ be a graph with $n_i$ vertices and $m_i$ edges for ${i = 1, 2}.$ Then the central edge join of two graphs $G_1$ and $G_2$ is the graph $G_1\veebar G_2$ is obtained from $C(G_1)$ and $G_2$ by joining each vertex corresponding to edges of $G_1$ with every vertex of $V(G_2)$.

Note that the central edge join $G_1\veebar G_2$ has $m_1+n_1+n_2$ vertices and $m_1+m_2+m_1n_2+\frac{n_1(n_1-1)}{2}$ edges.

Example 4.1. Let $G_1 = P_2$ and $G_2 = P_3$. Then the central vertex join $G_1\dot{\vee} G_2$ and central edge join $G_1\veebar G_2$ are depicted in Figure 1.

The next theorem gives the adjacency characteristic polynomial of $G_1\dot{\vee} G_2$ and $G_1\veebar G_2$, where $G_i$ is $r_i$-regular graph for ${i = 1, 2}.$

Theorem 4.1. Let $G_i$ be an $r_i$-regular graph with $n_i$ vertices and $m_i$ edges for ${i = 1, 2}.$ Then the adjacency characteristic polynomial of $G_1\dot{\vee} G_2$ is

Proof. Let $I(G_1)$ be the incidence matrix of $G_1.$ Then by a proper labeling of vertices, the adjacency matrix of $G_1\dot{\vee} G_2$ can be written as

The characteristic polynomial of $G_1\dot{\vee} G_2$ is

By Lemmas 2.1, 2.2, Definition 2.1 and Corollary 2.6, we have

Therefore the characteristic polynomial of $G_1\dot{\vee} G_2$ is

The following corollary describes the complete spectrum of $G_1\dot{\vee} G_2$ when $G_1$ and $G_2$ are regular graphs.

Corollary 4.2. Let $G_i$ be an $r_i$-regular graph with $n_i$ vertices and $m_i$ edges for ${i = 1, 2}.$ Then the adjacency spectrum of $G_1\dot{\vee} G_2$ consists of

1. $0$ with multiplicity $m_1-n_1$.

2. $\lambda_j(G_2), \; j = 2, 3, ..., n_2.$

3. Two roots of the equation $x^2+x+\lambda_i(G_1)x-(r_1+\lambda_i(G_1)) = 0 \; for\; i = 2, ..., n_1.$

4. Three roots of the equation $x^3+(-n_1+r_1+1-r_2)x^2+(-2r_1+r_2n_1-r_2-r_1r_2-n_1n_2)x+2r_1r_2 = 0.$

Corollary 4.3. Let $G_1$ be an $r_1$-regular graph with $n_1$ vertices and $m_1$ edges. Then the adjacency spectrum of $G_1\dot{\vee}K_{p, q}$ consists of

1. $0$ with multiplicity $m_1-n_1+p+q-2$.

2. Two roots of the equation $x^2+x+\lambda_i(G_1)x-(r_1+\lambda_i(G_1)) = 0\; for\; i = 2, ..., n_1.$

3. Four roots of the equation $x^4+(-n_1+r_1-pn_1-qn_1)x^3+(-2r_1-pq)x^2+(3pqn_1-pqr_1-pq)x+2pqn_1 = 0.$

The following corollary describes a construction of A-cospectral graphs.

Corollary 4.4. $(a)$ Let $G_1$ and $G_2$ be $A$-cospectral regular graphs and $H$ is a regular graph. Then $H\dot{\vee} G_1$ and $H\dot{\vee} G_2$ are $A$-cospectral.

$(b)$ Let $G_1$ and $G_2$ be $A$-cospectral regular graphs and $H$ is a regular graph. Then $G_1 \dot{\vee} H$ and $G_2 \dot{\vee} H$ are $A$-cospectral.

$(c)$ Let $G_1$ and $G_2$ be A-cospectral regular graphs, $H_1$ and $H_2$ are another A-cospectral regular graphs. Then $G_1 \dot{\vee} H_1$ and $G_2 \dot{\vee} H_2$ are A-cospectral.

Theorem 4.5. Let $G_i$ be an $r_i$-regular graph with $n_i$ vertices and $m_i$ edges for ${i = 1, 2}.$ Then the adjacency characteristic polynomial of $G_1\veebar G_2$ is

Proof. Let $I(G_1)$ be the incidence matrix of $G_1.$ Then by a proper labeling of vertices, the adjacency matrix of $A(G_1\veebar G_2)$ can be written as

The characteristic polynomial of $G_1\veebar G_2$ is

By Lemmas 2.1, 2.2, 2.7, Definition 2.1 and Corollary 2.6, we have

Thus the adjacency characteristic polynomial of $G_1\veebar G_2$ is

The following corollary describes the complete spectrum of $G_1\veebar G_2$ when $G_1$ and $G_2$ are regular graphs.

Corollary 4.6. Let $G_i$ be an $r_i$-regular graph with $n_i$ vertices and $m_i$ edges for ${i = 1, 2}.$ Then the adjacency spectrum of $G_1\veebar G_2$ consists of

1. $0$ with multiplicity $m_1-n_1-1$.

2. $\lambda_j(G_2)\; for\; j = 2, 3, ..., n_2.$

3. Two roots of the equation $x^2+x+\lambda_i(G_1)x-(r_1+\lambda_i(G_1)) = 0\; for\; i = 2, ..., n_1.$

4. Four roots of the equation $\Big(x^2+(-n_1+1+r_1)x-2r_1\Big)\Big(x^2-r_2x-m_1n_2\Big)-n_1n_2r_1^2 = 0.$

Corollary 4.7. Let $G_1$ be an $r_1$-regular graph with $n_1$ vertices and $m_1$ edges. Then the adjacency spectrum of $G_1\veebar K_{p, q}$ consists of

1. $0$ with multiplicity $m_1-n_1+p+q-2$.

2. Two roots of the equation $x^2+x+\lambda_i(G)x-(r_1+\lambda_i(G)) = 0\; for\; i = 2, ..., n_1.$

4. Four roots of the equation $x^4+(-m_1(p+q)-pq)x^2-2pqx-(p+q)n_1r_1^2 = 0.$

The following corollary is useful to construct non-isomorphic A-cospectral graphs.

Corollary 4.8. $(a)$ Let $G_1$ and $G_2$ be $A$-cospectral regular graphs and $H$ is a regular graph. Then $H\veebar G_1$ and $H\veebar G_2$ are $A$-cospectral.

$(b)$ Let $G_1$ and $G_2$ be $A$-cospectral regular graphs and $H$ is a regular graph. Then $G_1 \veebar H$ and $G_2 \veebar H$ are $A$-cospectral.

$(c)$ Let $G_1$ and $G_2$ be A-cospectral regular graphs, $H_1$ and $H_2$ are another A-cospectral regular graphs. Then $G_1 \veebar H_1$ and $G_2 \veebar H_2$ are A-cospectral.

Theorem 4.9. Let $G_1$ be an $r_1$-regular graph with $n_1$ vertices and $m_1$ edges and $G_2$ be an arbitrary graph with $n_2$ vertices and $m_2$ edges. Then the Laplacian characteristic polynomial of $G_1\dot{\vee} G_2$ is

Proof. Let $L(G_2)$ be the Laplacian matrix of $G_2$. Then by a proper labeling of vertices, the Laplacian matrix of $G_1\dot{\vee} G_2$ can be written as

The characteristic polynomial of $G_1\dot{\vee} G_2$ is \\

By Lemma 2.1 and Definition 2.1, we have

Using Definition 2.1, Lemma 2.1 and Corollary 2.6, we have

Again using Definition 2.1, we have

Note that $\mu_1(G_2) = 0$ and $\lambda_i(G_1) = r_i-\mu_i(G_1), i = 1, 2, ..., n.$ Thus the characteristic polynomial of $L(G_1\dot{\vee} G_2)$

The following corollary describes the complete Laplacian spectrum of $G_1\dot{\vee} G_2$ when $G_1$ is a regular graph and $G_2$ is an arbitrary graph.

Corollary 4.10. Let $G_1$ be an $r_1$-regular graph with $n_1$ vertices and $m_1$ edges and $G_2$ be an arbitrary graph with $n_2$ vertices and $m_2$ edges. Then the Laplacian spectrum of $G_1\dot{\vee} G_2$ consists of

1. $2$ with multiplicity $m_1-n_1$.

2. $n_1+\mu_j(G_2) \; for\; j = 2, 3, ..., n_2.$

3. Three roots of the equation $x^3-(n_2 +r_1+n_1+2)x^2+(2n_1+2n_2+n_1r_1)x = 0.$

4. Two roots of the equation $x^2-x(n_1+n_2+\lambda_i(G_1)+2)+2n_1+2n_2+\lambda_i(G_1)-r_1 = 0\; for\; i = 2, ..., n_1.$

As an application for Theorem 4.9 we give the expression for the number of spanning trees and Kirchhoff index of $G_1\dot{\vee} G_2$, for an $r_1$-regular graph $G_1$ and an arbitrary graph $G_2$.

Corollary 4.11. Let $G_1$ be an $r_1$-regular graph with $n_1$ vertices and $m_1$ edges and $G_2$ be an arbitrary graph with $n_2$ vertices and $m_2$ edges. Then the number of spanning trees of $G_1\dot{\vee} G_2$ is

Corollary 4.12. Let $G_1$ be an $r_1$-regular graph with $n_1$ vertices and $m_1$ edges and $G_2$ be an arbitrary graph with $n_2$ vertices and $m_2$ edges. Then the Kirchhoff index of $G_1\dot{\vee} G_2$ is

The following corollary describes a construction of L-cospectral graphs.

Corollary 4.13. $(a)$ Let $G_1$ and $G_2$ be $L$-cospectral regular graphs and $H$ is an arbitarary graph. Then $H\dot{\vee} G_1$ and $H\dot{\vee} G_2$ are $L$-cospectral.

$(b)$ Let $G_1$ and $G_2$ be $L$-cospectral regular graphs and $H$ is an arbitrary graph. Then $G_1 \dot{\vee} H$ and $G_2 \dot{\vee} H$ are $L$-cospectral.

$(c)$ Let $G_1$ and $G_2$ be L-cospectral regular graphs, $H_1$ and $H_2$ are another L-cospectral regular graphs. Then $G_1 \dot{\vee} H_1$ and $G_2 \dot{\vee} H_2$ are L-cospectral.

Theorem 4.14. Let $G_1$ be an $r_1$-regular graph with $n_1$ vertices and $m_1$ edges and $G_2$ be an arbitrary graph with $n_2$ vertices and $m_2$ edges. Then the Laplacian characteristic polynomial of $G_1\veebar G_2$ is

Proof. Let $L(G_2)$ be the Laplacian matrix of $G_2.$ Then by a suitable labeling of the vertices, the Laplacian matrix of $G_1\veebar G_2$ can be written as

The Laplacian characteristic polynomial of $G_1\veebar G_2$ is

By Lemma 2.1, we have

From Definition 2.1, Lemmas 2.1, 2.7 and Corollary 2.6, we have

After simplifying we get

The following corollary describes the complete Laplacian spectrum of $G_1\veebar G_2$ when $G_1$ is a regular graph and $G_2$ is an arbitrary graph.

Corollary 4.15. Let $G_1$ be an $r_1$-regular graph with $n_1$ vertices and $m_1$ edges and $G_2$ be an arbitrary graph with $n_2$ vertices and $m_2$ edges. Then the Laplacian spectrum of $G_1\veebar G_2$ consists of

1. $2+n_2$ with multiplicity $m_1-n_1-1$.

2. $m_1+\mu_j(G_2) \; for\; j = 2, 3, ..., n_2.$

3. Two roots of the equation $x^2-(n_1+\lambda_i(G_1)+2+n_2)x +2n_1+\lambda_i(G_1)+n_1n_2+n_2\lambda_i(G_1)-r_1 = 0 \; for\; i = 2, ..., n_1.$

4. Four roots of the equation $((x-2-n_2)(x-r_1)-2r_1)(x^2-(2+n_2+m_1)x+2m_1)-r_1^2n_1n_2 = 0.$

Corollary 4.16. Let $G_1$ be an $r_1$-regular graph with $n_1$ vertices and $m_1$ edges and $G_2$ be an arbitrary graph with $n_2$ vertices and $m_2$ edges. Then the number of spanning trees of $G_1\veebar G_2$ is

Corollary 4.17. Let $G_1$ be an $r_1$-regular graph with $n_1$ vertices and $m_1$ edges and $G_2$ an arbitrary graph with $n_2$ vertices and $m_2$ edges. Then the Kirchhoff index of $G_1\veebar G_2$ is

The following corollary describes a construction of L-cospectral graphs.

Corollary 4.18. $(a)$ Let $G_1$ and $G_2$ be $L$-cospectral regular graphs and $H$ is a regular graph. Then $H\veebar G_1$ and $H\veebar G_2$ are $L$-cospectral.

$(b)$ Let $G_1$ and $G_2$ be $L$-cospectral regular graphs and $H$ is an arbitrary graph. Then $G_1 \veebar H$ and $G_2 \veebar H$ are $L$-cospectral.

$(c)$ Let $G_1$ and $G_2$ be L-cospectral regular graphs, $H_1$ and $H_2$ are another L-cospectral regular graphs. Then $G_1 \veebar H_1$ and $G_2 \veebar H_2$ are L-cospectral.

Theorem 4.19. Let $G_i$ be an $r_i$-regular graph with $n_i$ vertices and $m_i$ edges for ${i = 1, 2}.$ Then the signless Laplacian characteristic polynomial of $G_1\dot{\vee} G_2$ is

Proof. Let $Q(G_2)$ be the signless Laplacian matrix of $G_2$. Then by a proper labeling of vertices, the Laplacian matrix of $G_1\dot{\vee} G_2$ can be written as

The signless Laplacian characteristic polynomial of $G_1\dot{\vee} G_2$ is

By using the same arguments as in the proof of Theorem 4.9, we get the desired result.

Corollary 4.20. Let $G_i$ be an $r_i$-regular graph with $n_i$ vertices and $m_i$ edges for ${i = 1, 2}.$ Then the signless Laplacian spectrum of $G_1\dot{\vee} G_2$ consists of

1. $2$ with multiplicity $m_1-n_1$.

2. $n_1+\nu_j(G_2) \; for\; j = 2, 3, ..., n_2.$

3. Three roots of the equation $(x-n_1-2r_1)(x^2-(2n_1+n_2-r_1)x+4n_1+2n_2-4-4r_1)-n_1^2(x-2) = 0.$

4. Two roots of the equation $(x-2)\big(x-n_1-n_2+2+\lambda_i(G_1)\big)+\nu_i(G_1) = 0.\; for\; i = 2, ..., n_1.$

The following corollary describes a construction of Q-cospectral graphs.

Corollary 4.21. $(a)$ Let $G_1$ and $G_2$ be $Q$-cospectral regular graphs and $H$ is a regular graph. Then $H\dot{\vee} G_1$ and $H\dot{\vee} G_2$ are $Q$-cospectral.

$(b)$ Let $G_1$ and $G_2$ be $Q$-cospectral regular graphs and $H$ is a regular graph. Then $G_1 \dot{\vee} H$ and $G_2 \dot{\vee} H$ are $Q$-cospectral.

$(c)$ Let $G_1$ and $G_2$ be Q-cospectral regular graphs, $H_1$ and $H_2$ are another Q-cospectral regular graphs. Then $G_1 \dot{\vee} H_1$ and $G_2 \dot{\vee} H_2$ are Q-cospectral.

Theorem 4.22. Let $G_i$ be an $r_i$-regular graph with $n_i$ vertices and $m_i$ edges for ${i = 1, 2}.$ Then the signless Laplacian characteristic polynomial of $G_1\veebar G_2$ is

Proof. Let $Q(G_2)$ be the signless Laplacian matrix of $G_2.$ Then by a suitable labeling of the vertices, the signless Laplacian matrix of $G_1\veebar G_2$ can be written as

The signless Laplacian characteristic polynomial of $G_1\veebar G_2$ is

By using the same arguments as in the proof of Theorem 4.14, we get the desired result.

Corollary 4.23. Let $G_i$ be an $r_i$-regular graph with $n_i$ vertices and $m_i$ edges for ${i = 1, 2}.$ Then the signless Laplacian spectrum of $G_1\veebar G_2$ consists of

1. $2+n_2$ with multiplicity $m_1-n_1-1$.

2. $m_1+\nu_j(G_2) \; for\; j = 2, 3, ..., n_2.$

3. Two roots of the equation $(x-2-n_2)\big(x-n_1+2+\lambda_i(G_1)\big)-\lambda_i(G_1)-r_1 = 0 \; for\; i = 2, ..., n_1.$

4. Four roots of the equation $\bigg((x^2-x(2n_1-2-r_1+2+n_2)+4n_1-4-4r_1+2n_1n_2-2n_2-n_2r_1-2r_1)(x^2-(2r_1+2+n_2+m_1)x+2m_1+4r_1+2r_1n_2)-n_1n_2r_1^2\bigg) = 0.$

By Theorem 4.22 enables us to construct infinitely many pairs of $Q$-cospectral graphs.

Corollary 4.24. $(a)$ Let $G_1$ and $G_2$ be $Q$-cospectral regular graphs and $H$ is a regular graph. Then $H\veebar G_1$ and $H\veebar G_2$ are $Q$-cospectral.

$(b)$ Let $G_1$ and $G_2$ be $Q$-cospectral regular graphs and $H$ is a regular graph. Then $G_1 \veebar H$ and $G_2 \veebar H$ are $Q$-cospectral.

$(c)$ Let $G_1$ and $G_2$ be Q-cospectral regular graphs, $H_1$ and $H_2$ are another Q-cospectral regular graphs. Then $G_1 \veebar H_1$ and $G_2 \veebar H_2$ are Q-cospectral.

Construction of integral graphs are very difficult. Here we present an infinite family of integral graphs.

5.   Some new integral graphs

The following propositions give the necessary and sufficient condition for the $G_1\dot{\vee} G_2$ and $G_1\veebar G_2$ are A-integral graphs.

Proposition 5.1. Let $G_1$ be an $r_1$-regular graph with $n_1$ vertices and $G_2$ be an $r_2$-regular graph with $n_2$ vertices. Then $G_1\dot{\vee} G_2$ is A-integral graph if and only if $G_2$ is A-integral and the roots of the equations $x^2+x+\lambda_i(G_1)x-(r_1+\lambda_i(G_1)) = 0 \; for\; i = 2, ..., n_1$ and $x^3+(-n_1+r_1+1-r_2)x^2+(-2r_1+r_2n_1-r_2-r_1r_2)x-2r_1r_2-n_1n_2 = 0$ are integers.

Proposition 5.2. Let $G_1$ be an $r_1$-regular graph with $n_1$ vertices and $G_2$ be an $r_2$-regular graph with $n_2$ vertices. Then $G_1\veebar G_2$ is A-integral graph if and only if $G_2$ is A-integral and the roots of the equations $x^2+x+\lambda_i(G_1)x-(r_1+\lambda_i(G_1)) = 0 \; for\; i = 2, ..., n_1$ and $\big(x^2+x(-n_1+1+r_1)-2r_1\big)(x^2-r_2x-n_1n_2r_1^2) = 0$ are integers.

The following are integral graphs.

1. From Corollary 3.3, we have $C(K_n)$ is A-integral if and only if $2n-2$ and $n-2$ are perfect squares. For example $C(K_3), C(K_{51}), C(K_{1683}), C(K_{57123})$ are A-integral graphs.

2. $C(K_{n, n})$ is A-integral if and only if $1+8n$ and $1+4n$ are perfect squares.

For example $C(K_{6, 6})$ and $C(K_{210,210}), C(K_{242556, 242556})$ are A-integral graphs.

3. Let $G$ be an $r$-regular A-integral graph with $n$ vertices and $l$ is a non negative integer. Then $lK_1\dot{\vee} G$ is A-integral if and only if the roots of the equation $x^3+(-l+1-r)x^2+r(l-1)x-nl = 0$ are integers.

4. Let $G$ be an $r$-regular A-integral graph with $n$ vertices. Then $G\dot{\vee} K_{n^2}$ is A-integral if and only if the roots of the equation $x^3-rx^2-2(n-1)x-2r(n-1) = 0$ are integers.

5. Let $G$ be an $r$-regular A-integral graph and $l$ is a non negative integer. Then $lK_1{\veebar } G$ is A-integral if and only if $\frac{l-1-r\pm\sqrt{(l-1-r)^2+8r}}{2}$ is an integer.

6. Let $G$ be an $r$-regular A-integral graph with $n$ vertices. Then $\bar K_{n}{\veebar G }$ is A-integral.

7. Let $G$ be a complete graph on $n$ vertices. Then $C(K_n)$ is Laplacian and signless Laplacian integral for every $n$.

6.   Conclusion

In this paper, we determine the different spectra of central graphs. Also, we define two new joins of graphs namely central vertex join and central edge join and obtain their spectra. As applications, these results enable us to construct infinitely many pairs of $A$-cospectral, $L$-cospectral and $Q$-cospectral graphs. In addition, we discussed the number of spanning trees and Kirchhoff index of central graphs, central vertex join and central edge join of graphs. Using the spectra of central graphs, central vertex join and central edge join of regular graphs, a new infinite family of A-integral (L-integral, Q-integral) graphs is obtained.

Conflict of interest

Authors declare there is no conflicts of interest in this paper.