Mild solutions for a multi-term fractional differential equation via resolvent operators

1.   Introduction

In the last two decades, differential equations involving fractional derivatives, have been used in many mathematical models to describe a wide variety of phenomena, including problems in viscoelasticity, signal and image processing, engineering, economics, epidemiology and among others, and the study of this kind of equations has been a topic of interest in recent years. See ^{[9,16,19,25,37,41,42,43,45]} and the references therein.

In this paper, we consider the following multi-term fractional differential equations

and

where $A$ is a closed linear operator defined in a Banach space $X, \; 1 < \alpha, \beta < 2, \; T > 0,$ and $f$ is a suitable continuous function. Here, for $\gamma > 0$ the derivatives $\partial^\gamma u$ and $\partial^\gamma_t u,$ denote the Weyl and Caputo fractional derivatives, respectively.

Although the definition of the fractional derivatives in the sense of Weyl (defined on $\mathbb{R}$) and Caputo (defined on $[0, \infty))$ are different, we notice that the mild solution to Eqs (1.1) and (1.2) can be written in terms of the same resolvent family. In fact, if $A$ is the generator of the fractional resolvent family $\{S_{\alpha, 1}(t)\}_{t\geq 0}$ (see its definition in Section 2) then the mild solutions to Eqs (1.1) and (1.2) are defined, respectively, by

and

where $x = u(0)$ and $y = u'(0)$ are the initial conditions in Eq (1.2), and the families $\{S_{\alpha, \beta}(t)\}_{t\geq 0},$ and $\{S_{\alpha, 2}(t)\}_{t\geq 0},$ are given respectively by

Here, the $\ast$ denotes the usual finite convolution and for $\gamma > 0$ the function $g_\gamma$ is defined by $g_\gamma(t): = t^{\gamma-1}/\Gamma(\gamma),$ where $\Gamma(\cdot)$ is the Gamma function. The fractional resolvent family $\{S_{\alpha, 1}(t)\}_{t\geq 0}$ is defined by

where $\Gamma$ is a suitable complex path where the resolvent operator $(\lambda^\alpha-A)^{-1}$ is well defined. By the uniqueness of the Laplace transform it is easy to see that

for all $t\geq 0.$ The existence of mild solutions to Eq (1.1) in case $\beta = 1$ has been widely studied in the last years, see for instance ^[4,12,13,24] and references therein. In these mentioned papers, the operator $A$ is assumed to be an $\omega$-sectorial operator of angle $\theta$ (see definition in Section 2). In this case, $A$ generates a resolvent family $\{E_{\alpha}(t)\}_{t\geq 0}$ (see ^[11,28]) which satisfies

where $C$ is a positive constant depending only on $\alpha$ and $\theta.$ This decay of $\{E_{\alpha}(t)\}_{t\geq 0}$ provides also some tools to obtain many and interesting consequences on the study of qualitative properties of solutions to fractional (and integral) differential (and difference) equations. See for instance ^{[4,7,8,29,31,44]} and the references therein for further details. We notice that, by the uniqueness of the Laplace transform, the resolvent families $\{E_\alpha(t)\}_{t\geq 0}$ and $\{S_{\alpha, 1}(t)\}_{t\geq 0}$ are the same for $1 < \alpha < 2.$

On the other hand, the existence of mild solutions to fractional differential equations with nonlocal conditions has been studied by several authors in the last years. The concept of nonlocal initial condition was introduced by L. Byszewski ^[6] to extend the study of classical initial value problems. This notion results more suitable to describe more precisely several phenomena in applied sciences, because it considers additional information in the initial data. More concretely, the nonlocal conditions have the form $u(0)+g(u) = u_0$ instead $u(0) = u_0,$ where $g$ is an appropriate function that represents the additional information in the system and provides a better description of the initial state of the system than the classical initial value problem. The theory of nonlocal Cauchy problems has been developed rapidly and has been studied widely in the last years, see for instance ^[3,33,38] and the references therein for more details.

There exists a wide recent literature on the existence of mild solutions to fractional differential equations with nonlocal initial conditions. More specifically, the problem

where $T > 0, \; A$ is a closed linear operator defined in a Banach space $X, \; 0 < \alpha\leq 1, \; u_0\in X, \; f$ is a suitable semilinear continuous function has been studied extensively in recent years. See for instance ^{[1,2,10,26,30,35]}. Since the fractional derivative $\partial_t^\alpha$ for $\alpha = 1$ is the usual derivative $\frac{d}{dt},$ the case $\alpha = 1$ in (1.3) corresponds precisely to the semilinear Cauchy problem introduced in the seminal paper ^[6] and the theory of $C_0$-semigroups of linear operators is the main tool to obtain the existence of solutions in this case. Similarly, for $\alpha > 0$ the theory of fractional resolvent families represents one of the main tools to study the existence of mild solutions to (1.3). Indeed, if $0 < \alpha\leq 1$ and $A$ generates a resolvent family $\{S_{\alpha, \alpha}(t)\}_{t\geq 0},$ then the mild solution to (1.3) is given by

where $S_{\alpha, 1}(t): = (g_{1-\alpha}\ast S_{\alpha, \alpha})(t),$ see for instance ^[30]. We notice that the variation of constant formula (1.4) coincides with the case $\alpha = 1$ introduced in [6,Section 3]. Similarly, for $1 < \alpha < 2$ and $\beta = 1$ or $\beta = \alpha,$ the Eq (1.2) subject to the nonlocal conditions $u(0)+g(u) = u_0,$ and $u'(0)+h(u) = u_1,$ where $g, h:C(I, X) \to X$ are continuous and $u_0, u_1$ belong to $X,$ ($I: = [0, T]$) has been considered by several authors in the last years. See for instance ^[2,22] for the case $\beta = 1$ and ^[33,34] in case $\beta = \alpha.$

In this paper, our concern is the study of existence of mild solutions to the fractional differential Eqs (1.1) and (1.2). Here, we assume certain conditions on the operator $A$ and on the parameters $\alpha$ and $\beta$ in order to ensure that $A$ is the generator of a fractional resolvent family $\{S_{\alpha, \beta}(t)\}_{t\geq0}.$

More specifically, in Eq (1.1) we consider the Weyl fractional derivative, because it is defined for functions on $\mathbb{R}.$ More precisely, we show that if the function $f$ in (1.1) is an almost periodic or an almost automorphic (among others) vector-valued function, then the Eq (1.1) has a unique almost periodic or almost automorphic function mild solution, respectively, which is given in terms of $\{S_{\alpha, \beta}(t)\}_{t\geq0}.$

On the other hand, in Eq (1.2) the derivative is taken in the sense of Caputo, because it is defined on the positive real axis $[0, \infty).$ Under the the nonlocal conditions $u(0)+g(u) = u_0,$ and $u'(0)+h(u) = u_1$ we prove that (1.2) has at least one mild solution. Here, the properties of the fractional resolvent family $\{S_{\alpha, \beta}(t)\}_{t\geq 0}$ are again an important tool to obtain the result.

This paper is organized as follows. The Section 2 gives the preliminaries on fractional calculus, sectorial operators, fractional resolvent families and some subspaces of bounded and continuous functions. Section 3 is devoted to the existence of mild solutions to (1.1). In Section 4 is studied the existence of mild solutions to the nonlocal problem (1.2). Finally, in Section 5 we give some examples.

2.   Preliminaries

For a Banach space $(X, \|\cdot\|),$ the space of all bounded and linear operators form $X$ into $X$ is denoted by $\mathcal{B}(X).$ If $A$ is a closed linear operator defined on $X$ we denote by $\rho(A)$ the resolvent set of $A$ and $R(\lambda, A) = (\lambda-A)^{-1}$ its resolvent operator, which is defined for all $\lambda\in\rho(A).$ For $1\leq p < \infty, \; L^p(\mathbb{R}_+, X)$ denotes the space of all Bochner measurable functions $g:\mathbb{R}_+\to X$ such that

We recall that a strongly continuous family $\{S(t)\}_{t\geq 0}\subset \mathcal{B}(X)$ is said to be exponentially bounded if there exist two constants $M > 0$ and $w\in\mathbb R$ such that $\|S(t)\|\leq Me^{wt}$ for all $t > 0.$

A closed and densely defined operator $A,$ defined on a Banach space $(X, \|\cdot\|),$ is said to be $\omega$ -sectorial of angle $\phi,$ if there exist $\phi\in [0, \pi/2)$ and $\omega\in \mathbb{R}$ such that its resolvent exists in the sector $\omega+\Sigma_\phi: = \left\{\omega+\lambda: \lambda\in \mathbb{C}, |\arg(\lambda)| < \frac{\pi}{2}+\phi\right\}\setminus\{\omega\}$ and $\|R(\lambda, A)\|\leq \frac{M}{|\lambda-\omega|}$ for all $\lambda \in \omega+\Sigma_\phi.$ See ^[17] and ^[18] for further details.

Now, we review some results on fractional calculus. We recall that for $\gamma > 0,$ the function $g_\gamma$ is defined by $g_\gamma(t) = \frac{t^{\gamma-1}}{\Gamma(\gamma)}$ for all $t\geq 0.$ For $\gamma > 0, \; \lceil \gamma \rceil$ denotes the smallest integer greater than or equal to $\gamma$, and $[\gamma]$ denotes the integer part of $\gamma$. As usual, the finite convolution of $f$ and $g$ is defined by $(f\ast g)(t) = \int_0^t f(t-s)g(s)ds.$

Definition 1. Let $\alpha > 0$ and $n = \lceil \alpha \rceil.$ The Caputo fractional derivative of order $\alpha$ of a function $u:[0, \infty)\to X$ is defined by

Definition 2. Let $\alpha > 0$ and $n = [ \alpha ]+1.$ The Weyl fractional derivative of order $\alpha$ of a function $u: \mathbb{R}\to X$ is defined by

where for $\gamma > 0, \; \partial^{-\gamma} u(t): = \int_{-\infty}^t g_\gamma(t-s)u(s)ds$ for all $t\in \mathbb{R}.$

It is a well known fact that if $\alpha\in\mathbb{N},$ then $\partial_t^n = \partial^n = \frac{d^n}{dt^n},$ that is, the Caputo and Weyl fractional derivatives coincide with the usual derivative if $\alpha\in\mathbb{N}.$ Moreover, if $\alpha, \beta\in \mathbb{R},$ then $\partial^\alpha \partial^\beta u = \partial^\beta\partial^\alpha u = \partial^{\alpha+\beta}u.$ See ^[25,41] and ^[42] for more details and applications on fractional differential calculus.

Now, we recall the resolvent families of operators generated by an operator $A.$

Definition 3. Let $A$ be closed linear operator with domain $D(A),$ defined on a Banach space $X, \; 1\leq\alpha\leq 2$ and $0 < \beta\leq 2.$ We say that $A$ is the generator of an $(\alpha, \beta)$-resolvent family, if there exists $\nu\geq 0$ and a strongly continuous and exponentially bounded function $S_{\alpha, \beta}: [0, \infty)\to \mathcal{B}(X)$ such that $\left\{\lambda^\alpha: {\rm Re} \lambda > \nu\right\}\subset \rho(A),$ and for all $x\in X,$

In this case, $\{S_{\alpha, \beta}(t)\}_{t\geq 0}$ is called the $(\alpha, \beta)$-resolvent family generated by $A.$

If we compare Definition 3 with the notion of $(a, k)$-regularized families introduced in ^[21], then we notice that $t\mapsto S_{\alpha, \beta}(t),$ is a $(g_\alpha, g_\beta)$-regularized family. Moreover, the family $\{S_{\alpha, \beta}(t)\}_{t\geq 0}$ is well known in some cases. For example, $S_{1, 1}(t)$ is a $C_0$-semigroup, $S_{2, 1}(t),$ corresponds to a cosine family and $S_{2, 2}(t)$ is a sine family. In the scalar case, that is, when $A = \varrho\mathcal{I},$ where $\varrho\in \mathbb{C}$ and $\mathcal{I}$ denotes the identity operator, then by the uniqueness of the Laplace transform, $S_{\alpha, \beta}(t)$ corresponds to the function $t^{\beta-1}E_{\alpha, \beta}(\varrho t^\alpha),$ where for $z\in\mathbb{C}$ the generalized Mittag-Leffler function is defined by $E_{\alpha, \beta}(z) = \sum_{k = 0}^\infty\frac{z^k}{\Gamma(\alpha k+\beta)},$ see for instance ^[39,40]. See also ^[41] and ^[42] for an interesting and recent discussion on the theory of general fractional derivatives and its applications.

We have also the following result. Its proof follows similarly as in [20,Proposition 3.7].

Proposition 4. Let $1\leq\alpha, \beta\leq 2.$ Let $S_{\alpha, \beta}(t)$ be the $(\alpha, \beta)$-resolvent family generated by $A.$ Then:

1. $S_{\alpha, \beta}(t)x\in D(A)$ and $S_{\alpha, \beta}(t)Ax = AS_{\alpha, \beta}(t)x$ for all $x\in D(A)$ and $t\geq 0$.

2. If $x\in D(A)$ and $t\geq 0,$ then

3. If $x\in X, t\geq0,$ then $\int_0^t g_\alpha(t-s)S_{\alpha, \beta}(s)xds\in D(A)$ and $S_{\alpha, \beta}(t)x = g_\beta(t)x+A\int_0^t g_\alpha(t-s)S_{\alpha, \beta}(s)xds.$

In particular, $S_{\alpha, \beta}(0) = g_\beta(0)\mathcal{I}$.

The next result gives sufficient conditions on $\alpha, \beta$ and $A$ to obtain generators of $(\alpha, \beta)$-resolvent families.

Theorem 5. ^[28] Let $1 < \alpha < 2$ and $\beta\geq 1$ such that $\alpha-\beta+1 > 0.$ Assume that $A$ is $\omega$-sectorial of angle $\frac{(\alpha-1)\pi}{2},$ where $\omega < 0.$ Then $A$ generates an exponentially bounded $(\alpha, \beta)$-resolvent family.

Theorem 6. ^[28] Let $1 < \alpha < 2$ and $\beta\geq 1$ such that $\alpha-\beta+1 > 0.$ Assume that $A$ is $\omega$-sectorial of angle $\frac{(\alpha-1)}{2}\pi,$ where $\omega < 0.$ Then, there exists a constant $C > 0,$ depending only on $\alpha$ and $\beta,$ such that

Finally, we recall some spaces of functions. For a given Banach space $(X, \|\cdot\|),$ let $BC(X): = \{f:\mathbb{R}\to X: \|f\|_\infty: = \sup_{t\in\mathbb{R}}\|f(t)\| < \infty\}$ be the Banach space of all bounded and continuous functions. For $T > 0$ fixed, $P_T(X)$ denotes the space of all vector-valued periodic functions, that is, $P_T(X): = \{f\in BC(X):f(t+T) = f(t), \mbox{ for all } t\in\mathbb{R}\}.$ We denote by $AP(X)$ to the space of all almost periodic functions (in the sense of Bohr), which consists of all $f\in BC(X)$ such that for every $\varepsilon > 0$ there exists $l > 0$ such that for every subinterval of $\mathbb{R}$ of length $l$ contains at least one point $\tau$ such that $\|f(t+\tau)-f(t)\|_\infty\leq \varepsilon.$ A function $f\in BC(X)$ is said to be almost automorphic if for every sequence of real numbers $(s'_n)_{n\in\mathbb{N}}$ there exists a subsequence $(s_n)_{n\in \mathbb{N}} \subset (s'_n)_{n\in \mathbb{N}}$ such that

is well defined for each $t \in \mathbb{R},$ and

We denote by $AA(X)$ the Banach space of all almost automorphic functions.

On the other hand, the space of compact almost automorphic functions is the space of all functions $f\in BC(X)$ such that for all sequence $(s'_n)_{n\in \mathbb{N}}$ of real numbers there exists a subsequence $(s_n)_{n\in \mathbb{N}}\subset(s'_n)_{n\in \mathbb{N}}$ such that $g(t): = \lim_{n\to \infty} f(t + s_n)$ and $f(t) = \lim_{n\to \infty} g(t-s_n)$ uniformly over compact subsets of $\mathbb{R}$.

We notice that $P_T(X), AP(X), AA(X)$ and $AA_c(X)$ are Banach spaces under the norm $||\cdot||_\infty$ and

We notice that all these inclusions are proper. Now we consider the set $C_0(X): = \{f\in BC(X): \lim_{|t|\to \infty} ||f(t)|| = 0\},$ and define the space of asymptotically periodic functions as $AP_T(X): = P_T(X)\oplus C_0(X).$ Analogously, we define the space of asymptotically almost periodic functions,

the space of asymptotically compact almost automorphic functions,

and the space of asymptotically almost automorphic functions,

We have the following natural proper inclusions

For more details on this function spaces, we refer to reader to ^[23,27].

Throughout, we will use the notation $\mathcal{N}(X)$ to denote any of the function spaces $AP_T(X), \; AAP(X), \; AAA_c(X)$ and $AAA(X)$ defined above. Finally, we define the set $\mathcal{N}(\mathbb{R} \times X; X)$ which consists of all functions $f:\mathbb{R}\times X\to X$ such that $f(\cdot, x)\in \mathcal{N}(X)$ uniformly for each $x\in K$, where $K$ is any bounded subset of $X$. Moreover, we have the following result.

Theorem 7. ^[23] Let $\{S(t)\}_{t\geq 0}\subset \mathcal{B}(X)$ be a strongly continuous and uniformly $1$-integrable family, that is $\int_0^\infty \|S(t)\|dt < \infty.$ If $f\in \mathcal{N}(X),$ then the function $u: \mathbb{R}\to X$ defined by

belongs to $\mathcal{N}(X).$

3.   Bounded mild solutions to Eq (1.1)

Let $1 < \alpha < 2$ and $\beta\geq 1.$ In this section, we first consider the linear version of the Eq (1.1), that is,

Definition 8. A function $u\in C(\mathbb{R}, X)$ is called a mild solution to Eq (3.1) if the function $s\mapsto S_{\alpha, \beta}(t-s)f(s)$ is integrable on $(-\infty, t)$ for each $t\in\mathbb{R}$ and

We notice that (3.1) can be considered as the limiting equation of the following integro-differential equation with singular kernels

in the sense that the mild solution to Eq (3.3) converges to the mild solution of (3.1) as $t\to \infty.$ In fact, if $\omega < 0$ and $A$ is an $\omega$-sectorial operator of angle $\theta = \frac{(\alpha-1)}{2}\pi,$ then taking Laplace transform in (3.3) we obtain

which is equivalent to

Therefore the solution of problem (3.3) can be written as

where $\{S_{\alpha, \beta}(t)\}_{t\geq 0}$ is the family of operators given by

On the other hand, by [28,Corollary 3.9] the function $t\mapsto S_{\alpha, \beta}(t)$ is uniformly $1$-integrable and therefore if $f$ is a bounded continuous function (for example, if $f$ belongs to $\mathcal{N}(X)$), then the mild solution to Eq (1.1) is given by

Since

we conclude by [28,Corollary 3.8], that $v(t)-u(t)\to 0$ as $t\to \infty.$

Let $1 < \alpha < 2, \; \beta\geq 1$ such that $\alpha-\beta+1 > 0,$ $\omega < 0$ and assume that $A$ is an $\omega$-sectorial operator of angle $\theta = \frac{(\alpha-1)}{2}\pi.$ By Theorem 5, the operator $A$ generates a resolvent family $\{S_{\alpha, \beta}(t)\}_{t\geq 0}.$ Take a bounded and continuous function $f:\mathbb{R}\to X,$ (for example, we can take $f\in \mathcal{N}(X)$). Define the function $\phi(t)$ by

By Theorem 6 we have $||\phi||_\infty\leq ||S_{\alpha, \beta}||_1\, ||f||_\infty.$ If $f(t)\in D(A)$ for all $t\in\mathbb{R}$, then $\phi(t)\in D(A)$ for all $t\in \mathbb{R}$ (see [5,Proposition 1.1.7]). Assume that $\partial^\alpha \phi$ exists. The Proposition 4 and Fubini's theorem imply that

for all $t\in \mathbb{R}.$ This means that, $\phi$ is a (strong) solution to Eq (3.1). We recall that a function $u\in C(\mathbb{R}, X)$ is called a strong solution of (3.1) on $\mathbb{R}$ if $u\in C(\mathbb{R}, D(A)),$ the fractional derivative of $u, \; \partial^\alpha u,$ exists and (3.1) holds for all $t\in\mathbb{R}.$ If merely $u(t)$ belongs to $X$ instead of the $D(A),$ then $u$ is a mild solution to the Eq (3.1) according to Definition 8. As consequence of the above computation we have the following result.

Theorem 9. Let $1\leq \beta < \alpha < 2$ and $\omega < 0.$ Assume that $A$ is an $\omega$-sectorial operator of angle $\theta = \frac{(\alpha-1)}{2}\pi.$ Then for each $f\in \mathcal{N}(X)$ there is a unique mild solution $u\in\mathcal{N}(X)$ of Eq (3.1) which is given by

Proof. By Theorem 5, the operator $A$ generates a resolvent family $\{S_{\alpha, \beta}(t)\}_{t\geq 0}$ and by [28,Corollary 3.9] the function $t\mapsto S_{\alpha, \beta}(t)$ is uniformly $1$-integrable. By Theorem 7 the function $u(t) = \int_{-\infty}^t S_{\alpha, \beta}(t-s)f(s)ds$ belongs to $\mathcal{N}(X)$ and it is the mild solution to (3.1).

Next, we consider the semilinear Eq (1.1).

Definition 10. A function $u\in C(\mathbb{R}, X)$ is called a mild solution to Eq (1.1) if the function $s\mapsto S_{\alpha, \beta}(t-s)f(s, u(s))$ is integrable on $(-\infty, t)$ for each $t\in\mathbb{R}$ and

Theorem 11. Let $1\leq \beta < \alpha < 2, \; \omega < 0$ and $A$ is an $\omega$-sectorial operator of angle $\theta = \frac{(\alpha-1)}{2}\pi.$ If $f \in \mathcal{N}(\mathbb{R}\times X, X)$ satisfies

where $L < \frac{\alpha}{C}|\omega|^{\beta/\alpha}B\left(\frac{\beta}{\alpha}, 1-\frac{\beta}{\alpha}\right)^{-1},$ and $C$ is the constant given in Theorem 6, and $B(\cdot, \cdot)$ denotes the Beta function, then the Eq (1.1) has a unique mild solution $u\in \mathcal{N}(X).$

Proof. Define the operator $F:\mathcal{N}(X)\to \mathcal{N}(X)$ by

By [28,Corollary 3.9] we have

and [23,Theorems 3.3 and 4.1], $F$ is well defined, that is, $F\phi\in \mathcal{N}(X)$ for all $\phi\in \mathcal{N}(X).$ For $\phi_1, \phi_2 \in \mathcal{N}(X)$ and $t\in \mathbb{R},$ by (3.9), we have:

This proves that $F$ is a contraction, so by the Banach fixed point theorem there exists a unique $u\in \mathcal{N}(X)$ such that $Fu = u$.

Theorem 12. Let $1\leq \beta < \alpha < 2, \; \omega < 0$ and $A$ is an $\omega$-sectorial operator of angle $\theta = \frac{(\alpha-1)}{2}\pi.$ If $f \in \mathcal{N}(\mathbb{R}\times X, X)$ satisfies

where $\mathfrak{L}(\cdot)\in L^1(\mathbb{R}, \mathbb{R}_+)$, then the Eq (1.1) admits a unique mild solution $u\in\mathcal{N}(X).$

Proof. It easily follows by Theorem 6 that $\|S_{\alpha, \beta}(t)\|\leq\widetilde{C}: = \max\left\{C, \dfrac{C}{|\omega|}\right\}$. Define the operator $F$ as (3.8). For $u, v\in \mathcal{N}(X)$ and $t\in \mathbb{R}$, we have

Generally, we have

Since $\dfrac{(\|\mathfrak{L}\|_{1}\widetilde{C})^n }{n!} < 1$ for sufficiently large $n$, by the contraction principle $F$ admits a unique fixed point $u\in \mathcal{N}(X)$.

4.   Mild solutions to Eq (1.2) with nonlocal conditioins

Assume that $A$ is an $\omega$-sectorial operator of angle $\theta = \frac{(\alpha-1)}{2}\pi.$ By Theorem 5 the operator $A$ generates a resolvent family $\{S_{\alpha, \beta}(t)\}_{t\geq 0}.$ If $h:C(I, X) \to X$ is a continuous function, $f(0, u(0)) = 0$ and $u_1\in X,$ then it is well known that the mild solution to problem

is given by means of the variation-of-constant formula

We assume the following

● H1. The function $f$ satisfies the Carathéodory condition, that is $f(\cdot, u)$ is strongly measurable for each $u\in X$ and $f(t, \cdot)$ is continuous for each $t\in I: = [0, T].$

● H2. There exists a continuous function $\mu : I\to \mathbb{R}_+$ such that

and $f(0, u(0)) = 0.$

● H3. The function $h:C(I, X)\to X$ is continuous and there exists $L_h > 0$ such that

● H4. The set $\mathcal{K} = \{S_{\alpha, \beta}(t-s)f(s, u(s)): u\in C(I, X), 0\leq s\leq t\}$ is relatively compact for each $t\in I.$

Proposition 13. Let $1 < \alpha < 2$ and $1 < \beta\leq 2$ such that $\alpha-\beta+1 > 0.$ If $A$ is an $\omega$-sectorial operator of angle $\theta = \frac{(\alpha-1)}{2}\pi,$ where $\omega < 0,$ then the function $t\mapsto S_{\alpha, \beta}(t)$ is continuous in $\mathcal{B}(X)$ for all $t > 0.$

Proof. It proof follows similarly to [30,Proposition 11]. We omit the details.

We recall the following results.

Lemma 14 (Mazur's Theorem). If $K$ is a compact subset of a Banach space $X,$ then its convex closure $\overline{{\rm conv}(K)}$ is compact.

Lemma 15 (Leray-Schauder Alternative Theorem). Let $C$ be a convex subset of a Banach space $X.$ Suppose that $0\in C.$ If $F:C\to C$ is a completely continuous map, then either $F$ has a fixed point, or the set $\{x\in C:x = \lambda F(x), 0 < \lambda < 1\}$ is unbounded.

Lemma 16 (Krasnoselskii Theorem). Let $C$ be a closed convex and nonempty subset of a Banach space $X.$ Let $Q_1$ and $Q_2$ be two operators such that

i) If $u, v\in C,$ then $Q_1u+Q_2v\in C.$

ii) $Q_1$ is a mapping contraction.

iii) $Q_2$ is compact and continuous.

Then, there exists $z\in C$ such that $z = Q_1z+Q_2z.$

We have the following existence theorem.

Theorem 17. Let $1 < \alpha < 2$ and $1 < \beta < 2$ such that $\alpha-\beta+1 > 0.$ Assume that $A$ is an $\omega$-sectorial operator of angle $\theta = \frac{(\alpha-1)}{2}\pi,$ where $\omega < 0.$ Under assumptions H1-H4, the problem (4.1) has at least one mild solution.

Proof. By Theorem 5, the operator $A$ generates a resolvent family $\{ S_{\alpha, 1}(t)\}_{t\geq 0}.$ By the uniqueness of the Laplace transform we have $S_{\alpha, 2}(t) = (g_1\ast S_{\alpha, 1})(t)$ and $S_{\alpha, \beta}(t) = (g_{\beta-1}\ast S_{\alpha, 1})(t)$ for all $t\geq 0.$ Moreover, by Theorem 6 there exists a constant $M > 0$ such that $\|S_{\alpha, 2}(t)\|\leq M$ and $\|S_{\alpha, \beta}(t)\|\leq M$ for all $t\geq 0.$ Now, we define the operator $\Gamma : C(I, X)\to C(I, X)$ by

Let $B_r: = \{u\in C(I, X): \|u\|\leq r\},$ where $r > 0.$ We shall prove that $\Gamma$ has at least one fixed point by the Leray-Schauder fixed point theorem. We will consider several steps in the proof.

Step 1. The operator $\Gamma$ sends bounded sets of $C(I, X)$ into bounded sets of $C(I, X).$ In fact, take $u\in B_r$ and $G: = \sup_{u\in B_r}\|h(u)\|$. Then

Therefore $\Gamma B_r \subset B_R$.

Step 2. $\Gamma$ is a continuous operator.

Let $u_n, u \in B_r$ such that $u_n\to u$ in $C(I, X)$. Then we have

We notice that the function $s\mapsto \mu(s)$ is integrable on $I.$ By the Lebesgue's Dominated Convergence Theorem, $\int_{0}^{t}\|f(s, u_n(s))-f(s, u(s))\|ds\to 0$ as $n\to \infty.$ Since $u_n\to u$ we obtain that $\Gamma$ is continuous in $C(I, X).$

Step 3 The operator $\Gamma$ sends bounded sets of $C(I, X)$ into equicontinuous sets of $C(I, X)$.

In fact, let $u\in B_r$, with $r > 0$ and take $t_1, t_2\in I$ with $t_2 < t_1.$ Then we have

Observe that

Using the norm continuity of $t\mapsto S_{\alpha, 2}(t)$ (see Proposition 13) we obtain that $\lim_{t_1\to t_2}I_1 = 0.$

On the other hand,

and therefore $\lim_{t_1\to t_2}I_2 = 0.$ Finally, for $I_3$ we have

Since

and $S_{\alpha, \beta}(t_1-s)-S_{\alpha, \beta}(t_2-s)\to 0$ in $\mathcal{B}(X),$ as $t_1\to t_2$ (see Proposition 13) we obtain by the Lebesgue's dominated convergence theorem that $\lim_{t_1\to t_2}I_3 = 0.$ The proof of the claim is finished.

Step 4. The function $\Gamma$ maps $B_r$ into relatively compact sets in $X$.

The hypothesis and Lemma 14 imply that $\overline{{\rm conv}(\mathcal{K})}$ is compact. Moreover, for $u\in B_r$, by the Mean-Value Theorem for the Bochner integral (see [15,Corollary 8,p. 48]), we get

for all $t\in [0, T].$ Thus the set $\overline{\{\Gamma u(t); u\in B_r\}}$ is compact in $X$ for every $t\in [0, T].$

We conclude from Steps 1–4, that $\Gamma$ is continuous and compact by the Arzela-Ascoli's theorem, which means that the function $\Gamma$ is completely continuous.

Step 5. The set $\Omega : = \{u\in B_r: u = \lambda \Gamma u, \; 0 < \lambda < 1\}$ is bounded. In fact, since $0\in \Omega$ we obtain that $\Omega \not = \emptyset.$ For $u\in \Omega$ we have

for all $t\in [0, T],$ which means that $\Omega$ is a bounded set.

Therefore, by Lemma 15 we conclude that $\Gamma$ has a fixed point, and the proof of the Theorem is finished.

The same method of proof can be used to prove the next result. We omit the details.

Theorem 18. Let $1 < \alpha < 2.$ Assume that $A$ generates the resolvent family $\{S_{\alpha, 1}(t)\}_{t\geq 0}.$ Under assumptions H1–H4, the problem (4.1) has at least one mild solution.

Now, we consider the problem

where $g, h:C(I, X) \to X$ are continuous, $f(0, u(0)) = 0$ and $u_0, u_1\in X.$ By (2.2) in Theorem 6, there exists a constant $M > 0$ such that

Thus

Under the same assumptions H1–H3 and

● H3'. The function $g:C(I, X)\to X$ is continuous and there exists $L_g > 0$ such that

we have the following result.

Theorem 19. Let $1 < \alpha < 2$ and $1 < \beta < 2$ such that $\alpha-\beta+1 > 0.$ Assume that $A$ is an $\omega$-sectorial operator of angle $\theta = \frac{(\alpha-1)}{2}\pi,$ where $\omega < 0.$ Suppose that $M\|\mu\|_\infty T^{\beta} < 1$ and $M(L_g+TL_h) < 1,$ where $M$ is the constant in (4.4). Assume that $(\lambda^\alpha-A)^{-1}$ is compact for all $\lambda > \nu^{1/\alpha},$ where $\nu$ is a positive constant. Under assumptions H1–H3 and H3', the problem (4.2) has at least one mild solution.

Proof. By Theorem 5, the operator $A$ generates the resolvent family $\{ S_{\alpha, 1}(t)\}_{t\geq 0},$ and $S_{\alpha, 2}(t) = (g_1\ast S_{\alpha, 1})(t)$ and $S_{\alpha, \beta}(t) = (g_{\beta-1}\ast S_{\alpha, 1})(t)$ for all $t\geq 0.$ Then, the mild solution to problem (4.2) is given by

Let $B_r: = \{u\in C(I, X): \|u\|\leq r\},$ where

On $B_r$ we define the operators $\Gamma_1, \Gamma_2$ by

where $u\in B_r.$ We claim that $\Gamma: = \Gamma_1+\Gamma_2$ has at least one fixed. To prove this, we will consider several steps.

Step 1. We claim that if $u, v\in B_r,$ then $\Gamma_1 u+\Gamma_2 v\in B_r.$ In fact,

Thus $\Gamma_1 u+\Gamma_2 v\in B_r$ for all $u, v\in B_r.$

Step 2. $\Gamma_1$ is a contraction on $B_r.$ In fact, if $u, v\in B_r,$ then

Since $M(L_g+TL_h) < 1,$ we get that $\Gamma_1$ is a contraction.

Step 3. $\Gamma_2$ is completely continuous.

Firstly, we prove that $\Gamma_2$ is a continuous operator on $B_r.$ Let $u_n, u \in B_r$ such that $u_n\to u$ in $B_r$. We notice that by (4.3)

Moreover, the function $s\mapsto \mu(s)$ is integrable on $[0, T].$ The Lebesgue's Dominated Convergence Theorem implies that $\int_{0}^{t}\|f(s, u_n(s))-f(s, u(s))\|ds\to 0$ as $n\to \infty.$ Since $u_n\to u$ we obtain that $\Gamma_2$ is continuous in $B_r.$

Now, we prove that $\{\Gamma_2 u:\, u\in B_r\}$ is a relatively compact set. In fact, by the Ascoli-Arzela theorem we only need to prove that the family $\{\Gamma_2 u:\, u\in B_r\}$ is uniformly bounded and equicontinuous, and the set $\{\Gamma_2 u(t):\, u\in B_r\}$ is relatively compact in $X$ for each $t\in[0, T].$ For each $u\in B_r$ we have $\|\Gamma_2 u\|\leq MT^{\beta}r\|\mu\|_\infty,$ which implies that $\{\Gamma_2 u:\, u\in B_r\}$ is uniformly bounded.

Next, we prove the equicontinuity. For $u\in B_r$ and $0\leq t_2 < t_1\leq T$ we have

Observe that for $I_1,$ by (4.3) we have $I_1 \leq MT^\beta\int_{t_2}^{t_1} \mu(s)\|u(s)\|ds \leq MT^\beta r \|\mu\|_{\infty}(t_1-t_2),$ and thus $\lim_{t_1\to t_2}I_1 = 0.$ On the other hand, for $I_2$ we have

By (4.4) we have $\mu(\cdot)\|S_{\alpha, \beta}(t_1-\cdot)-S_{\alpha, \beta}(t_2-\cdot)\|\leq 2T^{\beta-1} M\mu(\cdot)\in L^1([0, T], \mathbb{R}),$ and by Proposition 13 the function $t\mapsto S_{\alpha, \beta}(t)$ is norm continuous. This implies that if $t_1\to t_2,$ then $S_{\alpha, \beta})(t_1-s)-S_{\alpha, \beta})(t_2-s)\to 0$ in $\mathcal{B}(X).$ By the Lebesgue's dominated convergence theorem we conclude that $\lim_{t_1\to t_2}I_2 = 0.$ Therefore, $\{\Gamma_2 u:u\in B_r\}$ is an equicontinuous family.

Finally, we prove that $H(t): = \{\Gamma_2 u(t):\, u\in B_r\}$ is relatively compact in $X$ for each $t\in[0, T].$ Clearly, $H(0)$ is relatively compact in $X.$ Now, we take $t > 0.$ For $0 < \varepsilon < t$ we define on $B_r$ the operator

By [30,Theorem 14] we have that $S_{\alpha, \beta}(t)$ is a compact operator for all $t > 0.$ Thus $\mathcal{K}_\varepsilon: = \{S_{\alpha, \beta}(t-s)f(s, u(s)):\, u\in B_r, 0\leq s\leq t-\varepsilon\}$ is a compact set for all $\varepsilon > 0.$ By Lemma 14, $\overline{{\rm conv}(\mathcal{K}_\varepsilon)}$ is also a compact set. The Mean-Value Theorem for the Bochner integrals (see [15,Corollary 8,p. 48]), implies that $(\Gamma_2^\varepsilon u)(t)\in t\overline{{\rm conv}(\mathcal{K}_\varepsilon)},$ for all $t\in [0, T].$ Therefore, the set $H_{\varepsilon}(t): = \{(\Gamma_2^\varepsilon u)(t):\, u\in B_r\}$ is relatively compact in $X$ for all $\varepsilon > 0.$ Since

and the function $s\mapsto \mu(s)$ belongs to $L^1([t-\varepsilon, t], \mathbb{R}_+)$ we conclude by the Lebesgue dominated convergence Theorem that $\lim_{\varepsilon\to 0}\|(\Gamma_2 u)(t)-(\Gamma_2^\varepsilon u)(t)\| = 0.$ Therefore the set $\{\Gamma_2 u(t):\, u\in B_r\}$ is relatively compact in $X$ for each $t\in(0, T].$ The Ascoli-Arzela theorem implies that the set $\{\Gamma_2 u:\, u\in B_r\}$ is relatively compact. We conclude that $\Gamma_2$ is a completely continuous operator. By Lemma 16 we have that $\Gamma = \Gamma_1+\Gamma_2$ has a fixed point on $B_r,$ and therefore the problem (4.2) has a mild solution.

5.   Examples

Example 20.

On the Banach space $X = \mathbb{C},$ let $A$ be the scalar operator $A = \varrho I,$ where $\varrho\in\mathbb{R}.$ Consider the multi-term fractional differential equation

where $1\leq \beta < \alpha < 2$ and $f(t)$ is the almost periodic function $f(t) = \sin(t)+\sin(\sqrt{2}t),$ see [14,p. 80]. By Theorem 9 the solution $u$ to (5.1) is an almost periodic function, and it is given by

where $S_{\alpha, \beta}(t) = t^{\beta-1}E_{\alpha, \beta}(\varrho t^\alpha).$ By Theorem 6, we can write

Now, we notice that if $g(t) = e^{\mu t},$ where $\mu\in \mathbb{C}$ and $\delta > 0,$ then

and therefore, for $h(t) = \sin(at) = \frac{e^{ait}-e^{-ait}}{2i},$ where $a > 0,$ we have

This implies that

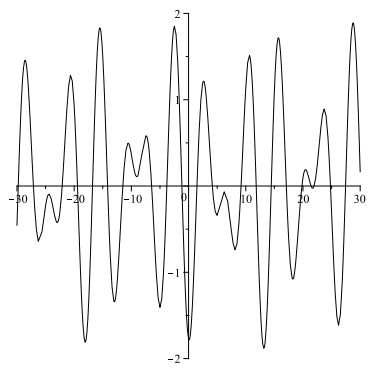
In Figure 1, we have the solution $u$ for (5.1) for $\varrho = -1$ and $\alpha = 1.5, \beta = 1.3$ on the interval $[-30, 30].$

Example 21.

Consider the following partial differential equation with fractional temporal derivatives

where $1 < \alpha, \beta < 2, \; 0\leq t_1 < ... < t_n\leq T, \; u_1\in L^2(\mathbb{R}),$ and $c_i$ are real constants.

On the Banach space $X = L^2(\mathbb{R}),$ let $A$ be the second order operator $Av = v''$ with domain $D(A) = W^{2, 2}(\mathbb{R}).$ By [36,Example 1.2.2,p. 3063], $A$ generates a cosine family $\{S_{2, 1}(t)\}_{t\in\mathbb{R}}$ on $X,$ and by the Subordination Principle [32,Corollary 3.3], $A$ is the generator of the resolvent family $\{S_{\alpha, 1}(t)\}_{t\geq 0}$ given by

where $\psi_{\frac{\alpha}{2}, 1-\frac{\alpha}{2}}$ is the Wright type function defined by

for $\theta\in(\pi-\frac{2}{\alpha}, \pi/2).$ Define,

Then, (5.2) can be reformulated as the abstract problem (4.1). Moreover, an easy computation shows that the hypotheses H1–H3 hold with $\mu(t) = 1$ and $L_h = \sum_{i = 1}^n |c_i|.$ Since

we obtain by (2.2) that the set $\mathcal{K} = \{S_{\alpha, \beta}(t-s)\sin(s, u(s)): u\in C(I, X), 0\leq s\leq t\}$ is relatively compact for each $t\in I,$ and therefore H4 holds. We conclude, by Theorem 18, that the problem (4.1) has at least one mild solution $u.$

6.   Conclusions

In this paper, some sufficient conditions are established for the existence of mild and bounded solutions to a multi-term fractional differential equation. Under the theory of fractional resolvent families in Banach spaces, the mild solutions are given in term of these operator families. The main results are given for the Caputo and Weyl fractional derivatives.

Acknowledgements

The authors thank the anonymous referees for reading the manuscript and for making suggestions that have improved the previous version of this paper. This work was partially supported by NSF of Shaanxi Province (2020JM-183).

Conflict of interest

The authors declare no conflict of interest.