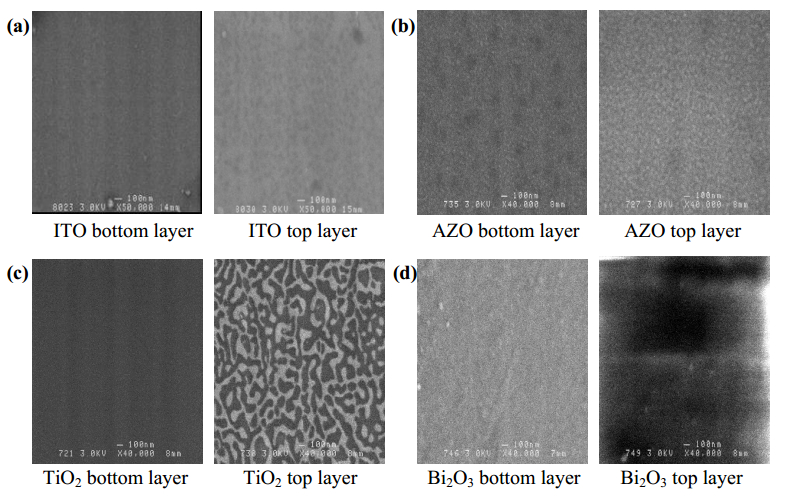
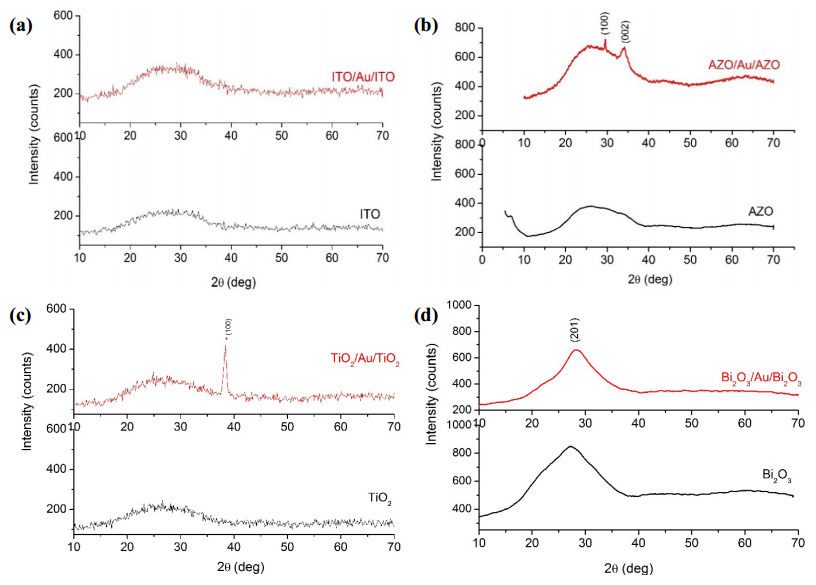
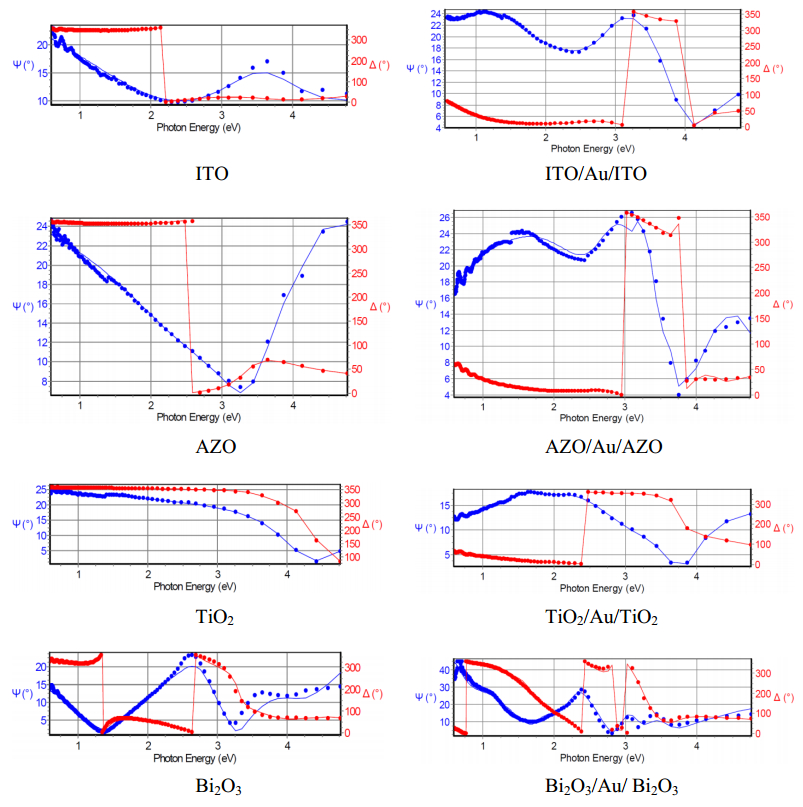
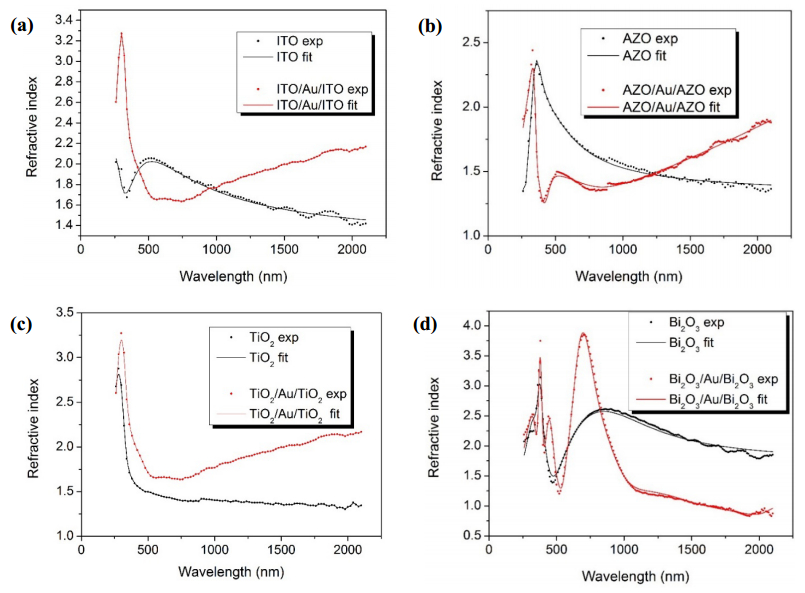
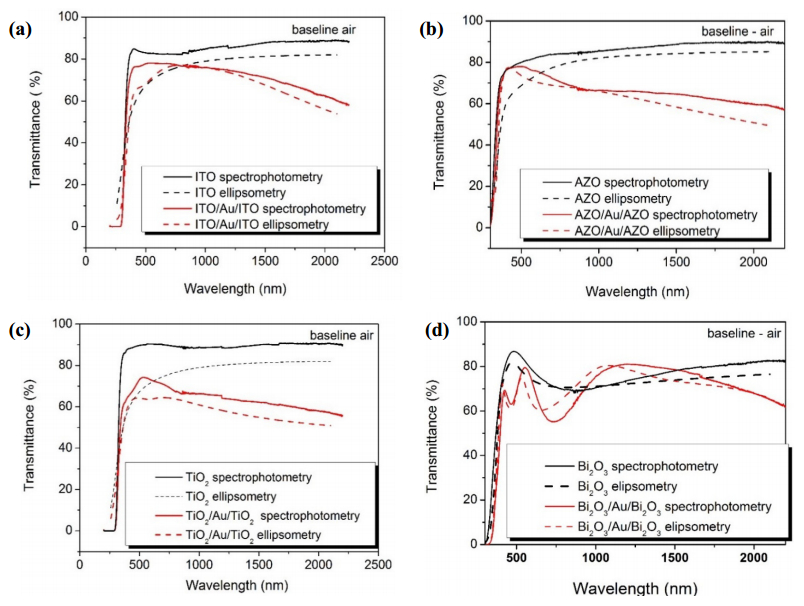
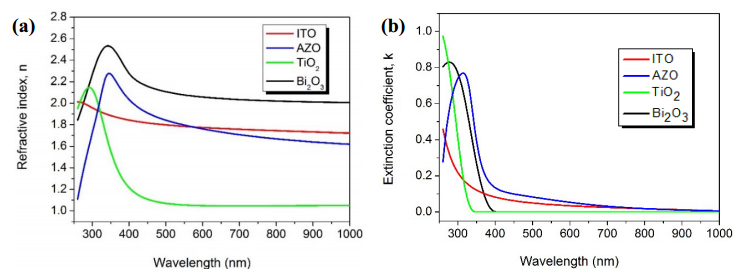
A comprehensive study of a class of Oxide/Metal/Oxide (Oxide = ITO, AZO, TiO2 and Bi2O3, Metal = Au) thin films was done by correlating the spectrophotometric studies with the ellispometric models. Films were deposited by successive sputtering from metallic targets In:Sn, Zn:Al, Ti and Bi in reactive atmosphere (for the oxide films) and respective inert atmosphere (for the metallic Au interlayer films) on glass substrates. The measurements of optical constants n—the refractive index and k—the extinction coefficient, at different incident photon energies for single oxide films and also for the three layers films oxide/metal/oxide samples were made using the spectroscopic ellipsometry (SE) technique. The ellipsometry modelling process was coupled with the recorded transmission spectra data of a double beam spectrophotometer and the best fitting parameters were obtained not only by fitting the n and k experimental data with the dispersion fitting curves as usual is practiced in the most reported data in literature, but also by comparing the calculated the transmission coefficient from ellipsometry with the experimental values obtained from direct spectrophotometry measurements. In this way the best dispersion model was deduced for each sample. Very good correlations were obtained for the other different thin films characteristics such as the films thickness, optical band gap and electrical resistivity obtained by other measurements and calculation techniques. The ellipsometric modelling, can hence give the possibility in the future to predict, by ellipsometric simulations, the proper device architecture in function of the preferred optical and electrical properties.
1.
Introduction
In this paper, we study the following general class of functional differential equations with distributed delay and bistable nonlinearity,
Many mathematical models issued from ecology, population dynamics and other scientific fields take the form of Eq (1.1) (see, e.g., [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15] and references therein). When a spatial diffusion is considered, many models are studied in the literature (see, e.g., [16,17,18,19,20,21,22]).
For the case where g(x)=f(x) has not only the trivial equilibrium but also a unique positive equilibrium, Eq (1.1) is said to be a problem with monostable nonlinearity. In this framework, many authors studied problem (1.1) with various monostable nonlinearities such as Blowflies equations, where f(x)=μx and g(x)=βxe−αx, and Mackey-Glass equations, where f(x)=μx and g(x)=βx/(α+xn) (see, e.g., [1,3,6,7,9,10,11,12,13,21,23,24,25,26,27]).
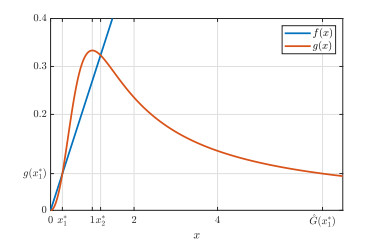
When g(x) allows Eq (1.1) to have two positive equilibria x∗1 and x∗2 in addition to the trivial equilibrium, Eq (1.1) is said to be a problem with bistable nonlinearity. In this case, Huang et al. [5] investigated the following general equation:
The authors described the basins of attraction of equilibria and obtained a series of invariant intervals using the decomposition domain. Their results were applied to models with Allee effect, that is, f(x)=μx and g(x)=βx2e−αx. We point out that the authors in [5] only proved the global stability for x∗2≤M, where g(M)=maxs∈R+g(s). In this paper, we are interested in the dynamics of the bistable nonlinearity problem (1.1). More precisely, we will present some attracting intervals which will enable us to give general conditions on f and g that ensure global asymptotic stability of equilibria x∗1 and x∗2 in the both cases x∗2<M and x∗2≥M.
The paper is organized as follows: in Section 2, we give some preliminary results including existence, uniqueness and boundedness of the solution as well as a comparison result. We finish this section by proving the global asymptotic stability of the trivial equilibrium. Section 3 is devoted to establish the attractive intervals of solutions and to prove the global asymptotic stability of the positive equilibrium x∗2. In Section 4, we investigate an application of our results to a model with Allee effect. In Section 5, we perform numerical simulation that supports our theoretical results. Finally, Section 6 is devoted to the conclusion.
In the whole paper, we suppose that the function h is positive and
We give now some standard assumptions.
(T1) f and g are Lipschitz continuous with f(0)=g(0)=0 and there exists a number B>0 such that maxv∈[0,s]g(v)<f(s) for all s>B.
(T2) f′(s)>0 for all s≥0.
(T3) g(s)>0 for all s>0 and there exists a unique M>0 such that g′(s)>0 for 0<s<M, g′(M)=0 and g′(s)<0 for s>M.
(T4) There exist two positive constants x∗1 and x∗2 such that g(x)<f(x) if x∈(0,x∗1)∪(x∗2,∞) and g(x)>f(x) if x∈(x∗1,x∗2) and g′(x∗1)>f′(x∗1).
Let C:=C([−τ,0],R) be the Banach space of continuous functions defined in [−τ,0] with ||ϕ||=supθ∈[−τ,0]|ϕ(θ)| and C+={ϕ∈C;ϕ(θ)≥0,−τ≤θ≤0} is the positive cone of C. Then, (C,C+) is a strongly ordered Banach space. That is, for all ϕ,ψ∈C, we write ϕ≥ψ if ϕ−ψ∈C+, ϕ>ψ if ϕ−ψ∈C+∖{0} and ϕ>>ψ if ϕ−ψ∈Int(C+). We define the following ordered interval:
For any χ∈R, we write χ∗ for the element of C satisfying χ∗(θ)=χ for all θ∈[−τ,0]. The segment xt∈C of a solution is defined by the relation xt(θ)=x(t+θ), where θ∈[−τ,0] and t≥0. In particular, x0=ϕ. The family of maps
such that
defines a continuous semiflow on C+ [28]. For each t≥0, the map Φ(t,.) is defined from C+ to C+ which is denoted by Φt:
The set of equilibria of the semiflow, which is generated by (1.1), is given by
2.
Preliminary results
In this section, we first provide existence, uniqueness and boundedness of solution to problem (1.1). We then present a Lyapunov functional and show the global asymptotic stability of the trivial equilibrium. We begin by recalling a useful theorem related to a comparison principle (see Theorem 1.1 in page 78 in [29]).
We consider the following problem:
where F:Ω→R is continuous on Ω, which is an open subset of C. We write x(t,ϕ,F) for the maximal defined solution of problem (2.1). When we need to emphasize the dependence of a solution on initial data, we write x(t,ϕ) or x(ϕ).
Theorem 2.1. Let f1, f2 :Ω→R be continuous, Lipschitz on each compact subset of Ω, and assume that either f1 or f2 is a nondecreasing function, with f1(ϕ)≤f2(ϕ) for all ϕ∈Ω. Then
holds for all t≥0, for which both are defined.
The following lemma states existence, uniqueness and boundedness of the positive solution to problem (1.1). For the proof, see [15,30,31].
Lemma 2.2. Suppose that (T1) holds. For any ϕ∈C+, the problem (1.1) has a unique positive solution x(t):=x(t,ϕ) on [0,∞) satisfying x0=ϕ, provided ϕ(0)>0. In addition, we have the following estimate:
Moreover, the semiflow Φt admits a compact global attractor, which attracts every bounded set in C+.
The following lemma can be easily proved (see also the proof of Theorem 1.1 in [29]).
Lemma 2.3. Let ϕ∈C be a given initial condition, x(ϕ) be the solution of problem (1.1) and xϵ(ϕ), ϵ>0 be the solution of problem (1.1) when replacing f by f±ϵ. Then
We focus now on the global stability of the trivial equilibrium. For this purpose, we suppose that
Lemma 2.4. Assume that (T1) and condition (2.2) hold. For a given ϕ∈C[0,x∗1]∖{x∗1}, the solution x(ϕ) of problem (1.1) satisfies
provided that one of the following hypotheses holds:
(i) g is a nondecreasing function over (0,x∗1).
(ii) f is a nondecreasing function over (0,x∗1).
Proof. Without loss of generality, we assume that ϕ(0)<x∗1. Suppose that (ⅰ) holds. We first claim that x(t)<x∗1 for all t≥0. Let xϵ(ϕ) be the solution of problem (1.1) by replacing f by f+ϵ. We prove that xϵ(t):=xϵ(t,ϕ)≤x∗1 for all t>0. Suppose, on the contrary, that there exists t1>0 such that xϵ(t1)=x∗1, xϵ(t)≤x∗1 for all t≤t1 and x′ϵ(t1)≥0. Then, using the equation of xϵ(t1), we have
which leads to a contradiction. Now, applying Lemma 2.3, we obtain x(t)≤x∗1 for all t≥0. Next, set X(t)=x∗1−x(t). We then have
Since x(t)≤x∗1 for all t≥0 and g is a nondecreasing function, we have, for t≥τ,
where L is the Lipschitz constant of f. Consequently, X(t)≥X(0)e−Lt and the claim is proved. Finally, to prove inequality (2.3), we suppose, on the contrary, that there exist an increasing sequence (tn)n, tn→∞, t0≥τ and a nondecreasing sequence (x(tn))n, such that x(tn)→x∗1 as tn→∞, x(tn)<x∗1 for some n and x(tn)=maxt∈[0,tn]x(t). Then, in view of condition (2.2), the equation of x(tn) satisfies
which is a contradiction.
Suppose now that (ⅱ) holds. Let xϵ(t) be the solution of problem (1.1) by replacing f(s) by f(s)+ϵ and g(s) by g+(s)=maxσ∈[0,s]g(σ).
Then, again by contradiction, suppose that there exists t1>0 such that xϵ(t1)=x∗1, xϵ(t)≤x∗1 for all t≤t1 and x′ϵ(t1)≥0. Then, arguing as above, we obtain
Since f is a nondecreasing function and g+ is the smallest nondecreasing function that is greater than g, we obtain f(s)>g+(s) for all s∈(0,x∗1). This contradicts with inequality (2.4). Further, by combining Theorem 2.1 and Lemma 2.3, we get x(t)≤x∗1 for all t≥0. Finally, following the same arguments as in the first part of this proof, the lemma is proved.
We now prove the global asymptotic stability of the trivial equilibrium.
Theorem 2.5. Assume that (T1) and condition (2.2) hold. Suppose also that ϕ∈C[0,x∗1]∖{x∗1}. The trivial equilibrium is globally asymptotically stable if one of the following hypotheses holds:
(i) g is a nondecreasing function over (0,x∗1).
(ii) f is a nondecreasing function over (0,x∗1).
Proof. Suppose that (ⅰ) holds. Let V be the Lyapunov functional defined by
where ψ(a)=∫τah(σ)dσ. The derivative of V along the solution of problem (1.1) gives
From condition (2.2) and Lemma 2.4, we have dV(xt)/dt<0 and thus, the result is reached by classical Lyapunov theorem (see [6]). Next, suppose that (ⅱ) holds. Let the function V be defined in (2.5) by replacing g(s) by g+(s):=maxσ∈[0,s]g(σ). Since g+(s)<f(s) for all s∈(0,x∗1), we can employ the same argument as above to get dV(xt)/dt<0. Finally the result is obtained by applying Theorem 2.1 and Lemma 2.4. This completes the proof.
Now, suppose that
Using the same proof as in Theorem 2.5, we immediately obtain the following theorem.
Theorem 2.6. Assume that (T1) and condition (2.6) hold. The trivial equilibrium is globally asymptotically stable for all ϕ∈C+.
3.
Global stability of the positive equilibrium x∗2
The following theorem concerns the global stability of x∗2 in the case where g is a nondecreasing function.
Theorem 3.1. Suppose that ϕ∈C[x∗1,sups∈[−τ,0]ϕ]∖{x∗1} and g is a nondecreasing function. Assume also that (T1) and (T4) hold. Then, the positive equilibrium x∗2 is globally asymptotically stable.
Proof. Without loss of generality, suppose that ϕ(0)>x∗1. We first claim that lim inft→∞x(t)>x∗1. For this, let xϵ(t):=xϵ(t;ϕ) be the solution of problem (1.1) when replacing f by f−ϵ. To reach the claim, we begin by proving that xϵ(t)>x∗1 for all t≥0. Otherwise, there exists t1>0 such that xϵ(t1)=x∗1, xϵ(t)≥x∗1 for all t≤t1 and x′ϵ(t1)≤0. Then, the equation of xϵ(t1) satisfies
This reaches a contradiction and thus xϵ(t)>x∗1 for all t>0. This result, together with Lemma 2.3, gives x(t)≥x∗1 for all t≥0.
Next, for X(t)=x(t)−x∗1 and since f(x∗1)=g(x∗1), the equation of X(t) satisfies
this leads to
where L is the Lipschitz constant of f. Consequently x(t)>x∗1 for all t>0.
Now, suppose that there exist an increasing sequence (tn)n, tn→∞, t0≥τ and a nonincreasing sequence (x(tn))n, such that x(tn)→x∗1 as tn→∞, x(tn)>x∗1 for some n and x(tn)=mint∈[0,tn]x(t). In view of (T4), the equation of x(tn) satisfies
which is a contradiction. The claim is proved.
To prove that x∗2 is globally asymptotically stable, we consider the following Lyapunov functional
with ψ(a)=∫τah(σ)dσ. By a straightforward computation, the derivative of V along the solution of problem (1.1) gives, for all t>0,
Note that ψ(τ)=0. In view of (T4), we have dV(xt)/dt≤0. If g is an increasing function, then the result is reached by using a classical Lyapunov theorem (see, e.g., [30]). If g is a nondecreasing function, then the result is proved by using the same argument as in the proof of Theorem 2.6 in [31]. This completes the proof.
We focus now on the case where g is non-monotone. Suppose that there exists ˆG(x) such that ˆG(x)=ˆg−1og(x), where ˆg(.) denotes the restriction of g to the interval [M,∞). Then, ˆG(x)=x for x∈[M,∞) and ˆG(x)>M>x for x∈[0,M).
Lemma 3.2. Assume that (T1)–(T4) hold. For a given ϕ∈C[x∗1,ˆG(x∗1)]∖{x∗1}, let x be the solution of problem (1.1). Then, the following assertions hold:
(i) if x∗2<M, then x∗1<x(t)<ˆG(x∗1) for all t>0.
(ii) if x∗2≥M and f(ˆG(x∗1))>g(M), then x∗1<x(t)<ˆG(x∗1) for all t>0.
Proof. Without loss of generality, suppose that x∗1<ϕ(0)≤ˆG(x∗1). First, observe that, for a given ϕ∈C[x∗1,ˆG(x∗1)]∖{x∗1}, we have f(ˆG(x∗1))>f(x∗1)=g(x∗1)=g(ˆG(x∗1)), and thus, there exists ϵ>0 such that f(ˆG(x∗1))−ϵ>g(ˆG(x∗1)). We begin by proving that x∗1≤x(t)≤ˆG(x∗1) for all t>0. To this end, in view of Lemma 2.3, we only need to prove that x∗1<xϵ(t):=xϵ(t;ϕ)≤ˆG(x∗1) for all t>0, where xϵ(t):=xϵ(t;ϕ) is the solution of problem (1.1) when replacing f by f−ϵ. Let yϵ:=yϵ(ϕ) be the solution of
with g+(s)=maxσ∈[0,s]g(σ). Since f is an increasing function, we have f(ˆG(x∗1))−ϵ>g+(ˆG(x∗1)). Accordingly, the function ˆG(x∗1) is a super-solution of problem (3.1). Finally, in view of Theorem 2.1, we obtain xϵ(t)≤yϵ(t)≤ˆG(x∗1) for all t≥0.
We now prove that xϵ(t)>x∗1. Suppose, on the contrary, that there exists t1>0 such that xϵ(t1)=x∗1, xϵ(t)≥x∗1 for all t≤t1, and thus, x′ϵ(t1)≤0. Then, the equation of xϵ(t1) satisfies
since g(s)≥g(x∗1) for all s∈[x∗1,ˆG(x∗1)]. This reaches a contradiction. Further, from Lemma 2.3, we obtain x(t)≥x∗1. The claim is proved.
Next, let X(t)=x(t)−x∗1. Since f(x∗1)=g(x∗1), the equation of X(t) satisfies
We know that g(s)≥g(x∗1) for all s∈[x∗1,ˆG(x∗1)]. We then have
where L is the Lipschitz constant of f. Consequently x(t)>x∗1 for all t>0.
Next, we prove that x(t)<ˆG(x∗1) for all t>0. Suppose, on the contrary, that there exists t1>0 such that x(t1)=ˆG(x∗1), x(t)≤ˆG(x∗1) for all t≤t1 and x′(t1)≥0. Then
Since f is an increasing function and ˆG(x∗1)>M, we have
Now, the assertion x∗2<M implies that g(M)<f(M), which leads to a contradiction.
When x∗2≥M, inequality (3.2) gives a contradiction by hypothesis. The Lemma is proved.
Using Lemma 3.2, we next prove the following lemma.
Lemma 3.3. Suppose that ϕ∈C[x∗1,ˆG(x∗1)]∖{x∗1}. Assume also that (T1)–(T4) hold. Let x be the solution of problem (1.1). Then, we have
provided that one of the following assertions holds:
(i) x∗2<M.
(ii) x∗2≥M and f(ˆG(x∗1))>g(M).
Proof. Firstly, from Lemma 3.2, both assertions imply that x∗1<x(t)<ˆG(x∗1) for all t≥0. Next, observe that there exists ϵ>0 such that
with θϵ∈(M,ˆG(x∗1)) and g(θϵ)=g(x∗1+ϵ). Indeed, in view of (T3), minσ∈[x∗1+ϵ,θϵ]g(σ)=g(θϵ)=g(x∗1+ϵ) and from (T4), we have g(x∗1+ϵ)>f(x∗1+ϵ).
Now, consider the following nondecreasing function:
where x∗1<ˉm<x∗1+ϵ, which is a constant satisfying g(ˉm)=f(x∗1+ϵ). From (T2) and (T4), we get g_(s)>f(s) for x∗1<s<x∗1+ϵ and g_(s)<f(s) for s>x∗1+ϵ. Let y(ϕ) be the solution of problem (1.1) when replacing g by g_. Then, according to Theorem 2.1, we have y(t;ϕ)≤x(t;ϕ). In addition, using Theorem 3.1, we obtain
The lemma is proved.
Under Lemma 3.3, we prove the following theorem on the global asymptotic stability of the positive equilibrium x∗2<M.
Theorem 3.4. Suppose that ϕ∈C[x∗1,ˆG(x∗1)]∖{x∗1} and x∗2<M. Assume also that (T1)–(T4) hold. Then, the positive equilibrium x∗2 is globally asymptotically stable.
Proof. We first claim that there exists T>0 such that x(t)≤M for all t≥T. Indeed, let xϵ:=xϵ(ϕ) be the solution of problem (1.1) when replacing f by f+ϵ. First, suppose that there exists T>0 such that xϵ(t)≥M for all t≥T. So, since x∗2<M, we have from (T4) that g(M)<f(M). Combining this with (T2), the equation of xϵ satisfies
which contradicts with xϵ(t)≥M. Hence there exists T>0 such that xϵ(T)<M. We show that xϵ(t)<M for all t≥T. In fact, at the contrary, if there exists t1>T such that xϵ(t1)=M and so x′ϵ(t1)≥0 then,
which is a contradiction. Further, according to Lemma 2.3, there exists T>0 such that x(t)≤M for all t≥T and the claim is proved. Finally, since g is a nondecreasing function over (0,M), the global asymptotic stability of x∗2 is proved by applying Theorem 3.1.
Remark 3.5. In the case where g(s)>g(x∗1) for all s>x∗1, the above theorem holds true. In fact, it suffices to replace ˆG(x∗1) in C[x∗1,ˆG(x∗1)]∖{x∗1} by sups∈[−τ,0]ϕ(s).
We focus now on the case where x∗2≥M. To this end, suppose that there exists a unique constant A such that
Lemma 3.6. Assume that (T1)–(T4) hold. Suppose also that x∗2≥M and f(A)≥g(M). For a given ϕ∈C[x∗1,ˆG(x∗1)]∖{x∗1} and the solution x:=x(ϕ) of problem (1.1), there exists T>0 such that
Proof. We begin by claiming that x∗2≤A. On the contrary, suppose that x∗2>A≥M. Then, due to (T2) and (T3), we have g(x∗2)<g(A)=f(M)<f(x∗2)=g(x∗2), which is a contradiction. The claim is proved.
Next, for a given ϕ∈C[x∗1,ˆG(x∗1)]∖{x∗1}, let xϵ:=xϵ(ϕ) be the solution of problem (1.1) when replacing f by f+ϵ. Since xϵ converges to x as ϵ tends to zero, we only need to prove that there exists T>0 such that xϵ(t)<A for all t≥T. To this end, let yϵ:=yϵ(ϕ) be the solution of problem (1.1), when replacing f by f+ϵ and g by g+ with g+(s)=maxσ∈[0,s]g(σ). By Theorem 2.1, we have xϵ(t)≤yϵ(t) for all t≥0, and thus, we only need to show that yϵ(t)<A for all t≥T. On the contrary, we suppose that yϵ(t)≥A for all t>0. Then, combining the equation of yϵ and the fact that g+(s)=g(M) for all s≥M, we obtain
which is a contradiction. Then, there exists T>0 such that yϵ(T)<A. We further claim that yϵ(t)<A for all t≥T. Otherwise, there exists t1>T such that yϵ(t1)=A and y′ϵ(t1)≥0. Substituting yϵ(t1) in Eq (1.1), we get
which is a contradiction. Consequently, by passing to the limit in ϵ, we obtain that x(t)≤A for all t≥T.
We focus now on the lower bound of x. First, define the function
where x∗1<ˉm<M, which is the constant satisfying g(ˉm)=f(M). Note that, due to (T2)–(T4) and condition (3.5), the function g_ is nondecreasing over (0,A) and satisfies
Let y(ϕ) be the solution of problem (1.1) when replacing g by g_. From Theorem 2.1, we have y(t;ϕ)≤x(t;ϕ) for all t>0, and from Theorem 3.1, we have limt→∞y(t;ϕ)=M. Consequently, lim inft→∞x(t)≥M. In addition, if, for all T>0, there exists (tn)n such that tn>T, y(tn)=M, ˉm<y(s)≤A for all 0<s≤tn and y′(tn)<0, then, for tn>T+τ,
which is a contradiction. The lemma is established.
Denote ˉf=f|[M,A] the restriction function of f over [M,A] and G(s):=ˉf−1(g(s)) for s∈[M,A]. Now, we are ready to state our main theorem related to x∗2≥M.
Theorem 3.7. Under the assumptions of Lemma 3.6, the positive equilibrium x∗2 of problem (1.1) is globally asymptotically stable, provided that one of the following conditions holds:
(H1) fg is a nondecreasing function on [M,A].
(H2) f+g is a nondecreasing function over [M,A].
(H3) (GoG)(s)s is a nonincreasing function over [M,x∗2],
(H4) (GoG)(s)s is a nonincreasing function over [x∗2,A],
Proof. Denote x∞:=lim inft→∞x(t) and x∞:=lim supt→∞x(t). First, suppose that either x∞≤x∗2 or x∞≥x∗2. For x∞≤x∗2, we introduce the following function:
Let y(ϕ) be the solution of problem (1.1) when replacing g by g_. Since g_(s)≤g(s) for all x∗1≤s≤x∗2, we have y(t)≤x(t) for all t>0. Further, in view of Theorem 3.1, we obtain
The local stability is obtained by using the same idea as in the proof of Theorem 2.6 in [31].
Now, for x∞≥x∗2, we introduce the function
and let y(ϕ) be the solution of problem (1.1) when replacing g by ˉg. As above, we have x(t)≤y(t) for all t>0 and y(t) converges to x∗2 as t goes to infinity. Next, we suppose that x∞<x∗2<x∞ and we prove that it is impossible. Indeed, according to Lemma 3.6, we know that, for every solution x of problem (1.1), we have M≤x∞≤x∞≤A.
Now, using the fluctuation method (see [28,32]), there exist two sequences tn→∞ and sn→∞ such that
and
Substituting x(tn) in problem (1.1), it follows that
Since g is nonincreasing over [M,A], we obtain, by passing to the limit in Eq (3.8), that
Similarly, we obtain
Multiplying the expression (3.9) by g(x∞) and combining with inequality (3.10), we get
This fact, together with the hypothesis (H1), gives x∞≤x∞, which is a contradiction. In a similar way, we can conclude the contradiction for (H2). Now, suppose that (H3) holds. First, notice that G makes sense, that is, for all s∈[M,A], the range of g is contained in [ˉf(M),ˉf(A)] since ˉf is strictly increasing over [M,A]. In fact, for all s∈[M,A] and since g is non-increasing over [M,A], we have g(A)≤g(s)≤g(M). Now, using the fact that f(A)≥f(M), we show that
Therefore, the function G is nonincreasing and maps [M,A] to [M,A]. In view of inequalities (3.9) and (3.10) and the monotonicity of ˉf, we arrive at
and
with G(s)=ˉf−1(g(s)). Now, applying the function G to inequalities (3.11) and (3.12), we find
which gives
Due to (H3), it ensures that x∗2≤x∞, which is impossible. Using the same arguments as above, we obtain a contradiction for (H4). The theorem is proved.
Remark 3.8. In the case where g(s)>g(A) for all s>M, the two above results hold true. In fact, it suffices to replace A in Lemma 3.6 and Theorem 3.7 by B defined in (T1).
For the tangential case where two positive equilibria x∗1 and x∗2 are equal, we have the following theorem
Theorem 3.9. Suppose that (T1)–(T3) hold. Suppose that, in addition to the trivial equilibrium, problem (1.1) has a unique positive equilibrium x∗1. Then
(i) for ϕ∈C+, there exists T>0 such that 0≤x(t)≤M for all t≥T.
(ii) ϕ∈C[x∗1,ˆG(x∗1)]∖{x∗1} implies that x∗1<x(t)≤M for all t≥T.
(iii) if ϕ∈C[x∗1,ˆG(x∗1)], then x∗1 attracts every solution of problem (1.1) and x∗1 is unstable.
Proof. The uniqueness of the positive equilibrium implies that x∗1≤M and g(x)<f(x) for all x≠x∗1. For (ⅰ), suppose that there exists t0>0 such that x(t)≥M for all t≥t0. Then, by substituting x in Eq (1.1), we get
This is impossible and then there exists T>0 such that x(T)<M. Next, if there exists t1>T such that x(t1)=M and x(t)≤M for all t≤t1, then
Consequently (ⅰ) holds. We argue as in the proof of Lemma 3.2 (ⅰ), to show (ⅱ). Concerning (ⅲ), we consider the following Lyapunov functional
where ψ(a)=∫τah(σ)dσ. As in the proof of Theorem 3.1, the derivative of V along the solution of problem (1.1) gives
Since g(s)<f(s) for all s≠x∗1 and g(s)≥g(x∗1) for all s∈[x∗1,M], we have dV(xt)/dt≤0. By LaSalle invariance theorem, x∗1 attracts every solution x(ϕ) of problem (1.1) with ϕ∈C[x∗1,ˆG(x∗1)]. From Theorems 2.5 and 3.4, we easily show that x1 is unstable.
4.
Application to a model with Allee effect and distributed delay
In this section, we apply our results to the following distributed delay differential equation:
where μ, k are positive constants. The variable x(t) stands for the maturated population at time t and τ>0 is the maximal maturation time of the species under consideration. h(a) is the maturity rate at age a. In this model, the death function f(x)=μx and the birth function g(x)=kx2/(1+2x3) reflect the so called Allee effect. Obviously, the functions f and g satisfy the assumptions (T1)–(T3) and g reaches the maximum value k/3 at the point M=1. The equilibria of Eq (4.1) satisfies the following equation:
Analyzing Eq (4.2), we obtain the following proposition.
Proposition 4.1. Equation (4.1) has a trivial equilibrium x=0. In addition
(i) if μ>2133k, then Eq (4.1) has no positive equilibrium;
(ii) if μ=2133k, then Eq (4.1) has exactly one positive equilibrium x∗1;
(iii) if 0<μ<2133k, then Eq (4.1) has exactly two positive equilibria x∗1<x∗2. Moreover,
(iii)-a if k3<μ<2133k, then 0<x∗1<x∗2<1;
(iii)-b if 0<μ≤k3, then 0<x∗1<1≤x∗2.
Using Theorem 2.6, we obtain
Theorem 4.2. If μ>2133k, then the trivial equilibrium of Eq (4.1) is globally asymptotically stable for all ϕ∈C+.
For the case (ⅲ)-a in Proposition 4.1 we have the following result
Theorem 4.3. Suppose that k3<μ<2133k. Then
(i) The trivial equilibrium of Eq (4.1) is globally asymptotically stable for all ϕ∈C[0,x∗1]∖{x∗1}.
(ii) The positive equilibrium x∗1 is unstable and the positive equilibrium x∗2 is globally asymptotically stable for all ϕ∈C[x∗1,ˆG(x∗1)]∖{x∗1}, where ˆG(x∗1)∈[1,∞) satisfies g(ˆG(x∗1))=g(x∗1).
(iii) There exists two heteroclinic orbits X(1) and X(2) connecting 0 to x∗1 and x∗1 to x∗2, respectively.
Proof. (ⅰ) and (ⅱ) directly follow from Proposition 4.1, (ⅲ)-a and Theorems 2.5 and 3.4.
For (ⅲ), we follow the same proof as in Theorem 4.2 in [5]. See also [18]. For the sake of completeness, we rewrite it. Let K={x∗1}. Clearly, K is an isolated and unstable compact invariant set in C[0,x∗1] and C[x∗1,ˆG(x∗1)].
By applying Corollary 2.9 in [33] to Φ|R+×C[0,x∗1] and Φ|R+×C[x∗1,ˆG(x∗1)], respectively, there exist two pre-compact full orbits X(1):R→C[0,x∗1]∖{0} and X(2):R→C[x∗1,ˆG(x∗1)]∖{0} such that α(X1)=α(X2)=K. This together with statements (i) and (ii) gives ω(X(1))={0} and ω(X(2))={x∗2}. In other words, there exist two heteroclinic orbits X(1) and X(2), which connect 0 to x∗1 and x∗1 to x∗2, respectively. This completes the proof.
For the case (ⅲ)-b in Proposition 4.1, we first show that
Lemma 4.4. Suppose that f(s)=μs. Condition (4.3) holds if and only if M≤1μg(1μg(M)).
Proof. It follows that f(A)≥g(M) if and only if A≥f−1(g(M))≥M. Since g is a decreasing function over [M,∞), we have g(A)≤g(f−1(g(M)))=g(1μ(g(M))). Moreover, since g(A)=f(M), we have M≤1μg(1μg(M)). The lemma is proved.
Lemma 4.5. For Eq (4.1), condition (4.3) holds if and only if
Proof. By a straightforward computation, we have f−1(x)=x/μ. For G(x)=g(x)/μ, we obtain
where p=k3/μ3. By applying Lemma 4.4, it is readily to see that 1≤(GoG)(1) holds if and only if
and thus, 0<μ≤k/3.
Theorem 4.6. Suppose that 0<μ≤k3. Then, the positive equilibrium x∗2 of Eq (4.1) is globally asymptotically stable for all ϕ∈C[x∗1,ˆG(x∗1)]∖{x∗1}, where ˆG(x∗1)∈[1,∞) satisfies g(ˆG(x∗1))=g(x∗1).
Proof. Observe that, in view of Lemmas 4.4 and 4.5, all hypotheses of Lemma 3.6 are satisfied. Now, in order to apply Theorem 3.7, we only show that (GoG)(x)x is nonincreasing over [M,A]. Indeed, by a simple computation, we have
where p=k3/μ3. Finally, observe that
for x≥1. In this case, both of (H3) and (H4) in Theorem 3.7 hold. This completes the proof.
Finally, for the tangential case, we have
Theorem 4.7. Suppose that μ=2133k. Then
(i) The trivial equilibrium of Eq (4.1) is globally asymptotically stable for all ϕ∈C[0,x∗1]∖{x∗1}.
(ii) If ϕ∈C[x∗1,ˆG(x∗1)]∖{x∗1}, then the unique positive equilibrium x∗1 is unstable and attracts every solution of Eq (4.1).
Proof. The proof of this theorem follows immediately from Theorems 2.5 and 3.9.
5.
Numerical simulation
In this section, we perform numerical simulation that supports our theoretical results. As in Section 4, we assume that
and confirm the validity of Theorems 4.2, 4.3, 4.6 and 4.7. In what follows, we fix
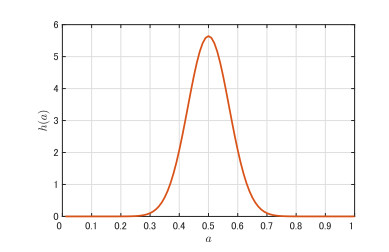
and change μ and ϕ. Note that 2133k≈0.42 and h is a Gaussian-like distribution as shown in Figure 1.
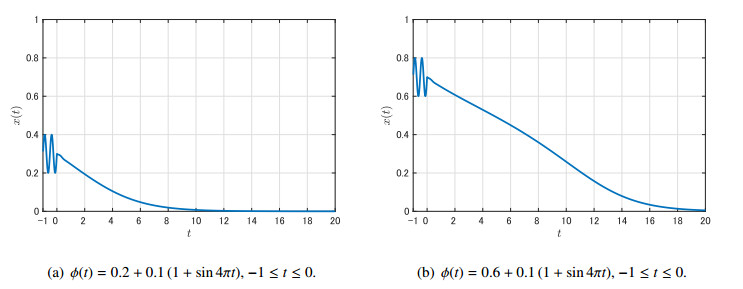
First, let μ=0.5. In this case, μ>2133k and thus, by Theorem 4.2, the trivial equilibrium is globally asymptotically stable. In fact, Figure 2 shows that x(t) converges to 0 as t increases for different initial data.
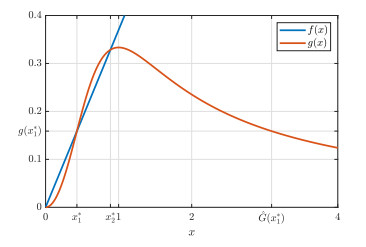
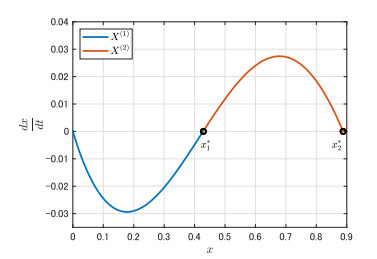
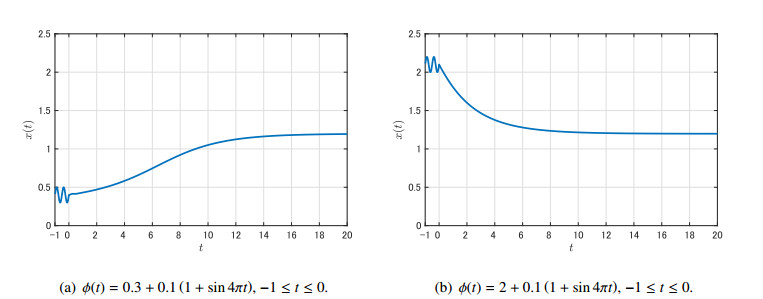
Next, let μ=0.37. In this case, k3<μ<2133k and x∗1≈0.43, x∗2≈0.89 and ˆG(x∗1)≈3.09 (Figure 3). Hence, by Theorem 4.3, we see that the trivial equilibrium is globally asymptotically stable for ϕ∈C[0,x∗1]∖{x∗1}, whereas the positive equilibrium x∗2 is so for ϕ∈C[x∗1,ˆG(x∗1)]∖{x∗1}. In fact, Figure 4 shows such a bistable situation. Moreover, heteroclinic orbits X(1) and X(2), which were stated in Theorem 4.3 (ⅲ), are shown in Figure 5.
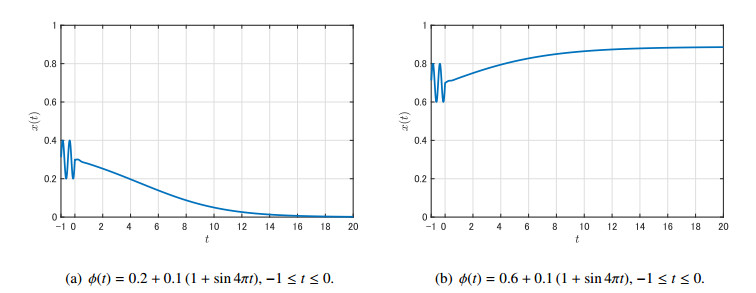
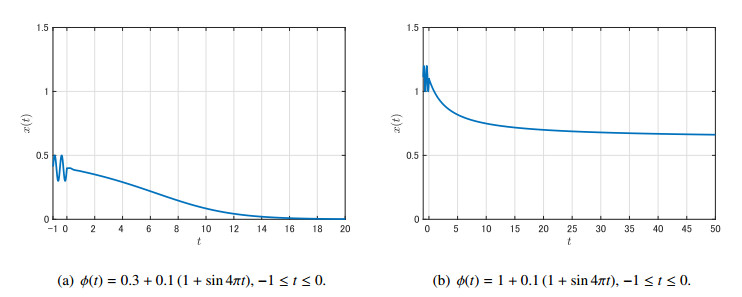
Thirdly, let μ=0.27<k3≈0.33. In this case, we have x∗1≈0.28, x∗2≈1.2 and ˆG(x∗1)≈6.52 (Figure 6). Thus, by Theorem 4.6, we see that the positive equilibrium x∗2 is globally asymptotically stable for ϕ∈C[x∗1,ˆG(x∗1)]∖{x∗1}. In fact, Figure 7 shows that x(t) converges to x∗2 as t increases for different initial data.
Finally, let μ=2133k≈0.42. In this case, we have x∗1≈0.63 and ˆG(x∗1)≈1.72 (Figure 8). By Theorem 4.7, we see that the trivial equilibrium is globally asymptotically stable for ϕ∈C[0,x∗1]∖{x∗1}, whereas x∗1 is unstable and attracts all solutions for ϕ∈C[x∗1,ˆG(x∗1)]∖{x∗1}. In fact, Figure 9 shows such two situations.
6.
Conclusions
In this paper, we studied the bistable nonlinearity problem for a general class of functional differential equations with distributed delay, which includes many mathematical models in biology and ecology. In contrast to the previous work [5], we considered both cases x∗2<M and x∗2≥M, and obtained sufficient conditions for the global asymptotic stability of each equilibrium. The general results were applied to a model with Allee effect in Section 4, and numerical simulation was performed in Section 5. It should be pointed out that our theoretical results are robust for the variation of the form of the distribution function h. This might suggest us that the distributed delay is not essential for the dynamical system of our model. We conjecture that our results still hold for τ=∞, and we leave it for a future study. Extension of our results to a reaction-diffusion equation could also be an interesting future problem.
Acknowledgments
We deeply appreciate the editor and the anonymous reviewers for their helpful comments to the earlier version of our manuscript. The first author is partially supported by the JSPS Grant-in-Aid for Early-Career Scientists (No.19K14594). The second author is partially supported by the DGRSDT, ALGERIA.
Conflict of interest
All authors declare no conflicts of interest in this paper.









 DownLoad:
DownLoad: