The fractional Malmheden theorem

1.   Introduction

In 1934, Harry William Malmheden ^[13] proved a simple algorithm to compute the value of a harmonic function at a point of $B_1$, knowing its value on the boundary.

The Malmheden theorem makes use of two fundamental geometric ingredients:

$1)$ the notion of affine interpolation between the values of a given function at two different points of the space,

$2)$ the projections of a point inside a ball to the boundary in a given direction.

Hence, to state the Malmheden theorem explicitly, we now formalize these two notions into a precise mathematical setting. We start by introducing a notation for the affine interpolation between the values of some given function. That is, given a set $K\subseteq \mathbb{R}^n$, a function $f:K\to \mathbb{R}$, two distinct points $a$, $b\in K$, and a point $x$ on the segment $L$ joining $a$ and $b$, we define $\mathcal{L}_{f}^{a, b}(x)$ as the affine function on $L$ such that $\mathcal{L}_{f}^{a, b}(a) = f(a)$ and $\mathcal{L}_{f}^{a, b}(b) = f(b)$.

Of course, one can write this affine function explicitly by using the analytic expression

One can call $\mathcal{L}_{f}^{a, b}(x)$ the "affine function of $f$ with extrema $a$ and $b$ evaluated at the point $x$".

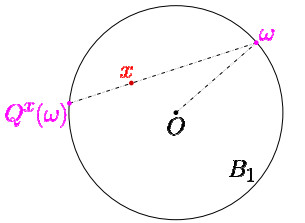
Now we discuss the notation regarding the projections of a point inside a ball to the boundary of the ball in a given direction. For this, given a point $x\in B_1$ and a direction $e\in \partial B_1$ we consider the intersections $Q_{+}^x(e)$ and $Q_{-}^x(e)$ of $\partial B_1$ with the straight line passing through $x\in B_1$ with direction $e$, with the convention that $Q_{+}^x(e)-Q_{-}^x(e)$ has the same orientation of $e$, see Figure 1.

Clearly, from the analytic point of view, one can write explicitly these projections in the form

where

We note from Eqs (1.2) and (1.3) that $Q_{\pm}^x(e)$ are continuous functions in $(x, e)\in B_1\times \partial B_1$. Moreover,

for each $e\in \partial B_1$. This tells us that the maps $Q_{\pm}^x$ simply reduce to $\pm id_{\partial B_1}$ when $x = 0$.

Given a boundary datum $f:\partial B_1\to \mathbb{R}$, the core of the Malmheden Theorem is thus to consider, for every point $x\in B_1$ and every direction $e\in\partial B_1$, the affine function of $f$ with extrema $Q_{-}^x(e)$ and $Q_{+}^x(e)$, namely the function

and then to average in all directions $e$.

The remarkable result by Malmheden is that this averaging procedure of linear interpolations produces precisely the solution of the classical Dirichlet problem in $B_1$ with boundary datum $f$, according to the following classical statement (see ^[13]):

Theorem 1.1 (Malmheden theorem). Let $n \geqslant2$ and $f:\partial B_1\rightarrow \mathbb{R}$ be continuous. Then

is the harmonic function in $B_1$ with boundary datum $f$.

As usual, here above and in the rest of this paper, we denoted by $H^{n-1}$ the $(n-1)$-Hausdorff measure (hence, the integral on the right hand side of (1.6) is simply the spherical integral along $\partial B_1$; we kept the explicit notation with the Hausdorff measure to have a typographical evidence of the surface integrals, to be distinguished by the classical volume ones).

We remark that Theorem 1.1 contains the Mean Value Theorem for harmonic functions as a particular case: indeed, in light of (1.1) and (1.4), if we take $x: = 0$ then (1.6) reduces to

which is the content of the Mean Value Theorem.

We also stress that an elegant result such as Theorem 1.1 is specific for balls and cannot be extended in general to other domains, as pointed out in ^[1].

Interestingly, Theorem 1.1 contains as a particular case a classical result due to Hermann Amandus Schwarz ^[18] about the Dirichlet problem in the plane and related to conformal mappings in the complex framework.

To state Schwarz result it is convenient to introduce the reflection of a point $\omega\in\partial B_1$ through a point $x\in B_1$, see Figure 2. More precisely, given $x\in B_1$ and $\omega\in \partial B_1$ we define

Comparing with (1.2), one sees that if $e: = \frac{\omega-x}{|\omega-x|}$ then $Q_+^x(e) = \omega$ and $Q_-^x(e) = Q^x(\omega)$.

In this setting, the result by Schwarz is that the average of the boundary datum composed with the above reflection returns the solution of the Dirichlet problem in the ball. More explicitly:

Theorem 1.2 (Schwarz theorem). Let $n = 2$ and $f:\partial B_1\rightarrow \mathbb{R}$ be continuous. Then

is the harmonic function in $B_1$ with external datum $f$.

Theorem 1.2 can be proved in several ways using either complex or real analysis (see e.g., ^[9,15,17]), but it is also a direct consequence of Theorem 1.1, see e.g., ^[8] for a detailed presentation of this classical argument.

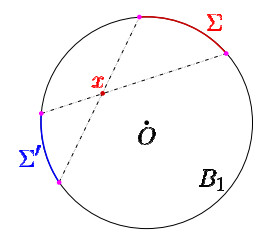
Example 1.3. A very neat application of Theorem 1.2 (see e.g., ^[16]) consists in the determination of the stationary temperature $u$ at a point $x$ in a plate (say $B_1$) when the temperature along the boundary of the plate is $1$ along some arc $\Sigma$ and $0$ outside. In this case, the reflection in (1.8) sends $\Sigma$ into an arc $\Sigma'$ (the symmetric of $\Sigma$ through $x$, see Figure 3) and it therefore follows from Theorem 1.2 that

where $|\Sigma'|$ is the length of the arc $\Sigma'$, thus providing an elementary geometric construction to solve a problem of physical relevance.

The objective of this paper is to obtain a fractional counterpart for the Malmheden and Schwarz theorems.

We will thus replace the notion of harmonic functions in $B_1$ with that of $s$-harmonic functions, namely functions whose fractional Laplacian vanishes in $B_1$, that is, for all $x\in B_1$,

where the integral above is intended in the principal value sense. Here above and throughout the paper the fractional parameter $s\in(0, 1)$.

Rather than a boundary value along $\partial B_1$, as usual in the nonlocal setting, we complement (1.10) with an external condition of the type $u = f$ in $\mathbb{R}^n\setminus B_1$.

We recall that in general $s$-harmonic functions behave way more wildly that their classical counterparts, see e.g., ^[7]. Therefore, in principle one cannot easily expect that a "simple formulation" such as the one in Theorems 1.1 and 1.2 accounts for all the complex situations arising in the fractional setting.

However, we will prove that a counterpart of Theorems 1.1 and 1.2 carries over to the case of the fractional Laplacian, considering the following structural modifications:

$1)$ the classical spherical averages are replaced by suitable weighted averages on spheres of radius larger than $1$,

$2)$ the geometric transformations in (1.2) and (1.8) are scaled in dependence of the radius of each of these spheres.

To clarify these points, and thus reconsider (1.5) in a nonlocal setting, given $\rho > 1$ and $f: \mathbb{R}^n\setminus B_1\to \mathbb{R}$, for all $x\in\partial B_1$ we define

Hence, in the notation of (1.1), we define

Notice that when $\rho = 1$ the above setting reduces to (1.5), otherwise one is considering here a similar framework but for a rescaled version of the function $f$ and rescaled points.

To detect the long-range effect of the fractional Laplacian, it is also useful to consider the kernel

where

For our purposes, the kernel ${\mathcal{E}}$ will play the role of a suitable spherical average^* of a fractional Poisson kernel and the constant $c(n, s)$ is merely needed for normalization purposes.

^*More precisely, the intuition behind ${\mathcal{E}}$ is \label{INTUK} that it satisfies, for each $x\in B_1$ and $\rho\in(1, +\infty)$,

where $P$ is the fractional Poisson kernel.

This relation can be obtained as a consequence of our fractional Malmheden theorem. Though our approach does not pass explicitly through this identity, for the sake of completeness we provide an independent proof in Appendix A.1.

We also define the space

With this, we can state the main result of this paper as follows:

Theorem 1.4 (fractional Malmheden theorem). Let $n \geqslant 2$, $s\in (0, 1)$, $R > 1$and $f\in L^\infty(B_{R}\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$.

Then, the unique solution (up to a zero measure subset of $\mathbb{R}^n\setminus B_1$) to the problem

can be written, for each $x\in B_1$, as

As a fractional counterpart of the observation in (1.7), we point out that Theorem 1.4 entails as a straightforward consequence the Mean Value Formula for $s$-harmonis functions. Indeed, by the changes of variable $e: = \omega/|\omega|$ and $y: = \rho\omega/|\omega|$,

which is the Mean Value Formula for $s$-harmonis functions, see e.g., formula (1.3) in ^[4].

We consider Theorem 1.4 as the natural fractional counterpart of Theorem 1.1 and we mention that indeed one can "recover" Theorem 1.1 in the limit as $s\nearrow1$, according to the following result:

Proposition 1.5. Let $n \geqslant 2$, $s_0\in (0, 1)$, $R > 1$and $f\in C(B_{R}\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$ for each $s\in (s_0, 1]$. Then, for each $x\in B_1$, it holds that

where $u_f^{(s)}$ and $u_f$ are defined in $(1.17)$ and $(1.6)$, respectively.

As a straightforward consequence of the classical Malmheden theorem (Theorem 1.1) and its fractional formulation theorem (Theorem 1.4), we deduce the following result.

Theorem 1.6 (An $s$-harmonic function is the superposition of classical harmonic functions). Let $n \geqslant 2$, $s\in (0, 1)$ and $f\in C(\mathbb{R}^n\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$. For each $\rho > 1$ we define ${u}_{f_\rho}$ as the unique solution to the Dirichlet problem

where $f_\rho$ is defined in $(1.11)$.

Then the unique solution $u_f^{(s)}$ to $(1.16)$ can be written as

The interest of Theorem 1.6 is that it allows us to write an $s$-harmonic function in $B_1$ as a weighted integral of classical harmonic functions, where the weight coincide with $\mathcal{E}(x, \rho)$. Besides being interesting in itself, this result is very useful to deduce properties of $s$-harmonic functions, as the Harnack inequality (see Section 4), starting from their local counterpart.

As a matter of fact, as a consequence of Theorem 1.6 one obtains a new proof of the Harnack inequality for $s$-harmonic functions in $B_1$. The result goes as follows:

Theorem 1.7 (Harnack inequality). Let $n \geqslant 2$, $s\in (0, 1)$, $R > 1$ and $u$ be non negative, $s$-harmonic in $B_1$ and such that $u \in L^\infty(B_{R}\setminus B_1)\cap L_s^1(\mathbb{R}^n \setminus B_1)$.

Then, for each $r\in(0, 1)$ and $x\in B_r$,

The constants in $(1.20)$ are optimal, and for $s\nearrow 1$ they converge to the optimal constants of the classical Harnack inequality in $B_r$ for harmonic functions in $B_1$.

For different proofs of the fractional Harnack inequality see ^[6,11,12] and the references therein.

Another consequence of Theorem 1.4 is the fractional version of Schwarz result:

Theorem 1.8 (fractional Schwarz theorem). Let $n = 2$, $s\in (0, 1)$, $R > 1$and $f\in L^\infty(B_R\setminus B_1)\cap L_s^1(\mathbb{R}^2\setminus B_1)$.Then, the unique solution (up to a zero measure subset of $\mathbb{R}^2\setminus B_1$) to the problem

can be written, for each $x\in B_1$, as

This is a fractional counterpart of Theorem 1.2, in the sense of Proposition 1.9 below. Proposition 1.9 is a straightforward consequence of Theorems 1.2 and 1.8 and Proposition 1.5.

Proposition 1.9. Let $n = 2$, $s_0\in (0, 1)$, $R > 1$and $f\in C(B_R\setminus B_1)\cap L_s^1(\mathbb{R}^2\setminus B_1)$ for each $s\in (s_0, 1]$. Then, for each $x\in B_1$, it holds that

where $u_f^{(s)}$, $u_f$ are defined in $(1.21)$ and $(1.9)$, respectively.

Remark 1.10. It is worth pointing out that from Theorem 1.4 we can evince the identity

for each $x\in B_1$. Indeed, if we consider as external data $f = 1$ in $\mathbb{R}^n\setminus B_1$, then the unique solution to the problem (1.16) is $u = 1$ in $\mathbb{R}^n$. Therefore, according to (1.17) and the fact that in this case the linear interpolation $\mathcal{L}_{1, e, \rho}(x) = 1$ for each $x\in B_1$, we obtain that

which gives (1.22).

As an application of Theorem 1.8, we have:

Example 1.11. Let $n = 2$ and take an arc $\Sigma\subset\partial B_1$. Consider the function defined on $\mathbb{R}^2\setminus B_1$ as

It is clear that $\tilde{\chi}_{\Sigma}$ is positively homogeneous of degree zero, and furthermore $\tilde{\chi}_{\Sigma}\in L^\infty (\mathbb{R}^2\setminus B_1)\subset L_s^1(\mathbb{R}^2\setminus B_1)$. Then by Theorem 1.8 we get that for each $x\in B_1$

where $\Sigma_{x/\rho}'$ is the projected arc of $\Sigma$ on $\partial B_1$ through the focal point $x/\rho$, as constructed in Example 1.3. We denoted with $\vert \Sigma_{x/\rho}'\vert$ its length.

This gives a simple geometrical procedure to compute the solution of

at a point $x$ of the two dimensional disc when the non local boundary condition is given by (1.23). Note that as $\rho$ is getting larger, the measure of $\Sigma_{x/\rho}'$ reaches the one of $\Sigma$, or more precisely

If $x = 0$, formula (1.24) boils down to

where we have applied identity (1.22). This example can be seen as the fractional counterpart of Example 1.3.

This paper is organized as follows. In Section 2 we give some preliminary results on the $s$-harmonic function written as a convolution with the fractional Poisson kernel.

Section 3 is devoted to the proofs of the fractional Malmheden and Schwarz results, that is Theorems 1.4 and 1.8, and of the convergence result in Proposition 1.5.

In Section 4 we use these results to provide a proof of the well-known Harnack inequality for $s$-harmonic functions under some regularity assumptions on the external datum $f:\mathbb{R}^n\setminus B_1\rightarrow \mathbb{R}$, that is we prove Theorem 1.7.

2.   Preliminary results on the fractional Poisson kernel

In this section, we revisit the well-established result according to which fractional harmonic functions can be represented as an integral of the datum outside the domain against a suitable Poisson kernel. For completeness, we extend this result to the case in which the datum is not necessarily continuous, so to be able to present the results of this paper in a suitable generality. Notice that the extension to functions that are not necessarily continuous is also useful for us to comprise situations as in Example 1.11.

The framework that we consider is the following. For $n \geqslant2$ and $s\in (0, 1)$, we consider the space $L_s^1(\mathbb{R}^n\setminus B_1)$ as defined in (1.15). Given $f\in L_s^1(\mathbb{R}^n\setminus B_1)$, we denote the norm on $L_s^1(\mathbb{R}^n\setminus B_1)$ by

Furthermore, we define the following fractional Poisson kernel in the unit ball

for $x\in B_1$ and $y\in \mathbb{R}^n\setminus B_1$, and $c(n, s)$ is the normalizing constant in (1.14).

As customary, the role of the constant $c(n, s)$ is to normalize the Poisson kernel, namely we have that

see e.g., formula (1.14) and Lemma A.5 in ^[3].

We also remark that

and, for every $\rho\in(0, 1)$, $\alpha\in \mathbb{N}^n$ and $y\in \mathbb{R}^n\setminus B_1$,

where $C_\rho > 0$ depends only on $\rho$, $n$ and $s$ and, as usual, we have denoted the length of the multi-index $\alpha$ as $|\alpha|: = \alpha_1+\dots+\alpha_n$.

Then, we define

and we have the following result on the representation of $s$-harmonic functions:

Theorem 2.1. Let $n \geqslant2$, $s\in (0, 1)$ and $f\in C(\mathbb{R}^n\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$. Then the function in $(2.4)$ is the unique pointwise continuous solution to the problem

For a proof of Theorem 2.1 see e.g. Theorem 2.10 in ^[3].

We now generalize Theorem 2.1 by allowing external data that are not necessarily continuous:

Proposition 2.2. Let $n \geqslant2$, $s\in (0, 1)$, $R > 1$ and $f\in L^\infty(B_{R}\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$. Then the function defined in $(2.4)$ is the unique solution (up to a zero measure subset of $\mathbb{R}^n\setminus B_1$) to the problem in $(2.5)$.

Proof. We argue by approximation, owing to Theorem 2.1. The gist is indeed to take a sequence of continuous functions $f_k$ approaching $f$ as $k\to+\infty$, use Theorem 2.1 and then pass to the limit. To implement this idea, one needs to take care of some regularity issues.

The details of this technical argument go as follows. By (2.3), for each $x\in B_1$ and multi-index $\alpha$ we have that

As a consequence, we obtain that $u_f^{(s)}(x)$ in (2.4) is well defined and smooth inside $B_1$.

To complete the proof of Proposition 2.2, we need to show that $u_f^{(s)}$, as defined in (2.4), is the unique solution of (2.5). To do so, we start by checking that $u_f^{(s)}$ is $s$-harmonic in $B_1$. We consider a sequence $\lbrace f_k\rbrace_{k}\subset C(\mathbb{R}^n\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$, such that

More specifically, we take $f_k: = (\chi_{B_k} \tilde{f})*\eta_{\frac{1}{k}}$ with $k \geqslant 2$, where $\tilde{f}$ is defined as

and $\eta_{\frac{1}{k}}$ is a mollifier of radius $\frac{1}{k}$, while $\chi_{B_k}$ is the characteristic function of $B_k$. We also let $u_{f_k}^{(s)}$ be the unique pointwise continuous solution to the problem (2.5), according to Theorem 2.1.

Then we have that for each multi-index $\alpha$

Indeed for each multi-index $\alpha$ and $g\in L^\infty(B_R\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$ one finds that

for each $x\in B_1$, and therefore, choosing $R_0\in(1, R)$, we see that, for every $x\in B'$ with $B'\Subset B_1$,

where $c$ is a positive constant depending on $\alpha$, $R_0$, $n$, $s$ and $B'$. The first term in the third line in (2.8) converges to zero as $k\to+\infty$, thanks to (2.6). We also observe that, if $y\in B_{R_0}\setminus B_1$, then

and therefore, by the dominated convergence theorem, we have that also the second term in the third line in (2.8) converges to zero as $k\to+\infty$. These considerations prove (2.7).

Furthermore note that if $\alpha = 0$, taking $R_0\in(1, R)$ and using also (2.2), we have that, for all $x\in B_{1}$,

where $C$ is a positive constant depending on $R_0$, $n$ and $s$. Now, we observe that the sequence $\Vert f_k\Vert_{L_s^1(\mathbb{R}^n \setminus B_1)}$ is uniformly bounded, thanks to (2.6). Accordingly, from (2.9) we see that

Now, if $x\in B_1$, taking $\delta\in\left(0, 1-|x| \right)$, we have that

where

Notice that

which converges to zero as $k\to+\infty$, thanks to (2.7).

Furthermore, we observe that if $y\in\mathbb{R}^n\setminus B_1$ then

and thus

which, in light of (2.6), converges to zero as $k\to+\infty$.

Moreover, from (2.7) and the dominated convergence theorem, we see that the quantity $C+D$ converges to zero as $k\to+\infty$.

Finally, recalling (2.10) and making again use of the dominated convergence theorem, we have that $F$ converges to zero as $k\to+\infty$.

These considerations and (2.11) give that $(-\Delta)^s u_{f_k}^{(s)}(x)$ converges to $(-\Delta)^s u_f^{(s)}(x)$ as $k\to+\infty$ for every $x\in B_1$. Since, by Theorem 2.1, we know that $(-\Delta)^su_{f_k}^{(s)}(x) = 0$ for each $x\in B_1$, we conclude that $(-\Delta)^s u_f^{(s)}(x) = 0$. This proves that $u_f^{(s)}$ solves (2.5).

It is only left to show the uniqueness statement. Suppose that there exists $u_1:\mathbb{R}^n\rightarrow \mathbb{R}$ satisfying

Then both $v: = u_f^{(s)}-u_1$ and $-v = u_1- u_f^{(s)}$ are solutions to

and therefore by the maximum principle for the fractional Laplacian (see e.g., Theorem 2.3.2. in ^[5]) we have that $v = 0$ in $B_1$, leading to uniqueness.

3.   Proof of the fractional Malmheden and Schwarz theorems

In this section we provide the proofs of the fractional Malmheden and Schwarz results, as stated in Theorems 1.4 and 1.8, and of the convergence result in Proposition 1.5.

We start with the main argument to prove Theorem 1.4. For this, we employ the following change of variable result (see Lemma 2.13.3 in ^[8] for the proof of it):

Lemma 3.1. Let $n \geqslant 2$, $x\in B_1$ and $Q_{\pm}^x$ and $r_{\pm}^x$be defined as in $(1.2)$ and $(1.3)$, respectively. Then for each $e\in \partial B_1$ it holdsthat

and for each continuous $f:\partial B_1\rightarrow \mathbb{R}$ we have that

With this notation, Theorem 1.4 will be a consequence of the following statement.

Theorem 3.2. Let $n \geqslant 2$, $s\in (0, 1)$, $R > 1$and $f\in L^\infty(B_R\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$.Let $u_f^{(s)}$ be as in $(2.4)$.

Then, for each $x\in B_1$,

where the notation in $(1.12)$ and $(1.13)$ has been used.

Furthermore, if $f$ is positively homogeneous of degree $\gamma$ for some $\gamma \geqslant 0$, then we have that

Proof. We first suppose that $f\in C(\mathbb{R}^n\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$. Let $x\in B_1$, then, using polar coordinates, from (2.1) and (2.4) we get that

Hence, defining

and applying Lemma 3.1 we obtain that

From Eqs (1.2) and (1.3) we deduce that

and also (see formula (2.13.25) in ^[8])

which, together with (3.4), gives that

We now observe that

From (1.2), (1.3), (1.11) and (1.12) we also deduce that

Plugging this information into (3.6) we find that

which in turn, together with (3.5), gives that

Thus, recalling (1.13), this and (3.3) establish the desired result in (3.1) under the additional assumption that $f$ is continuous in $\mathbb{R}^n\setminus B_1$.

Now we remove the continuity assumption on $f$ by an approximation argument. Given $f\in L^\infty(B_R\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$, we consider a sequence of functions $\lbrace f_k\rbrace_k\subset C(\mathbb{R}^n\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$ as in (2.6) and we let $u_{f_k}^{(s)}$ be the unique pointwise continuous solution to the problem (2.5), according to Theorem 2.1.

By (2.7),

Therefore we have that, for each $x\in B_1$,

From this, we claim that there exists a subsequence $\lbrace f_{k_j}\rbrace_j$, such that

For the convenience of the reader, the technical proof of (3.8) can be found in Appendix A.2.

The claim in (3.8) establishes the desired result in (3.1) also for non continuous functions.

To prove the claim in (3.2), we use (1.1) and (1.12) to see that

The claim in (3.2) then follows from this and (3.1). This concludes the proof of Theorem 3.2.

Proof of Theorem 1.4. From Proposition 2.2, we know that, under the hypotheses of Theorem 1.4, the function defined in (2.4) is the unique solution (up to a zero measure subset of $\mathbb{R}^n\setminus B_1$) to the problem (1.16). Then, the desired result in Theorem 1.4 follows from this and Theorem 3.2.

We now give the proof of the Schwarz result in Theorem 1.8.

Proof of Theorem 1.8. We first suppose that $f\in C(\mathbb{R}^2\setminus B_1)\cap L_s^1(\mathbb{R}^2\setminus B_1)$. Under these assumptions we can apply Theorem 1.6 in dimension $2$ and get

where $\tilde{u}_{f_\rho}$ has been defined in the statement of Theorem 1.6. Therefore, when $n = 2$ we can apply Theorem 1.2 to $\tilde{u}_{f_\rho}$ and get

which leads to (1.21) in the case in which $f\in C(\mathbb{R}^2\setminus B_1)$.

Suppose now that $f\in L^\infty(B_{R}\setminus B_1)\cap L_s^1(\mathbb{R}^2\setminus B_1)$, and consider a sequence of functions $\lbrace f_k\rbrace_k\subset C(\mathbb{R}^2\setminus B_1)\cap L_s^1(\mathbb{R}^2\setminus B_1)$ as in (2.6). We let $u_{f_k}^{(s)}$ be the unique pointwise continuous solution to the problem (2.5), according to Theorem 2.1. From the previous step, we have that, for each $x\in B_1$,

By this and (3.7), we have that, for each $x\in B_1$,

From this, one sees that there exists a subsequence $\lbrace f_{k_j}\rbrace_{j}$ such that

For the facility of the reader, a detailed proof of (3.9) is given in Appendix A.3.

We observe that the proof of Theorem 1.8 is completed, thanks to (3.9).

We now deal with the convergence result in Proposition 1.5.

Proof of Proposition 1.5. Let $f\in C(B_R\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$, for each $s\in (s_0, 1]$. Furthermore let $u_f^{(s)}$ and ${u}_f$ as in the statement of Proposition 1.5. Then, Theorem 1.1 implies that the following identity holds for each $\rho\in (1, R)$

thanks to (1.12), where ${u}_{f_\rho}$ is the unique solution to the classical Dirichlet problem (1.18).

In particular, we have that

Now, using (1.17), (1.22) and (3.10) we obtain for each $x\in B_1$ and $R_0\in(1, R)$ the following identity

By the continuity of ${u}_f$ and $f$, we have that for each $\delta > 0$ there exists some $R_0\in (1, R)$ such that for each $\rho\in(1, R_0)$

Also, we point out that

thanks to (1.12).

Therefore, from this, (3.10), (3.11), and (3.13), for all $x\in B_1$, we deduce that, if $\rho\in(1, R_0)$,

Now, if $x\in B_1$ and $\rho\in(1, R_0)$, we see that

and thus, according to (1.1),

and therefore, plugging this information into (3.14), we obtain that, if $x\in B_1$ and $\rho\in(1, R_0)$,

Furthermore, employing the change of variable $e: = \omega/|\omega|$ and recalling (1.13),

We also deduce from (1.1) the following pointwise estimate

We claim that

Indeed, if we define the following function

we see that it is $C^1$ and invertible. Note that, recalling also the limits in (1.4), one finds that

for some positive constant $C$, depending on $x$. Therefore by applying Theorem 2 in Section 3.3.3 of ^[10], we obtain that

This and the fact that $f\in L_s^1(\mathbb{R}^n\setminus B_1)$ for each $s\in (s_0, 1]$ entail that (3.20) holds true.

As a consequence of (3.19) and (3.20) we have that the integrals in (3.18) are finite and bounded in $s$.

Using this information and (3.17), we deduce from (3.12) that for each $\delta > 0$ there exists some $R_0\in (1, R)$ such that for each $\rho\in (1, R_0)$ we have

where ${C}(x, R_0)$ depends only on $x$ and $R_0$.

By taking the limit as $s\nearrow 1$, we see that

since $c(n, s)\to 0$ for $s\nearrow 1$ by (1.14). As a consequence

This and the arbitrariness of $\delta$ give the desired claim in Proposition 1.5.

4.   Harnack inequality

In this section we provide a new proof of the Harnack inequality for $s$-harmonic functions as stated in Theorem 1.7. Our strategy is to use the Fractional Malmheden Theorem to show that this result can be directly inferred from the classical Harnack inequality for harmonic functions.

Proof of Theorem 1.7. For convenience we call $u|_{\mathbb{R}^n\setminus B_1} = f$. Let us first assume that $f\in C(\mathbb{R}^n\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$. Under this assumption, we can apply Theorem 1.6 and obtain that

for each $x\in B_1$, where ${u}_{f_\rho}$ has been defined in the statement of Theorem 1.6. Therefore, we have that

Now we fix $r\in(0, 1)$ and we consider $x\in B_r$. Applying the Harnack inequality for classical harmonic functions to ${u}_{f_\rho}$, we have that

From this, (1.13) and (4.1) we obtain that

where for convenience we have called $t: = \vert x\vert$ in the last line and defined

with $(\rho, t)\in [1, \infty)\times [0, r]$.

Since $g(\rho, t)$ is decreasing in $\rho$ and increasing in $t$, we have that

Therefore, from this, (1.19) and (4.2) we obtain that

which establishes the first inequality in (1.20).

To prove the second inequality in (1.20), we make use of the Harnack inequality for harmonic functions, thus obtaining that

Using this and (1.13) into (4.1), we find that

Using again the notation $t: = \vert x\vert$, we define the following function

with $(\rho, t)\in [1, \infty)\times [0, r]$, and we see that

Since $g_1$ is increasing in $\rho$, we have that, for all $(\rho, t)\in [1, \infty)\times [0, r]$,

Notice also that $g_2$ is decreasing, and therefore, for all $(\rho, t)\in [1, \infty)\times [0, r]$,

Plugging this information into (4.4) and recalling (1.19), we get

which completes the proof of (1.20) under the additional continuity assumption on $f$.

To deal with the general case, we perform an approximation argument, whose details go as follows. If $f\in L^\infty(B_R\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$, we take a sequence $\lbrace f_k\rbrace_k\subset C(\mathbb{R}^n\setminus B_1)\cap L_s^1(\mathbb{R}^n\setminus B_1)$ as in (2.6). Then for $u_{f_k}^{(s)}$ the two-sided inequality in (1.20) holds true, thanks to the previous step. Also, by (3.7), we have the local uniform convergence

and therefore we deduce the two sided inequality (1.20) also in this case.

It is only left to prove that the constants provided in equation (1.20) are optimal. To show this let us fix some direction $e\in \partial B_1$, a constant $\epsilon > 0$ and the function

Then the function

is $s$-harmonic in $B_1$, as a consequence of Proposition 2.2. Therefore, if we fix $x = -re$ for $r\in (0, 1)$, we have that

and thus, by Lebesgue Differentiation Theorem, we conclude that

This proves that the constant on the left hand side inequality in (1.20) is optimal.

Similarly, taking $x = re$, one sees that

which shows that the constant on the right hand side inequality in (1.20) is also optimal. This concludes the proof of Theorem 1.7.

Acknowledgments

SD and EV are members of AustMS. Supported by the Australian Laureate Fellowship FL190100081 "Minimal surfaces, free boundaries and partial differential equations" and by the Australian Research Council DECRA DE180100957 "PDEs, free boundaries and applications".

Conflict of interest

The authors declare no conflict of interest.

Appendices

A.1.   Averaging the fractional Poisson kernel

Here we give a direct proof of the identity pointed out in the footnote on page 5. Namely, we establish that, if $x\in B_1$ and $\rho > 1$,

being $P$ the fractional Poisson kernel in (2.1).

The identity in (A.1) has its own interest and it can be deduced from our fractional Malmheden theorem, by taking a datum $f$ concentrating along a given sphere $\partial B_\rho$. For the sake of completeness however, we provide here an independent proof, only based on elementary computations and standard integral formulas.

More specifically, we aim at showing that

Indeed, once (A.2) is established, we deduce from it, (1.13) and (2.1) that

and this would complete the proof of (A.1).

Hence, we focus now on proving (A.2). To this end, we use spherical coordinates on $\partial B_1$, with $\theta$, $\theta_1$, $\dots$, $\theta_{n-3}\in[0, \pi]$ and $\theta_{n-2}\in[0, 2\pi)$, see e.g., Eq (A.23) in ^[3] or pages 60-61 in ^[14], which correspond to $\omega = \omega(\theta)$ of the form

and produce a surface element of the form

Also, up to a rotation, to prove (A.2) we can assume that $x = (0, \dots, 0, |x|)$. In this way, we find that

whence it follows that

Thus, we use the notation $\tau: = \frac{\rho}{|x|}\in(1, +\infty)$ and, by Proposition A.9 in ^[3], we deduce that

This and Proposition A.10 in ^[3] yield that

The proof of (A.2) is thereby complete.

A.2.   Proof of (3.8)

We recall (1.13) and we employ the change of variable $e: = \omega/|\omega|$ to see that

It also follows from (2.6) that, for a.e. $y\in \mathbb{R}^n\setminus B_1$,

Now we take $R_0\in(1, R)$ and we deduce from (A.3) that

Recalling the computation in (3.16), for $k$ large enough we have that

Consequently, using this, (A.4) and the dominated convergence theorem,

Also, we claim that there exists a subsequence $\lbrace f_{k_j}\rbrace_j$ such that

To show (A.7), we recall (3.15) and we observe that, for every $x\in B_1$ and $y\in\mathbb{R}^n\setminus B_{R_0}$,

Moreover, by (2.6) there exists a subsequence $\lbrace f_{k_j}\rbrace_j$ and a function $h\in L_s^1(\mathbb{R}^n\setminus B_{1})$ such that $\vert f_{k_j}(y)\vert \leqslant h(y)$ for a.e. $y\in \mathbb{R}^n\setminus B_1$ (see for instance Theorem 4.9 in ^[2]). Therefore, using this information into (A.8), we have

for a.e. $y\in \mathbb{R}^n\setminus B_{R_0}$.

Now we recall the map $F_\pm$ defined in (3.21), which is $C^1$ and invertible, and therefore, by Theorem 2 in Section 3.3.3 of ^[10] and (3.22), we get that

Accordingly, we deduce that

This, the bound in (A.9), the pointwise convergence in (A.4) and the dominated convergence theorem lead to (A.7), as desired.

Hence, putting together (A.5)–(A.7), we obtain that

for each $x\in B_1$, which completes the proof of (3.8).

A.3.   Proof of (3.9)

The proof of (3.9) is similar to the one of (3.8). We provide here the details for the convenience of the reader.

From (1.13) with $n = 2$ and the change of variable $e: = \omega/|\omega|$,

By (2.6), we have that, for a.e. $y\in \mathbb{R}^n\setminus B_1$,

Now we take $R_0\in(1, R)$ and we get from (A.10) that

Notice that, for $k$ large enough,

As a consequence,

Furthemore, recalling (2.6) (see also Theorem 4.9 in ^[2]) we deduce the existence of a subsequence $\lbrace f_{k_j}\rbrace_j$ and of a function $h\in L_s^1(\mathbb{R}^n\setminus B_{1})$ such that

for a.e. $y\in \mathbb{R}^n\setminus B_{R_0}$.

Furthermore, we claim that

Indeed, the function

is $C^1$ and invertible. Moreover, since

we find that

for some positive constant $\tilde{C} > 0$.

From this and Theorem 2 in Section 3.3.3 of ^[10] we have that

which establishes (A.15).

From (A.11), (A.14) and (A.15) and the Dominated Convergence Theorem, we deduce that

Gathering together this, (A.12) and (A.13), we conclude that

This finishes the proof of (3.9).