1.
Introduction
Mosquito-borne infectious diseases are spread by mosquitoes and are prevalent worldwide, including malaria, dengue fever, filariasis, yellow fever, and other highly harmful infectious diseases. Research shows that there are more than 80 such diseases, many of which have caused significant losses to human lives and health in different countries and regions. In recent years, the risk of mosquito-borne infectious disease outbreaks has increased due to climate change, as well as the rise of commercial trade and personnel exchanges.
Due to the lack of an effective vaccine for mosquito-borne diseases, controlling the population of mosquitoes has become crucial in curbing the spread of such diseases. Traditional physicochemical control methods have significant disadvantages such as negative environmental effects and drug resistance. In recent years, new biological control approaches such as insect sterility technology (SIT) and its derivatives have been extensively studied and practiced. These approaches involve the release of sterile or Wolbachia-infected mosquitoes into the wild to disrupt or suppress the development of the wild mosquito population. Certain studies specifically explored the use of sterile insect release for targeted population control [1,2,3]. Other research considered how the endosymbiotic bacterium Wolbachia can induce resistance to the dengue virus in Aedes aegypti [4], and there has been research into the control of particular mosquito-borne diseases [5]. Furthermore, in [6], a combination of incompatible and sterile insect techniques has been implemented to eradicate wild mosquitoes. During the exploration of these technologies, detailed analyses have been conducted on specific population characteristics such as population diffusion, periodic changes, the Allee effect, and others [7,8,9,10,11]. To ensure successful suppression of mosquito populations, continuous release over a long period is often necessary. Researchers have studied release strategies for different situations through various methods. For instance, uninterrupted continuous release strategies, based on ODE or DDE models, have undergone extensive examination [12,13,14,15]. More recently, impulsive release strategies, which better align with practical operations, have received considerable attention. Switched systems have been widely employed in simulating these release strategies [16,17,18,19,20]. In addition, many researchers have shown a preference for impulsive differential dynamical systems [21,22,23,24]. Some have integrated the duration of sexual activity of sterile mosquitoes with impulsive release behavior to investigate release strategies that effectively suppress mosquito populations [25,26,27].
Typically, male sterile mosquitoes are released into the wild to mate with female wild mosquitoes, rendering them unable to lay or hatch eggs. In [18,26,27,28], the authors proposed a mathematical model that considers the sexual lifespan of sterile mosquitoes, including only those within their sexually active period. Due to the short sexual lifespan of sterile mosquitoes, natural deaths during the sexual activity period are often neglected. Instead of using a single equation to describe the change in the number of sterile mosquitoes, the number of sterile mosquitoes is used as a control function [15,18,26,27,28,29]. Based on these ideas, the authors provided a nearly complete characterization of the release strategy using the following model.
where ω(t) and g(t) represent the number of wild mosquitoes and the number of sterile male mosquitoes in their sexually active period. The birth rate per wild mosquito, denoted by a, follows logistic growth. Additionally, ξ and μ represent the density-dependent and independent death rates, respectively.
We know that mosquito growth includes three aquatic stages, namely egg, larva, and pupa, as well as an adult stage. Intraspecific competition significantly affects the aquatic stages, while the adult stage is rarely affected. Building on model (1.1), the authors in [30] proposed and studied a stage-structured model for suppressing mosquito populations as follows:
where J and A represent the number of wild mosquitoes in the aquatic and adult stages, respectively, while g represents the number of sterile males. The birth rate of adults is denoted by β. μi and ξ represent the natural mortality and intraspecific competition coefficient, respectively, where i=0,1. The parameter α represents the emergence rate from larvae to adults.
As mentioned earlier, in order to suppress the wild mosquito population, male sterile mosquitoes are released in most scenarios. In addition, the role of female and male mosquitoes in interacting with sterile mosquitoes is different. Motivated by the previous research works, this paper aims to further investigate and develop a wild mosquito suppression model with both stage and sex structure.
The paper is organized as follows: In Section 2, we develop a mosquito population suppression model with both stage and sex structure. The model is a time-switched system with the number of sexually active sterile males serving as a control function. In Section 3, we first define two important thresholds for the release amount and then determine the conditions for the stability of the wild mosquito extinction equilibrium and the existence of positive periodic solutions. In Section 4, we provide numerical results to validate our theoretical findings. Finally, we conclude with a brief summary in Section 5.
2.
Model formulation
L. Almeida et al. in [31] describes the dynamics of the mosquito population as follows
where E(t), L(t), P(t), F(t) and M(t) stands for the numbers of eggs, larvae, pupa, adult females and adult males at time t, respectively. The parameters βE, K, and τE represent the oviposition rate, environmental capacities and hatching rate for eggs, respectively. Pupas will develop into males or females, and ν∈(0,1) reflects the corresponding proportion. τL, τP are transition rates, c is the intraspecific competition of larvae, and δE, δL, δP, δF and δM are the corresponding death rates.
To simplify the mosquito population model, we make the following assumptions, similar to those in [31]: (i) the dynamics of larvae and pupae are fast, and intraspecific competition at the larvae stage is negligible (i.e.,c≪1); (ii) the probability of a pupa developing into a female is the same as that of a male (ν=12); (iii) male and female mosquitoes have the same death rate (δF=δM).
With these assumptions and the third and fourth functions of system (2.1), we obtain the equilibrium equations for larvae and pupae, which are:
After substituting Eq (2.2) into system (2.1) and introducing a release function u(⋅) for sterile male mosquitoes, we can derive the following interactive dynamical system describing the population dynamics of two kinds of mosquitoes
where Ms(t) is the number of sterile males in the field, γ measures the mating competitiveness of sterile males, and βF=τPτLτE(τP+δP)(τL+δL).
In this paper, we also only consider sexually active sterile mosquitoes in the dynamics. Let Ms(t) be the number of sexually active sterile males at time t and ignore their natural death as in previous works [15,18,26,27,28,29]. By taking Ms(t) as a control function, we convert the system (2.1) into
Let T be the waiting time between two consecutive release, and sterile mosquitoes are released with an amount of m at time t=kT, k=0,1,2,⋯. We use ˉT to represent the sexual lifespan of sterile mosquitoes, ignoring their natural death as in [15,18,26,27,28,29]. There are three possible cases for T and ˉT: T=ˉT, T>ˉT, and T<ˉT. Previous works [30] studied the effect of releasing sterile mosquitoes on wild mosquito populations in the first two cases.
In this work, we mainly study the last case: T<ˉT. In fact, experimental studies have indicated that the lifespan of sterile mosquitoes can be as long as 51 days [32] such as the Wolbachia-infected mosquitoes, and their sexual lifespan can reach 14 days [33]. Nonetheless, the release of sterile mosquitoes is relatively frequent, such as in the experimental field of Shazai Island in Guangzhou, China, where they were released three times a week [32]. Hence, it is meaningful to consider the situation where the sexual lifespan of sterile mosquitoes exceeds the release period, that is T<ˉT.
Similar to the discussion in [17], when T<ˉT, there must exist a unique positive integer p and a non-negative number q that satisfy
where q∈[0,T) and p=[ˉT/T], which is the integer closest to ˉT/T and not greater than ˉT/T. Since pT≤ˉT, we can obtain the first p releases
where k=0,1,2,⋯,p−1. From the (p+1)th release, it is easy to get
where k=p,p+1,⋯. If q=0, then Ms(t)=pm, and the characteristics of the system are completely consistent with the simple system of constant value continuous release. In the present work, we assume that 0<q<T. As we are studying the asymptotic behavior of the system, for the sake of analysis convenience and without loss of generality, we can further assume that for k=0,1,2,⋯,
Based on the above assumption, the model (2.4) is transformed as follows:
where k=0,1,2,⋯.
Population dynamics of wild mosquitoes with sterile mosquito release then depend on the continuous switching between systems (2.7) and (2.8). In this paper, based on the switched systems (2.7) and (2.8), we mainly investigate the extinction and periodic change of wild mosquito population.
3.
Dynamics of the switched system
3.1. preliminaries
First, we consider the dynamic behavior of the wild mosquito population without the release of sterile mosquitoes. Let Ms(t)≡0, then the system (2.4) or (2.7) and (2.8) can be rewritten as follows:
Let Ω={(E,F)|0≤E≤K,0≤F}. Obviously, Ω is a positive invariant set of systems (3.1), (2.7) and (2.8), and every solution of systems (3.1), (2.7) and (2.8) with nonnegative initial values is positive and bounded. Therefore, in this work, we will investigate the dynamics of these systems in Ω. Additionally, it is easy to verify that system (3.1) is a monotonic system on Ω, so there are no closed orbits. By defining the basic offspring number of the wild mosquito as
we list the following conclusions about the system (3.1):
Lemma 1. (i) If R0≤1, then the extinction equilibrium A0(0,0) is the unique equilibrium of system (3.1) that is globally asymptotically stable in Ω.
(ii) If R0>1, then A0(0,0) is unstable, and the unique positive equilibrium A∗(E∗,F∗) of system (3.1) is globally asymptotically stable in Ω, where
According to the necessity of releasing sterile mosquitoes, we will only consider the case R0>1 in this paper.
For the convenience of illustration, the following system is introduced to uniformly express the two subsystems (2.7) and (2.8)
where ˜p=p+1 or p.
To compute the jacobian matrix of the system (3.2), we obtain
It has non-negative extra-diagonal coefficients on Ω={(E,F)|0≤E≤K,0≤F}, indicating that the system (3.2) is monotone on Ω.
Clearly, the wild mosquito extinction equilibrium A0(0,0) is an equilibrium of both systems (2.7) and (2.8). By using the general form (3.2) of systems (2.7) and (2.8), we can now calculate the positive equilibria by finding the positive roots of the following equations,
which can be further equivalently transformed into
It is easy to know that the discriminant of the above equation is
then we define the following release threshold for the system (3.2)
Lemma 2. Let R0>1. The trivial equilibrium A0 of system (3.2) is always locally asymptotically stable. Moreover,
(i) There exists m∗(˜p)>0 such that the system (3.2) admits no positive equilibrium if m>m∗(˜p), one positive equilibrium if m=m∗(˜p), and two positive equilibria A⋅j(E⋅j(˜p),F⋅j(˜p)),j=1,2 if 0<m<m∗(˜p), where 5
(ii) If m>m∗(˜p), then the trivial equilibrium A0 is globally asymptotically stable in Ω. If 0<m<m∗(˜p), then A⋅1(E⋅1(˜p),F⋅1(˜p)) is unstable, while A⋅2(E⋅2(˜p),F⋅2(˜p)) is locally asymptotically stable. If m=m∗(˜p), then the unique positive equilibrium is a saddle node.
Proof. To calculate the Jacobian matrix of the system (3.2) at the trivial equilibrium A0(0,0), we have
It has two negative eigenvalues, therefore the trivial equilibrium A0 is always locally asymptotically stable.
(i) By calculating the discriminant Δ in Eq (3.5), we can see that Δ=0 when m=m∗(˜p), Δ<0 when m>m∗(˜p), and Δ>0 when 0<m<m∗(˜p). Moreover, the root of the quadratic equation (3.4) must be positive roots if it exists. These observations lead to the conclusions in (i).
(ii) Since the system (3.2) is monotone on Ω, it has no closed orbits. If m>m∗(˜p), then the extinction equilibrium A0 is the unique equilibrium which is stable, so it is globally asymptotically stable in Ω.
If 0<m<m∗(˜p), we calculate Jacobian matrix of the system (3.2) at A⋅j,j=1,2 by using jacobian matrix (3.3) and get
We can easily get trJA⋅j=−νβEβFF⋅jδF(m1+F⋅j)−δF<0 and
where Φ(F)=−G(F)γ˜pm+F.
Since −Φ′(F)=−G(F)(γ˜pm+F)2+G′(F)(γ˜pm+F), G(F⋅1)=G(F⋅2)=0 and G′(F⋅1)>0,G(F⋅2)<0, we can obtain |J(A⋅1)|<0 and |J(A⋅2)|>0. Therefore, A⋅1(E⋅1,F⋅1) is an unstable saddle, while A⋅2(E⋅2,F⋅2) is locally asymptotically stable.
If the release amount m increases to m=m∗(˜p), then the two positive equilibria A⋅1 and A⋅2 merge into a single semi-stable saddle node. The proof is completed.
Remark 1. According to Lemma 2, the constant continuous release system (3.2) has a threshold m(˜p) for the release amount m. When the release amount m>m(˜p), the wild mosquito population in the field will be successfully suppressed.
Referring to the two subsystems (2.7) and (2.8), we denote their corresponding release thresholds as m∗1 and m∗2, respectively, given by:
When there are two positive equilibria, the positive equilibria corresponding to systems (2.7) and (2.8) are denoted by A1j(E1j,F1j) and A2j(E2j,F2j),j=1,2, respectively, where
Lemma 2 provides insights into the behavior of systems (2.7) and (2.8). We can now state the following result:
Lemma 3. Let R0>1. The trivial equilibrium A0 of system (2.7) (or (2.8)) is always locally asymptotically stable. In addition,
(i) If 0<m<m∗1, system (2.7) (or (2.8)) has two positive equilibria A11(E11,F11) and A12(E12,F12) (or A21(E21,F21) and A22(E22,F22)), where A11(E11,F11) (or A21(E21,F21)) is unstable, and A12(E12,F12) (or A22(E22,F22)) is locally asymptotically stable.
(ii) If m∗1<m<m∗2, system (2.8) has two positive equilibria A21(E21,F21) and A22(E22,F22), where A21(E21,F21) is unstable, and A22(E22,F22) is locally asymptotically stable. However, system (2.7) has no positive equilibrium.
(iii) If m>m∗2, both systems (2.7) and (2.8) have no positive equilibrium, and the unique equilibrium A0(0,0) is globally asymptotically stable in Ω.
3.2. Periodic and impulsive releases
When the release period of sterile mosquitoes is shorter than their sexual lifespan, that is, T<ˉT, the number of sterile mosquitoes that are sexual active will continually switch between two levels, as shown in function (2.6). The population dynamics of wild ones will then depend on the switched systems (2.7) and (2.8).
With the appearance of continual switching, the extinction equilibrium A0(0,0) becomes the unique equilibrium of the systems (2.7) and (2.8). We will first discuss its stability.
Since R0=νβEβFδF(τE+δE)>1, βF=τPτLτE(τP+δP)(τL+δL) and ν∈(0,1), we get νβEβF>δF(τE+δE) and βF<τE, and then τE+δE>νβF. Let
then for any given 0<σ<1, denote
Theorem 1. Assuming R0>1 and ˉT=pT+q, for any solution (E(t),F(t)) of systems (2.7) and (2.8) with 0<E(0)+F(0)<ℓ1, the inequality
holds, where r=(1−σ)α>0. Thus, the extinction equilibrium A0(0,0) of systems (2.7) and (2.8) is exponentially asymptotically stable.
Proof. Suppose (E(t),F(t)) is a solution of the systems (2.7) and (2.8) in Ω with 0<E(0)+F(0)<ℓ1 for fixed σ∈(0,1). Let P(t):=E(t)+F(t) for t≥0. According to the first subsystem (2.7), we have for t∈[kT,kT+q], k=0,1,2,⋯,
We claim that if P(kT)<ℓ1<ℓ, then P(t)<ℓ1 for all t∈[kT,kT+q], k=0,1,2,⋯. Let's prove this by contradiction. Suppose it does not holds, then there must exist ˉt∈(kT,kT+q] such that P(t)<ℓ1<ℓ for t∈[kT,ˉt) and P(ˉt)=ℓ1, then P′(ˉt)≥0. However, from inequality (Eq 3.10), we deduce
which leads a contradiction. Therefore, we have P(t)<ℓ1 for all t∈[kT,kT+q] and P′(t)≤(βEγ(p+1)mℓ1−α)P(t)=−(1−pp+1σ)αP(t)<0. Then we can further deduce
Similarly, for the second subsystem (2.8), we can obtain
Then if P(kT+q)<ℓ1, then P(t)<ℓ1 for all t∈[kT+q,(k+1)T], k=0,1,2,⋯. In addition,
Combining with inequalities (Eq 3.11) and (Eq 3.12), we know that
where ξ:=e−(1−σ)αT<1.
Thus,
If t∈[kT,kT+q], then from inequality (Eq 3.11) we get
If t∈[kT+ˉT,(k+1)T], then from inequality (Eq 3.12) we get
By combining inequalities (Eq 3.13) and (Eq 3.14), we can see that the inequality (Eq 3.9) holds and A0(0,0) is exponentially asymptotically stable. The proof is completed.
In Theorem 1, we show the exponential asymptotic stability of A0(0,0). However, this stability is only a local feature. Due to the periodic release of sterile mosquitoes, we are concerned about the possibility of a periodic change in the wild mosquito population, specifically, whether the systems (2.7) and (2.8) has a positive periodic solution. In the following discussion, we focus on the existence of positive periodic solutions.
Based on Lemma 3, when R0>1, T<ˉT, and 0<m<m∗1, both subsystems (2.7) and (2.8) have a pair of positive equilibria, namely A11(E11,F11), A12(E12,F12) and A21(E21,F21), A22(E22,F22). It is easy to verify that F22>F12>F11>F21>0 and K>E22>E12>E11>E21>0. Let Ω0:=[E12,E22]×[F12,F22] be a rectangle in the EF-phase plane. Then we can get the following result.
Theorem 2. If R0>1 and 0<m<m∗1, then the systems (2.7) and (2.8) has a T-periodic solution in Ω0. Suppose (E(t),F(t)) is a solution of the systems (2.7) and (2.8) with E(t0)≥E11 and F(t0)≥F11 for some t0≥0, then E(t)≥E11 and F(t)≥F11 for all t>t0, and dist((E(t),F(t)),Ω0)→0 as t→∞. Moreover, if such a solution is T-periodic, then (E(t),F(t))∈Ω0 for all t≥0.
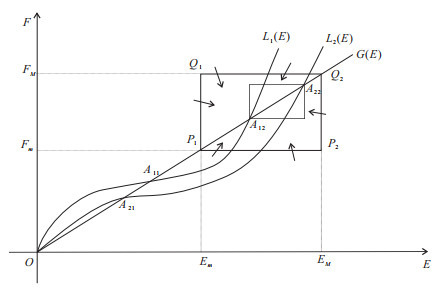
Proof. For the conditions R0>1, T<ˉT, and 0<m<m∗1, we obtain the existence of Aij, i,j=1,2. Let η(E):=KE(τE+τE)K−E, E∈[0,K]. The vertical isoclines E′(t)=0 of systems (2.7) and (2.8) in the first quadrant of the EF-phase plane are given by
respectively (see Figure 1). Since L1(E)>L2(E), the curve of F=L1(E) is strictly above the curve of F=L2(E) for E>0. Additionally, F=G(E):=νβEEδF is the shared horizontal isocline of systems (2.7) and (2.8).
Select any two positive numbers Em and EM satisfying E11<Em<E12<E22<EM<K, then Fm=G(Em) and FM=G(EM). Due to the strict monotonic increase property with respect to E of G(E), it follows that Fm<FM. Let Ω1 be the closed rectangle with the vertices P1,P2,Q1 and Q2, where P1=(Em,Fm), P2=(EM,Fm), Q1=(Em,FM), and Q2=(EM,FM).
Clearly, F11<Fm<F12<F22<FM and Ω0⊂Ω1. Since G(E) is strictly monotone increasing with respect to E, the segment ¯P1P2 lies strictly below the isocline F=G(E) except for the point P1, while the segment ¯Q1Q2 lies strictly above the isocline F=G(E) except for the point Q2. According to the second equation of systems (2.7) and (2.8), we have
Thus, the vector fields of (2.7) and (2.8) point into the closed rectangle Ω1 on ¯P1P2 and ¯Q1Q2.
In addition, since L1(E) and L2(E) are both strictly monotone increasing with respect to E on [0,K], we can see that the entire segment ¯P1Q1 lies strictly above the isocline F=L1(E), and the entire segment ¯P2Q2 lies strictly below the isocline F=L2(E). From system (2.7), we obtain
where L−1(E)=12βE[−η(E)+√η(E)2+4γ(p+1)mβEη(E)]>0.
From the system (2.8), we can obtain
where L−2(E)=12βE[−η(E)+√η(E)2+4γpmβEη(E)]>0.
Clearly, on the segment ¯P1Q1, we have E≡Em, so F>L1(Em) and F>L2(Em). As a result, dEdt>0 holds for both systems (2.7) and (2.8) on ¯P1Q1. Similarly, on the segment ¯P2Q2, we have F<L1(EM) and F<L2(EM), leading to dEdt<0 for both systems (2.7) and (2.8) on this segment. Therefore, the vector fields of systems (2.7) and (2.8) point towards the inside of Ω1 on both ¯P1Q1 and ¯P2Q2.
In summary, Ω1 represents a positive invariant set of the switched systems (2.7) and (2.8), and any point A∈Ω1 will have the trajectory of the systems (2.7) and (2.8) starting from it stay within Ω1.
We define a map Φ:Ω1→Ω1 as given in [30]:
Here, ϕt(A) and ψt(A) are the solutions of systems (2.7) and (2.8) starting from the point A, respectively. Since Φ is continuous and maps Ω1 to Ω1, by Brouwer's fixed point theorem, we know that Φ has a fixed point ˉA∈Ω1. Then, the solution of systems (2.7) and (2.8) passing through the point ˉA is a continuous T-periodic solution whose trajectory lies entirely in Ω1.
It is worth noting the arbitrariness of Em and EM. If Em→E12 and EM→E22, then Ω1→Ω0, and the continuous T-periodic solution of systems (2.7) and (2.8) in Ω1 lies in Ω0. If Em→E11 and EM→∞, then Ω1→[E11,∞)×[F11,∞). Since Ω1 is an attraction domain, [E11,∞)×[F11,∞) is also a positively invariant set of systems (2.7) and (2.8). Then, for any solution of systems (2.7) and (2.8) with E(t0)≥E11 and F(t0)≥F11, it follows that E(t)≥E11 and F(t)≥F11 for all t>t0. Moreover, dist((E(t),F(t)),Ω0)→0 as t→∞. Furthermore, if this solution is periodic, then (E(t),F(t))∈Ω0. Thus, the proof is completed.
When we increase the release amount m to exceed the smaller threshold m∗1, that is, m∗1≤m<m∗2, we obtain the following result:
Theorem 3. Suppose R0>1 and m∗1<m<m∗2. Then there exist positive constants T0>0 and δ0>0 such that the systems (2.7) and (2.8) has a continuous and positive T-periodic solution when
Proof. According to Lemma 3, when R0>1, T<ˉT and m∗1<m<m∗2, the subsystem (2.8) has two positive equilibria A21 and A22, while (2.7) has only the extinction equilibrium.
Similar to Theorem 2, we first select two arbitrary positive numbers Em and EM such that 0<Em<E22<EM and G(EM)<L1(Em) (G(E) and Li(E),i=1,2 are defined in the proof of Theorem 2). Let Fm=G(Em) and FM=G(EM). Then we construct a closed rectangle Ω1 with vertices at P1=(Em,Fm), P2=(EM,Fm), Q1=(Em,FM), and Q2=(EM,FM). In addition, let P3 be the intersection point of the segment P1P2 and the isocline F=L2(E), and for any E0∈(Em,E3), denote points P0:=(E0,Fm) and Q0:=(E0,FM) (see Figure 2). Then Ω1 can be regarded as a rectangle composed of two parts, namely, Ω1=Ω11⋃Ω21, where Ω11 is the rectangle with P1, P0, Q1, and Q0 as its vertices, and Ω21 is the rectangle with P0, P2, Q0, and Q2 as its vertices.
We define ω1 and ω2 as follows:
where G1(E,F) and G2(E,F) are the right-hand side functions of the first equations of systems (2.7) and (2.8), respectively.
We assume that
where T0:=E0−Em−ω1, δ0:=E0−Emω2.
In the following, we will prove that the Poincare map Φ defined in Theorem 2 is continuous and maps Ω21 to itself. Then, similarly to the proof of Theorem 2, we can deduce that the systems (2.7) and (2.8) has a continuous and positive T-periodic solution in Ω21.
The vector fields of system (2.7) point towards the interior of Ω1 along the segments ¯P1P2, ¯Q1Q2, and ¯P2Q2. Therefore, for any solution of system (2.7) with initial value (E(0),F(0))∈Ω21, it can only cross the segment ¯P0Q0 if it leaves Ω1. We will now show that this is impossible by contradiction.
Suppose there exists a time t0∈[0,q) such that (E(t0),F(t0))∈¯P0Q0 and (E(t),F(t))∈Ω21 for t∈[0,t0). Then, we have E′(t0)<0, which means that the solution immediately enters Ω11 after t0. When the solution leaves Ω11, it can only go through the segment ¯P1Q1.
Now, assume there exists a time ˜t∈(t0,q] such that (E(˜t),F(˜t))∈¯P1Q1 and (E(t),F(t))∈Ω11 for t∈[t0,˜t). Then, we have E′(t)=G1(E(t),F(t))≥ω1 for t∈[t0,˜t], and E(˜t)=Em≥E(t0)+ω1(˜t−t0)≥E0+ω1q. Since ω1<0 and q<T0, we have Em<E0+ω1T0, which is a contradiction. Therefore, any solution (E(t),F(t)) of system (2.7) with (E(0),F(0))∈Ω21 will remain in Ω1 for t∈[0,q].
Next, we show that for any solution of system (2.8) with (E(q),F(q))∈Ω1, the solution will stay in Ω1 for t∈[q,T] with (E(T),F(T))∈Ω21. Noting that the vector fields of system (2.8) point to the inside of both Ω1 and Ω21, we see that both the rectangles Ω1 and Ω21 are positive invariant sets of system (2.8). Therefore, (E(q),F(q))∈Ω1 implies (E(t),F(t))∈Ω1 for all t∈[q,T]. Furthermore, we can claim that (E(T),F(T))∈Ω21. If this does not hold, then (E(t),F(t))∈Ω11 for all t∈[q,T] due to the properties of the invariant set Ω21. This implies that E′(t)≥ω2 for t∈[q,T] and E(T)≥E(q)+ω2(T−q). We further deduce that E0−Em≥E(T)−E(q)≥ω2(T−q), which contradicts the inequality (3.18).
In summary, we have shown that for any solution (E(t),F(t)) of systems (2.7) and (2.8) starting from the initial point (E(0),F(0))∈Ω21, the solution will stay in Ω1 for t∈[0,T] and (E(T),F(T))∈Ω21. Thus, the Poincare map Φ:Ω21→Ω21 is well-defined and has at least one fixed point A∗ in Ω21. Then the solution of systems (2.7) and (2.8) through the point A∗ is a continuous and positive T-periodic solution. The proof is completed.
Remark 2. If m=m∗1, then the two equilibria A11(E11,F11) and A12(E12,F12) of (2.7) coincide into one, denoted by A∗1c(E∗1c,F∗1c). By slightly modifying the proof of Theorem 3.3 and selecting values of Em and EM such that E∗1c<Em<E22<EM and G(EM)<L1(Em), we can similarly prove that the systems (2.7) and (2.8) has a positive T-periodic solution.
Next, we consider the case where the release amount m is further increased until m≥m∗2, and we present the following result.
Theorem 4. If R0>1 and m>m∗2, then the unique extinction equilibrium A0 of systems (2.7) and (2.8) is globally asymptotically stable.
Proof. According to Theorem 1, if R0>1 and m>m∗2, the extinction equilibrium A0(0,0) of systems (2.7) and (2.8) is locally asymptotically stable. We will now prove that it is also globally attractive.
To this end, we construct the following system:
Similar to the discussion about system (3.1), we can show that system (3.19) is monotone on Ω. Furthermore, we have
Thus, system (3.19) can be regarded as a comparison system for systems (2.7) and (2.8). With the same initial values ˜E(0)=E(0) and ˜F(0)=F(0), we obtain
From Lemma 2, we know that if R0>1 and m>m∗2, the trivial equilibrium (0,0) of system (3.19) is globally asymptotically stable. That is, for any non-negative initial value (˜E(0),˜F(0)), the solution of system (3.19) from it satisfies
By the comparison theorem, we can easily see that for any non-negative initial value (E(0),F(0)), the solution of systems (2.7) and (2.8) from it satisfies
Thus, the extinction equilibrium A0(0,0) of systems (2.7) and (2.8) is also globally attractive. The proof is completed.
Remark 3. If m=m∗2, then the extinction equilibrium A0(0,0) of system (2.7) is globally asymptotically stable, meaning that every solution of system (2.7) will tend to the extinction equilibrium A0(0,0). Moreover, the two equilibria A21(E21,F21) and A22(E22,F22) of system (2.8) coincide into one, denoted by A∗2c(E∗2c,F∗2c). According to Lemma 2, this case presents a possible semi-stability, where the basin of attraction of A0(0,0) contains the interval [0,A∗2c), denoted as {(E,F)∈R+2:0≤E<E∗2c,0≤F<F∗2c}, and the basin of attraction of A∗2c(E∗2c,F∗2c) contains the interval (A∗2c,∞), denoted as {(E,F)∈R+2:E>E∗2c,F>F∗2c}. Due to the continual switching between two subsystems (2.7) and (2.8), any solution of systems (2.7) and (2.8) must enter [0,A∗2c) after some time and will eventually tend to the extinction equilibrium A0(0,0). Therefore, the extinction equilibrium A0(0,0) of systems (2.7) and (2.8) is globally asymptotically stable.
Remark 4. According to Theorems 1–4 and Remark 3, the periodic and impulsive release systems (2.7) and (2.8) has a threshold m∗2 for the release amount m, and only when the release amount m≥m∗2, can the wild mosquito population in the field be successfully suppressed.
4.
Numerical simulation
This section aims to illustrate the theoretical results developed in the previous section through several numerical examples. We use the values of most model parameters from [31,34], which are summarized in Table 1.
Another important parameter to be determined is the carrying capacity, denoted by K. We consider an island with an area of 74 hectares, as in [31,34], with an estimated male population of about 69 males per hectare. If there are no sterile mosquitoes in the field and the wild population reaches a stable state, the number of adult wild males is assumed to be M∗=F∗=69×74=5106. Then, the number of eggs at the stable state is estimated as E∗=δFF∗νβF=40848, and the carrying capacity can be calculated as
For the sexual lifespan of sterile mosquitoes, we take the value from [17], and set ˉT=14. Through direct calculation, we can get
When no sterile ones are released, the unique positive equilibrium A∗(E∗,F∗) is globally and uniformly asymptotically stable (see Figure 3(a)), which is consistent with the result in Lemma 1.
For the constant release system (3.2), we can take ˜p=4 without loss of generality, yielding m∗(4)≈4667. When 0<m<m∗(4), the system (3.2) displays bistability, as shown in Figure 3(b), where the extinction equilibrium A0 and a positive equilibrium are locally stable. Increasing the release amount to m=m∗(4) results in a unique positive equilibrium that is semi-stable (see Figure 3(c)). However, if m>m∗(4), then the unique equilibrium A0 becomes globally stable (see Figure 3(d)).
For the switched systems (2.7) and (2.8), textcolor{red}{we choose the release period T=3 }and set ˉT=pT+q with p=4 and q=2. After a simple calculation, we obtain m∗1=m∗(p+1)≈3735 and m∗2=m∗(p)≈4667.
Firstly, let m=3250<m∗1 and we note that the systems (2.7) and (2.8) displays bistability. In addition to the locally stable extinction equilibrium, there also exists a stable positive periodic solution (see Figure 4 (a)). This is consistent with the conclusions of Theorems 1 and 2. As shown in Figures 4(b, c), when the release amount increases to m∗1<m<m∗2, the systems (2.7) and (2.8) continues to exhibit bistability, which is consistent with the conclusions of Theorems 1 and 3. Furthermore, we can see that the attraction region of the extinction equilibrium increases with the release amount m until the release amount is not less than m∗2. If m<m∗2, the extinction equilibrium becomes globally attractive (see Figure 4(d)).
5.
Conclusions
In this work, we constructed and analyzed a two-dimensional switched system of the wild mosquito population with stage structure and periodic releases of sterile mosquitoes. To consider the role of sterile mosquitoes in the interaction between the two mosquito populations, we referred to some previous studies and only included sexually active sterile males in the system. Existing research data suggests that the sexual lifespan ˉT of sterile mosquitoes can be shorter or longer than their release period T, and in [30], the authors studied the case ˉT≤T. Therefore, we focused on investigating the case ˉT>T based on the switched systems (2.7) and (2.8).
We first investigated the non-release system (3.1) and the general form (3.2) of the two subsystems. We gave conditions for the existence and stability of their equilibria (Lemmas 2 and 3), which prepared for the later study of the switched system. Two important release amount thresholds (m∗1 and m∗2) were determined. Then, we proved that the extinction equilibrium A0(0,0) of systems (2.7) and (2.8) must be exponentially asymptotically stable (Theorem 1). This differs from the conclusion in [30] when ˉT<T since, in the latter case, the extinction equilibrium may be unstable. As systems (2.7) and (2.8) has no positive equilibrium, we further discussed the existence of positive periodic solutions. In Theorems 2 and 3, through the analysis of vector fields and using the fixed-point theorem, we show that systems (2.7) and (2.8) has a positive periodic solution when 0<m<m∗1 and m∗1≤m<m∗2. Further increasing the release of sterile mosquitoes, and when m≥m∗2, we confirmed that the extinction equilibrium A0(0,0) of systems (2.7) and (2.8) becomes globally asymptotically stable. This is a previously unconfirmed dynamic phenomenon when studying the case of ˉT<T.
Regarding the periodic switched systems (2.7) and (2.8), due to the complexity of its model construction, we only prove the existence of positive periodic solutions using the fixed-point theorem. However, neither the number nor the stability of the positive periodic solutions are determined. In future research, we will try more methods to solve these problems, such as constructing Lyapunov functions to study the stability of periodic solutions [35,36,37]. In addition, we mainly focus on the theoretical study and the validation of the related results in this work. For the numerical analysis, we used data from a mosquito population on a 74-hectare island. Due to the relatively small number of wild mosquitoes on the island, the amplitude of the periodic fluctuation in the mosquito population is indeed small. In future research, we will collect and organize relevant data from other scenarios with large mosquito populations, and apply our research results to the development of management strategies for wild mosquito populations.
Acknowledgements
This work is supported by the National Natural Science Foundation of China (11901502), Program for Science & Technology Innovation Talents in Universities of Henan Province (21HASTIT026), Program for Innovative Research Team (in Science and Technology) in University of Henan Province (21IRTSTHN014) and Graduate Research Innovation Fundation of XYNU(2022KYJJ055).
Conflict of interest
The authors declare no conflicts of interest in this paper.









 DownLoad:
DownLoad: