Novel closed-loop controllers for fractional nonlinear quadratic systems

1.   Introduction

The dynamic behavior of a physical system is described by differential equations. Most physical systems are nonlinear ^[1]. Many nonlinear optimal control problems do not have solutions that can be easily computed ^[2]. Due to many advantages, engineers prefer the closed-loop controllers for the optimal control problems ^[3]. We know that it may not be possible to find analytically the optimal control law for a real-world nonlinear optimal control problem. There are some numerical methods like the methods of ^[4,5] to find the closed-loop controllers of integer order systems. In ^[4], a polynomials-based method by integrating from $0$ to $t$ for finding the feedback gain of the linear integer control system with the quadratic performance index was presented in which the user must solve a set of equations. But in this work, we propose a method by which the resulting equation is a static equation and the terminal condition is imposed directly to the equation. In ^[5], another method for finding a nonlinear closed-loop control of linear/nonlinear integer control systems was presented in which there are some necessary modifications for applying the method and the controller is nonlinear. ^[6] extended the Riccati theory, unifying continuous-time linear-quadratic optimal permanent and sampled-data control problems in finite and infinite time horizons.

Many of physical systems have been modeled by fractional differential equations ^[7,8]. Since the existence of the fractional order systems is shown in the literature, we need to study the fractional nonlinear optimal control systems. For a fractional plant, finding the optimal control law becomes more complicated than an integer plant, because of the fractional nature of its state equation. Also, analyzing such systems is complicated.

The stability analysis and the control of fractional-order systems have been widely investigated ^[9]. There are two different perspectives in designing the feedback controls of the fractional systems. In the first, we design the controller, then by adjusting some parameters we find the elements of the controller. There are useful procedures in designing the feedback control like, feedback controller based on linear matrix inequalities ^[10], event-triggered control ^[11], adaptive composite dynamic surface neural control ^[12] and so on. In the second perspective, we are dealing with fractional optimal control ^[13] in which the feedback controller must satisfy some conditions and equations. There are many texts in the fractional optimal control that have only researched open-loop solutions. Also, some of the illustrative examples of these texts are not optimal control problems.

The methods extended for the classic optimal control problems may not be applicable to the fractional optimal control problems. In ^[13], the study on the optimal control of the fractional systems was presented. ^[14] presented the control law for the fractional optimal control problem, in which the closed-loop controller is given in terms of the state, costate and the Mittag-Leffler function. ^[15] presented new nonlinear and linear fractional order controllers based on synergetic control theory in the Riemann–Liouville sense. ^[16] presented a novel solution to the state estimation problem for a class of nonlinear commensurate fractional-order systems. ^[17] presented a method for finding a suboptimal controller for a multi-fractional linear quadratic regulator system in the presence of known disturbance.

In this research, we present a nonlinear suboptimal feedback control of fractional nonlinear optimal control problems. Its main advantages are: its generality, which means it can be applied to a fractional system with different values of the fractional orders; it can be applied to the integer order nonlinear optimal control problems; for the integer order problems, it is linear; it can be used for large scale problems; moreover, it will be applicable in a nonlinear problem in which the terminal cost weighted matrix is not a zeros matrix. In this paper, we present the Riccati matrix for all illustrative examples, while this matrix and the Kalman gain were not presented in very few works in this research filed.

The framework of this study is as follows. First, we present some concepts to transform the nonlinear optimal control problem into a sequence of linear optimal control problems. Next, we introduce the optimality conditions of the problem under consideration and a formulation for the optimal control law. The fractional matrix differential Riccati equation of the original problem is formulated and a method for solving the equation is presented. By using the polynomial properties, we obtain the open-loop and closed-loop controllers. Finally, we redesign the linear optimal control law for fractional plants and we apply it to several problems.

2.   Fractional nonlinear quadratic optimal control

Consider the general fractional nonlinear optimal control problem as minimizing the real-valued performance index

subject to a nonlinear system

with the initial condition ${\bf x}(0) = {\bf x}_{0}$. Here, $t \in [0, t_{f}]$, $t_{f}$ as the final time is fixed, ${\bf T}, {\bf Q}(t) \in \mathbb{R}^{q \times q}$ are symmetric, positive semi-definite matrices, ${\bf R}(t)\in \mathbb{R}^{r \times r}$ is a symmetric, positive definite matrix, $^{C}_{0}D^{\alpha}_{t}$ is the left Caputo fractional derivative of order $\alpha$, $\alpha \in (0, 1]$, ${\bf x} \colon [0, t_f] \to \mathbb{R}^q$ and ${\bf u} \colon [0, t_f] \to \mathbb{R}^r$ are the state and control vectors, and ${\bf f} \in \mathbb{R}^{q}$ is a nonlinear (differentiable) Lipschitz vector function.

2.1.   Necessary conditions for fractional nonlinear optimal control problems

Lemma 2.1. The fractional nonlinear optimal control problem under assumptions that there are not any undecomposed terms and that the nonlinearity satisfies a local Lipschitz condition, can be replaced by a sequence of fractional linear time-varying systems, which converges to a solution as: for $i \ge 1$ minimize the performance index

subject to the linearized system equation

where "$[i]$" represents the iteration,

Proof. See ^[18]. □

Lemma 2.2. The fractional nonlinear optimal control system (2.2), under assumptions of Lemma 2.1, can be replaced by a sequence of fractional linear time-varying systems whose solutions converge to the solution of the original nonlinear system. Also, the solution is bounded.

Proof. The idea of proof is similar to that given in ^[13]. □

Theorem 2.1. By taking

and

the necessary optimality conditions for the fractional nonlinear optimal control problems, in which $\alpha \in (0.9, 1]$, are

and

where "$i^{*}$" is the number of the iteration (in each method) by which we have reached the final optimal solutions.

Proof. The proof is based on using Lemmas 2.1 and 2.2 in the necessary optimality conditions, such as (2.5)–(2.7) as derived for the fractional linear optimal control problems in ^[13]. □

Remark 2.1. Here, we have a necessary condition on $\alpha$; that is, $\alpha \in (0.9, 1]$, but, as we shall see later, by using the proposed controller we overcome this limitation and we can choose $\alpha \in (0, 1]$.

2.2.   Fractional matrix differential Riccati equation and optimal control law for a nonlinear system

Theorem 2.2. The closed-loop optimal control for the fractional nonlinear optimal control problem of minimizing the performance index (2.3) subject to the dynamical system (2.4) is obtained from

Also, the linearized fractional differential Riccati equation of the nonlinear system becomes as (2.9),

where $i^{*}$ is the same as that in Theorem 2.1.

Proof. From Lemma 2.1, by linearization of the original system as

when we find the final optimal solutions for which $i = i^{*}$, we take

Thus, we have the linear system

Now, from Theorem 2.1 and by setting

and differentiating (2.10), we can obtain (2.8) and (2.9). □

Theorem 2.3. The solution of the fractional Riccati equation for the linearized system is obtained from

as

where

and $i^{*}$ is the same as before.

Proof. The proof is like that of Theorem 2.2. When we reach the final optimal solutions, by differentiating ${\bf Y}(t){\bf X}^{-1}(t)$ and right multiplying ${\bf X}(t)$, we get

By setting

and

in the latter equation, we find that a similar matrix fractional differential equation as (2.11) is obtained. □

2.3.   Open-loop and closed-loop optimal controls

As mentioned in ^[13], we cannot use $N = 1$ for the wavelets definitions, because we return to polynomials concepts. By choosing $N = 1$, we have the shifted Legendre polynomials $\bar{P}_{m}(t)$ and the shifted Chebyshev polynomials $\bar{T}_{m}(t)$ instead of Legendre and Chebyshev wavelets. By using the symbol $\varphi_{m}(t)$ for any of these polynomials, we expand a function $f(t)$ on $[0, 1]$ as

where

and

The coefficients $\{f_{m}^{\varphi}\}$ for $w_{\varphi}(t)$ as the weight function, which is

or

are given by

For ${\bf f}_{\varphi}$ in (2.14) and ${\bf{ \pmb{\mathsf{ φ}} }}(t)$ as a vector of $\bar{P}_{m}(t)$ or $\bar{T}_{m}(t)$, we have ^[13]

${}^{RL}_{\; \; \; }I^{\alpha}_{}$ denotes the Riemann-Liouville integral of order $\alpha$.

2.3.1.   Open-loop optimal controllers

Theorem 2.4. The open-loop optimal control for the fractional nonlinear optimal control problem of minimizing the performance index (2.3) subject to the dynamical system (2.4) is obtained from: for $i \ge 1,$

subject to

where

Proof. From Lemma 2.1,

and

Since ${\bf A}^{[i-1]}$ and ${\bf B}^{[i-1]}$ are known, we can write

and

Also, ${\bf x}_{0}$, ${\bf Q}(t)$ and ${\bf R}(t)$ can be expanded as

and

Now, we expand the state and control vectors of each iteration as

and

where ${\bf x}^{[i]}_{\varphi}$ and ${\bf u}^{[i]}_{\varphi}$ are unknown. Thus, using(2.15)–(2.17), we see the original nonlinear optimization problem is transformed into the sequence of quadratic programming (QP) problems given in (2.19) and (2.20) until the condition given in ^[18] is reached. □

Remark 2.2. We have used the subscript "${\varphi}$" in the blocks of the QP model (2.19) and (2.20) to indicate that these blocks must be obtained from ${\varphi}$ and they differ significantly for each polynomial. We can also find the open-loop solutions by using fractional derivative properies like the method given in ^[7].

2.3.2.   Closed-loop optimal controllers

Theorem 2.5. By

the solution of (2.11)–(2.13) in the terms of the deired polynomials is

where $i^{*}$ is given in Theorem 2.1 and

Proof. Using the polynomials expansions,

Applying the right Riemann-Liouville integral on (2.11), using (2.18), and from the procedure given in ^[13],

Finally, by finding ${\bf P}^{[i^{*}-1]}(t)$ from (2.12) and using (2.8), we are able to obtain the closed-loop optimal control of the fractional nonlinear system. □

Remark 2.3. When ${\bf A}^{[i-1]}$ or ${\bf B}^{[i-1]}$ is not a function of ${\bf x}^{[i-1]}$ or ${\bf u}^{[i-1]}$, we replace $\tilde{{\bf A}}^{[i-1]}$ or $\tilde{{\bf B}}^{[i-1]}$ by $\tilde{{\bf A}}$ or $\tilde{{\bf B}}$, respectively. That means the matrix remains unchanged in each iteration.

2.3.3.   Redesigned closed-loop optimal control

Due to the local error of the approximation process and in order to overcome the issue given in Remark 2.1, we use a suboptimal controller to find an optimal control that has many advantages. By using the idea given in ^[13], the redesigned closed-loop optimal control is

where ${\bf K}^{[i^{*}-1]}(t)$ is the same as before and only the term ${\bf l}(t)$ is imposed to compensate the local error.

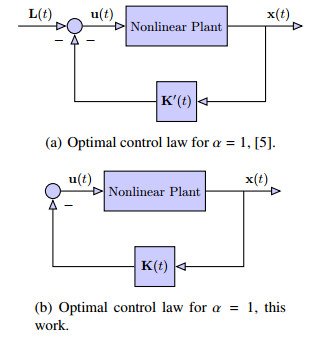
Corollary 2.1. If $\alpha = 1$, we have ${\bf l}(t) = {\bf 0}$ in (2.24); hence, the proposed controller is linear, as shown in Figure 1b. Also, we have

Theorem 2.6. Assume that for the problem given in (2.1) and (2.2),

is the exact closed-loop controller for $\alpha \ne 1$, then the controlled system with the redesigned closed-loop optimal control has the same behaviors as that with the exact controller.

Proof. The proof consists of transforming the problem into a number of linear problems to which the open-loop controller can be applied and then using the results. □

Theorem 2.7. Assume that for the function ${\bf f}({\bf x}(t), {\bf u}(t), t)$ in (2.2), there exists constants $\nu_{1}, \nu_{2} \ge 0$ such that

and

where ${\bf x}, \bar{{\bf x}} \in \mathbb{R}^{q}$ and ${\bf u}, \bar{{\bf u}} \in \mathbb{R}^{r}$ and the state ${\bf x}(t)$ is bounded. For a control, ${\bf x}(\cdot |{\bf u})$ is continuous dependence.

Proof. We have

Since $||{{\bf K}}(t)||$ is also bounded, we take

where $\nu_2' \ge0$. From

we abtain

Hence, the state of the nonlinear system depends continuously on the functions involved. □

3.   Simulation studies

In this section, we apply the proposed method for finding the optimal control law to some fractional nonlinear optimal control problems. First, we apply it to an example studied in ^[5] for $\alpha = 1$ and a nonlinear controller was obtained. Here, by using our method, a linear controller for this nonlinear system is obtained (see Figure 1), then we study its fractional order plant. Also, we study the optimal control problem of a two-stage nonlinear continuous stirred-tank reactor as a highly nonlinear, large scale system. Finally, an example for showing the effectiveness of the proposed method is investigated.

3.1.   Example 1

The problem is finding the optimal control law for the (fractional order) Van der Pol oscillator problem. Consider the system

where $x_{1}(0) = 1$ and $x_{2}(0) = 0$. The performance index is

This problem for $\alpha = 1$ was studied in ^[5] in which the author has proposed a nonlinear optimal control law in the from

see ^[5]. This is shown in Figure 1(a). However, in our method, a linear optimal control law as

is proposed and its block diagram is shown in Figure 1(b). Clearly, implementation of our proposed controller is easier due to its linear nature. We found $J^* = 1.449390$; by considering Corollary 2.1, we have

The Riccati matrix coefficients and Kalman gains are shown in Figures 2 and 3 for $\alpha = 1$ and $\alpha = 0.9$. Moreover, the results for $\alpha = 0.8$ are given in Figure 4.

3.2.   Example 2

A two-stage nonlinear continuous stirred-tank reactor (CSTR) as a highly nonlinear system described by time-delay differential equations was used for optimal control studies in ^[19]. The authors transformed the system into the delay-free system; for more details, see ^[20]. Here, we use a fractional version of the modified CSTR with the new state equations as (3.1), where $\tau = 0.1$,

and

The fractional optimal control problem consists of determining the optimal control law, which minimizes the performance index

First, we linearize CSTR in the form (2.4), then by calculating the elements of the quadratic programming (2.19) and (2.20) from (2.21)–(2.23), we obtain the open-loop solutions. Next, by finding the Riccati matrix ${\bf P}^{[i^{*}-1]}(t)$ in (2.12) from solving (2.11), (2.13) and using (2.8) and (2.24), we find the optimal control law.

The results are shown in Figure 5. For the Riccati matrix, we found 16 coefficients. In order to see the symmetry of the specific values, we used the same colors for such values. The optimal control law is

Also, we change the performance index to verify the effectiveness of the method as

The new results are shown in Figure 6. The differences are obvious. We see that the method can handle the fractional nonlinear system with the Bolza type performance index.

3.3.   Example 3

Finally, in order to check effectiveness of the method, we apply it to the fractional type of an example as an unstable zero equilibrium in ^[21] that its integer order for finding the state estimate was solved by using the method of this reference and that of ^[22]. Consider the system

where ${\bf x}(0) = [1, 1]^{\top}$.

However, some of the given assumptions are not satisfied, but we apply the method. We choose the weighting matrices given in the text to apply the proposed method. The value of the control weighting matrix has no effect on the solutions. Clearly, the elements of the Kalman gain in this problem are zeros. Hence, we just show the Riccati coefficients for $\alpha = 1$ and $\alpha = 0.94$ obtained by the method in Figures 7 and 8. We can see that even for this system, the method provides the solution for the Riccati matrix.

4.   Conclusions

A new method has been introduced to find the closed-loop optimal control, the optimal state and performance index of a fractional nonlinear system. The optimally conditions of fractional nonlinear optimal control problems has been presented. By deriving the fractional linearized Riccati equation, a new method for finding the Riccati coefficient has been presented. A linear feedback controller for the integer order nonlinear optimal control problem has been introduced. As can been seen, the method can handle highly nonlinear optimal control problems. The future work can be: extending the proposed method to fractional nonlinear systems having undecomposed terms and known disturbances, extending the results of this paper to the case where $\alpha \in (1, 2)$, and considering the fractional optimal control problem with a Riemann-Liouville performance index ^[23].

Use of AI tools declaration

The author declares he has not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The author would like to express his sincere thanks to the editor and referees for all helpful suggestions on this article.

Conflict of interest

The author declares that there is no conflict of interest in this paper.