We prove the existence of a solution to a quasilinear system of degenerate equations, when the datum is in a Marcinkiewicz space. The main assumption asks the off-diagonal coefficients to have support in the union of a geometric progression of squares.
Citation: Patrizia Di Gironimo, Salvatore Leonardi, Francesco Leonetti, Marta Macrì, Pier Vincenzo Petricca. Existence of solutions to some quasilinear degenerate elliptic systems with right hand side in a Marcinkiewicz space[J]. Mathematics in Engineering, 2023, 5(3): 1-23. doi: 10.3934/mine.2023055
| [1] | David Cruz-Uribe, Michael Penrod, Scott Rodney . Poincaré inequalities and Neumann problems for the variable exponent setting. Mathematics in Engineering, 2022, 4(5): 1-22. doi: 10.3934/mine.2022036 |
| [2] | Aleksandr Dzhugan, Fausto Ferrari . Domain variation solutions for degenerate two phase free boundary problems. Mathematics in Engineering, 2021, 3(6): 1-29. doi: 10.3934/mine.2021043 |
| [3] | Antonio Vitolo . Singular elliptic equations with directional diffusion. Mathematics in Engineering, 2021, 3(3): 1-16. doi: 10.3934/mine.2021027 |
| [4] | Isabeau Birindelli, Giulio Galise . Allen-Cahn equation for the truncated Laplacian: Unusual phenomena. Mathematics in Engineering, 2020, 2(4): 722-733. doi: 10.3934/mine.2020034 |
| [5] | La-Su Mai, Suriguga . Local well-posedness of 1D degenerate drift diffusion equation. Mathematics in Engineering, 2024, 6(1): 155-172. doi: 10.3934/mine.2024007 |
| [6] | Chiara Gavioli, Pavel Krejčí . Deformable porous media with degenerate hysteresis in gravity field. Mathematics in Engineering, 2025, 7(1): 35-60. doi: 10.3934/mine.2025003 |
| [7] | Italo Capuzzo Dolcetta . The weak maximum principle for degenerate elliptic equations: unbounded domains and systems. Mathematics in Engineering, 2020, 2(4): 772-786. doi: 10.3934/mine.2020036 |
| [8] | Lucio Boccardo . A "nonlinear duality" approach to $ W_0^{1, 1} $ solutions in elliptic systems related to the Keller-Segel model. Mathematics in Engineering, 2023, 5(5): 1-11. doi: 10.3934/mine.2023085 |
| [9] | Mirco Piccinini . A limiting case in partial regularity for quasiconvex functionals. Mathematics in Engineering, 2024, 6(1): 1-27. doi: 10.3934/mine.2024001 |
| [10] | Tiziano Penati, Veronica Danesi, Simone Paleari . Low dimensional completely resonant tori in Hamiltonian Lattices and a Theorem of Poincaré. Mathematics in Engineering, 2021, 3(4): 1-20. doi: 10.3934/mine.2021029 |
We prove the existence of a solution to a quasilinear system of degenerate equations, when the datum is in a Marcinkiewicz space. The main assumption asks the off-diagonal coefficients to have support in the union of a geometric progression of squares.
In this article we consider the following quasilinear boundary value problem
| {−div(a(x,u(x))Du(x))=f(x),x∈Ωu=0,x∈∂Ω | (1.1) |
where Ω⊂Rn, with n≥3, f,u:Ω→RN, with N≥2, and a:Ω×RN→RN2n2 is a matrix valued function whose entries are aα,βi,j(x,u) with i,j∈{1,…,n} and α,β∈{1,…,N}. Therefore the first line in (1.1) is a system of N equations of the form
| −n∑i=1Di(n∑j=1N∑β=1aα,βi,j(x,u)Djuβ)=fαα=1,…,N. | (1.2) |
For the treated problem there is an extensive literature in the scalar case N=1.
In particular, for the existence of a suitably defined solution, the Reader can refer to the papers [9,10,14,58,69] while, relatively to uniqueness and a priori estimates, we can quote respectively the papers [67] and [3]. For what concerns the regularity of a solution we cite the works [35,38]. Moreover, similar conclusions for the nonlinear case can be found in [2,8,12] and for the anisotropic case in [4,36]. Subsequently the aforementioned results have been extended to the operator with lower order terms too (see also [15,16,17,20,51]). In this context one can also see [7,11,12,24,25,30]. Furthermore, in [37] the right hand side appears in divergence form, that is f=−divF and in [1] the biharmonic operator is studied.
For further regularity results concerning elliptic operators the Reader is invited to refer to the foundamental works [5,6,26,27,28,41,42,43,44,45,46,47,48,64] and the survey [65].
As it is shown by the De Giorgi's counterexample [29], see also [39,40,49,60,61], the good regularity properties obtained in the scalar case can not be in general extended to the vectorial one, unless new structural assumptions are introduced.
An existence result of bounded weak solution for nonlinear degenerate elliptic systems is obtained in [55], using a componentwise coercivity condition. In several other papers, conditions on the support of the off-diagonal coefficients aα,βi,j(x) have been used to address different problems. Let us mention that a maximum principle result is obtained in [66] where the assumption is aα,βi,j(x,y)=0 for α≠β when yα is large and in [52] where different shapes of support are considered. Hölder continuity of the solutions is proved in [70] for a tridiagonal system, aα,βi,j=0 for β>α. L∞ regularity results are obtained in [53] for an oblique type of support for the coefficients and in [54] for a butterfly support. Measure data problems are faced in [56] and [57] where the support of aα,βi,j(x,y) is contained in squares along the yα=±yβ diagonals.
These kind of assumptions on the coefficients have been recently employed also to deal with degenerate elliptic systems. In this context there are results on problem (1.1) when the datum f∈Lm, which extend the ones contained in [14] for the scalar case. Namely, in [31] the existence of a bounded solution is proved when m>n2, assuming a butterfly support for the off-diagonal coefficients; moreover in [32] the case of a datum f with an intermediate grade of integrability (m<n2) is treated, thanks to an appropriate choice of the support for the off-diagonal coefficient.
In this paper we extend to the degenerate vectorial problem (1.1) an existence result concerning degenerate scalar operators, with the datum f in a suitable Marcinkiewicz space, contained in [14,62,63] (see also [21,22]). Since we are dealing with the vectorial case the support of the coefficients is required to have a particular structure. In Section 2 we give the precise notions of degenerate ellipticity and Marcinkiewicz spaces, see respectively (A2) and definition 1, while the assumption on the shape of the support of the coefficients is stated in (A3).
Also in this context the extension to the vectorial case of the known result in the scalar one is not obvious. Indeed, starting from De Giorgi's counterexample, it is possible to construct an example of an elliptic system with datum f∈Lp for every p<n, whose unique solution is unbounded and has low integrability, see [31] for details on the counterexample.
When dealing with systems of N equations, like (1.2), whose coefficients are only measurable with respect to x, little is known. Most articles are devoted to study existence or regularity of solutions of systems with right hand side fα∈Lm, either when m is large, or when m is small. When m is large, namely m>n2, existence of bounded solutions is obtained in [31].
When m is small, namely m=1, or even when fα is a measure, existence of solutions have been studied for general systems
| −n∑i=1Di(Aαi(x,u,Du))=fα,α=1,…,N, | (1.3) |
under structure conditions on Aαi. Namely, in [33] and [34], authors assume that
| 0≤n∑i=1Aαi(x,y,ξ)((Id−b×b)ξ)αi | (1.4) |
for every b∈RN with |b|≤1. On the other hand, in [71], the author assumes the componentwise sign condition
| 0≤n∑i=1Aαi(x,y,ξ)ξαi | (1.5) |
for every α=1,…,N. When N=2, (1.4) implies (1.5): it is enough to take first b=(1,0), then b=(0,1). Note that, in the present paper, we address the quasilinear case
| Aαi(x,y,ξ)=N∑β=1n∑j=1aα,βi,j(x,y)ξβj; | (1.6) |
in this case, as far as one off-diagonal coefficient a˜α,˜β˜i,˜j(x,y) is non zero, then (1.5) is no longer true: it is enough to take α=˜α, ξβj=0 if β∉{˜α,˜β}, ξ˜αi=0 if i≠˜i, ξ˜α˜i=1, ξ˜βj=0 if j≠˜j, ξ˜β˜j=ta˜α,˜β˜i,˜j(x,y)|a˜α,˜β˜i,˜j(x,y)|2 with t→−∞. When N=2, failure of (1.5) implies failure of (1.4). We recall that the study of quasilinear systems (1.2) with fα∈L1 is contained in [57] under the assumption that the support of off-diagonal coefficients is contained in a sequence of squares with side lenght r along the diagonals of the yα−yβ plane.
Concerning existence and regularity of suitable defined solutions of linear ellitptic systems
| −n∑i=1Di(Aαi(x)Du)=fα,α=1,…,N, |
with VMO coefficients and datum f=(fα) in a Lebesgue space Lγ with γ∈(1,2nn+2] (i.e., below the duality exponent) or in a suitable Lorentz-Morrey space one can refer also to [50]. While if f belongs to the natural dual Lebesgue space but the linear operator in not coercive due to the presence of a lower order term, called "drift term",
| −n∑i=1Di[Aαi(x)Du−Eαi(x)u]=fα(x) |
then existence and regularity results can be found in [19]. The above result has been extended to non linear operator under the so-called Landes condition (similar to (1.4)) with datum being in L1 or in L2nn+2 respectively in [18,23].
In the present work we address the existence of a regular solution to (1.2) when fα has an intermediate degree of integrability, namely, fα∈Mm with 1<2nn+2−θ(n−2)<m<n2 and Mm is the Marcinkiewicz space. In this case, the higher degree of integrability of the right hand side fα allows us to weaken the condition on the support of off-diagonal coefficients.
In the next section we present assumptions on the coefficients and on the datum f and our result. In Section 3 we consider a sequence of approximating non degenerate problems and we prove estimates on their weak solutions; then, with a limit procedure, we get the result for our problem.
For all i,j∈{1,…,n} and all α,β∈{1,…,N} we assume that aα,βi,j:Ω×RN→R satisfies the following conditions:
(A0) x↦aα,βi,j(x,y) is measurable and y↦aα,βi,j(x,y) is continuous;
(A1) (boundedness of all the coefficients) there exists c>0 such that
| |aα,βi,j(x,y)|≤c |
for almost every x∈Ω and for all y∈RN;
(A2) (degenerate ellipticity of all the coefficients) there exist constants ν>0 and θ∈(0,1) such that
| N∑α,β=1n∑i,j=1aα,βi,j(x,y)ξαiξβj≥νN∑α=1|ξα|2(1+|yα|)θ, |
for almost every x∈Ω, for all y∈RN and ξ∈RN×n;
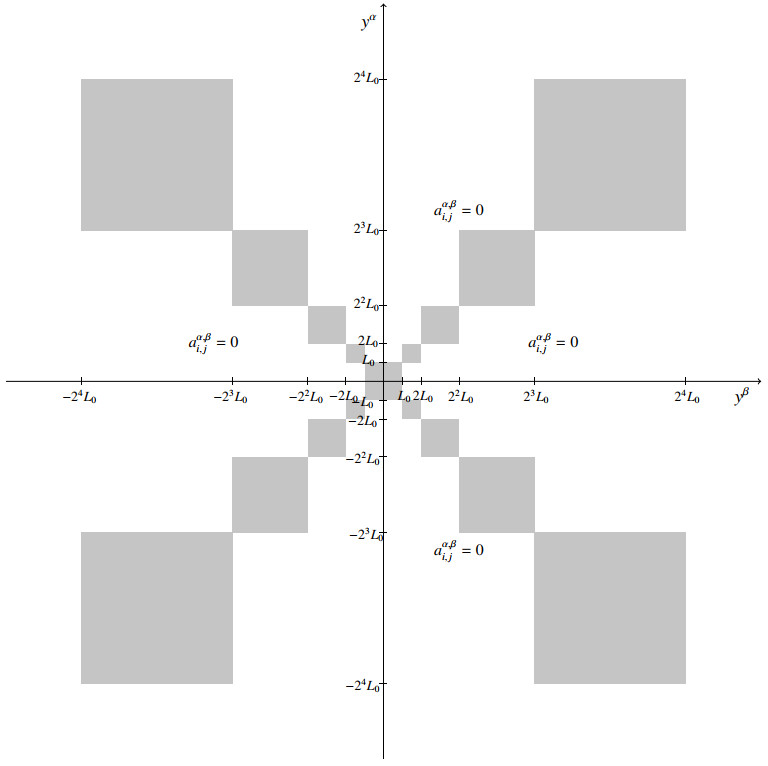
(A3) (support of off-diagonal coefficients) there exists L0≥1 such that (A′3) and (A″3) hold, where
(A′3) (support of off-diagonal coefficients contained in a central square) if aα,βi,j(x,y)≠0 and 0≤|yα|<L0, then it holds also 0≤|yβ|<L0;
(A″3) (support of off-diagonal coefficients contained in the union of a geometric progression of squares) if aα,βi,j(x,y)≠0 and there exists t∈N∪{0} such that 2tL0≤|yα|<2t+1L0, then it holds also 2tL0≤|yβ|<2t+1L0.
Let us remark that from assumption (A2) it follows that we have degeneracy in the α equation when uα is large. In [13] is treated for N=2 the case in which degeneracy in the α equation arises when uβ is large, with β≠α.
Note that (A′3) and (A″3) are always fulfilled when α=β. On the contrary, when α≠β, (A3) forces the support of aα,βi,j(x,y) to be contained in the union of infinite squares along the diagonals, see grey region in Figure 1.
On f we assume that it belongs to the Marcinkiewicz space Mm(Ω,RN), with
| 2nn+2−θ(n−2)<m<n2. |
For the convenience of the Reader, we recall the definition of Marcinkiewicz spaces, also known as weak Lebesgue spaces.
Definition 2.1. Let m be a positive number. We say that a measurable function f:Ω→R belongs to the Marcinkiewicz space Mm(Ω,R) if there exists a positive constant c sucht that
| |{x∈Ω:|f(x)|>t}|<ctm,∀t>0; | (2.1) |
in such a case we set
| Mm(f,Ω)=(inf |
M^m(\Omega, \mathbb{R}^N) is the space of functions f = (f^1, \ldots, f^N) such that f^i \in M^m(\Omega, \mathbb{R}) for each i . Moreover M_m(f) = \sum_{\alpha = 1}^N M_m(f^{\alpha}) .
We recall some properties on Marcinkiewicz spaces:
| \begin{equation} \begin{split} & L^m(\Omega) \subset M^m(\Omega) \subset L^{m- \varepsilon} (\Omega), \; \, \forall \, m > 1, \, \forall \, 0 < \varepsilon \leq m-1 \end{split} \end{equation} | (2.2) |
and
| \begin{equation} \int_{E} |f| dx \leq M_m(f, \Omega) |E|^{1-\frac{1}{m}}, \quad \forall f \in M^m(\Omega), \quad \forall E \subset \Omega. \end{equation} | (2.3) |
For more details on Marcinkiewicz space see [10,68].
Let us explicitly remark that, being 0 < \theta < 1 , from (2.2) it follows that
| \begin{equation} f \in L^{\frac{2n}{n+2}} (\Omega), \quad \forall f \in M^m(\Omega) \;{\rm{ with }} \;m > \frac{2n}{n+2-\theta (n-2)}. \end{equation} | (2.4) |
Under our set of assumptions we prove the following theorem:
Theorem 2.1. Assume (\mathcal{A}_0) , (\mathcal{A}_1) , (\mathcal{A}_2) , (\mathcal{A}_3) , with n \geq 3 . If f \in M^m(\Omega, \mathbb{R}^N) , with \frac{2n}{n+2-\theta(n-2)} < m < \frac{n}{2} , then there exists u \in W_0^{1, 2}(\Omega, \mathbb{R}^N) \cap M^r(\Omega, \mathbb{R}^N) , with
| \begin{equation} r = \frac{nm(1-\theta)}{n-2m}, \end{equation} | (2.5) |
weak solution of the problem (1.1), that is such that
| \begin{equation} \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n a_{i,j}^{\alpha, \beta} (x, u(x)) D_j u^\beta (x) D_i \varphi^\alpha (x) dx = \int_{\Omega} \sum\limits_{\alpha = 1}^N f^\alpha (x) \varphi^\alpha (x) dx \end{equation} | (2.6) |
for all \varphi \in W_0^{1, 2}(\Omega, \mathbb{R}^N) .
We set for all k \in \mathbb{N}
| \begin{equation*} \tilde{a}_{i,j,k}^{\alpha, \beta} (x, y) = a_{i,j}^{\alpha, \beta} (x, y)+ \frac{1}{k} \delta_{\alpha, \beta} \delta_{i,j} \end{equation*} |
with
| \begin{equation*} \delta_{i, j} = \begin{cases} 1 & {\rm{ if }}\; i = j \\ 0 & {\rm{ if }}\; i \ne j . \end{cases} \end{equation*} |
We consider the following family of approximating problems
| \begin{equation} \begin{cases} -\sum\limits_{i = 1}^n D_i \left( \sum\limits_{j = 1}^n \sum\limits_{\beta = 1}^N \tilde{a}_{i,j,k}^{\alpha, \beta} (x, u_k) D_j u_k^{\beta} \right) = f^\alpha ,& x \in \Omega \\ u_k = 0, & x \in \partial \Omega. \end{cases} \end{equation} | (\mathcal{\tilde{P}}_k) |
We want to show the existence of a weak solution for each problem ( \mathcal{\tilde{P}}_k ), that is a function u_k \in W_0^{1, 2}(\Omega, \mathbb{R}^N) such that
| \begin{equation} \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n \tilde{a}_{i,j,k}^{\alpha, \beta} (x, u_k(x)) D_j u_k^\beta (x) D_i \varphi^\alpha (x) dx = \int_{\Omega} \sum\limits_{\alpha = 1}^N f^\alpha (x) \varphi^\alpha (x) dx \end{equation} | (3.1) |
for all \varphi \in W_0^{1, 2}(\Omega, \mathbb{R}^N) .
Let us first show some properties of the coefficients \tilde{a}_{i, j, k}^{\alpha, \beta} . From assumption ( \mathcal{A}_1 ) it follows that
| \begin{equation} |\tilde{a}_{i,j,k}^{\alpha, \beta} (x, y)| \leq c+1. \end{equation} | (\mathcal{\tilde{A}}_1) |
Using assumption ( \mathcal{A}_2 ) we have the following non degenerate ellipticity condition
| \begin{equation} \tag{ \mathcal{\tilde{A}}_2 } \begin{split} \sum\limits_{\alpha, \beta = 1}^N & \sum\limits_{i,j = 1}^n \tilde{a}_{i,j,k}^{\alpha, \beta} (x, y) \xi_i^\alpha \xi_j^\beta = \\ & = \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n a_{i,j}^{\alpha, \beta} (x, y) \xi_i^\alpha \xi_j^\beta + \frac{1}{k} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n \delta_{\alpha, \beta}\delta_{i, j} \xi_i^\alpha \xi_j^\beta \geq \\ & \geq \nu \sum\limits_{\alpha = 1}^N \frac{|\xi^\alpha|^2}{(1+|y^\alpha|)^\theta} + \frac{1}{k} |\xi|^2. \end{split} \end{equation} | (3.4) |
Now let us show that for all f \in M^m(\Omega, \mathbb{R}^N) , with m > \frac{2n}{n+2-\theta(n-2)} , the linear operator
| \begin{equation*} \begin{split} F : W_0^{1,2}(\Omega, \mathbb{R}^N) & \to \mathbb{R} \\ v \quad & \mapsto \int_{\Omega} \sum\limits_{\alpha = 1}^N f^{\alpha}(x) v^{\alpha}(x) dx \end{split} \end{equation*} |
is continuous. Indeed, using Hölder inequality, (2.4) and Sobolev embedding, we have for a suitable constant C > 0
| \begin{equation*} \begin{split} |F(v)| & = \left|\int_{\Omega} \sum\limits_{\alpha = 1}^N f^{\alpha}(x) v^{\alpha}(x) dx\right| \leq \sum\limits_{\alpha = 1}^N \int_{\Omega}|f^{\alpha}(x) v^{\alpha}(x)| dx \leq \\ & \leq \sum\limits_{\alpha = 1}^N \|f^{\alpha}\|_{L^{\frac{2n}{n+2}}} \|v^{\alpha}\|_{L^{\frac{2n}{n-2}}} \leq \\ & \leq C \sum\limits_{\alpha = 1}^N \|f^{\alpha}\|_{L^{\frac{2n}{n+2}}} \|v\|_{W_0^{1,2}(\Omega, \mathbb{R}^N)} \end{split} \end{equation*} |
and the continuity of F is proved. Therefore we can apply the surjectivity result of Leray-Lions, see [59], and we have the existence of a weak solution u_k for the problem (\mathcal{\tilde{P}}_k) , that is, there exists u_k \in W^{1, 2}_0(\Omega, \mathbb{R}^N) such that (3.1) holds true for every \varphi \in W^{1, 2}_0(\Omega, \mathbb{R}^N) .
In the next Lemma 3.1, arguing as in [14], we prove that the sequence \{u_k\}_{k \in \mathbb{N}} is bounded in W_0^{1, 2}(\Omega, \mathbb{R}^N) \cap M^r(\Omega, \mathbb{R}^N) . We first recall the following elementary inequalities that will be used in the proof of Lemma 3.1. We have
| \begin{align} & \sum\limits_{\alpha = 1}^M a_{\alpha}^p \leq M \left(\sum\limits_{\alpha = 1}^M a_{\alpha}\right)^p , \end{align} | (3.2) |
| \begin{align} & \left( \sum\limits_{\alpha = 1}^M a_{\alpha} \right)^p \leq M^p \sum\limits_{\alpha = 1}^M (a_{\alpha} )^p, \end{align} | (3.3) |
| \begin{align} & \sum\limits_{\alpha = 1}^M ( a_{\alpha} \, b_{\alpha}) \leq \left(\sum\limits_{\alpha = 1}^M a_{\alpha}\right) \left(\sum\limits_{\alpha = 1}^M b_{\alpha}\right), \end{align} | (3.4) |
provided a_{\alpha}, b_{\alpha} \geq 0 for all \alpha \in \{ 1, \dots, M\} and p > 0 .
Lemma 3.1. Assume that f \in M^m(\Omega, \mathbb{R}^N) with \frac{2n}{n+2-\theta(n-2)} < m < \frac{n}{2} and let u_k be a weak solution of (\mathcal{\tilde{P}}_k) . Then the sequences \|u_k\|_{W_0^{1, 2}(\Omega, \mathbb{R}^N)} and M_r(u_k, \Omega) , with r given in (2.5), are bounded by a positive constant which depends only on L_0 , \theta , m , n , N , \nu , |\Omega| and M_m(f, \Omega) .
Proof. For any t \in \mathbb{N} \cup \{0\} and for L_0 \geq 1 given by assumption (\mathcal{A}_3) , we define the following functions
| \begin{equation*} G_{2^t L_0}(s) = \begin{cases} 0 & {\rm{ if }}\; |s| \leq 2^t L_0 \\ s - 2^t L_0 \frac{s}{|s|} & {\rm{ if }}\; |s| > 2^t L_0 \end{cases} \end{equation*} |
and
| \begin{equation*} T_{2^t L_0}(s) = \begin{cases} s & {\rm{ if }}\; -2^tL_0 \leq s \leq 2^t L_0 \\ 2^t L_0 & {\rm{ if }}\; s > 2^t L_0 \\ - 2^t L_0 & {\rm{ if }}\; s < - 2^t L_0. \end{cases} \end{equation*} |
We consider as test function in (3.1) the function \varphi_t \in W_0^{1, 2}(\Omega, \mathbb{R}^N) defined as
| \begin{equation} \varphi_t = (\varphi_t^1, \ldots , \varphi_t^N) = (T_{2^t L_0}(G_{2^t L_0}(u_k^1)), \ldots, T_{2^t L_0}(G_{2^t L_0}(u_k^N))). \end{equation} | (3.5) |
We introduce the sets
| \begin{equation*} A_{k,2^t L_0}^{\alpha} = \{ x\in \Omega: \vert u_k^{\alpha} \vert \ge 2^t L_0 \} \; {\rm{ and }} \; B_{k,2^t L_0}^{\alpha} = \{ x\in \Omega : 2^t L_0\le \vert u_k^{\alpha} \vert < 2^{t+1} L_0 \} . \end{equation*} |
For all \alpha \in \{1, \ldots, N\} we have
| \begin{equation} {\rm{supp}} \, \varphi_t^{\alpha} \subset A_{k,2^t L_0}^{\alpha}, \quad |\varphi_t^{\alpha}| \leq 2^t L_0 \; \quad {\rm{ and }} \quad D_i \varphi_t^{\alpha} = D_i u_k^{\alpha} \; \mathbb{I}_{B_{k,2^t L_0}^{\alpha}}, \end{equation} | (3.6) |
where \mathbb{I}_B(x) = 1 if x \in B and \mathbb{I}_B(x) = 0 otherwise. Moreover, using (\mathcal{A''}_3) , we have
| \begin{equation} a_{i,j}^{\alpha, \beta} (x, u_k(x)) \mathbb{I}_{B_{k,2^t L_0}^{\alpha}} (x) = a_{i,j}^{\alpha, \beta} (x, u_k(x)) \mathbb{I}_{B_{k,2^t L_0}^{\alpha}} (x) \mathbb{I}_{B_{k,2^t L_0}^{\beta}}(x). \end{equation} | (3.7) |
Indeed, if a_{i, j}^{\alpha, \beta} (x, u_k(x)) = 0 or x\notin B_{k, 2^t L_0}^{\alpha} , then the (3.7) is obvious. If a_{i, j}^{\alpha, \beta} (x, u_k(x)) \neq 0 and x\in B_{k, 2^t L_0}^{\alpha} , that is 2^t L_0\le \vert u_k^{\alpha} \vert < 2^{t+1} L_0 , then for (\mathcal{A''}_3) we have 2^t L_0 \le \vert u_k^{\beta} \vert < 2^{t+1} L_0 so that x\in B_{k, 2^t L_0}^{\beta} .
From (3.6), (3.7) and (\mathcal{A}_2) we have
| \begin{equation} \begin{split} & \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n \tilde{a}_{i,j,k}^{\alpha, \beta} (x, u_k(x)) D_j u_k^\beta (x) D_i \varphi_t^\alpha (x) = \\ & = \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n (a_{i,j}^{\alpha, \beta} (x, u_k(x)) + \frac{1}{k} \delta_{\alpha, \beta} \delta_{i,j})D_j u_k^\beta (x) D_i u_k^\alpha (x) \mathbb{I}_{B_{k,2^t L_0}^{\alpha}}(x) = \\ & = \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n a_{i,j}^{\alpha, \beta} (x, u_k(x)) D_j u_k^\beta (x) \mathbb{I}_{B_{k,2^t L_0}^{\beta}}(x) D_i u_k^\alpha (x) \mathbb{I}_{B_{k,2^t L_0}^{\alpha}}(x) + \\ & \qquad + \sum\limits_{\alpha = 1}^N \sum\limits_{i = 1}^n \frac{1}{k}|D_i u_k^\alpha (x)|^2 \mathbb{I}_{B_{k,2^t L_0}^{\alpha}} \geq \\ & \geq \nu \sum\limits_{\alpha = 1}^N \frac{|D u_k^\alpha (x) \mathbb{I}_{B_{k,2^t L_0}^{\alpha}}(x)|^2}{(1+|u_k^{\alpha}(x)|)^{\theta}} . \end{split} \end{equation} | (3.8) |
Then, replacing in the left side of (3.1) the test function (3.5) and using (3.8), we get
| \begin{equation} \begin{split} & \int_{\Omega}\sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n \tilde{a}_{i,j,k}^{\alpha, \beta} (x, u_k(x)) D_j u_k^\beta (x) D_i \varphi_t^\alpha (x) dx \geq \\ &\geq \nu \sum\limits_{\alpha = 1}^N \int_{B_{k,2^t L_0}^{\alpha}} \frac{|D u_k^\alpha (x) |^2 }{(1+|u_k^{\alpha}(x)|)^{\theta}}dx \ge \nu \sum\limits_{\alpha = 1}^N \int_{B_{k,2^t L_0}^{\alpha}} \frac{|D u_k^\alpha (x) |^2}{(1+2^{t+1}L_0)^{\theta}} dx \\ & = \frac{\nu} {(1+2^{t+1}L_0)^{\theta}} \sum\limits_{\alpha = 1}^N \int_{B_{k,2^t L_0}^{\alpha}}|D u_k^\alpha (x) |^2 dx. \end{split} \end{equation} | (3.9) |
Combining (3.9) with (3.1), we get
| \begin{equation} \begin{split} & \sum\limits_{\alpha = 1}^N \int_{B_{k,2^t L_0}^{\alpha}} \vert Du_k^{\alpha}(x) \vert^2 dx \le \frac{(1+2^{t+1}L_0)^{\theta}}{\nu} \int_{\Omega} \sum\limits_{\alpha = 1}^N f^\alpha (x) \varphi_t^\alpha (x) dx = \\ & = \frac{(1+2^{t+1}L_0)^{\theta}}{\nu} \sum\limits_{\alpha = 1}^N \int_{A_{k,2^t L_0}^{\alpha}} f^\alpha (x) \varphi_t^\alpha (x) dx . \end{split} \end{equation} | (3.13) |
Using Sobolev's embedding and (3.10) we have
| \begin{equation} \begin{split} & \left( \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^\ast} dx \right)^{\frac{2}{2^{\ast}}} = \left( \int_{\Omega} |\varphi_t^\alpha (x)|^{2^\ast} dx \right)^{\frac{2}{2^{\ast}}} \leq \\ & \leq C_S \int_{\Omega} |D\varphi_t^\alpha (x)|^{2} dx = C_S \int_{\Omega} \sum\limits_{i = 1}^n |D_i\varphi_t^\alpha (x)|^{2} dx = \\ & = C_S \int_{\Omega} \sum\limits_{i = 1}^n |D_i u_k^\alpha (x)\mathbb{I}_{B^{\alpha}_{k,2^t L_0}}|^{2} dx = C_S \int_{B_{k,2^t L_0}^{\alpha}} \sum\limits_{i = 1}^n |D_iu_k^\alpha (x)|^{2} dx = \\ & = C_S \int_{B_{k,2^t L_0}^{\alpha}} |D u_k^\alpha (x)|^{2} dx, \end{split} \end{equation} | (3.11) |
where C_S is the Sobolev embedding constant. Summing on \alpha in (3.11) and using (3.10), we have
| \begin{equation} \begin{split} & \sum\limits_{\alpha = 1}^N \left( \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^\ast} dx \right)^{\frac{2}{2^{\ast}}} \leq C_S \sum\limits_{\alpha = 1}^N \int_{B_{k,2^t L_0}^{\alpha}} |D u_k^\alpha (x)|^{2} dx \leq \\ & \leq C_S \frac{(1+2^{t+1}L_0)^{\theta}}{\nu} \sum\limits_{\alpha = 1}^N \int_{A_{k,2^t L_0}^{\alpha}} f^\alpha (x) \varphi_t^\alpha (x) dx . \end{split} \end{equation} | (3.12) |
From (2.4) we have f \in L^{\frac{2n}{n+2}}(\Omega) and, by Sobolev immersion, we have also \varphi_t \in L^{2^{\ast}} = L^{\frac{2n}{n-2}} . Then, using the Hölder inequality with exponents \frac{2n}{n+2} and \frac{2n}{n-2} = 2^{\ast} and applying (2.3) to the function |f^{\alpha}|^{\frac{2n}{n+2}} \in M^{\frac{(n+2)m}{2n}}(\Omega) , we deduce for all \alpha = 1, \ldots, n
| \begin{equation} \begin{split} & \int_{A_{k,2^t L_0}^{\alpha}} f^\alpha (x) \varphi_t^\alpha (x) dx \leq \\ & \leq \left(\int_{A_{k,2^t L_0}^{\alpha}} |f^\alpha (x)|^\frac{2n}{n+2} \right)^{\frac{n+2}{2n}} \left( \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^{\ast}} dx \right)^{\frac{1}{2^{\ast}}} \leq \\ & \leq M_m(f^{\alpha}, \Omega) |A_{k,2^t L_0}^{\alpha}|^{\frac{n+2}{2n}(1-\frac{2n}{(n+2)m})} \left( \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^{\ast}} dx \right)^{\frac{1}{2^{\ast}}}. \end{split} \end{equation} | (3.13) |
From (3.12) and (3.13) it follows that
| \begin{equation*} \begin{split} & \sum\limits_{\alpha = 1}^N \left( \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^\ast} dx \right)^{\frac{2}{2^{\ast}}} \leq \\ & \leq C_S \frac{(1+2^{t+1}L_0)^{\theta}}{\nu} \sum\limits_{\alpha = 1}^N M_m(f^{\alpha}, \Omega) |A_{k,2^t L_0}^{\alpha}|^{\frac{mn+2m-2n}{2nm}} \left( \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^{\ast}} dx \right)^{\frac{1}{2^{\ast}}} \leq \\ & \leq C_1 (2^{t}L_0)^{\theta} \sum\limits_{\alpha = 1}^N |A_{k,2^t L_0}^{\alpha}|^{\frac{mn+2m-2n}{2nm}} \left( \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^{\ast}} dx \right)^{\frac{1}{2^{\ast}}}, \end{split} \end{equation*} |
where C_1 is a constant depending only on C_s , \nu , M_m(f, \Omega) .
Now, using last inequality and (3.3), (3.4), (3.2), we have
| \begin{equation*} \begin{split} & \left( \sum\limits_{\alpha = 1}^N \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^\ast}dx \right)^{\frac{2}{2^{\ast}}} \leq N^{\frac{2}{2^{\ast}}} \sum\limits_{\alpha = 1}^N \left( \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^\ast} dx \right)^{\frac{2}{2^{\ast}}} \leq \\ & \leq N^{\frac{2}{2^{\ast}}} C_1 (2^t L_0)^{\theta} \sum\limits_{\alpha = 1}^N |A_{k,2^t L_0}^{\alpha}|^{\frac{mn+2m-2n}{2nm}} \left( \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^{\ast}} dx \right)^{\frac{1}{2^{\ast}}} \leq \\ & \leq N^{\frac{2}{2^{\ast}}} C_1 (2^t L_0)^{\theta} \left(\sum\limits_{\alpha = 1}^N |A_{k,2^t L_0}^{\alpha}|^{\frac{mn+2m-2n}{2nm}} \right) \left[ \sum\limits_{\alpha = 1}^N \left( \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^{\ast}} dx \right)^{\frac{1}{2^{\ast}}} \right] \leq \\ & \leq N^{1+\frac{2}{2^{\ast}}} C_1 (2^t L_0)^{\theta} \left(\sum\limits_{\alpha = 1}^N |A_{k,2^t L_0}^{\alpha}|^{\frac{mn+2m-2n}{2nm}} \right) \left( \sum\limits_{\alpha = 1}^N \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^{\ast}} dx \right)^{\frac{1}{2^{\ast}}}; \end{split} \end{equation*} |
Therefore
| \begin{equation} \begin{split} & \left( \sum\limits_{\alpha = 1}^N \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^\ast}dx \right)^{\frac{1}{2^{\ast}}} \leq N^{1+\frac{2}{2^{\ast}}} C_1 (2^t L_0)^{\theta} \sum\limits_{\alpha = 1}^N |A_{k,2^t L_0}^{\alpha}|^{\frac{mn+2m-2n}{2nm}}. \end{split} \end{equation} | (3.14) |
Since \varphi_t^{\alpha} = T_{2^t L_0} (G_{2^t L_0}(u_k^{\alpha})) , for all t \in \mathbb{N} \cup \{0\} , we have
| \begin{equation*} \begin{split} & \left( \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^{\ast}} dx \right)^{\frac{1}{2^{\ast}}} \geq \left( \int_{A_{k,2^{t+1} L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^{\ast}} dx \right)^{\frac{1}{2^{\ast}}} = \\ & = \left( \int_{A_{k,2^{t+1} L_0}^{\alpha}} (2^t L_0)^{2^{\ast}} dx \right)^{\frac{1}{2^{\ast}}} = 2^t L_0 |A_{k,2^{t+1} L_0}^{\alpha}|^{\frac{1}{2^{\ast}}}; \end{split} \end{equation*} |
Then, summing on \alpha and using (3.2) we have
| \begin{equation} \begin{split} & \left( \sum\limits_{\alpha = 1}^N \int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^{\ast}} dx \right)^{\frac{1}{2^{\ast}}} \geq \frac{1}{N} \sum\limits_{\alpha = 1}^N \left(\int_{A_{k,2^t L_0}^{\alpha}} |\varphi_t^\alpha (x)|^{2^{\ast}} dx \right)^{\frac{1}{2^{\ast}}} \geq \\ & \geq \frac{1}{N} \sum\limits_{\alpha = 1}^N 2^t L_0 |A_{k,2^{t+1} L_0}^{\alpha}|^{\frac{1}{2^{\ast}}}. \end{split} \end{equation} | (3.15) |
From (3.14) and (3.15) it follows that
| \begin{equation*} \frac{1}{N} \sum\limits_{\alpha = 1}^N 2^t L_0 |A_{k,2^{t+1} L_0}^{\alpha}|^{\frac{1}{2^{\ast}}} \leq N^{1+\frac{2}{2^{\ast}}} C_1 (2^t L_0)^{\theta} \sum\limits_{\alpha = 1}^N |A_{k,2^t L_0}^{\alpha}|^{\frac{mn+2m-2n}{2nm}} \end{equation*} |
and then
| \begin{equation*} \sum\limits_{\alpha = 1}^N |A_{k,2^{t+1} L_0}^{\alpha}|^{\frac{1}{2^{\ast}}} \leq\frac{1}{(2^t L_0)^{1-\theta}} N^{2+\frac{2}{2^{\ast}}} C_1 \sum\limits_{\alpha = 1}^N |A_{k,2^t L_0}^{\alpha}|^{\frac{mn+2m-2n}{2nm}} . \end{equation*} |
From the last inequality and using (3.3) and (3.2) we have
| \begin{equation*} \begin{split} & \left(\sum\limits_{\alpha = 1}^N |A_{k,2^{t+1} L_0}^{\alpha}|\right)^{\frac{1}{2^{\ast}}} \leq N^{\frac{1}{2^{\ast}}} \sum\limits_{\alpha = 1}^N |A_{k,2^{t+1} L_0}^{\alpha}|^{\frac{1}{2^{\ast}}} \leq \\ & \leq \frac{1}{(2^t L_0)^{1-\theta}} N^{2+\frac{3}{2^{\ast}}} C_1 \sum\limits_{\alpha = 1}^N |A_{k,2^t L_0}^{\alpha}|^{\frac{mn+2m-2n}{2nm}} \leq \\ & \leq \frac{1}{(2^t L_0)^{1-\theta}} N^{3+\frac{3}{2^{\ast}}} C_1 \left(\sum\limits_{\alpha = 1}^N |A_{k,2^t L_0}^{\alpha}|\right)^{\frac{mn+2m-2n}{2nm}} ; \end{split} \end{equation*} |
Therefore
| \begin{equation} \sum\limits_{\alpha = 1}^N |A_{k,2^{t+1} L_0}^{\alpha}| \leq \frac{C_2}{(2^t L_0)^{(1-\theta)2^{\ast}}} \left(\sum\limits_{\alpha = 1}^N |A_{k,2^t L_0}^{\alpha}|\right)^{\frac{mn+2m-2n}{m(n-2)}}, \end{equation} | (3.16) |
where C_2 is a positive constant depending only on N , n , C_S , \nu and M_m(f, \Omega) .
Let us set
| \begin{equation*} \gamma = \frac{mn+2m-2n}{m(n-2)} \in (0,1) \end{equation*} |
and let us remark that for r given in (2.5) the following equality holds
| \begin{equation} r-(1- \theta) 2^{\ast} = r \gamma. \end{equation} | (3.17) |
Now, for all h > 0 and for r given in (2.5), let us define
| \begin{equation} \rho (h) = h^r \sum\limits_{\alpha = 1}^N |A_{k,h}^{\alpha}|. \end{equation} | (3.18) |
For all t \in \mathbb{N} \cup \{0\} , it follows from (3.16) that
| \begin{equation*} \begin{split} & \rho (2^{t+1} L_0) = (2^{t+1} L_0)^r \sum\limits_{\alpha = 1}^N |A_{k,2^{t+1} L_0}^{\alpha}| \leq \\ & \leq 2^r (2^t L_0)^r \frac{C_2}{(2^t L_0)^{(1-\theta)2^{\ast}}} \left(\sum\limits_{\alpha = 1}^N |A_{k,2^t L_0}^{\alpha}|\right)^{\frac{mn+2m-2n}{m(n-2)}} = \\ & = 2^r C_2 (2^t L_0)^{r \gamma} \left(\sum\limits_{\alpha = 1}^N |A_{k,2^t L_0}^{\alpha}|\right)^{\gamma} = \\ & = 2^rC_2 \left( (2^t L_0)^{r } \sum\limits_{\alpha = 1}^N |A_{k,2^t L_0}^{\alpha}|\right)^{\gamma} = 2^r C_2 [\rho(2^t L_0)]^{\gamma}. \end{split} \end{equation*} |
Therefore we obtain that there exists a constant C_3 = \max (1, 2^r C_2) \geq 1 , depending only on N , n , C_S , \nu , M_m(f, \Omega) , \theta and m such that, for all t \in \mathbb{N} \cup \{0\} , we have
| \begin{equation*} \rho (2^{t+1} L_0) \leq C_3 [\rho(2^t L_0)]^{\gamma} \end{equation*} |
and, arguing by induction, it follows that
| \begin{equation} \rho (2^{s} L_0) \leq C_3^{\sum\limits_{h = 0}^{s-1}\gamma^h} [\rho(L_0)]^{\gamma^s} \leq C_3^{\sum\limits_{h = 0}^{+\infty}\gamma^h} [\rho(L_0)]^{\gamma^s}, \quad \forall s \in \mathbb{N}. \end{equation} | (3.19) |
Being \gamma < 1 and \rho(L_0) \geq 0 , the elementary inequality
| \begin{equation} [\rho(L_0)]^{\gamma^s} \leq 1 +\rho(L_0), \quad \forall s \in \mathbb{N} \end{equation} | (3.20) |
holds. Using the notation C_4 = C_3^{\sum_{h = 0}^{+\infty}\gamma^h} = C_3^{\frac{1}{1-\gamma}} and putting together (3.19) and (3.20), we have
| \begin{equation} \rho (2^{s} L_0) \leq C_4 (1 +\rho(L_0) ), \quad \forall s \in \mathbb{N}, \end{equation} | (3.21) |
where C_4 \geq 1 is a constant depending only on N , n , C_S , \nu , M_m(f, \Omega) , \theta and m .
Using (3.21), we want to prove that there exists a constant C_5 depending only on N , n , C_S , \nu , M_m(f, \Omega) , \theta , m , L_0 and |\Omega| such that
| \begin{equation} \rho(h) = h^r \sum\limits_{\alpha = 1}^N |A_{k,h}^{\alpha}| \leq C_5, \quad \forall h \geq L_0. \end{equation} | (3.22) |
Indeed, for h \in [L_0, 2 L_0] , we have
| \begin{equation} \rho(h) = h^r \sum\limits_{\alpha = 1}^N |A_{k,h}^{\alpha}| \leq (2L_0)^r \sum\limits_{\alpha = 1}^N |\Omega| = (2L_0)^r N |\Omega| . \end{equation} | (3.23) |
For all h \geq 2 L_0 there exists s \in \mathbb{N} and w \in [L_0, 2 L_0) such that h = 2^s w . Then, using (3.21) and (3.23), we have for all h \geq 2 L_0
| \begin{equation} \begin{split} & \rho(h) = \rho(2^s w) = (2^s w)^r \sum\limits_{\alpha = 1}^N |A_{k,2^s w}^{\alpha}| \leq \\ & \leq (2^{s+1} L_0)^r \sum\limits_{\alpha = 1}^N |A_{k,2^s L_0}^{\alpha}| = 2^r(2^{s} L_0)^r \sum\limits_{\alpha = 1}^N |A_{k,2^s L_0}^{\alpha}| = \\ & = 2^r \rho(2^s L_0) \leq 2^r C_4 (1 +\rho(L_0) ) \leq \\ & \leq 2^r C_4 (1 +(2L_0)^r N |\Omega| ) : = C_5. \end{split} \end{equation} | (3.24) |
From (3.23) and (3.24) follows (3.22).
For all h \geq L_0 , using (3.22), we have
| \begin{equation} \sum\limits_{\alpha = 1}^N |\{ x \in \Omega : |u_k^{\alpha}| > h \}| \leq \sum\limits_{\alpha = 1}^N |A_{k,h}^{\alpha}| = \frac{\rho(h)}{h^r} \leq \frac{C_5}{h^r}; \end{equation} | (3.25) |
for h \in (0, L_0) we have
| \begin{equation} \sum\limits_{\alpha = 1}^N |\{ x \in \Omega : |u_k^{\alpha}| > h \}| \leq \sum\limits_{\alpha = 1}^N |\Omega| = N \Omega \leq \frac{N |\Omega|L_0^r}{L_0^r} < \frac{N |\Omega|L_0^r}{h^r}. \end{equation} | (3.26) |
Then, setting C_6 = \max (C_5, N |\Omega|L_0^r) , from (3.25) and (3.26), we get
| \begin{equation*} \sum\limits_{\alpha = 1}^N |\{ x \in \Omega : |u_k^{\alpha}| > h \}| \leq \frac{C_6}{h^r}, \quad \forall h > 0, \end{equation*} |
proving the boundness of the sequence \{u_k\}_{k \in \mathbb{N}} in M^r(\Omega) .
It remains to prove that the sequence \{u_k\}_{k \in \mathbb{N}} is bounded in W^{1, 2}_0(\Omega, \mathbb R^N).
From (3.10), for all t\in \mathbb N\cup \{0\} , we have
| \begin{equation} \begin{split} &\sum\limits_{\alpha = 1}^N \int_{\Omega}\vert Du_k^{\alpha}(x)\vert^2dx = \sum\limits_{\alpha = 1}^N \int_{\{ \vert u_k^{\alpha}\vert < L_0 \} } \vert Du_k^{\alpha}(x)\vert^2dx+\sum\limits_{\alpha = 1}^N \int_{\{ \vert u_k^{\alpha}\vert \ge L_0 \} } \vert Du_k^{\alpha}(x)\vert^2dx = \\ & = \sum\limits_{\alpha = 1}^N \int_{\{ \vert u_k^{\alpha}\vert < L_0 \} } \vert Du_k^{\alpha}(x)\vert^2dx+\sum\limits_{t = 0}^{+\infty} \sum\limits_{\alpha = 1}^N \int_{\{2^tL_0\le \vert u_k^{\alpha}\vert < 2^{t+1}L_0 \}}\vert Du_k^{\alpha}(x)\vert^2dx = \\ & = \sum\limits_{\alpha = 1}^N \int_{\{ \vert u_k^{\alpha}\vert < L_0 \} } \vert Du_k^{\alpha}(x)\vert^2dx+\sum\limits_{t = 0}^{+\infty} \sum\limits_{\alpha = 1}^N \int_{B_{k,2^tL_0}^{\alpha} }\vert Du_k^{\alpha}(x)\vert^2dx\le\\ &\le \sum\limits_{\alpha = 1}^N \int _{\{ \vert u_k^{\alpha} \vert < L_0 \} } \vert Du_k^{\alpha}(x)\vert^2dx+\sum\limits_{t = 0}^{+\infty} \frac{(1+2^{t+1}L_0)^{\theta}} {\nu} \sum\limits_{\alpha = 1}^N\int_{A_{k,2^tL_0}^{\alpha}} f^{\alpha}(x)\varphi_t^{\alpha}(x)dx.\\ \end{split} \end{equation} | (3.27) |
Now we estimate the right hand side of (3.27).
Observing that \vert \varphi_t^{\alpha}(x) \vert\le 2^tL_0 , for all x and for all t\in \mathbb{N}\cup \{0 \} , and using (2.3), we have
| \begin{equation*} \begin{split} & \int_{A_{k,2^tL_0}^{\alpha}} f^{\alpha}(x)\varphi_t^{\alpha}(x)dx \le 2^tL_0 \int_{A_{k,2^tL_0}^{\alpha}} \vert f^{\alpha}(x)\vert dx \le\\ & \le 2^tL_0 M_m(f^{\alpha},\Omega)\vert A_{k,2^tL_0}^{\alpha} \vert^{1-\frac{1}{m}} \leq 2^t L_0 M_m(f,\Omega)\vert A_{k,2^tL_0}^{\alpha} \vert^{1-\frac{1}{m}} . \end{split} \end{equation*} |
Summing on \alpha = 1, .., N the previous inequality, by (3.2), the definition of \rho in (3.18) and (3.22), we get
| \begin{equation*} \begin{split} &\sum\limits_{\alpha = 1}^N \int_{A_{k,2^tL_0}^{\alpha}} f^{\alpha}(x)\varphi_t^{\alpha}(x)dx \le 2^tL_0M_m(f,\Omega)\sum\limits_{\alpha = 1}^N \vert A_{k,2^tL_0}^{\alpha} \vert^{1-\frac{1}{m}}\le \\ &\le 2^tL_0M_m(f,\Omega) N \Big( \sum\limits_{\alpha = 1}^N \vert A_{k,2^tL_0}^{\alpha} \vert \Big)^{1-\frac{1} {m}} = 2^tL_0M_m(f,\Omega) N \Big (\frac {\rho(2^tL_0)}{(2^tL_0)^r} \Big)^{1-\frac{1}{m} } \le \\ & \le C_5^{1-\frac{1}{m}}2^tL_0 M_m(f,\Omega) N (2^{-tr})^{1-\frac{1}{m}} L_0^{-r(1-\frac{1}{m})} = \\ & = C_5^{1-\frac{1}{m}} M_m(f,\Omega) N (2^{1-r(1-\frac{1}{m})} )^t L_0^{1-r(1-\frac{1}{m})} .\\ \end{split} \end{equation*} |
From this inequality it follows that
| \begin{equation} \begin{split} &\sum\limits_{t = 0}^{+\infty} \frac{(1+2^{t+1}L_0)^{\theta}}{\nu} \sum\limits_{\alpha = 1}^N \int_{A_{k,2^tL_0}^{\alpha}} f^{\alpha}(x)\varphi_t^{\alpha}(x)dx \le \\ & \le \sum\limits_{t = 0}^{+\infty} \frac{(1+2^{t+1}L_0)^{\theta}} {\nu}C_5^{1-\frac{1} {m}} M_m(f,\Omega) N (2^{1-r(1-\frac{1}{m})} )^t L_0^{1-r(1-\frac{1}{m})} = \\ & = \frac { C_5^{1-\frac{1} {m}}M_m(f,\Omega) N L_0^{1-r(1-\frac{1}{m})} } {\nu} \sum\limits_{t = 0}^{+\infty} (1+2^{t+1}L_0)^{\theta} (2^{1-r(1-\frac{1}{m})})^t \le \\ & \le \frac { C_5^{1-\frac{1} {m}}M_m(f,\Omega) N L_0^{1-r(1-\frac{1}{m})} } {\nu} \sum\limits_{t = 0}^{+\infty} (2^{t+2}L_0)^{\theta} (2^{1-r(1-\frac{1}{m})})^t = \\ & = \frac { C_5^{1-\frac{1} {m}}M_m(f,\Omega) N L_0^{1-r(1-\frac{1}{m})} } {\nu} \sum\limits_{t = 0}^{+\infty} 2^{2\theta} 2^{t\theta} L_0^{\theta} (2^{1-r(1-\frac{1}{m})})^t = \\ & = \frac { C_5^{1-\frac{1} {m}}M_m(f,\Omega) N 2^{2\theta} L_0^{1-r(1-\frac{1}{m})+\theta} } {\nu} \sum\limits_{t = 0}^{+\infty} \big( 2^{\theta +1-r(1-\frac{1}{m})} \big)^t.\\ \end{split} \end{equation} | (3.28) |
Since \frac{2n}{n+2-\theta (n-2)} < m < \frac{n}{2} , it results that \theta +1-r(1-\frac{1}{m}) < 0 and the series in the right side of the last inequality converges; we have
| \begin{equation} \sum\limits_{t = 0}^{+\infty} \frac{(1+2^{t+1}L_0)^{\theta}}{\nu} \sum\limits_{\alpha = 1}^N \int_{A_{k,2^tL_0}^{\alpha}} f^{\alpha}(x)\varphi_t^{\alpha}(x)dx \le C_7, \end{equation} | (3.29) |
where C_7 is a positive constant depending only on n, N, m, \theta, \nu, M_m(f, \Omega), \vert \Omega \vert, C_S, L_0.
Now, let us prove that \sum_{\alpha = 1}^N \int _{\{ \vert u_k^{\alpha} \vert < L_0 \} } \vert Du_k^{\alpha}(x)\vert^2dx is bounded.
To this aim we use \psi = \big(\psi^1, ..., \psi^N\big) = \big(T_{L_0}(u_k^1), ..., T_{L_0}(u_k^N)\big) as a test function in the weakly formulation (3.1) of problem (\tilde P_k) . Observing that
| \begin{equation*} D_i\psi^{\alpha} = D_iu_k^{\alpha}\mathbb{I}_{B_{k,0}^{\alpha}}(x) \end{equation*} |
where B_{k, 0}^{\alpha} = \{ x\in \Omega : 0\le \vert u_k^{\alpha}(x)\vert < L_0\} , we have
| \begin{equation} \begin{split} &\int_{\Omega} \sum\limits_{\alpha = 1}^N f^{\alpha }(x)\psi^{\alpha}(x)dx = \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i, j = 1}^n {\tilde a}_{i,j,k}^{\alpha, \beta}(x, u_k(x))D_ju_k^{\beta}(x) D_i\psi^{\alpha}(x)dx = \\ & = \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i, j = 1}^n \big( a_{i,j}^{\alpha, \beta} (x, u_k(x)) +\frac{1}{k} \delta_{\alpha, \beta}\delta_{i,j} \big)D_ju_k^{\beta}(x) D_iu_k^{\alpha}(x) \mathbb{I}_{B_{k,0}^{\alpha}} dx = \\ & = \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i, j = 1}^n a_{i,j}^{\alpha, \beta} (x, u_k(x)) D_ju_k^{\beta}(x) D_iu_k^{\alpha}(x) \mathbb{I}_{B_{k,0}^{\alpha}} dx+ \\ &+ \int_{\Omega} \sum\limits_{\alpha = 1}^N \sum\limits_{i = 1}^n \frac{1}{k}\vert D_iu_k^{\alpha}(x)\vert^2 \, \mathbb{I}_{B_{k,0}^{\alpha}}dx\ge\\ &\ge \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i, j = 1}^n a_{i,j}^{\alpha, \beta} (x, u_k(x)) D_ju_k^{\beta}(x) D_iu_k^{\alpha}(x) \mathbb{I}_{B_{k,0}^{\alpha}} dx.\\ \end{split} \end{equation} | (3.30) |
From (\mathcal A'_3) , we get
| \begin{equation} a_{i,j}^{\alpha, \beta} (x, u_k(x)) \mathbb{I}_{B_{k,0}^{\alpha}} = a_{i,j}^{\alpha, \beta} (x, u_k(x)) \mathbb{I}_{B_{k,0}^{\alpha}}(x) \mathbb{I}_{B_{k,0}^{\beta}}(x). \end{equation} | (3.31) |
Combining (3.30), (3.31) and (\mathcal A_2) , we deduce that
| \begin{equation} \begin{split} &\int_{\Omega} \sum\limits_{\alpha = 1}^N f^{\alpha }(x)\psi^{\alpha}(x)dx\ge \\ & \ge \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i, j = 1}^n a_{i,j}^{\alpha, \beta} (x, u_k(x)) D_ju_k^{\beta}(x) D_iu_k^{\alpha}(x) \mathbb{I}_{B_{k,0}^{\alpha}} dx = \\ & = \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i, j = 1}^n a_{i,j}^{\alpha, \beta} (x, u_k(x)) D_ju_k^{\beta}(x) \mathbb{I}_{B_{k,0}^{\beta}}(x) D_iu_k^{\alpha }(x) \mathbb{I}_{B_{k,0}^{\alpha}}(x) dx\ge\\ & \ge \int_{\Omega} \nu \sum\limits_{\alpha = 1}^N \frac{\vert Du_k^{\alpha}(x)\vert^2\mathbb{I}_{B_{k,0}^{\alpha}}(x)} {(1+\vert u_k^{\alpha}(x) \vert )^{\theta}} dx\ge \frac {\nu}{(1+L_0)^{\theta}} \sum\limits_{\alpha = 1}^N \int_{B_{k,0}^{\alpha}}\vert Du_k^{\alpha} \vert^2 dx.\\ \end{split} \end{equation} | (3.32) |
From (3.32) and (2.3), it follows that
| \begin{equation} \begin{split} & \sum\limits_{\alpha = 1}^N \int_{B_{k,0}^{\alpha}}\vert Du_k^{\alpha} \vert^2 dx\le \frac{(1+L_0)^{\theta}} {\nu} \int_{\Omega} \sum\limits_{\alpha = 1}^N f^{\alpha }(x)\psi^{\alpha}(x)dx \le \\ &\le \frac{(1+L_0)^{\theta}L_0 } {\nu} \sum\limits_{\alpha = 1}^N \int_{\Omega}\vert f^{\alpha}(x) \vert dx\le \\ &\le \frac{(1+L_0)^{\theta}L_0 } {\nu} \sum\limits_{\alpha = 1}^NM_m(f,\Omega) \vert \Omega \vert^{1-\frac{1}{m}} = \\ & = \frac{(1+L_0)^{\theta}L_0 N} {\nu} M_m(f,\Omega)\vert \Omega \vert^{1-\frac{1}{m}}.\\ \end{split} \end{equation} | (3.33) |
Therefore, combining (3.27), (3.29) and (3.33), we have
| \begin{equation*} \begin{split} & \sum\limits_{\alpha = 1}^N \int_{\Omega} \vert Du_k^{\alpha}(x)\vert^2dx\le\\ &\le \sum\limits_{\alpha = 1}^N \int_{\{ \vert u_k^{\alpha}\vert < L_0 \}}\vert Du_k^{\alpha}(x) \vert^2dx+\sum\limits_{t = 0}^{+\infty} \frac{(1+2^{t+1}L_0)^{\theta}}{\nu} \sum\limits_{\alpha = 1}^N\int_{A_{k,2^tL_0}^{\alpha}} f^{\alpha}(x)\varphi_t^{\alpha}(x)dx \le\\ &\le C_7+ \frac{(1+L_0)^{\theta}L_0 N} {\nu} M_m(f,\Omega)\vert \Omega \vert^{1-\frac{1}{m}}: = C_8, \end{split} \end{equation*} |
where C_8 is a positive constant depending only on n, N, m, \theta, \nu, M_m(f, \Omega), \vert \Omega \vert, C_S, L_0, and the boundedness of u_k in W_0^{1, 2}(\Omega, \mathbb{R^N}) is proved.
Proof of Theorem 2.1
Proof. Let u_k be a solution of (\tilde {P}_k) . Lemma 3.1 states that the sequence of \{u_k \} is uniformly bounded in M^r(\Omega, \mathbb{R}^N) and in W^{1, 2}_0(\Omega, \mathbb{R}^N) . Then there exists a positive constant C such that M_r(u_k, \Omega) \leq C and \|u_k\|_{W^{1, 2}_0(\Omega, \mathbb{R}^N)} \leq C for all k \in \mathbb{N} . Being \{u_k \} bounded in W^{1, 2}_0(\Omega, \mathbb{R}^N) there exists a subsequence \{u_{k_{\lambda}} \} weakly converging in W^{1, 2}_0(\Omega, \mathbb{R}^N) to a function u \in W^{1, 2}_0(\Omega, \mathbb{R}^N) . Moreover, by Rellich-Kondrachov embedding Theorem, Sobolev space W_0^{1, 2}(\Omega, \mathbb{R}^N) is compactly embedded in L^2(\Omega, \mathbb{R}^N) ; then, there exists a subsequence, not relabeled, also in the sequel, strongly converging to u in L^{2} . From L^2 convergence we get pointwise convergence almost everywhere, up to a further subsequence. Briefly we write
| \begin{align} & u_{k_{\lambda}} \rightharpoonup u \quad {\rm{ in }}\; W_0^{1,2}(\Omega), \\ & u_{k_{\lambda}} \to u \quad {\rm{ in }}\; L^2(\Omega), \\ & u_{k_{\lambda}} (x) \to u(x) \quad {\rm{ almost\; everywhere\; in }}\; \Omega, \\ & M_r(u_k, \Omega) \leq C, \quad \| u_{k_{\lambda}}\|_{W^{1,2}_0(\Omega, \mathbb{R}^N)} \leq C. \end{align} | (3.34) |
Now, we pass to the limit as \lambda \rightarrow +\infty , in the weak formulation of problem (\tilde {P}_k) , written when k = k_{\lambda} , to prove that u solves problem (2.6). More precisely, we verify that for all \varphi \in W_0^{1, 2}(\Omega, \mathbb{R}^N)
| \begin{equation*} \begin{split} \lim\limits_{\lambda \to +\infty} \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n \tilde{a}_{i,j,k_{\lambda}}^{\alpha, \beta} (x, u_{k_{\lambda}}(x)) D_j u_{k_{\lambda}}^\beta (x) D_i \varphi^\alpha (x) dx = \\ = \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n a_{i,j}^{\alpha, \beta} (x, u(x)) D_j u^\beta (x) D_i \varphi^\alpha (x) dx. \end{split} \end{equation*} |
To this aim, we estimate
| \begin{align*} & \left| \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n \tilde{a}_{i,j,k_{\lambda}}^{\alpha, \beta} (x, u_{k_{\lambda}}(x)) D_j u_{k_{\lambda}}^\beta (x) D_i \varphi^\alpha (x) dx + \right. \\ & \quad \left. - \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n a_{i,j}^{\alpha, \beta} (x, u(x)) D_j u^\beta (x) D_i \varphi^\alpha (x) dx \right| \leq \\ & \leq \left| \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n [{a}_{i,j}^{\alpha, \beta} (x, u_{k_{\lambda}}(x)) - a_{i,j}^{\alpha, \beta}(x, u(x))] D_j u_{k_{\lambda}}^\beta (x) D_i \varphi^\alpha (x) dx \right| + \\ & \quad + \left| \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n a_{i,j}^{\alpha, \beta} (x, u(x)) [D_j u_{k_{\lambda}}^{\beta} (x)- D_j u^\beta (x)] D_i \varphi^\alpha (x) dx \right| + \\ & \quad +\left| \int_{\Omega} \sum\limits_{\alpha = 1}^N \sum\limits_{i = 1}^n \frac{1}{k_{\lambda}} D_i u_{k_{\lambda}}^\alpha (x) D_i \varphi^\alpha (x) dx \right| : = \\ & = I_{k_{\lambda}} +II_{k_{\lambda}} +III_{k_{\lambda}}. \end{align*} |
We obtain the result by proving that I_{k_{\lambda}}, II_{k_{\lambda}}, III_{k_{\lambda}} tend to zero as \lambda \rightarrow +\infty. We start to estimate I_{k_{\lambda}}. Using Hölder inequality and boundedness of the sequence \{u_{k_{\lambda}}\} in W^{1, 2}_0(\Omega) we have
| \begin{equation} \begin{split} &I_{k_{\lambda}} = \left| \int_{\Omega} \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n [{a}_{i,j}^{\alpha, \beta} (x, u_{k_{\lambda}}(x)) - a_{i,j}^{\alpha, \beta}(x, u(x))] D_j u_{k_{\lambda}}^\beta (x) D_i \varphi^\alpha (x) dx \right| \leq \\ & \leq \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n \left( \int_{\Omega} |{a}_{i,j}^{\alpha, \beta} (x, u_{k_{\lambda}}(x)) - a_{i,j}^{\alpha, \beta}(x, u(x))|^2 | D_i \varphi^\alpha (x)|^2 dx \right)^{\frac{1}{2}} \| D_j u_{k_{\lambda}}^\beta\|_{L^2} \leq \\ & \leq C \sum\limits_{\alpha, \beta = 1}^N \sum\limits_{i,j = 1}^n \left( \int_{\Omega} |{a}_{i,j}^{\alpha, \beta} (x, u_{k_{\lambda}}(x)) - a_{i,j}^{\alpha, \beta}(x, u(x))|^2 | D_i \varphi^\alpha (x)|^2 dx \right)^{\frac{1}{2}}. \end{split} \end{equation} | (3.35) |
For any i, j = 1, \ldots, n and for any \alpha, \beta = 1, \ldots, N , using pointwise convergence in (3.34) and continuity of functions y \to a_{i, j}^{\alpha, \beta}(x, y) we have that
| \begin{equation*} |{a}_{i,j}^{\alpha, \beta} (x, u_{k_{\lambda}}(x)) - a_{i,j}^{\alpha, \beta}(x, u(x))|^2 | D_i \varphi^\alpha (x)|^2 \to 0 \quad {\rm{ as }}\; \lambda \to +\infty; \end{equation*} |
moreover from (\mathcal{A}_1) we get
| \begin{equation*} \begin{split} & |{a}_{i,j}^{\alpha, \beta} (x, u_{k_{\lambda}}(x)) - a_{i,j}^{\alpha, \beta}(x, u(x))|^2 | D_i \varphi^\alpha (x)|^2 \leq \\ & \leq (|{a}_{i,j}^{\alpha, \beta} (x, u_{k_{\lambda}}(x)) |+ |a_{i,j}^{\alpha, \beta}(x, u(x))|)^2 | D_i \varphi^\alpha (x)|^2 \leq \\ & \leq (c+c)^2 | D_i \varphi^\alpha (x)|^2 \in L^1(\Omega); \end{split} \end{equation*} |
therefore, by dominated convergence theorem, we obtain that
| \begin{equation*} \left( \int_{\Omega} |{a}_{i,j}^{\alpha, \beta} (x, u_{k_{\lambda}}(x)) - a_{i,j}^{\alpha, \beta}(x, u(x))|^2 | D_i \varphi^\alpha (x)|^2 dx \right)^{\frac{1}{2}} \to 0 \quad {\rm{ as }}\; \lambda \to +\infty. \end{equation*} |
The above limit and (3.35) imply that I_{k_{\lambda}} tends to zero as \lambda \to +\infty .
Observing that u_{k_{\lambda}}^{\beta} \rightharpoonup u^{\beta} in W^{1, 2}_0(\Omega) , for any i, j = 1, \ldots, n and for any \alpha, \beta = 1, \ldots, N , we have
| \begin{equation*} \int_{\Omega} a_{i,j}^{\alpha, \beta} (x, u(x)) [D_j u_{k_{\lambda}}^{\beta} (x)- D_j u^\beta (x)] D_i \varphi^\alpha (x) dx \to 0 \quad {\rm{ as }}\; \lambda \to +\infty , \end{equation*} |
hence II_{k_{\lambda}} tends to zero as \lambda \to +\infty .
Using Hölder inequality and (3.34), it results that
| \begin{equation*} \begin{split} &III_{k_{\lambda}} = \left| \int_{\Omega} \sum\limits_{\alpha = 1}^N \sum\limits_{i = 1}^n \frac{1}{k_{\lambda}} D_i u_{k_{\lambda}}^\alpha (x) D_i \varphi^\alpha (x) dx \right| \leq \frac{1}{k_{\lambda}} \sum\limits_{\alpha = 1}^N \sum\limits_{i = 1}^n \| D_i u_{k_{\lambda}}^\alpha \|_{L^2} \| D_i \varphi^\alpha \|_{L^2} \leq \\ & \leq \frac{1}{k_{\lambda}} n N C \|\varphi\|_{W^{1,2}_0(\Omega, R^N)}. \end{split} \end{equation*} |
Passing to the limit as \lambda \rightarrow +\infty , we obtain that III_{k_{\lambda}} tends to zero and the proof is completed.
We acknowledge the support of GNAMPA, INdAM, MIUR, UNⅣAQ. S. Leonardi has been supported by Piano della Ricerca di Ateneo 2020-2022–PIACERI: Project MO.S.A.I.C. "Monitoraggio satellitare, modellazioni matematiche e soluzioni architettoniche e urbane per lo studio, la previsione e la mitigazione delle isole di calore urbano", Project EEEP & DLaD. S. Leonardi is member of the Gruppo Nazionale per l'Analisi Matematica, la Probabilità e le loro Applicazioni (GNAMPA) of the Istituto Nazionale di Alta Matematica (INdAM), codice CUP_E55F22000270001.
The authors declare no conflict of interest.
| [1] | S. Aouaoui, Solutions to quasilinear equations of N-biharmonic type with degenerate coercivity, Electronic Journal of Differential Equations, 2014 (2014), 228. |
| [2] |
A. Alvino, L. Boccardo, V. Ferone, L. Orsina, G. Trombetti, Existence results for nonlinear elliptic equations with degenerate coercivity, Annali di Matematica, 182 (2003), 53–79. https://doi.org/10.1007/s10231-002-0056-y doi: 10.1007/s10231-002-0056-y

|
| [3] | A. Alvino, V. Ferone, G. Trombetti, A priori estimates for a class of non uniformly elliptic equations, Atti Sem. Mat. Fis. Univ. Modena, 46 (1998), 381–391. |
| [4] | H. Ayadi, F. Mokhtari, Nonlinear anisotropic elliptic equations with variable exponents and degenerate coercivity, Electronic Journal of Differential Equations, 2018 (2018), 45. |
| [5] |
P. Baroni, M. Colombo, G. Mingione, Regularity for general functionals with double phase, Calc. Var., 57 (2018), 62. https://doi.org/10.1007/s00526-018-1332-z doi: 10.1007/s00526-018-1332-z

|
| [6] |
L. Beck, G. Mingione, Lipschitz bounds and nonuniform ellipticity, Commun. Pure Appl. Math., 73 (2020), 944–1034, https://doi.org/10.1002/cpa.21880 doi: 10.1002/cpa.21880

|
| [7] | L. Boccardo, Quasilinear elliptic equations with natural growth terms: the regularizing effect of the lower order terms, J. Nonlinear Convex Anal., 7 (2006), 355–365. |
| [8] |
L. Boccardo, Marcinkiewicz estimates for solutions of some elliptic problems with nonregular data, Annali di Matematica, 188 (2009), 591–601. https://doi.org/10.1007/s10231-008-0090-5 doi: 10.1007/s10231-008-0090-5

|
| [9] | L. Boccardo, H. Brézis, Some remarks on a class of elliptic equations with degenerate coercivity, Boll. Unione Mat. Ital., 6 (2003), 521–530. |
| [10] | L. Boccardo, G. Croce, Elliptic partial differential equations: Existence and regularity of distributional solutions, Berlin: De Gruyter, 2013. https://doi.org/10.1515/9783110315424 |
| [11] |
L. Boccardo, G. Croce, L. Orsina, Existence of solutions for some noncoercive elliptic problems involving derivatives of nonlinear terms, Differential Equations & Applications, 4 (2012), 3–9. https://doi.org/10.7153/dea-04-02 doi: 10.7153/dea-04-02

|
| [12] |
L. Boccardo, G. Croce, L. Orsina, Nonlinear degenerate elliptic problems with W^{1, 1}_0(\Omega) solutions, Manuscripta Math., 137 (2012), 419–439. https://doi.org/10.1007/s00229-011-0473-6 doi: 10.1007/s00229-011-0473-6

|
| [13] | L. Boccardo, G. Croce, C. Tanteri, An elliptic system with degenerate coercivity, Rend. Mat. Appl. (7), 36 (2015), 1–9. |
| [14] | L. Boccardo, A. Dall'Aglio, L. Orsina, Existence and regularity results for some elliptic equations with degenerate coercivity, Atti Sem. Mat. Fis. Univ. Modena, 46 (1998), 51–81. |
| [15] |
P. Cianci, G. R. Cirmi, S. D'Asero, S. Leonardi, Morrey estimates for solutions of singular quadratic nonlinear equations, Annali di Matematica, 196 (2017), 1739–1758. https://doi.org/10.1007/s10231-017-0636-5 doi: 10.1007/s10231-017-0636-5

|
| [16] |
G. R. Cirmi, S. D'Asero, S. Leonardi, Fourth-order nonlinear elliptic equations with lower order term and natural growth conditions, Nonlinear Anal. Theor., 108 (2014), 66–86. https://doi.org/10.1016/j.na.2014.05.014 doi: 10.1016/j.na.2014.05.014

|
| [17] |
G. R. Cirmi, S. D'Asero, S. Leonardi, Morrey estimates for a class of elliptic equations with drift term, Adv. Nonlinear Anal., 9 (2020), 1333–1350. https://doi.org/10.1515/anona-2020-0055 doi: 10.1515/anona-2020-0055

|
| [18] |
G. R. Cirmi, S. D'Asero, S. Leonardi, On the existence of weak solutions to a class of nonlinear elliptic systems with drift term, J. Math. Anal. Appl., 491 (2020), 124370. https://doi.org/10.1016/j.jmaa.2020.124370 doi: 10.1016/j.jmaa.2020.124370

|
| [19] |
G. R. Cirmi, S. D'Asero, S. Leonardi, Morrey estimates for a class of noncoercive elliptic systems with VMO-coefficients, Atti Accad. Naz. Lincei Rend. Lincei Mat. Appl., 32 (2021), 317–334. https://doi.org/10.4171/RLM/938 doi: 10.4171/RLM/938

|
| [20] |
G. R. Cirmi, S. D'Asero, S. Leonardi, M. M. Porzio, Local regularity results for solutions of linear elliptic equations with drift term, Adv. Calc. Var., 15 (2022), 19–32. https://doi.org/10.1515/acv-2019-0048 doi: 10.1515/acv-2019-0048

|
| [21] |
G. R. Cirmi, S. Leonardi, Regularity results for the gradient of solutions linear elliptic equations with L^{1, \lambda} data, Annali di Matematica, 185 (2006), 537–553. http://doi.org/10.1007/s10231-005-0167-3 doi: 10.1007/s10231-005-0167-3

|
| [22] |
G. R. Cirmi, S. Leonardi, Regularity results for solutions of nonlinear elliptic equations with L^{1, \lambda} data, Nonlinear Anal. Theor., 69 (2008), 230–244. https://doi.org/10.1016/j.na.2007.05.014 doi: 10.1016/j.na.2007.05.014

|
| [23] |
G. R. Cirmi, S. Leonardi, Higher differentiability for the solutions of nonlinear elliptic systems with lower-order terms and L^{1, \theta}-data, Annali di Matematica, 193 (2014), 115–131. https://doi.org/10.1007/s10231-012-0269-7 doi: 10.1007/s10231-012-0269-7

|
| [24] |
G. Croce, An elliptic problem with degenerate coercivity and a singular quadratic gradient lower order term, Discrete Contin. Dyn. Syst. S, 5 (2012), 507–530. https://doi.org/10.3934/dcdss.2012.5.507 doi: 10.3934/dcdss.2012.5.507

|
| [25] | G. Croce, The regularizing effects of some lower order terms in an elliptic equation with degenerate coercivity, Rendiconti di Matematica, Serie Ⅶ, 27 (2007), 299–314. |
| [26] |
G. Cupini, F. Giannetti, R. Giova, A. Passarelli di Napoli, Regularity results for vectorial minimizers of a class of degenerate convex integrals, J. Differ. Equations, 265 (2018), 4375–4416. https://doi.org/10.1016/j.jde.2018.06.010 doi: 10.1016/j.jde.2018.06.010

|
| [27] |
G. Cupini, P. Marcellini, E. Mascolo, Regularity of minimizers under limit growth conditions, Nonlinear Anal. Theor., 153 (2017), 294–310. https://doi.org/10.1016/j.na.2016.06.002 doi: 10.1016/j.na.2016.06.002

|
| [28] |
C. De Filippis, G. Mingione, Lipschitz bounds and nonautonomous integrals, Arch. Rational Mech. Anal., 242 (2021), 973–1057. https://doi.org/10.1007/s00205-021-01698-5 doi: 10.1007/s00205-021-01698-5

|
| [29] | E. De Giorgi, Un esempio di estremali discontinue per un problema variazionale di tipo ellittico, Boll. Un. Mat. Ital. Ser. Ⅳ, 1 (1968), 135–137. |
| [30] |
F. Della Pietra, G. Di Blasio, Comparison, existence and regularity results for a class of non-uniformly elliptic equations, Differential Equations & Applications, 2 (2010), 79–103. https://doi.org/10.7153/dea-02-07 doi: 10.7153/dea-02-07

|
| [31] | P. Di Gironimo, F. Leonetti, M. Macrì, P. V. Petricca, Existence of bounded solutions for some quasilinear degenerate elliptic systems, Minimax Theory and its Applications, 6 (2021), 321–340. |
| [32] | P. Di Gironimo, F. Leonetti, M. Macrì, P. V. Petricca, Existence of solutions to some quasilinear degenerate elliptic systems when the datum has an intermediate degree of integrability, Complex Var. Elliptic Equ., in press. https://doi.org/10.1080/17476933.2022.2069753 |
| [33] |
G. Dolzmann, N. Hungerbuhler, S. Muller, Non-linear elliptic systems with measure-valued right hand side, Math. Z., 226 (1997), 545–574. https://doi.org/10.1007/PL00004354 doi: 10.1007/PL00004354

|
| [34] |
V. Ferone, N. Fusco, VMO solutions of the N-Laplacian with measure data, Comptes Rendus de l'Académie des Sciences Series I-Mathematics, 325 (1997), 365–370. https://doi.org/10.1016/S0764-4442(97)85618-2 doi: 10.1016/S0764-4442(97)85618-2

|
| [35] |
H. Gao, M. Huang, W. Ren, Regularity for entropy solutions to degenerate elliptic equations, J. Math. Anal. Appl., 491 (2020), 124251. https://doi.org/10.1016/j.jmaa.2020.124251 doi: 10.1016/j.jmaa.2020.124251

|
| [36] |
H. Gao, F. Leonetti, W. Ren, Regularity for anisotropic elliptic equations with degenerate coercivity, Nonlinear Anal., 187 (2019), 493–505. https://doi.org/10.1016/j.na.2019.06.017 doi: 10.1016/j.na.2019.06.017

|
| [37] |
D. Giachetti, M. M. Porzio, Existence results for some nonuniformly elliptic equations with irregular data, J. Math. Anal. Appl., 257 (2001), 100–130. https://doi.org/10.1006/jmaa.2000.7324 doi: 10.1006/jmaa.2000.7324

|
| [38] |
D. Giachetti, M. M. Porzio, Elliptic equations with degenerate coercivity: gradient regularity, Acta Math. Sinica, 19 (2003), 349–370. https://doi.org/10.1007/s10114-002-0235-1 doi: 10.1007/s10114-002-0235-1

|
| [39] | W. Hao, S. Leonardi, J. Nečas, An example of irregular solution to a nonlinear Euler-Lagrange elliptic system with real analytic coefficients, Annali della Scuola Normale Superiore di Pisa (Ⅳ), 23 (1996), 57–67. |
| [40] | W. Hao, S. Leonardi, M. Steinhauer, Examples of discontinuous, divergence-free solutions to elliptic variational problems, Comment. Math. Univ. Carolin., 36 (1995), 511–517. |
| [41] |
J. Kristensen, G. Mingione, The singular set of \omega-minima, Arch. Rational Mech. Anal., 177 (2005), 93–114. https://doi.org/10.1007/s00205-005-0361-x doi: 10.1007/s00205-005-0361-x

|
| [42] |
J. Kristensen, G. Mingione, The singular set of minima of integral functionals, Arch. Rational Mech. Anal., 180 (2006), 331–398. https://doi.org/10.1007/s00205-005-0402-5 doi: 10.1007/s00205-005-0402-5

|
| [43] |
J. Kristensen, G. Mingione, Boundary regularity in variational problems, Arch. Rational Mech. Anal., 198 (2010), 369–455. https://doi.org/10.1007/s00205-010-0294-x doi: 10.1007/s00205-010-0294-x

|
| [44] |
T. Kuusi, G. Mingione, Universal potential estimates, J. Funct. Anal., 262 (2012), 4205–4269. https://doi.org/10.1016/j.jfa.2012.02.018 doi: 10.1016/j.jfa.2012.02.018

|
| [45] |
T. Kuusi, G. Mingione, Linear potentials in nonlinear potential theory, Arch. Rational Mech. Anal., 207 (2013), 215–246. https://doi.org/10.1007/s00205-012-0562-z doi: 10.1007/s00205-012-0562-z

|
| [46] |
T. Kuusi, G. Mingione, Guide to nonlinear potential estimates, Bull. Math. Sci., 4 (2014), 1–82. https://doi.org/10.1007/s13373-013-0048-9 doi: 10.1007/s13373-013-0048-9

|
| [47] |
T. Kuusi, G. Mingione, The Wolff gradient bound for degenerate parabolic equations, J. Eur. Math. Soc., 16 (2014), 835–892. https://doi.org/10.4171/JEMS/449 doi: 10.4171/JEMS/449

|
| [48] |
T. Kuusi, G. Mingione, Vectorial nonlinear potential theory, J. Eur. Math. Soc., 20 (2018), 929–1004. https://doi.org/10.4171/JEMS/780 doi: 10.4171/JEMS/780

|
| [49] |
S. Leonardi, On constants of some regularity theorems. De Giorgi's type counterexample, Math. Nachr., 192 (1998), 191–204. https://doi.org/10.1002/mana.19981920111 doi: 10.1002/mana.19981920111

|
| [50] |
S. Leonardi, Gradient estimates below duality exponent for a class of linear elliptic systems, Nonlinear Differ. Equ. Appl., 18 (2011), 237–254. http://doi.org/10.1007/s00030-010-0093-y doi: 10.1007/s00030-010-0093-y

|
| [51] |
S. Leonardi, Morrey estimates for some classes of elliptic equations with a lower order term, Nonlinear Anal., 177 (2018), 611–627. https://doi.org/10.1016/j.na.2018.05.010 doi: 10.1016/j.na.2018.05.010

|
| [52] |
S. Leonardi, F. Leonetti, C. Pignotti, E. Rocha, V. Staicu, Maximum principles for some quasilinear elliptic systems, Nonlinear Anal., 194 (2020), 111377. https://doi.org/10.1016/j.na.2018.11.004 doi: 10.1016/j.na.2018.11.004

|
| [53] | S. Leonardi, F. Leonetti, C. Pignotti, E. Rocha, V. Staicu, Local boundedness for weak solutions to some quasilinear elliptic systems, Minimax Theory and its Applications, 6 (2021), 365–378. |
| [54] |
S. Leonardi, F. Leonetti, E. Rocha, V. Staicu, Butterfly support for off diagonal coefficients and boundedness of solutions to quasilinear elliptic systems, Adv. Nonlinear Anal., 11 (2022), 672–683. https://doi.org/10.1515/anona-2021-0205 doi: 10.1515/anona-2021-0205

|
| [55] |
F. Leonetti, P. V. Petricca, Existence of bounded solutions to some nonlinear degenerate elliptic systems, Discrete Contin. Dyn. Syst. B, 11 (2009), 191–203. https://doi.org/10.3934/dcdsb.2009.11.191 doi: 10.3934/dcdsb.2009.11.191

|
| [56] |
F. Leonetti, E. Rocha, V. Staicu, Quasilinear elliptic systems with measure data, Nonlinear Anal. Theor., 154 (2017), 210–224. https://doi.org/10.1016/j.na.2016.04.002 doi: 10.1016/j.na.2016.04.002

|
| [57] |
F. Leonetti, E. Rocha, V. Staicu, Smallness and cancellation in some elliptic systems with measure data, J. Math. Anal. Appl., 465 (2018), 885–902. https://doi.org/10.1016/j.jmaa.2018.05.047 doi: 10.1016/j.jmaa.2018.05.047

|
| [58] |
F. Leonetti, R. Schianchi, A remark on some degenerate elliptic problems, Ann. Univ. Ferrara, 44 (1998), 123–128. https://doi.org/10.1007/BF02828019 doi: 10.1007/BF02828019

|
| [59] |
J. Leray, J. L. Lions, Quelques résultats de Višik sur les problèmes elliptiques non linéaires par les méthodes de Minty-Browder, Bulletin de la Société Mathématique de France, 93 (1965), 97–107. https://doi.org/10.24033/bsmf.1617 doi: 10.24033/bsmf.1617

|
| [60] |
V. G. Maz'ja, Examples of nonregular solutions of quasilinear elliptic equations with analytic coefficients, Funct. Anal. Appl., 2 (1968), 230–234. https://doi.org/10.1007/BF01076124 doi: 10.1007/BF01076124

|
| [61] |
G. Mingione, Regularity of minima: An invitation to the dark side of the calculus of variations, Appl. Math., 51 (2006), 355–426. https://doi.org/10.1007/s10778-006-0110-3 doi: 10.1007/s10778-006-0110-3

|
| [62] |
G. Mingione, The Calder\acute{o}n-Zygmund theory for elliptic problems with measure data, Ann. Scuola Norm. Sup. Pisa Cl. Sci. (5), 6 (2007), 195–261. https://doi.org/10.2422/2036-2145.2007.2.01 doi: 10.2422/2036-2145.2007.2.01

|
| [63] |
G. Mingione, Gradient estimates below the duality exponent, Math. Ann., 346 (2010), 571–627. https://doi.org/10.1007/s00208-009-0411-z doi: 10.1007/s00208-009-0411-z

|
| [64] |
G. Mingione, Gradient potential estimates, J. Eur. Math. Soc., 13 (2011), 459–486. https://doi.org/10.4171/JEMS/258 doi: 10.4171/JEMS/258

|
| [65] |
G. Mingione, G. Palatucci, Developments and perspectives in nonlinear potential theory, Nonlinear Anal., 194 (2020), 111452. https://doi.org/10.1016/j.na.2019.02.006 doi: 10.1016/j.na.2019.02.006

|
| [66] | J. Nečas, J. Stará, Principio di massimo per i sistemi ellittici quasi-lineari non diagonali, Boll. Un. Mat. Ital. Ser. Ⅳ, 6 (1972), 1–10. |
| [67] | A. Porretta, Uniqueness and homogenization for a class of non coercive operators in divergence form, Atti Sem. Mat. Fis. Univ. Modena, 46 (1998), 915–936. |
| [68] | E. Stein, G. Weiss, Introduction to Fourier analysis on Euclidean spaces, Princeton University Press, 1971. |
| [69] | C. Trombetti, Existence and regularity for a class of non-uniformly elliptic equations in two dimensions, Differential Integral Equations, 13 (2000), 687–706. |
| [70] | Z. Q. Yan, Everywhere regularity for solutions to quasilinear elliptic systems of triangular form, In: Partial differential equations, Berlin: Springer, 1988,255–261. https://doi.org/10.1007/BFb0082938 |
| [71] | S. Zhou, A note on nonlinear elliptic systems involving measures, Electronic Journal of Differential Equations, 2000 (2000), 08. |
| 1. | Patrizia Di Gironimo, Francesco Leonetti, Marta Macrì, Existence and regularity for solutions of quasilinear degenerate elliptic systems, 2024, 245, 0362546X, 113562, 10.1016/j.na.2024.113562 | |
| 2. | Patrizia Di Gironimo, Salvatore Leonardi, Francesco Leonetti, Marta Macrì, Existence of distributional solutions to some quasilinear degenerate elliptic systems with low integrability of the datum, 2025, 1864-8258, 10.1515/acv-2023-0065 |