1.
Introduction
In this note we study existence of positive radial solutions of fully nonlinear elliptic partial differential equations in the form
where p>1, a>−1, 0<λ≤Λ, and Ω is either an annulus or an exterior domain in RN for N≥3. Additionally, we suppose ˜N+>2 in the case of M+λ,Λ, where ˜N± are the dimension-like numbers
Here M±λ,Λ are the Pucci's extremal operators, which play an essential role in stochastic control theory and mean field games. We deal with classical solutions of (1.1) that are C2 in Ω.
In [9] nonexistence results in exterior domains for weighted equations as in (1.1) via a dynamical system approach were established. However, proving the existence of such solutions, as well as solutions in annuli, is a difficult task in terms of that approach, since the orbits in the flow there present blow-up discontinuities. Our goal in this work is to complement the analysis in [9], by showing existence of radial solutions in exterior domains and annuli.
We mention that the analysis of the associated ODE problem for proving existence of annular or exterior domain solutions has been performed in many papers in semilinear cases [1,8,11].
In the case when a=0, results of this nature were obtained in [5,6]. The analysis in [5] was performed in light of the change of variables in [4]. In [9] we have already noticed that employing quadratic dynamical systems is effective to deal with these problems, in a simple and unified way. Here, our shooting arguments to obtain existence of annular solutions are inspired by those in [6]. On the other hand, in what concerns the existence in exterior domains we introduce an alternative dynamical system, which is different from those in [5] and [9], of quadratic type which do not present blow-up discontinuities.
For solutions in annuli, our main result reads as follows.
Theorem 1.1. For any p>1, and 0<a<b<+∞, problem (1.1) has a positive radial solution in the annulus
Note that solutions of (1.1) may not be radial in general, for instance the Gidas-Ni-Nirenberg type symmetry result of [3] does not hold for annular domains. Moreover, since v=−u solves M∓λ,Λ(v)+|x|a|u|p−1u in Ω, then Theorem 1.1 also proves the existence of a negative solution for the corresponding problem.
The proof of Theorem 1.1 relies on a careful study of the ODE problem, shooting method and energy arguments. We mention that solutions in annuli can be identified in correspondence with orbits in the dynamical system, but the interior and exterior radii are not explicit in that approach neither can be obtained for an arbitrary annulus via rescaling.
As far as exterior domain solutions are concerned, we obtain the following result. We recall p∗a± are the critical exponents defined in [9] for the operators M±λ,Λ. They are the threshold for the existence and nonexistence of radial positive regular solutions, that is, solutions differentiably defined at r=0.
Theorem 1.2 (Exterior domain). For any p>p∗a± and R>0, by setting Ω=RN∖BR, it holds:
(i) there exists a unique fast decaying solution of (1.1);
(ii) there exist infinitely many solutions of (1.1) with either slow or pseudo-slow decay.
In addition, the ranges where pseudo-slow exterior domain solutions exist are the same where pseudo-slow decay regular solutions exist in [9], see also [12].
The choice of using quadratic systems to treat weighted equations is categorical since the new dynamical system variables do not see the weight, as in [9]. It is worth mentioning that earlier methods employed might be much more involved, meanwhile a simple variable change which eliminates the weight is not available for Pucci operators.
The text is organized as follows. In Section 2 we recall some preliminary tools on radial solutions and shooting method. In Section 3 we write the corresponding quadratic system and use it to prove Theorem 1.2, while Section 4 is devoted to the proof of Theorem 1.1.
2.
Preliminaries
We start by recalling that Pucci's extremal operators M±λ,Λ, for 0<λ≤Λ, are defined as
where A,X are N×N symmetric matrices, and I is the identity matrix. Equivalently, if we denote by {ei}1≤i≤N the eigenvalues of X, we can define the Pucci's operators as
From now on we will drop writing the parameters λ,Λ in the notations for the Pucci's operators.
When u is a radial function, for ease of notation we set u(|x|)=u(r) for r=|x|. If u is C2, the eigenvalues of the Hessian matrix D2u are {u′′,u′(r)r,…,u′(r)r} where u′(r)r is repeated N−1 times.
The Lane-Emden system (1.1) for M+ is written in radial coordinates as
while for M− one has
where M± and m± are the Lipschitz functions
Equations (P+) and (P−) are understood in the maximal interval where u is positive.
Remark 2.1. A positive function u cannot be at the same time convex and increasing in an interval, since u′′(r)<0 as long as u′(r)≥0. In particular, any critical point of a positive solution u is a local strict maximum point for u.
By solution in annulus or exterior domain solution we mean a solution u of (P+) or (P−) defined in an interval [a,ρ), for a∈(0,+∞) and ρ≤+∞, and verifying the Dirichlet condition u(a)=limr→ρ−u(r)=0. We look at the initial value problem
The equations (P+), (P−), together with (2.4) were studied in [5,6].
For any p>1 and for each δ>0, by ODE theory there exists a unique solution u=uδ defined in a maximal interval (a,ρδ) where u is positive, a<ρδ≤+∞.
If ρδ=+∞ we get a positive radial solution in the exterior of the ball Ba. In the second case, a positive solution in the annulus (a,ρδ) is produced. Note that Eq (2.4) is not invariant by rescaling.
Remark 2.2. All results obtained for δ>0 will be also true for δ<0. Indeed, negative shootings for an operator F can be seen as positive shootings for the operator G defined as G(x,X)=−F(x,−X), which is still elliptic and satisfies all the properties we considered so far. In particular, the negative solutions of M+ are positive solutions of M− in the same domain, and viceversa.
Remark 2.3. Let u be a positive radial solution of (2.4) with u=uδ, for some positive constant δ in [a,ρ). Then the rescaled pair
for α as in (2.6), produces a positive solution pair of the same equation in (aγ−1α,ργ−1α) with initial values uγ(aγ−1α)=0, as well as u′γ(aγ−1α)=γ1+1αδ.
Notation. Whenever p>1 and ˜N+>2, we set
3.
The dynamical system and exterior domain solutions
Let u,v be a positive solution pair of (P+) or (P−). Thus we can define the new functions
for t=ln(r), whenever r>0 is such that u>0. The phase space is contained in R2. Throughout the text, we denote the first quadrant as
Since we are studying positive solutions, the points (x(t),z(t)) belong to 1Q when u′<0. Apart from 1Q, we set
that is, 2Q is the region in R2 such that the corresponding u satisfies u′>0. In particular, u′′<0 in 2Q.
As a consequence of this monotonicity, the problems (P+) and (P−) become in 1Q as:
In terms of the functions (3.1), we derive the following autonomous dynamical system, corresponding to (3.2) for M+, where the dot ˙ stands for ddt,
Likewise one has for M−, associated to (3.3),
We stress that (3.4) and (3.5) correspond to positive, decreasing solutions of (P+) and (P−).
On the other hand, given a trajectory τ=(x,z) of (3.4) or (3.5) in 1Q, we define
and then we deduce
Since x∈C1, then u∈C2. Moreover, u satisfies either (P+) or (P−) from the respective equations for ˙x,˙z in the dynamical system.
In other words, (x,z) is a solution of Eq (3.4) or (3.5) in 1Q if and only if u defined by (3.6) is a positive pair solution of (P+) or (P−) with u′<0.
An important role in the study of our problem is played by the following line for M+,
which corresponds to the vanishing of u′′, see also [9]. It allows us to define the following regions
which represent the sets where the function u is concave or convex. More precisely, R+λ is the region of strictly concavity of u, while R−λ is the region of strictly convexity of u.
The respective notations for the operator M− are
At this stage it is worth observing that the systems (3.4) and (3.5) are continuous on ℓ+ and ℓ−, respectively. More than that, the right hand sides are locally Lipschitz functions of x,z, so the usual ODE theory applies. Then one recovers existence, uniqueness, and continuity with respect to initial data as well as continuity with respect to the parameter p.
Since we are considering positive solutions of (1.1), and u′>0 implies u′′<0, one finds out the following ODEs:
Now, in terms of the corresponding dynamical system, we get
On the other hand, for the operator M− one has
Remark 3.1. No stationary points exist in 2Q. Indeed, since u′>0 and u>0 yield u′′<0 then ˙x>0 in 2Q. In other words, we do not have stationary points outside 1Q when we are considering positive solutions u of (1.1).
One may write the dynamical systems (3.4) and (3.5) in terms of the following ODE first order autonomous equation
For instance, in the case of the operator M+, then f,g are given by
We first recall some standard definitions from the theory of dynamical systems.
A stationary point Q of (3.15) is a zero of the vector field (f,g). If σ1 and σ2 are the eigenvalues of the Jacobian matrix (Df(Q),Dg(Q)), then Q is hyperbolic if both σ1,σ2 have nonzero real parts. If this is the case, Q is a source if Re(σ1),Re(σ2)>0, and a sink if Re(σ1),Re(σ2)<0; Q is a saddle point if Re(σ1)<0<Re(σ2).
Next we recall a result on the local stable and unstable manifolds near saddle points of the system (3.15); see [7,theorems 9.29,9.35]. We will see that the usual theory for autonomous planar systems applies since each stationary point Q possesses a neighborhood strictly contained in R−λ in the first quadrant where the function f is C1. In turn, g is always a C1 function.
Sometimes we denote α(τ), i.e., the α-limit of the orbit τ, as the set of limit points of τ(t) as t→−∞. Similarly one defines ω(τ) i.e., the ω-limit of τ at +∞.
We observe that the x axis is invariant by the flow in the sense that z=0 implies ˙z=0. Next, the set where ˙x=0 for the system (3.4), with respect to the operator M+, is given by the parabola
Note that a respective parabola {(x,z):z=λ(N−2)x−λx2} where ˙x=0 on the region R+λ does not exist, since it lies entirely below the concavity line ℓ+, namely
Moreover, the parabola π+1 itself in (3.16) lies below the concavity line ℓ+, so belonging to the region R−λ, that is,
since ˜N+−2<˜N+−1=λΛ(N−1). Analogously, for the operator M−, the parabola
represents the set where ˙x=0, which is contained in the region R−Λ. Also, we define the line
which is the set where ˙z=0 and z>0 for both operators M±.
Lemma 3.2. The stationary points of the dynamical systems (3.4), (3.5), (3.13), and (3.14) are:
where x0=α, and z0=αΛ(˜N+−pα+a)=αΛ(˜N+−2−α);
where x0=α and z0=αλ(˜N–pα+a)=αλ(˜N–2−α).
Proof. The stationary points are given by the intersection of the parabola π±1 with the lines π2 and {(x,z)∈R2:z=0}.
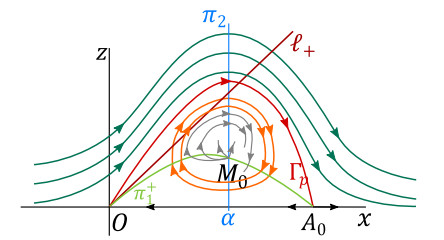
Next we analyze the directions of the vector field F in (3.15) on the x,z axes, on the concavity lines ℓ±, and on the sets π±1 and π2, see Figure 1 below.
Proposition 3.3. The systems (3.4) and (3.5) enjoy the following properties:
(1) Every trajectory of (3.4) in 1Q crosses the line ℓ+ transversely except at the point P=1+ap(1,λ(N−1)). It passes from R+λ to R−λ if x>1+ap, while it moves from R−λ to R+λ if x<1+ap. A similar statement holds for (3.5), via ℓ−, P=1+ap(1,Λ(N−1)), R+Λ, R−Λ;
(2) The vector field at the point P in item (1) is parallel to the line ℓ+ (resp. ℓ−), and an orbit can only reach such a point from R−λ (resp. R−Λ);
(3) The flow induced by (3.15) on the x axis points to the left for x∈(0,˜N±−2), and to the right when x>˜N±−2. On the z axis it always moves up and to the right;
(4) The vector field (f,g) on the parabola π±1 is parallel to the z axis whenever x≠α. It points up if x<α, and down if x>α.
(5) On the line π2 the vector field (f,g) is parallel to the x axis for z≠z0, where z0 is the z-coordinate of M0 in Lemma 3.2. It moves to the left if z<z0, and to the right if z>z0.
Proof. Let us consider the operator M+ since for M− it will be analogous.
(1)–(2) We have ˙x>0 on ℓ+ since π+1 is below the line ℓ+. By the inverse function theorem, t is a function of x on ℓ+, and so is z. In order to detect the transversality, one looks at dzdx on ℓ+ and compare it with the slope of ℓ+. Note that
Then dzdx>λ(N−1) for x<1+ap, while dzdx<λ(N−1) for x>1+ap.
Now we infer that a change of concavity does not happen at x=1+ap. Indeed, if τ=(x,z) is a trajectory and t0 is such that τ(t0)=P, then dzdx(1+ap)=λ(N−1) i.e., d˜zdx(1+ap)=0, where ˜z(x)=z(x)−λ(N−1)x. Then ˜z has a maximum point at x=1+ap, and so τ stays in R−λ in a neighborhood of the point P.
(3) Since the x axis is contained in R−λ then ˙x=x(x−(˜N+−2)) which is positive for x>˜N+−2 and negative when x<˜N+−2. On the other hand, the z axis is contained in R+λ, so ˙x=zλ>0 and ˙z=z(2+a)>0 on x=0 for a>−2.
(4) ˙z=(p−1)z(α−x) on π+1 is positive for x<α, and negative when x>α.
(5) On π2∩R−λ, ˙x=α(α−(˜N+−2))+zΛ is positive for z>z0 and negative when z<z0, for z0 given in Lemma 3.2. On π2∩(R+λ∪ℓ+) we have ˙x≥α(α+1)>0.
The next proposition gives us a study on a local analysis of the stationary points. It follows by the correspondence with the variables (X,Z) in [9], and Propositions 2.2, 2.7, and 2.8, in addition to the appendix in there.
We recall that X:=x and Z(t):=−r1+aup(r)/u′(r), r=et from [9]. Note that z=XZ.
Proposition 3.4 (M±). The following properties are verified for the systems (3.4) and (3.5).
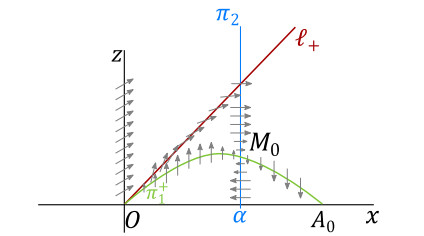
1) For every p>1 the origin O is a saddle point, whose unstable direction is given by
Moreover, there is a unique trajectory coming out from O at −∞ with slope as above, which we denote by Γp, that is, for all p>1, Γp is such that α(Γp)=O.
2) For p>ps,a± the point A0 is a saddle point whose linear stable direction is
where A+=Λ[(˜N+−2)p−(2+a)] for M+, while A−=λ[(˜N–2)p−(2+a)] for M−. Further, there exists a unique trajectory arriving at A0 at +∞ with slope as above, which we denote by Υp i.e., for all p>ps,a±, Υp is such that ω(Υp)=A0.
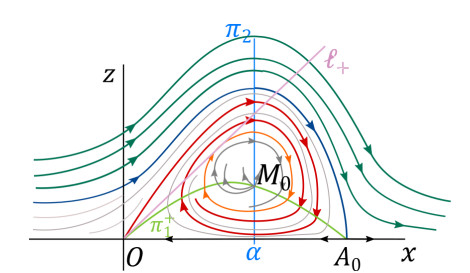
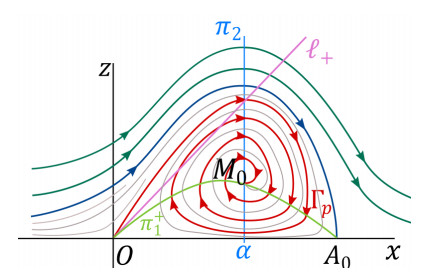
3) At p=ps,a± the point A0 coincides with M0 and belongs to the x axis, while for p<ps,a± the point A0 is a source and M0 belongs to the fourth quadrant. Also, M0∈1Q⇔p>ps,a± in which case: M0 is a source if ps,a±<p<pp,a±; M0 is a sink for p>pp,a±; M0 is a center at p=pp,a±.
The trajectories Γp and Υp uniquely determine the global unstable and stable manifolds of the stationary points O and A0, respectively. They are graphs of functions in a neighborhood of the stationary points in their respective ranges of p. The tangent direction at O is always above the parabola π±1 with ˙z>0, while the tangent direction at A0 is above π±1 with ˙z<0 for all p>ps,a±.
Next we translate the results obtained in [9] into the new variables in what concerns periodic orbits, a priori bounds and blow-ups. We use the one to one correspondence between the orbits in the system (X,Z) in [9] and our (x,z).
Since ˙x>0 on 2Q then periodic orbits may only exist in 1Q. From this, we automatically recover the following proposition from [9]. Recall that pp,a−<paΔ<pp,a+ from (2.6).
Proposition 3.5 (Dulac's criterion). Let λ<Λ. In the case of the operator M+ there are no periodic orbits of (3.4) when 1<p≤paΔ or p>pp,a+. In the case of M− no periodic orbits of (3.5) exist if 1<p<pp,a− or p≥paΔ. In addition, for M+,
(i) there are no periodic orbits strictly contained in the region R+λ∪ℓ+ (resp. R+Λ∪ℓ− for M−), for any p>1;
(ii) periodic orbits contained in R−λ∪ℓ+ (resp. R−Λ∪ℓ−) are admissible only at p=pp,a±. Also, no periodic orbits at pp,a± can cross the concavity line ℓ± twice;
(iii) other limit cycles θ are admissible by the dynamical system as far as they cross ℓ± twice.
By Poincaré-Bendixson theorem, if a trajectory of (3.4) or (3.5) does not converge to a stationary point neither to a periodic orbit, either forward or backward in time, then it necessarily blows up. In the next propositions we prove that a blow up may only occur in finite time. The admissible blow-ups for x in forward time are again in correspondence with X in [9]. However, blow-ups in Z in 1Q from [9] do not occur in our system for z, since a blow-up there occurs at a finite time T where u′(T)=0, R=eT, and for this we have z(T)=R2+aup−1(R)∈(0,+∞).
It remains to characterize the types of blow-up for x in 2Q backward in time.
Lemma 3.6. Any trajectory τ of (3.4)–(3.13) or (3.5)–(3.14) which passes through 2Q, with τ(t)=(x(t),z(t)), is such that x(t)→−∞ and z(t)→0 as t→t1 for some t1∈R. Also, the vector field in 2Q always points to the right and upwards, with ˙x>0 and ˙z>0.
Proof. Let us consider the operator M+. In 2Q one uses the systems (3.13) to write
since x<0 and z>0. Moreover, if x(t0)<0 for some t0∈R, we write ˙xx(x+2−˜N−)≥1 and so
By integrating in the interval [t,t0] we get
and in particular x blows up at the finite time t1=t0+ln(1/c0)˜N–2<t0.
Regular or singular positive solutions of (P+) and (P−) enjoy the monotonicity u′<0 since they belong to ¯1Q. Now we obtain a priori bounds for trajectories of (3.4) or (3.5) defined for all t in intervals of type (ˆt,+∞) or (−∞,ˆt).
Proposition 3.7. Let τ be a trajectory of (3.4) or (3.5) in 1Q, with τ(t)=(x(t),z(t)) defined for all t∈(ˆt,+∞), for some ˆt∈R. Then x(t)∈(0,˜N±−2) for all t≥ˆt. If instead, τ is defined for all t∈(−∞,ˆt), for some ˆt∈R, then
In particular, if a global trajectory is defined for all t∈R in 1Q then it remains inside the box (0,˜N+−2)×(0,λα(N+a)) in the case of M+; it stays in (0,˜N–2)×(0,Λα(N+a)) for M−.
Proof. Since z>0, ˙x≥x(x+2−N)inR+λ and ˙x≥x(x+2−˜N+)inR−λ, the bound for x for a trajectory defined for all forward time is accomplished as in the proof of [9,Proposition 2.11].
Meanwhile, with respect to the bound for z, we first claim that if a trajectory τ intersects the line z=λ(N+a)x then the trajectory τ must cross the z axis. Indeed, in this case Z would attain the value Z=λ(N+a), and so a blow up at a backward time t0∈R in Z would occur by the proof of (2.26) in [9,Proposition 2.11]. Thus, X(t)→0 as t→t+0, from which u′(t0)=0 with u(t0)>0. Thus, x(t0)=0. So the claim is true. Next, we observe that a trajectory defined for all forward time attains a maximum value for z at the line π2, therefore the a priori bound (3.20) for z is verified.
Proof of Theorem 1.2. The existence follows by the existence of trajectories produced by the dynamical system in (x,z), which in turn comes from the dynamical system analysis for (X,Z) in [9] properly glued via the flow in 2Q originated by x,z. More precisely, by [9,Lemma 4.11], for any p>p∗a±, the orbit Υp defined in Proposition 3.4(2) has a blow-up in Z backwards at finite time T, which corresponds to a trajectory in (x,z) which crosses the vertical z axis at T. Then, by Lemma 3.6, Υp has a blow-up in x at t0<T such that x(t)→−∞ as t→t+0. The trajectory Υp corresponds to a fast decaying exterior domain solution of (1.1) in RN∖Br0, with r0=et0.
The remaining slow decaying and pseudo-slow decaying exterior domain solutions come from the trajectories displayed in [9,Figures 5(b) and 6], which are again glued through the z axis by our dynamical system (x,z), analogously.
Even though solutions in annuli can be identified by the trajectories blowing up both in backward (x→−∞) and forward (x→+∞) times in Figures 2–4, the existence of an annular solution for an arbitrary annulus (a,b) is not ensured. Recall that the scaling in Remark 2.3 does not work in this case since it changes both extrema of the annulus. This will be accomplished in the next section, by using the shooting method and energy functions.
4.
Energy analysis and solutions in annuli
In this section we introduce some energy functions and use them to establish existence of solutions in the annulus.
Proposition 4.1. For each δ>0, and uδ solution of (2.4), we set
Then the energy function
is piecewisely monotone decreasing in {u′≠0} whenever ˜N+≥3/2.
Proof. To fix the ideas let δ>0 and the operator M+. For simplicity, we write
where ms is the step function defined through mss=m+(s), for s=u′(r) or s=u′′(r), whenever u′′≠0. Here m+(s) is the Lipschitz function given in (2.2).
Set ˆN−1:=mu′mu′′(N−1) which is either N−1, ˜N+−1 or ˜N–1, whenever u′′≠0. We have σ=Λ≥mu′′ when uu′>0; while σ=λ≤mu′′ when uu′<0. Anyways it yields uu′σ≤uu′mu′′, then
whenever u′′≠0 and u′≠0 and 2(ˆN−1)+a>0. The latter is ensured for instance when a>−1 and ˜N+≥3/2. Note that at a point r0 where u′′(r0)=0 we have u′(r0) and u(r0) with opposite signs since u′ and u cannot be both equal to zero by ODE existence and uniqueness of the initial value problem for Lipschitz nonlinearities.
From the dynamical system we obtain a complete characterization of monotonicity for solutions uδ of (2.4) as follows. Specially in this section we keep the notation in [6] for τ=τδ as a radius (and not for trajectories as in the rest of the text).
Lemma 4.2. For any δ>0 such that uδ is a positive solution of (2.4) in [a,ρ], with ρ=ρδ≤+∞, there exists a unique number τ=τ(δ) with τ∈(a,ρ), such that
Proof. Let us observe that a first critical point exists for u. To see this we look at the dynamical system driven by X,Z. In this case, the behavior at the third quadrant X,Z<0 is given by ˙X>0 and ˙Z<0, with a blow up at finite time T such that u′(eT)=0 by [9,Remark 3.3], and so τ=eT. At such time we have u(τ)>0, and so X(T)=x(T)=0 and z(T)∈(0,+∞). The uniqueness of τ follows by Remark 2.1.
If ρδ=+∞ then limr→∞uδ(r)=0. This comes from the a priori bounds in Proposition 3.7. Thus, for any δ>0 either ρδ=+∞ and limr→∞uδ(r)=0, or there exists some ρδ<+∞ such that u(ρδ)=0. Moreover, by continuous dependence on the initial data, the function δ↦ρδ is continuous in a neighborhood of any δ>0 where ρδ<+∞ whenever p>1.
We shall omit the dependence on the parameter δ>0 whenever it is clear from the context.
Proposition 4.3. For any pair δ>0, and u of (2.4), the energy functions
are monotone increasing, where Eσ is given in (4.1) for σ∈{λ,Λ}.
Proof. Let us consider the operator M+. We recall that in the interval [a,τ] we have u′≥0, u′′≤0, and so
On the other hand, in [τ,ρ] we have u′≤0, and
where (mu′′,ˆN) is either (λ,N) or (Λ,˜N+).
Set σ=λ if r∈[a,τ] and σ=Λ if r∈[τ,ρ]. In any case, for A=2(˜N−−1) we obtain
where the inequality comes from 2(˜N−−1)+a≥0, which holds for a>−1 and N≥2.
In the remaining of the section we prove Theorem 1.1. This is reduced to show that, for any given +∞>b>a>0, there exists a parameter δ>0 such that ρδ=b in addition to u(b)=0.
We start analyzing the behavior of the solutions uδ as δ approaches to 0 and +∞.
Lemma 4.4. If δ→0 then we have u(τδ)→0 and ρδ→+∞.
Proof. By Proposition 4.1 we have EΛ(r)≤EΛ(a) for all r≤τ, that is,
since uu′≥0 in [a,τ]. In particular, at r=τ=τδ,
Next we write the equation for u in [a,τ] as (u′r˜N−−1)′=−raλupr˜N−−1, and so integrating from a to τ produces
By combining the estimate for u in (4.2) and equality (4.3) we obtain
where C0 depends only on a,p,a,N,λ,Λ, and so
In particular, ρδ≥τδ→+∞ as δ→0.
Lemma 4.5. If δ→+∞ then ρδ→a and u(τδ)→+∞. Moreover, for every C0>0 there exists a positive constant c0 depending only on C0,a,N,p,λ,Λ such that
Proof. We denote Ar,s=Br∖¯Bs for any r>s and fix the operator M+, for M− it will be analogous.
Step 1) u(τδ)→+∞ when δ→∞.
Assume by contradiction that there exists a sequence δk→∞ with respective solutions uk=uδk of (2.4), with τk=τδk, ρk=ρδk, and uk≤M for all k.
Since Eλ(r)≥Eλ(a) for all r∈[a,τk] by Proposition 4.3, then
Since uk≤M, then τk→∞ as k→∞. In particular, τk≥a+1 for large k. Take ε∈(0,1) with ε≤a˜N−−1 and r∈[a,a+ε]⊂[a,τk]. Then we use Taylor expansion of uk at the point a to write
Now we notice that
Moreover, since u′k is decreasing in (a,r), we have u′k(ck)≤δk and so, by the second order PDE in (P+) and the fact that uk is increasing in (a,r), we deduce
Putting this estimate into (4.5) one finds
Finally, by evaluating it at r=a+ε it yields
since ˜N−−12aε≤12. But this is impossible since δk→+∞ and uk is bounded. This shows Step 1.
Step 2) ρδ→a as δ→+∞.
We first show that τδ→a as δ→+∞. This will be a consequence of Step 1 and the estimate
where C0 depends on a,a,p,λ. In order to prove (4.6), we write for r∈[a,τ] where τ=τδ,
and by integrating it in [r,τ], for r∈[a,τ), one gets
where C depends on a,a,p,λ. Another integration in [a,τ] yields
By using s=u(r) and u′dr=ds we get
by taking σ=su(τ) and dσ=dsu(τ), from which we deduce (4.6).
Now it is enough to prove that
If not, then there exists ϵ>0 and a sequence δk→∞, with ρk=ρδk≤+∞ and τk=τδk such that ρk>(1+ϵ)τk for the solutions uk=uδk of (2.4). In particular, uk is positive and decreasing in the interval [τk,(1+ϵ)τk].
For r∈(τk,(1+ϵ)τk] we consider the annulus Ak=Aτk,r where uk solves
where
Now, by the definition of first eigenvalue λ+1(D)=λ+1(M+,D) for the fully nonlinear Lane-Emden equation driven by M+ in the domain D with respect to the weight |x|a (see [2,10,13]), we have
Note that the following scaling holds
In fact, if λ+1,ϕ+1 are a positive eigenvalue and eigenfunction for the operator M+ with weight |x|a in A1,1+ϵ i.e.,
then μ+1,ψ+1, where μ+1=λ+1s−2−a and ψ+1(x)=ϕ+1(xs) are a positive eigenvalue and eigenfunction in As,s(1+ϵ) for M+ with weight |x|a.
Then, by combining (4.7) and (4.8) one finds
Using EΛ(τk)≤EΛ(r) for r∈[τk,ρk), it comes
Since τk→a as k→+∞ then
Now, by putting the latter and (4.9) into (4.10) we derive
where
and Jk→+∞ as k→∞ by Step 1. Hence
Via integration we get
which contradicts (4.9).
Step 3) δ≤C0 implies ρδ≥a+c0.
Let us prove the contrapositive, that is, if ρδ→a then δ→+∞.
As in Step 2, if s=maxAa,ρup−1=up−1(τ) then u solves
Now, by the maximum principle for the fully nonlinear equation through the characterization of the first eigenvalue in [2,13] (see also [10] for the weighted version) it follows
In fact, if we had s<λ+1(M+,Aa,ρ) then by the mentioned maximum principle we would obtain u≤0 in Aa,ρ which is impossible.
Using the scaling for the eigenvalue in (4.8), (4.2), and (4.11), we derive
Again by the scaling as in Step 2, λ+1(M+,D)→+∞ as |D|→0. Then ρδ→a implies δ→+∞. As a consequence, the ratio ρδ/a remains bounded away from 1 whenever δ is bounded from above.
Proof of Theorem 1.1. We fix the annulus Aa,b for some 0<a<b. For every δ>0, recall that uδ is the unique radial solution of the initial value problem (2.4), with a maximal radius of positivity given by ρδ∈(a,+∞]. Here, u(ρδ)=0 if ρδ<+∞, while u(r)→0 as r→+∞ is ρδ=+∞.
The mapping δ→ρδ is continuous by ODE continuous dependence on initial data. In particular, the set
is open. By Lemma 4.5, D is nonempty and contains an open neighborhood of +∞.
Let δ∗=δ∗(p) be the infimum of the unbounded connected component of D. Since D is open, if δ∗>0 then ρδ∗=+∞. If δ∗=0 then limδ→0ρδ≥limδ→0τδ=+∞ by Lemma 4.4.
The function δ↦ρδ is well defined and leads the interval (δ∗,+∞) onto (a,+∞) by the second part of Lemma 4.5. Then there exists δ>0 such that ρδ=b. The existence of negative solutions follows by Remark 2.2.
Remark 4.6. For the non weighted case a=0, in [5] it was shown that δ∗=infD for all p, that is D=(δ∗,+∞) is an open interval. Moreover, they prove there that at δ∗ only a fast decaying solution is admissible.
Acknowledgments
The authors would like to thank Carmem Maia Gilardoni for kindly making the figures, and for the precise comments of the anonymous referee.
L. Maia was supported by FAPDF, CAPES, and CNPq grant 309866/2020-0. G. Nornberg was supported by FAPESP grant 2018/04000-9, São Paulo Research Foundation.
Conflict of interest
The authors declare no conflict of interest.









 DownLoad:
DownLoad: