An inherently discrete–time $SIS$ model based on the mass action law for a heterogeneous population

1.   Introduction

As data gradually increase from various industries around the world, its status as an important production element and strategic production resource is increasingly emerging. As a flourishing technology, big data plays a significant role in improving the management level and increasing data utilization in the process of integrating into highway management. By using the toll collection system of an expressway network, the integration of information can be effectively realized. Based on this, it is more important to use effective methods to deal with the problem of freight volume forecasting, and to improve the forecasting accuracy with scientific and reliable of data analysis. Therefore, it is necessary to establish a targeted prediction model to provide a certain reference for future economic development planning, to guide the future planning and investment of the transportation network, and to ensure the application efficiency of the toll collection system of the expressway network.

The accuracy of existing artificial intelligence prediction methods is usually higher than that of traditional prediction methods although they have low requirements when selecting raw data, the reason is that they can analyze the optimal logical relationship between data by using the ideas of induction, deduction and analogy. For example, Zhang et al. proposed an EMD-LSTM model to grasp water quality in the drainage network; as a result, the model has achieved the highest R2 values and the lowest root mean square error values in the prediction process ^[1]. Sigakova et al. used a regression tree and other machine learning methods to study the close relationship between various influencing factors, which improved the freight volume forecast ^[2]; Nuzzolo and Comi joined the existing logistics infrastructure of a city into the influencing factors; and then used the city freight volume of Rome to verify the proposed model; the results showed that the accuracy when predicting freight-delivery-vehicle flow by using generalized least squares and maximum likelihood estimation is higher than that by traditional methods ^[3]; Ran constructed a backpropagation (BP) neural network prediction model with the help of Nanning road freight volume data over the years. Compared with the trend extrapolation, exponential smoothing, gray prediction and exponential regression method, their model was able to fit the original data more accurately and have a smaller relative error ^[4]. Wang and Shao not only constructed a road freight volume prediction model based on a support vector machine by using historical data of industries and economic indicators of Beijing such as import and export trade volume and so on, but they also analyzed their impacts on the results. Apart from the results showing that the proposed model has high effectiveness and feasibility, it can be extended to practical applications ^[5]. Based on this, Li et al. also used variables such as population number, vehicle ownership and various economic indicators to establish a generalized regression neural network. After simplifying the adjustable parameters, the proposed model was found to have high efficiency in the forecast of highway freight volume in Nanjing ^[6]; Wang compared and analyzed a variety of traditional forecasting methods with the proposed BP neural network freight volume forecasting model by using economic indicators; the results showed that the proposed model has better applicability ^[7]. In a word, the above prediction models mainly focus on the impact of various macro factors on freight volume, but they cannot solve the short-term prediction problem of freight volume. Still and all, they also provide a new idea for establishing intelligent prediction models.

In recent years, the use of big data has become more and more popular in the forecast of freight volume ^[8,9,10]. For example, Liang et al. proposed a hybrid kernel function support vector machine model after performing a grey correlation analysis on various influencing factors ^[11]. Considering the characteristics of freight data in different periods, Cheng et al. established a multivariate long short-term memory (LSTM) prediction model based on monthly and daily freight volume data, then, they used the freight volume data from 2010 to 2017 on the Guangzhou Railway to verify the proposed model ^[12]. Since many scholars have begun to focus on road freight transportation, machine learning algorithms are beginning to enter the research field. Different from the traditional road freight volume statistics method using the sampling survey ^[13], the calculation of regional short-term freight volume generally relies on real-time toll station receipts, which overcomes the shortcomings of incomplete data ^[14]. With the help of a highway network toll collection system ^[15,16], after analyzing the temporal and spatial variation of highway traffic flow ^[17,18], some researchers began to use the deep learning model to predict the highway freight volume ^[19,20], Wang and Chen even used a graph convolutional network to predict the origin-destination (OD) freight volume matrix according to the toll station data ^[21]. Inspired by this, the above models have also laid a foundation for predicting freight volume from the perspective of spatio-temporal information importance.

Expressway freight volume has strong regional and seasonal fluctuation characteristics. It has become an urgent problem in the logistics field to explore how to build data sets from the perspective of time and space, establish short-term prediction models by using relevant factors, and improve the accuracy of freight volume prediction. The LSTM network utilizes the sensitivity of orders and is especially suitable for processing and predicting time series with nonlinear properties. Using a quantum particle swarm optimization (QPSO) algorithm to tune the LSTM parameters can reduce redundancy and save simulation time. Through the improvement of LSTM, the model can capture patterns that may be ignored by LSTM. In addition to the prediction of highway freight volume, as a common LSTM variant, the QPSO-LSTM algorithm proposed in this paper can be applied for the prediction of continuous variables such as short-term traffic flow, future temperature and stock trends. With the help of the Jilin expressway online toll information system, we studied the application of a QPSO-LSTM network model based on spatial information importance in expressway freight volume prediction, which can be used as a supplement to the conventional freight volume prediction model.

2.   QPSO-LSTM model theory

2.1.   QPSO

PSO is a kind of bionic algorithm, which realizes random optimization by simulating the behavior of organisms. It is designed by imitating the process of bird groups looking for food. Particles can compare the individual optimal value and the global optimal value of the group according to the state in flight with the fitness value. After obtaining the results, the optimal solution can be searched in the solution space of the optimal particles. The disadvantage of PSO is that the speed and position of particles determine the current motion state of particles. In the process of particle evolution, the feasible solution of particles is a limited and shrinked region, and the particle speed is fixed. Hence, once the particle motion trajectory is fixed, it cannot cover the whole solution space, which leads to the phenomenon of local optimal values. In the QPSO algorithm, the quantum uncertainty theory in quantum mechanics theory is introduced. In quantum space, the velocity and position of particles cannot be determined at the same time, so the trajectory of particles is uncertain and can appear randomly in all solution spaces, which solves the problem of the particle trajectory of PSO algorithm being fixed and easy to fall into local optimal solutions ^{[22,23,24,25,26]}. In QPSO, the wave function is used to represent the motion state of particles. Since space and time are independent of each other in quantum space, particles corresponding to wave functions are also random. Besides, QPSO has the advantages of fewer parameters, a simple structure and fast convergence.

For the QPSO, the $mbest$ parameter is introduced to represent the average value of the historical best position, $pbest$ of the particle swarm. The particle update steps of the QPSO algorithm are as follows.

Step 1: Calculate $mbest$, i.e.,

where $M$ represents the size of the particle swarm; ${p}^{best\_i}$ represents the $i$ th $pbest$ in the current iteration.

Step 2: Update particle position u:

where $gbest$ represents the current global optimal particle; ${P}_{i}$ is used to update the position of the $i$ th particle.

The particle position update formula is

where ${x}_{i}$ represents the position of the $i$ th particle; $\alpha$ is the innovation parameter; $\varPhi$ and $\mu$ are uniformly distributed values on (0, 1), and the probability of taking both positive and negative is 0.5. It can be seen that there is only one innovative parameter setting ($\alpha$) in the QPSO algorithm, and $\alpha$ is generally not greater than 1.

2.2.   LSTM network structure

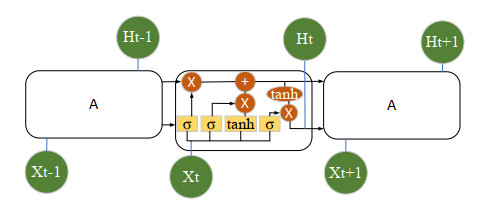
The LSTM network is a deep recurrent neural network proposed by Hochreiter and Schmidhuber ^[27]. It selectively affects the state of each moment in the neural network by relying on the "gate" structure, and it mainly includes a forget gate, input gate and output gate. Their roles, in turn, are to decide when to forget the previous state, add a new state and output the final state, respectively. The network structure of the LSTM network is shown in Figure 1.

As can be seen in Figure 1, each black line represents the transmission direction of the entire vector, ie from the output of one node to the input of other nodes. The rounded boxes represent computational operations; the boxes in the middle represent the learned neural network layer; joined lines represent vector connections; separated lines represent content being copied and then distributed to different locations. The core idea of LSTM is not only that the last state can be stored through the connection of the state of cells (A in the figure), but also that useful states can be stored through network learning. Regarding the iterative process, the update formulas for the forget gate, input gate and output gate are as follows:

where, ${W}_{f}$, ${W}_{i}$ and ${W}_{o}$ represent the corresponding weights of the forget gate, input gate and output gate respectively; ${b}_{f}$, ${b}_{i}$ and ${b}_{o}$ represent the bias vectors of the forget gate, input gate and output gate; ${C}_{t}$ represents the new cell state vector; ${C}_{t-1}$ represents the cell state vector at the previous moment; $\sigma$ represents the sigmoid function.

When using the QPSO algorithm for parameter tuning, particle initialization is transformed into a series of LSTM parameters, and its fitness is the MAE or MAPE value of the LSTM model when using initialization parameters, i.e.,

where $\stackrel{~}{{y}_{i}}$ represents the predicted value, ${y}_{i}$ represents the true value, and $N$ represents the number of training sets.

3.   Dataset construction

When forecasting freight volume, in addition to the selection of models, the role of data sets should not be underestimated. This is because there are abnormal, repeated, blank values in the original records of the expressway toll collection system. Since the information contained in the records constitutes the most original data, it is necessary to statistically analyze on them and extract effective information which can improve the prediction accuracy of the model. This method extracts the information that affects the freight volume from the perspective of spatial importance, and completes the reconstruction of the data set. Before the reconstruction of the data set, the main preparations are to convert the freight traffic records into freight traffic information of each spatial region, and then preprocess the input records to obtain the freight traffic information between each pair of regions. After all that work, the spatial influencing factors analysis for the regional freight traffic can be conducted further.

3.1.   Grid embedding

Definition 1: Divide the statistical time interval $T = [{T}_{a}, {T}_{b}]$ into a series of time series $\{{T}_{1}, {T}_{2}, ..., {T}_{M}\}$, and each time series is called a time slot. When the freight volume is counted, the time slot can be a year, month, day, or hour. According to the size of the time slot, freight volume forecasts can be divided into short-term and long-term forecasts.

Definition 2: Divide a spatial region, such as an administrative region, city, province or even a country, into $N$ non-overlapping grids $G = \{{g}_{1}, {g}_{2}, ..., {g}_{N}\}$. Each grid is called a grid, and its size is determined by the area of the region and the maximum number of grids $N$. Figure 2 depicts a region consistings of 16 grids. The data point ${M}_{i}$ of each grid ${g}_{i}$ in a given time slot ${T}_{i}$ is not only affected by the previous time slot ${T}_{1}, {T}_{2}, ...{T}_{i-1}$, but also by the data ${M}_{j}, j = 1, 2, ...N, j\ne i$ of other grids. For the freight volume, this occurrence within the grid is closely related to the cargo attraction of other regions.

Definition 3: For a grid ${g}_{i}$, when there is a cargo transportation record ${M}_{ij}$ with other grids ${g}_{j}$ ${g}_{j}, j = 1, 2, ...N, j\ne i$, ${g}_{j}$ is called a neighborhood of the grid ${g}_{i}$.

3.2.   Data preprocessing

The regional outline of Jilin Province is depicted in Figure 3 which shows its longitude and latitude. It includes highway toll stations, county-level administrative centers, county boundaries, provincial boundaries and its main highway lines in each administrative region.

The expressway toll collection system includes truck traffic records of 188 provincial toll stations and 24 provincial border toll stations. Each truck traffic record exists in the form of toll records which includes a series of valid variables, such as a vehicle entry time, entry toll station, driving lane, exit time, exit toll station, vehicle type, load, ETC charge or not, vehicle license plate, etc. The provincial border toll station mainly records the information of vehicles entering and leaving the provincial boundary, such as the time of entering and leaving the provincial boundary, the serial number of the toll station entering and leaving the provincial boundary, the license plate, etc. The description of the field attributes is shown in Table 1.

When organizing the input data of the model, the interception time interval for each pair of grids is set to 1 day, that is, the number of cargo exchanges in one day. The reason why one day is selected as the statistical standard is that among the truck trips involved, the medium and long trips occupy the vast majority. Taking December 1, 2020 as an example, if the cargo transportation volume from Dongfeng County to Changchun City is 486.2 tons, it means that the total cargo is 486.2 tons from all of the toll stations in Dongfeng County to all of the toll stations in Changchun City.

The specific process and steps of data preprocessing are shown in Figure 4.

Step 1: Perform data cleaning on the expressway operation data and gantry data, mainly including the processing of missing values and outliers. According to the administrative regionalization of Jilin Province, the whole region of Jilin City is divided into a series of grids ${G}_{i}, i = 1, 2, ..., N$, where each grid is an administrative region including all of the toll stations located within it; in the end a toll -station- grid map is established.

Figure 3 shows the location map of all toll stations (blue triangle) in Jilin Province. The freight volume of each grid is the sum of the freight volume records of all toll stations located in it. In this study, each county-level administrative region is regarded as a grid, and Jilin Province includes a total of 48 grids (41 county-level administrative regions and seven provincial toll stations). The entry and exit toll stations in each record are mapped to each grid; then, the records are organized into records of cargo exchange between grids. Days are used as the time slot for statistics, that is, each piece of data records the cargo exchange volume of a pair of grids in one day.

Step 2: Filter out all types of trucks from the original data set which includes six types, namely, Vehicle type I ~ Vehicle type VI.

Step 3: Calculate the average dead-weight of the six truck types.

Step 4: Correlate the provincial record and gantry record according to the license plate information and time information to form a complete data record.

Step 5: Take one grid ${g}_{i}$ as the origin point O, and the other grids ${g}_{j}, j = 1, 2, ..., N$ as the destination point D. Then take the exit time as the statistical standard; and calculate the total freight volume of different truck types for each day.

Step 6: Repeat Step 5 for all grids ${g}_{i}, i = 1, 2, ..., N$.

Step 7: Encode the input data into a two-dimensional matrix format, in which the columns are arranged in grid order, and the rows are arranged in a time series. Each row records the ${g}_{i}$ occurrence of cargo exchange between one grid with other grids in a time slot, and it satisfies the conditions of independent and identical distribution, finally the OD data record format is set as shown in Table 2.

Taking Changchun City as an example, Table 2 shows the cargo volume of within Changchun City and Changchun City to the other cities (such as Dehui, Yushu, Nong'an, Jilin, Jiaohe, Shulan, Panshi, Yongji, Siping, Gongzhuling, Shuangliao, Lishu, Yitong Manchu. Autonomous County, Liaoyuan, Dongfeng, Dongliao, Tonghua City, Tonghua County, Huinan, Liuhe, Meihekou, Baishan, Fusong, Jingyu, Songyuan, Qianguo Mongolian Autonomous County, Changling, Gan'an, Fuyu, Baicheng, Da'an, Zhenlai, Taonan, Yanji, Tumen, Dunhua, Hunchun, Longjing, Wangqing, Antu), as well as the Changchun borders, Siping borders, Liaoyuan borders, Tonghua borders, Songyuan borders, Baicheng borders and Yanji borders. The cargo volume sum of all grids is the occurrence of Changchun City.

3.3.   Modeling dataset reconstruction based on spatial importance

To address the fact that not all grids have goods exchange between them, screening the preprocessed data and appropriately selecting the spatial domain embedding dimension are the basis for regional freight volume forecasting. A QPSO-LSTM network model as developed by using this dataset, and it can predict the amount of freight in one grid in the future. According to the classification and regression tree (CART) algorithm, the importance of 46 spatial variables in the statistical time period is calculated respectively, and then the dimension selection is carried out according to the calculation results.

The CART algorithm is a typical nonparametric binary tree classifier, which is used to select the spatial embedding dimension of the input data. The CART algorithm first calculates the Gini index between the freight volume ${{M}_{i}}_{N}$ and the occurrence volume ${M}_{t}$ between different grids, and then it ranks the importance of ${M}_{t-i}$ to ${M}_{t}$.The input spatial embedding dimension is selected by setting an importance threshold. In order to analyze the impact of each grid on the future freight volume, the importance of the predictor variables is calculated by using Eq (9).

where $Gini\left(z\right({a}_{i}, t\left)\right)$ represents the Gini index of the $j$ th attribute of the $t$ th internal node; ${M}_{CART}$ represents the total freight volume; ${M}_{t}$ represents the cargo volume of the internal node at time $t$.

3.4.   Overall flow of the short-term freight volume hybrid forecasting algorithm

Combined with the mentioned Sections 3.1-3.3, the overall flow of the spatial importance-based QPSO-LSTM algorithm proposed in this paper is as follows.

1) The data of the expressway toll system is preprocessed first. Then, the appropriate time interval is selected; and the freight volume value between each pair of grids is obtained by statistical analysis.

2) After obtaining the freight volume information between grids, the OD transaction records of cargo with other grids are obtained for each grid.

3) For the OD transaction records in Step 2, the algorithm in Section 3.3 is used to calculate the importance of other grids to the starting grid. This is followed by, sorting the importance, setting the importance threshold, and delete other grid data whose importance is less than this threshold.

4) For the reconstructed dataset obtained by Step 3, use the QPSO algorithm to obtain the optimal tuning parameters for the LSTM model.

5) After selecting the length of the input space dimension, the optimal tuning parameters are input into the LSTM model for freight volume forecasting.

6) Using the QPSO-LSTM model to complete the prediction, compare the error prediction results with the conventional LSTM model. The overall prediction effect of the QPSO-LSTM model is finished.

4.   Result analysis and comparison

4.1.   Analysis of spatial influencing factors

Based on the preliminary analysis of expressway freight volume, it can be understood that the difference in spatial factors has obvious influence on the freight volume. If these influencing factors are included in the prediction model features, the robustness of the algorithm will be increased and the prediction accuracy will be improved accordingly. The algorithm in Section 3.3 was used to calculate the importance of other grids to the initial grid. In order to represent fairness, four grids were randomly selected as representatives; of the spatial importance of Hunchun City, Panshi City, Siping City and Tumen City, respectively, as shown in Figure 5 below.

Through the analysis of the spatial dimension, it can be found that each grid is differently affected by the freight volume of other grids. For example, Hunchun City is mainly affected by Tumen City, Yanji City, Jiaohe City, Jilin City, Antu City, Changchun City, Dunhua City, the Changchun Boundary and Tonghua. The impact ratios were 47.40, 19.11, 10.06, 9.44, 4.64, 2.22, 1.69, 1.51, and 1.45%, respectively. But, Panshi City is mainly influenced by Panshi City itself, Huinan County, Nong'an County, Dongfeng County, the Tonghua Boundary, Jilin City, Yongji County and Changchun City. The impact ratios were 33.96, 22.95, 13.22, 5.52, 4.13, 3.36, 3.33 and 2.58%, respectively. Siping City has relatively few spatial influence variables, such as Gongzhuling City, Lishu County, Changchun City and Siping City. Alternatively, Tumen City is greatly influenced by Antu City, Jilin City, Yanji City, Hunchun City and Changchun City. Their importance factors were calculated to be 39.10, 29.33, 12.65, 7.47 and 33.88%, 25.67, 20.26, 9.25 and 2.88% respectively. The freight volume relationship between different grids is closely related to the freight volume exchange between the grids. Therefore, it is essential to obtain the dynamic freight volume matrix between different grids to improve the LSTM model and predict the freight volume in future.

On the premise of taking into account the modeling accuracy level and efficiency, 10 grids whose importance is greater than 0.001 were selected to represent the length of the historical freight volume, that is, the grid embedding dimension of the QPSO-LSTM model was 10.

4.2.   Result analysis of QPSO-LSTM model

In order to fully verify the effectiveness and performance advantages of the LSTM model with on QPSO parameter tuning in short-term freight volume prediction, the QPSO algorithm simulation parameters were set as shown in Table 3. The experimental platform was a CPU Intel (R) core i5 and 12G RAM, Windows 10 (64 bit) and anaconda3 (64 bit). The QPSO parameter tuning simulation results for Changchun City, Jilin City, Siping City and Nong'an County are shown in Table 4. In the experiment, the original data were all from the records of the Jilin expressway toll system from January 2018 to June 2021, with a total of 62, 510, 817 records.

The results of parameter tuning includes four parameters. The first parameter N is the QPSO particle number, which means N-fold cross-validation for the LSTM model. The second parameter is the number of LSTM unit cells in the first layer, the third parameter is the number of LSTM unit cells in the second layer; and the fourth parameter is the number of batches per iteration. It can be seen in Table 4 that, when predicting the freight volume of Changchun City, the LSTM model for the freight volume was conducted with two-fold cross-validation and the model included two layers. The first layer included a cell unit, and the second layer included a cell unit. When the number of batches was 1, the prediction effect was the best.

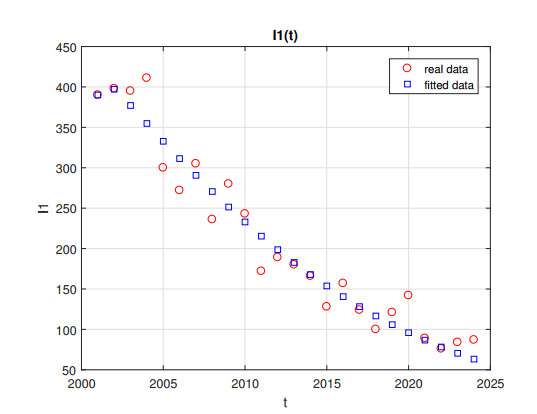
When building the LSTM network with the parameters in Table 4, the prediction results for Changchun City, Jilin City, Siping City and Nong'an County were as shown in Table 5 and Figure 6.

For the sake of fairness, five dates were randomly chosen for illustration in Table 5, for example, we will discuss Changchun City on 2020/05/25, 2020/08/31, 2020/10/18, 2020/11/08 and 2021/06/19. The relative errors between the actual values of the test set and the predicted values obtained by using the model were 13.12, 13.71, 1.63, 3.52 and 2.40% respectively. The relative errors of Jilin City on 2020/5/27, 2020/8/28, 2020/10/19, 2020/11/15 and 2021/6/15 are 20.22, 6.64, 6.09, 14.99 and 1.81%, respectively. The maximum and minimum errors of different grids were within the acceptable range. According to the comparison between the actual value and the predicted value within the whole-time range of the test set, the LSTM model optimized by QPSO algorithm can accurately predict the changing trend of shipment volume from 2020 to 2021 for different grids. In February, when the freight volume fell sharply, for example, for Changchun 2021/2/14, the actual freight volume was 9827.703 tons, and the predicted value was 10744.6536 tons, with a relative error of only 9.33%. The relative error for Jilin City, Siping City and Nong'an County on 2021/2/3 were -4.21, 8.93 and 9.88% respectively. This also shows that QPSO-LSTM model can still achieve a good prediction effect when the freight volume decreases sharply.

For all datasets, the training set and test set were divided as 0.64:0.36. For the four grids (Changchun, Jilin, Siping and Nong'an County), the iteration diagram of MAE and MAPE was as shown in Figure 10. It can be seen in Figure 10 that whether it is the MAE or MAPE, the four grids could converge within 10 rounds. The order of MAE was Jilin City > Siping City > Changchun City > Nong'an County, and the order of MAPE was Nong'an County > Changchun City > Siping City > Jilin City.

4.3.   Comparative analysis of QPSO-LSTM model and other models

Using the same dataset, another set of experiments was designed to evaluate a benchmark for comparative analysis, that is, the LSTM model that does not use the QPSO algorithm for parameter optimization. The experimental parameters were set randomly except that the proportion of the training set and the test set were the same; and the LSTM had the double-layer structure. In order to facilitate the comparison of models, the same four grids (Changchun City, Jilin City, Siping City and Nong'an County) were again selected for simulation. The simulation results for the four grids using the conventional LSTM model are shown in Figure 8, and the comparison results with the MAE and MAPE for the QPSO-LSTM model are shown in Figure 9.

It can be seen in Figures 6 and 8 that although the general changing trend of freight volume can also be predicted by using the conventional LSTM model, its prediction effect was worse than that of the QPSO-LSTM model for Changchun, Jilin, Siping and Nong'an. The error of the former was 40% higher than that of the latter, on average. The prediction value of the conventional LSTM model was relatively stable, but it could not cope with the sharp change in freight volume. For example, the relative errors from 2021/02/01 in Changchun, 2021/02/27 in Jilin, 2021/01/25 in Siping and 2021/02/24 in Nong'an County were 53.34, 134.56, 12.56 and 33.28% respectively. Therefore, when using the conventional LSTM model, although the internal correlation between the freight volume and spatial factors was also used, the influence of the length selection of the freight volume historical time information was ignored. Because the length of the historical time cannot be determined, the information was redundant and the prediction effect was poor.

Similarly, it can be seen in Figure 9 that the MAE and MAPE of the QPSO-LSTM model were lower than those of the conventional LSTM model. The MAE values of the four grids obtained by using the QPSO-LSTM model were 0.00367, 0.00578, 0.00381 and 0.0018, respectively. The MAE values of the four grids obtained by using the conventional LSTM model were 0.00558, 0.01029, 0.008 and 3.132e-4. The errors increase by 52.04, 78.03, 109.97 and 82.60%, respectively. The MAPE also decrease from 61.07905, 57.71179, 71.85626 and 51.63141 to 16.55851, 14.10185, 15.3475 and 17.00631, respectively, and the errors increased by 268.87, 309.25, 368.20 and 203.60% respectively. This also shows that the prediction effect of the QPSO-LSTM model is better than that of the conventional LSTM model, which is consistent with the conclusions in Figure 6 and Figure 8.

To sum up, the QPSO-LSTM model based on spatial information importance has the highest accuracy and stability for short-term freight volume prediction.

5.   Conclusions

Accurate freight volume prediction is necessary to reflect the contribution, quality and structure of transportation development. Aiming to solve the problem of highway freight volume prediction, we proposed an LSTM model whose parameters were tuned by a QPSO algorithm. In order to improve the calculation efficiency of the model, it uses a CART algorithm to obtain the importance factors of spatial information; and then screen the input information after exceeding the preset threshold; finally, the QPSO -LSTM model input data set is reconstructed, which can ensure the accuracy and reduce the complexity of the model at the same time.

In order to prove the effectiveness of the QPSO-LSTM model, two groups of experiments were designed. The first group of experiments proved the necessity of QPSO-LSTM from the perspective of spatial information importance. The second group of experiments compared the simulation effects of QPSO-LSTM with those of the conventional LSTM model, which still has a two-layer structure but the parameters are set randomly. The results showed that QPSO-LSTM not only well reflects the changing trend and law of highway freight volume, but it can also improve the prediction accuracy. The two groups of experiments were verified by taking the highway freight volume records of Jilin Province in China as an example, which shows that the proposed model can be widely used as a practical short-term highway freight volume prediction method. The next step will continue to carry out relevant research on dataset construction methods and prediction models.

Acknowledgments

This work was supported by the Young Teachers Research Project for Xi'an University of Technology: [Grant Number 256081921]; Special nature project of Shaanxi Provincial Department of Education [Grant Number 21JK0786]; Scientific research plan project of Shaanxi Provincial Department of Education [Grant Number 2021R025]; Scientific research plan project of Shaanxi Provincial Department of Education [Grant Number 21JZ005]; and Natural Science Basic Research Program of Shanxi: [Grant Number 2021JQ-279].

Conflict of interest

The authors declare that there is no conflicts of interest.