Mathematical analysis of synthesis chemical reactions for virus building block polymers in vivo

1.   Introduction

A virus is an infectious microbe containing nucleic acid (either DNA or RNA) and protein. A virus cannot replicate by itself; instead, it must infect human, animal or plant cells and use components of the host cell to give rise to numerous progenies. These progenies are usually genetically and structurally identical to the parent virus. Often, a virus ends up killing the host cell in its reproduction process, causing damage to the host organism. Well-known examples of viruses causing human disease include COVID-19, acquired immune deficiency syndrome (AIDS), smallpox, and measles. In particular, the COVID-19 pandemic is claiming millions of lives in only three years ^[1], and the World Health Organization said that 1.3 million people were newly infected worldwide in 2022. These viruses have brought huge losses to mankind.

All viruses depend on cells for their reproduction, and the styles of virus reproduction are numerous. Here, human immunodeficiency virus (HIV) for example shows the reproduction process for a kind of virus. HIV enters the human body. Once it encounters a T-cell, it actively attaches itself to the T-cell. Attachment is a specific binding between proteins on the viral surface and proteins that serve as receptors on the T-cell surface. Then viral fusion occurs and fusion allows the genetic material of the virus to be injected directly into the cell's cytoplasm. The cellular machinery is hijacked to produce the necessary components of HIV, such as new genetic material and structural proteins. These components are transported to the inside of the cell membrane. Structural proteins are assembled and begin to deform a section of the membrane to form the new viral envelope. Viral genetic material is gathered into the envelope, and a viral budder is formed. Lastly, it is released and a new virus is reproduced ^[2]. A lot of research has unraveled the ways to stop viral replication within human cells.

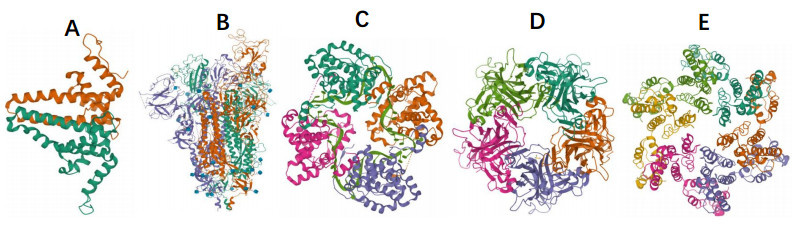
Assembly of structural proteins is a key process for a kind of viral replication. It is composed of two steps. The first step is that structural protein monomers are polymerized to building blocks. The second step is that these building blocks are assembled to virus capsid. The first step provides a foundation for the second step. Its aim is to form the building block to improve assembly efficiency of virus capsid. In this work, we focus on the first step. In general, building blocks formed in the first step include five types: dimer (for example, Hepatitis B virus ^[3]), trimer (for example, SARS-CoV-2 Omicron ^[4]), tetramer (for example, La Crosse virus ^[5]), pentamer (for example, Betapolyomavirus ^[6]), and hexamer (for example, HIV-1 ^[7]). The crystal structures ^[8] of building blocks for these viruses are shown in Figure 1.

Numerous biologists and pharmacists are interested in the synthesis chemical reactions of virus building blocks. Wang and Hou ^[9] analyzed drug and drug candidate building blocks. Chen et al. ^[10] presented a general approach to study modular self-assembly of biomolecules with protein building blocks. Christiansen et al. ^[11] found that the trimeric major capsid protein of Mavirus was stabilized by its interlocked N-termini enabling core flexibility for capsid assembly. Ni and Chau ^[12] constructed a spherical artificial virus by using building blocks and studied the intricate morphology and the intracellular transformation of the spherical virus. Liu et al. ^[13] studied dynamical analysis of synthesis reactions for these five building blocks including dimer, trimer, tetramer, pentamer and hexamer in vitro, where the total concentration of proteins for building blocks is constant.

Synthesis chemical reactions for virus building block polymers in vivo are more complex than that in vitro. In vivo, monomers will be produced continuously, and building blocks will decrease for assembly at the same time. As a result, mathematical models for synthesis chemical reactions in vivo are more complex than that in vitro. In this work, we will focus on synthesis chemical reactions in vivo for five types of building blocks, including dimers, trimers, tetramer, pentamer and hexamer. We will present mathematical models for these synthesis chemical reactions. Then the existence, uniqueness, and stability of the positive equilibrium solution of these models will be proved. Mathematical analysis of these models will be carried on one by one. These theoretical results will support that synthesis chemical reactions of virus building blocks in vivo will be tend to a stable state for any initial state.

2.   Synthesis reactions and properties

2.1.   Dimer building block

We first consider that the dimer is the building block of a kind of virus assembly in vivo. The example is shown in Figure 1(A). Two monomers are polymerized to a dimer, and their chemical reactions are as follows.

where $M_1$ is the monomer, $M_2$ is the dimer building block, $k_1^+$ is the on-rate constant, $k_1^-$ is the off-rate constant, $r$ is the assemble rate of virus by using dimer building blocks, and $\phi\stackrel{\mu}{\longrightarrow} M_1$ describes the production of the monomer with the rate $\mu$.

The concentration of monomers will decrease as they polymerize to dimers. They will increase as they are produced with the rate $\mu$. Moreover, they will also increase as dimers dissociate to them. Based on the mass action law and mass conservation law ^[14], we can get

where $[M_1]$ and $[M_2]$ are the concentrations of $M_1$ and $M_2$, respectively.

So similarly, we obtain the following mathematical model for the above polymerization chemical reactions in vivo.

The initial condition is

where $C_0$ is a constant.

Theorem 1. The mathematical model for dimer building block polymerization chemical reactions (2.2) exists a unique solution.

Proof. Let

We can get

Obviously, functions in Eq (2.4) are all continuous in $[0, +\infty]\times [0, C_0]\times [0, C_0]$. So, $f(t, [M_1], [M_2])$ satisfies the Lipschitz condition for all $[M_1], [M_2]$, $[M_1]', [M_2]'$

where $L$ is a constant.

Therefore, based on the Picard theorem, the mathematical model for dimer building block polymerization chemical reactions (2.2) exists a unique solution.

Theorem 2. The positive equilibrium point of the mathematical model for dimer building block polymerization chemical reactions (2.2) exists and is unique.

Proof. Let $\frac{d[M_i]}{dt} = 0, i = 1, 2$ for system (2.2). We can get

Based on the second equation of Eq (2.6), we can get

Substitute Eq (2.7) into the first equation of Eq (2.6). We can get

Add Eq (2.8) to Eq (2.7). We can get

Therefore, the positive equilibrium point of the mathematical model for dimer building block polymerization chemical reactions (2.2) exists and is unique.

Theorem 3. Mathematical model for dimer building block polymerization chemical reactions (2.2) is locally asymptotic stable.

Proof. The characteristic equation of Eq (2.2) is as follows.

For $k_1^+ r [M_1] > 0$, $(4k_1^+[M_1] + k_1^- +r) > \sqrt{(4k_1^+[M_1] +k_1^- +r)^2 - 16k_1^+r[M_1]}$. Therefore, the real parts of $\lambda_{1, 2}$ are all negative.

Therefore, the mathematical model for dimer building block polymerization chemical reactions (2.2) is locally asymptotic stable.

2.2.   Trimer building block

We consider that the trimer is the building block of a kind of virus assembly in vivo. The example is shown in Figure 1(B). The polymerization chemical reactions are as follows.

where $M_i$ is the protein with $i$ monomers, $k_i^+$ is the on-rate constant, $k_i^-$ is the off-rate constant $i = 1, 2, 3$, $\mu$ is the production rate of the monomers, and $r$ is the assemble rate of the virus.

The mathematical model for the polymerization chemical reactions in vivo is as follows ^[14].

where $[M_i]$ is the concentrations of $M_i, i = 1, 2, 3$.

The initial condition is

Theorem 4. The positive equilibrium point of the mathematical model for trimer building block polymerization chemical reactions (2.11) exists and is unique.

Proof. Let $\frac{d[M_i]}{dt} = 0, i = 1, 2, 3$ for system (2.11). We can get

Let the second equation of Eq (2.12) multiply by 2 and let the third equation of Eq (2.12) multiply by 3. Then, add them to the first equation of Eq (2.12), and we can get

Add the above equation to the last equation of Eq (2.12), we can get

Add Eq (2.13) and the last equation of Eq (2.12) to the second equation of Eq (2.12). We can get

Add Eq (2.15) to Eq (2.14). We can get

Let

Then

So $y = f([M_1])$ decreases monotonically in $\left [0, \sqrt{\frac{\mu}{9k_1^+}}\right ]$, and it increases monotonically in $\left [\sqrt{\frac{\mu}{9k_1^+}}, +\infty \right ]$. Moreover,

$y = f([M_1])$ has only one intersection with the positive horizontal axis. So Eq (2.16) has only one positive solution, denoted by $[M^*]$. Then,

Therefore, the positive equilibrium point of the mathematical model for trimer building block polymerization chemical reactions (2.11) exists and is unique.

Theorem 5. Mathematical model of the trimer building block polymerization reactions (2.11) is locally asymptotic stable.

Proof. The characteristic equation of mathematical model (2.11) is as follows.

By using the symbolic computational toolbox of the MATLAB software, we get

where

The Routh-Hurwitz matrix of the above characteristic equation is as follows

where $c_{11} = 1, c_{12} = b, c_{21} = a, c_{22} = c$.

We get values of the first column elements in Routh-Hurwitz matrix (2.17) by using the symbolic computational toolbox of the MATLAB software. For their expression is too complex, we only show their signs as follows.

Based on the Routh-Hurwitz rule, their real parts of all eigenvalues in the above characteristic equation are all negative. Therefore, based on the theorem ^[15], the trimer building block polymerization reaction system (2.11) is locally asymptotic stable.

2.3.   Tetramer building block

We consider that the tetramer is the building block of a kind of virus assembly in vivo. The example is shown in Figure 1(C). The polymerization reactions are as follows.

where $M_i$ is the protein with $i$ monomers, $k_i^+$ is the on-rate constant, $k_i^-$ is the off-rate constant $i = 1, 2, 3, 4$, $\mu$ is the production rate of the monomers, and $r$ is the assemble rate of the virus.

The mathematical model for the above polymerization chemical reactions in vivo {is as follows ^[14]}.

where $[M_i]$ is the concentration of $M_i, i = 1, 2, 3, 4$.

The initial condition is

Theorem 6. The positive equilibrium point of the mathematical model for the tetramer building block polymerization reaction system (2.19) exists and is unique.

Proof. Let $\frac{d[M_i]}{dt} = 0, i = 1, 2, 3, 4$ for mathematical model (2.19). We can get

Let the second equation of Eq (2.20) multiply by 2, let the third equation of Eq (2.20) multiply by 3, and let the fourth equation of Eq (2.20) multiply by 4. Then, add them to the first equation of Eq (2.20), and we can get

Add the above equation to the last equation of Eq (2.20). We can get

Add Eqs (2.21) and (2.22), and the fourth equation of Eq (2.20) to the third equation of Eq (2.20). We get

Add the third and fourth equation of Eq (2.20) to the second equation of Eq (2.20). We can get

Add Eqs (2.21) and (2.23) to Eq (2.24). We can get

Let

Because $k_i^{\pm} > 0, [M_1] > 0$, $f'([M_1]) > 0$. $f([M_1])$ increases monotonically in $\left (0, +\infty \right)$. Moreover,

Therefore, there is a unique positive solution denoted by $[M_1^*]$ for $f([M_1^*]) = 0$. Substitute $[M_1^*]$ to Eqs (2.22) and (2.23), and we can get the unique positive values $[M_2^*], [M_3^*]$. Therefore, the positive equilibrium point of mathematical model (2.19) exists and is unique.

Theorem 7. Mathematical model of tetramer building block polymerization reactions (2.19) is locally asymptotic stable.

Proof. The characteristic equation of mathematical model (2.19) is as follows.

where

By using the symbolic computational toolbox of the MATLAB software, we get

where

The Routh-Hurwitz matrix of the above characteristic equation is as follows

where $c_{11} = 1, c_{12} = b, c_{13} = d, c_{21} = a, c_{22} = c$.

We get values of the first column elements in the Routh-Hurwitz matrix (2.25) by using the symbolic computational toolbox of the MATLAB software. For their expression is too complex, we only show their signs as follows.

Based on the Routh-Hurwitz rule, real parts of all eigenvalues in the characteristic equation are all negative. Therefore, based on the theorem ^[15], the mathematical model of tetramer building block polymerization reactions (2.19) is locally asymptotic stable.

2.4.   Pentamer building block

We consider that the pentamer is the building block of a kind of virus assembly in vivo. The example is shown in Figure 1(D). The polymerization reactions are as follows.

where $M_i$ is the protein with $i$ monomers, $k_i^+$ is the on-rate constant, $k_i^-$ is the off-rate constant $i = 1, 2, ..., 5$, $\mu$ is the production rate of the monomers, and $r$ is the assemble rate of the virus.

The mathematical model for the above polymerization chemical reactions in vivo is as follows ^[14].

where $[M_i]$ is the concentration of $M_i, i = 1, 2, 3, 4, 5$.

The initial condition is

Theorem 8. The positive equilibrium point of the mathematical model for pentamer building block polymerization reaction Eq (2.27) exists and is unique.

Proof. The proof method is similar to the method of proving Theorem 6.

Theorem 9. The mathematical model of pentamer building block polymerization reaction systems (2.27) is locally asymptotic stable.

Proof. The proof method is similar to the method of proving Theorem 7.

2.5.   Hexamer building block

We consider that the hexamer is the building block of a kind of virus assembly in vivo. The example is shown in Figure 1(E). The polymerization reactions are as follows.

where $M_i$ is the protein with $i$ monomers, $k_i^+$ is the on-rate constant, $k_i^-$ is the off-rate constant $i = 1, 2, ..., 6$, $\mu$ is the production rate of the monomers, and $r$ is the assemble rate of the virus.

The mathematical model for the above polymerization chemical reactions in vivo is as follows ^[14].

where $[M_i]$ is the concentrations of $M_i, i = 1, 2, 3, 4, 5, 6$.

The initial condition is

Theorem 10. The positive equilibrium point of the mathematical model for hexamer building block polymerization reaction Eq (2.29) exists and is unique.

Proof. The proof method is similar to the method of proving Theorem 6.

Theorem 11. The mathematical model of hexamer building block polymerization reaction systems (2.29) is locally asymptotic stable.

Proof. The proof method is similar to the method of proving Theorem 7.

3.   Conclusions and discussion

Building blocks are necessary for capsid assembly for numerous viruses. Sufficient building blocks not only provide raw materials for virus assembly, but also ensure virus assembly efficiency. Here, we focus on mathematical analysis of polymerization chemical reactions of building blocks. Often, monomer numbers in most of virus building blocks are typically less than six. That is, building blocks include dimer, trimer, tetramer, pentamer, and hexamer. We comprehensively consider all five types of building block polymerization reactions in vivo. Then, we present the corresponding mathematical models of these chemical reactions. We prove that the positive equilibrium point of each mathematical model for building block polymerization reactions exists and is unique. Furthermore, we prove that all mathematical models of building block polymerization reactions are locally asymptotic stable. Therefore, we conclude that concentrations of intermediates and building blocks will tend to be stable for any initial concentrations, respectively. Moreover, these stable concentrations of intermediates and building blocks are constants, respectively. These obtained results can provide further insight into property of virus building block polymerization chemical reactions in vivo.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This work was supported by National Natural Science Foundation of China (No. 12371487).

Conflict of interest

The authors declare there is no conflict of interest.