1.
Introduction
A production system with a similar type of multi-product is gaining attention nowadays. A traditional economic production quantity (EPQ) produces each type of product separately [1]. This process uses a machine multiple times for a similar process. Production of multi-products in a shared production system can reduce machine usage and can produce the generic structure of multi-product. Agarwal [2] introduced an easy grouping concept under a common order cycle to solve a multi-product supply chain. They introduced a computation method to find the optimal value of the common order cycle. Rosenblatt and Rothblum [3] presented a multi-item production management policy under a single resource capacity constraint. Aliyu and Andizani [4] examined a multi-item production-inventory system with shortages, deterministic demand, deterioration, and capacity and budget constraints. They used a linear quadratic concept to find the value of the optimal control policy. Balkhi and Foul [5] discussed a multi-product production model in finite time periods where shortages and backorders are allowed for every product. For every product, they derived optimal production and restarting times for each period. Rahmani et al. [6] investigated a two-stage capacity-based production system with uncertain demand and production costs. An initial robust schedule was used by them. Chiu et al. [7] proposed a production model to find the production and shipment decisions, simultaneously, with the rework process. They considered a single-stage production process without involving the common intermediate part. Their outcomes helped managers to understand and control the effects of different system parameters on the optimal production-shipment policy. Additional studies related to multi-product production-inventory systems are found in the literature [8].
The evolution of industries over the past century has been characterized by the integration of supply chains (SCs), titled a supply chain integration (SCI) [9]. The SCI activities within an organization, correspond to the suppliers, the customers and the SC levels [10]. In other words, the SCI is an organizational process to integrate the suppliers, the customers, and the internal functional units to optimize the SC's total performance of the SC [11]. Rosenzweig et al., [12] further defined the SCI as the linkages among various SC elements. Many authors discussed the SCI as a common place for SCs [13]. These integration definitions have undergone various modifications owing to research from different perspectives. The SCI aims at coordinating processes in the SCs as an important competitive advantage over competitors [14] and [15]. The experts of the supply chain management (SCM) believe that the integration leads to higher performance for SC levels [16,17,18]. Generally, the global competition and the demand for better customer services have significantly increased the needs for SCI among the companies. The most well-established frameworks for studying SC relate to lot-sizing problems [19].
2.
Literature Review
Gharaei et al. [20] proposed the growth patterns for all dead and live-grown items, along with mortality and survival probabilities. Gharaei et al. [21] developed and optimized a lot-sizing policy in an integrated EPQ model with partial backorders and re-workable products. They considered linear and fixed backordering costs. Gharaei et al. [22] designed and optimized an integrated four-level SC, which contained a supplier, a producer, a wholesaler, and multiple retailers. Gharaei et al. [23] provided a new generation of inventory models, entitled economic growing quantity (EGQ), which focused on growing items of agricultural industries, such as fisheries, poultry, and livestock. Gharaei et al. [24] addressed the optimum number of stockpiles and the economic period length for inventories. Amjadian et al. [25] designed an integrated five-level SC, which contained a supplier, a producer, a wholesaler, multiple retailers, and a collector. Accordingly, a closed-loop supply chain (CLSC) with multi-stage products were designed by them with respect to the green production principles and quality control (QC) policy under backlogged and lost sale. Taleizadeh et al. [26] described optimal decisions and operational strategies in a logistics network considering two capital-constrained manufacturers. They produced products of different qualities, and sold them to a retailer with deterministic demand over a specific period. Gharaei et al. [27] proposed a multi-product, multi-buyer SC model with stochastic constraints. Moreover, the model differentiated between the holding costs for financial and non-financial components, in which the first included the investment in the market, and the second included the cost for physical storage, movement, and insurance of products.
In multi-item production system, if multiple products share a common intermediate part, vendors can be interested in evaluating a two-stage production scheme. The first-stage makes common intermediate parts and the second stage produces end products to reduce overall system costs and shorten the replenishment cycle time. Reduce costs along with shortening the refill cycle period. Gerchak et al. [28] created a model for an arbitrary number of products with a normal demand distribution. They explained the service level measure where the production of common components might be required. Garg and Tang [29] discussed that there are differences among similar types of multiple products. They created two replicas of products with a difference of more than one position. They decided on necessary conditions when one type of delayed differentiation was more beneficial than the other. They found that variations in demand and lead times have significant effects on determining which point of differentiation should be delayed. Graman [30] explained a two-product, single-term, order-up-to cost model to decide inventory levels of end products and postponement capacity. Non-linear programming was chosen to decide the optimal solutions to inventory levels and capacity that minimized the system costs. The study indicated that altering product value, holding cost, cost of postponement, packaging cost, and fill rate reduced expected total cost and increased postponement capacity. Other studies addressed various aspects of the multi-product production management system [31]. It is inevitable to produce defective items due to various uncontrolled factors in the production process. Quality assurance, quality inspections, rework, and elimination of imperfect items, are studied in several studies [32]. In contrast to a continuous review model, a period review model is important within a multi-product-based production system. Several aspects of the periodic review model and multi-shipment issues are discussed in the literature too [33].
Mukherjee et al. [34] estimated maximum product flow within a cross-dock. Mridha et al. [35] discussed a green product manufacturing system but did not discuss a multi-product system. Habib et al. [36] discussed a green product manufacturing system where raw materials were collected from multi-type waste products. Sarkar et al. [37] proposed a model that aimed to reduce waste by reworking defective products and maximizing profit. Saxena et al. [38] proposed an SC model for a single type of eco-designed product and solved the model using the Stackelberg-Nash game policy. Bachar et al. [39] described a production model where partial outsourcing of products was allowed to remove shortages from the system. Discussed studies formulated production and SC model single type of products without shared-production facility. This model expands on the earlier work of Chiu [7] for a period-review model flexible production system (Figure 1).
3.
Problem definition
The proposed model describes a flexible production system integrated with shared-production techniques and remanufacturing. The flexible production system has a single machine. The vendor's annual demand is ∑Mi=1δi for M number of different products. These M customized items are made using a two-stage shared-production system. Stage 1 makes only common components, and Stage 2 produces the final product with the rest of the components within sequence M. This two-stage production system has a common cycle time. The study aims to reduce machine usage by reducing the replenishment period and optimizing production quantity. The common parts are produced at the rate of q1,0 in Stage 1. Then, M different customized products are assembled (Figure 1) at a production rate q1,i. Here, i=0,1,2,...,M and i=0 indicates the shared-production process of Stage 1.
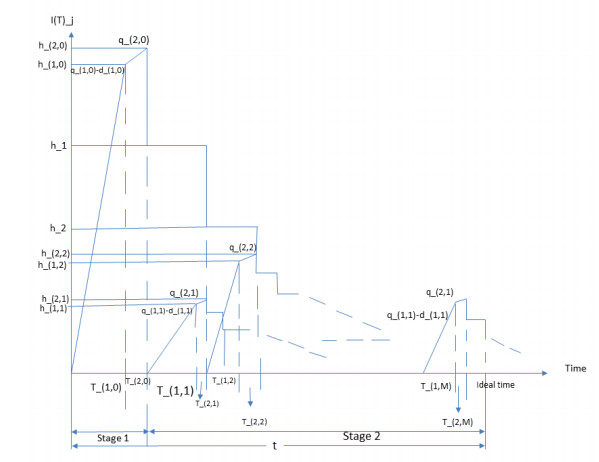
Material and development costs of each product are added in unit production cost of product i for production and remanufacturing as Fi=(Cm1,i+CD1,iq1,i+αq1,i)+(Cm2,i+CD2,iq2,i+αq2,i). The production process at each Stage randomly produce yi portion of defective products at the rate g1,i, where g1,i = q1,iyi. Production rate q1,i of Stage 2 is greater than (δi+g1,i), i.e., (q1,i−g1,i−δi)>0, i.e., (1−yi−δiq1,i)>0. All defective products are remanufactured in each stage. The remanufacturing process begins at a rate q2,i as soon as the production process ends in both stages (Figure 2).
Common components of all products are manufactured in Stage 1 in time T1,0 and remanufactured imperfect products at time T2,0. After completion of production and remanufacturing in Stage 1, M products are ready for the Stage 2. Total inventory from shared-production facility is represented in Figure 3. Production in Stage 2 happens in succession order, from i=1 to M. In Stage 2, customized production of all products takes (T1,i) time for product i and remanufacturing of finished products requires T2,i times. Then, products are sent for delivery in N number of shipments at time T3,i (Figure 4). The supply level of finished products from the flexible production system is represented in Figure 4.
4.
Notation
5.
Mathematical modeling
This section describes the mathematical modeling and total cost analysis of these study.
5.1. Illustration and investigation
A two-stage flexible production model produces M distinct multi-product with annual market demand δi. The production cycle is (Figure 1)
Stage 1 produces common components of all products in a lot size A0. It depends on the production batch Ai of product i. Then, the following (Figure 1) equations are found:
In Stage 2 (i=1,2,...,M), the following equations are found from Figures 2 to 4.
5.2. Cost analysis
Different costs for the two-stage flexible production system are developed as follows.
5.2.1. Setup cost (SEC)
Total setup cost is the sum of the setup amount for Stage 1 and Stage 2 for item i in a production cycle. Therefore, total setup cost for the production process can be formulated as
5.2.2. Production cost (PRC)
Unit production cost depends on metrical cost, development cost, and production rate, and remanufacturing rate of product i. Thus, the unit production cost of the product i for both Stages are given by
5.2.3. Remanufacturing cost (REC)
Imperfect products are produced through the production process of both stages for the product i. Those imperfect products are remanufactured right after the production process are finished. The corresponding remanufacturing cost is
5.2.4. Safety stock cost (SSC)
To overcome the stock out situation, some safety stock is required. Imperfect products are not send to the market as new products. The manufacturer uses the remanufactured products as safety stock to avoid shortages.
5.2.5. Inventory holding cost for new common components in Stage 1 (IHC)
IHC is used for holding common components, both manufactured and remanufactured product i, throughout T1,i and T2,i (Figures 1 and 2). Thus, the inventory holding cost is
5.2.6. Inventory holding cost for new customized products in Stage 2 (IHCF)
In Stage 2, IHCF is used for holding the production of customized product i (Figure 3). The associative cost is written as
5.2.7. Inventory holding cost of all remanufactured items (IHCI)
IHCI is used for holding imperfect products after remanufacturing until the time T2,i. The corresponding holding cost is
5.2.8. Holding cost of perfect customized products in Stage 2 (HRR)
Total perfect customized products after production and remanufacturing are stored until the time T2,i for product i. Besides, number of reworked items are stored until time T3,i. Total holding cost for perfect customized products is
5.2.9. Holding cost of defective customized products in Stage 2 (HCDI)
Defective customized product i is stored in every production cycle until the production up time T1,i. HCDIis given as follows:
5.2.10. Holding cost of average inventory for perfect customized items in Stage 2 (HCMQ)
Thus, the average holding cost of customized new items at the end of the production up time T1,i is HCMQ, which can be expressed as
5.2.11. Stock holding cost (SHC)
After finishing the production in two-stages, all finished products are stored for distribution. Then, products are sent in shipments. After sending product in shipment, other products are still stored. Thus, SHC is used to hold finished product i after production (Figure 4). Associative stock holding cost is
5.2.12. Fixed and variable delivery cost (FVD)
After Stage 2, finished products are sent to the market in N number of shipments. FVD is used for fixed transportation cost and IHC is used for variable transportation cost in T3,i. Corresponding transportation cost is
5.2.13. Expected total cost
The total cost (TC) of the flexible production system is TC(t,N,q1,i,q2,i), which can be written as
This is a period review model, i.e., inventory is checked in a certain time period. Substituting Eqs (5.1) to (5.16) in Eq (5.30), expected total cost (E[TCU]) for M number of products per cycle can be obtained as below.
Eq (5.31) states the expected total cost of the proposed production system. There are four decision variables t,N,q1,i, and q2,i. The paper gives a unique solution to the problem and finds the best strategy for the flexible production system.
6.
Solution methodology
A classical optimization technique is used to obtain the total cost E[TCU]. Solutions of decision variables are found by using first order derivatives. The convex nature of the objective function in Eq (5.31) are proved by the Hessian matrix. First order partial derivatives of Eq (5.31) with respect to t,N,q1,i and q2,i are given below.
First order derivatives in Eqs (6.1), (6.6), (6.10), and (6.13) give unique solutions after equating the equations to zero (necessary condition of classical optimization). Thus, unique solutions t∗,N∗,q∗1,i, and q∗2,i are
[See Appendix 1 for all the values]
The following proposition proves that the ETC cost of the flexible production system is a global minimum.
Proposition: Expected total cost of the production system in Eq (5.31) has a global minimum value at t∗,N∗,q∗1,i, and q∗2,i if the values principal minors of order one (H11), two (H22), three (H33), and four (H44) of the fourth order Hessian matrix are greater than zero.
Proof: The Hessian matrix of order four can be written as
The first order principal minor is
H11=∂2E∂t∗2=2B0t3+∑Mi=1(2Bit3+2NB1,it3)>0.
The first order principal minor is
H11=2B0t3+∑Mi=1(2Bit3+2NB1,it3)>0.
The second order principal minor is
H22=∂2E∂t∗2∂2E∂N∗2−(∂2E∂t∗∂N∗)2=(2B0t3+∑Mi=1(2Bit3+2NB1,it3))(∑Mi=1(−H1,itδ2iγ1,iN3+H3,itδ2iγ1,iN3))−(∑Mi=1(−B1,it2+H1,iγ1,iδ2i2N2−H3,iδ2iγ1,i2N2))2>0.
The third order principal minor is
H33=∂2E∂N∗2det(H22)−(∂2E∂t∗∂N∗)2(∂2E∂q∗1,i2)>0.
The fourth principal minor is
H44=∂2E∂q∗2,i2det(H33)−(∂2E∂t∗∂q∗2,i)2(∂2E∂N∗2)(∂2E∂q∗1,i2)>0.
Therefore, one can conclude that the unique solutions of the objective function provides a global minimum cost.
7.
Numerical example and discussions
The numerical examples are provided to investigate the outcomes of the mathematical model. Five distinct products are produced with a common component manufacturing rate β=q2,iq1,i. Associative input data are taken from Chiu et al. [7]. Annual demand of five products are δ1 = 3000 units/year, δ2 = 3200 units/year, δ3 = 3400 units/year, δ4 = 3,600 units/year, and δ5 = 3800 units/year. A linear relationship 1β is assumed for these relevant manufacturing rates. The relationship between the relevant amount of the common components and the participation rate β can be linear or nonlinear. All cases are investigated in the following subsections.
7.1. Case 1. Investigation of linear participation rate for the production of common components
The correlation between the common components production and the customized production of products is linear with the participation rate β = 0.5. Setup cost of Stage 1 (B0) = $8500/setup, remanufacturing cost of Stage 1 (FR,0) = $25/unit, holding cost (H1,0) = $5/unit/unit time, holding cost for safety stock cost for Stage 1 (H4,0) = $5/unit/unit time. Unit holding cost H1,1 = $10/unit/unit time, H1,2 = $15/unit/unit time, H1,3 = $20/unit/unit time, H1,4 = $25/unit/unit time, and H1,5 = $30/unit/unit time. Holding cost for remanufactured products for Stage 1 (H2,0) = $15/unit/unit time. Setup cost for Stage 2 are B1 = $8500/setup, B2 = $9000/setup, B3 = $9500/setup, B4 = $10,000/setup, B5 = $10,500/setup. Random defective rate in Stage 1 follows uniform distribution y0∼U[0, 0.04].
q1,i=11/q1,i−1/q1,0. Random defective rate in Stage 2 follows uniform distribution y1∼U[0, 0.01], y2∼U[0, 0.06], y3∼U[0, 0.11], y4∼U[0, 0.16], and y5∼U[0, 0.21]. Unit remanufacturing costs of Stage 2 are FR,1 = $25/unit, FR,2 = $30/unit, FR,3 = $35/unit, FR,4 = $40/unit, and FR,5 = $45/unit. q2,i=11/q2,i−1/q2,0. Unit holding cost of remanufactured product for Stage 2 are H2,1 = $30/unit/unit time, H2,2 = $35/unit/unit time, H2,3 = $40/unit/unit time, H2,4 = $45/unit/unit time, and H2,5 = $50/unit/unit time. Fixed delivery cost per shipment are B1,1 = $1800/shipment, B1,2 = $1900/shipment, B1,3 = $2000/shipment, B1,4 = $2100/shipment, and B1,5 = $2200/shipment. Unit variable delivery cost are FT,1 = $0.1/unit, FT,2 = $0.2/unit, FT,3 = $0.3/unit, FT,4 = $0.4/unit, and FT,5 = $0.5/unit. Holding cost of finished product after Stage 2 are H3,1 = $70/unit/unit time, H3,1 = $75/unit/unit time, H3,3 = $80/unit/unit time, H3,4 = $85/unit/unit time, and H3,5 = $90/unit/unit time. Holding cost of safety stock for Stage 2 are H4,1 = $10/unit/unit time, H4,2 = $15/unit/unit time, H4,3 = $20/unit/unit time, H4,4 = $25/unit/unit time, and H4,5 = $30/unit/unit time.
Annual demand for common components of products is δ0 = 17,000 units, which is obtained by applying Eqs (5.2) and (5.3). Then, by using Eqs (6.15) to (6.18), the optimum shipment number is obtained as N∗ = 4, optimum production cycle time t∗ = 0.6785 years, optimum production rate of Stage 1 q1,0=104,368unit/year, q1,1112,258 unit/year, q1,2 = 116,066 unit/year, q1,3 = 120,000 unit/year, q1,4 = 124,068 unit/year, and q1,5 = 128,276 units unit/year, optimum remanufacturing rate of of Stage 2 q2,0=85,752unit/year, q2,1 = 89,806 units/year, q2,2 = 92,852 units/year, q2,3 = 96,000 units/year, q2,4 = 99,254 units/year, and q2,5 = 102,621 units/year and the expected total cost is E[TCU] = $107,471,000/cycle. When the participation rate β rises, the total cost E[TCU] decreases 3.76% at β = 0.5 (total cost decreases from $111,511,910/cycle (β=1) to $107,471,000/cycle). These analytic results show that the expected total cost is a significantly useful investigation for manufacturers who produce multiple items through a shared-production facility. As the participation rate β=q2,iq1,i rises, the optimum cycle period t∗ reduces significantly. The optimum cycle period t∗ is decreased by 25.5% at β = 0.5 (declines from 0.8515 years (β=1) to 0.6785 years). Results indicate that the proposed two-stage multi-product flexible production system provides a reduced cycle length than with global minimum cost.
7.2. Case 2: Nonlinear correlation of applicable variables
This investigation examines the nonlinear relationship between shared-production and customized production with a participation rate β=q2iq1,i. Hence it has a more production rate than a linear participation rate. Using the new relation, parametric values are FR,0 = $40/unit, B0 = $13,493/setup, H1,0 = H4,0 = $8/unit time, H2,0 = $24/unit/unit time. Other parameters remain identical as expressed in Subsection 7.1. y0∼U[0, 0.04]. Therefore, Bi = $3507/setup, $4007/setup, $4507/setup, $5007/setup, and $5507/setup. FR,i = $10/unit, $15/unit, $20/unit, $25/unit, and $30/unit, and yi follows a uniform distribution with the interval [0, 0.01], [0, 0.06], [0, 0.11], [0, 0.16], and [0, 0.21], for five products, respectively.
If β1/3 is the nonlinear relation, then F0 = β1/3F1 = $63/unit. Using Eqs (6.15) to (6.18) and (5.31), one can get the optimum numeral values of the shipment N∗ = 4, optimum production cycle time t∗ = 0.6005 (years), optimum production rate q1,0 = 101,821 unit/year, q1,1 = 105,272 unit/year, q1,2 = 109,518 unit/year, q1,3 = 113,233 unit/year, q1,4 = 117,072 unit/year, q1,5 = 125,146 unit/year, optimum remanufacturing rate q2,0 = 83,659 unit/year, q2,1 = 87,614 unit/year, q2,2 = 90,586 unit/year, q2,3 = 93,657 unit/year, q2,4 = 96,832 unit/year, q2,5 = 100,117 unit/year, and the expected total cost is E[TCU] = $104,837,961/cycle. For the non-linear relationship of β, when β increases, total cost E[TCU] decreases and it decreases by 2.45% (i.e., the total cost reduces from $107,471,000/cycle for β = 0.5, to $104,837,961/cycle) correlated to the initial linear occurrence. For the nonlinear case, optimum cycle time t∗ decreases by 13.20% than the linear relationship β = 0.5 (it reduces from 0.6785 years to 0.5889 years). Hence, it shows that the proposed two-stage multi-product flexible production system is significantly useful for manufacturers for a short replenishment cycle. The manufacturer can provide multiple products with less cycle time. The analytic outcomes reveal that the shared-production has a higher cost than the customized production system. Besides, a nonlinear participation β1/3 provides less system cost than a linear relation. But, the optimum cycle period t∗ reduces significantly for a non-linear participation rate.
8.
Managerial implications
The managers aim to achieve a less cost-sensitive production system such that the system cost becomes low. In a high price-sensitive system, market demand decreases with a few price increases. The risk of borrowing from the online platform increases for high-price-sensitive products. Besides, a long cycle time can increase the risk of lost sales for a cost-sensitive system. Thus, a shared-production facility along with a flexible production system solve the problem by adjusting production and remanufacturing rate within a reduced cycle time. Thus, industry managers can reduce the risk of lost sales due to a flexible production system.
9.
Conclusions
A shared-production facility-based flexible production was discussed where multi-products were produced. The production system was a two-stage facility where each stage had a production and remanufacturing process. Multi-products were produced in the production process and imperfect products were remanufactured after finishing the production process. Both the production and remanufacturing processes had a single flexible machine. Thus, the shared production helped to produce common components of all products in Stage 1 and Stage 2 finished the rest. Results showed that the participation ratio of shared-production in the production process had a major impact on the system's cost and production cycle time. If the production cost of Stage 1 and Stage 2 became independent of one another, then the system cost was maximum. If the production cost of Stage 1 is linearly dependent on Stage 2, then the production cost of Stage 1 became less than Stage 2, and both the cycle time along with system cost were reduced. But, the maximum reduction in cost and cycle time happened when the relation β became non-linear. The flexible production system supported the whole process as the reduction of cycle time implies a fast production process in less amount of time. Adjustment of production and remanufacturing rate of the flexible production system helped the manager to decide on the new reduced cycle time. The present model developed a flexible production model by considering simultaneous scheduling and lot-sizing with a single machine. This study can be extended using parallel flexible machines [40]. The study can be extended for a supply chain scenario with multiple buyers. Moreover, consideration of uncertainty within the market demand will make the model more practical. Instead of linear relation [41], future research can be conducted using nonlinear control theory techniques [42,43]. Environmental issue of carbon emissions can be considered within the proposed system [44].
Acknowledgments
This research is not funded through any source.
Conflict of interest
There are no conflicts of interest.
Appendix 1
A1=(H1,iδ2i2(γ2,i−γ1,iN)+H2,iδ2iE[yi]22q2,i+H3,iδ2i2(1q1,i+E[yi]q2,i+γ1,iN)+H4,iδiE[yi]
B1=δiCD1,i2N+H3,itδ2iN+H1,itδ2iN+H1,itδ2i−H3,itδ2iN
C1=δiCD2,i2N+H3,itδ2iNE[yi]+H1,itδ2iNE[yi]+H1,itδ2iE[yi]−H3,itδ2i2E[yi]+NH2,itδ2iE[y2i]−NH1,itδ2iE[y2i]









 DownLoad:
DownLoad: