Coronary artery centerline extraction in cardiac computed tomography angiography (CTA) is an effectively non-invasive method to diagnose and evaluate coronary artery disease (CAD). The traditional method of manual centerline extraction is time-consuming and tedious. In this study, we propose a deep learning algorithm that continuously extracts coronary artery centerlines from CTA images using a regression method. In the proposed method, a CNN module is trained to extract the features of CTA images, and then the branch classifier and direction predictor are designed to predict the most possible direction and lumen radius at the given centerline point. Besides, a new loss function is developed for associating the direction vector with the lumen radius. The whole process starts from a point manually placed at the coronary artery ostia, and terminates until tracking the vessel endpoint. The network was trained using a training set consisting of 12 CTA images and the evaluation was performed using a testing set consisting of 6 CTA images. The extracted centerlines had an average overlap (OV) of 89.19%, overlap until first error (OF) of 82.30%, and overlap with clinically relevant vessel (OT) of 91.42% with manually annotated reference. Our proposed method can efficiently deal with multi-branch problems and accurately detect distal coronary arteries, thereby providing potential help in assisting CAD diagnosis.
Citation: Xintong Wu, Yingyi Geng, Xinhong Wang, Jucheng Zhang, Ling Xia. Continuous extraction of coronary artery centerline from cardiac CTA images using a regression-based method[J]. Mathematical Biosciences and Engineering, 2023, 20(3): 4988-5003. doi: 10.3934/mbe.2023231
| [1] | Sumati Kumari Panda, Abdon Atangana, Juan J. Nieto . New insights on novel coronavirus 2019-nCoV/SARS-CoV-2 modelling in the aspect of fractional derivatives and fixed points. Mathematical Biosciences and Engineering, 2021, 18(6): 8683-8726. doi: 10.3934/mbe.2021430 |
| [2] | A. D. Al Agha, A. M. Elaiw . Global dynamics of SARS-CoV-2/malaria model with antibody immune response. Mathematical Biosciences and Engineering, 2022, 19(8): 8380-8410. doi: 10.3934/mbe.2022390 |
| [3] | Tahir Khan, Roman Ullah, Gul Zaman, Jehad Alzabut . A mathematical model for the dynamics of SARS-CoV-2 virus using the Caputo-Fabrizio operator. Mathematical Biosciences and Engineering, 2021, 18(5): 6095-6116. doi: 10.3934/mbe.2021305 |
| [4] | A. M. Elaiw, Raghad S. Alsulami, A. D. Hobiny . Global dynamics of IAV/SARS-CoV-2 coinfection model with eclipse phase and antibody immunity. Mathematical Biosciences and Engineering, 2023, 20(2): 3873-3917. doi: 10.3934/mbe.2023182 |
| [5] | Wenhan Guo, Yixin Xie, Alan E Lopez-Hernandez, Shengjie Sun, Lin Li . Electrostatic features for nucleocapsid proteins of SARS-CoV and SARS-CoV-2. Mathematical Biosciences and Engineering, 2021, 18(3): 2372-2383. doi: 10.3934/mbe.2021120 |
| [6] | Junyuan Yang, Guoqiang Wang, Shuo Zhang . Impact of household quarantine on SARS-Cov-2 infection in mainland China: A mean-field modelling approach. Mathematical Biosciences and Engineering, 2020, 17(5): 4500-4512. doi: 10.3934/mbe.2020248 |
| [7] | Rahat Zarin, Usa Wannasingha Humphries, Amir Khan, Aeshah A. Raezah . Computational modeling of fractional COVID-19 model by Haar wavelet collocation Methods with real data. Mathematical Biosciences and Engineering, 2023, 20(6): 11281-11312. doi: 10.3934/mbe.2023500 |
| [8] | Somayeh Fouladi, Mohammad Kohandel, Brydon Eastman . A comparison and calibration of integer and fractional-order models of COVID-19 with stratified public response. Mathematical Biosciences and Engineering, 2022, 19(12): 12792-12813. doi: 10.3934/mbe.2022597 |
| [9] | Ali Al Khabyah, Muhammad Kamran Jamil, Ali N. A. Koam, Aisha Javed, Muhammad Azeem . Partition dimension of COVID antiviral drug structures. Mathematical Biosciences and Engineering, 2022, 19(10): 10078-10095. doi: 10.3934/mbe.2022471 |
| [10] | Darrak Moin Quddusi, Sandesh Athni Hiremath, Naim Bajcinca . Mutation prediction in the SARS-CoV-2 genome using attention-based neural machine translation. Mathematical Biosciences and Engineering, 2024, 21(5): 5996-6018. doi: 10.3934/mbe.2024264 |
Coronary artery centerline extraction in cardiac computed tomography angiography (CTA) is an effectively non-invasive method to diagnose and evaluate coronary artery disease (CAD). The traditional method of manual centerline extraction is time-consuming and tedious. In this study, we propose a deep learning algorithm that continuously extracts coronary artery centerlines from CTA images using a regression method. In the proposed method, a CNN module is trained to extract the features of CTA images, and then the branch classifier and direction predictor are designed to predict the most possible direction and lumen radius at the given centerline point. Besides, a new loss function is developed for associating the direction vector with the lumen radius. The whole process starts from a point manually placed at the coronary artery ostia, and terminates until tracking the vessel endpoint. The network was trained using a training set consisting of 12 CTA images and the evaluation was performed using a testing set consisting of 6 CTA images. The extracted centerlines had an average overlap (OV) of 89.19%, overlap until first error (OF) of 82.30%, and overlap with clinically relevant vessel (OT) of 91.42% with manually annotated reference. Our proposed method can efficiently deal with multi-branch problems and accurately detect distal coronary arteries, thereby providing potential help in assisting CAD diagnosis.
New insights on novel coronavirus 2019-nCoV/SARS-CoV-2 modelling in the aspect of fractional derivatives and fixed points by Sumati Kumari Panda, Abdon Atangana and Juan J. Nieto. Mathematical Biosciences and Engineering, 2021, 18(6): 8683-8726. doi: 10.3934/mbe.2021430.
We would like to submit the following corrections to our recently published paper [1]. We also corrected some typos in the text.
First and second paragraphs in Section 2 (An extended orthogonal space and fixed points) has been updated.
The accessible collected works show that there is a clear connection between Metric Fixed Point Theory and many academic fields including but not limited to engineering, sciences, and technology. One of the clear applications of this is found in the theory of ordinary and partial differential equations. These equations are used to model real-world problems, thus their exact solutions are requested to be compared with experimental data. However on many occasions, exact solutions are impossible to be obtained, thus researchers relied on numerical approximation to provide a numerical solution that can be used for comparison with data. However, it is at least useful to provide conditions under which the model has an exact solution, in this case, metric fixed point theory is very useful. Several extensions or generalizations have been made and extensions have been done to capture problems that are more complex.
First paragraph in Section 3 (Existence of solutions for novel coronavirus 2019-nCoV/SARS-CoV-2 model in fractional scenario) have been updated.
Although humans have advanced their technologies and their medicine to protect the mankind, it is important to note that there are still diseases that continue to spread across the world that up to now humans have not found a permanent solution to eradicating these diseases. A few examples can be noted including HIV, malaria, Ebola, and other well-known infectious diseases that continue to take much life daily. In addition to these existing diseases, humans continue to be surprised by new ones that lead them to double their efforts to make their environment more saver, however, diseases are known to be the main source of death across the world. For example, information released by the WHO in 2001 indicated that diseases have added 26% of global mortality. From 2003, the world has witnessed a rise of different types of viruses for example SARS-CoV in 2003, Mers-CoV in 2012, Ebola in 2014, and now SARS-CoV-2 and others that are not listed here. Researchers from different backgrounds have investigated the biological structures, mode of transmission, and life span of these viruses. On the other hand, to protect life and livelihood medical bodies have developed several vaccines that could help prevent the spread of these diseases. Nevertheless, to have an idea of what could happen in a near future, mathematicians have developed some mathematical models that could be used first to provide the reproductive number of these diseases, second to predict the numbers of infected, recovery, and death cases. This was also valid with the breakout of the new virus known as covid-19, several investigations have been done, vaccines have been developed and some mathematical models have been proposed in the last two years with the aim to predict future behaviors of the spread. It's important to note that the use of mathematical modeling in the analysis of epidemiological disorders has risen significantly. In this work, we propose solutions for fractional-order models of the novel coronavirus 2019-nCoV/SARS-CoV-2.
First paragraph in Section 4.1 (Atangana-Baleanu fractional derivative sense) have been updated.
It has been recognized that dynamical spread exhibits nonlocal behaviors resembling processes like that of a power-law, fatigue, and crossover. These patterns are well described by some mathematical functions, for example, the power-law function is known to replicate power-law behaviors as time and power change. The fatigue effect can be well explained using an exponential decay function. The generalized Mittag-Leffler has been recognized to depict a passage from stretched fatigue effect to power-law. From these functions and the convolution, concepts of fractional differentiation and integration have been introduced and applied in many problems in the last decades. In this section, we investigate the model of 2019-nCoV/SARS-CoV-2 using differential operators based on the generalized Mittag-Leffler kernel.
| [1] | World Health Organization, The top 10 causes of death, 2020. Available from: https://www.who.int/en/news-room/fact-sheets/detail/the-top-10-causes-of-death. |
| [2] |
F. Cademartiri, L. La Grutta, A. Palumbo, P. Malagutti, F. Pugliese, W. B. Meijboom, et al., Non-invasive visualization of coronary atherosclerosis: state-of-art, J. Cardiovasc. Med., 8 (2007), 129–137. https://doi.org/10.2459/01.JCM.0000260820.40145.a8 doi: 10.2459/01.JCM.0000260820.40145.a8
|
| [3] |
G. Mowatt, E. Cummins, N. Waugh, S. Walker, J. Cook, X. Jia, et al., Systematic review of the clinical effectiveness and cost-effectiveness of 64-slice or higher computed tomography angiography as an alternative to invasive coronary angiography in the investigation of coronary artery disease, Health Technol. Assess., 12 (2008), 3–143. https://doi.org/10.3310/hta12170 doi: 10.3310/hta12170
|
| [4] |
A. W. Leber, A. Becker, A. Knez, F. von Ziegler, M. Sirol, K. Nikolaou, et al., Accuracy of 64-slice computed tomography to classify and quantify plaque volumes in the proximal coronary system—A comparative study using intravascular ultrasound, J. Am. Coll. Cardiol., 47 (2006), 672–677. https://doi.org/10.1016/j.jacc.2005.10.058 doi: 10.1016/j.jacc.2005.10.058
|
| [5] | A. Kanitsar, D. Fleischmann, R. Wegenkittl, P. Felkel, M. E. Grö ller, CPR-curved planar reformation, in IEEE Visualization, 2002. VIS 2002, (2002), 37–44. https://doi.org/10.1109/VISUAL.2002.1183754 |
| [6] |
H. S. Hecht, Applications of multislice coronary computed tomographic angiography to percutaneous coronary intervention: How did we ever do without it, Catheterization Cardiovasc. Interventions, 71 (2008), 490–503. https://doi.org/10.1002/ccd.21427 doi: 10.1002/ccd.21427
|
| [7] |
M. F. Khan, S. Wesarg, J. Gurung, S. Dogan, A. Maataoui, B. Brehmer, et al., Facilitating coronary artery evaluation in MDCT using a 3D automatic vessel segmentation tool, Eur. Radiol., 16 (2006), 1789–1795. https://doi.org/10.1007/s00330-006-0159-8 doi: 10.1007/s00330-006-0159-8
|
| [8] | J. S. Shinbane, S. S. Mao, M. J. Girsky, R. J. Oudiz, S. Carson, J. Child, et al., Computed tomographic angiography can define three-dimensional relationships between coronary veins and coronary arteries relevant to coronary venous procedures, Circulation, 110 (2004), 702. |
| [9] |
Y. Shen, Y. Gao, P. Zhang, W. Yu, S. Zhou, B. Li, et al., Research on CCTA coronary lumen segmentation based on Polar1DMLP model, CT Theory Appl. Res., 29 (2020), 631–642. https://doi.org/10.15953/j.1004-4140.2020.29.06.01 doi: 10.15953/j.1004-4140.2020.29.06.01
|
| [10] | M. Umer, S. Sharma, P. Rattan, A survey of deep learning models for medical image analysis, in 2021 International Conference on Computing Sciences (ICCS), (2021), 65–69. https://doi.org/10.1109/iccs54944.2021.00021 |
| [11] | W. Huang, L. Huang, Z. Lin, S. Huang, Y. Chi, J. Zhou, et al., Coronary artery segmentation by deep learning neural networks on computed tomographic coronary angiographic images, in 2018 40th Annual international conference of the IEEE engineering in medicine and biology society (EMBC), (2018), 608–611. https://doi.org/10.1109/embc.2018.8512328 |
| [12] |
F. Isensee, P. F. Jaeger, S. A. A. Kohl, J. Petersen, K. H. Maier-Hein, nnU-Net: A self-configuring method for deep learning-based biomedical image segmentation, Nat. Methods, 18 (2021), 203–211. https://doi.org/10.1038/s41592-020-01008-z doi: 10.1038/s41592-020-01008-z
|
| [13] |
D. Jia, X. Zhuang, Learning-based algorithms for vessel tracking: A review, Comput. Med. Imaging Graphics, 89 (2021), 101840. https://doi.org/10.1016/j.compmedimag.2020.101840 doi: 10.1016/j.compmedimag.2020.101840
|
| [14] |
A. Dorobanțiu, V. Ogrean, R. Brad, Coronary centerline extraction from CCTA using 3D-UNet, Future Int., 13 (2021), https://doi.org/10.3390/fi13040101 doi: 10.3390/fi13040101
|
| [15] | S. Rjiba, T. Urruty, P. Bourdon, C. Fernandez-Maloigne, R. Delepaule, L. P. Christiaens, et al., CenterlineNet: Automatic coronary artery centerline extraction for computed tomographic angiographic images using convolutional neural network architectures, in 2020 Tenth International Conference on Image Processing Theory, Tools and Applications (IPTA), (2020), 1–6. https://doi.org/10.1109/IPTA50016.2020.9286458 |
| [16] |
J. M. Wolterink, R. W. van Hamersvelt, M. A. Viergever, T. Leiner, I. Išgum, Coronary artery centerline extraction in cardiac CT angiography using a CNN-based orientation classifier, Med. Image Anal., 51 (2019), 46–60. https://doi.org/10.1016/j.media.2018.10.005 doi: 10.1016/j.media.2018.10.005
|
| [17] | A. Mostafa, A. M. Ghanem, M. El-Shatoury, T. Basha, Improved centerline extraction in fully automated coronary ostium localization and centerline extraction framework using deep learning, in 2021 43rd Annual International Conference of the IEEE Engineering in Medicine & Biology Society (EMBC), (2021), 3846–3849. https://doi.org/10.1109/EMBC46164.2021.9629655 |
| [18] | H. Yang, J. Chen, Y. Chi, X. Xie, X. Hua, Discriminative coronary artery tracking via 3D CNN in cardiac CT angiography, in International Conference on Medical Image Computing and Computer-assisted Intervention, 11765 (2019), 468–476. https://doi.org/10.1007/978-3-030-32245-8_52 |
| [19] | R. Girshick, Fast R-CNN, in 2015 IEEE International Conference on Computer Vision, (2015), 1440–1448. https://doi.org/10.1109/iccv.2015.1697 |
| [20] |
C. Zhou, H. P. Chan, A. Chughtai, S. Patel, L. M. Hadjiiski, J. Wei, et al., Automated coronary artery tree extraction in coronary CT angiography using a multiscale enhancement and dynamic balloon tracking (MSCAR-DBT) method, Comput. Med. Imaging Graphics, 36 (2012), 1–10. https://doi.org/10.1016/j.compmedimag.2011.04.001 doi: 10.1016/j.compmedimag.2011.04.001
|
| [21] |
D. Han, H. Shim, B. Jeon, Y. Jang, Y. Hong, S. Jung, et al., Automatic coronary artery segmentation using active search for branches and seemingly disconnected vessel segments from coronary CT angiography, Plos One, 11 (2016), https://doi.org/10.1371/journal.pone.0156837 doi: 10.1371/journal.pone.0156837
|
| [22] |
M. Schaap, C. T. Metz, T. van Walsum, A. G. van der Giessen, A. C. Weustink, N. R. Mollet, et al., Standardized evaluation methodology and reference database for evaluating coronary artery centerline extraction algorithms, Med. Image Anal., 13 (2009), 701–714. https://doi.org/10.1016/j.media.2009.06.003 doi: 10.1016/j.media.2009.06.003
|
| [23] |
W. G. Austen, J. E. Edwards, R. L. Frye, G. G. Gensini, V. L. Gott, L. S. Griffith, et al., A reporting system on patients evaluated for coronary artery disease, Report of the Ad Hoc Committee for Grading of Coronary Artery Disease, Council on Cardiovascular Surgery, American Heart Association, Circulation, 51 (1975), 5–40. https://doi.org/10.1161/01.cir.51.4.5 doi: 10.1161/01.cir.51.4.5
|
| [24] |
Y. Geng, H. Liu, X. Wang, J. Zhang, Y. Gong, D. Zheng, et al., Effect of microcirculatory dysfunction on coronary hemodynamics: A pilot study based on computational fluid dynamics simulation, Comput. Biol. Med., 146 (2022), 105583. https://doi.org/10.1016/j.compbiomed.2022.105583 doi: 10.1016/j.compbiomed.2022.105583
|
| [25] | R. Gao, Z. Hou, J. Li, H. Han, B. Lu, S. K. Zhou, Joint coronary centerline extraction and lumen segmentation from Ccta using Cnntracker and vascular graph convolutional network, in 2021 IEEE 18th International Symposium on Biomedical Imaging, (2021), 1897–1901. https://doi.org/10.1109/isbi48211.2021.9433764 |
| [26] |
B. Jeon, Deep recursive bayesian tracking for fully automatic centerline extraction of coronary arteries in CT images, Sensors, 21 (2021), 6087. https://doi.org/10.3390/s21186087 doi: 10.3390/s21186087
|
| [27] |
P. A. L. Tonino, B. De Bruyne, N. H. J. Pijls, U. Siebert, F. Ikeno, M. vant Veer, et al., Fractional flow reserve versus angiography for guiding percutaneous coronary intervention, N. Engl. J. Med., 360 (2009), 213–224. https://doi.org/10.1056/NEJMoa0807611 doi: 10.1056/NEJMoa0807611
|
| [28] |
N. H. J. Pijls, B. de Bruyne, K. Peels, P. H. van der Voort, H. J. R. M. Bonnier, J. Bartunek, et al., Measurement of fractional flow reserve to assess the functional severity of coronary-artery stenoses, N. Engl. J. Med., 334 (1996), 1703–1708. https://doi.org/10.1056/nejm199606273342604 doi: 10.1056/nejm199606273342604
|
| [29] |
J. Knuuti, W. Wijns, A. Saraste, D. Capodanno, E. Barbato, C. Funck-Brentano, et al., 2019 ESC guidelines for the diagnosis and management of chronic coronary syndromes the task force for the diagnosis and management of chronic coronary syndromes of the European Society of Cardiology (ESC), Eur. Heart J., 41 (2020), 407–477. https://doi.org/10.1093/eurheartj/ehz425 doi: 10.1093/eurheartj/ehz425
|
| [30] | L. Yu, S. Wang, X. Li, C. W. Fu, P. A. Heng, Uncertainty-aware self-ensembling model for semi-supervised 3D left atrium segmentation, in International Conference on Medical Image Computing and Computer-assisted Intervention, (2019), 605–613. https://doi.org/10.1007/978-3-030-32245-8_67 |
| [31] | D. Nie, Y. Gao, L. Wang, D. Shen, ASDNet: Attention based semi-supervised deep networks for medical image segmentation, in International Conference on Medical Image Computing and Computer-assisted Intervention, (2018), 370–378. https://doi.org/10.1007/978-3-030-00937-3_43 |
| [32] |
A. Madani, J. R. Ong, A. Tibrewal, M. R. K. Mofrad, Deep echocardiography: data-efficient supervised and semi-supervised deep learning towards automated diagnosis of cardiac disease, NPJ Digital Med., 1 (2018), 59. https://doi.org/10.1038/s41746-018-0065-x doi: 10.1038/s41746-018-0065-x
|
| [33] |
V. Cheplygina, M. de Bruijne, J. P. W. Pluim, Not-so-supervised: A survey of semi-supervised, multi-instance, and transfer learning in medical image analysis, Med. Image Anal., 54 (2019), 280–296. https://doi.org/10.1016/j.media.2019.03.009 doi: 10.1016/j.media.2019.03.009
|
Figures(6) / Tables(4)
Xintong Wu, Yingyi Geng, Xinhong Wang, Jucheng Zhang, Ling Xia. Continuous extraction of coronary artery centerline from cardiac CTA images using a regression-based method[J]. Mathematical Biosciences and Engineering, 2023, 20(3): 4988-5003. doi: 10.3934/mbe.2023231
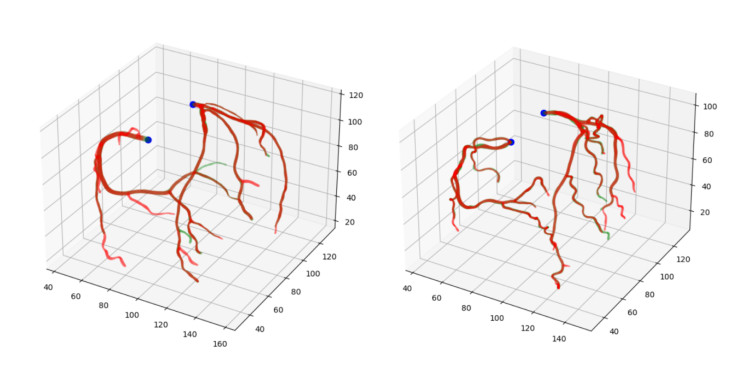
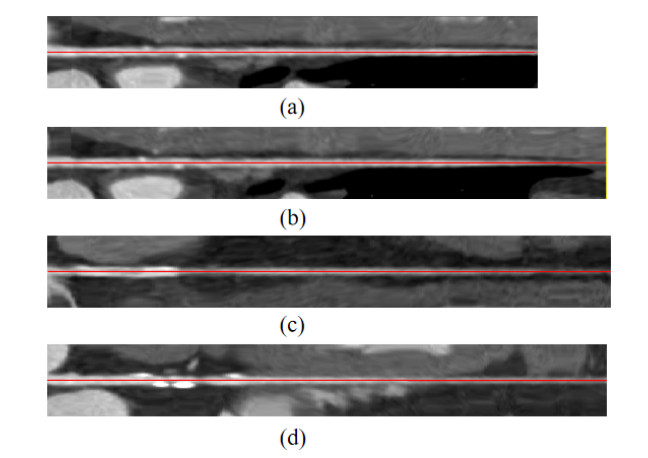
DownLoad: