1.
Properties of fluorescent proteins used for protein degradation assays
The impact of the green fluorescent protein (GFP) and its derivatives on cell biology has been tremendous. These molecules have provided insights on the localization and dynamics of single proteins, complexes, and organelles in living cells. Furthermore, fluorescent proteins (FPs) with emission colors spanning the whole visible spectrum have been developed in the past decades to follow independent events simultaneously. The aim of this ongoing process is to develop bright, monomeric, fast maturing, photostable FPs covering the full visible spectrum that are functional in all cellular compartments [1]–[3].
Although FPs are mostly used either as transcriptional reporters or to characterize the localization and/or dynamics of a specific protein, initial attempts to use GFP as a reporter in protein degradation assays began early on [4]. In this review, we will summarize the most common techniques in which FPs report protein degradation and highlight biophysical properties of FPs that are important for these assays. Initial studies used single FPs as readout for protein abundance. Later on, the methodology advanced to the usage of dual-color referencing, photoconvertible FPs, and fluorescent timers (FTs) to facilitate investigations. Researchers applied these more sophisticated degradation assays and proteome-wide screens to uncover whole protein degradation pathways by systems biology approaches. Regarding FTs, either specialized FPs that switch their color due to a second chromophore oxidation step or a tandem combination of a fast maturing FP with a slow maturing FP are used [5]–[7]. In both cases, ratiometric determination of fluorescence intensity reports the age of the whole fusion protein. Photoconvertible FPs are an alternative for in vivo pulse-chase experiments, as they allow for the labeling of an existing pool of proteins at a specific time point. Following this molecule pool over time is a convenient way to determine the stability of a protein. Moreover, de novo protein biosynthesis can be recorded after the photoconversion by the synthesis of unconverted FP molecules [8].
The biophysical features of the FP fused to the protein of interest (POI) could influence the characterization of protein stability. A critical feature in the context of protein degradation assays is the use of a monomeric FP in the fusion protein. The formation of dimers or tetramers, which were present in many older GFP and RFP variants, might lead to changes in a degradation pathway or the requirement of additional factors [9]. The maturation half-time of the FP is another characteristic with a huge impact on protein degradation assays. In the case that the maturation half-time of the FP is much longer than the half-life of the POI, most proteins in the degradation assay will be invisible to the observer due to FPs with non-matured chromophore [10]. If the two values are similar, about 50% of the POI will be undetected in the assay. Only if the maturation time is much shorter than the degradation half-life will most POIs be observable. Although this condition demands the usage of FPs with a short maturation time, FPs of distinct color with differences in maturation time are crucial for a novel type of reporter construct of protein age, the tandem FP timer [7].
The maturation time of an FP can also influence the data in other types of assays. In the case of a translational shut-off experiment, in which a translational inhibitor like cycloheximide is used to stop protein biosynthesis [4], maturation of the existing FPs continues for the duration of the experiment [11]. Consequently, the fraction of matured FPs increases over time. This ongoing maturation has to be considered in the interpretation of the experimental results if an FP with longer maturation time is used in an assay of relatively short time frame. Thus, not only the overall brightness, but also the maturation time of a given FP influences its successful application as a reporter in an assay. Therefore, the differences in biophysical properties of common FPs (Table 1) should be considered before constructs are generated.
A diverse array of protein degradation assays have been developed based on single FPs as well as their combinations [3], convertible FPs [89], and timers [5]–[7]. Next, we will give a short introduction into protein degradation followed by an overview of the different techniques used to monitor protein degradation and to critical biophysical properties of FPs that are important for these applications.
2.
Protein degradation by the ubiquitin proteasome system
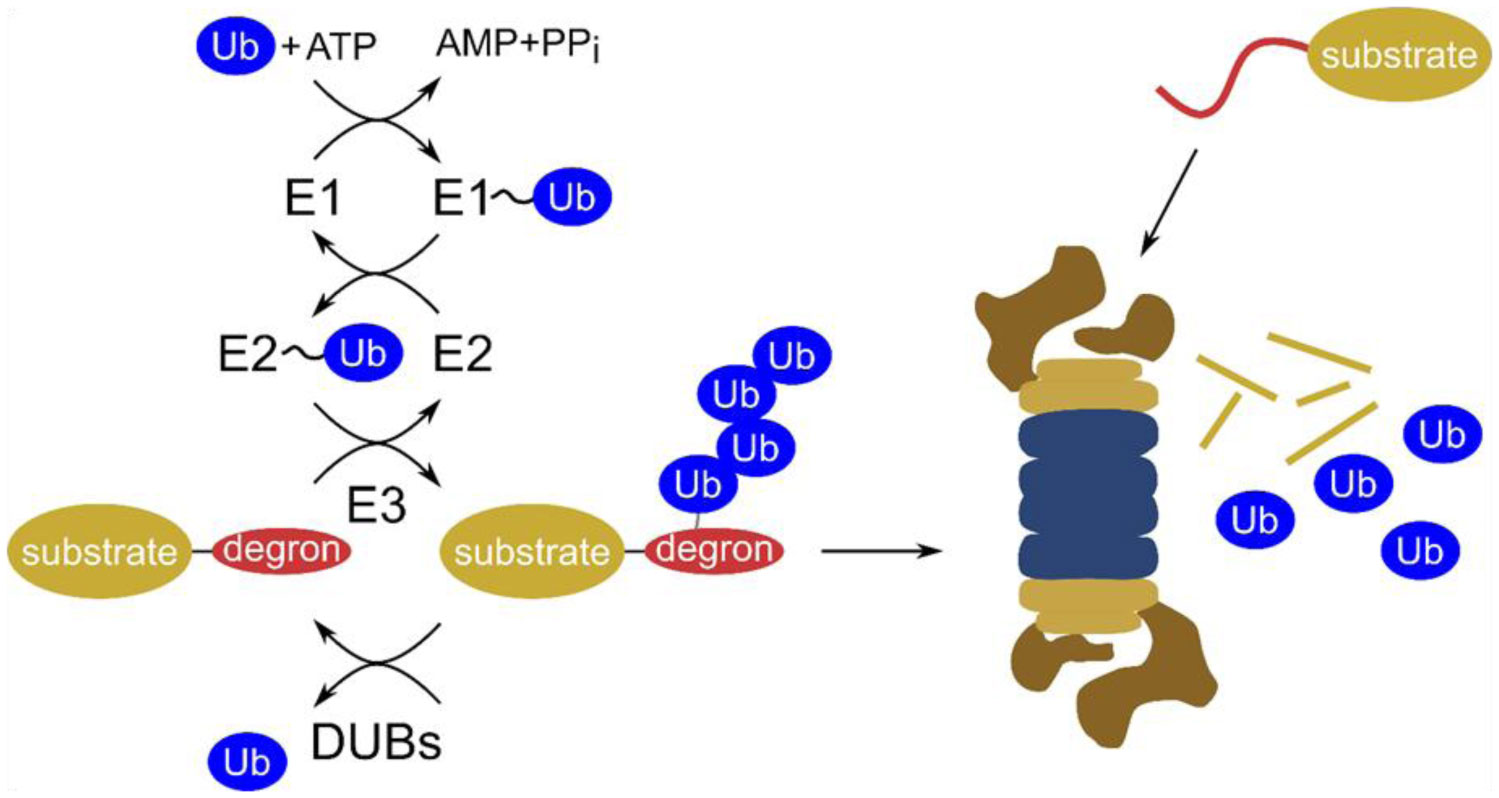
Regulated protein turnover plays important roles in eukaryotic cells, allowing them to shape their proteome in accordance to extracellular and intracellular signals and serving as a basic mechanism to ensure cellular viability. Deregulation of protein turnover or defects in proteolysis are connected to severe diseases as well as cellular and organismic aging in higher eukaryotes [90]. The characterization of basic degradation pathways as well as determinants of protein stability is therefore of high importance. In eukaryotes, one of the main machinery for regulated protein turnover is the ubiquitin-proteasome system (UPS; Figure 1). The small protein ubiquitin is a tag that can mark proteins for degradation by a protease complex, the so called proteasome [91],[92]. In brief, ubiquitin is activated by the ubiquitin-activating enzyme (E1) through adduct formation of the C-terminal glycine of ubiquitin with a cysteine in the E1 in an energy-rich thioester bond. Transesterification onto a cysteine in a ubiquitin-conjugating enzyme (E2) primes the activated ubiquitin for the transfer onto the substrate. In general, an E2 forms a complex with a ubiquitin-protein ligase (E3) that selects the substrate. Substrate binding by the E3 stimulates the transfer of ubiquitin to the substrate; usually, it is fused covalently to the ε-amino group of a lysine. This step may be repeated further through the addition of ubiquitin moieties to a ubiquitin already attached to the substrate, resulting in the formation of polyubiquitin chains. This elongation sometimes requires the action of a fourth enzyme (E4) for efficient ubiquitin chain formation. Moreover, several different mechanisms of ubiquitin transfer have been identified by biochemical studies. Excellent reviews provide detailed information about the basic mechanism of protein ubiquitylation [91]–[94].
In general, proteasomal proteolysis requires a chain of at least four ubiquitin moieties linked to each other via the C-terminus of a ubiquitin fused to a lysine at residue 48 in the previous ubiquitin or ultimately in the substrate [95]. Although such a linear ubiquitin chain is sufficient to target a ubiquitylated protein for degradation, the in vivo situation is more complex. Ubiquitin has seven lysines as well as the N-terminus for further ubiquitylation, which enables a variety of linear chains or branched complexes with diverse patterns as substrate ubiquitylation outcomes. Ultimately, the ubiquitylation pattern influences the fate of the modified protein, with linear chains linked through K11 or K48 signaling for proteasomal degradation [96],[97].
The polyubiquitin chain is recognized by subunits in the regulatory particle of the proteasome or by adapter proteins that bind to the polyubiquitin chain and to the regulatory particle. Substrate unfolding by AAA-ATPases requires an unfolded sequence stretch. Concomitantly, the substrate is transferred to the inner cavity of the proteasome where the proteolysis into peptides takes place. The polyubiquitin chain is removed from the substrate before or during degradation by a deubiquitylating enzyme and recycled for further rounds of ubiquitylation [98].
Substrate selection occurs mostly by E3s that recognize degradation sequences (degrons) in the substrate. A degron is defined as a part of a protein that is necessary and sufficient for degradation [99],[100]. In the context of the UPS, degrons are often short, transferable sequence motifs that are bound by an E3, which induces ubiquitylation and degradation. Thus, degrons typically contain several distinct features: a recognition motif for the E3, at least one lysine for the attachment of a polyubiquitin chain and a stretch of unfolded amino acids for the induction of proteasomal proteolysis [101]. Some specialized degrons are not bound by an E3 and do not require polyubiquitylation to induce proteasomal degradation. Instead, these degrons bind directly to the regulatory particle of the proteasome and induce proteolysis of the substrate. However, an unfolded region of sufficient length is still required in the substrate to allow unfolding and degradation of the protein. The best-studied example following this kind of degradation mechanism is the so-called cODC degron, derived from the carboxy terminus of murine ornithine decarboxylase (ODC). The degradation of cODC by the proteasome via this mechanism is conserved between diverse eukaryotes, as the degradation-inducing function of cODC has been observed in mammalian, plant, insect, and yeast cells [102],[103].
The mode of substrate selection, by an E3 or by the proteasome itself, cannot always be easily distinguished. Some substrates simply follow both routes like the well-studied transcription factor Rpn4 [104],[105]. Depending on the molecular or cellular environment, gradual changes in degradation route are possible. One example is the photosensitive degron (psd) module, an optogenetic tool for light-induced protein degradation that is useful for generating conditional mutants of essential proteins in yeast [39],[82],[106]. For activation of the psd module, a synthetic variant of the proteasome-recognized degron cODC becomes exposed following light-triggered structural changes of a photoreceptor. Whereas soluble psd-modified proteins seem to be mostly recognized directly by the proteasome, with only a minor fraction of the activated psd dependent on the E3 Doa10 for degradation, ER membrane proteins fused to psd are completely dependent on Doa10 for degradation [107]. Although the proteasome is active close to the ER membrane [108],[109], it does not seem to recognize the cODC degron of psd close to the membrane. These findings exemplify the necessity of mapping degradation pathways in detail and indicate a lack of knowledge concerning the recognition of substrates by components of the UPS.
FPs are excellent reporter proteins to test degrons and the different features of a degron, as they can be easily observed in living cells. Their natural stability against proteolysis makes them almost ideal reporters to investigate proteolysis and presents the possibility of identifying whole degradation pathways in large scale [42]. Non-FP based compounds have been employed to study the UPS as well, which have been reviewed elsewhere [110]–[113].
3.
Fluorescence reporters for the observation of protein kinetics
Diverse strategies have been used to follow protein degradation in eukaryotes with FPs. Common observation techniques for FPs were used, namely quantitative fluorescence microscopy, fluorescence detection by plate reader, and flow cytometry. Not surprisingly, the first applications in protein degradation relied on GFP as a reporter.
3.1. Fusion with a conventional FP
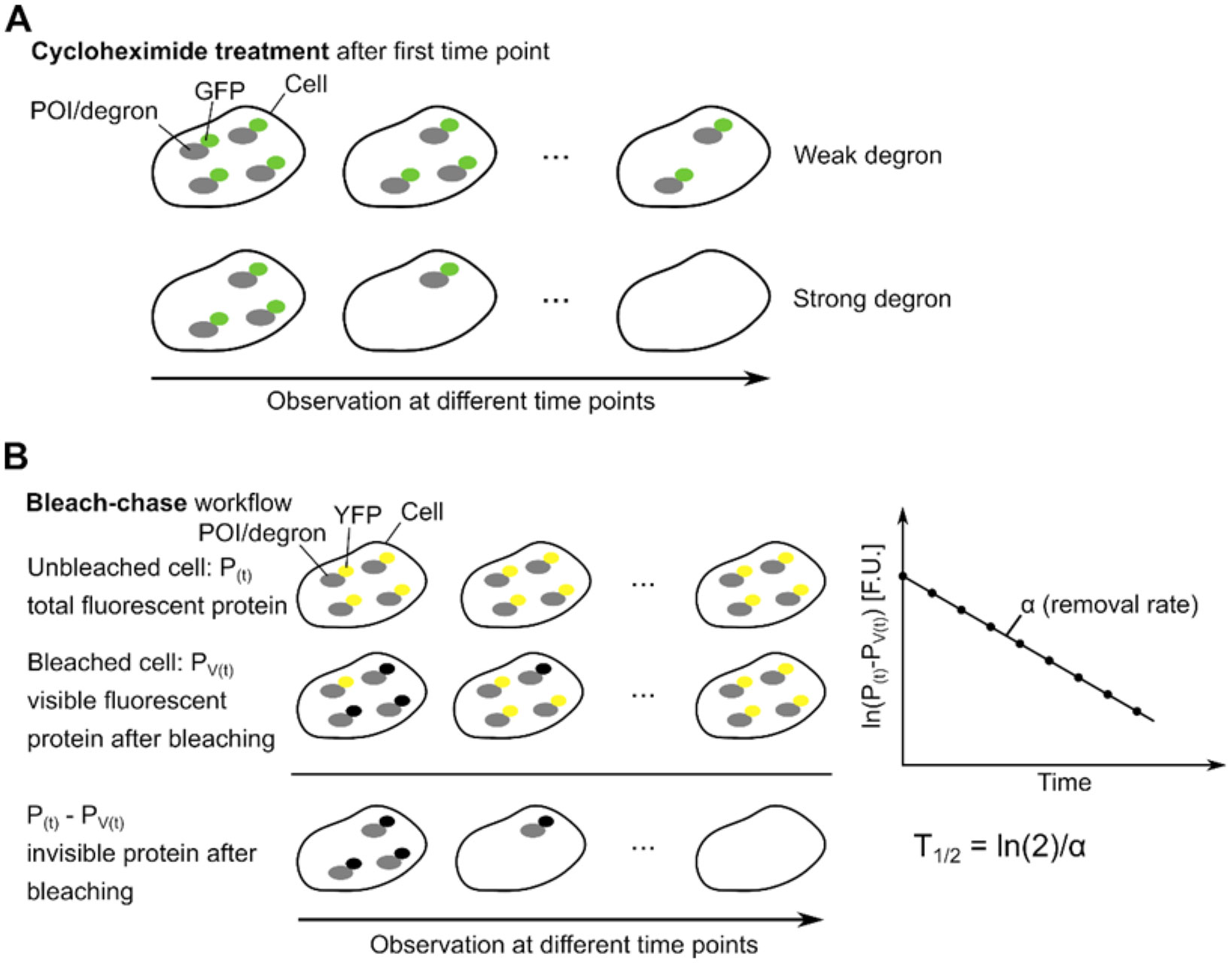
Historically, the usage of GFP in living cells and as a marker for protein localization was soon followed by application as a readout for protein degradation events [4],[114],[115]. Subsequently, fusions of GFP to proteins of interest and random mutagenesis were used to identify genes involved in turnover of the target in budding yeast [116],[117]. Similar approaches have also been undertaken in mammalian cells, either to identify degradation enhancing sequences or to study protein degradation on single cell level. An early investigation aimed at identifying novel degron sequences in mammalian cells used GFP as reporter and detection by flow cytometry [118]. Protein synthesis was stopped by the translational inhibitor cycloheximide, which is one of the classical techniques for determining protein half-lives (Figure 2A) [119].
Apart from the search for new degrons and degradation pathways, the usage of destabilized eGFP or other FPs coupled to the cODC degron as well as the application of reporter substrates like UbG76V-GFP as transcriptional reporter is widespread for investigating UPS activity or screening for proteasome inhibitors [120]. Quantification of the POI is usually achieved by fluorescence microscopy or flow cytometry and fluorescent reporters have been detected in single cells, living microarray format, or whole animals [41],[121]–[130]. A combination of automated high-throughput microscopy with a translational shut-off by cycloheximide was used to characterize protein degradation kinetics and cell-to-cell variations of destabilized eGFP in a large number of mouse fibroblast cells. [30],[41],[131]. One problematic aspect is that quantification of a single FP fused to the POI is affected by cell-to-cell differences in expression [132], which might influence the interpretation of the results.
Further applications of FPs as degradation reporters comprise characterization of known degrons and the search for novel ones. Usually, the implementation and quantification is performed in a similar way as pioneered by Hampton et al. 1996 [4]. However, combinations of FPs have also been used to track the abundance of the degron fused to an FP and to follow the expression of a protease simultaneously observing proteolytic cleavage of a substrate with concomitant degron activation [23],[24]. In microorganisms like budding yeast, FPs are directly observable in colonies and cell lawns [82],[116]. Detection of the fluorescence level allows one to screen for novel components of a degradation pathway or to search for degron variants with higher activity [39],[117]. Moreover, characterization of protein degradation at single cell level, the characterization of natural degrons, and the development of synthetic degrons relies heavily on the usage of FPs [13],[17],[38],[40],[76],[133]. Often, degrons are readily transferable between eukaryotes, due to the high conservation of the UPS [91],[103]. Depending on the cellular context, however, surprising effects have also been observed. One example is degrons that induce protein degradation in yeast or in mammalian cells, but lead to protein agammaegation in Caenorhabditis elegans [37],[134],[135]. In C. elegans, these sequences are not acting as specific proteasome-targeting signals. Instead, they alter the structure of the GFP resulting in agammaegation-prone proteins that are recognized by the chaperone system. Overloading the capacity of chaperone-based degradation pathways then leads to the formation of toxic agammaegates [37].
Localization of fully active proteasomes has been detected with bifunctional molecules. These molecules form a covalent bond to a threonine residue in the active center of a proteasome subunit and have a fluorophore attached to themselves by a linker [136]. Although mostly non-FP-based fluorescent compounds have been used for this purpose, it is a neat addition to the toolbox for visualizing localization of proteasomes engaged in protein degradation.
The usage of FPs in living cells is not always without obstacles, especially in cases where absolute or relative amounts of protein levels must be correlated with fluorescence intensities. Common challenges include the transfection efficiency of the reporter construct, which depends on cell type and size of the plasmid/construct. This influences how many cells in the assay are carrying the reporter and lead to variations in expression strength [137]–[140]. Although these factors do not influence the half-life of a reporter, they have an impact on fluorescence quantification. Below, we describe some methods that were developed to address some of these challenges, for example imaging of two cell lines together, usage of a photoconvertible FP, ratiometric reporters, or usage of tandem FP timers.
One characteristic of cells is that they exhibit fluorescence of metabolites and compounds of their own [141]–[143]. This autofluorescence signal will add to the signal of the FP and must be taken into account for quantification of the specific signal. Usually, compensation requires measurement of cells without the FP.
The bleach chase is a remarkable method relying only on single modification of the POI with one fluorophore (Figure 2B). Here, time-lapse analysis of undisturbed cells versus cells in which the FP has been bleached is used to calculate the protein removal rate [77]. Application requires a microscope that allows bleaching of the FP in selected cells, which is usually achieved by a laser. One should use a bright FP with relatively low photostability to minimize phototoxic effects during bleaching, but photostable enough to allow long-term observation of the cells. A fast-maturing FP is critical to avoid distortions when calculating the invisible molecule fractions. Other critical parameters for obtaining meaningful results include quantification of the autofluorescence and bleaching of the fluorophores during long-term observation of the cells.
In one study, a network implementation was used to obtain direct visualization of UPS activity. The genes of stable FPs were integrated into a genetic circuit with a destabilized transcriptional activator to link proteasomal activity with transcription of a fluorescent reporter. Thus, long-term investigation of proteasomal degradation and UPS activity is facilitated in mammalian cells [144].
The usage of fluorescence microscopy in protein degradation assays in order to identify novel degradation pathways in high throughput screens proves challenging due to the relatively low image acquisition speed and the need for proper image segmentation and fluorescence intensity quantification. Nevertheless, microscope automation has been used to circumvent these obstacles. In a high throughput microscopy-based search for targets of the E3 Grr1 in yeast, staining of yeast cells with a dye enabled cell detection. Yeast POIs were labelled with GFP and Grr1-dependent degradation was monitored in a mix of two cell lines for each GFP-marked POI, one with endogenous Grr1 and one lacking Grr1 that was labelled by RFP. Both cell lines were imaged simultaneously, positive hits were identified by high abundant GFP fluorescence in RFP-containing cells [64]. Thus, side-by-side comparison of cells with and without operational Grr1 was used to identify POIs degraded via the E3 Grr1. This method circumvented the necessity for precise quantification of the POI, which could be affected by fluctuations in fluorescence intensities. Usage of FPs as reporters is a very common technique; however, the last example of high-throughput screening also exemplifies the problems that one has to face: cell-to-cell variations in fluorescence that are invoked by extrinsic and intrinsic factors [132]. Coupled with fluctuations in fluorescence detection, these variations impede the elucidation of protein degradation pathways. In the search for Grr1 substrates, simultaneous detection of cells with and without Grr1 was used to minimize this problem. Another possible solution is using a reference fluorophore that normalizes the fluorescence to an internal control.
3.2. Conversion of fluorescent proteins
The development of photoconvertible FPs facilitated characterization of protein dynamics, among them also protein degradation. Photoconvertible FPs change their fluorescence color in response to irradiation with light of a specific wavelength like Dendra2 or switch from non-fluorescent proteins to fluorescent ones like PA-GFP (photoactivatable GFP). Some examples are given in Table 2.
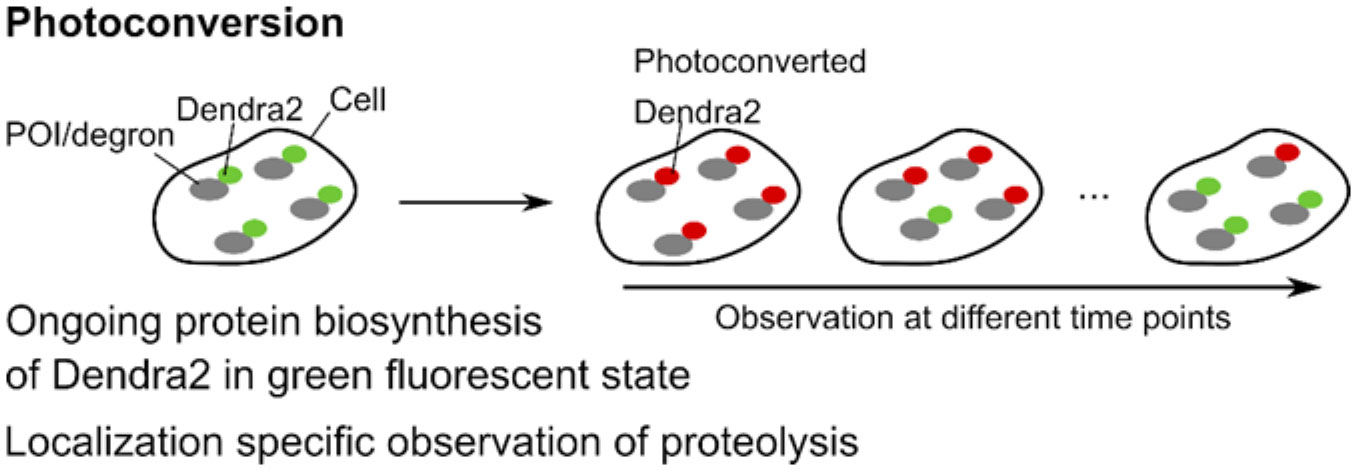
After photoconversion, the decrease in the specific fluorescence signal, which depends on the degradation rate, can be measured without the use of inhibitors of protein biosynthesis (Figure 3). Photoconvertible FPs have proven useful for monitoring real time protein degradation on a single-cell level and have shown potential for high throughput analysis [8],[151]. The green-to-red photoconvertible FP Dendra2 was successfully used to determine different degron-mediated protein half-lives in cultured cells [8] as well as for the measurement of UPS activity dependent on cell-type and age in C. elegans [149]. Moreover, photoconvertible FPs were used in cultured cells as well as in Drosophila, mouse, and zebrafish embryos to measure protein turnover dynamics [152]–[155].
Similar to the usage of a single FP as degradation reporter, measurements are affected by cell-to-cell variations in protein expression. However, in comparison to conventional FPs, photoconversion has the clear advantage that autofluorescence of individual cells can be measured before photoconversion. This leads to more reliable measurements of the degradation of the POI. In order to ensure that a large fraction of the molecules in a cell are highlighted during the assay, the photoconvertible FP should ideally exhibit high brightness in the converted state and fast maturation into the non-converted state. Unfortunately, most photoconvertible FPs are less bright than conventional FPs and maturation times to the non-converted fluorescent state are not always available. Complete photoconversion of all molecules in a cell is not necessary to measure the degradation rate. For most proteins, the intracellular decay rate follows first order kinetics and is independent from the amount of molecules present at the beginning [156]–[158]. Nonetheless, the better the photoconversion rate, the better is the signal to noise ratio, which facilitates quantification of the converted FP signal.
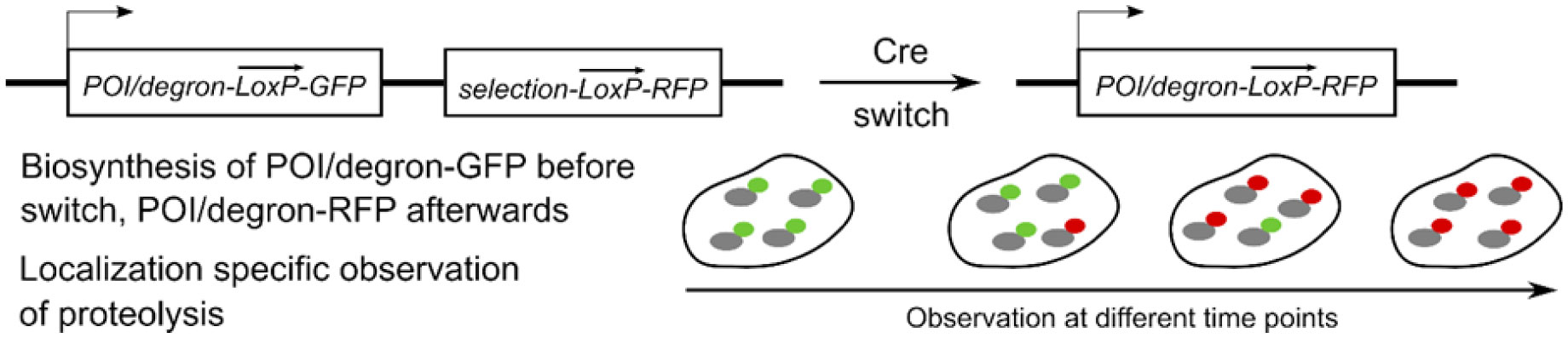
A variation of FP conversion is the recombination-induced tag exchange (RITE), which relies on a genetic switch in the FP fused to the protein of interest by the Cre recombinase. Here, the Cre recombinase removes a sequence stretch containing a GFP gene as well as a selection marker in order to bring an RFP gene in frame with the gene of interest [159]. Thus, the protein of interest is synthesized before the Cre switch with GFP and afterwards all newly synthesized molecules are fused to RFP. Time-lapse recording coupled with quantification of GFP and RFP signals before and after the switch achieves monitoring of protein turnover as well as biosynthesis of the protein of interest (Figure 4). FPs with fast maturation times are important in order to avoid distortion of the degradation measurement and delay in observation of the newly synthesized molecules after switching.
RITE has been applied in mammalian cell culture to investigate cell cycle dependence and cell to cell variation of proteasomal proteolysis, degradation of nuclear inner membrane proteins, and long-lived proteins in postmitotic cells [55],[160],[161]. In budding yeast, the method has been used to investigate the distribution of proteasomes in aged and starving cells, destruction of mitochondria in aged cells, and the behavior of long-lived proteins [162]–[164].
3.3. Ratiometric reporter using a second fluorescent protein
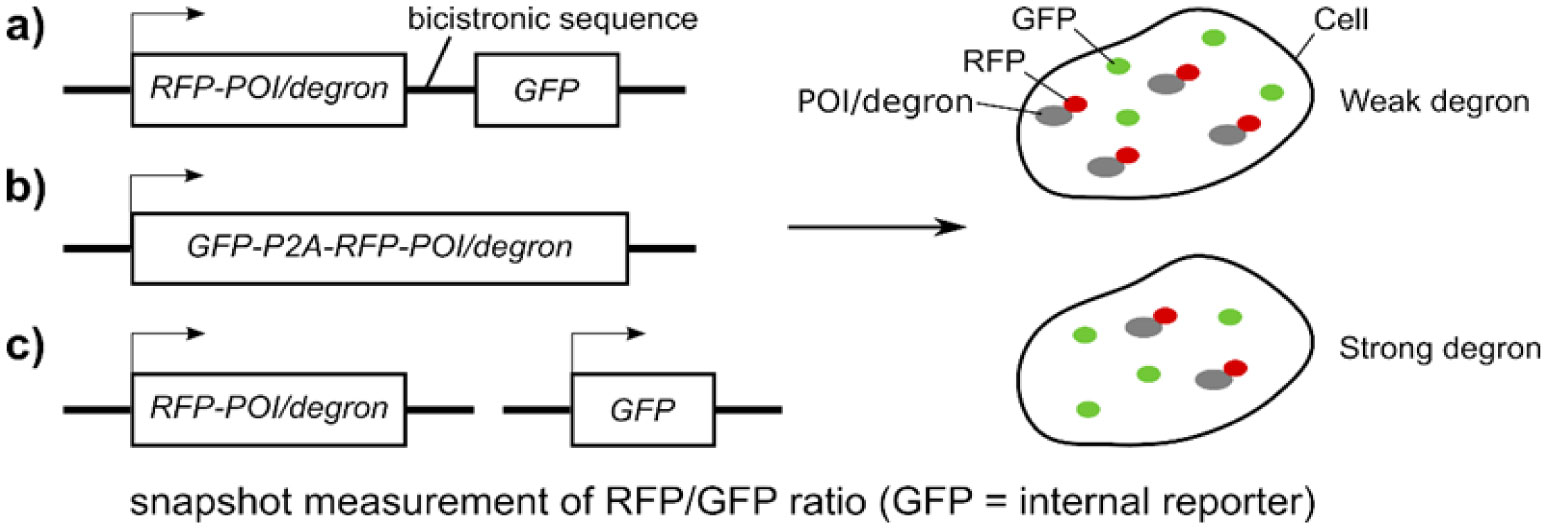
Another approach to characterizing protein degradation is to monitor the fluorescence intensity ratio of two different FPs. This system uses two FPs that are translated from a bicistronic mRNA. Separation of the coupled proteins at translation level in eukaryotes can be achieved by inserting different sequences between the two genes. For example, an internal ribosomal entry sequence (IRES), a viral 2A-peptide sequence, or two constitutive promoters have been used for the fluorescence-based characterization of protein stability in mammalian and yeast cells (Figure 5) [25],[31],[58].
In relation to the IRES or the 2A-peptide sequence, expression levels will not necessarily be the same, but a fixed ratio will be achieved. One FP acts as an internal reporter, whereas the other one is fused to the POI. Changes in the fluorescence intensity ratio of both proteins are correlated to the degradation of the POI and are not altered by fluctuations in transcription or translation. Thus, ratiometric measurements enable snap-shot measurements that facilitate screening of many POIs in a short time. Proteome-wide association studies to decipher degradation pathways are feasible with this approach. Important features of the FPs include fast maturation of both FPs in order to ensure correct calculation of the ratio.
Such a bicistronic approach based on IRES combined with the hORFeome proteome library, microarray technique and fluorescent-activated cell sorting enabled the investigation of a larger set of proteins in mammalian cells. This so-called fluorescence-based global protein stability (GPS) profiling is a systematic, high throughput approach to monitor protein turn over, which allows the observation of dynamic changes in protein stability dependent from stimuli or genetic manipulation. For GPS profiling and identification of substrates of the Skp, Cullin, F-box containing complex (SCF) in cultured cells, the FP DsRed was used as an internal reporter and an eGFP was fused to the POI. Bicistronic expression was obtained by using an IRES sequence placed between the RFP and the GFP construct [31],[32],[42]. In addition, this methodology has been successfully used to screen for C-degrons in mammalian cells [34],[165] and to develop a system for induced protein degradation using proteasome adaptors in mammalian cells [166].
It should be taken into account that the existing mechanisms to generate bicistronic mRNAs in eukaryotes have certain imperfections. A reduced expression efficiency of the downstream open reading frame (orf) has been observed for IRES sequences [167]. This behavior can be minimized with IRES sequences that mediate different expression rates of the downstream orf and repetition of certain IRES sequences [168]–[171]. In addition, the types of orfs flanking the IRES sequence as well as the length and secondary structure of the non-coding inter cistronic sequence between the orfs influence the expression rate [172],[173]. Despite these issues, it has been successfully implemented to obtain large-scale information about protein degradation pathways in mammalian cells.
Another ratiometric method was used to compare efficiencies of degron-module variations in yeast. Here, super-folder GFP (sfGFP) was used as reference and mCherry as reporter that was destabilized by fusion to various degron-modules. Bicistronic expression was achieved by an insertion of a viral 2A-peptide sequence [25]. The viral 2A-peptide sequence causes ribosomal skipping during translation, which results in two separated proteins [174]. Nonetheless, it has been observed that these sequences do not always result in complete separation of both proteins and that the production of the second protein is reduced [175]. There are different 2A-peptide sequences with varying cleavage efficiencies that also depend on the used cell type as well as an optimization strategy via modification of the 2A codons [174],[176]. Despite these issues, the method has been used to compare protein stabilities and to monitor dynamic changes invoked by external factors [25],[177]–[180].
A third approach was used to compare the strength of commonly used degrons in Saccharomyces cerevisiae. In this case, the RFP DsRed was expressed as reference from a constitutive promoter and a YFP fused with the different degrons from another promoter. Quantification of degron strength was achieved by ratiometric snap-shot measurements [58]. This technique was also used to investigate the importance of disordered regions and ubiquitin-like domains in proteasomal proteolysis [181]. Overall, the diverse examples of successful usage of internal controls demonstrate the robustness of this approach. The viral 2A and IRES sequences promise small and compact constructs at the expense of non-fully separated constructs or unequal production of the POI compared to the internal control. In contrast, usage of two expression cassettes results in considerably larger constructs and equal expression of reporter and degron construct depends on the promoters in use.
3.4. Fluorescent timers
The tandem fluorescent timer (tFT) consists of two FPs with distinct maturation rates fused to a POI. In most implementations, the fast maturing sfGFP (maturation half-time: 6 min) is coupled to the slower maturing mCherry (two step maturation: 17 min and 30 min) [7]. Thus, a POI is marked by the sfGFP and its average age can be deduced by the maturation state of mCherry in relation to the fluorescence intensity of the GFP (Figure 6).
Shortly after protein biosynthesis, the fusion protein will show only green fluorescence, due to the fast maturation rate of sfGFP, resulting in a low mCherry/sfGFP intensity ratio. Over time, the slower maturing mCherry becomes fluorescent and the ratio increases with protein age. POIs with a fast turnover will have a very low mCherry/sfGFP fluorescence ratio, as the POI is degraded before mCherry maturation is complete. Proteins with slower turnover rates will have a higher ratio due to the increased number of molecules with matured mCherry. As only snap-shot measurements are necessary to characterize the age of a POI, proteome wide investigation of protein turnover is easily achieved with libraries of tFT-tagged proteins. Different time ranges can be achieved through coupling FPs with different maturation times together [7],[35]. One of the FPs should always have a fast maturation time. Changes in the FP with slower maturation kinetics adapts the tFT to match the characteristics of the process of interest. By measuring the fluorescence intensities and calculating the mCherry/sfGFP intensity ratio, it is possible to determine protein kinetics, spatio-temporal localizations, and protein degradation kinetics with tFTs. Importantly, the tFT is fused to the POI in a way that the GFP is placed distal to the degradation site, as sfGFP is known to be incompletely degraded by the proteasome [35]. However it should be noted that the maturation times can also be influenced by different cellular conditions or the cellular localization [7].
The tFT assay was used in yeast to assay the stability of POIs in dependence to o-glycosylation, to reveal the degradation pathway of mitochondrial membrane proteins, and to investigate protein degradation of mislocalized tail-anchored membrane proteins [53],[54],[62]. Pooled libraries of tFT tagged proteins containing different N-terminal sequences were used to study N-terminal degrons in single cells with fluorescence–activated cell sorting analysis [61]. In addition, the tFTs were successfully utilized in yeast to investigate the turnover rates of several putative targets of the inner nuclear membrane-associated degradation (INMAD) Asi complex and the endoplasmic reticulum associated protein degradation (ERAD) system [60]. Moreover, a tandem FP timer with sfGFP coupled to mOrange was used to study cell cycle dependence of proteasomal degradation as well as cell-to-cell variation of proteolysis in mammalian cells [55]. Here, it was shown that time-lapse recordings can be used to reveal changes in degradation and biosynthesis rate of a POI. The system was also applied in plants (Nicotiana benthamiana & Arabidopsis thaliana), demonstrating its value to characterize protein half-lives in different genetic backgrounds via live cell imaging [56].
The utility of the tFT approach was also demonstrated in animals. Dona et al. used tFT-tagged G-protein coupled receptors to investigate their turnover rate as well as to track the signal transduction of cytokines in migrating cells in Zebra fish embryos [59]. In Drosophila, the tandem fluorescence timer was adapted to investigate the precise manner of bicoid gradient formation in embryos, highlighting the role of protein degradation in embryo morphogenesis [52].
The diverse applications of the tFT method, the usability in single cells or whole animals, and the possibility to infer the age of a protein, solely by snap-shot imaging argues for the importance of this method. As the age of a protein is directly related to its degradation rate, simple measurements are enough to assess protein stability at a given time point. Time-lapse recordings of tFTs incorporate information like changes in degradation and biosynthesis rates. Comparison of such data in whole animals or developing embryos multiplies the applicability and demonstrates the valence of this approach.
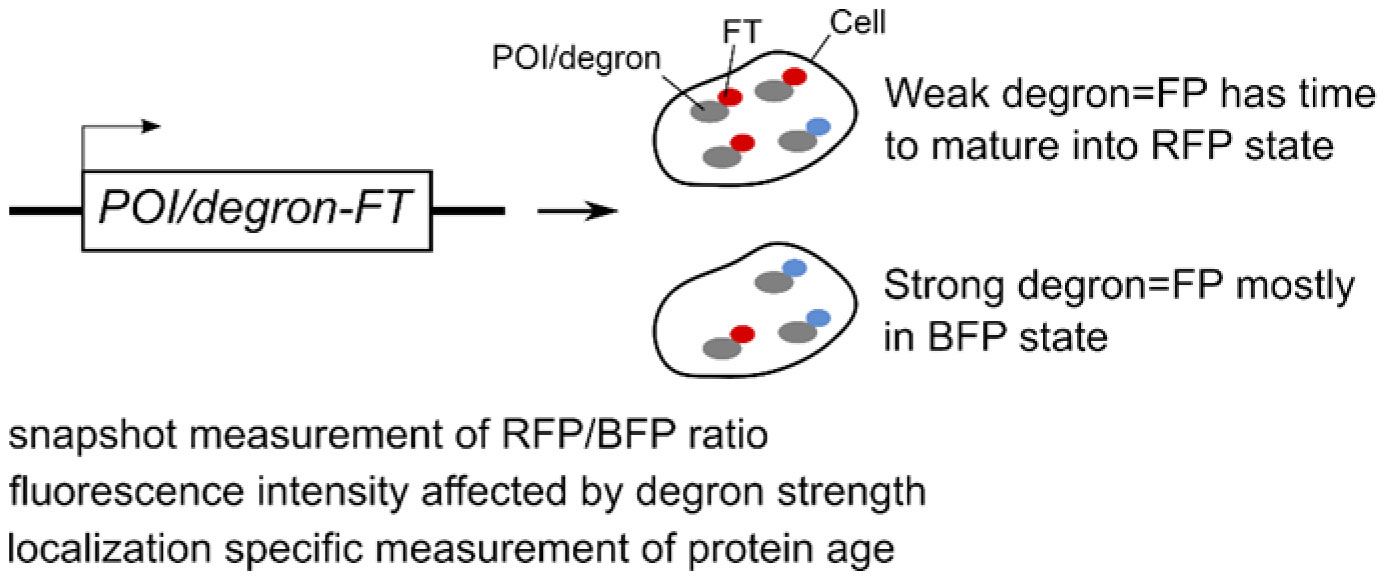
Recently, single FP timers have been described that show a blue-to-red fluorescence conversion [6] (Figure 7, Table 3). They have been applied in mammalian cells to study proteasomal proteolysis, endosomal protein degradation and to generate a degron toolbox [36],[79],[182]. Although these timers are relatively slow in changing their fluorescence, the maturation time seems to be suited to follow proteolysis of certain events in mammalian cells. Tailored FPs like these timers with high brightness and different switching times might be a fruitful direction for further developments. The possible application range is similar to that of tFTs.
4.
Concluding remarks
Usage of FPs to study protein degradation has resulted in characterization of numerous degradation pathways and the diverse fluorescence-based methods are valuable additions to the toolbox for investigating UPS-dependent protein degradation [42],[113]. Yet, certain features of FPs have to be taken into account. One issue is the intrinsic stability of the β-barrel fold of FPs. It has been noted with different types of proteasomal substrates and in different organisms that an FP destined for degradation and already engaged by a proteasome might escape its fate [33],[35]. The tight folding of the β-barrel might exceed the unfolding capability of the proteasome sometimes and a certain percentage of FPs escapes the degradation process. Moreover, the FP is released from the proteasome devoid of the degron sequence in this case, which protects it from further degradation attempts in the future. Thus, kinetic measurements relying solely on fluorescent measurements might be slightly shifted due to the presence of escaped FPs that accumulate over time. Especially K63-linked ubiquitin chains were found to be inefficient for proteasomal degradation of GFP [33],[183].
However, not only the type of ubiquitylation is critical for efficient proteolysis of an FP, but also the stability of the β-barrel and the type of FP, e.g. sfGFP resists proteasomal degradation considerably whereas neonGreen is degraded more efficiently [35]. In bacteria, a stepwise unfolding of sfGFP has been suggested for the degradation by the ClpXP protease, which might indicate that partially digested proteins are able to escape the protease in-between the unfolding steps [184]. In line with such a view, changing the starting point for unfolding and proteolysis of the β-barrel by circular permutation has been demonstrated to minimize inefficient proteolysis of sfGFP by ClpX in vitro as well as by the proteasome in vivo [35],[184]. Thus, usage of an FP that is efficiently processed by the protease of choice is a critical consideration prior to an investigation aiming at determination of protein stability. This is not only the case for usage of a tFT, but also for assays that use ratiometric generation of an internal control. In the latter case, an inefficiently digested FP like sfGFP should be avoided as direct fusion partner of the POI. For a tFT assay, placement of sfGFP distal to the degradation initiation site is recommended [35].
For the purpose of protein degradation assays, it would be advantageous to have a more thorough biophysical characterization of the permutated sfGFP variants with respect to maturation time and brightness. At the moment, the available information is incomplete [35],[51]. Moreover, incomplete proteolysis of other fluorophores might confer a problem if they are used together with e.g. sfGFP. For mCherry this might not be an issue, as incomplete proteasomal degradation was not detected in the context of tFTs [35]. Yet, no information is available about in vivo proteolysis of other, more modern RFPs like Scarlet or Scarlet-I that are increasingly used instead of mCherry due to their higher brightness [84],[185]–[187]. Another issue that needs to be considered before the usage of fluorophores is autofragmentation of FPs [15],[188]. This may not be a problem during fluorescent assays, but it can hamper other techniques like immunodetection. For eGFP, the fragments are a byproduct of autocatalytic chromophore formation. As different FPs show differences in fragment formation, future improvement of FPs may address this issue as well.
Biophysical properties of FPs have to be considered for many applications. These include fluorescence changes induced by pH and oxidation, which depend on the cellular environment of the FP or differences in fluorescence lifetime [189],[190]. FPs sensitive to changes in pH and oxidation state have been developed and characterized based on these changes in fluorescence intensity [191]–[194]. Careful consideration of these properties is required for the interpretation of results originating from large-scale protein degradation assays in system biology. An FP fused to POIs that originate from different cellular environments with differences in pH or redox conditions might show a huge POI-dependent change in fluorescence. Failure to compensate for such influences might lead to an increase of false positive or false negative hits. Usage of organelle specific probes that are optimized for a distinct cellular environment would be one possible solution for such issues. However, properties like pH-dependent shifts in fluorescence lifetime of GFP have been used to monitor events like bacterial phagocytosis [195], which exemplifies the possibilities that careful considerations of biophysical properties of GFP and related proteins are offering.
Moreover, application of FPs in the secretory system is challenged by mechanisms like glycosylation and disulfide bond formation, which both might interfere with FP folding or lead to oligomerization [196]. Oligomerization of eGFP by disulfide bond formation has been observed in endocrine cells, which was resolved by mutation of two cysteines (Cys49, Cys71) [197]. However, cell type specific factors and the exact FP variant might play a role as well, as GFPuv has been reported as a tool to investigate secretion in cultivated insect cells and Aequorea victoria GFP has been used to image the secretory pathway in tobacco leaves [198],[199]. A toolbox of common FPs and photoswitchable FPs have been developed that are optimized for organelles of the secretory pathways and as fusion partners for membrane proteins [65],[200]–[202]. Yet, variants of the FP Venus or its split variant have been developed whose fluorescence relies on a cycle of glycosylation and deglycosylation [203]. These variants were used to investigate protein degradation via the ERAD pathway. Another method to detect protein-protein interactions, the so called fluorescence resonance energy transfer (FRET), has been used to determine specificity of protein agammaegation [204]. The latter process is induced upon failure of proteasomal degradation for certain proteins [205]. During FRET, energy is transferred nonradiatively from a donor FP in its excited state to an excitable FP (the acceptor) and the transfer is highly sensitive to the distance and orientation of the FPs [206]. This aspect is often problematic for successful in vivo applications, but the high sensitivity of the method reports accurately about changes in protein-protein interactions.
The researcher investigating protein degradation has a demand for fast-maturing FPs with high brightness, high photostability, no tendency for oligomerization, and smooth proteasomal proteolysis. Yet, the wealth of data produced by tFT assays demonstrates that FPs with diverse maturation times are also interesting for generating reporters with distinct features optimal for illuminating the biological question of interest.









 DownLoad:
DownLoad: