Nomenclature: PV: Photovoltaic; DC: Direct Current; PCM: Phase Change Material; kWh: kilowatt-hours; η: Efficiency; Cu: Copper; Al: Aluminium; wt%: Weight Percentage; nm: Nanometre; W: Watts; Wp: Watt Peak; TEG: Thermoelectric Generator; TEC: Thermoelectric Cooler; HSTEG: Heat Sink Thermoelectric Generator
1.
Introduction
The reliance on fossil fuels for energy generation within the modern era of globalization has demonstrably caused severe environmental damage. The scientific community has recognized the crucial importance of sustainable energy sources, especially solar power, because of their distinct benefits. These merits include its remarkable abundance, economic viability, and straightforward implementation across various applications [1,2,3,4]. Solar energy possesses the unique distinction of being both a clean and dependable energy source, representing the most ancient form of energy harnessed by humankind [5,6]. The widespread adoption of solar water heaters in the early 1960s marked a significant inflection point, and presently, photovoltaic (PV) technology finds application in diverse sectors, including electricity generation, irrigation systems, building temperature control, and food dehydration [7]. Given the escalating global energy demands, solar energy is a practical and ecologically responsible solution, free from the acoustic pollution associated with conventional generators [8]. Solar energy collection is based on the core principle of transforming solar radiation, carried by photons, into usable direct current (DC) electricity through the photovoltaic effect. This conversion is achieved using PV panels made of semiconductor materials that can absorb incoming solar photons [9]. These modules' limited ability to convert solar irradiance into usable electrical energy is a significant challenge. The efficiency of this conversion, which varies based on the specific type of PV panel used, generally ranges between 12% and 25% [10]. Numerous factors contribute to this inefficiency, including the intensity of sunlight, wind speed, ambient humidity, dust accumulation on the module surface, shading, and the module's operating temperature. Elevated operating temperature has been recognized as the most prominent factor leading to efficiency degradation. Consequently, implementing effective cooling strategies to lessen thermal buildup within photovoltaic panels is vital. Research has shown that reducing the surface temperature of PV panels can improve their efficiency and overall operational longevity [11]. Although various cooling strategies for PV panels have been explored in the literature, most reviews tend to incorporate specific techniques, such as nanofluids or PCMs, without providing a comprehensive evaluation of diverse cooling methods [12,13,14,15,16].
1.1. Research gap
Despite significant advancements in PV cooling technologies, existing research lacks a holistic and comparative analysis of multiple emerging cooling methods. The absence of an extensive evaluation across multiple emerging technologies—such as heat pipes, heat sinks, holographic films, nanofluids, phase change materials (PCMs), thermoelectric modules, biomaterials, and hybrid cooling systems—limits the understanding of their relative effectiveness. Furthermore, they often provide generalized insights without systematically addressing key challenges such as scalability, cost-effectiveness, material sustainability, integration complexities, and real-world feasibility in diverse climatic conditions. A structured, critical analysis is needed to bridge this knowledge gap and guide the development of optimized, commercially viable, and sustainable cooling solutions for PV systems. This gap limits the development of optimized, commercially viable, and sustainable cooling solutions for PV systems. By addressing these shortcomings, this study seeks to bridge the divide between theoretical advancements and practical implementation, offering a roadmap for future research and innovation.
1.2. Research objective
This study aims to comprehensively and systematically evaluate emerging cooling technologies for photovoltaic (PV) panels, focusing on their effectiveness in enhancing thermal management, efficiency, and power output. Unlike conventional reviews that often emphasize a single cooling technique, this paper provides a comparative analysis of multiple advanced cooling strategies, including heat pipes, heat sinks, holographic films, nanofluids, phase change materials (PCMs), thermoelectric modules, biomaterials, and hybrid cooling systems.
Our primary objectives are to:
• Critically analyze the effectiveness of each cooling approach in terms of strength, integration challenges, and material suitability
• Compare key performance metrics, such as temperature reduction, power enhancement, and overall efficiency improvement.
• Identify gaps in existing research and propose future research directions, focusing on scalable, cost-effective, and sustainable cooling solutions suitable for real-world deployment.
1.3. Novelty statement
This review distinguishes itself by offering a comprehensive, comparative, and structured evaluation of multiple cooling technologies rather than focusing on a single approach. Unlike previous works, this study provides the synergistic potential of hybrid cooling solutions, explores material innovations for thermal management, and assesses these technologies' economic feasibility and large-scale applicability. Moreover, this review outlines practical and actionable future research directions, emphasizing advancements in design, scalability, material selection, and long-term field performance. By addressing both theoretical and applied perspectives, this study serves as a strategic guide for researchers, engineers, and policymakers, contributing to the development of next-generation thermal management solutions for photovoltaic applications. Additionally, the inclusion of recent advancements (2020–2024) ensures that the findings are aligned with the latest scientific and technological trends, making this review a timely and relevant resource for the field.
2.
Environmental factors influencing the performance of photovoltaic panels
2.1. Solar irradiance
Incident solar irradiance, measured in watts per square meter (W/m²), represents the spectral photon flux density emitted by the sun within the ultraviolet (UV) to near-infrared (IR) range, typically from 0.3 µm to 3 µm [17]. The positioning of a PV panel significantly impacts its capture of this irradiance. Established research demonstrates a positive correlation between the spectral irradiance and the electrical power output generated by a PV panel. In other words, as the intensity of incident solar irradiance increases, the corresponding electrical power output of the PV panel also rises. Figure 1 visually depicts this relationship.
2.2. Wind speed
Researchers have identified a positive correlation between wind velocity and the operational temperature of PV panels. As wind speeds rise, a corresponding decline in the module's operating temperature is observed. This phenomenon translates to an increase in power output from the PV panel. Figure 2 provides a visual representation of the inverse relationship between wind speed and module temperature. The underlying principle is that higher wind speeds promote a more efficient convective heat transfer rate away from the photovoltaic panel surface [19,20].
2.3. Humidity
Atmospheric humidity is the amount of water vapor contained in the air. Regions with high humidity levels, such as Malaysia (annual relative humidity: 75%–95%) and Singapore (relative yearly humidity: 82%) [22], might encounter the development of a thin layer of moisture on the surface of photovoltaic panels. This phenomenon can lead to a reduction in photovoltaic panel efficiency, as illustrated in Figure 3.
2.4. Dust accumulation
While environmental factors influence photovoltaic panels' efficiency, dust accumulation often receives less attention despite its significant impact. A layer of dust on the photovoltaic panel surface blocks the solar irradiance from reaching the underlying photovoltaic cells, thereby hindering power generation. Several studies have investigated the detrimental effects of dust deposition on PV panel performance. These studies reveal a substantial decrease in power output with increasing dust accumulation on the panel surface [24]. Figure 4 visually depicts the photovoltaic panel output power decline as dust accumulation increases from a clean state.
2.5. Shading
The power output of a PV panel exhibits a marked sensitivity to shadows cast upon its surface. These shadows effectively inhibit solar irradiance from reaching the panel, compromising its ability to generate electricity. Due to the series connection of individual cells within a PV panel, shading on any portion of the panel interrupts the flow of current across both the shaded and unshaded regions [19,26,27]. Figure 5 serves as a visual representation of the detrimental effect of shading on a PV panel's energy production (kWh).
2.6. Surface temperature
Operating temperature is arguably the most critical factor within the context of PV panel performance degradation. While some of the incident solar radiation is transformed into usable energy, the rest is converted into heat, increasing the module's temperature. This phenomenon is well-established within the scientific community, with a consensus that elevated surface temperatures demonstrably degrade photovoltaic panel performance. The impact of temperature fluctuations manifests across several key parameters like open-circuit voltage (Voc), short-circuit current (Isc), efficiency (η), and fill factor (FF). As temperature increases, the overall efficiency decreases [9,19,29]. Figure 6 visually depicts this inverse relationship between temperature and efficiency.
3.
Effects of a cooling system on photovoltaic panel temperature
3.1. Classification of different cooling techniques
Various cooling methods, including active, passive, and hybrid systems, have been developed to mitigate this issue and enhance the performance of PV panels [31]. The general classification of various cooling techniques is presented in Figure 7.
Active
Active solar cooling systems employ mechanical or electrical components to dissipate heat from photovoltaic panels. The key advantage of active cooling is its ability to provide consistent and controlled cooling, which can substantially improve the efficiency of PV panels by reducing their operating temperatures. However, the increased complexity and energy consumption of active cooling systems can also raise the overall system cost and maintenance requirements. Common active cooling approaches include using fans to blow air across the module surfaces or circulating coolant liquids through the modules to absorb and remove excess heat [16,32,33,34].
Passive
Passive cooling strategies for PV panels exploit natural heat transfer processes to remove heat without relying on external power sources. These systems typically employ heat sinks, fins, or other materials to absorb and dissipate heat generated by the PV panel. The inherent simplicity, low cost, and lack of energy consumption make passive cooling attractive. However, it is essential to acknowledge that environmental conditions can influence the effectiveness of these methods. In scenarios with peak solar irradiance, passive cooling might not consistently deliver sufficient temperature regulation for optimal PV performance [16,32,33,34].
Hybrid
Hybrid cooling systems combine active and passive cooling methods to enhance the thermal management of photovoltaic panels. These systems are designed to integrate these techniques strategically, focusing on maintaining ideal temperatures for photovoltaic panels while reducing energy consumption. By leveraging components from active and passive cooling strategies, hybrid systems have demonstrated more significant efficiency improvements than those achieved using only one approach. Research indicates hybrid systems yield better efficiency gains than purely active or passive cooling methods [35,36].
3.2. How might the temperature drop benefit the photovoltaic panel?
Expanding upon the findings presented in Section 2, this section focuses on the vital importance of thermal management in enhancing the efficiency and longevity of photovoltaic panels. Given that solar irradiance is an external factor that cannot be controlled, the main objective is to address the negative impacts of increasing module temperatures. An efficient cooling system is essential to optimize performance and mitigate these effects. This strategy improves efficiency and results in cost reductions due to the prolonged lifespan of photovoltaic panels. Three primary cooling methods are utilized: Active, passive, and hybrid. Active cooling methods, which include techniques using water or nanofluids, depend on external equipment to assist in heat dissipation. In contrast, passive cooling approaches, such as holographic films or natural convection heat sinks, function without external energy sources. Hybrid cooling systems effectively integrate aspects of both active and passive cooling techniques. In the following section, we comprehensively analyze these cooling strategies [37].
4.
Review of various cooling techniques
Heat pipe-based cooling system
The foundational research on heat pipes was initially conducted by Gaugler in 1944 and Trefethen in 1962. These devices operate as passive conduits for heat transfer, leveraging the latent heat of vaporization of a working fluid to attain remarkably high thermal conductivities without needing an external power source. The choice of working fluid and the design configuration of the heat pipe play a significant role in determining its operational temperature range [38].
In a published work by Basri et al. (2022) [39], a novel passive cooling approach utilizing heat pipes for PV panels was investigated to enhance their efficiency. The study employed two monocrystalline silicon photovoltaic panels, each with a rated power output of 10 watts. As depicted in Figure 8, one panel functioned as a reference module, while the other was integrated with a thermal management system consisting of flat heat pipes and heat sinks. Each cooling unit incorporated two flat plate heat pipes to facilitate thermal transfer between a pair of aluminum heat sinks, thereby promoting accelerated heat dissipation.
Eshghi et al. [40] conducted an experimental study using a 26.5 W monocrystalline photovoltaic panel to evaluate the cooling performance of a 10 mm diameter copper thermosyphon heat pipe mounted on the rear side of the panel. To optimize heat dissipation, an aluminum plate was integrated to enhance thermal conductivity between the heat pipe to the back of the photovoltaic panel. Distilled water, with varying filling ratios of 25%, 45%, and 65%, which is stored in a PVC tank for circulation, served as the working fluid. The findings were compared against a conventional photovoltaic panel with identical specifications. Detailed schematic diagrams of the cross-section and the experimental setup are provided in Figure 9(a,b), respectively.
Praveenkumar et al. [41] investigated commercially available CPU heat pipes for passive thermal management of PV panels. They employed two identical 30 W polycrystalline photovoltaic panels, one equipped with a cooling system and the other serving as a control. Figure 10(a) shows the test setup of water cooling, air cooling, and a CPU heat sink utilizing a heat pipe for passive heat transfer. The complete experimental configuration, including the pyranometer for solar irradiance measurement and the data logger for recording measurements, is presented in Figure 10(b).
Al-Amri et al. [42] performed unique research by studying both active and passive cooling methods. In the passive cooling approach, two PV panels were integrated with six heat sinks, with one set of heat sinks embedded in high-melting-point paraffin wax PCM to enhance thermal regulation. For the active cooling system, four heat pipes were affixed to the back of the PV panel, testing it with and without liquid immersion. The liquids used for immersion were water, ethylene glycol, and engine oil. A thermal image depicting the experimental results is shown in Figure 11.
Kaneesamkandi et al. [43] investigated the performance of a heat pipe thermosiphon (HPT) system in cooling PV panels, employing both theoretical modeling and experimental validation. The research utilized two 350 Wp polycrystalline photovoltaic panels, with one functioning as a control and the other integrated with a heat pipe thermosiphon that uses acetone as the working fluid. Acetone was selected for its relatively high boiling point of 56 ℃. The experiments employed a copper heat pipe (HPT) filled with varying amounts of acetone: 25 mL, 50 mL, 75 mL, and 100 mL, which corresponded to filling ratios of 3.39%, 6.79%, 10.18%, and 13.58%, respectively. The analytical findings were 2.61% consistent with the experimental outcomes. The experimental setup of the reference and HPT system is shown in Figure 12(a,b), respectively. The extended section of the copper heat pipe was also designed to facilitate effective heat transfer from the working fluid.
Sabry et al. [44] aimed to explore the performance of a multi-junction concentrator photovoltaic (CPV) cell combined with a copper heat pipe, a thermoelectric generator (TEG), and an aluminum heat sink for cooling purposes. They evaluated four distinct configurations: a long heat pipe (25 cm) paired with a heat sink (LHP + HS), a short heat pipe (15 cm) paired with a heat sink (SHP + HS), a long heat pipe with two TEGs connected to heat sinks (LHP + HS + TEG), and a short heat pipe with two TEGs connected to heat sinks (SHP + HS + TEG).
The five experiments, encompassing the heat pipe cooling technique, have been summarized in Table 1.
From the summary, the flat heat pipe combined with the heat sink technique shows the best output in terms of temperature reduction and cell efficiency improvement. When using two heat sinks with forced convection, 11.39 W power is obtained from the cooled panel, whereas only 9.73 W is delivered from the uncooled reference panel.
Heat Sink-based cooling system
Heat sinks are passive heat exchangers featuring extended fins with optimized shapes, for the thermal management of PV panels. Heat sinks utilize the principles of natural convection to dissipate heat through the increased surface area provided by the fins. To maximize thermal efficiency and keep the operating temperatures of the PV cells low, these heat sinks are commonly made from materials with high thermal conductivity, such as copper or aluminum. Copper is often preferred due to its exceptional heat conduction properties. Additionally, a heat sink's overall dimensions and fin design are crucial in determining its effectiveness. Much research has been conducted on employing heat sinks as a passive cooling solution to improve the efficiency of PV panels.
In a comprehensive investigation published by Arifin et al. [45], a combined computational and experimental approach was employed to evaluate the efficacy of air-cooled aluminum heat sinks for PV panel thermal management. The researchers utilized two 50 W polycrystalline photovoltaic panels. Figure 13(a) depicts the experimental setup while Figure 13(b) illustrates the configuration of the heat sink affixed to the rear surface of the PV panel. The experiment adopted a differential temperature measurement technique to quantify the heat sink's cooling effectiveness. This involved comparing the average temperature profiles of two panels: a reference panel without any cooling and a test panel equipped with an aluminum heat sink.
In a study by Arifin et al. [46], the effect of heat sink design on the thermal management of PV panels was studied. The investigation involved a 50 W polycrystalline silicon photovoltaic panel, which had a baseline efficiency of 14%. A reference panel lacking a heat sink was compared with a panel featuring various heat sink configurations. A reference panel without a heat sink was compared to one with various heat sink configurations. The experimental setup is illustrated in Figure 14. The researchers focused on how the number of fins and the heat sink material influenced cooling efficiency. Four different material pairings were tested: copper-copper (Cu-Cu), copper-aluminum (Cu-Al), aluminum-copper (Al-Cu), and aluminum-aluminum (Al-Al). Each combination was evaluated with 0, 5, 10, and 15 fins. The findings indicated that the number of fins had a more substantial impact on temperature reduction than the thermal conductivity of the heat sink material. This implies that the additional fins provide greater surface area, crucial for effective heat dissipation.
In their investigation into enhancing photovoltaic panel output using passive cooling techniques, Hudisteanu et al. [47] employed a monocrystalline silicon PV panel with a rated power of 320 Wp and a conversion efficiency of 19.30%. A copper heat sink was directly attached to the backside of the PV panel to facilitate heat dissipation. Two primary heat sink configurations were evaluated: one featuring horizontally oriented, non-perforated fins (Figure 15(a)), and the other utilizing vertically aligned, non-perforated fins (Figure 15(b)). For each fin orientation (horizontal and vertical), three variations were tested: (ⅰ) Non-perforated fins, (ⅱ) fins with perforations of 30 mm diameter, and (iii) fins with perforations of 60 mm diameter (Figure 15(c)).
Salehi et al. [48] experimented with the photovoltaic panel system's performance under two cooling systems: Natural convection and thermoelectric modules coupled with an anodized aluminum heat sink for a beat cooling effect using silicone adhesive. The outputs of the thermoelectric modules were connected in series and mounted to the back of one of the two 10 W polycrystalline photovoltaic modules. The thermoelectric module used is shown in Figure 16(a). The anodized aluminum heat sink is displayed in Figure 16(b).
In their research study, Krstic et al. [49] investigated the effectiveness of aluminum heat sinks of 36 different configurations in improving the performance of PV panels (Figure 17). One 100 Wp monocrystalline panel is used to compare the identical PV panel with 36 heat sinks with different configurations for cooling purposes. A thermal imaging camera was used to capture the temperature distribution of the front and rear sides of the panels. To validate and understand the heat transfer of the heat sinks in-depth, ANSYS Fluent software was utilized to simulate the temperature and airflow around the three best heat sinks obtained from the thermal imaging camera. The average difference of 1 ℃ between the experimental and numerical data is well accepted. The three best heat sinks, namely B1, B2, and B3, have different lengths and configurations. B1 has the most extended fin, smallest base, and large space between fins. B2 has a lower height than B1 and B3, longer fins, and a thinner base than B3. On the other hand, B3 has a similar structure and characteristics to B2 and a shorter fin length compared to B1.
Elminshawy et al. [50] bring novelty to their work in terms of the PV panel used. The researchers utilized 83 W polycrystalline to demonstrate the effectiveness of a heat sink in reducing the surface temperature of floating PV (FPV). Three different configurations have been introduced in this work: A bare floating PV (FPV), partially submerged PV (PSPV), and partially submerged PV attached with an aluminum heat sink (PSPV—AF). An uncertainty of 0.034% has been attained for the electrical efficiency, which indicates that the experimental data is reliable.
All five experiments using the heat sink cooling technique have been summarized in Table 2.
From the above summary, the horizontal fins with 30 mm diameter holes perform the best to reduce 14 ℃ of the surface temperature of the PV panel by improving 6.49% of power output to the base case scenario. This is due to the perforated holes in the heat sink, which allows more airflow to dissipate the heat from the heat sink.
Holographic film-based cooling system
We explore a novel light management strategy utilizing a transparent holographic film. This film incorporates an array of microscopic diffractive elements, technically termed "prismacons". The underlying principle revolves around the interaction of sunlight with a PV panel. As solar radiation heats the PV panel, the integrated array of equal-sided prisms within the film captures the incident light and redirects it via refraction before it reaches the panel's surface [51].
Kirpichnikova et al. [51] investigated the thermal performance of a PV panel integrated with a heat-protective holographic film using an experimental and numerical modeling approach. A regression model also has been developed between the PV panel temperature and ambient temperature which poses a strong positive linear relationship. They employed two monocrystalline silicon PV panels with identical 100 W power ratings. One module was equipped with the protective film, while the other served as a control without the film. The experimental setup is depicted in Figure 18. The authors utilized MATLAB to model the current-voltage (I-V) and power-voltage (P-V) characteristics of the film-integrated panel. Subsequently, a numerical model was validated between the experimental data and the simulation results. An uncertainty of ±1 has been obtained from the uncertainty test, which indicates the results are reliable.
The experiment using a holographic film-based cooling technique has been summarized in Table 3.
Nanofluid-based cooling system
Nanofluids are specially formulated suspensions of solid nanoparticles, generally measuring less than 100 nm in at least one dimension, distributed within base fluids like water, ethylene glycol, or oil. The idea of integrating nanoparticles into conventional heat transfer fluids was pioneered by Masuda in 1993. Subsequent experimental and theoretical investigations have shown that nanofluids often demonstrate improved thermal conductivity compared to their base fluids. For instance, studies indicate that adding just a 0.3% volume fraction of copper nanoparticles to ethylene glycol can enhance its thermal conductivity by up to 40%. This significant enhancement in heat transfer properties has led to considerable research interest in utilizing nanofluids as cooling agents for photovoltaic panels, as noted in [52].
In their investigation, Murtadha et al. [53] evaluated the efficacy of a two-pass circulation system employing titanium dioxide (TiO2) nanofluid for enhancing the thermal management of PV panels. The experiment adopted a comparative approach, analyzing the performance of five monocrystalline silicon photovoltaic panels: an uncooled reference panel, a panel with a conventional water-cooling system, and three panels employing nanofluid cooling systems with varying concentrations (1 wt%, 2 wt%, and 3 wt%). The detailed setup and sequence of the PV system utilized in the experiment are provided in Figure 19(a,b), respectively. A critical aspect of the experimental design was the implementation of a two-pass fluid circulation system, as illustrated in Figure 19(c). This configuration effectively doubled the coolant flow path, significantly enhancing the average temperature differential between the coolant and the panel surface. Consequently, this approach facilitated a more efficient heat transfer process.
Hamdan [54] investigated the cooling effects of water-based aluminum oxide (Al2O3) nanofluid and aluminum radiators functioning as heat sinks on the thermal regulation of photovoltaic panels. The experiment was conducted with four 345 Wp polycrystalline photovoltaic panels, where the first panel was the reference panel; the second panel was coated with Al2O3 nanofluid, leveraging its enhanced thermal conductivity to dissipate heat; the third panel was integrated with aluminum heat sinks attached to its rear side to facilitate passive cooling; and the fourth panel combined both strategies, incorporating heat sinks coated with Al2O3 nanofluid for improved heat dissipation.
Ibrahim et al. [55] investigated the impact of using water and aluminum oxide (Al2O3) nanofluid as cooling mediums in different photovoltaic panels, compared to a non-cooling system photovoltaic panel. A serpentine coil heat exchanger, constructed from semi-rectangular copper tubes welded onto a copper sheet, was installed to the back of the photovoltaic panels to facilitate heat dissipation. The concentration of the nanofluid changes from 0.01%, 0.03%, and 0.05%, with a differing mass flow rate of 0.03 kg/s and 0.07 kg/s for both water and nanofluid. Their findings revealed that irrespective of the cooling medium, reducing the mass flow rate led to a decline in cooling efficiency, resulting in higher panel surface temperatures even when the nanofluid concentration remained unchanged.
Jose et al. [56] intended to study the performance of a serpentine copper tube heat exchanger integrated with aluminum oxide (Al2O3) nanofluids at varying concentrations of 0.1% and 0.2%. The study was carried out using a 100W PV thermal (PVT) collector, where the cooling efficiency of nanofluids was compared against distilled water. To evaluate the impact of increasing nanoparticle concentration, the three fluids (distilled water, 0.1% Al2O3, and 0.2% Al2O3) were provided at mass flow rates of 0.015, 0.0133, and 0.0117 kg/s, respectively.
Salehi et al. [57] researched the effectiveness of aluminum nanofluid coupled with a heat sink in mitigating the overheating issue of the photovoltaic module. Another cooling system, water cooling, has been experimented with by the research team to compare surface temperature, power output, and conversion efficiency. A 2% volumetric water concentration was mixed with these 30 nm aluminum nanoparticles. To get a better understanding of the setup for this experiment, Figure 20(a,b) displays the experimental setup for the 10 W monocrystalline photovoltaic panel.
The nanofluid-based cooling system summary shows that the most power output and efficiency are achieved when Titanium Oxide (TiO2) nanofluid is utilized with its highest concentration of 3 wt%. The power and efficiency increase reached 45 W and 19%, respectively. However, the best nanofluid to be considered is the Aluminium Oxide (Al2O3) nanofluid when integrated with the aluminum heat sink. This novel combination achieves a 13 ℃ temperature reduction, which is much higher than TiO2. Al2O3 coupled with heat sink increases the output power by 13.7% and efficiency by 13.5%. Even though this is lower than TiO2, the lower cost and higher thermal conductivity of Al2O3 provide more merit. The five experiments using the nanofluid cooling technique have been summarized in Table 4.
PCM-based cooling system
Schlutz and Wren were among the first to implement PCMs in thermal management systems. One of the key benefits of PCMs is their substantial latent heat of fusion, which enables them to absorb considerable thermal energy with only a slight increase in temperature. This characteristic significantly improves the efficiency of cooling systems. Many researchers have investigated using PCMs specifically for cooling photovoltaic panels [58].
Velmurugan et al. [59] proposed a novel radiative cooling technique for PV panels employing a PCM matrix. This design deviates from conventional methods by incorporating a distinct cylindrical PCM matrix that maintains a contactless configuration with the rear surface of the PV panel by placing it at different distances from the panel, which are 6 mm, 9 mm, and 12 mm. This eliminates the potential for thermal and mechanical stress on the panel that may arise from direct physical contact. The detailed schematic and experimental configuration for the polycrystalline photovoltaic panel are depicted in Figure 21.
Rubaiee et al. [60] used three 50 W photovoltaic panels to investigate the performance of a passive PCM cooling system. Of the three panels, one has been made as the reference panel with no cooling system integrated, and the next panel is designed with a multi-pipe frame made from copper injected with paraffin wax as the PCM; moreover, Zinc Oxide (ZnO) is doped with 1% paraffin wax, which is also inserted into the copper frame with multiple pipes for the third-panel cooling method. The model of the experiment is shown in Figure 22.
Agarwal et al. [61] conducted an experimental study integrated with copper tubes made from 0.8 mm thin copper sheets filled with 90% RT27 PCM to enhance the thermal regulation of a 180 Wp photovoltaic panel. The experiment was structured into four configurations, a reference module (A), a module with 91 copper tubes filled with PCM (B), a module with 181 copper tubes (C), and a module with 271 copper tubes (D). In configurations B, C, and D, the copper tubes were positioned vertically downward beneath the photovoltaic panel to facilitate effective heat dissipation.
Durez et al. [62] conducted simulated three organic PCMs with different melting temperatures (RT21, RT35, and RT44) on a 10W monocrystalline photovoltaic panel model. These PCMs were selected based on previous studies showing efficiency improvements of over 17%. The computational model was validated against experimental results from Ciulla et al. (2012). The simulation was carried out over five months (April to August) across three geographically diverse locations, which were Bahawalpur, Bhadla, and Arizona. These locations were chosen due to their high solar irradiance and extreme temperature variations, which provided valuable insights into the thermal regulation effectiveness of PCM-based PV systems. They used a genetic algorithm in MATLAB to validate their model, which accurately predicted panel temperatures. The results showed RT21 as the best PCM for various climates. Figure 23 illustrates the PCM-based PV model.
Sheikh et al. [63] explored an innovative multi-layered PCM cooling system by integrating organic PCM (OPCM) and metallic PCM (MPCM). Initially, two organic PCMs, RT44 and RT64, were considered, but after further analysis, RT44 was selected for OPCM, while CERROLOW-117 alloy was used for MPCM. The numerical model was validated against experimental data from Biwole et al. (2013). They installed two layers of PCM at the back of the panel, placing an aluminum sheet under each layer. A total of ten configurations were tested, varying PCM type, layer combinations, and thicknesses to determine the most effective cooling strategy. The detailed experimental configurations are outlined in Table 5. After identifying the best combination of PCM, they aimed to determine the optimal tilt angle to maximize the cooling system's potential.
The five experiments utilizing the PCM cooling technique are summarized in Table 6.
According to the summary of the PCM-based cooling system, RT21 is the best option for PCM as the maximum temperature reduction is 29 ℃ with its maximum power output and maximum efficiency of 10.5% and 5.34%, respectively. Paraffin wax also performs well in reducing the PV temperature by 15% and increasing the power by 16 W. It is worth noting that RT21 PCM and Paraffin wax are more likely to share the same properties, but RT21 has a lower melting point of 21 ℃. RT21 PCM is engineered to obtain a higher thermal conductivity than Paraffin wax.
Thermo-electric (TEC)—based cooling system
TECs exploit the Peltier effect, which describes thermal energy transfer at the junction of dissimilar semiconductors due to an applied electric current. This phenomenon differs from the Seebeck effect, the principle behind thermoelectric generators, where a temperature difference generates a voltage. Discovered 13 years after Seebeck's work, the Peltier effect signifies heat absorption at one junction and heat release at the other when current flows through the circuit. The contemporary understanding of thermoelectricity employs a non-equilibrium approach, attributing a thermocouple's electromotive force (EMF) to a temperature-dependent gradient in the metal's electron concentration [64].
Metwally et al. [65] used ANSYS FLUENT to conduct a numerical analysis of an active water-cooling system designed to act as a heat sink for a thermoelectric generator (TEG). For the 40 × 40 mm TEG, they constructed a model with an 8 mm thick water module and a 1 mm water channel. Figure 24 illustrates the construction details of the photovoltaic panel. The numerical model was validated against experimental data from Kossyvakis et al. (2017), which indicates a good match between the simulation and experimental data. The performance of the active cooling system, coupled with the TEG, was simulated under three seasons, summer, winter, and spring, and two weather conditions, fair and sunny.
Khan et al. [66] developed a novel cooling system for the photovoltaic module using bismuth-telluride-based thermoelectric generators (TEG). The basic principle of harvesting the heat of the TEG, which aids in cooling, becomes the main idea of this research, where the researchers compared the performance of an uncooled PV panel with the PV panel attached to ten thermoelectric generators. Two 10 W polycrystalline photovoltaic panels were employed for this study. Figure 25(a) shows the uncooled PV panel used as the reference panel in this experiment. Ten TEGs attached to the rear side of the panel are displayed in Figure 25(b).
Praveenkumar et al. [67] studied the effectiveness of thermoelectric coolers (TECs) attached to the heat sink in lowering the operational temperature of the PV panel using two 30 W PV panels. 4 TECs were first attached to an aluminum sheet before being pasted behind the PV panel. Heat sinks and fans were also attached to the TECs' surface to enhance the cooling effect. The visual representations in Figure 26(a,b) give a better understanding of the cooling system approach, which has been investigated in this study.
Faheem et al. [68] explored a new method for hybrid solar thermoelectric generation (HSTEG) by integrating two types of thermoelectric modules (TEMs), which are thermoelectric coolers (TECs) and thermoelectric generators (TEGs). They configured four cases: A reference panel, active cooling with TECs connected to an additional power source, passive cooling with TEGs and a heat sink, and a hybrid combination of TECs and TEGs with a heat sink, known as the HSTEG system. The schematic diagram of the experiment is shown in Figure 27.
The five experiments encompassing the thermo-electric cooling technique are summarized in Table 7.
From the thermo-electric cooling system summary, it can be concluded that the thermo-electric is a good system to be utilized for cooling and obtaining additional power during sunny days. To maximize the potential of thermo-electric modules in cooling a PV panel, an additional system has to be added. When an aluminum heat sink is attached to the thermo-electric module, it provides better cooling. Among the thermoelectric coolers (TECs) and thermoelectric generators (TEGs), heat sink integrated TEG (HSTEG) reduced the temperature of the PV by 16.7 ºC and improved the efficiency of the system by 15.5.
Biomaterial-based cooling system
Various biomaterials, including naturally derived and synthetic alternatives, can be engineered for specific functionalities, including efficient heat transfer. This property, coupled with biocompatibility and minimal environmental impact, positions biomaterials as highly promising candidates for thermal management applications.
Ramkiran et al. [69], a comparative analysis was conducted to evaluate the efficacy of various sustainable passive cooling strategies for PV panels [36]. The investigation employed a 50 W polycrystalline PV panel to assess the impact of five distinct passive cooling techniques on cell temperature and electrical power output. These techniques included Plant cooling: This strategy leverages the evapotranspiration process of surrounding vegetation to achieve a cooling effect (Figure 28(b)) Greenhouse cooling: A greenhouse structure is constructed around the PV panel, facilitating natural air circulation and promoting heat dissipation (Figure 28(c)); Greenhouse cooling + plant cooling: This approach combines the benefits of both greenhouse cooling and plant evapotranspiration for enhanced thermal management (Figure 28(d)); Coir pith cooling: Coir pith, a natural fiber derived from coconut husks, is utilized as a cooling medium due to its high water-holding capacity and evaporative potential (Figure 28(e)); PCM cooling with paraffin wax: Paraffin wax, a PCM with a high latent heat of fusion, is incorporated into the system to absorb excess thermal energy during a phase change (Figure 28(f)). The performance of each cooling strategy was compared to an identical reference PV panel without any cooling mechanism (Figure 28(a)).
Dwivedi et al. [70] carried out three experiments, all with 30 W Polycrystalline photovoltaic panels but integrated with different cooling technologies. The researchers intended to study the performance of biomaterial products in cooling the photovoltaic module. For this reason, moist coconut fiber is chosen. A polyurethane sheet is used to encapsulate the wet coir pith. For comparison, an oscillatory flow design PVT system where the water flows at a rate of 0.02 kg/s and a reference module is employed. These two experiments encompassing the thermo-electric cooling technique are summarized in Table 8.
From the biomaterials used for the cooling system, the moist coconut pith technique is the best in reducing the temperature of the PV panel surface by about 15 ℃ from the reference panel. 24.21 W of power is exhibited from the moist coconut pith technique, which is 9.56 W higher than the reference PV panel.
Hybrid cooling system
In a hybrid system, a single PV panel contains multiple cooling systems. Researchers have explored a hybrid cooling system that utilizes two working fluids within a single PVT unit. This approach has demonstrably enhanced the efficiency of PVT systems and even regular photovoltaic panels with cooling.
Rukman et al. [71] examined the effect of an innovative bi-fluid cooling system combining air and water on the electrical performance of flexible PV panels. The investigation involved comparing the electrical properties of the panels equipped with the bi-fluid system to those without it, all under consistent solar irradiance of 800 W/m². Monocrystalline flexible PV panels rated at 100 W were used in both configurations. The bi-fluid cooling system maintained a constant water mass flow rate of 0.025 kg/s, while the air mass flow rate ranged from 0.04 kg/s to 0.10 kg/s. The study's main aim was to assess how the cooling system influenced the efficiency of the flexible PV panels.
Agyekum et al. [72] developed a unique hybrid cooling system that combines direct water cooling for the front surface and evaporative cooling for the rear side of a photovoltaic panel. Water flows from a PVC tank through 16 mm PVC pipes with 1 mm perforated holes, which cools the front of the 30 W photovoltaic panel. The water then collects in a basin and is recirculated back to the PVC tank using a water pump. The photovoltaic panel, tilted at 45° and facing south, features a cotton mesh wick on its rear surface. This wick, wrapped around the end of the PVC pipe from the storage tank, absorbs water via capillary action to cool the panel. To collect excess water from the wick, a perforated aluminum sheet is placed behind the panel. Figure 29 illustrates the schematic diagram of this experimental setup.
Chiang et al. [73] investigated a new hybrid approach that combines a loop thermosyphon, heat exchanger, and water-based heat recovery system for a PVT setup. The loop thermosyphon is attached to the back of the PV/T system using R600a refrigerant as the working fluid. The refrigerant transfers heat from the PV/T system to a shell and tube heat exchanger, where the vaporized heat is then transferred to water for hot water usage. Afterward, the refrigerant condenses and returns to the thermosyphon to repeat the process.
Azmi et al. [74] examined a conventional and straightforward hybrid cooling system using water and air. In their setup, three 50 W photovoltaic panels are tested outdoors. The first panel is a reference, while the second panel is equipped with a water-cooling system using two water sprinklers to cool the top surface. The third panel features a bi-fluid cooling system that combines the two water sprinklers with two air exhaust blowers to cool the back surface of the panel. This Arduino-based automated cooling system activates when the photovoltaic panel's surface temperature exceeds 50 ºC.
Madurai Elavarasan et al. [75] investigated a novel hybrid cooling approach that solely relies on passive cooling techniques. This system combines HS29 PCM, aluminum heat sinks, and still water. The study involved three experimental setups, the first being a reference panel, the second featuring a combination of PCM and aluminum heat sinks, and the third integrating PCM, and aluminum heat sinks, with the PV panel submerged in still water.
These five experiments encompassing the hybrid cooling technique are summarized in Table 9.
All the hybrid techniques reviewed show a 48.15 ℃ surface temperature for a bi-fluid, water, and air combination cooling system. The power output and efficiency are maximized at 0.025 kg/s of water flow and 0.08 kg/s air flow with a value of 7.384 W and 15.95%, respectively.
5.
Summary of research work and future research directions
5.1. Critical insights into various techniques
5.1.1. Heat pipe
Heat pipes are recognized for their exceptional thermal conductivity and efficiency in heat transfer, operating passively without needing external power. This has made them a prominent interest for researchers exploring thermal management solutions in photovoltaic panels. Researchers have examined various working fluids such as water, air, and acetone, with acetone emerging as the most effective choice due to its lower boiling point of 56 ℃. This enables acetone to efficiently dissipate excess heat from photovoltaic modules, while its high thermal conductivity further enhances the cooling process.
One of the most significant advantages of heat pipes is their passive operation, which does not require any additional power to operate the cooling system. This makes the heat pipe cooling technique an ideal solution for off-grid and remote solar installations. Unlike active cooling systems that involve moving parts, heat pipes operate silently, reducing mechanical wear and maintenance requirements. Additionally, their compact design enables them to be integrated into space-constrained solar modules, which makes them a better choice over the bulky traditional heat sinks. Their rapid thermal response ensures that heat is efficiently dissipated and minimizes hotspots. Furthermore, heat pipes demonstrate high thermal reliability, as their ability to function efficiently across varied temperature ranges makes them particularly suitable for regions with extreme climatic fluctuations.
Despite these advantages, heat pipes face significant challenges, primarily the installation cost. As heat pipes involve precision manufacturing and material selection to ensure optimal thermal performance and longevity, they often will be expensive. Another critical concern is system complexity. Heat pipes require careful thermal integration with PV panels, necessitating customized designs based on module size, orientation, and environmental conditions. Improper thermal coupling or inadequate heat pipe design can lead to inefficient heat dissipation. Manufacturing defects, such as improper sealing, can also lead to fluid leakage or clogging, which compromises the long-term functionality of the system.
5.1.2. Heat sink
Heat sinks are widely regarded as a cost-effective and efficient method for passive thermal management, relying on conduction and convection to dissipate heat. Extensive research on photovoltaic panel cooling has focused on optimizing heat sink designs, investigating materials such as aluminum and copper, and exploring diverse fin configurations to maximize heat dissipation. Effective thermal management is crucial for maintaining optimal photovoltaic panel temperatures, which improves efficiency and prolongs lifespan. The modular design of heat sinks allows for scalability, making them adaptable to various PV system configurations. Additionally, their passive cooling mechanism eliminates the need for external power sources, reducing operational costs and maintenance requirements. This also makes it a simple system to be installed.
Despite these advantages, heat sinks present several limitations. One of the primary challenges is their bulkiness, which restricts their integration into compact solar setups, such as rooftop solar installations. Furthermore, their effectiveness depends on natural convection, hindering their performance in low-wind environments. Another critical issue is the thermal contact between the heat sink and the PV panel. A small gap between the heat sink and the PV panel can reduce the heat dissipation efficiency. Material selection also poses a challenge, as aluminum, though cost-effective, has moderate thermal conductivity, while copper, despite its efficiency, is expensive and heavy. Additionally, environmental exposure can degrade heat sink performance over time making it not suitable for a longer period.
5.1.3. Holographic film
Holographic films hold the potential to enhance light absorption in photovoltaic panels while providing passive cooling by redirecting sunlight and lowering panel temperatures. These films can increase energy generation by concentrating light onto high-efficiency areas of the solar cells. However, only one experiment has been conducted solely using holographic films to regulate the temperature of a photovoltaic panel.
One of the primary advantages of holographic films is their passive cooling capability, which helps regulate PV panel temperatures without requiring additional energy input. This can lead to higher efficiency and prolonged PV panel lifespan. Additionally, holographic films are lightweight and thin, making them less intrusive compared to bulky cooling systems. Their ability to direct light towards the PV can also enhance energy conversion efficiency, particularly in multi-junction solar cells that benefit from targeted wavelength absorption.
Despite these advantages, the durability of holographic films is a major concern as it can lead to performance degradation over time. These films are prone to wear and tear, leading to reduced cooling effectiveness. Another critical drawback is the limited real-world validation of holographic films in PV panel cooling applications.
5.1.4. Nanofluid
Nanofluids, comprising nanoparticles dispersed in a base fluid, offer a promising advancement in enhancing heat transfer for photovoltaic panel cooling systems. By significantly increasing thermal conductivity, nanofluids can substantially improve the cooling efficiency of photovoltaic modules. Extensive research has focused on leveraging nanofluids to address the thermal management challenges of photovoltaic panels, making this approach one of the most extensively studied in the field. Among the various nanofluids investigated, Silicon Carbide (SiC) and Copper Oxide (CuO) are favored for their superior thermal conductivity, whereas Titanium Dioxide (TiO₂) and Aluminum Oxide (Al₂O₃) are less commonly used due to their lower thermal performance. Notably, SiC nanofluids demonstrate exceptional heat transfer capabilities and enhanced suspension stability compared to other nanofluids.
The ability of nanofluids to rapidly transfer heat away from solar panels is particularly valuable in regions with high solar irradiance, where excessive heat buildup can significantly reduce PV efficiency. Additionally, the small particle size and large surface area of nanofluids enable better thermal interaction with the base fluid, further boosting heat exchange efficiency.
Despite these advantages, one of the major concerns is the high cost of nanoparticles. Additionally, maintaining the long-term stability of nanoparticles in the base fluid remains a significant challenge. The potential clogging of cooling channels increases maintenance requirements. Unlike passive cooling methods, nanofluid-based cooling often requires active circulation systems involving additional components, which can increase system complexity. Additionally, environmental concerns related to the disposal of used nanofluids and the potential toxicity of certain nanoparticles need to be addressed. Some nanoparticles may pose health and ecological risks if not properly managed, making it essential to develop biodegradable or non-toxic nanofluids.
5.1.5. PCM
PCMs are vital for thermal energy storage, absorbing excess heat through phase transitions from solid to liquid to maintain efficient operation during high temperatures. Paraffin wax is commonly used in photovoltaic panel applications due to its superior thermal stability and cost-effectiveness compared to alternatives like calcium chloride hexahydrate. By undergoing a phase change from solid to liquid, PCMs effectively reduce thermal stress on PV panels, thereby enhancing efficiency and prolonging their operational lifespan.
One of the primary limitations of PCMs is their low thermal conductivity, particularly paraffin wax. This means that while PCMs can store heat effectively, they struggle to release it quickly, reducing their ability to maintain stable PV panel temperatures. Additionally, PCM leakage during repeated phase transitions presents a long-term durability concern. This leads to material loss and system degradation. The risk of leakage during phase changes poses a critical challenge for PCM adoption. Additionally, in extreme climates, PCMs may experience degradation over time, losing their phase change properties and requiring regular maintenance or replacement.
5.1.6. Thermoelectric modules
Thermoelectric modules offer a promising approach by converting excess heat into electricity, providing cooling and power generation. Their ability to convert excess heat into electricity offers a unique dual-benefit approach, making them an attractive option for enhancing PV efficiency and energy yield. One of the primary advantages of thermoelectric modules is that no moving parts are involved in this operation. This results in low maintenance and long operational life compared to mechanical cooling systems. Furthermore, their compact size and lightweight nature make them suitable for integration into rooftop PV. Additionally, thermoelectric modules can function in a passive mode when paired with PCMs or heat sinks. This enables them to dissipate excess heat more effectively without external power consumption.
Despite these advantages, thermoelectric modules suffer from several critical limitations. Low conversion efficiency remains the most significant challenge. Furthermore, their reliance on high-temperature gradients poses a significant obstacle, as PV panels rarely operate under such extreme thermal conditions. Additionally, thermoelectric materials are expensive due to the scarcity of raw materials. Moreover, hot-spot formation on PV panels due to non-uniform heat dissipation can degrade panel performance over time. Another engineering challenge is ensuring structural durability under outdoor conditions.
5.1.7. Biomaterials
Biomaterials, such as natural fibers and bio-based composites, offer an eco-friendly and sustainable alternative for cooling photovoltaic panels, reducing the environmental impact of their production. The primary advantage of biomaterials lies in their moisture retention capability, which enables passive evaporative cooling, thereby lowering the surface temperature of PV modules. Coir pith (coconut fiber) has emerged as a promising candidate due to its high water-holding capacity and slow evaporation rate, making it an effective passive cooling medium. Similarly, other organic materials, such as hay, have been considered; however, their lower water retention capabilities make them less effective for prolonged cooling applications.
One of the most significant limitations of biomaterials is their low thermal conductivity, which restricts them from dissipating heat efficiently. Additionally, durability concerns pose a major challenge. Natural fibers and bio-based composites are prone to degradation due to microbial growth. This can compromise their structural integrity and long-term performance.
5.1.8. Hybrid
Hybrid photovoltaic panel cooling systems combine multiple cooling techniques to enhance efficiency and mitigate the limitations of individual methods. These systems integrate passive and active cooling strategies, such as heat sinks with thermoelectric modules (TECs), PCMs with heat pipes, or air and water cooling with nanofluids, to achieve superior thermal regulation.
The primary advantage of hybrid cooling lies in its ability to leverage the strengths of multiple cooling technologies. This ensures greater temperature reduction, improved electrical efficiency, and extended module lifespan compared to a single cooling technique. One of the primary concerns is the increased cost and system complexity resulting from integrating multiple components. Moreover, higher maintenance requirements due to the involvement of multiple components can lead to operational difficulties and higher long-term expenses.
5.2. Practical implementation potential
5.2.1. Heat pipe
The practical deployment of heat pipe cooling is hindered by long-term reliability, heat pipes are prone to degradation due to environmental exposure, particularly in harsh climates. The risk of corrosion and deterioration can reduce thermal performance over time. Furthermore, scalability remains a significant challenge, as deploying heat pipes across large-scale solar farms would substantially increase costs and integration complexity. Heat pipes require precision engineering and customization to fit specific PV configurations, adding further to deployment costs.
5.2.2. Heat sink
The size and weight constraints of the heat sinks hinder their large-scale deployment. Real-world implementation requires maintenance which increases the cost. The limitations related to airflow dependency, material constraints, and thermal contact resistance remain a challenge in the practical implementation of heat sinks. The corrosion and durability issues make it difficult to install heat sink technology on a large scale.
5.2.3. Holographic film
The holographic film's practical implementation faces several technological and economic barriers. Furthermore, unlike well-established cooling methods such as heat pipes or nanofluid-based cooling, holographic films lack industry-standard performance benchmarks, making it difficult to compare their efficiency against alternative solutions. From a manufacturing standpoint, scalability remains a key barrier to widespread adoption.
5.2.4. Nanofluid
The practical challenge that hinders the widespread adoption of nanofluid-based cooling is the expensive large-scale implementation. The synthesis, functionalization, and stabilization of nanoparticles require sophisticated techniques that contribute to production costs. The risk of corrosion of system components, especially when using highly reactive nanoparticles, further complicates implementation. The energy required to circulate nanofluids must be carefully optimized to ensure that it does not significantly reduce the net energy gain from the PV system.
5.2.5. PCMs
The high cost of advanced PCMs makes them less economically viable for large-scale PV applications. Another practical challenge is the weight and volume of PCM-based systems, which could increase the structural load on PV installations. This limits their application in rooftop or lightweight solar panels. The degradation of PCM reduces the system reliability making it a crucial hindrance in practical implantation.
5.2.6. Thermoelectric module
Several challenges hinder their large-scale adoption, particularly their low efficiency, high material costs, and dependence on significant temperature differentials to function optimally. In real-world applications, temperature differentials on PV surfaces typically range between 20–50 ℃, which is often insufficient to generate meaningful thermoelectric output. The complex fabrication processes make large-scale production expensive compared to conventional cooling systems. Practical implementation challenges further complicate the integration of thermoelectric modules into PV systems. Even small gaps can significantly reduce heat transfer.
5.2.7. Biomaterials
Unlike conventional cooling techniques, biomaterials rely on external environmental factors, which can affect their cooling efficiency unpredictably. Furthermore, biomaterials exhibit high variability in composition and quality. This unpredictable efficiency and inconsistency make it difficult to achieve standardized performance across different applications, limiting their scalability for large-scale PV cooling solutions.
5.2.8. Hybrid
The real-world implementation of hybrid cooling also demands extensive customization based on environmental conditions. In hot and arid regions, passive cooling alone may be insufficient, requiring supplementary active cooling mechanisms. Conversely, in humid environments, nanofluid-based cooling or evaporative cooling could be more effective. However, they pose risks of fluid instability, fouling, and potential environmental concerns. Scalability remains a key issue, as hybrid cooling strategies that work efficiently in small-scale setups may not deliver proportional benefits when implemented in large solar farms.
5.3. Future research directions
5.3.1. Heat pipe
Future research on heat pipes must focus on cost-reduction strategies through the use of alternative materials and simplified manufacturing processes. Innovations in self-regulating heat pipes, which can adapt to varying heat loads, could improve scalability and reliability. Additionally, improving sealing techniques and anti-corrosion coatings could enhance long-term durability. This will significantly reduce maintenance challenges. Furthermore, novel working fluids that offer superior thermal properties and lower environmental impact should be identified. Hybrid approaches, integrating heat pipes with PCMs or thermoelectric modules, could further enhance performance.
5.3.2. Heat sink
To overcome these challenges, researchers should focus on innovations such as micro-fin or thin-film heat sinks, which could enhance cooling performance. Additionally, the exploration of advanced materials, such as graphene-infused aluminum, could improve thermal conductivity without adding excessive weight. Applying corrosion-resistant coatings may further enhance durability, ensuring long-term reliability in harsh environments. Another promising research direction in the future is the integration of heat sinks with hybrid cooling solutions, such as holographic film. Additionally, improving thermal interface materials (TIMs) to maintain better contact between the heat sink and photovoltaic panels, even under environmental stress, is key to minimizing performance degradation. Long-term field studies are essential to validate the real-world performance of heat sinks under diverse climatic conditions. By focusing on optimized design configurations, future advancements can significantly enhance the practicality and efficiency of heat sinks.
5.3.3. Holographic film
For holographic films to become a viable component in PV cooling systems, several research directions need to be explored. Hybrid integration strategies should be prioritized, where holographic films are combined with other cooling technologies to enhance their thermal regulation capabilities. Additionally, material innovation is crucial to developing coatings or treatments that can improve weather resistance and longevity. Research into self-cleaning or anti-reflective coatings could further enhance their practicality. To realize their full potential, long-term field studies are necessary.
5.3.4. Nanofluid
To overcome these challenges, researchers should focus on optimizing nanofluid formulations by exploring cost-effective and environmentally friendly nanoparticles. Incorporating self-cleaning or anti-fouling additives can help minimize maintenance challenges. Moreover, large-scale field testing under real-world conditions is essential to evaluate long-term performance and durability.
5.3.5. PCM
To overcome these issues, enhancing PCM performance is a key area of research. One promising approach is incorporating high-conductivity additives, such as graphene and carbon nanotubes. These additives can significantly improve the thermal conductivity of PCMs and enhance their ability to dissipate heat efficiently. Additionally, hybrid cooling solutions that integrate PCMs with active cooling systems, such as thermoelectric modules, could further improve heat dissipation and system reliability. Additionally, long-term field studies are necessary to evaluate the durability and real-world effectiveness of these improved PCM technologies.
5.3.6. Thermoelectric module
To make thermoelectric cooling a viable solution for PV panels, several research advancements are necessary. Material innovation is crucial, with ongoing research into nanostructured thermoelectric materials and hybrid composites aimed at improving efficiency at lower temperature gradients. Cost reduction strategies, including scalable fabrication techniques and the use of abundant, low-cost materials, are essential for commercial viability. Field testing under varying environmental conditions will provide valuable insights into their real-world performance. Additionally, techno-economic assessments are necessary to evaluate whether the energy recovered from thermoelectric generation can justify its high initial investment costs.
5.3.7. Biomaterials
To overcome these limitations, researchers should focus on enhancing the thermal properties and durability of biomaterials. Additionally, the development of composite biomaterials that integrate synthetic binders or protective coatings could improve their longevity and resistance to microbial degradation. Hybrid approaches that combine biomaterials with passive or active cooling methods such as PCMs or water-based cooling systems could provide more reliable and efficient cooling solutions.
5.3.8. Hybrid
To advance hybrid PV cooling technology, future research should focus on optimizing design configurations, reducing costs, and improving reliability. Computational modeling and simulation techniques can help predict system performance under different environmental conditions. Material innovations, such as highly conductive, lightweight PCMs and durable nanofluids, could enhance long-term efficiency. Moreover, field testing in diverse climates is crucial for evaluating practical feasibility. Rigorous experimental validation of these strategies will help translate theoretical advancements into real-world applications, ensuring the successful deployment of next-generation photovoltaic panel cooling solutions.
Table 10 presents a detailed comparative analysis of the reviewed cooling techniques, including the suitable materials for each method, their respective advantages and limitations, as well as performance benchmarks. These benchmarks include temperature reduction, power consumption increase, and efficiency enhancement.
5.4. Economic and environmental aspects of a cooling technique
Economic feasibility plays a crucial role in determining the practical implementation of cooling technologies for PV systems. Among the economic metrics, the Levelized Cost of Electricity (LCOE) serves as a key parameter for evaluating the cost-effectiveness of a given cooling technique. LCOE represents the cost of generating 1 kWh of electricity from a PV system over its lifetime. When assessing the feasibility of a PV cooling system, the cost of the cooling mechanism itself must be incorporated into the LCOE calculation, ensuring a comprehensive cost-benefit analysis [76].
Generally, PV panels without cooling systems tend to have a lower initial LCOE, as they do not incur additional costs. In contrast, while cooling systems may increase the initial cost, they can enhance power output and extend panel lifespan, potentially offsetting the added expense over the system's operational period. Despite the significance of LCOE in economic evaluations, very few studies have conducted an in-depth analysis of the LCOE implications of PV cooling systems. The lack of comprehensive LCOE assessments creates a knowledge gap, making it difficult to determine the long-term financial viability of integrating cooling technologies into PV applications [76].
Kumar et al. [77] conducted a comprehensive revision and update of the Levelized Cost of Energy (LCOE) for various passive cooling technologies used in PV systems. The updated findings serve as a valuable benchmark, which is presented in Table 11.
Hamed et al. [78] investigated three combinations of cooling techniques, which integrate with an aluminum heat sink and silica gel along with the reflector. A detailed cost analysis was performed for each component to study the economic feasibility of the proposed cooling system. In the study, it is revealed that the PV panel was priced at $0.80 and $0.90 for the aluminum heat sink, 1 kg silica gel at $1.50, and the 1 m2 reflector costs $2.01. The LCOE of each cooling configuration is summarized in Table 11.
The higher LCOE in Hamed et al. study for the PVs and aluminum heat sink is strongly associated with the system design, type of material, and implementation scale. Ramesh et al. suggest that heat sink cooling is the most feasible, while Hamad et al. suggest that the cooling technique integrated with silica gel is the best. However, the hybrid solution of silica gel and heat sink has a higher LCOE than the passive technique of heat sink. It is most acceptable that a hybridization of a cooling technique often requires higher costs as it possesses better benefits, including PV surface temperature reduction, power, and efficiency improvisation. Researchers should focus on long-term field validation, material cost reduction, and hybrid system efficiency improvements to achieve a balance between performance gains and economic feasibility.
In addition to economic considerations, the environmental impact of cooling systems must also be evaluated, particularly in terms of their potential to reduce CO₂ emissions. This aspect is critical for assessing the sustainability and ecological benefits of implementing such technologies [50].
Kumar et al. [77] introduced an innovative hybrid cooling system combining a thermoelectric generator (TEG), heat pipes, and radiative cooling. Through a comprehensive simulation study using COMSOL Multiphysics, they demonstrated that this system could significantly reduce CO2 emissions by an estimated 396 tons. This substantial reduction highlights the potential of integrating advanced cooling mechanisms to achieve both performance enhancement and environmental sustainability in photovoltaic systems. However, while the results are promising, the study's reliance on simulation-based data may necessitate further experimental validation to confirm its real-world applicability and scalability.
In contrast, Elminshawy et al. [50] explored a different approach by focusing on floating PV systems. They implemented aluminum heat sinks on the rear side of floating PV panels to lower operating temperatures and improve performance. While this experimental cooling technique successfully enhanced the system's efficiency, its environmental impact was relatively modest, mitigating only 1.83 tons of CO2. This stark difference in CO₂ reduction compared to Ramesh et al.'s system underscores the variability in environmental benefits across cooling strategies. It also raises questions about the trade-offs between system complexity, cost, and environmental impact.
These studies collectively emphasize the importance of balancing technological innovation with environmental and economic feasibility. Future research should focus on optimizing cooling systems to maximize both performance gains and CO2 reduction while ensuring cost-effectiveness and practical implementation in diverse photovoltaic applications. Additionally, comparative analyses of different cooling techniques, supported by both simulations and experimental data, could provide deeper insights into their relative merits and limitations.
6.
Conclusions
6.1. Key findings
Recent photovoltaic panel cooling technology advancements, focusing on experiments conducted between 2020 and 2024 are analyzed. A comprehensive overview of these techniques and their effectiveness in photovoltaic panel thermal energy management are provided. Key findings and practical implications are discussed below.
• Heat pipes provide an efficient and straightforward method for heat dissipation in photovoltaic panels, especially when paired with heat sinks to accelerate heat transfer to the environment. Despite their effectiveness, practical implementation is often constrained by maintenance challenges, including potential blockages and the need for frequent fluid replenishment, which may limit broader adoption. Future research should prioritize the development of low-maintenance or self-regulating heat pipe systems to improve their long-term performance and commercial feasibility.
• Heat sinks, crucial for thermal management, are available in various configurations and materials. While copper offers superior thermal conductivity, aluminum's lower cost, lighter weight, and compatibility with extrusion processes make it the preferred choice for most applications. Moreover, including strategically placed small holes in aluminum heat sinks can further enhance heat dissipation, ensuring optimal system performance. This balance of efficiency and practicality makes aluminum a versatile solution in thermal management systems.
• The findings revealed that holographic film, a simple and affordable solution, offers a promising approach to enhancing photovoltaic panel efficiency by refracting excess sunlight and focusing it more effectively. While it requires complementary technologies for long-term performance, its ease of implementation and minimal maintenance make it a viable option for industrial applications. Further research into optimizing holographic film performance is essential to maximize its potential benefits.
• Nanofluid cooling, while promising for its efficiency, presents practical challenges. The higher cost and need for external power may deter adoption in large-scale applications like solar power plants. Among nanofluids, Aluminium Oxide (Al2O3) nanofluid has demonstrated superior performance. However, nanoparticle concentration significantly impacts cooling efficiency, necessitating careful optimization to balance heat dissipation with potential clogging risks.
• While PCMs offer efficient cooling for photovoltaic panels, their high cost and potential maintenance issues limit their widespread adoption. RT21 PCM and Paraffin wax enhance the performance of the PV panel. However, the encapsulation and scaling challenges associated with PCMs necessitate further research and development to make this technology more economically viable and practical.
• The integration of thermoelectric modules with heat sinks enhances their cooling efficiency and electricity generation. While this technique shows potential for future applications, further research is needed to optimize this synergistic approach and fully realize its potential for sustainable energy production.
• A highly sustainable and eco-friendly approach to photovoltaic panel cooling involves the use of biomaterials, particularly moist coir pith. With its excellent water retention capacity, coir pith presents a promising solution for effective heat dissipation. However, challenges remain regarding its long-term durability and the need for frequent replacement, which may increase maintenance costs and limit its large-scale implementation in industrial applications.
• Hybrid water-air cooling systems demonstrate superior thermal performance compared to traditional methods. By combining the advantages of water and air cooling, these systems offer an efficient and sustainable solution for managing the heat generated by photovoltaic panels, particularly in regions with limited water resources.
• Hybrid cooling systems, combining the strengths of various cooling methods, represent the future of photovoltaic module temperature management. These systems offer superior performance and economic advantages while minimizing environmental impact. However, a comprehensive environmental assessment ensures these systems align with sustainability goals.
• This research highlights the need for significant improvements in the material properties of cooling systems and emphasizes the critical importance of real-world field testing.
6.2. Limitations
• We focused on a specific subset of research literature, potentially narrowing the findings' scope and limiting the conclusions' comprehensiveness.
• Variations in experimental methodologies and conditions across the reviewed studies challenge the establishment of consistent comparisons and the drawing of generalized conclusions.
• We did not extensively address the economic feasibility or provide a thorough environmental assessment of the cooling technologies discussed, which restricts understanding their practical applicability and long-term sustainability in real-world scenarios.
Use of AI tools declaration
During the preparation of this work, the author(s) used AI tools to improve the language and clarity of the text. After using the AI tool, the author(s) reviewed and edited the content as needed and took full responsibility for the content of the publication.
Acknowledgements
The authors would like to acknowledge the financial support received under the Internal Research grant (Grant Number: RDU223310) from Universiti Malaysia Pahang Al-Sultan Abdullah. The authors also acknowledge the scientific testing and technical facilities from the Fluid Centre and the Automotive Engineering Centre, UMPSA.
Conflict of interest
The authors declare that they have no known competing financial interests or personnel relationships that could have appeared to influence the work reported in this article.
Author contributions
Kaovinath Appalasamy: Conceptualization, Data curation, Investigation, Methodology, Resources, Software, Visualization, Roles/Writing—original draft. R Mamat: Formal analysis, Funding acquisition, Project administration, Resources, Supervision, Validation, Writing—review & editing. Sudhakar Kumarasamy: Conceptualization, Formal analysis, Methodology, Project administration, Resources, Supervision, Validation, Writing—review & editing.
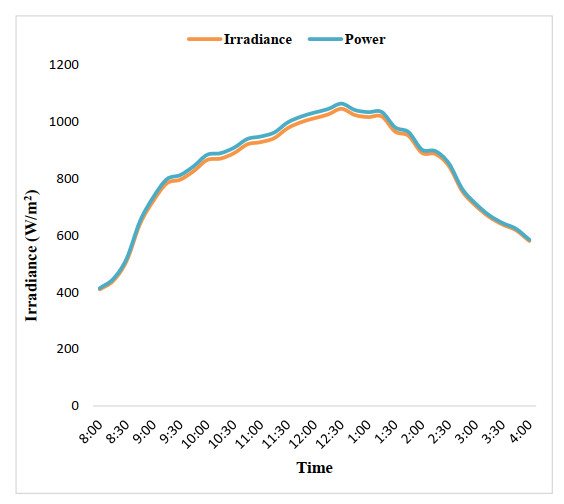









 DownLoad:
DownLoad: