Search Advanced

Mathematical Biosciences and Engineering

2021, Volume 18, Issue 5: 6386-6409. doi: 10.3934/mbe.2021317
Previous Article Next Article
Review Special Issues

Development of surrogate models in reliability-based design optimization: A review

  • 1.
    Henan Key Laboratory of Mechanical Equipment Intelligent Manufacturing, School of Mechanical and Electrical Engineering, Zhengzhou University of Light Industry, Zhengzhou, MO 450002, China
  • 2.
    Zhengzhou Railway Vocational and Technical College, Zhengzhou, MO 451460, China
  • 3.
    China Railway Engineering Equipment Group Co., Ltd, Zhengzhou, MO 450002, China
  • Received: 27 May 2021 Accepted: 06 July 2021 Published: 21 July 2021

  • Reliability-based design optimization (RBDO) is applied to handle the unavoidable uncertainties in engineering applications. To alleviate the huge computational burden in reliability analysis and design optimization, surrogate models are introduced to replace the implicit objective and performance functions. In this paper, the commonly used surrogate modeling methods and surrogate-assisted RBDO methods are reviewed and discussed. First, the existing reliability analysis methods, RBDO methods, commonly used surrogate models in RBDO, sample selection methods and accuracy evaluation methods of surrogate models are summarized and compared. Then the surrogate-assisted RBDO methods are classified into global modeling methods and local modeling methods. A classic two-dimensional RBDO numerical example are used to demonstrate the performance of representative global modeling method (Constraint Boundary Sampling, CBS) and local modeling method (Local Adaptive Sampling, LAS). The advantages and disadvantages of these two kinds of modeling methods are summarized and compared. Finally, summary and prospect of the surrogate–assisted RBDO methods are drown.

    Citation: Xiaoke Li, Qingyu Yang, Yang Wang, Xinyu Han, Yang Cao, Lei Fan, Jun Ma. Development of surrogate models in reliability-based design optimization: A review[J]. Mathematical Biosciences and Engineering, 2021, 18(5): 6386-6409. doi: 10.3934/mbe.2021317

    Related Papers:

  • Reliability-based design optimization (RBDO) is applied to handle the unavoidable uncertainties in engineering applications. To alleviate the huge computational burden in reliability analysis and design optimization, surrogate models are introduced to replace the implicit objective and performance functions. In this paper, the commonly used surrogate modeling methods and surrogate-assisted RBDO methods are reviewed and discussed. First, the existing reliability analysis methods, RBDO methods, commonly used surrogate models in RBDO, sample selection methods and accuracy evaluation methods of surrogate models are summarized and compared. Then the surrogate-assisted RBDO methods are classified into global modeling methods and local modeling methods. A classic two-dimensional RBDO numerical example are used to demonstrate the performance of representative global modeling method (Constraint Boundary Sampling, CBS) and local modeling method (Local Adaptive Sampling, LAS). The advantages and disadvantages of these two kinds of modeling methods are summarized and compared. Finally, summary and prospect of the surrogate–assisted RBDO methods are drown.


    加载中


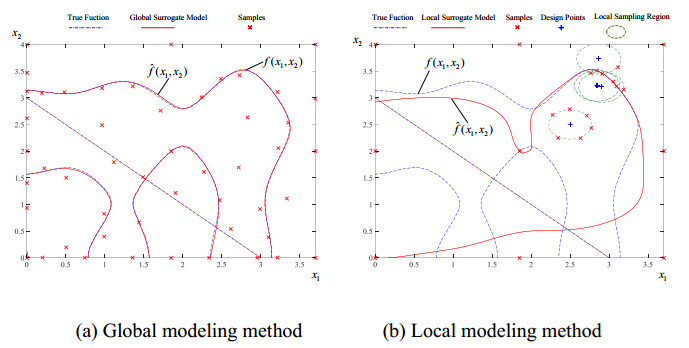
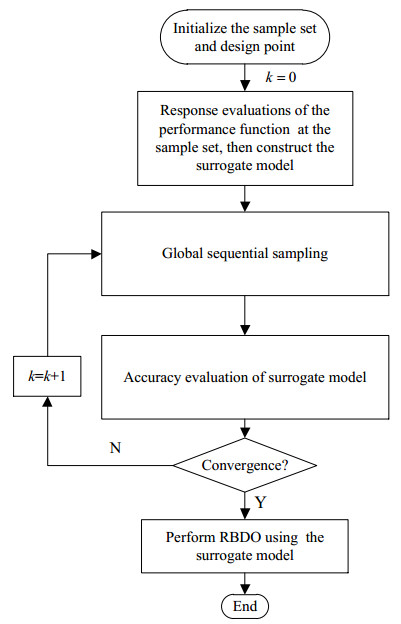
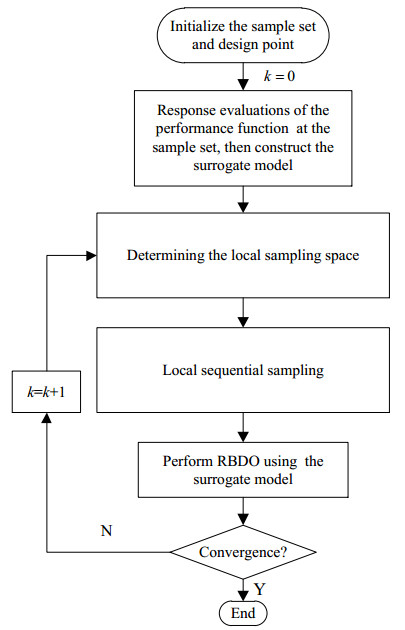
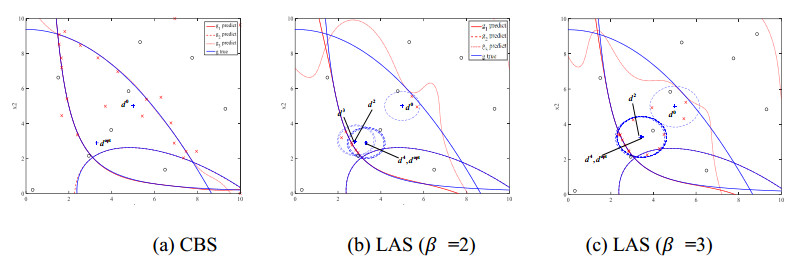
    [1] A. T. Beck, W. J. D. Gomes, A comparison of deterministic, reliability-based and risk-based structural optimization under uncertainty, Probabilist. Eng. Mech., 28 (2012), 18-29.
    [2] T. Zou, S. Mahadevan, A direct decoupling approach for efficient reliability-based design optimization, Struct. Multidiscip. O., 31 (2006), 190-200. doi: 10.1007/s00158-005-0572-7
    [3] R. H. Lopez, A. T. Beck, Reliability-based design optimization strategies based on FORM: A review, J. Braz. Soc. Mech. Sci., 34 (2012), 506-514. doi: 10.1590/S1678-58782012000400012
    [4] Z. Z. Chen, H. B. Qiu, L. Gao, P. G. Li, An optimal shifting vector approach for efficient probabilistic design, Struct. Multidiscip. O., 47 (2013), 905-920. doi: 10.1007/s00158-012-0873-6
    [5] L. Shi, S. P. Lin, A new RBDO method using adaptive response surface and first-order score function for crashworthiness design, Reliab. Eng. Syst. Safe, 156 (2016), 125-133. doi: 10.1016/j.ress.2016.07.007
    [6] B. D. Youn, K. K. Choi, L. Du, Enriched performance measure approach for reliability-based design optimization, AIAA J., 43 (2005), 874-884. doi: 10.2514/1.6648
    [7] S. Goswami, S. Chakraborty, R. Chowdhury, T. Rabczuk, Threshold shift method for reliability-based design optimization, Struct. Multidiscip. O., 60 (2019), 2053-2072. doi: 10.1007/s00158-019-02310-x
    [8] X. P. Du, W. Chen, A most probable point-based method for efficient uncertainty analysis, J. Design. Manuf. Autom., 4 (2001), 47-66. doi: 10.1080/15320370108500218
    [9] X. P. Du, W. Chen, Y. Wang, Most probable point-based methods, A. Singhee, R. Rutenbar (eds), Extreme Statistics in Nanoscale Memory Design, Boston, MA, 2010.
    [10] J. E. Hurtado, D. A. Alvarez, A method for enhancing computational efficiency in Monte Carlo calculation of failure probabilities by exploiting FORM results, Comput. Struct., 117 (2013), 95-104. doi: 10.1016/j.compstruc.2012.11.022
    [11] A. E. Ismail, A. K. Ariffin, S. Abdullah, M. J. Ghazali, Probabilistic Assessments of the Plate Using Monte Carlo Simulation, IOP. Conf. Ser. Mater. Sci. Eng., 17 (2011), 012029.
    [12] R. H. Lopez, J. E. S. de-Cursi, D. Lemosse, Approximating the probability density function of the optimal point of an optimization problem, Eng. Optimiz., 43 (2011), 281-303. doi: 10.1080/0305215X.2010.489607
    [13] Z. Liang, Reliability-based design optimization using surrogate model with assessment of confidence level, The University of Iowa, 2011.
    [14] E. Zio, Reliability engineering: Old problems and new challenges, Reliab. Eng. Syst. Safe., 94 (2009), 125-141. doi: 10.1016/j.ress.2008.06.002
    [15] T. W. Lee, B. M. Kwak, A reliability-based optimal design using advanced first order second moment method, Mech. Struct. Mach., 15 (1987), 523-542. doi: 10.1080/08905458708905132
    [16] M. Hohenbichler, S. Gollwitzer, W. Kruse, R. Rackwitz, New light on first-and second-order reliability methods, Struct. Saf., 4 (1987), 267-284. doi: 10.1016/0167-4730(87)90002-6
    [17] J. Lim, B. Lee, I. Lee, Second‐order reliability method‐based inverse reliability analysis using Hessian update for accurate and efficient reliability‐based design optimization, Int. J. Numer. Meth. Eng., 100 (2014), 773-792. doi: 10.1002/nme.4775
    [18] J. F. Zhang, X. P. Du, A second-order reliability method with first-order efficiency, J. Mech. Design, 132 (2010), 101006.
    [19] G. Lee, S. Yook, K. Kang, D. H. Choi, Reliability-based design optimization using an enhanced dimension reduction method with variable sampling points, Int. J. Precis. Eng. Man., 13 (2012), 1609-1618. doi: 10.1007/s12541-012-0211-3
    [20] G. Bird, Monte-Carlo simulation in an engineering context, Rare. Gas. Dynam., 1 (1981), 239-255.
    [21] M. A. Valdebenito, G. I. Schueller, A survey on approaches for reliability-based optimization, Struct. Multidiscip. O., 42 (2010), 645-663. doi: 10.1007/s00158-010-0518-6
    [22] B. Echard, N. Gayton, M. Lemaire, AK-MCS: An active learning reliability method combining Kriging and Monte Carlo Simulation, Struct. Saf., 33 (2011), 145-154. doi: 10.1016/j.strusafe.2011.01.002
    [23] P. W. Glynn, D. L. Iglehart, Importance sampling for stochastic simulations system, Manage. Sci., 35 (1989), 1367-1392.
    [24] S. K. Au, J. L. Beck, A new adaptive importance sampling scheme for reliability calculations, Struct. Saf., 21 (1999), 135-158. doi: 10.1016/S0167-4730(99)00014-4
    [25] I. Depina, T. M. H. Le, G. Fenton, G. Eiksund, Reliability analysis with Metamodel Line Sampling, Struct. Saf., 60 (2016), 1-15. doi: 10.1016/j.strusafe.2015.12.005
    [26] S. F. Song, Z. Z. Lu, W. W. Zhang, Z. Y. Ye, Reliability and Sensitivity Analysis of Transonic Flutter Using Improved Line Sampling Technique, Chinese. J. Aeronaut., 22 (2009), 513-519. doi: 10.1016/S1000-9361(08)60134-X
    [27] P. Bjerager, Probability integration by directional simulation, J. Eng. Mech-Asce., 114 (1988), 1285-1302. doi: 10.1061/(ASCE)0733-9399(1988)114:8(1285)
    [28] O. Ditlevsen, R. E. Melchers, H. Gluver, General multi-dimensional probability integration by directional simulation, Comput. Struct., 36 (1990), 355-368. doi: 10.1016/0045-7949(90)90134-N
    [29] S. k. Au, J. L. Beck, Subset simulation and its application to seismic risk based on dynamic analysis, J. Eng. Mech-Asce., 129 (2003), 901-917. doi: 10.1061/(ASCE)0733-9399(2003)129:8(901)
    [30] I. Papaioannou, W. Betz, K. Zwirglmaier, D. Straub, MCMC algorithms for subset simulation, Probabilist. Eng. Mech., 41 (2015), 89-103.
    [31] S. F. Song, Z. Z. Lu, H. W. Qiao, Subset simulation for structural reliability sensitivity analysis, Reliab. Eng. Syst. Safe., 94 (2009), 658-665. doi: 10.1016/j.ress.2008.07.006
    [32] M. Rosenblatt, Remarks on a multivariate transformation, Ann. Math. Stat., 23 (1952), 470-472. doi: 10.1214/aoms/1177729394
    [33] P. L. Liu, A. D. Kiureghian, Multivariate distribution models with prescribed marginals and covariances, Probabilist. Eng. Mech., 1 (1986), 105-112. doi: 10.1016/0266-8920(86)90033-0
    [34] P. T. Lin, H. C. Gea, Y. Jaluria, A modified reliability index approach for reliability-based design optimization, J. Mech. Design, 133 (2011), 044501.
    [35] I. Enevoldsen, J. D. Sørensen, Reliability-based optimization in structural engineering, Struct. Saf., 15 (1994), 169-196. doi: 10.1016/0167-4730(94)90039-6
    [36] S. C. Kang, H. M. Koh, J. F. Choo, Reliability-based design optimisation combining performance measure approach and response surface method, Struct. Infrastruct. E., 7 (2011), 477-489. doi: 10.1080/15732479.2010.493335
    [37] B. D. Youn, K. K. Choi, L. Du, Enriched performance measure approach for reliability-based design optimization, AIAA J., 43 (2005), 874-884. doi: 10.2514/1.6648
    [38] J. Tu, K. K. Choi, Y. H. Park, A new study on reliability-based design optimization, J. Mech. Design, 121 (1999), 557-564. doi: 10.1115/1.2829499
    [39] H. O. Madsen, P. F. Hansen, A comparison of some algorithms for reliability based structural optimization and sensitivity analysis, Springer, Berlin, Heidelberg, (1992), 443-451.
    [40] N. Kuschel, R. Rackwitz, Two basic problems in reliability-based structural optimization, Math. Method. Oper. Res., 46 (1997), 309-333. doi: 10.1007/BF01194859
    [41] H. Agarwal, C. K. Mozumder, J. E. Renaud, L. T. Watson, An inverse-measure-based unilevel architecture for reliability-based design optimization, Struct. Multidiscip. O., 33 (2007), 217-227. doi: 10.1007/s00158-006-0057-3
    [42] C. Jiang, H. B. Qiu, L. Gao, X. W. Cai, P. G. Li, An adaptive hybrid single-loop method for reliability-based design optimization using iterative control strategy, Struct. Multidiscip. O., 56 (2017), 1271-1286. doi: 10.1007/s00158-017-1719-z
    [43] Y. Aoues, A. Chateauneuf, Benchmark study of numerical methods for reliability-based design optimization, Struct. Multidiscip. O., 41 (2010), 277-294. doi: 10.1007/s00158-009-0412-2
    [44] M. Yang, D. Q. Zhang D, X. Han. Enriched single-loop approach for reliability-based design optimization of complex nonlinear problems, Eng. Comput-Germany., 2020.
    [45] D. Lehký, O. Slowik, D. Novák, Reliability-based design: Artificial neural networks and double-loop reliability-based optimization approaches, Adv. Eng. Softw., 117(2018), 123-135. doi: 10.1016/j.advengsoft.2017.06.013
    [46] J. Ni, K. H. Yu, Z. F. Yue, Reliability-based multidisciplinary design optimization for turbine blade using double loop approach, J. Aerospace. Power., 24 (2009), 2051-2056.
    [47] W. Li, Y. Li, An effective optimization procedure based on structural reliability, Comput. Struct., 52 (1994), 1061-1067. doi: 10.1016/0045-7949(94)90090-6
    [48] H. Agarwal, J. E. Renaud, New decoupled framework for reliability-based design optimization, AIAA J., 44 (2006), 1524-1531. doi: 10.2514/1.13510
    [49] K. Y. Chan, S. J. Skerlos, P. Papalambros, An adaptive sequential linear programming algorithm for optimal design problems with probabilistic constraints, J. Mech. Design, 129 (2007), 140-149. doi: 10.1115/1.2337312
    [50] G. D. Cheng, L. Xu, L. Jiang, A sequential approximate programming strategy for reliability-based structural optimization, Comput. Struct., 84 (2006), 1353-1367. doi: 10.1016/j.compstruc.2006.03.006
    [51] Y. T. Wu, W. Wang, Efficient probabilistic design by converting reliability constraints to approximately equivalent deterministic constraints, J. Integr. Des. Process. Sci., 2 (1998), 13-21.
    [52] X. P. Du, W. Chen, Sequential optimization and reliability assessment method for efficient probabilistic design, J. Mech. Design, 126 (2004), 225-233. doi: 10.1115/1.1649968
    [53] C. Jiang, H. B. Qiu, X. K. Li, Z. Z. Chen, L. Gao, P. G. Li, Iterative reliable design space approach for efficient reliability-based design optimization, Eng. Comput-Germany., 36 (2020), 151-169.
    [54] Z. Z. Chen, H. B. Qiu, L. Gao, L. Su, P. G. Li, An adaptive decoupling approach for reliability-based design optimization, Comput. Struct., 117 (2013), 58-66. doi: 10.1016/j.compstruc.2012.12.001
    [55] T. M. Cho, B. C. Lee, Reliability-based design optimization using convex linearization and sequential optimization and reliability assessment method, Struct. Saf., 33 (2011), 42-50. doi: 10.1016/j.strusafe.2010.05.003
    [56] X. P. Du, Saddlepoint Approximation for Sequential Optimization and Reliability Analysis, J. Mech. Design, 130 (2008), 011011.
    [57] G. Kharmanda, A. Mohamed, M. Lemaire, Efficient reliability-based design optimization using a hybrid space with application to finite element analysis, Struct. Multidiscip. O., 24 (2002), 233-245. doi: 10.1007/s00158-002-0233-z
    [58] G. Kharmanda, S. Sharabaty, H. Ibrahim, A. El-Hami, Reliability-based design optimization using semi-numerical methods for different engineering application, Int. J. Cad/Cam., (2009).
    [59] A. Mohsine, G. Kharmanda, A. El-Hami, Improved hybrid method as a robust tool for reliability-based design optimization, Struct. Multidiscip. O., 32 (2006), 203-213. doi: 10.1007/s00158-006-0013-2
    [60] A. Mohsine, A. El-Hami, A robust study of reliability-based optimization methods under eigen-frequency, Comput. Method. Appl. M., 199 (2010), 1006-1018. doi: 10.1016/j.cma.2009.11.012
    [61] G. Kharmanda, M. H. Ibrahim, A. A. Al-Kheer, F. Guerin, A. El-Hami, Reliability-based design optimization of shank chisel plough using optimum safety factor strategy, Comput. Electron. Agr., 109 (2014), 162-171. doi: 10.1016/j.compag.2014.09.001
    [62] G. Kharmanda, N. Olhoff, Extension of optimum safety factor method to nonlinear reliability-based design optimization, Struct. Multidiscip. O., 34 (2007), 367-380. doi: 10.1007/s00158-007-0107-5
    [63] A. Yaich, G. Kharmanda, A. El-Hami, L. Walha, M. Haddar, Reliability based design optimization for multiaxial fatigue damage analysis using robust hybrid method, J. Mech., 34 (2018), 551-566. doi: 10.1017/jmech.2017.44
    [64] K. Dammak, A. Yaich, A. El-Hami, L. Walha, An efficient optimization based on the robust hybrid method for the coupled acoustic-structural system, Mech. Adv. Mater. Struc., 27 (2019), 1816-1826.
    [65] B. Debich, A. El-hami, A. Yaich, W. Gafsi, L. Walha, M. Haddar, An efficient reliability-based design optimization study for PCM-based heat-sink used for cooling electronic devices, Mech. Adv. Mater. Struc., (2020), 1-13.
    [66] A. Kamel, K. Dammak, A. Yaich, A. El-Hami, M. Ben-Jdidia, L. Hammami, M. Haddar, A modified hybrid method for a reliability-based design optimization applied to an offshore wind turbine, Mech. Adv. Mater. Struc., 6 (2020), 1-14.
    [67] A. Garakani, M. Bastami, An evolutionary approach for structural reliability, Struct. Eng. Mech., (2019).
    [68] R. Yadav, R. Ganguli, Reliability based and robust design optimization of truss and composite plate using particle swarm optimization, Mech. Adv. Mater. Struc., 6 (2020), 1-11.
    [69] C. Tong, H. L. Gong, A hybrid reliability algorithm using PSO-optimized Kriging model and adaptive importance sampling, IOP. Conf. Ser. Earth. Environ. Sci., 128 (2018), 012094.
    [70] J. Q. Chen, X. S. Zhang, Z. Jing, A cooperative PSO-DP approach for the maintenance planning and RBDO of deteriorating structures, Struct. Multidiscip. O., 58 (2018), 95-113. doi: 10.1007/s00158-017-1879-x
    [71] X. N. Fan. J. X. Zhou, A Reliability-based Design optimization of Crane Metallic Structure based on Ant colony optimization and LHS, 13th World Congress on Intelligent Control and Automation (WCICA), Changsha, PRC, 1470-1475, 2018.
    [72] M. G. C. Santos, J. L. Silva, A. T. Beck, Reliability-based design optimization of geosynthetic-reinforced soil walls, Geosynth. Int., 25 (2018), 442-455. doi: 10.1680/jgein.18.00028
    [73] N. M. Okasha, Reliability-Based Design Optimization of Trusses with Linked-Discrete Design Variables using the Improved Firefly Algorithm, Engineering-Prc., 6 (2016), 964-971.
    [74] C. Jiang, Z. Hu, Y. Liu, Z. P. Mourelatos, P. Jayakumar, A sequential calibration and validation framework for model uncertainty quantification and reduction, Comput. Method. Appl. M., 368 (2020), 113172.
    [75] K. Dammak, A. Elhami, Thermal reliability-based design optimization using Kriging model of PCM based pin fin heat sink, Int. J. Heat. Mass. Tran., 166 (2021), 120745.
    [76] K. Dammak, A. Elhami, Multi-objective reliability-based design optimization using Kriging surrogate model for cementless hip prosthesis, Comput. Method. Biomec., 23 (2020), 854-867. doi: 10.1080/10255842.2020.1768247
    [77] X. K. Li, F. H. Yan, J. Ma, Z. Z. Chen, X. Y. Wen, Y. Cao, RBF and NSGA-Ⅱ based EDM process parameters optimization with multiple constraints, Math. Biosci. Eng., 16 (2019), 5788-5803. doi: 10.3934/mbe.2019289
    [78] Y. S. Yeun, B. J. Kim, Y. S. Yang, W. S. Ruy, Polynomial genetic programming for response surface modeling Part 2: adaptive approximate models with probabilistic optimization problems, Struct. Multidiscip. O., 29 (2005), 35-49. doi: 10.1007/s00158-004-0461-5
    [79] Y. Shi, Z. Lu, R. He, Y. Zhou, S. Chen, A novel learning function based on Kriging for reliability analysis, Reliab. Eng. Syst. Safe, 198 (2020), 106857.
    [80] B. H. Ju, B. C. Lee, Reliability-based design optimization using a moment method and a Kriging metamodel, Eng. Optimiz., 40 (2008), 421-438. doi: 10.1080/03052150701743795
    [81] J. Ma, X. Y. Han, Q. Xu, S. H. Chen, W. B. Zhao, X. K. Li, Reliability-based EDM process parameter optimization using kriging model and sequential sampling, Math. Biosci. Eng., 16 (2019), 7421-7432. doi: 10.3934/mbe.2019371
    [82] M. Q. Chau, X. Han, C. Jiang, Y. C. Bai, T. N. Tran, V. H. Truong, An efficient PMA-based reliability analysis technique using radial basis function, Eng. Computation., 31 (2014), 1098-1115. doi: 10.1108/EC-04-2012-0087
    [83] Y. Wang, X. Q. Yu, X. P. Du, Improved reliability-based optimization with support vector machines and its application in aircraft wing design, Math. Probl. Eng., 17(2015), 3127-3141.
    [84] Q. Zhou, Y. Wang, S. K. Choi, P. Jiang, X. Y. Shao, J. X. Hu, A sequential multi-fidelity metamodeling approach for data regression. Knowl-Based. Syst., 134 (2017), 199-212.
    [85] T. D. Robinson, M. S. Eldred, K. E. Willcox, R. Haimes, Surrogate-based optimization using multifidelity models with variable parameterization and corrected space mapping, AIAA J., 46 (2008), 2814-2822. doi: 10.2514/1.36043
    [86] S. E. Gano, J. E. Renaud, H. Agarwal, A. Tovar, Reliability-based design using variable-fidelity optimization, Struct. Infrastruct. E., 2 (2006), 247-260. doi: 10.1080/15732470600590408
    [87] X. K. Li, H. B. Qiu, Z. Jiang, L. Gao, X. Y. Shao, A VF-SLP framework using least squares hybrid scaling for RBDO, Struct. Multidiscip. O., 55 (2017), 1629-1640. doi: 10.1007/s00158-016-1588-x
    [88] M. G. Fernández-Godino, C. Park, N. H. Kim, R. T. Haftka, Issues in deciding whether to use multifidelity surrogates, AIAA J., (2019), 1-16.
    [89] E. Acar, M. Rais-Rohani, Ensemble of metamodels with optimized weight factors, Struct. Multidiscip. O., 37 (2009), 279-294. doi: 10.1007/s00158-008-0230-y
    [90] T. Goel, R. T. Haftka, W. Shyy, N. V. Queipo, Ensemble of surrogates, Struct. Multidiscip. O., 33 (2007), 199-216.
    [91] X. K. Li, J. G. Du, Z. Z. Chen, W. Y. Ming, Y. Cao, W. B. He, J. Ma, Reliability-based NC milling parameters optimization using ensemble metamodel, Int. J. Adv. Manuf. Tech., 97 (2018), 3359-3369. doi: 10.1007/s00170-018-2211-7
    [92] L. M. Chen, H. B. Qiu, C. Jiang, X. W. Cai, L. Gao, Ensemble of surrogates with hybrid method using global and local measures for engineering design, Struct. Multidiscip. O., 57 (2017), 1711-1729.
    [93] K. Crombecq, E. Laermans, T. Dhaene, Efficient space-filling and non-collapsing sequential design strategies for simulation-based modeling, Eur. J. Oper. Res., 214 (2011), 683-696. doi: 10.1016/j.ejor.2011.05.032
    [94] T. Golshani, E. Jorjani, C. S. Chehreh, S. Z. Shafaei, H. Y. Nafechi, a Modeling and process optimization for microbial desulfurization of coal by using a two-level full factorial design, Int. J. Min. Sci. Techno., 23 (2013), 261-265. doi: 10.1016/j.ijmst.2013.04.009
    [95] M. Buragohain, C. Mahanta, A novel approach for ANFIS modelling based on full factorial design, Appl. Soft. Comput., 8 (2008), 609-625. doi: 10.1016/j.asoc.2007.03.010
    [96] P. G. Duan, Y. Y. Wang, Y. Yang, L. Y. Dai, Optimization of Adiponitrile Hydrolysis in Subcritical Water Using an Orthogonal Array Design, J. Solution. Chem., 38 (2009), 241-258. doi: 10.1007/s10953-008-9362-3
    [97] J. Liu, Q. B. Wang, H. T. Zhao, J. A. Chen, Y. Qiu, Optimization design of the stratospheric airship's power system based on the methodology of orthogonal experiment, J. Zhejiang. Univ-Sc. A., 14 (2013), 38-46. doi: 10.1631/jzus.A1200138
    [98] K. T. Fang, D. K. J. Lin, Uniform design in computer and physical experiments, Springer, Tokyo, (2008), 105-125.
    [99] K. T. Fang, Z. H. Yang, On uniform design of experiments with restricted mixtures and generation of uniform distribution on some domains, Stat. Probabil. Lett., 46 (2000), 113-120. doi: 10.1016/S0167-7152(99)00095-4
    [100] B. G. M. Husslage, G. Rennen, E. R. V. Dam, D. D. Hertog, Space-filling Latin hypercube designs for computer experiments, Optim. Eng., 4 (2006), 611-630.
    [101] G. G. Wang, Adaptive response surface method using inherited latin hypercube design points, J. Mech. Design, 125 (2003), 210-220. doi: 10.1115/1.1561044
    [102] Z. Z. Chen, H. B. Qiu, L. Gao, X. K. Li, P. G. Li, A local adaptive sampling method for reliability-based design optimization using Kriging model, Struct. Multidiscip. O., 49 (2014), 401-416. doi: 10.1007/s00158-013-0988-4
    [103] C. Jiang, H. B. Qiu, Z. Yang, L. M. Chen, L. Gao, P. G. Li, A general failure-pursuing sampling framework for surrogate-based reliability analysis, Reliab. Eng. Syst. Safe., 183 (2019), 47-59. doi: 10.1016/j.ress.2018.11.002
    [104] X. Li, H. B. Qiu, Z. Z. Chen, L. Gao, X. Y. Shao, A local Kriging approximation method using MPP for reliability-based design optimization, Comput. Struct., 162 (2016), 102-115. doi: 10.1016/j.compstruc.2015.09.004
    [105] N. C. Xiao, M. J. Zuo, C. N. Zhou, A new adaptive sequential sampling method to construct surrogate models for efficient reliability analysis, Reliab. Eng. Syst. Safe., 169 (2018), 330-338. doi: 10.1016/j.ress.2017.09.008
    [106] L. Zhao, K. K. Choi, I. Lee, L. Du, Response surface method using sequential sampling for reliability-based design optimization, International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, San Diego, California, USA, 2009.
    [107] S. Mahadevan, R. Rebba, Inclusion of model errors in reliability-based optimization, J. Mech. Design, 128 (2006), 936-994. doi: 10.1115/1.2204973
    [108] C. Currin, T. Mitchell, M. Morris, D. Ylvisaker, A Bayesian Approach to the Design and Analysis of Computer Experiments, Office of scientific & technical information technical reports, USA, 1988.
    [109] G. Li, V. Aute and S. Azarm, An accumulative error based adaptive design of experiments for offline metamodeling, Struct. Multidiscip. O., 40 (2010), 137-155. doi: 10.1007/s00158-009-0395-z
    [110] C. Jiang, H. B. Qiu, L. Gao, D. P. Wang, Z. Yang, L. M. Chen, Real-time estimation error-guided active learning Kriging method for time-dependent reliability analysis, Appl. Math. Model., 77 (2020), 82-98. doi: 10.1016/j.apm.2019.06.035
    [111] M. E. Johnson, L. M. Moore, D. Ylvisaker, Minimax and maximin distance designs, J. Stat. Plan. Infer., 26 (1990), 131-148.
    [112] S. L. Xu, H. T. Liu, X. F. Wang, X. M. Jiang, A robust error-pursuing sequential sampling approach for global metamodeling based on voronoi diagram and cross validation, J. Mech. Design, 136 (2014), 69-74.
    [113] T. H. Lee, J. J. Jung, A sampling technique enhancing accuracy and efficiency of metamodel-based RBDO: Constraint boundary sampling, Comput. Struct., 86 (2008), 1463-1476. doi: 10.1016/j.compstruc.2007.05.023
    [114] Z. Meng, D. Q. Zhang, Z. T. Liu, G. Li, An adaptive directional boundary sampling method for efficient reliability-based design optimization, J. Mech. Design, 140 (2018), 121406.
    [115] B. J. Bichon, M. S. Eldred, L. P. Swiler, S. Mahadevan, J. M. McFarland, Efficient global reliability analysis for nonlinear implicit performance functions, AIAA J., 46 (2008), 2459-2468. doi: 10.2514/1.34321
    [116] S. Q. Shan, G. G. Wang, Survey of modeling and optimization strategies to solve high-dimensional design problems with computationally-expensive black-box functions, Struct. Multidiscip. O., 41 (2010), 219-241. doi: 10.1007/s00158-009-0420-2
    [117] G. Shieh, Improved shrinkage estimation of squared multiple correlation coefficient and squared cross-validity coefficient, Organ. Res. Methods., 11 (2008), 387-407. doi: 10.1177/1094428106292901
    [118] C. J. Willmott, K. Matsuura, Advantages of the mean absolute error (MAE) over the root mean square error (RMSE) in assessing average model performance, Clim. Res., 30 (2005), 79-82. doi: 10.3354/cr030079
    [119] X. K. Li, X. Y. Han, Z. Z. Chen, W. Y. Ming, Y. Cao, J. Ma, A multi-constraint failure-pursuing sampling method for reliability-based design optimization using adaptive Kriging, Eng. Comput-Germany., 2020.
    [120] I. Lee, K. K. Choi, D. Gorsich, Sensitivity analyses of FORM-based and DRM-based performance measure approach (PMA) for reliability-based design optimization (RBDO), Int. J. Numer. Meth. Eng., 82 (2010), 26-46. doi: 10.1002/nme.2752
    [121] Z. Z. Chen, S. P. Peng, X. K. Li, H. B. Qiu, H. D. Xiong, L. Gao, P. G. Li, An important boundary sampling method for reliability-based design optimization using kriging model, Struct. Multidiscip. O., 52 (2015), 55-70. doi: 10.1007/s00158-014-1173-0
    [122] L. Zhao, K. K. Choi, I. Lee, D. Gorsich, Conservative surrogate model using weighted kriging variance for sampling-based RBDO, J. Mech. Design, 135 (2014), 1-10.
    [123] M. Moustapha, B. Sudret, Surrogate-assisted reliability-based design optimization: a survey and a new general framework, Struct. Multidiscip. O., 60 (2019), 2157-2176. doi: 10.1007/s00158-019-02290-y
    [124] B. J. Bichon, S. Mahadevan, M. S. Eldred, Reliability-based design optimization using efficient global reliability analysis, 50th AIAA/ASME/ASCE/AHS/ASC Structures, Structural Dynamics, and Materials Conference, 2009.
    [125] A. Basudhar, C. Dribusch, S. Lacaze, S. Missoum, Constrained efficient global optimization with support vector machines, Struct. Multidiscip. O., 46 (2012), 201-221. doi: 10.1007/s00158-011-0745-5
    [126] B. J. Bichon, M. S. Eldred, S. Mahadevan, J. M. McFarland, Efficient Global Surrogate Modeling for Reliability-Based Design Optimization, J. Mech. Design, 135 (2013), 011009.
    [127] K. Crombecq, D. Gorissen, D. Deschrijver, T. Dhaene, A novel hybrid sequential design strategy for global surrogate modeling of computer experiments, Siam. J. Sci. Comput., 33 (2011), 1948-1974. doi: 10.1137/090761811
    [128] X. K. Li, H. B. Qiu, Z. Z. Chen, L. Gao, X. Y. Shao, A local sampling method with variable radius for RBDO using Kriging, Eng. Computation., 32 (2015), 1908-1933. doi: 10.1108/EC-09-2014-0188
    [129] X. Liu, Y. Z. Wu, B. X. Wang, J. W. Ding, H. X. Jie, An adaptive local range sampling method for reliability-based design optimization using support vector machine and Kriging model, Struct. Multidiscip. O., 55 (2017), 2285-2304. doi: 10.1007/s00158-016-1641-9
    [130] I. Lee, K. K. Choi, L. Zhao, Sampling-based RBDO using the stochastic sensitivity analysis and Dynamic Kriging method, Struct. Multidiscip. O., 44 (2011), 299-317. doi: 10.1007/s00158-011-0659-2
    [131] X. Liu, Y. Z. Wu, B. X. Wang, Q. Yin, J. J. Zhao, An efficient RBDO process using adaptive initial point updating method based on sigmoid function, Struct. Multidiscip. O., 58 (2018), 2583-2599. doi: 10.1007/s00158-018-2038-8
  • Reader Comments
  • © 2021 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)
通讯作者: 陈斌, bchen63@163.com
  • 1. 

    沈阳化工大学材料科学与工程学院 沈阳 110142

  1. 本站搜索
  2. 百度学术搜索
  3. 万方数据库搜索
  4. CNKI搜索
Mathematical Biosciences and Engineering
3.9

Metrics

Article views(4643) PDF downloads(563) Cited by(2)

Preview PDF
Download XML
Export Citation
Article outline

Figures and Tables

Figures(8)  /  Tables(3)

Other Articles By Authors

Related pages

Tools

/

DownLoad:  Full-Size Img  PowerPoint
Return
Return

Catalog