Research on meme transmission based on individual heterogeneity

1.   Introduction

The term meme was first introduced by Dawkins ^[1], which means something similar to the role played by genes in the process of biological evolution in transmission of language, ideas, beliefs, and behavior patterns. It refers to a new gene copy, cultural transmission unit or imitation unit. A meme is a replicator in the field of culture; therefore, it is used to spread and achieves evolution of ideology such as culture and behavior from the perspective of ontology. Susan ^[2] further explored the concept of memes from a broader perspective, and reported that all information that can be copied through imitation can be called meme. The concept of memes has evolved from a basic cultural replication unit to anything that can be copied and transmitted. In the modern network environment, a catchword, a song, a video, news and an advertisement can all be regarded as a meme. The emergence of memes provides a clear path for information dissemination and cultural inheritance.

The era of social network media has changed the way of information transmission in traditional media. Individuals have more rights to autonomy, participation and communication. Therefore, the complex social network relations and individual heterogeneity can easily integrate into the information communication and become important factors affecting information communication. Communication is no longer a simple viral information transmission in a specific context, but has changed to replicable and mutable meme transmission with a basic frame structure, and pays more attention to individual participation and interaction. Kate Distin ^[3] reported that a meme evolves based on the survival of the fittest as the case of biological evolution, and conforms to the concept and behavior of the meme host, such as intentionality and consciousness. Reproduction and propagation of any meme inevitably involves understanding and deduction of the subject. Transmission of information and instructions contained in a meme is accompanied by obscurity and distortion in expression. Therefore, intentionality of the subject and the way of understanding and decoding plays an important role in communication ^[4].

2.   Research review

Study of meme communication ^[5,6] has attracted attention of scholars from physics, sociology, communication, management and other disciplines. A large number of meme communication studies from the field of social physics are available based on theories such as complex adaptive system and information communication dynamics due to advances in the complex network theory.

Bauckhage C ^[7] used the stochastic model, lognormal model and infectious disease model to fit the propagation trend of meme. The findings of the study showed that the propagation trend of a meme can be well fitted with lognormal distribution ^[8]. This study verified and predicted the propagation development trend of some Internet memes very accurately. Wang L et al. ^[9] reported that a meme is a 'contagious' cultural factor and used SIR model to model and explore if the trend of meme transmission was consistent with that of infectious diseases. Analysis showed a state of peak rise in the early stage and a gradual decline in the middle and late stage. Miller I and Cupchik G ^[10] used the SIS infectious disease model to simulate the transmission process of a meme in the network. The findings showed that the transmission process of Internet meme was similar to the sharing process. Wei X et al. ^[11] used the improved SI²S infectious disease model to simulate the transmission process of a competitive meme. Freitag D et al. ^[12] analyzed users' clicks on meme information on the network, and used SIR model to fit the propagation trend and propagation process of Internet memes. Towers S et al. ^[13] conducted comparative experiments with different types of video memes, and reported that the SIR chamber model accurately fits the promotion or inhibition effects of memes in the transmission process. Kwiatkow ska J et al. ^[14] divided Facebook users into susceptible group, disseminators and correctors groups, and performed a simulation and verification of Internet meme transmission on small-world networks. Smailhodzic A et al. ^[15] analyzed the popular Internet memes on the Twitter platform and used the SIR infectious disease model, and the results effectively predicted the spread of Ebola virus. Sun W et al. ^[16] added the P-state group to SIR model, simulated the network meme transmission process, generated the SIPR compartment model, and reported that the interference rate affected the final distribution of the system population. Zhang D ^[17] reports that individual innovation behavior has a significant effect on Internet meme transmission. A previous study constructed a SIInR model to simulate meme propagation based on the traditional SIR model. The simulation results showed that high infection rate, contact rate, low recovery rate, innovation rate and immunity rate can promote sustainable development of a meme. Sachak-Patwa R et al. ^[18,19] used Multi-group SEIRS delay differential equation epidemic model for the popularity evolution of viral videos. The findings showed that the multi-group epidemic modeling approach including time delays and coupling between videos is a useful tool for understanding dynamics of viral videos which are connected.

In studies on the propagation model construction of meme, most studies set the propagation of meme to be analogous to the spread of infectious diseases, and conduct research and simulation using chamber model. Stability of the basic structure of meme indicates that the transmission process of meme is similar to the transmission of infectious diseases. A meme is transmitted as an information regardless of its form, and the difference only lies in the form of expression. Analysis of meme transmission shows that not all individuals can become transmitters after receiving memes. Most individuals immediately ignore memes based on individual heterogeneity judgment. This shows that not all memes can achieve large-scale 'contagion'. Compartment model is mostly used for simulation in studies of meme transmission. Although the diffusion situation of meme transmission is restored to a certain extent, the heterogeneity of individuals in meme transmission is ignored. Complex behaviors, attitudes and other individual heterogeneity characteristics occur due to differences in education, personality and other basic characteristics among individuals. These feature expressions are quickly integrated into the network transmission, and affect the propagation and evolution of the meme. Therefore, this study sought to construct a meme transmission model based on the classic SEIR model using the internal mechanism of meme transmission and the expression of individual heterogeneity factors.

3.   Model construction of meme transmission

3.1.   SIR-EIR chamber model

Spread of an epidemic is based on the probability of exposure and infection. In the classic SEIR model, nodes are compartmentalized as susceptible, exposed, infected, and recovered states. Susceptible individuals are people susceptible to the disease. Exposed individuals are infected individuals and subjects currently in the latent period without infections. Infected individuals are subjects spreading disease. Individuals in the recovered state are subjects who have recovered from the disease. Susceptible individuals first change to the exposed state, then transform into the infection state, and may obtain immunity with a certain probability. Previous studies have achieved advances in propagation dynamics analysis of SEIR model, model expansion and evolution based on different situations. These models include ordinary differential equation model ^[20,21], reaction diffusion model ^[22] and time-delay model ^[23,24]. The aim of most studies is to determine the global stability of the system based on the basic regenerative number R₀. Han S et al. ^[25] explored a diffuse SEIR epidemic model with nonlinear incidence form, and evaluated the global stability of epidemic equilibrium and disease-free equilibrium by constructing an appropriate Lyapunov function. Ávila-Vales E et al. ^[26] explored a class of periodic SEIRS epidemic models with general morbidity and reported that the basic regenerative number determines the global stability of the model and is a threshold parameter for persistence of the disease. The basic reproduction number is estimated by numerical simulation using nonlinear incidence.

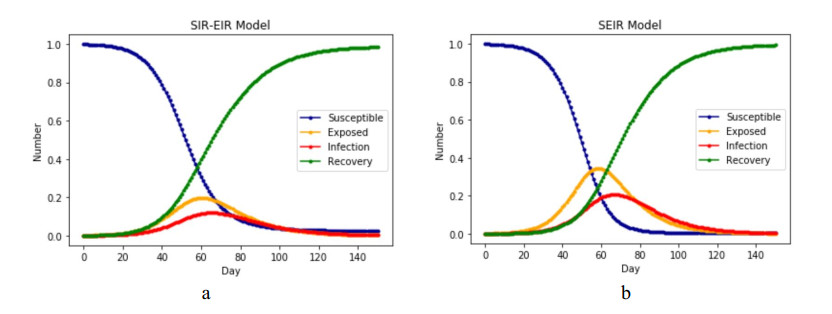
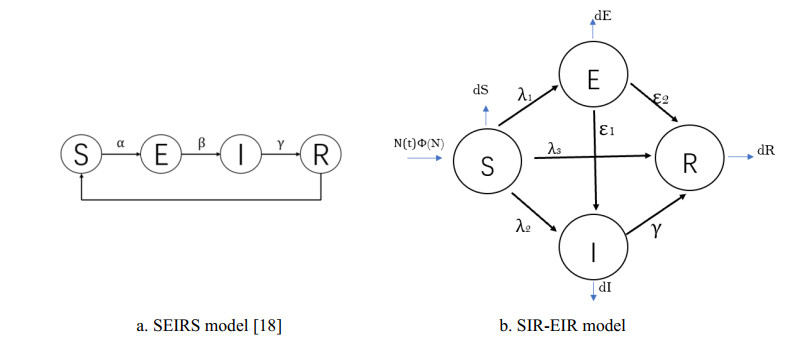
Some studies report that the recovered state is only a process, and will be re-infected. Previous studies proposed the SEIRS delay differential equation epidemic model to describe the popularity evolution of viral videos ^[18,19]. In this model, individuals move through the susceptible, exposed, infected and recovered states and back to the susceptible state, where the cycle repeats itself (Figure 1a). The models incorporate time-delay to accurately describe the virtual contact process between individuals and temporary immunity of individuals to videos after they get tired of watching them. In addition, time delay is added to accurately describe the virtual contact process between individuals, however, it does not consider the perception and judgment of the video or information for individuals with subjective initiative and other possible paths for individual state transitions.

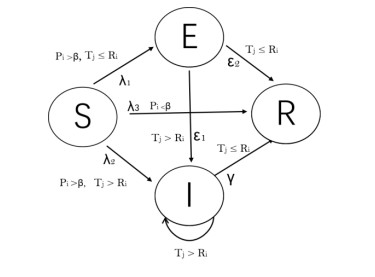
However, during transmission of a meme, individuals as the carriers have the right to decide whether to spread the meme or not. When individuals are exposed to memes with a certain probability, they make judgments based on their own preferences, trust, risk perception and other individual heterogeneity. Not all the memes they are exposed to are transmitted. The process of individual judgment is a process of individual heterogeneity expression, and is the fundamental difference between meme transmission and epidemic transmission. Individuals have different processing results for meme information during meme transmission based on the topic and credibility of the meme. Susceptible nodes can be directly transformed into infection state transmission nodes with infectious ability through judgment, and can be temporarily transformed into hesitating state nodes. In addition, susceptible nodes which are not interested in memes can be directly transformed into immune state. Hesitating nodes are not contagious, but have known the information of the meme. They may receive the meme from the neighbor nodes and turn into infection nodes, or they can be transformed into immune nodes due to interference from other factors. Communication intention of individuals mainly depends on the transmission capacity of meme and individual heterogeneity factors without consideration of the effect of network structure. The specific influence mode of individual heterogeneity is not considered in the current case. A warehouse model containing all possible transmission state paths is constructed. Three new warehouse transfer paths $\mathrm{S}\to \mathrm{I}$, $\mathrm{S}\to \mathrm{R}$, $\mathrm{E}\to \mathrm{R}$ are added to construct a meme transmission model named SIR-EIR model based on the SEIR model (Figure 1b).

Susceptible nodes have extra two transfer paths $\mathrm{S}\to \mathrm{I}$, $\mathrm{S}\to \mathrm{R}$ in the current model compared with the classical SEIR model. Individuals in the E-state can change to I-state based on the infection rate of ${\varepsilon }_{1}$, or directly change to the R-state based on the immunity rate of ${\varepsilon }_{2}$. This implies that the transition of $\mathrm{S}\to \mathrm{E}$ is a progressive transition state, indicating that two selective iterations are given to some individuals of S-state. Moreover, the transfer path of $\mathrm{E}\to \mathrm{R}$ is added, and the E-state individual can be transferred directly to the R-state. The transfer of $\mathrm{S}\to \mathrm{E}$ can be considered if the nodes fail to respond to the information in time due to the time-delay reception and understanding reaction after they are exposed to the transmitted meme in the actual network. The individual may not immediately respond due to personal interest perception, attention, trust, risk perception and other reasons of meme. At the time of t + n, these nodes may be transferred to the I-state propagation state or the R-state immune state due to propagation of neighbor nodes or influence by external factors.

The differential equation of SIR-EIR was constructed based on the theory of system dynamics modeling as shown below;

The total amount of $\mathrm{N}\left(\mathrm{t}\right)$ in the system is equal to $S\left(t\right)+E\left(t\right)+I\left(t\right)+R\left(t\right)$, therefore, it can be concluded that, ${N}^{\text{'}}\left(t\right) = N\left(t\right)\varPhi \left(N\right)-dN\left(t\right)$. Considering a single factor growth rate of $\mathrm{b}\mathrm{N}\left(\mathrm{t}\right)$, the equation below is obtained

3.2.   SIR-EIR Model Solution

The terms $s = S/N, e = E/N, i = I/N, r = R/N,$ are set to represent proportions of node states in the total number of users. The mean field method is used to solve the basic regenerative number and stable solution of the system.

The domain of definition is $\Omega = \left\{\left(s, e, i, r\right)\in {R}_{+}^{4}| 0\le s+e+i+r\le 1\right\}$. The system of rail line will eventually enter or stay in the area in the boundary of Ω and won't leave, therefore, Ω is positive invariant set of the system. To solve the system of equations the following steps are used;

Get $I = \frac{\varPhi \left(N\right)}{\frac{\lambda s}{1-s}-\alpha \varPhi \left(N\right)},$ then $i = I/N = \frac{\varPhi \left(N\right)}{\left[\frac{\lambda s}{1-s}-\alpha \varPhi \left(N\right)\right]N}$ , $e = \frac{{\lambda }_{1}\varPhi \left(N\right)\left(1-s\right)}{\lambda ({\varepsilon }_{1}+{\varepsilon }_{2}+\varPhi \left(N\right))}$,

We can get:

Set $\varPhi \left(N\right)\left(1-s\right)F\left(s\right) = 0$, then $F\left(s\right) = \frac{{\lambda }_{2}}{\lambda }+\frac{{\varepsilon }_{1}{\lambda }_{1}}{\lambda \left[{\varepsilon }_{1}+{\varepsilon }_{2}+\varPhi \left(N\right)\right]}-\frac{\gamma +\varPhi \left(N\right)}{\left[\lambda s-\alpha \varPhi \left(N\right)\left(1-s\right)\right]N}$. The steady state equilibrium point exists within Ω and ${P}_{0}(\mathrm{1, 0}, \mathrm{0, 0})$.

${R}_{0}$ is the basic regeneration number of the system. When ${R}_{0}>$ 1, $\mathrm{F}\left(\mathrm{s}\right)$ is monotone increasing, and $F\left(1\right)>0$, so $F\left(s\right) = 0$ only exist when interval (0, 1) have the only positive root, implying that there exists unique non-zero system steady state equilibrium point. Therefore, the system steady state equilibrium point ${P}^{*}({s}^{*}, {e}^{*}, {i}^{*}, {r}^{*})$ is obtained:

Therein: $\mathrm{N}\left(\mathrm{t}\right) = \frac{K(b-d)}{b+[\frac{K\left(b-d\right)}{{N}_{0}}-b]{e}^{-(b-d)t}}$.

When the basic regenerative number ${R}_{0}\le 1$, the equilibrium point of the system ${P}^{*}({s}^{*}, {e}^{*}, {i}^{*}, {r}^{*})$ is globally stable, and when ${R}_{0}>$ 1, the equilibrium point ${P}^{*}({s}^{*}, {e}^{*}, {i}^{*}, {r}^{*})$ is globally stable inside Ω.

3.2.   SIR-EIR model simulation and parameter influence analysis

The simulation experiment was carried out using Python 3.7 to verify the equilibrium point, stability and propagation of the improved model, and explore the influence of parameters such as contact probability, transfer probability and immunity probability on the evolution of meme propagation. The initial setting of each parameter was as follows, $b = 0.001, K = 1.2, {\lambda }_{1} = 0.4, {\lambda }_{2} = 0.1, {\lambda }_{3} =$ 0.1, ${\varepsilon }_{1} = 0.05, {\varepsilon }_{2} = 0.02,$ $\gamma = 0.1$, $\alpha = 0.002$, ${N}_{0} = 1, {I}_{0} = 0.001, {R}_{0} = 0, {E}_{0} = 0$ .

Evaluation indexes of the degree of meme transmission were defined as follows:

(1) Spreading range $\mathrm{Z}\left(\mathrm{t}\right)$ : $Z\left(t\right) = E\left(t\right)+I\left(t\right)+R\left(t\right) = N-S\left(t\right)$ ; Transmission range refers to the number of all individuals in the system affected by meme transmission at time t, including hesitant individuals, transmission individuals and immune individuals.

(2) Conductivity: $z\left(t\right) = \frac{Z\left(t\right)}{N} = \frac{N-S\left(t\right)}{N} = 1-s\left(t\right)$

(3) Propagation ratio $\mathrm{i}\left(\mathrm{t}\right)$ : $i\left(t\right) = I\left(t\right)/N,$ propagation ratio refers to the proportion of all individuals in the state of transmission at time t.

(4) Transmission ratio: $e\left(t\right)+i\left(t\right)$ represents the proportion of potential and transmitted individuals in the system, and the proportion of individuals affected by memes.

3.3.1.   Difference between SIR-EIR model and SEIR model

The SIR-EIR model has significant difference in the time interval of system propagation peak compared with the traditional SEIR model. The connected path of $\mathrm{S}\to \mathrm{R}$ and $\mathrm{S}\to \mathrm{I}$ are increased in the system, therefore, the propagation speed of SIR-EIR model is faster compared with that of SEIR model, and the peak value of propagation range is smaller compared with that of the SEIR model. SIR-EIR model is more consistent with the actual situation compared with SEIR models. In the process of network information transmission, only a small number of nodes can freely transmit and carry out the transmission (Figure 2).

3.3.2.   Influence of transition probability ${\mathrm{\lambda }}_{1}, {\mathrm{\lambda }}_{2}, {\mathrm{\lambda }}_{3}$

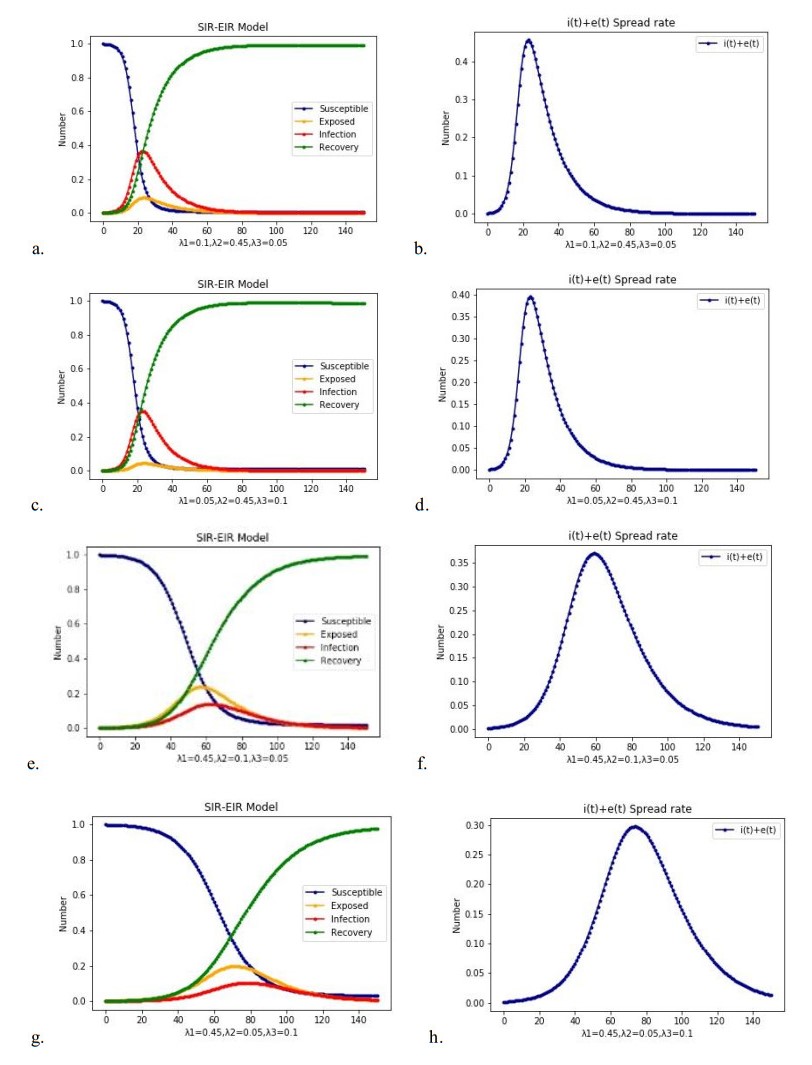
The initial parameters were not changed, and different values were set for state transition probability ${\lambda }_{1}, {\lambda }_{2}, {\lambda }_{3}$, to explore the change of system propagation, as shown in Figure 3.

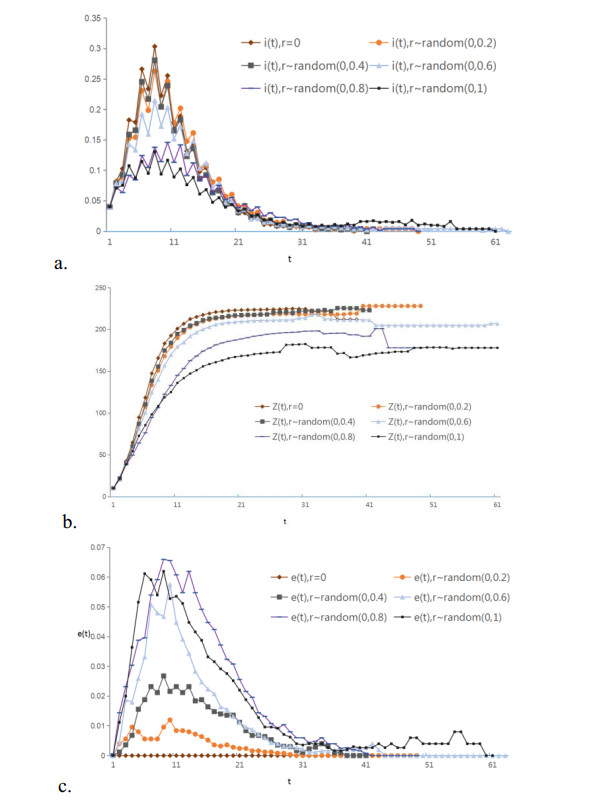
The changes of each state of the system are significantly different (Figure 3a and 3c). The value of ${ \lambda }_{2}$ can significantly affect the equilibrium state and the propagation velocity of the system. When ${\lambda }_{3}$ is the same, the value of $e\left(t\right)+i\left(t\right)$ increases with increase in ${\lambda }_{2}$, and the propagation velocity increases significantly. Analysis showed that ${\lambda }_{2}$ has a greater positive effect on the transmission speed and transmission range of meme information compared with ${\lambda }_{1}$ (Figure 3b and 3d). This phenomenon is observed because after some nodes contact the meme information, they can directly transform into I-state, which is stronger in the following transmission range and infectivity compared with when they only transform into the E-state. Therefore, a strong transmission force of meme is strong enough and increase in sensitivity of nodes to the meme increases the transmission. The transmission range of meme can be significantly improved by directly transforming the individual state from the susceptible state to the transmission state. Insensitivity and uncertain perception of individual meme results in slow propagation speed of a and a small conduction range.

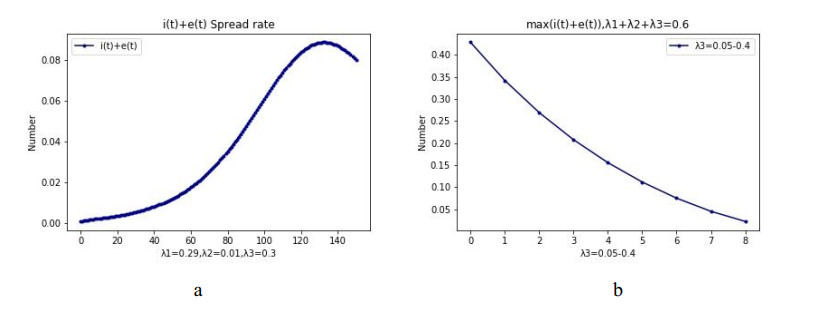
Changes of each state are basically the same, however, the propagation influence range and peak value of $e\left(t\right)+i\left(t\right)$ vary significantly (Figure 4a and 4b), indicating that ${\lambda }_{3}$ is negatively correlated with the propagation range and peak value of the system. The experiment explored the continuous change curve of ${\lambda }_{3}$, and controls were not changed ${\lambda }_{1}+{\lambda }_{2}+{\lambda }_{3} = 0.6$. When ${\lambda }_{3}\ge 0.3$, the propagation range of the whole system $e\left(t\right)+i\left(t\right)$ is less than 15.2%, and the propagation speed slows down rapidly with increase of ${\lambda }_{3}$, implying that ${\lambda }_{3}$ is negatively correlated with the network propagation speed. In addition, this shows that if the sensitivity and topic degree of the meme are not strong, and the public's interest in the meme is not high, the spread range and speed of the meme will be significantly affected.

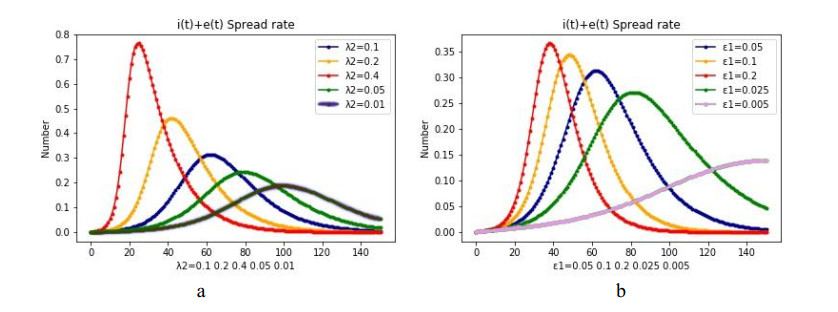
Adjustment of the values of ${\lambda }_{2}$ and ${\varepsilon }_{1}$ showed that the scaling of ${\mathrm{\lambda }}_{2\mathrm{ }}$ value by one-fold had a multiplier effect on the peak value of information transmission range $e\left(t\right)+i\left(t\right)$ (Figure 5). However, scaling of ${\varepsilon }_{1}$ had no significant effect on the peak value of $e\left(t\right)+i\left(t\right)$, indicating that ${\lambda }_{2}$ was more sensitive compared with ${\varepsilon }_{1}$. The transfer probability of ${\lambda }_{2}$ mainly depends on the infectivity of the meme itself, whereas the value of ${\varepsilon }_{1}$ depends on the adjacent network structure. This indicates that the intervention and regulation in the actual transmission process are significantly less compared with the intervention in the initial state of meme transmission.

Analysis showed that the SEIR model is improved by adding $S\to I$, $S\to R$, $E\to R$ three paths, which have a significant effect on the propagation of the whole system. Propagation of meme is dependent on factors such as the quality of the meme itself and its ability to be transmitted, and the individual heterogeneity of nodes, such as individual interest, attention, trust and risk perception. Therefore, this study introduces individual heterogeneity into the SIR-EIR model and builds a meme transmission model based on individual heterogeneity based on the judgment process of individuals' generation of meme communication intention.

4.   Meme propagation model considering individual heterogeneity

In the process of meme transmission, individuals play a role as the transmission subject. Therefore, they complete the screening of the attention of meme due to the heterogeneity of their individual preferences. Even for very popular memes, there are always individuals who have no interest in them. Individual perception of the source credibility of the meme, and individual perception of the risk that may arise from the spread of the meme are key factors that determine the spread of a meme. When individuals are exposed to a meme and interested in the meme, they seek information related to the meme and make complex judgments based on the information acquired and the knowledge accumulated by individuals. If the individual's perception of trust for the meme is greater compared with his perception of the risk of meme transmission, then the individual spreads the meme. In this case, the trust and risk perception changes; otherwise, they tend to handle it prudently, enter the state of hesitation, waiting for other individuals to share meme-related information to supplement their meme-related cognition, or enter the state of immunity. When individuals in a hesitant state are exposed to similar information of meme again, they carry out judgement through the same mechanism. If the trust perception is greater than the risk perception, then the individuals in a hesitant state will change into a transmission state. Individuals in the transmission state will lose interest in the transmitted meme with a certain probability, change into an immune state and stop the transmission of the meme. Individual heterogeneity parameters such as interest perception, risk perception and trust perception based on SIR-EIR model were introduced to construct the IHSEIR meme transmission model which included individual heterogeneity. The model was simulated by the Multi-Agent method based on characterization of individual heterogeneity.

4.1.   IHSEIR model and description

The judgment rule of individual intention behavior was added to the current model based on the warehouse propagation model. The model considers the transition of node state, and the process of node state change (Figure 6).

(1) Heterogeneous attribute of individual i

${T}_{i}$, Trust perception, which represents heterogeneity of the degree of credibility shown by the individual to others; ${T}_{i}$ ∈ [0, 1].

${R}_{i}$, Risk perception, representing the heterogeneity of individual risk judgment and negative benefit perception; ${R}_{i}$ ∈ [0, 1].

${P}_{i}$, Degree of interest, which indicates the degree of interest in meme; ${P}_{i}$ ∈ [0, 1].

(2) Transmission rules (taking S state as an example)

If ${T}_{j}\left(t\right)>{R}_{i}\left(t\right)$, then the individual i changes from S state to I state with probability λ₂, and propagates the meme.

${R}_{i}(t+1) = {R}_{i}\left(t\right)-\sigma {R}_{j}\left(t\right)$; ($\mathrm{\sigma }$ is the propagation perception coefficient)

Elseif ${T}_{j}\left(t\right)\le {R}_{i}\left(t\right)$, then the individual i changes from S state to I state with probability λ₁, and end of this update.

$\omega = randscr(-\mathrm{1, 1})$, implies that after the individual receives the information, the risk perception of the individual may not be reduced, but can be enhanced. Using asynchronous update mode, after traversing the full part of the transmission node, into the next process.

4.2.   IHSEIR meme propagation model simulation and parameter influence analysis

In this study, Netlogo 6.0 software was used for simulation. Initial parameters were set as shown in Table 2. A small-world network with 250 nodes was used and the average degree of network was 6. The model operation results are shown in Figure 7.

4.2.1.   Effect of risk perception heterogeneity ${R}_{i}$ on meme transmission

Parameters presented in Table 2 were used to explore the effect of heterogeneity changes the risk perception on transmission. Initial risk perception heterogeneity was set at 0, random(0, 0.2), random(0, 0.4), random(0, 0.6), random(0, 0.8), random(0, 1), and the number of update iterations was set as 100. 50 independent random experiments were chosen, and the average value was calculated to determine the change of meme transmission ratio and transmission range.

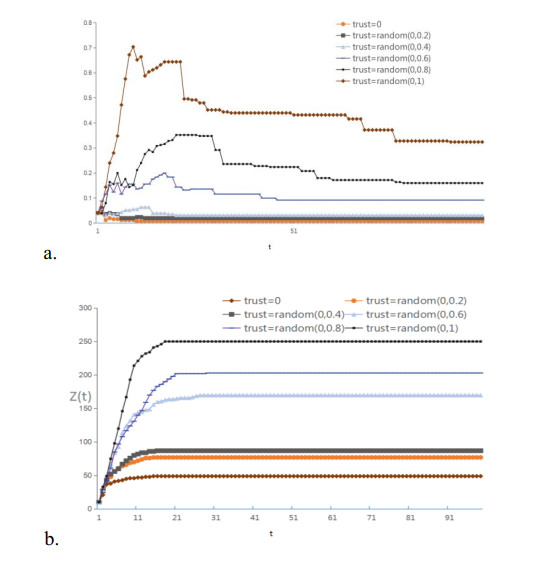
Gradual decrease in the distribution range and mean value of individual risk perception results in increase in the maximum transmission ratio in the process of meme propagation (Figure 8a). The meme transmission ratio and the information conductivity are the highest when the individual risk perception value ${R}_{i}$ = 0. The transmission ratio and the conductivity decrease with increase in the individual risk perception value. Gradual increase in the initial distribution range and mean value of individual risk perception results in decrease in the transmission range and transmission ratio (Figure 8b). Notably, there is a significant difference in the time for the system evolution to reach the asymptotically stable state. Therefore, high overall risk perception of the group in the network is correlated with a large risk perception of the individual for the transmission of meme, which is not conducive to the transmission of meme. A gradual decrease of the distribution range and mean value of individual risk perception results in a gradual increase in the maximum proportion of individuals in the hesitating state during the process of meme transmission. Risk perception significantly affects the number of hesitant individuals. Although a high-risk perception coefficient decreases the number of transmission individuals in the system, it significantly increases the number of hesitant individuals. This indicates that the effect of individual risk perception heterogeneity on reduction of meme transmission ratio is greater compared with the effect on the transition of individual hesitation state. A large risk perception atmosphere significantly affects the spread of meme, and can lead to more hesitant individuals, making the spread process difficult to predict.

4.2.2.   Influence of initial trust heterogeneity ${T}_{i}$ on meme transmission

Similar parameters as described in Table 2 were used to explore the effect of heterogeneity changes in trust perception on transmission. The initial trust perception heterogeneity was set at 0, random(0, 0.2), random(0, 0.4), random(0, 0.6), random(0, 0.8), and random(0, 1), respectively. The number of update iterations was set to 100 steps. A total of 50 independent random experiments were selected, and the average value was calculated to determine the change in meme transmission ratio and transmission range.

The experimental results are shown in Figure 9. Gradual increase in initial trust value distribution range and mean value of individuals resulted in gradual increase in the maximum transmission ratio in the process of meme propagation. This is observed because a small value of individual trust value ${T}_{i}$ results in decreased in the external credibility of an individual, which is not enough to counter the risk perception of other individuals. Therefore, the meme cannot be transmitted. Transmission ratio and conductivity increase with increase in individual trust value. Gradual increase in the initial trust value distribution range and mean value of individuals results in gradual increase in the transmission range and transmission ratio after the system (Figure 9b). However, a significant difference in the time is observed when the system reaches the asymptotically stable state. When both individual trust and risk perception follow $random\left(\mathrm{0, 1}\right)$ distribution, the transmission rate of information increases close to 100%, and the transmission can reach most of the nodes. Individual trust value decreases information transmission shows torsion irregularity when $T < random\left(\mathrm{0, 0.4}\right)$ . In addition, most nodes change to the non-inductive state, which is insufficient to change the other nodes. Therefore, trust degree in the system has a significant effect on the transmission of meme. The propagation ratio and conductivity of meme conduction increase with increase in level of the overall environmental trust.

4.2.3.   Effect of interest preference threshold β on meme transmission

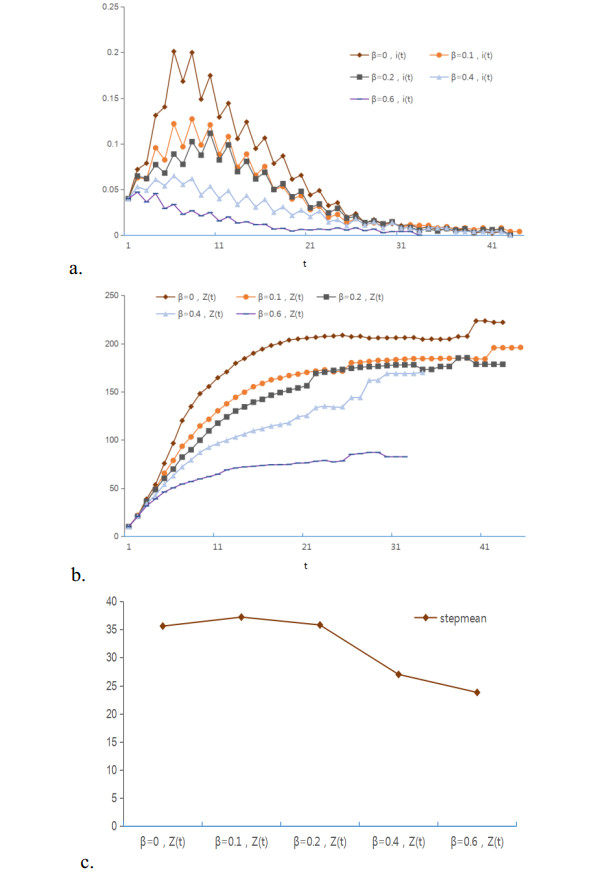
Effect of individual interest and preference heterogeneity on meme transmission was explored using similar parameters presented in Table 2. Distribution of individual interest preference was $P = random\left(\mathrm{0, 1}\right)$. The threshold of individual interest was set at β = 0, 0.1, 0.2, 0.4, 0.6. The number of update iterations was set to 100 steps. A total of 50 independent random experiments were selected, and the average value was calculated to explore the change in meme transmission ratio and transmission range.

Increase in individual interest degree threshold β resulted in gradual decrease in maximum transmission in the process of memes spread $i\left(t\right)max$ (Figure 10a). Meme diffusion is affected by whether the information topic degree fits the audience's individual interest degree perception. Individuals will not change into immune state when the threshold of individual interest β = 0 due to lack of interest in meme, however, they change into hesitating state and transmitting state. This is equivalent to increasing the number of transformation individuals in the propagation state, so that more individuals have the opportunity to turn into the transformation. A high threshold of individual interest degree is correlated with a low individual interest perception of meme, therefore, it is difficult to meet the individual's interest perception, leading to direct transformation of most unknown individuals into immune states. This is equivalent to reducing the number of transformation nodes, thus the number of transmission individuals in the system decreases significantly.

Increase in individual interest threshold β, significantly decreases the propagation range Z(t) when the system is stable (Figure 10b). This is observed because increase in individual interest threshold decreases individuals' interest perception of meme, leading to loss of interest of most unknown individuals, blocking the diffusion path of information. As a result, it increases the number of individuals who are do not receive the meme. The slope of the range, $Z\text{'}\left(t\right) = \underset{∆t\to 0}{\mathit{lim}}∆Z\left(t\right)/∆t$, shows the speed of meme diffusion. Increase in the threshold β of individual interest degree results in gradual decrease in diffusion speed of meme in the system (Figure 10b). This finding indicates that the process of meme diffusion becomes slow, and is proved from the side that the patency of the diffusion path of the meme is low. Meme diffusion time of the system is significantly decreased when the threshold of individual interest $\beta >0.2$ (Figure 10c). This is because after being exposed to the meme, a large number of individuals directly transform into the immune state due to their low interest perception, blocking the transmission path of the meme. As a result, most unknown individuals are unable to receive the information, thus reducing the diffusion time of the meme.

5.   Conclusions

This study constructed a fully connected chamber model of meme transmission by adding three transfer states $\mathrm{S}\to \mathrm{I}$, $\mathrm{S}\to \mathrm{R}$, $\mathrm{E}\to \mathrm{R}$ to the SEIR model, based on analysis of individual state transfer in the process of meme transmission, and named it the SIR-EIR model. In addition, the study solved the basic reproduction number R₀ and stable solution of the new model. Three heterogeneous factors including individual interest preference, perception of trust and perception of risk, were added to construct a HISEIR meme propagation model based on the SIR-EIR model. Simulation was carried out using the Multi-Agent method.

The following conclusions and some management details were obtained from the study:

1.Low individual's interest perception of meme results in most individuals with susceptible states directly transforming into immune states. This significantly reduces the transmission base and affects the transmission effect. Therefore, effectiveness of meme diffusion can be significantly improved by improving the topic of the meme to fit the interests and preferences of the audience.

2.Effect of regulation and intervention in the actual transmission process is less significant compared with the intervention at the initial stage of meme transmission. In the process of meme transmission, an early intervention is correlated with a high effect on the whole communication process.

3.Effect of individual risk perception heterogeneity on the reduction of meme transmission ratio is greater compared with the effect on the transition of individual hesitation state. A larger risk perception atmosphere results in more hesitant individuals, making the propagation process of meme difficult to predict and significantly limits the propagation of the meme. A low overall environmental trust level is not conducive for transmission of a meme. A higher overall environmental trust can significantly affect the transmission effect of meme.

Conflict of interest

The authors declare there is no conflict of interest.