Mathematical analysis of a simplified general type-2 fuzzy PID controller

1.   Introduction

In real control problems, there exited many uncertainties, like model structure, measurement, external disturbance and so on, tradition PID and type-1 fuzzy controller can’t deal with these uncertainties ^[1,2,3,4,5]. Type-2 fuzzy controller can handle uncertainties more robust than PID and type-1 fuzzy controller for it was described by type-2 fuzzy sets proposed by Zadeh in 1975 ^[6]. Type-2 fuzzy sets mainly included interval type-2 fuzzy sets whose secondary membership degree was 1 and general type-2 fuzzy sets whose secondary membership degree was decided by a function, such as triangular, Gaussian, trapezoid. As the secondary membership degree of interval type-2 fuzzy sets was 1, so it was easily to be implemented and Karnik-Mendel (KM) algorithm was the most widely applied type reduction for interval type-2 fuzzy sets ^[7]. Interval type-2 fuzzy logic systems has been applied in many applications, like face recognition ^[8], prediction problems ^[9,10,11], pattern recognition ^[12], clustering ^[13], intelligent control ^[14], industrial ^[15], neuro-fuzzy systems ^[16], interval type-2 fuzzy PID controller ^[17,18], sculpting the state space ^[19], peer-to-peer e-commerce ^[20], classification ^[21,22], regression ^[23], diagnosis problems ^[24], metaheuristics ^[25], gravitational search algorithm ^[26], healthcare problem ^[27], unmanned aerial vehicles ^[28], deep neural network ^[29], pursuit evasion game ^[30], analytical structure of interval type-2 fuzzy controller ^[31,32,33] and so on.

As the secondary membership degree of general type-2 fuzzy sets was determined by a function rather than 1, so general type-2 fuzzy sets contained more uncertain information than interval type-2 fuzzy sets. And general type-2 fuzzy logic systems had more design parameters when describing reality. Thus, general type-2 fuzzy systems can obtain a better performance in some control systems with high uncertainties. Now there existed some efficient type reduction algorithms for general type-2 fuzzy sets, for example, α-plane representation method ^[34,35,36], zSlices-based representation method ^[37,38], sample method ^[39], geometric method ^[40,41], hierarchical collapsing method ^[42] and so on. In these algorithms, α-plane representation method was widely applied in general type-2 fuzzy sets type reduction. By α-plane representation, general type-2 fuzzy sets will be assembled by some interval type-2 fuzzy sets (α-planes). Some exiting interval type-2 fuzzy sets type reduction algorithms can be applied to these α-planes, like KM, EKM ^[46], IASC ^[47] or EIASC ^[48]. General type-2 fuzzy logic systems have been applied in many situations, like: mobile robots ^{[38,46,47,48,49,50,51]}, water tank ^[52], traffic signal scheduling ^[53], inverted pendulum plant ^[54], 5-agents system ^[55], nonlinear power systems ^[56], water level and DC motor speed ^[57], aerospace ^[58], airplane flight ^[59], steam temperature ^[60], power-line inspection robots ^[61,62], fractional order general type-2 fuzzy controller ^[63,64], medical diagnosis ^[65,66,67], fuzzy classifier and clustering ^[68,69], sculpting the state space ^[70], similarity measures ^[71], forecasting ^[72], brain-machine interface ^[73] and so on. ^[74,75,76] made a detailed introduction on type 2 fuzzy logic applications.

The type reduction of general type-2 fuzzy sets was converted to type reduction of several interval type-2 fuzzy sets. And KM type reduction algorithm was applied to these interval type-2 fuzzy sets in most applications. KM algorithm was an iterative process without analytic solution. The number of α-planes and iterative process of KM algorithm decided the execution time of general type-2 fuzzy sets type reduction. Thus the real time of general type-2 fuzzy controller was weaker than type-1 and interval type-2 fuzzy controller. In according with these problems, a simplified general type-2 fuzzy PID (SGT2F-PID) controller is studied. The SGT2F-PID controller applies triangular function as the primary and secondary membership function. The inputs of SGT2F-PID controller are error and error derivative, and each input defines 2 fuzzy membership functions in fuzzy domains, thus only 4 rules will be derived in this SGT2F-PID controller. This paper mainly contains the following 3 contributions:

Ⅰ). The primary membership degree of apex for secondary membership degree is applied to get the centroid of SGT2F-PID controller. Then the real time of SGT2F-PID controller is almost the same as conventional type-1 fuzzy PID (T1F-PID) controller and better than interval and general type-2 fuzzy PID controller.

Ⅱ). The primary membership degree of apex for secondary membership degree is decided by the up and low bounds of footprint of uncurtains, which inherits the benefits of type-2 fuzzy PID controller. So the SGT2F-PID controller contains more design freedom and handles uncertainties better than PID or type-1 fuzzy PID controller.

Ⅲ). The accurate mathematical expression of SGT2F-PID controller is obtained and compared with mathematical expressions of interval type-2 fuzzy PID controller (IT2F-PID) and conventional T1F-PID controller. The mathematical expressions indicate that these 3 fuzzy PID controllers are all PID type controller. Furthermore, we obtain the relationship of controller gains and explain why SGT2F-PID controller can get better controlling effects.

2.   Basic Concepts

2.1.   Fuzzy sets

A type-1 fuzzy set in the universe X is characterized by a membership function μ_A(x) as Eq (1).

where ${\mu _\boldsymbol{A}}:\boldsymbol{X} \to [0, 1]$, and μ_A(x) represents the membership degree of the element x∈X to the set A.

A general type-2 fuzzy sets $\boldsymbol{\tilde A}$ defined in universal sets X can be described as Eq (2) ^[77].

u is the primary membership degree and ${\mu _{\boldsymbol{\tilde A}}}(x, u)$ is the secondary membership degree related to input variable x and primary membership degree u.

If the secondary membership degrees ${\mu _{\boldsymbol{\tilde A}}}(x, u)$ are set to 1, then interval type-2 fuzzy sets can be shown as Eq (3).

Figure 1 shows the definition of type-1 fuzzy sets, interval type-2 fuzzy sets and general type-2 fuzzy sets whose secondary membership function is triangular.

2.2.   α-planes representation for general type-2 fuzzy sets

Liu introduced an α-plane representation for general type-2 fuzz sets ^[34], and pointed that α-plane denoted as ${\boldsymbol{\tilde A}_\alpha }$ can be defined as Eq (4).

If assemble all α-planes ${\boldsymbol{\tilde A}_\alpha }$, then general type-2 fuzzy sets $\boldsymbol{\tilde A}$ can be described as Eq (5).

The centroid of general type-2 fuzzy sets can be calculated by the centroids of its all α-planes ${\boldsymbol{\tilde A}_\alpha }$.

${l_{{{\boldsymbol{\tilde A}}_\alpha }}}$ and ${r_{{{\boldsymbol{\tilde A}}_\alpha }}}$ are the left and right end points of interval type-2 fuzzy sets ${\boldsymbol{\tilde A}_\alpha }$ whose secondary membership degree is α.

3.   General type-2 fuzzy logic systems

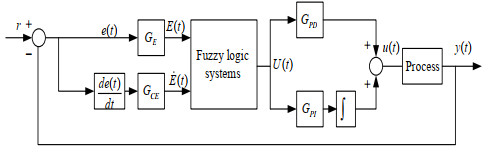
The general structure of fuzzy PID controller can be depicted as Figure 2 ^[76]. The antecedent parts can be type-1, interval type-2 or general type-2 fuzzy sets and the consequent parameters are crisp values.

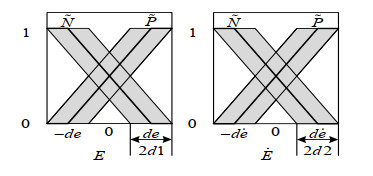
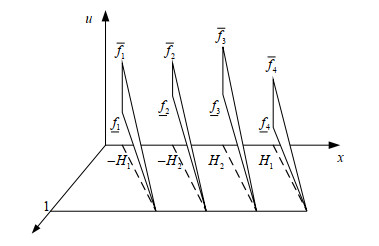
In this paper, triangular primary function is applied. The inputs of general type-2 fuzzy PID controller are normalize error (E) defined in [-de-d1, de+d1] and error derivative ($\dot E$) defined in $[ - d\dot e - d2, d\dot e + d2]$, which is shown as Figure 3. d1 and d2 decide the footprint of uncertain for primary membership degree, for simplify, $de = d\dot e$ and d1 = d2.

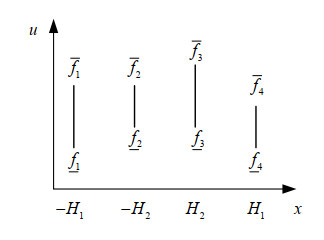
The consequent parameters are symmetric and from Figure 3, 4 rules will be generated as follows, here H₁ > H₂ > -H₂ > -H₁.

Rule 1: If $\dot E$ is $\tilde N$ and E is $\tilde N$, then ${y_1} = - {H_1}$

Rule 2: If $\dot E$ is $\tilde P$ and E is $\tilde N$, then ${y_2} = - {H_2}$

Rule 3: If $\dot E$ is $\tilde N$ and E is $\tilde P$, then ${y_3} = {H_2}$

Rule 4: If $\dot E$ is $\tilde P$ and E is $\tilde P$, then ${y_4} = {H_1}$

Around the steady state, that is in interval [-de+d1, de-d1] for error and $[ - d\dot e{\rm{ + }}d{\rm{2}}, d\dot e - d2]$ for error derivative, the upper and lower bounds of the primary membership degree of error and error derivative can be calculated by Eqs (8–11).

By fuzzy inference of interval type-2 fuzzy logic systems and product operation, the fired membership degrees of fuzzy rules can be described as Eq (12).

4.   Simplified general type-2 fuzzy PID controller

4.1.   Type-1 fuzzy PID controller

The triangular membership function of type-1 fuzzy PID controller is depicted as Figure 4, also for simplify, $de = d\dot e$.

In interval [-de, de] for error and $[ - d\dot e, d\dot e]$ for error derivative, the membership degree of error and error derivative can be calculated as Eqs (13–16).

So the fired membership degrees of fuzzy rules for type-1 fuzzy PID controller can be described as Eq (17).

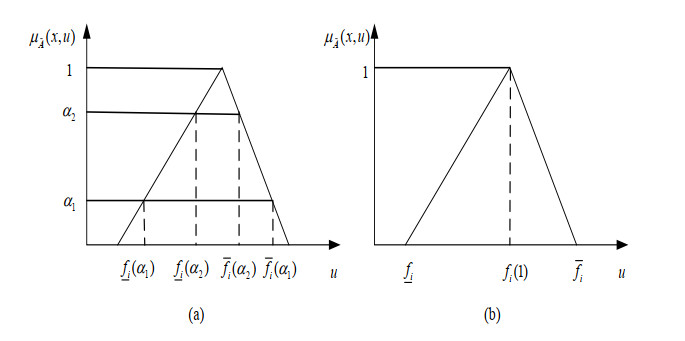
Figure 5 shows an example of membership degrees for fuzzy rules corresponding to consequent parameters using TIF-PID.

From Figure 5 and defuzzification process of type-1 fuzzy sets, the output of type-1 fuzzy inference U(t) in Figure 2 can be calculated as Eq (18).

where, f_i is described as Eq (17) and y_i = [-H₁, -H₂, H₂, H₁]. By the mathematical expression of Eqs (13–17) and y_i, the final solution of U_T1 can be expressed as Eq (19).

According to Eq (19) and Figure 2, the final output of T1F-PID controller can be expressed as Eq (20).

Combine Eq (19) and Eq (20), the output of T1F-PID controller is a PID type controller as Eq (21).

where:

Figure 6 shows the shape of control surface of type-1 fuzzy controller, here H₁ = 1 and H₂ = 0.

4.2.   Interval type-2 fuzzy PID controller

For KM algorithm didn’t have analytic solution, so NT type reduction ^[78,79] algorithm will be applied to get the mathematical expression of IT2F-PID controller. Figure 7 shows an example of upper and lower bounds for fuzzy rules corresponding to consequent parameters using IT2F-PID controller.

From Figure 7, by defuzzification process and NT algorithm, the output of interval type-2 fuzzy inference U(t) in Figure 2 can be calculated as Eq (22).

where, $[{\bar f_i}, {\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{f} _i}]$ is described as Eq (12) and y_i = [-H₁, -H₂, H₂, H₁]. By the mathematical expression of Eqs (8–12) and y_i, the final solution of U can be expressed as Eq (23).

According to Eq (23) and Figure 2, the final output of IT2F-PID controller can be expressed as Equation (24).

Combine Eq (23) and Eq (24), the output of IT2F-PID controller can be calculated as Eq (25).

where:

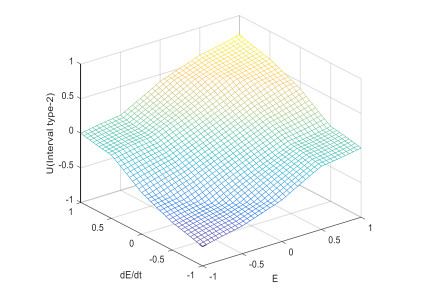
Figure 8 shows the shape of control surface of interval type-2 fuzzy controller, here H₁ = 1 and H₂ = 0.

4.3.   Simplified general type-2 fuzzy PID controller

For type reduction of general type-2 fuzzy sets was converted to type reduction of several interval type-2 fuzzy sets, so the number of α-planes will affect the real time of GT2F-PID controller.

Figure 9 shows an example of membership degrees for fuzzy rules corresponding to consequent parameters using GT2F-PID controller.

The differences of GT2F-PID and SGT2F-PID controller can be seen from Figure 10.

From Figure 10, GT2F-PID controller firstly fixes the number of α-planes, that is D. Then derives D intervals $[{\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{f} _i}({\alpha _j}), {\bar f_i}({\alpha _j})]$ (j = 1, 2, …, D) whose secondary membership degree is α_j. KM or other interval type-2 type reduction algorithm will be applied for each interval type-2 fuzzy sets. And the last, assembles all the centroids of α-plane and gets the output of GT2F-PID controller.

In this paper, the SGT2F-PID controller adapts the primary membership degree of α-plane (α = 1) as the membership degree of fuzzy rules, which is calculated as Eq (26).

here, w is an adjustable parameter.

The output of simplified general type-2 fuzzy inference U(t) in Figure 2 can be calculated as Equation (27).

where, $[{\bar f_i}, {\underset{\raise0.3em\hbox{$\smash{\scriptscriptstyle-}$}}{f} _i}]$ is described as Eq (12) and y_i = [-H₁, -H₂, H₂, H₁]. By the mathematical expression of Eqs (8–12) and y_i, the final solution of U can be expressed as Eq (28).

According to Eq (28) and Figure 2, the final output of SGT2F-PID controller can be expressed as Eq (29).

Combine Eq (28) and Eq (29), the output of SGT2F-PID controller can be calculated as Eq (30).

where:

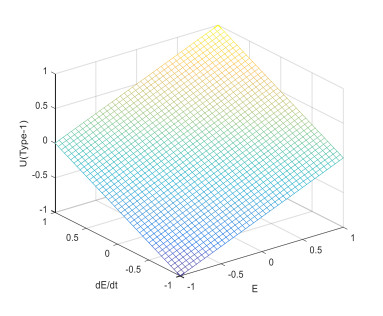
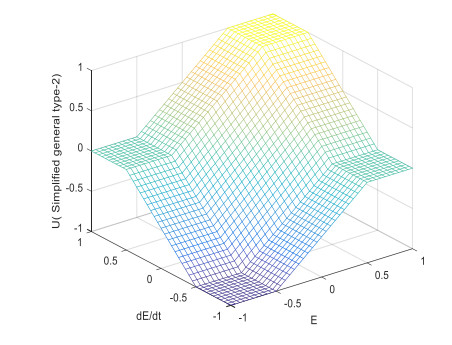
Figure 11 shows the shape of control surface of simplified general type-2 fuzzy controller, here H₁ = 1, H₂ = 0 and w = 0.

From the control surface curve of T1-FPID, IT2-FPID and SGT2-FPID controller, when the system error is near the endpoint, the output of SGT2-FPID controller is larger than T1-FPID and IT2-FPID, so the SGT2-FPID controller has the faster rising time. When the error is near zero, the output of SGT2-FPID controller is smoother than T1-FPID and IT2-FPID, so the SGT2-FPID controller has faster steady time and smaller overshoot.

4.4.   Controller analysis

In summary, the unified T1-FPID, IT2F-PID and SGT2F-PID controller mathematical expressions can be indicated as Eq (31).

where:

If calculate the derivative of K_SGT2 to w, then the partial derivative is Eq (33).

From Eq (33), K_SGT2 is a decreasing function of w and in general, w is in range [0, 1]. So the ranges of K_SGT2 is denoted as Eq (34).

For de > d1, so, $K_{SGT2}^{\max } > {K_{T1}} > {K_{IT2}}$ and $K_{SGT2}^{\min } < {K_{IT2}} < {K_{T1}}$, Figure 12 shows the curve of K_SGT2 as w is rising from 0 to 1.

when w = w₁, K_SGT2 = K_T1 and w = w₂, K_SGT2 = K_IT2, then w₁ = (de-d1)/(2de) and w₂ = 0.5.

According to the characteristics of PID controller, the advantage of proportional action is timely. If increase proportional gain, then the system response speed will be enhanced (that is reducing the rising time and steady time) but the system overshoot will be increased. The integral action can eliminate static error, if increase integral gain, the system overshoot will be decreased. The differential action also has the advantage of timely, which is belonging to ‘future control’. If increase differential gain, the steady time and system overshoot will be reduced.

From above analysis, if a control system maintains both faster response speed and smaller overshoot, the PID controller should chose larger proportional gain, integral gain and differential gain. Figure 12 shows that if w < w₁, then the proportional gain, integral gain and differential gain of SGTF-PID are larger than T1F-PID and IT2F-PID.Thus the controlling efforts of SGTF-PID will be better than T1F-PID and IT2F-PID, which is proved by section 5 of four simulation examples.

5.   Simulations

In simulations, 3 plants and a practical inverted pendulum system are tested to demonstrate the robustness and efficiency of SGT2F-PID. The controlling efforts of SGTF-PID are also compared with PID, T1F-PID, and IT2F-PID controller using NT type reduction algorithm.

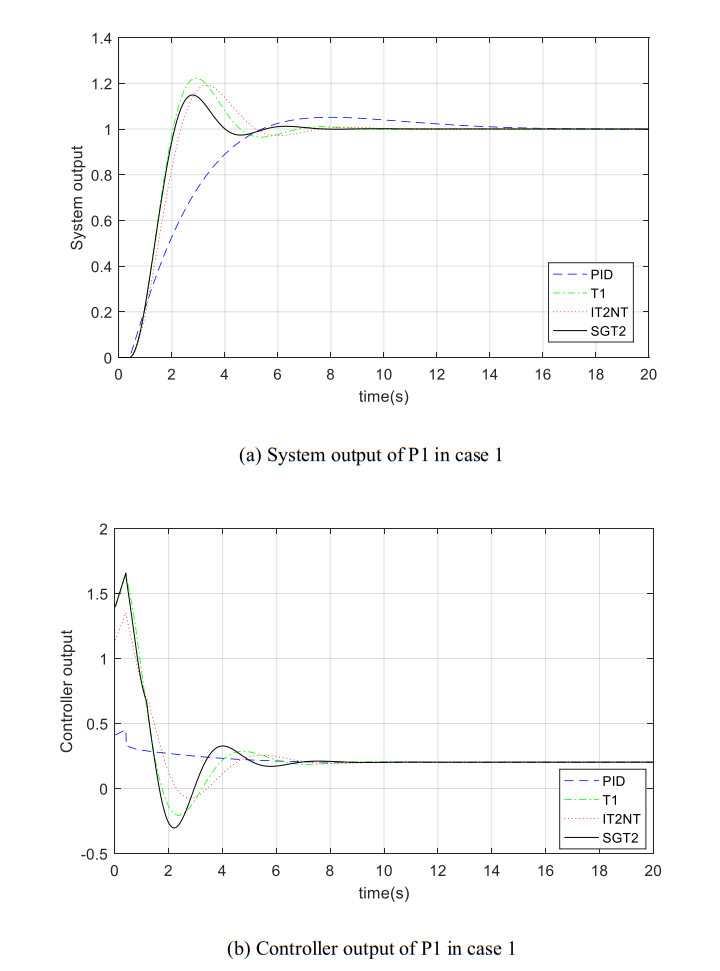
5.1.   Second order stable linear plant (P1)

The tuning PID controller parameters are K_P = 0.4088, K_I = 0.1084, K_D = 0.3547 under case 1 plant parameters. Fuzzy PID controller parameters are G_E = 0.7757, G_CE = 0.7442, G_PD = 3.5336, G_PI = 0.6996, $de = d\dot e = 1$, d1 = d2 = 0.5, H₁ = 1, H₂ = 0, w = 0.1.

Case 1: ζ = 1.125, ω_n = 0.45, L = 0.4.

Case 2: ζ = 1.6875, ω_n = 0.225, L = 0.4.

Case 3: ζ = 0.5624, ω_n = 0.675, L = 0.4.

Case 4: ζ = 1.6875, ω_n = 0.675, L = 0.6.

Table 1 summarizes some controlling performance comparisons of SGT2F-PID controller with other 3 controllers. In Table 1, t_s is steady state time, t_ris rising time, OS is system overshoot and three error integral criterions ISE, ITSE, ITAE.

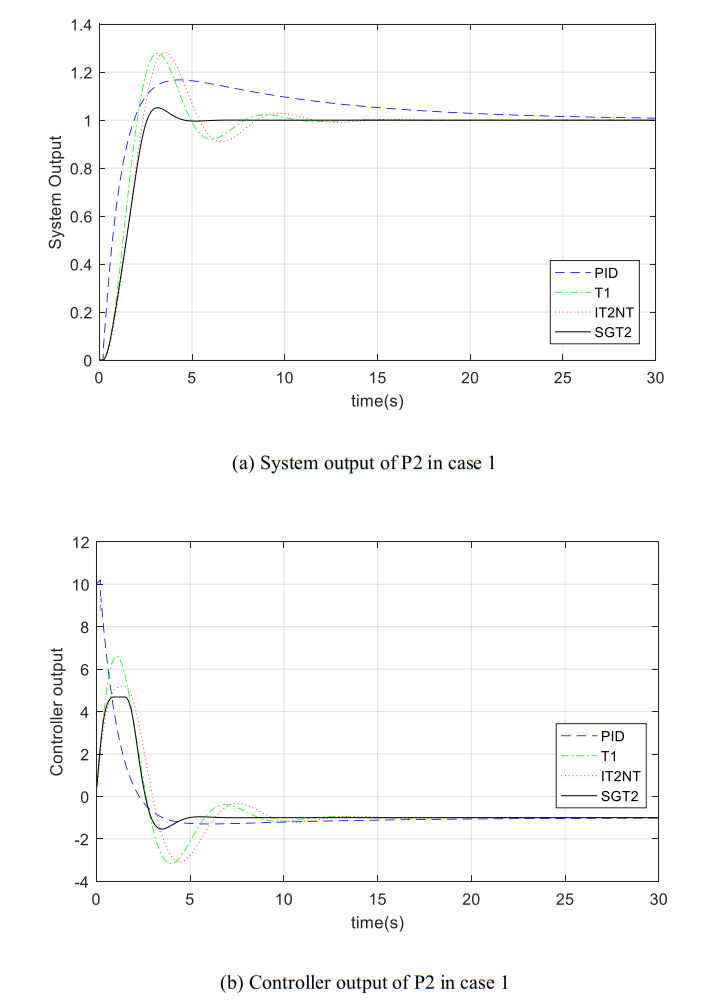
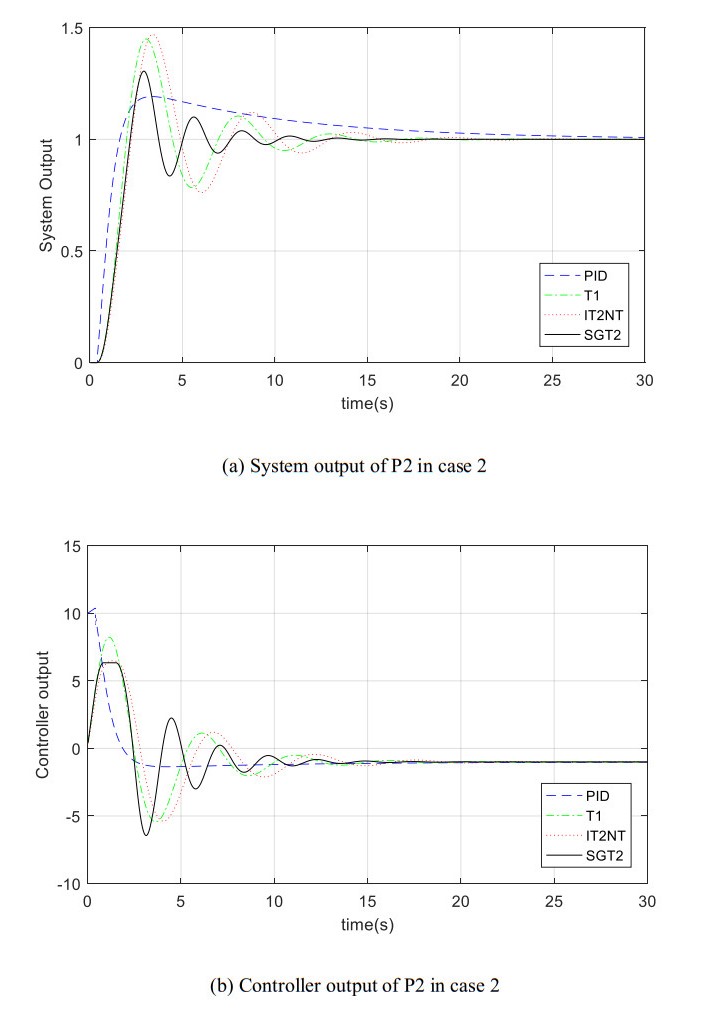
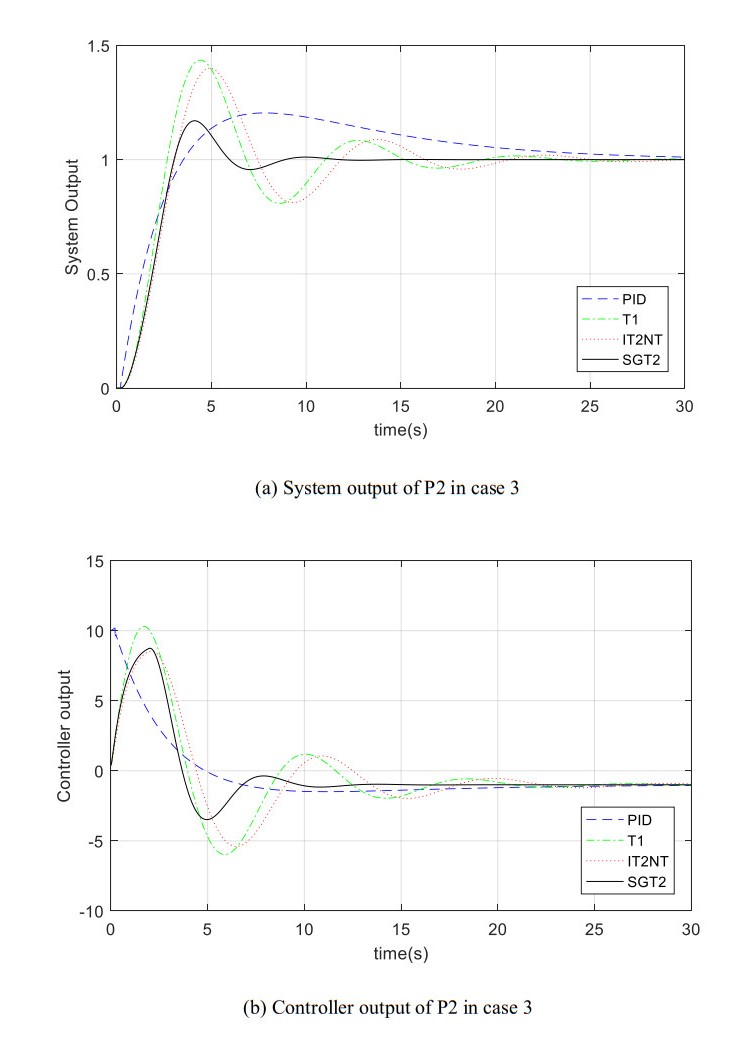
5.2.   First order linear unstable plant (P2)

The tuning PID controller parameters are K_P = 9.999, K_I = 0.9483, K_D = 0.2785 under case 1 plant parameters. Fuzzy PID controller parameters are G_E = 1.9956, G_CE = 0.9387, G_PD = 0.2532, G_PI = 20.0573, $de = d\dot e = 1$, d1 = d2 = 0.5, H₁ = 1, H₂ = 0, w = 0.

Case 1: K = 1, T = 10, L = 0.2.

Case 2: K = 1, T = 10, L = 0.4.

Case 3: K = 1, T = 20, L = 0.2.

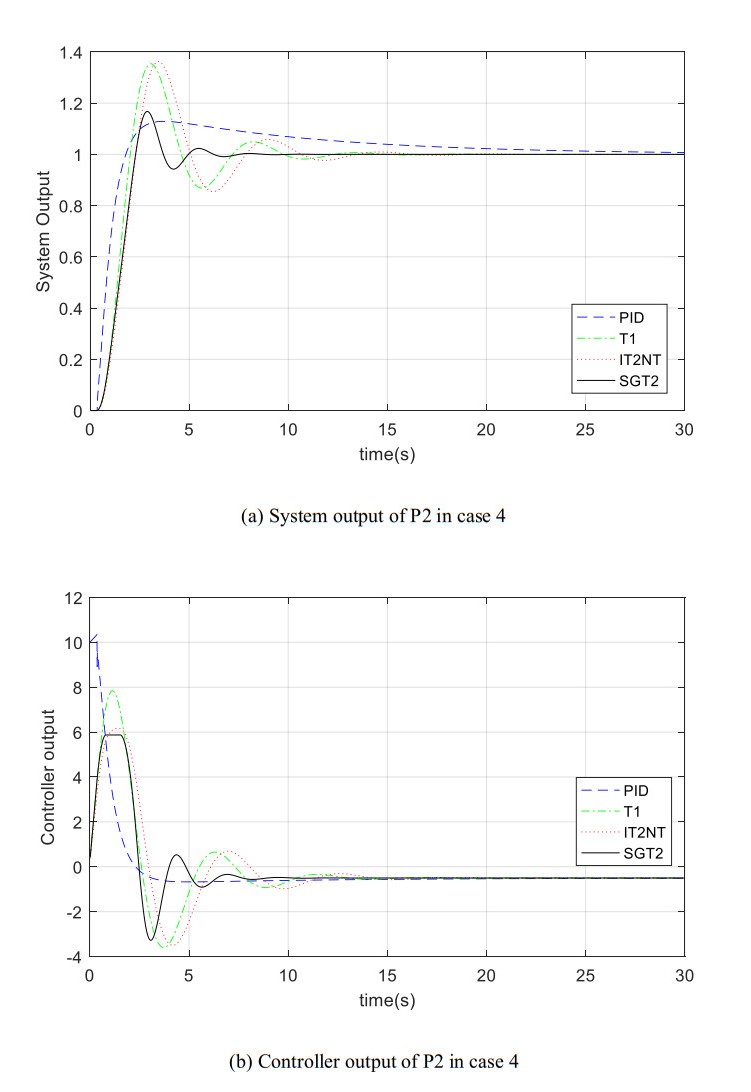
Case 4: K = 2, T = 20, L = 0.35.

Table 2. shows the P2 controlling performance comparisons of SGT2F-PID controller with other 3 controllers.

5.3.   Second order nonlinear plant (P3)

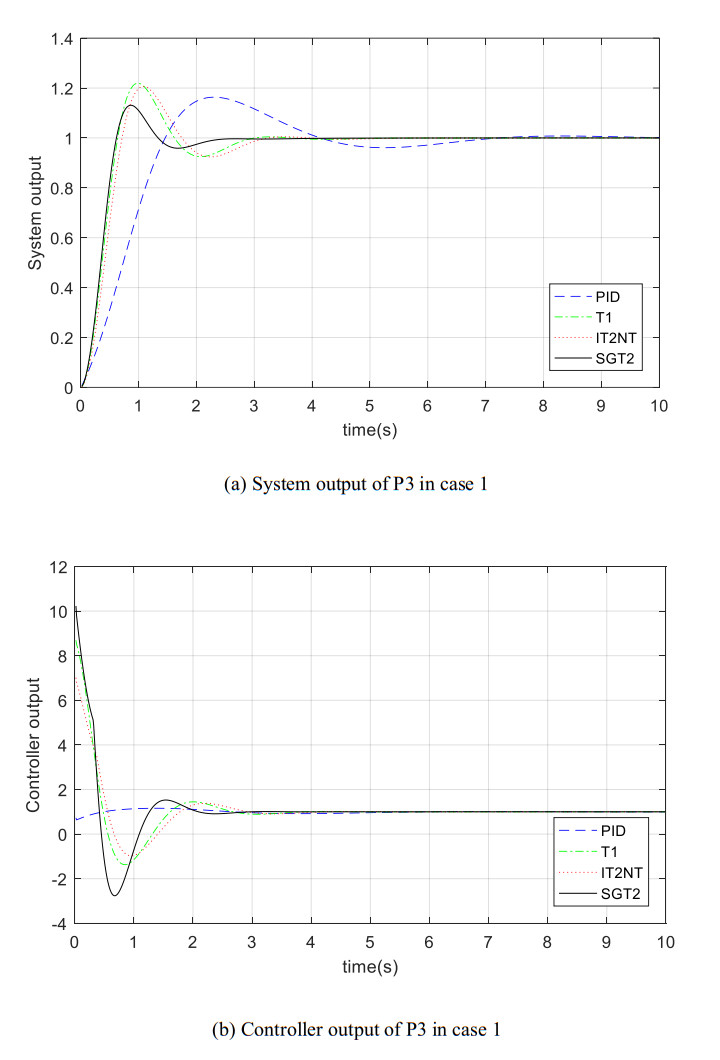
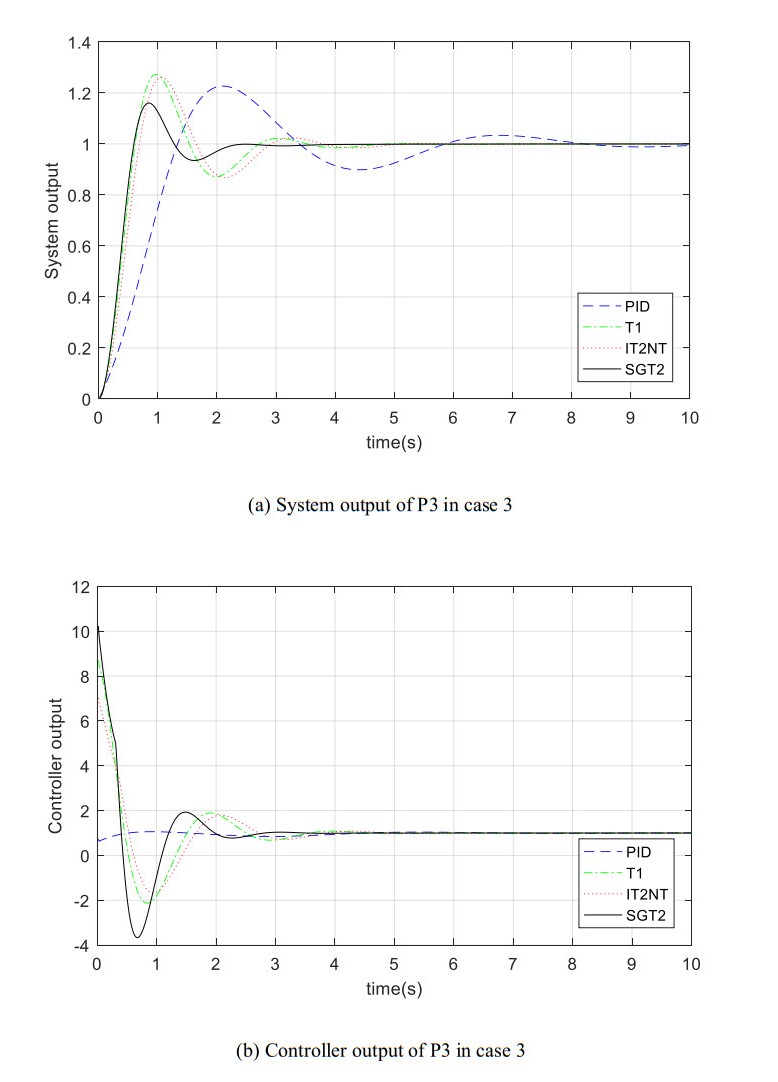
PID controller parameters are K_P = 0.8028, K_I = 1.8548, K_D = 0.4609 selected from article ^[1] optimized by hybridized ABC-GA algorithm. Fuzzy PID controller parameters are G_E = 0.8359, G_CE = 0.1944, G_PD = 20.5501, G_PI = 20.2681, $de = d\dot e = 1$, d1 = d2 = 0.5, H₁ = 1, H₂ = 0, w = 0.

Case 1: ε = 1, σ = 1, L = 0.

Case 2: ε = 1, σ = 1, L = 0.1.

Case 3: ε = 1, σ = 0.7, L = 0.

Case 4: ε = 1.3, σ = 1, L = 0.

Table 3 shows the P3 controlling performance comparisons of SGT2F-PID controller with other 3 controllers.

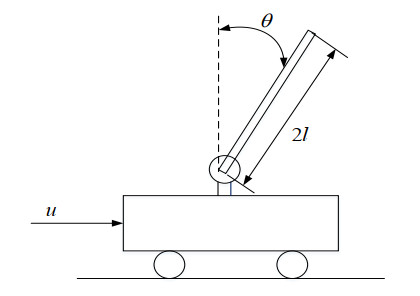
5.4.   Nonlinear inverted pendulum system (P4)

The inverted pendulum system was often applied to demonstrate the reliability of a new controller, as shown in Figure 25.

The inverted pendulum system is consisted of a cart and a pendulum, the controlling aim is to keep pendulum angle at a certain value under external force. Equation (38) describes the state equations of the inverted pendulum system ^[80].

In (38), x₁is the pendulum angle θ and x₂ is the pendulum angular velocity $\dot \theta$. u is the external force horizontally acted on the cart and the unit of u is Newton. m_cis the cart mass, m_p is the pendulum mass and 2l is the pendulum length. The values of these parameters are m_c = 0.5kg, m_c = 0.2kg, l = 0.5m, g = 9.8m/s². Δm_pand ΔA are respectively the uncertainty of pendulum mass and inverted pendulum structure.

PID controller parameters are K_P = 40, K_I = 100, K_D = 8. Fuzzy PID controller parameters are G_E = 0.1009, G_CE = 0.1944, G_PD = 30.5501, G_PI = 30.2681, $de = d\dot e = 0.2$, d1 = d2 = 0.1, H₁ = 10, H₂ = 5, w = 0.04.

Case 1: Normal case.

The initial conditions x₁ = 0.1rad and x₂ = 0rad/s, the setting value is x₁ = 0rad. In normal case, Δm_p = 0 and $\Delta A = \left[ {\begin{array}{*{20}{c}} 0&0 \\ 0&0 \end{array}} \right]$.

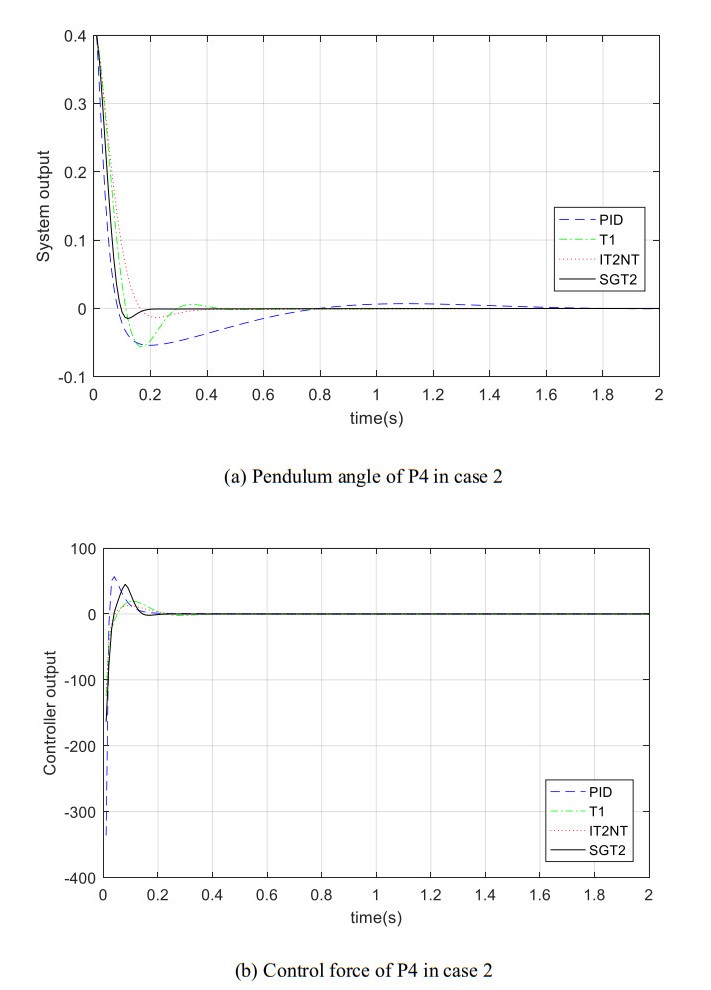
Case 2: Normal case.

The initial conditions x₁ = 0.4rad and x₂ = 0rad/s, the setting value is x₁ = 0rad. In normal case, Δm_p = 0 and $\Delta A = \left[ {\begin{array}{*{20}{c}} 0&0 \\ 0&0 \end{array}} \right]$.

From case 3 to case 6, we will indicate the controlling effects of SGT2-FPID controller when the system adding uncertainties.

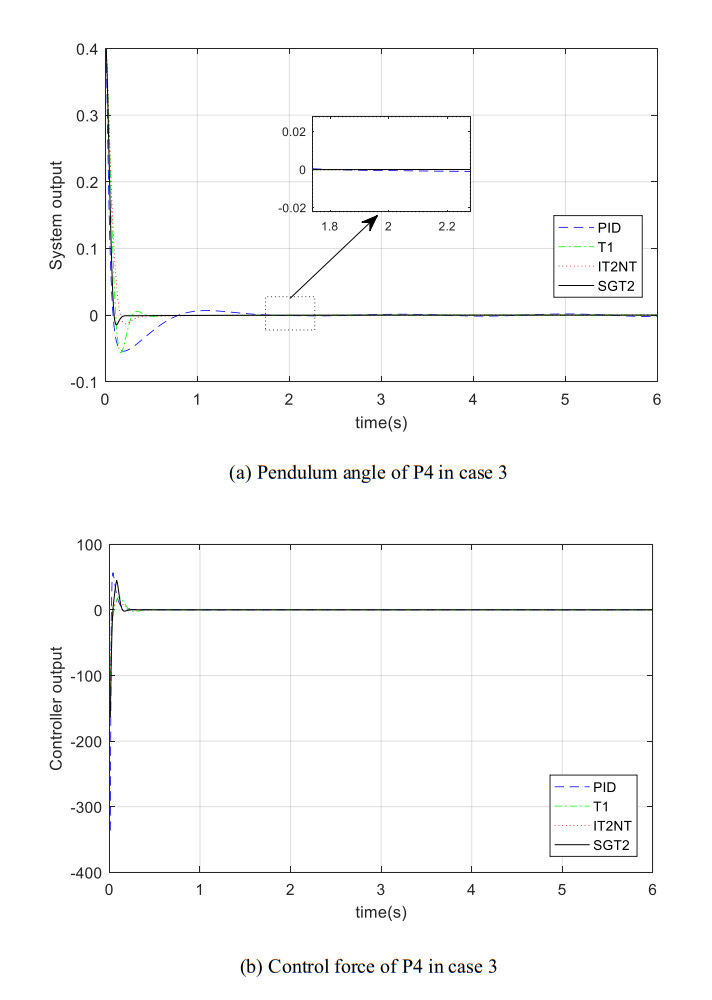
Case 3: Pendulum mass uncertainty.

Here, we will add pendulum mass uncertainty (Δm_p = 2.7kg) at 2s.

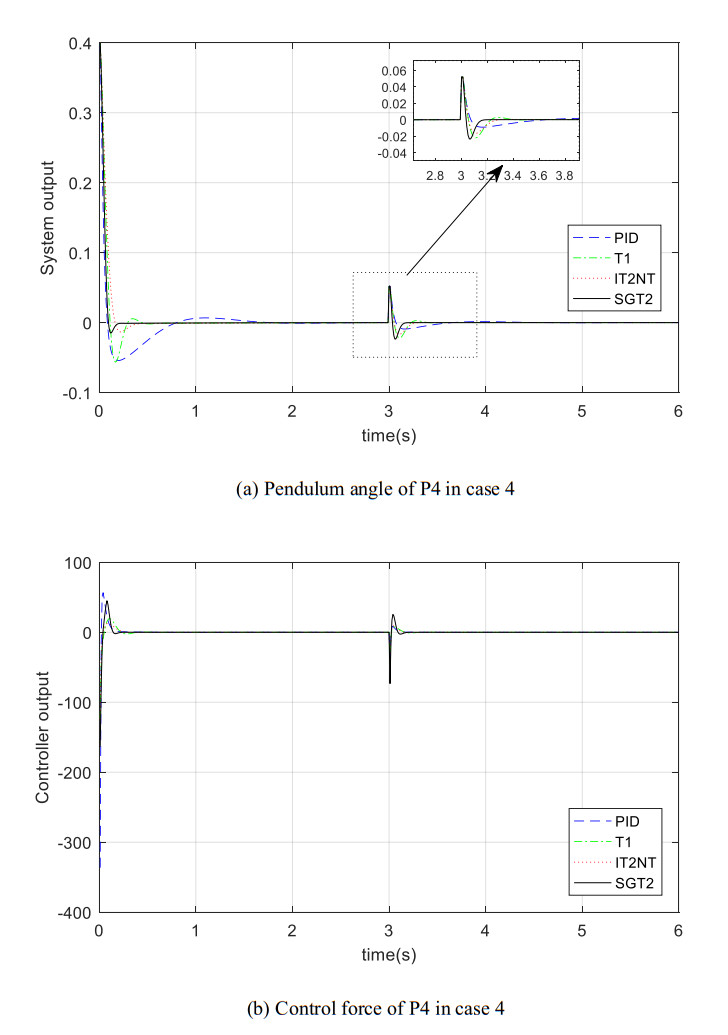
Case 4: Measurement uncertainty in pendulum angle.

Here, we will add measurement uncertainty in pendulum angle θ (∆x₁ = 0.052) at 3s.

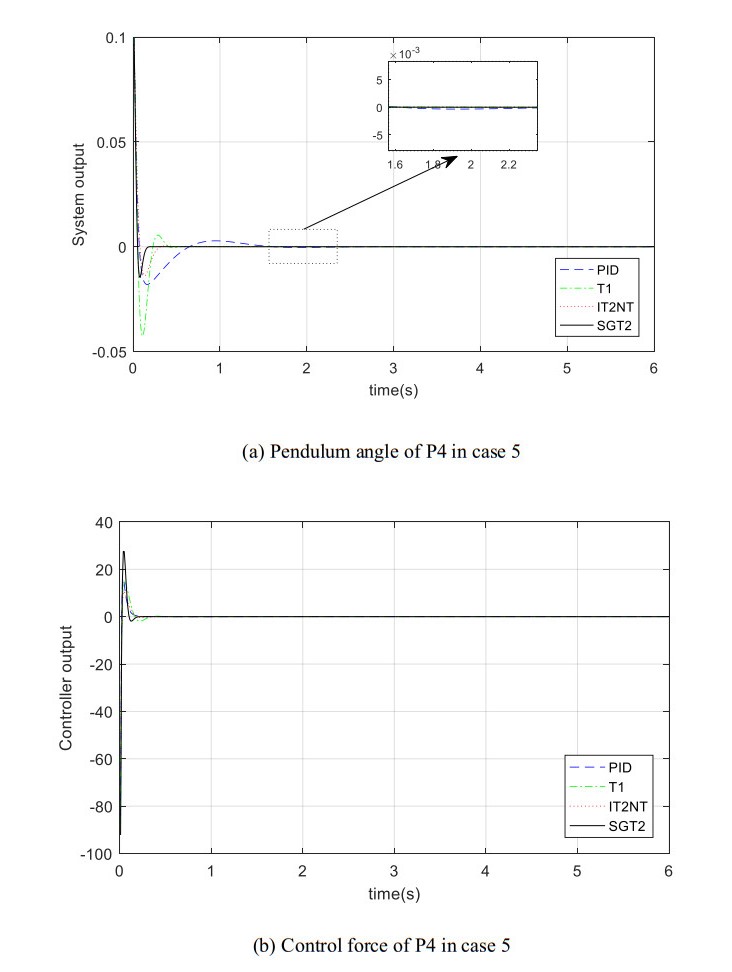
Case 5: Structure uncertainty. Here, we will add structural uncertainty in the inverted pendulum as 2s ($\Delta A = \left[ {\begin{array}{*{20}{c}} {0.03}&{0.03} \\ {0.03}&{0.03} \end{array}} \right]$), and the initial conditions x₁ = 0.1rad and x₂ = 0rad/s, the setting value is x₁ = 0rad.

Case 6: External disturbance uncertainty.

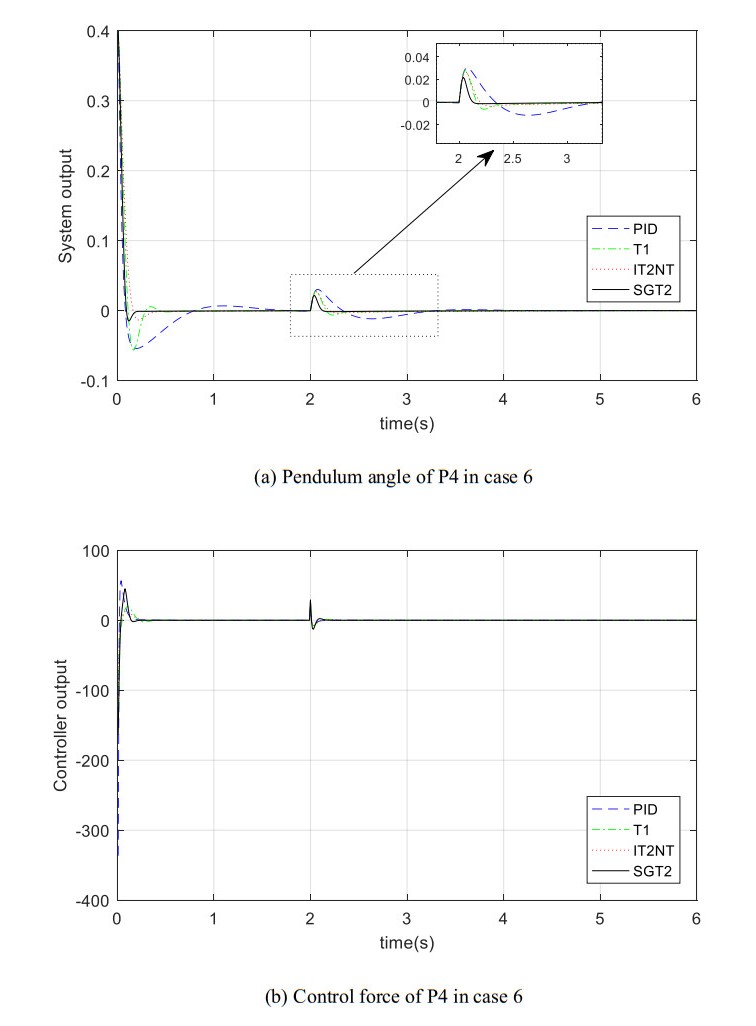
Here, we will add an external disturbance of controlling force at 2s (∆d = 29N).

Table 4 shows the P4 controlling performance comparisons of SGT2F-PID controller with other 5 controllers for case 1 and case 2. As compares with controlling performances of ^[80] and ^[81], another two error integral criterions are added as follows.

Table 5 shows the P4 controlling performance comparisons of SGT2F-PID controller with other 4 controllers for case 3 to case 6.

6.   Conclusions

We discuss 3 kinds of fuzzy PID controllers and derive the mathematical expressions of TIF-PID, IT2F-PID and SGT2F-PID described by Eq (21), Eq (25) and Eq (30). The SGT2F-PID controller contains more adjustable parameters and only 4 fuzzy rules are generated. For the primary membership degree of α-plane (α = 1) is used to get the defuzzification result of SGT2F-PID controller, thus the SGT2F-PID controller maintains the ability of handing uncertainties as general type-2 fuzzy controller and higher real-time. By the mathematical expressions of TIF-PID, IT2F-PID and SGT2F-PID controller, the controlling performance is discussed and explains why SGT2F-PID controller has better controlling effects than TIF-PID and IT2F-PID controller.

And 4 simulations including a second order linear plant, an unstable first order linear plant and two second order nonlinear plants are tested. In addition, the controller parameters of each plant are the same when the plant parameters are changed, which demonstrate the robustness of SGT2F-PID controller. From the 4 simulation results, when the controlled object changes, the SGT2F-PID controller can still maintain small overshoot, faster response time and stable time. Also the controller performance evaluation indexes (ISE, ITSE, ITAE) of SGT2F-PID controller are better than other 3 compared controllers. The results of simulation 4 indicates that, when the controlled object exists uncertainties of measurement, structure and external disturbance, the SGT2F-PID controller can handle these uncertainties more robust than PID, TIF-PID and IT2F-PID controller.

The next researches will focus on the following 4 aspects:

Ⅰ). Although SGT2F-PID controller can achieve better control performances, but the determined parameters are more than other controllers. How to determine the appropriate parameters will be a major work.

Ⅱ). Triangular function is applied as primary and secondary membership function, other membership function like Gaussian, trapezoid will be discussed in the future.

Ⅲ). In this paper, we fix the parameters de and d1 and discuss the influence of w on the controller parameters gains. In the future, we will study the influence of de and d1 on the controller parameters gains.

Ⅳ). The fractional order simplified general type-2 fuzzy PID controller will be investigated and compared with existing PID and fuzzy PID controllers.

Acknowledgments

This study was funded by the scientific research fund project of Nanjing Institute of Technology (YKJ201523, QKJ201802).

Conflict of Interest

The authors declare there is no conflict of interest.