Research on decision-making of emergency plan for waterlogging disaster in subway station project based on linguistic intuitionistic fuzzy set and TOPSIS

1.   Introduction

In recent years, global warming has led to more frequent rainstorms in some regions. Developing countries with inadequate water control infrastructure, including China, suffer from frequent flooding ^[1,2]. According to the 2017 China Eco-Environmental Status Bulletin issued by the Ministry of Ecology and Environment of China, 55.15 million people were affected by waterlogging in 2017, and 316 people died. Waterlogging disaster directly led to a loss of 214.3 billion RMB. A construction project is necessarily an open environment, which is susceptible to the adverse effects of the surrounding environment. Also, the construction site of a subway station project is often 20–30 m below the surface. Rainwater will naturally converge to the construction site of a subway station project, which may cause serious safety hazards ^[3].

Decision-making in an emergency plan for waterlogging disasters in subway station projects is complex. It must be known that a waterlogging disaster is about to occur or is occurring and project managers from multiple units (e.g., construction, design, and survey units) will be involved. The research issue is interdisciplinary, involving civil engineering, management, mathematics, and environmental science, requiring high-level project management practice ^[4].

In the practice of subway station engineering project management, personnel will expand the pre-programmed and principled emergency plans for waterlogging disasters into multiple, highly operable, and complete emergency plans based on specific conditions of the project. If the selected emergency plan is not scientific and effective, emergency management cannot quickly reduce disaster losses and resume construction activities ^[5,6]. Therefore, the decision-making for an emergency plan for a waterlogging disaster in subway station projects plays an essential role in the mitigation and mediation of waterlogging disasters.

Compared with the decision-making of routine events, the decision-making for an emergency plan for rainstorm waterlogging disaster in subway station projects has the prominent characteristics of short decision-making time and great decision-making pressure ^[7]. These characteristics reflect rapid disaster development speed, high uncertainty, and large potential losses. Therefore, it is difficult for decision-makers to provide accurate numerical evaluation values or probability values, which is an important basis for assessing the possibility of accidents and making decisions ^[8]. In engineering practice, decision-makers usually use language term information ^[9] as an evaluation value, such as poor, average, or good.

In recent years, to solve the problems of systematic evaluation and decision-making under linguistic terminology information, many researchers have tried to combine linguistic terminology set and fuzzy set theory to form a series of fuzzy linguistic terminology sets, and have achieved good theoretical and application results ^[10]. In the interval intuitionistic fuzzy set, the definition of language term set is expanded and the corresponding calculation rules are defined, namely, the language interval intuitionistic fuzzy set (LIVIFs) ^[10]. This can not only qualitatively present the decision-makers' understanding, attitude, and preference to emergency plans with the help of language terminology information, but also comprehensively and intuitively represent the uncertainty of decision-makers' understanding, attitudes, and preferences from dimensions of membership and non-membership degree. Therefore, compared with the decision-making method of quantitative data analysis ^[11,12], the decision-making method under the framework of linguistic interval intuitionistic fuzzy sets is more suitable for decision-making problems for rainstorm waterlogging disasters in subway station engineering.

Weight calculation is another important step in this decision-making. The commonly used weight calculation methods can be divided into two categories: Subjective and objective. The structural entropy weight method combines the Delphi expert investigation method, fuzzy analysis method, and entropy weight method ^[13]. The basic idea is to first collect experts' ranking and selection of the importance of indicators, then use the entropy weight method to correct the uncertainty and transform and analyze experts' opinions ^[14]. The structural entropy weight method retains the advantages of the subjective weight method with the strong explanatory power and high calculation precision of the objective weight method. In recent years, the structural entropy weight has achieved good application results in the field ^[15].

In the general structural entropy weight method, it is considered that the expert weights of all experts are the same. But many participants are involved in the decision-making of an emergency response plan for rainstorm and waterlogging disasters in subway station projects, and their characteristics vary greatly ^[14]. Decision-making experts have different educational backgrounds, experience (working years), knowledge level, and other characteristics, and their awareness of decision-making problems varies. Decision-making experts from different units should also have different expert weights and influence on the weight calculation results in varied ways ^[16]. From the perspective of reducing disaster risk loss, the experts of the work unit that bears the greater risk loss are likely eager and incentivized to reduce disaster loss, and thus should have greater expert weight. That is, the expert weight for emergency decision-making in construction projects should not be the same among decision-making experts and should be specifically calculated. This is influenced by the practical needs of construction project management, and also reflects differences between the decision-making of a construction disaster emergency plan and other emergency decisions. In this study, the expert knowledge and the knowledge blindness of the structural entropy weight method are improved to fully consider the characteristics of experts.

The last main component of this paper is to extend the application of the technique for order preference by similarity to ideal solution (TOPSIS) method in linguistic interval intuitionistic fuzzy sets. TOPSIS is a widely used, multi-scheme, decision-making method, which has the advantages of convenient calculation and no need for preset decision-making and evaluation grades ^[17,18]. The positive ideal scheme, the optimal combination of evaluation indexes in all schemes, and the negative ideal scheme, the most unfavorable combination of evaluation indexes in all schemes, are the core concepts in the TOPSIS method ^[19]. To accurately select the positive and negative ideal scheme, this paper selects a scientific and effective sorting method for the linguistic interval intuitionistic fuzzy numbers of each index under the background of linguistic interval intuitionistic fuzzy sets. The core of the TOPSIS method applied to decision-making is to calculate the distance between all schemes and ideal schemes, which is an important basis for calculating the relative closeness between each scheme and ideal schemes. This paper presents a distance measure formula for calculating these distances in the context of intuitionistic fuzzy sets of language intervals.

Based on the preceding analysis, this paper proposes a decision-making model of emergency plans for rainstorm waterlogging disasters in subway station engineering based on the guidelines of intuitionistic fuzzy sets and TOPSIS. The main contributions of this paper are as follows. (1) Decision-makers' understanding of, attitudes about, and preferences for emergency plans are quantitatively presented via the use of language terminology information, and the uncertainties of decision-makers' understanding, attitudes, and preferences are intuitively represented by the dimensions of the degrees of membership and non-membership in terms of uncertainty. (2) In this work, the improved structural entropy weight method is used to calculate the weights of indexes, which not only fully considers the subjective and objective factors in the calculation of weights, but also accurately describes the influences of experts' working units, educational backgrounds, working years, knowledge levels, and other characteristics via the improved expert average cognitive degree and knowledge blindness. (3) The TOPSIS method with a linguistic intuitionistic fuzzy set is extended, which effectively coordinates the complicated relationships among multiple targets in the decision-making of emergency plans for rainstorm waterlogging disasters in subway station projects, and overcomes the difficulties of scientific and effective decision-making and evaluation grade presetting in emergency decision-making. The model resolution of the TOPSIS method with a linguistic intuitionistic fuzzy set is also significantly higher than that of the classical TOPSIS method.

The remainder of this paper is organized as follows. Section 2 summarizes the research results related to the existing research work. Section 3 focuses on the establishment of the index system and emergency decision-making model, which are the critical explorations of this paper. In addition, the section 3 analyzes a classic decision-making problem as a benchmark and verification case. In the fourth section, the Huilong Road West Station of Chengdu Metro Line 11 in China is selected for a case study. Section 5 discusses the influences of different operators on the calculation results, and compares the results with those of the classical TOPSIS method. Section 6 presents the conclusion of this work.

2.   Related works

The research of emergency decisions about accidents attaches great importance to the reduction of the loss caused by emergencies. In recent years, relevant scholars have obtained some in-depth research results. After analyzing the entire process of waterlogging disasters, Wang et al. ^[20] proposed an emergency decision-making model for urban rail transit systems based on the regret theory. Chen et al. ^[21] put forward a disaster response program for urban waterlogging based on game theory, and comprehensively utilized both qualitative and quantitative information on urban waterlogging disasters. Cheong et al. ^[22] assessed the impacts of different cartographic representations on emergency route planning during flood response and found that, although complex map information contains most of the required information, it is not conducive to the rational planning of emergency routes due to the urgency of emergency decision-making. Ren et al. ^[6] established an evaluation index system of earthquake emergency plans from the perspectives of the operation mechanism, emergency response, and emergency support, and implemented the evaluation of earthquake emergency plans via the Hesitation Analytic Hierarchy Process (HAHP). However, most of this existing research was based on quantitative data analysis, which is problematic for the description of the uncertainty of decision-makers' understanding of, attitudes about, and preferences for emergency decision-making. To the best of the authors' knowledge, research on the decision-making of emergency plan for waterlogging disaster in subway station project has not yet been reported.

In addition, while the Analytic Hierarchy Process (AHP) method has often been used to calculate the weights in previous related research, it is highly subjective. Liang et al. ^[14] used the structural entropy weight method, which comprehensively considers both subjective and objective factors, to scientifically and effectively calculate the index weights of mined-out area pipelines. Liu et al. ^[15] employed the structural entropy weight method to determine the weights of the safety performance index of a fire protection system in a building, and verified the scientificity and effectiveness of this method via comparison with the provisions of relevant laws, regulations, and design specifications.

Multi-attribute decision-making methods based on qualitative language term sets are more flexible and applicable, and relevant approaches have become increasingly used in various fields ^[23]. Xu et al. ^[24] proposed a dynamic emergency decision-making method for large-group risks based on cumulative prospect theory, and used the clustering method to cluster the preferences of decision-making groups to determine the corresponding experts' weights. Chang et al. ^[25] developed a new model based on intuitionistic fuzzy sets to solve the complexity and uncertainty in the decision-making process. Two operators based on intuitionistic fuzzy sets were proposed, and the feasibility and effectiveness of the model were proven via empirical research. In addition, Ferdous et al. ^[26] pointed out that fuzzy sets and other soft computing methods could better characterize the uncertainty related to expert knowledge. Grosse ^[27] analyzed in detail the sources of uncertainty in an emergency management plan in Sweden, and presented an open framework of the systematic influence of uncertainty. Zhang et al. ^[28] used the fuzzy multi-criteria group decision-making method (FMCGDM) to construct a decision-making model of emergency response plans, and extended the TOPSIS method with FMCGDM to effectively coordinate the complicated relationships between multiple targets in emergency plan decision-making. This work provided substantial inspiration for the present research. According to the characteristics of emergency decision-making in crisis management, Gao et al. ^[29] proposed a dynamic decision-making method based on fuzzy sets of hesitation probability to overcome the shortage of information, uncertainty, and dynamic trends. Aimed at addressing the problems of insufficient risk identification, incomplete and inaccurate data, and the different preferences of decision-makers, Sun and Ma ^[30] established a new model by combining soft set theory with classical fuzzy rough set theory. Meng et al. ^[31] discussed how to use linguistic membership and linguistic non-membership to express the qualitative preference and non-preference judgments of decision-makers. Ou et al. ^[32] expressed uncertainty evaluation information in the form of linguistic intuitionistic fuzzy sets, and presented the calculation method of the scheme distance in the TOPSIS method.

3.   Methodology

3.1.   Establishment of the index system

Project decision-makers often synthesize expert opinions from multiple work units and quickly generate several emergency plans based on pre-set outlines. But pre-set emergency plans often lack completeness and operability. The main reason is that the construction of subway station projects is a dynamic process. Waterlogging that occurs at different times would need different construction measures, e.g., the number of people and machinery ^[33]. As such, the project management staff cannot prepare an emergency plan with strong operability and detailed content in advance. Also, plans should have the flexibility to accommodate changing conditions of waterlogging disasters.

Based on the above analysis, this article starts from the four aspects of scientific planning, completeness of the content, operability, and flexibility, and uses expert interviews, literature research, and other methods to build a decision index system for emergency plans for rainstorm and waterlogging disasters in subway stations. The indicator system has 4 primary indicators (scientific basis, completeness, operability, and flexibility) and 15 secondary indicators.

The uncertainty and time pressure for emergency plan decision-making make it difficult for decision-makers to obtain accurate and complete quantitative data promptly ^[34]. Therefore, all indicators in this article are qualitative indicators. Experts use the qualitative language of “extremely poor, very poor, poor, relatively poor, average, good, relatively good, very good, and excellent” to describe the score of each indicator. Also, all secondary indicators in Table 1 are value types, which means the better the index comments, the higher the score, and the better the solution.

3.2.   Establishment of the decision-making method for waterlogging disaster in subway station project based on the linguistic intuitionistic fuzzy set and TOPSIS

According to previous analyses, the decision-making method for waterlogging disasters in the subway station project mainly includes three parts: LIVIFS and related operators, index weight calculation methods based on improved structural entropy weight method, and the TOPSIS method under linguistic interval intuitionistic fuzzy sets. In the four sub-sections below, four independent and different symbols are used to describe the related sets and variables in each.

3.2.1.   Basic concepts of LIVIFS and related operators

Let $S = \left\{ {{s_\theta }|\theta = 0, 1, \cdots , h} \right\}$, where $h$ is a positive integer, ${s_\theta }$ represents the possible value of a language variable ^[35].

(1) If $\theta > \delta$, then ${s_\theta } > {s_\delta }$.

(2) There is a complement operator $neg$, which makes $neg\left( {{s_\theta }} \right) = {s_{h - \theta }}$.

$S$ is called a discrete set of languages.

Experts described the scores of each index with the qualitative language of “extremely poor, very poor, poor, a little poor, average, a little good, good, very good, and extremely good”.

To facilitate the calculation between languages, discrete language sets are often expanded into continuous language sets ${S_{\left[ {0, h} \right]}} = \left\{ {{s_\theta }|\theta \in \left[ {0, h} \right]} \right\}$ ^[36].

Let $X$ be the given set, then the intuitionistic fuzzy set $I$ on $X$ is defined as follows:

In Eq (2), ${u_I}\left( {{x_i}} \right)$ and, ${v_I}\left( {{x_i}} \right)$, respectively, represent the membership and non-membership of element ${x_i}$ to set $I$ ^[37]. The binary group $\left( {{u_I}\left( {{x_i}} \right), {v_I}\left( {{x_i}} \right)} \right)$ is called the intuitionistic fuzzy number. For convenience, it's abbreviated as $\beta = \left( {{u_I}, {v_I}} \right)$, where ${u_I}, {v_I} \in \left[ {0, 1} \right]$ and ${u_I} + {v_I} \leqslant 1$.

Let $X$ be a given set, and ${S_{\left[ {0, h} \right]}}$ be a continuous set of linguistic terms, then $\tilde A$ is a linguistic interval-valued intuitionistic fuzzy set defined on $X$ :

where ${s_{{{\tilde u}_{\tilde A}}}}\left( x \right) = \left[ {{s_{\tilde u_{_{\tilde A}}^L}}\left( x \right), {s_{\tilde u_{\tilde A}^U}}\left( x \right)} \right]$ represents the degree of membership of the language interval and ${s_{{{\tilde v}_{\tilde A}}}}\left( x \right) = \left[ {{s_{\tilde v_{\tilde A}^L}}\left( x \right), {s_{\tilde v_{\tilde A}^U}}\left( x \right)} \right]$ represents the degree of non-membership of the language interval. For any $x \in X$, ${s_{\tilde u_{\tilde A}^U}}\left( x \right){\rm{ + }}{s_{\tilde v_{\tilde A}^U}}\left( x \right) \leqslant {s_h}$ holds. The binary group $\left( {{s_{{{\tilde u}_{\tilde A}}}}\left( x \right), {s_{{{\tilde v}_{\tilde A}}}}\left( x \right)} \right)$ is called a linguistic interval-valued intuitionistic fuzzy number (LIVIFN), which can be abbreviated as $\tilde \alpha {\rm{ = }}\left( {{s_{{{\tilde u}_{\tilde A}}}}, {s_{{{\tilde v}_{\tilde A}}}}} \right)$, where ${s_{{{\tilde u}_{\tilde A}}}}, {s_{{{\tilde v}_{\tilde A}}}} \in {S_{\left[ {0, h} \right]}}$, and $\tilde u_{\tilde A}^U + \tilde v_{\tilde A}^U \leqslant h$ ^[38].

Four algorithms ^[39] were defined between LIVIFNs by T-norm and S-norm.

Suppose ${\tilde \alpha _i} = \left( {\left[ {{s_{{a_i}}}, {s_{{b_i}}}} \right], \left[ {{s_{{c_i}}}, {s_{{d_i}}}} \right]} \right)$ and $i = 1, 2$ are two LIVIFNs, such that:

where ${S_A}$ and ${T_A}$ are S-norm and T-norm on the unit interval, satisfying ${S_A}\left( {x, y} \right) = 1 - \left( {1 - x} \right)\left( {1 - y} \right)$ and ${T_A}\left( {x, y} \right) = xy$. ${f_A}:\left[ {0, 1} \right] \to \left[ {0, \infty } \right]$ and ${g_A}:\left[ {0, 1} \right] \to \left[ {0, \infty } \right]$ are generators of ${S_A}$ and ${T_A}$, respectively satisfying ${f_A} = - \operatorname{In} \left( {1 - x} \right)$ and ${g_A} = f\left( {N\left( x \right)} \right)$, where $N$ is the standard negative function $N\left( x \right) = 1 - x$ on the unit interval ^[40].

Let $\tilde \alpha = \left( {\left[ {{s_{{a_i}}}, {s_{{b_i}}}} \right], \left[ {{s_{{c_i}}}, {s_{{d_i}}}} \right]} \right)$ be a group of intuitionistic fuzzy numbers of language intervals, where $i = 1, 2, \cdots , n$.

The linguistic interval-valued intuitionistic fuzzy weighted average operator (LIVIFWA) is defined as follows ^[39]:

The linguistic interval-valued intuitionistic fuzzy weighted geometry operator (LIVIFWG) is defined as follows ^[39]:

3.2.2.   Index weight calculation method based on the improved structural entropy weight method

In this study, there are two kinds of weights, index and expert. The index weight reflects the importance of the index to the decision-making results. The greater the index weight, the greater the impact. Expert weight refers to the influence of experts on the weight calculation results. The greater the expert weight of an expert, the greater the influence of the expert's opinion on the result of weight calculation with indicators.

In this paper, the structural entropy weight method is used to calculate the index weight. The expert cognitive degree of the structural entropy weight method is improved to include the influence of the work unit of the decision-making expert on the weight. Also, this paper improves knowledge blindness to fully consider the impact of the educational background, experience (working years), knowledge level, and other characteristics of decision-making experts on the uncertainty of experts' cognition ^[6,14].

The detailed steps of the index weight calculation method based on the improved structural entropy weight method are as follows:

Step 1: Typical ranking based on experts' opinions.

Through the Delphi expert survey method, the relative importance ranking of indicators ${a_{ij}}$ is collected to form an expert ranking opinion. This ${a_{ij}}$ indicates the importance evaluation of the $i$ expert ($1 \leqslant i \leqslant k$) on the $j$ index ($1 \leqslant j \leqslant n$).

Step 2: Weight correction of typical ranking.

According to the idea of entropy weight method, the uncertainty of experts' typical ranking is corrected. This is the most important step in the structural entropy weight method.

(1) Quantitative transformation of qualitative typical sorting

Define the membership function of sorting transformation as follows:

where ${p_n}\left( I \right){\rm{ = }}\frac{{m - I}}{{m - 1}}$ and $\lambda {\rm{ = }}\frac{1}{{\operatorname{In} \left( {m - 1} \right)}}$. $I$ is the qualitative ranking number given by experts after evaluating an index according to the format of typical ranking, $1 \leqslant I \leqslant j$. The $m$ is transformation parameters, and $m = j + 2$ is generally taken ^[14].

With $\mu \left( I \right) = \chi \left( I \right)\left( {\frac{{m - 1}}{{m - I}}} \right) - 1$, then $\mu \left( I \right)$ can be reduced as follows:

The ranking quantitative conversion value ${b_{ij}} = \mu \left( {{a_{ij}}} \right)$ can be obtained by bringing the ranking ${a_{ij}}$ of the indexes by experts into Eq (11).

(2) According to the results of quantitative transformation, experts' recognition of the importance of indicators is defined. The degree of recognition here is the weight of experts, which refers to the influence of different experts on the weight calculation results.

At present, when the structural entropy weight method is used in relevant researches, it is often assumed that all experts have the same degree of understanding, and the corresponding formula is as follows ^[14]:

Different construction units play different roles in the management of waterlogging disasters in subway station projects and bear different disaster losses. From the perspective of reducing disaster losses, the units that bear the most losses have more eager and subjective expert opinions and thus the higher these expert weights should be. For example, under the most common BT construction mode in the world, all personnel and property losses within a construction site caused by waterlogging disaster shall be borne by the unit itself, and losses caused to engineering entities shall be borne by the construction unit. Also, the cost incurred by the construction enterprise in the process of emergency rescue shall be borne by the owner unit. From the perspective of disaster loss-bearing, it can be qualitatively considered that the construction unit bears the greatest risk of waterlogging disaster loss. Thus, under BT mode, experts from construction units are more eager to reduce (and subjective in reducing) disaster losses, and their expert weight should be greater.

It is worth mentioning that different project management modes or different contractual agreements will lead to different disaster consequences for all parties. However, we should always attach importance to the opinions of experts from units that bear more losses. From the perspective of the engineering management discipline, under different project management modes, the emergency disaster risk commitment of all participating units is shown in Table 2. In Table 2, the expert weight of the unit that bears the most risks is set to 3, the unit that bears the second most risks is set to 2, and the unit that bears the least risks is set to 1.

The formula for calculating the improved experts' recognition of the importance of the index is as follows:

where $w{z_j}$ is the expert importance score of the $j$ expert, which can be obtained from Table 2 according to the work unit of the expert.

(3) The uncertainty of experts due to cognition is defined as knowledge blindness. In previous research results, it is often believed that different experts have the same uncertainty due to cognition.

The formula for calculating the parameters of knowledge blindness is as follows ^[15]:

However, in the practice of emergency decision-making, the educational background, working years and knowledge level of emergency decision-makers are often different, and the uncertainty of decision-making due to cognition is also different. Also, some scholars believe that the uncertainty caused by experts' cognition is a normal distribution. However, only when the number of experts is sufficient can we approximately think that the uncertainty of different experts due to cognition is normally distributed. The precondition of the normal distribution is difficult to meet in the emergency decision of sudden natural disasters in engineering projects.

Generally speaking, experts with higher academic qualifications, longer working years and higher level of knowledge can be considered to have a clearer understanding of decision-making issues and less uncertainty. According to the results of previous studies, different experts are assigned scores according to their achievements. Based on the management practice of the construction project, its "Educational qualification" was replaced by the time of directly participating in the project.

The formula for calculating the parameter ${Q_j}$ of the improved knowledge blindness is as follows:

where $w{m_k}$ is the expert uncertainty score of the $j$ expert. The higher the score, the lower the uncertainty of the expert due to cognition in this decision-making. According to the personal information of the expert, the score of each index can be obtained from Table 3, and the sum is the expert uncertainty score.

(4) Define the overall understanding ${x_j}$ of $k$ expert to the $j$ index as follows:

The evaluation vector $X = {\left( {{x_1}, {x_2}, \cdots , {x_n}} \right)^T}$ of the index set was obtained by $k$ experts.

Step 3: Normalization processing

Then $W = {\left( {{\omega _1}, {\omega _2}, \cdots , {\omega _n}} \right)^{\mathop{\rm T}\nolimits} }$ is the weight vector of the expert to the index set $U = {\left( {{\mu _1}, {\mu _2}, \cdots , {\mu _n}} \right)^T}$.

3.2.3.   The TOPSIS method under the LIVIFS

Based on the previous theoretical analysis on linguistic interval intuitionistic fuzzy sets, this paper combines the previous research results to expand the TOPSIS method in the context of linguistic interval intuitionistic fuzzy sets.

Detailed steps, including solutions to two key technical problems, are as follows:

(1) Calculating a comprehensive matrix

LIVIFWA ^[39]:

LIVIFWG ^[39]:

(2) Obtain the positive ideal value and the negative ideal value of each index.

Positive ideal value:

Negative ideal value:

where $i = 1, 2, \cdots , m$, $j = 1, 2, \cdots , n$.

The key to calculating the positive ideal value and negative ideal value lies in the sorting of pairs. This paper selects the sorting method proposed and shown below ^[18].

If $Sco\left( {{{\tilde \alpha }_1}} \right) < Sco\left( {{{\tilde \alpha }_2}} \right)$, then ${\tilde \alpha _1} \prec {\tilde \alpha _2}$.

If $Sco\left( {{{\tilde \alpha }_1}} \right) = Sco\left( {{{\tilde \alpha }_2}} \right)$ and $Acc\left( {{{\tilde \alpha }_1}} \right) < Acc\left( {{{\tilde \alpha }_2}} \right)$, then ${\tilde \alpha _1} \prec {\tilde \alpha _2}$.

(3) Calculating the sum of separation measures, $Sep_i^ -$ and $Sep_i^ +$, of all schemes ${x_i}\left( {i = 1, 2, \cdots , m} \right)$ :

where $d\left( {\tilde \alpha _{ij}^{pos}, {{\tilde \alpha }_{ij}}} \right)$ represents the distance between the evaluation value ${\tilde \alpha _{ij}}$ and the positive ideal solution $\tilde \alpha _{ij}^{pos}$, and $d\left( {\tilde \alpha _{ij}^{neg}, {{\tilde \alpha }_{ij}}} \right)$ represents the distance between the evaluation value ${\tilde \alpha _{ij}}$ and the negative ideal solution $\tilde \alpha _{ij}^{neg}$.

Let ${\tilde \alpha _i} = \left( {\left[ {{s_{{a_i}}}, {s_{{b_i}}}} \right], \left[ {{s_{{c_i}}}, {s_{{d_i}}}} \right]} \right)$ and $i = 1, 2$ be intuitionistic fuzzy numbers between two groups of languages. The relevant distance measures are defined as follows ^[25,30]:

(4) Calculating the closeness degree $C{O_i}$ of the scheme ${x_i}\left( {i = 1, 2, \cdots , m} \right)$

The greater the closeness $C{O_i}$, the better the actual performance of the corresponding plan ${x_i}\left( {i = 1, 2, \cdots , m} \right)$; the less the closeness $C{O_i}$, the worse the actual performance of the corresponding plan ${x_i}\left( {i = 1, 2, \cdots , m} \right)$.

3.2.4.   Decision-making method of emergency plans

Based on the language interval intuitionistic fuzzy set and its agammaegation operator mentioned above, the index weight calculation model based on the improved structural entropy weight method and the language interval intuitionistic fuzzy TOPSIS method, the decision-making method of an emergency plan is constructed. The detailed steps are as follows:

Step 1: Collect evaluation information and obtains the individual evaluation matrix ${D_k} = {\left( {\tilde \alpha _{ij}^k} \right)_{m \times n}}$ of all experts, where $k = 1, 2, \cdots , t$.

Step 2: Perform the index weight calculation model in Section 3.2.2 to obtain a comprehensive weight vector ${\lambda ^ * } = {\left( {{\lambda _1}, {\lambda _2}, \cdots , {\lambda _t}} \right)^{\rm{T}}}$.

Step 3: Agammaegate the evaluation matrix ${D_k} = {\left( {\tilde \alpha _{ij}^k} \right)_{m \times n}}$ and the comprehensive weight vector ${\lambda ^ * } = {\left( {{\lambda _1}, {\lambda _2}, \cdots , {\lambda _t}} \right)^{\rm{T}}}$ into a comprehensive matrix $D = {\left( {\tilde \alpha _{ij}^k} \right)_{m \times n}}$.

Step 4: Execute the language interval intuitionistic fuzzy TOPSIS method in section 3.2.3 to obtain the closeness degree $C{O_i}$ of each evaluation scheme, and obtains the ranking of the scheme ${x_i}\left( {i = 1, 2, \cdots , m} \right)$ according to the value of the closeness degree $C{O_i}$.

The steps of the method are shown in Figure 1.

3.3.   Benchmark and verification case

Before the formal case study, a benchmark and verification case was conducted. Due to the lack of research on the emergency decision-making of subway station engineering or rainstorm waterlogging disasters via the use of interval intuitionistic fuzzy sets and TOPSIS, the decision-making of real estate location was selected as a benchmark and verification case. This classical decision-making problem, which has many existing research results, has been frequently used as an example for the verification of the TOPSIS algorithm.

The relevant data for this problem are reported in previous research ^[41,42]. The method proposed in this paper was used for calculation and sorting, and the calculation results are reported in Table 4. The results of the traditional TOPSIS method ^[41] and a multi-attribute decision-making method based on balanced expectations ^[42] are also presented in Table 4.

From the calculation results in Table 4, it is evident that, for this classical decision-making problem, the rankings of the four methods were basically the same, and the best location was C. The ranking results of the four methods were only different for the selection of the fourth and fifth locations. Compared with the classical TOPSIS method and the balanced expectation method used in the field of economics, the research methods proposed in this paper (LIVIFWA and LIVIFWG) are both scientific and effective.

In addition, the methods proposed in this paper were found to have stronger resolution. In the results of the classical TOPSIS method, the $CO$ values of C and B were very close; the difference was only 0.004 (0.47% relative deviation). However, in the results of the proposed methods, there were significant differences between the $CO$ values of C and B; the difference for LIVIFWA was 0.166 (40.29% relative deviation) and the difference for LIVIFWG was 0.85 (19.50% relative deviation). It is evident that the proposed methods were able to highlight the difference between each scheme and the ideal solution, and the resolutions of the scheme ranking results were stronger than that of the classical TOPSIS method. Better resolution indicates that the model is easier to use and has a wider application range, which is a major advancement of the research method employed in this paper.

4.   Case study

4.1.   Background

The first phase of Chengdu Metro Line 11 is located in Chengdu, with a total length of 22.0 km and a total of 18 underground stations. The total investment of this project is 1.33 billion yuan. The project was scheduled to start in 2017 and start operations in December 2020. Huilong Road West Station is a typical station in this project, which is an underground, two-story, island platform station with a two-story, cast-in-place, and frame structure. The total length of this station is 510.8 m, and the total width of the standard section is 21.10 m. The maximum depth of this station is 21.26 m, which is the deepest station project of the first phase of Chengdu Metro Line 11. There are many abandoned sewage pipes around Huilong Road West Station, and Qinglan Ditch, which has been flooded many times, passes through this station. The surrounding areas of the station are mostly farmland and wasteland, with good water absorption capacity and poor drainage capacity.

The owner unit of Huilong Road West Station is Chengdu Rail Transit Group Co., Ltd.; the construction unit is China National Construction Corporation. The design unit is mainly China Railway Fourth Survey and Design Institute Group Co., Ltd. The project management mode of this project is BT. According to the relevant laws of China and the contract of the project, the storm and waterlogging disaster is a “force majeure event”. All personnel and property losses in the construction site caused by the waterlogging disaster are borne by the unit itself, and the losses caused to the engineering entity are attributed to the construction unit. The cost of construction enterprises in the process of emergency rescue and emergency rescue is borne by the owner.

Beginning on July 6, 2018, Chengdu, Sichuan Province, China began to receive continuous rainfall. The average 24-hour rainfall in the urban area of Chengdu reached 60–80 mm, and the rainfall in some areas exceeded 250 mm. At 10:00 am on July 11, 2018, Chengdu officially released a rainstorm orange warning signal, which is the highest signal for a rainstorm warning in China. At this time, the topography of Huilong Road West Station on Chengdu Metro Line 11 was low, and it was currently in a critical period of main structure construction. There were a large number of on-site construction workers, mechanical equipment, and building materials, which are prone to waterlogging disasters, causing huge casualties and property losses.

After receiving the Provincial Level Ⅱ Flood Control Emergency Response initiated by the government authorities, the participating units of the Huilong Road West Station Station Project were quickly organized to formulate four emergency plans $Y = \left\{ {{y_1}, {y_2}, {y_3}, {y_4}} \right\}$, as shown in Table 5.

Six experts were organized to participate in the rainstorm and waterlogging disaster emergency plan decisions of the project. The basic situation of the six experts in the expert evaluation group $E = \left\{ {{e_1}, {e_2}, \cdots , {e_6}} \right\}$ is shown in Table 6.

4.2.   The emergency plan for the waterlogging disaster of Huilong Road West Station project

In this section, the decision-making method given in section 3.2.4 was adopted to give the detailed calculation process of case analysis with a step-by-step approach.

4.2.1.   Collecting evaluation information and obtaining an individual evaluation matrix

Six experts comprehensively evaluated the four emergency plans according to the index system in Table 1. By using the language term set extreme pool (${s_0}$), very pool (${s_1}$), pool (${s_2}$), little pool (${s_3}$), average (${s_4}$), little good (${s_5}$), good (${s_6}$), very good (${s_7}$), and extremely good (${s_8}$) the evaluation matrix ${D_k} = {\left( {\tilde \alpha _{ij}^k} \right)_{m \times n}}$ of existing plans $X = \left\{ {{x_1}, {x_2}, {x_3}, {x_4}} \right\}$ under each index in the form of linguistic interval intuitionistic fuzzy sets were generated. The evaluation information is shown in Table 7.

4.2.2.   Obtaining a comprehensive weight vector

According to the personal information of the six experts in Table 6, this can be obtained by referring to Tables 2 and 3, as shown in Table 8.

The calculation process and results of calculating the weight of each index based on the improved structural entropy weight method are shown in Table 9.

From Table 9, it is clear that P3 (operability) had the largest weight of the four primary indexes, which indicates that operability is the most critical factor in the decision-making of emergency plans for waterlogging disasters in subway station projects. Regarding the secondary indexes, the comprehensive weight of C13 (flexible response to the changes in the emergency response level) was 0.1070, and this is therefore the most important factor. The weights of C1 (compliance with relevant regulations and policies) and C12 (effectiveness of reducing disasters) were also large. The factors C5 (a reasonable command system), C11 (quickness in treating the overwhelmed), and C6 (full use of emergency rescue supplies and personnel) had the smallest weights. Based on the analysis of the weight results, the following suggestions are provided: The preparation and decision-making of emergency plans should focus on operability, the flexibility of the emergency plan for disaster changes, the consistency with relevant laws and policies, and the rescue efficiency. Based on these suggestions, the analysis and demonstration of the rationality of the command system, and the speed of treating the wounded, could be reduced in the preparation, decision-making, and execution of emergency plans.

4.2.3.   Getting a comprehensive matrix by assembling the evaluation matrix and the comprehensive weight vector

The calculation results based on LIVIFWA are shown in Table 10.

4.2.4.   Obtaining the closeness degree and ranking of each evaluation scheme

Perform the language interval intuitionistic fuzzy TOPSIS method in section 3.2.3 to obtain the closeness degree $C{O_i}$ of each evaluation scheme and obtain the ranking of schemes ${x_i}\left( {i = 1, 2, \cdots , m} \right)$ according to the value of closeness degree $C{O_i}$.

(1) Using the ranking method based on scoring function and accuracy function, the positive ideal value $\tilde \alpha _{ij}^{pos}$ and the negative ideal value $\tilde \alpha _{ij}^{neg}$ of all secondary indexes are obtained.

C₁: $\tilde \alpha _{i1}^{pos} = \max \left\{ {{{\tilde \alpha }_{i1}}} \right\} = \left( {\left[ {{s_{5.490}}, {s_{6.843}}} \right], \left[ {{s_{0.792}}, {s_{1.301}}} \right]} \right)$, $\tilde \alpha _{i1}^{neg} = \min \left\{ {{{\tilde \alpha }_{i1}}} \right\} = \left( {\left[ {{s_{3.707}}, {s_{5.173}}} \right], \left[ {{s_{0.792}}, {s_{2.092}}} \right]} \right)$.

C₂: $\tilde \alpha _{i2}^{pos} = \max \left\{ {{{\tilde \alpha }_{i2}}} \right\} = \left( {\left[ {{s_{5.342}}, {s_{6.197}}} \right], \left[ {{s_{0.785}}, {s_{1.736}}} \right]} \right)$, $\tilde \alpha _{i2}^{neg} = \min \left\{ {{{\tilde \alpha }_{i2}}} \right\} = \left( {\left[ {{s_{4.201}}, {s_{5.450}}} \right], \left[ {{s_{2.331}}, {s_{2.783}}} \right]} \right)$.

C₃: $\tilde \alpha _{i3}^{pos} = \max \left\{ {{{\tilde \alpha }_{i3}}} \right\} = \left( {\left[ {{s_{5.039}}, {s_{5.836}}} \right], \left[ {{s_{1.432}}, {s_{2.143}}} \right]} \right)$, $\tilde \alpha _{i3}^{neg} = \min \left\{ {{{\tilde \alpha }_{i3}}} \right\} = \left( {\left[ {{s_{3.810}}, {s_{5.016}}} \right], \left[ {{s_{1.601}}, {s_{2.092}}} \right]} \right)$.

C₄: $\tilde \alpha _{i4}^{pos} = \max \left\{ {{{\tilde \alpha }_{i4}}} \right\} = \left( {\left[ {{s_{5.131}}, {s_{6.642}}} \right], \left[ {{s_{0.728}}, {s_{1.224}}} \right]} \right)$, $\tilde \alpha _{i4}^{neg} = \min \left\{ {{{\tilde \alpha }_{i4}}} \right\} = \left( {\left[ {{s_{3.837}}, {s_{5.615}}} \right], \left[ {{s_{0.950}}, {s_{1.702}}} \right]} \right)$.

C₅: $\tilde \alpha _{i5}^{pos} = \max \left\{ {{{\tilde \alpha }_{i5}}} \right\} = \left( {\left[ {{s_{5.457}}, {s_{6.401}}} \right], \left[ {{s_{0.537}}, {s_{1.531}}} \right]} \right)$, $\tilde \alpha _{i5}^{neg} = \min \left\{ {{{\tilde \alpha }_{i5}}} \right\} = \left( {\left[ {{s_{5.157}}, {s_{6.488}}} \right], \left[ {{s_{1.430}}, {s_{2.031}}} \right]} \right)$.

C₆: $\tilde \alpha _{i6}^{pos} = \max \left\{ {{{\tilde \alpha }_{i6}}} \right\} = \left( {\left[ {{s_{5.313}}, {s_{6.419}}} \right], \left[ {{s_{0.726}}, {s_{1.241}}} \right]} \right)$, $\tilde \alpha _{i6}^{neg} = \min \left\{ {{{\tilde \alpha }_{i6}}} \right\} = \left( {\left[ {{s_{5.157}}, {s_{6.488}}} \right], \left[ {{s_{1.430}}, {s_{2.031}}} \right]} \right)$.

C₇: $\tilde \alpha _{i7}^{pos} = \max \left\{ {{{\tilde \alpha }_{i7}}} \right\} = \left( {\left[ {{s_{4.842}}, {s_{5.614}}} \right], \left[ {{s_{0.631}}, {s_{1.711}}} \right]} \right)$, $\tilde \alpha _{i7}^{neg} = \min \left\{ {{{\tilde \alpha }_{i7}}} \right\} = \left( {\left[ {{s_{5.157}}, {s_{6.488}}} \right], \left[ {{s_{1.430}}, {s_{2.031}}} \right]} \right)$.

C₈: $\tilde \alpha _{i8}^{pos} = \max \left\{ {{{\tilde \alpha }_{i8}}} \right\} = \left( {\left[ {{s_{5.004}}, {s_{6.138}}} \right], \left[ {{s_{0.979}}, {s_{1.330}}} \right]} \right)$, $\tilde \alpha _{i8}^{neg} = \min \left\{ {{{\tilde \alpha }_{i8}}} \right\} = \left( {\left[ {{s_{4.102}}, {s_{5.315}}} \right], \left[ {{s_{1.027}}, {s_{2.237}}} \right]} \right)$.

C₉: $\tilde \alpha _{i9}^{pos} = \max \left\{ {{{\tilde \alpha }_{i9}}} \right\} = \left( {\left[ {{s_{4.804}}, {s_{6.103}}} \right], \left[ {{s_{0.970}}, {s_{1.472}}} \right]} \right)$, $\tilde \alpha _{i9}^{neg} = \min \left\{ {{{\tilde \alpha }_{i9}}} \right\} = \left( {\left[ {{s_{4.029}}, {s_{5.104}}} \right], \left[ {{s_{1.338}}, {s_{2.340}}} \right]} \right)$.

C₁₀: $\tilde \alpha _{i10}^{pos} = \left( {\left[ {{s_{4.859}}, {s_{6.107}}} \right], \left[ {{s_{1.441}}, {s_{2.006}}} \right]} \right)$, $\tilde \alpha _{i10}^{neg} = \left( {\left[ {{s_{3.223}}, {s_{5.076}}} \right], \left[ {{s_{1.467}}, {s_{2.324}}} \right]} \right)$.

C₁₁: $\tilde \alpha _{i11}^{pos} = \left( {\left[ {{s_{4.859}}, {s_{6.107}}} \right], \left[ {{s_{1.441}}, {s_{2.006}}} \right]} \right)$, $\tilde \alpha _{i11}^{neg} = \left( {\left[ {{s_{3.746}}, {s_{5.577}}} \right], \left[ {{s_{1.200}}, {s_{1.582}}} \right]} \right)$.

C₁₂: $\tilde \alpha _{i12}^{pos} = \left( {\left[ {{s_{3.458}}, {s_{5.260}}} \right], \left[ {{s_{1.798}}, {s_{2.638}}} \right]} \right)$, $\tilde \alpha _{i12}^{neg} = \left( {\left[ {{s_{3.223}}, {s_{5.076}}} \right], \left[ {{s_{1.467}}, {s_{2.324}}} \right]} \right)$.

C₁₃: $\tilde \alpha _{i13}^{pos} = \left( {\left[ {{s_{4.859}}, {s_{6.107}}} \right], \left[ {{s_{1.441}}, {s_{2.006}}} \right]} \right)$, $\tilde \alpha _{i13}^{neg} = \left( {\left[ {{s_{3.223}}, {s_{5.076}}} \right], \left[ {{s_{1.467}}, {s_{2.324}}} \right]} \right)$.

C₁₄: $\tilde \alpha _{i14}^{pos} = \left( {\left[ {{s_{4.859}}, {s_{6.107}}} \right], \left[ {{s_{1.441}}, {s_{2.006}}} \right]} \right)$, $\tilde \alpha _{i14}^{neg} = \left( {\left[ {{s_{2.723}}, {s_{4.710}}} \right], \left[ {{s_{1.452}}, {s_{2.674}}} \right]} \right)$.

C₁₅: $\tilde \alpha _{i15}^{pos} = \left( {\left[ {{s_{4.859}}, {s_{6.107}}} \right], \left[ {{s_{1.441}}, {s_{2.006}}} \right]} \right)$, $\tilde \alpha _{i15}^{neg} = \left( {\left[ {{s_{3.535}}, {s_{5.018}}} \right], \left[ {{s_{1.036}}, {s_{2.420}}} \right]} \right)$.

(2) Separately calculating the separation measures $Sep_i^ -$ and $Sep_i^ +$ of all schemes.

$Sep_1^{\rm{ + }}{\rm{ = }}\frac{1}{{15}}\sum\limits_{j = 1}^{15} {d\left( {\tilde a_{ij}^{pos}, {{\tilde a}_{1j}}} \right)} = 0.067$, $Sep_1^ - {\rm{ = }}\frac{1}{{15}}\sum\limits_{j = 1}^{15} {d\left( {\tilde a_{ij}^{neg}, {{\tilde a}_{1j}}} \right)} = 0.087$.

$Sep_2^{\rm{ + }}{\rm{ = }}\frac{1}{{15}}\sum\limits_{j = 1}^{15} {d\left( {\tilde a_{ij}^{pos}, {{\tilde a}_{2j}}} \right)} = 0.043$, $Sep_2^ - {\rm{ = }}\frac{1}{{15}}\sum\limits_{j = 1}^{15} {d\left( {\tilde a_{ij}^{neg}, {{\tilde a}_{2j}}} \right)} = 0.185$.

$Sep_3^{\rm{ + }}{\rm{ = }}\frac{1}{{15}}\sum\limits_{j = 1}^{15} {d\left( {\tilde a_{ij}^{pos}, {{\tilde a}_{3j}}} \right)} = 0.183$, $Sep_3^ - {\rm{ = }}\frac{1}{{15}}\sum\limits_{j = 1}^{15} {d\left( {\tilde a_{ij}^{neg}, {{\tilde a}_{3j}}} \right)} = 0.067$.

$Sep_4^{\rm{ + }}{\rm{ = }}\frac{1}{{15}}\sum\limits_{j = 1}^{15} {d\left( {\tilde a_{ij}^{pos}, {{\tilde a}_{4j}}} \right)} = 0.153$, $Sep_4^ - {\rm{ = }}\frac{1}{{15}}\sum\limits_{j = 1}^{15} {d\left( {\tilde a_{ij}^{neg}, {{\tilde a}_{4j}}} \right)} = 0.086$.

(3) Calculating the closeness $C{O_i}$ of all schemes.

$C{O_1}{\rm{ = }}\frac{{Sep_1^ - }}{{Sep_1^{\rm{ + }}{\rm{ + }}Sep_1^ - }}{\rm{ = }}0.565$, $C{O_2}{\rm{ = }}\frac{{Sep_2^ - }}{{Sep_2^{\rm{ + }}{\rm{ + }}Sep_2^ - }}{\rm{ = 0}}{\rm{.811}}$,

$C{O_3}{\rm{ = }}\frac{{Sep_3^ - }}{{Sep_3^{\rm{ + }}{\rm{ + }}Sep_3^ - }}{\rm{ = }}0.268$, $C{O_4}{\rm{ = }}\frac{{Sep_4^ - }}{{Sep_4^{\rm{ + }}{\rm{ + }}Sep_4^ - }}{\rm{ = }}0.360$.

According to the closeness degree of each plan, the order is ${y_2} \succ {y_1} \succ {y_4} \succ {y_3}$, and the optimal plan is ${y_2}$.

5.   Discussions

In this work, a decision-making model of emergency plans for waterlogging disasters in subway station projects was constructed based on linguistic intuitionistic fuzzy sets and TOPSIS. However, the relationship between the allocation of disaster loss and experts' weights was only described qualitatively, which was the major limitation in this study.

LIVIFWA and LIVIFWG are two operators in the LIVIFS. The calculation results based on these two operators were compared, and the results are presented in Table 11. In addition, the results of experts' surveys presented in Table 7 were processed into the classical TOPSIS, and the results are also exhibited in Table 11.

From Table 11, it is evident that the order based on the LIVIFWG operator was ${y_2} \succ {y_1} \succ {y_3} \succ {y_4}$ and the optimal plan was ${y_2}$. However, the ranking of schemes ${y_3}$ and ${y_4}$ was different from that based on LIVIFWA; the reason for this might be that LIVIFWG increased the interference of extreme evaluation information on the decision results ^[43]. The order based on the classical TOPSIS was ${y_2} \succ {y_1} \succ {y_4} \succ {y_3}$. However, because the classical TOPSIS does not consider the uncertainty of indexes in the evaluation, the relative closeness results of schemes ${y_1}$ and ${y_4}$ were very close, and the reliability and effectiveness of the final ranking results were difficult to guarantee. The decision-making model proposed in this paper is able to fully describe the decision-makers’ understanding of, attitudes about, and preferences for emergency plans via the linguistic intuitionistic fuzzy set, and can comprehensively and intuitively represent the uncertainty of the decision-makers’ understanding, attitudes, and preferences from the dimensions of the membership and non-membership degrees with uncertainty. Compared with the classical TOPSIS, the method proposed in this paper can more comprehensively and accurately describe the target attribute information.

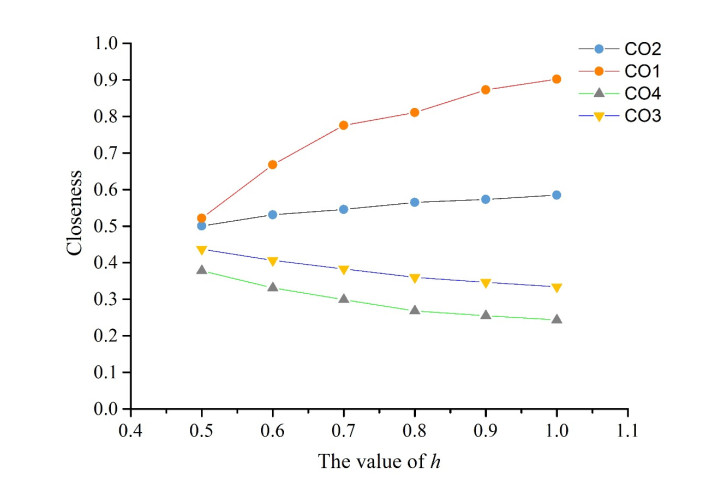
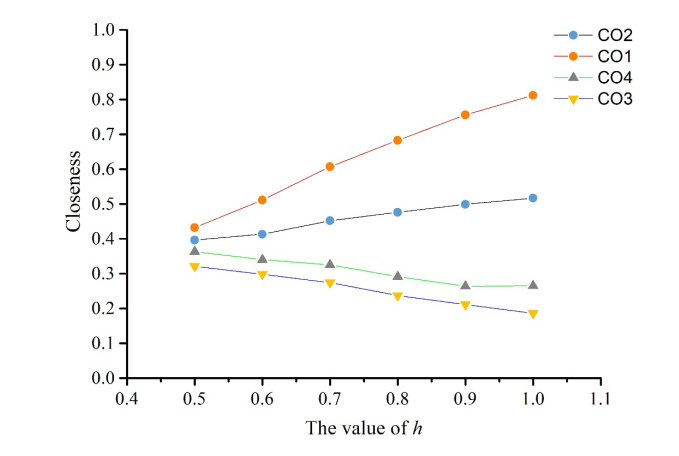
The uncertainty analysis of data is the content in related research on which focus should be placed ^[44]. In this paper, $h$ is an important parameter in interval intuitionistic fuzzy sets, and expresses the upper limit of certainty in expert cognition; $h{\rm{ = }}1$ means that there is no uncertainty in expert cognition, while $h{\rm{ = 0}}$ means that there is no certainty in expert cognition. Additionally, $h{\rm{ < }}0.5$ means that the expert's uncertainty is greater than the certainty, and it can be considered that the decision-making based on expert cognition is invalid ^[45]. The calculation results of $h = 1, 0.9, 0.8, 0.7, 0.6, 0.5$ are presented in Figures 2 and 3.

As presented in Figures 2 and 3, within the range of h = 0.5–1, the CO ranking of each scheme did not change with the variation of h. This suggests that the uncertainty in this research did not affect the evaluation results. It is worth noting that the range of h = 0–0.5 has no discussion value, as the uncertainty is greater than the certainty at this time ^[45]. Regardless of which operator was used, with the increase of h, the difference between the CO value of each scheme also increased. Therefore, the greater the certainty of the research problem, the stronger the CO sorting resolution of each scheme.

6.   Conclusions

In consideration of the strong uncertainty in the decision-making of emergency plans for waterlogging disasters in subway station projects, this paper used the membership and non-membership degrees in a linguistic intuitionistic fuzzy set to represent the uncertainties of the decision-makers' understanding, attitudes, and preferences. An evaluation index system with 15 secondary indicators was constructed based on four aspects, namely a scientific basis, completeness, operability, and flexibility. In this work, the improved structural entropy weight method was employed to calculate the index weights, which not only fully considers the subjective and objective factors in the process of weight calculation, but also accurately describes the influence of experts' working units, educational backgrounds, working years, knowledge levels, and other characteristics via the improved expert average cognitive degree and knowledge blindness. To overcome the fact that it is difficult to scientifically and effectively preset decision-making and evaluation grades for emergency decision-making, the TOPSIS method was extended with a linguistic intuitionistic fuzzy set, and the intricate relationships between multiple targets in the decision-making of emergency plans for waterlogging disasters in subway station projects were effectively coordinated. The calculation results of benchmark and verification case highlight the rationality and scientificity of the method proposed in this paper. The results of a case study demonstrated that the key to the preparation and decision-making of emergency plans is the plans’ operability. Compared with the classical TOPSIS, the model proposed in this paper was proven to exhibit better reliability and effectiveness. A future research direction is the quantitative analysis of the relationship between the allocation of disaster loss and expert weights to provide a more comprehensive approach.

Acknowledgments

This paper is supported by the Science and Technology Project of Wuhan Urban and Rural Construction Bureau, China (201943).

Conflict of Interests

The authors declare there is no conflict of interests.