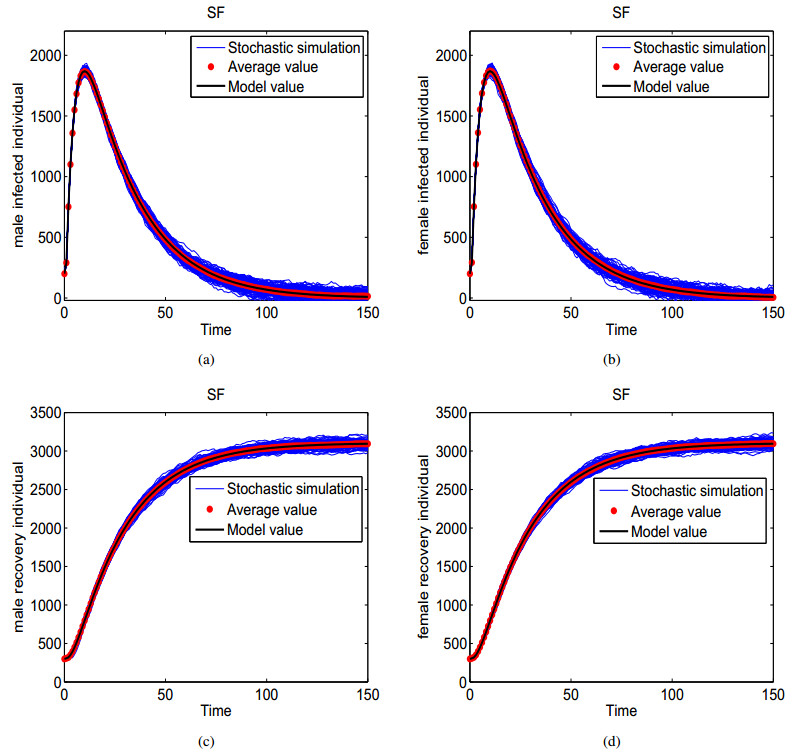
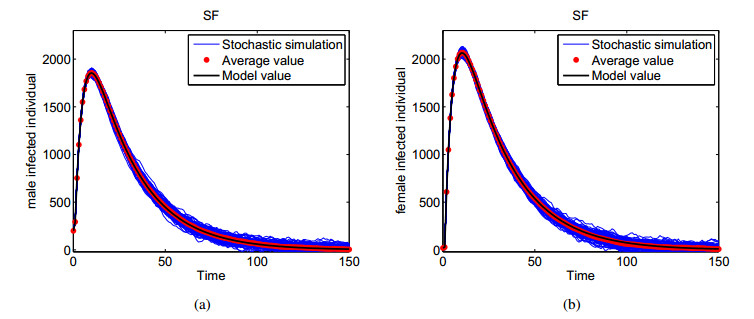
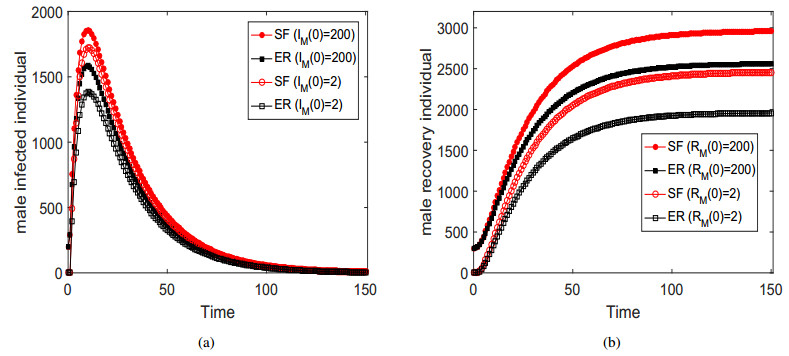
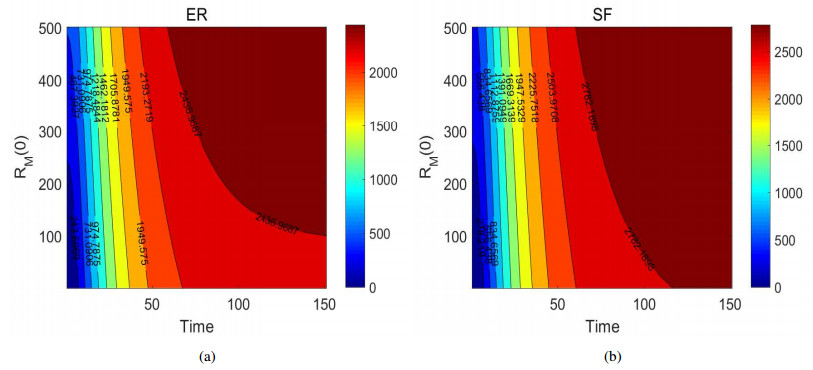
A new edge-based sexually transmitted SEIR model on the contact network is introduced in this paper. The contact infection between the opposite sex and no infectivity during the latent period on bipartite networks are included. The basic reproduction number and the equations of the final size of epidemic are derived. The dynamics of our model with arbitrary initial conditions are further studied. Sensitivity analysis on several parameters and numerical results of the model are derived. We show that the length of the latent period has an effect on arrival time and size of disease peak, but does not affect the final epidemic size and the basic reproduction number of the disease.
1.
Introduction
Inflammatory myofibroblastic tumors (IMT), a rare kind of tumor, is widely accepted as "intermediate malignancy" for its distinct clinical, pathological and molecular features [1,2]. Traditionally, we use a generic term "Inflammatory pseudotumour (IP)" to describe a variety of neoplastic and non-neoplastic lesions including IMT that shares a similar histopathological appearance [1]. It was not until 2002 that the lesion IMT was defined by WHO and described as "a kind of mesenchymal tumor composed of differentiated myofibroblast spindle cells with a prominent, usually plasmocyte and/or lymphocyte infiltration" [3].
IMT used to be classified as a benign tumor [4]. However, evidences showed that chromosomal aberrations involving the anaplastic lymphoma kinase (ALK) gene, which locates on chromosome 2p23, were commonly present [5,6]. In addition, the lesions possessed a more agammaessive biological behavior with local infiltration and recurrence as well as distant metastasis [7,8]. Recently, a majority of literatures were more inclined to classify IMT as an intermediate malignant potential neoplasm.
It has been reported that IMT occurs in virtually all organs/systems, including lung, mesentery, omentum, soft tissue, liver, spleen, pancreas, colon, spermatic cord, prostate, orbit, peripheral and central nerves, in which the most common anatomical sites are lung, mesentery and omentum [1,6,7,9]. According to some literature, children and young adults form the majority of patient population, and the lesions affect females more frequently than males [3,6]. Other literature reveals an inverse male to female ratio.
The best treatments for IMT are still controversial. Some studies suggested that surgical resection, especially complete resection should be the first choice if not prohibited by delicate anatomic location, multiple lesions or complication [1,4,7,8]. Other Studies recommended that adjuvant therapies, including radiotherapy, chemotherapy, immunomodulatory and immunosuppressors such as corticosteroids, were also optimal choices [4,7,8].
In this study, we analyzed 92 real cases extracted from SEER database between 2002 and 2014 and provided a systematic overview on tumor demographical, clinical, biological features as well as treatment efficacy.
2.
Materials and method
The source of patient population data was extracted from the Surveillance, Epidemiology, and End Results (SEER), an authoritative and comprehensive source of population-based information on cancer incidence and survival in the United States. We select all patients diagnosed with IMT by histopathological findings (Histologic Type ICD-O-3 is 8825) between 2002 and 2014 and finally generate 92 confirmed cases.
Patient demographics and case series data were acquired from chart review, including sex, age, race, survival time, tumor primary site, biological behavior, tumor size, distant metastasis and treatment (surgery, radiotherapy and chemotherapy). There was no specific histomorphology and molecular features among the data we obtained, we don't cover these aspects in this paper.
Among these 92 cases, 7 patients (4 male and 3 female) were confirmed dead of more than one malignant tumor including IMT, which meant the correlation between death and IMT was ambiguous. 3 of these patients had a survival time less than 1 month. These 10 cases were excluded in survival analyses.
2.1. Statistical analysis
Statistical analyses were performed using IBM SPSS version 25.0 and the statistical software package R version 3.4.2. Hazard ratios and their 95% confidence intervals (95% CI) were computed. All P values were 2 sided, and P < 0.05 was considered statistically significant.
3.
Results
3.1. Patient characteristics
Characteristics analyses included sex, ethnicity and ages of onset. The concrete data and characteristics of the study cohort were generalized in Table 1. 48.91% were male (45/92) and 51.09% were female patients (47/92). The sex ratio was 0.96:1 (male: female ratio), close to 1. The White race accounted for the highest proportion adding up to 75.00% (69/92), followed by the Black 11.96% (11/92). The average age was 47.4 ± 22.4y (ranged from 0 to 83y). We did a detailed statistical analysis of age and found that around 1/3 of the patients were in a middle age (from 41y to 64y), which was the most common age bracket in IMT tumorigenesis. The young patients (from 18y to 40y) and elderly patients (more than 65y) shared the similar proportion near to 28%. Children and adolescents (no more than 17y) accounted for only 10%, which was controversial to previous reports [1,10,11,12].
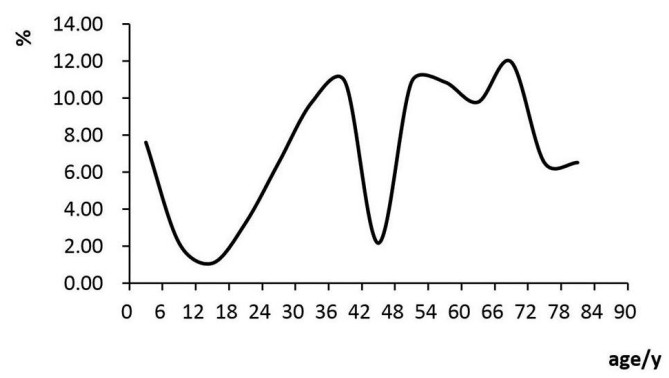
A curve was made according to the age at diagnosis in Figure 1. According to Figure 1, we found that there were three peak age brackets of high IMT incidence, including children around 0-5y, youth around 36-40y and middle-aged and elderly patients more than 50y.
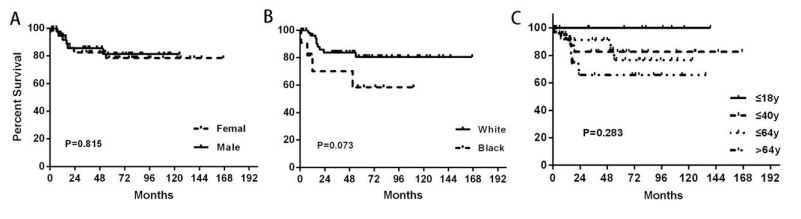
Univariate regression analysis was performed between survival time and the following three variables: sex (male versus female, Figure 2A), ethnicity (White versus Black, Figure 2B) and age of diagnosis (no more than 17y, 18y to 40y, 41y to 64y and more than 65y, Figure 2C). However, we did not find any difference of these variables.
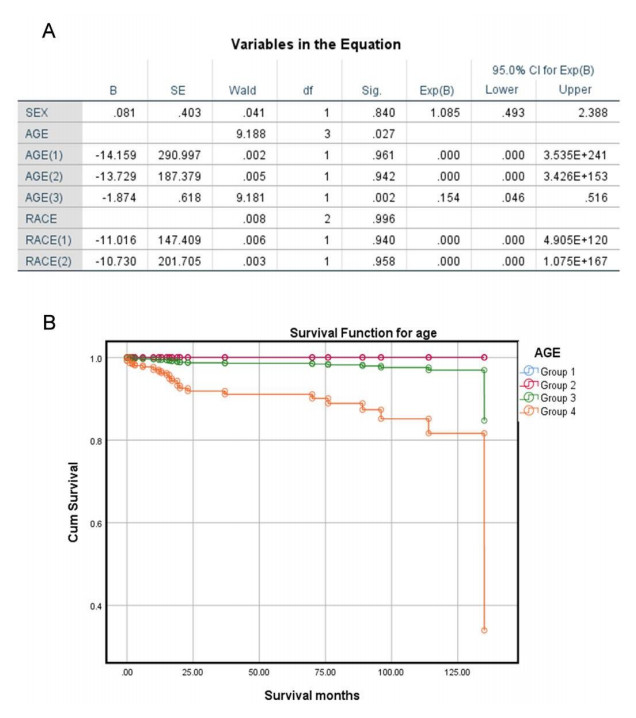
Cox proportional hazards model analysis was used to test for associations with survival and the 3 variables (sex, ethnicity, age). In this model, we adjusted the age as 4 groups: group 1, age < 20; group 2, 20≤age < 40; group 3, 40≤age < 60, group 4, age≥60. We found that age was a predictive factor of survival in this multivariate regression analysis, the p value was 0.027 (Figure 3).
3.2. Clinical characteristics
The anatomical locations of the cohort were summarized in Table 2. The most common anatomical locations were the connective, subcutaneous and other soft tissues of the limbs and hip or shoulder, accounting for about 1/3 in total 92 cases, followed by abdominal and pelvic viscera, such as intestinal tract, pancreas, uterus and retroperitoneum, accounting for approximately 14%. Other predilection sites included nervous system and skeletal system, especially the cerebral meninges, accounting for about 10% and 8%, respectively.
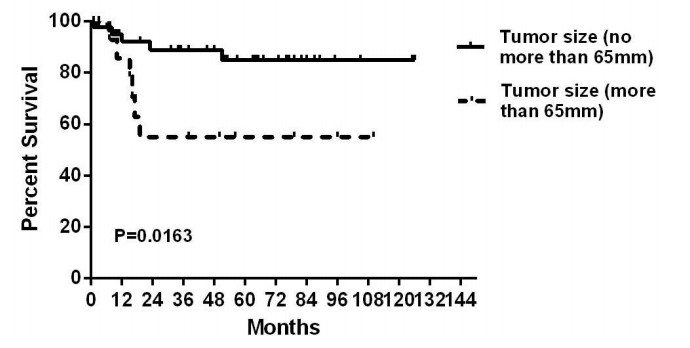
Tumor sizes ranged from 1cm to 25cm and the average was 6.5 ± 5.3cm. Around 2/3 were in the scope of 1.5cm–6.5cm. Survival was better in patients with tumor size no more than 6.5cm compared with those more than 6.5cm (Figure 4) (P < 0.05).
Traditionally, most of IMTs were considered as a benign mass on the basis of its histopathologic nature. However, an almost completely opposite conclusion was made in this study. The majority of IMTs (81/92, 88.04%) were with malignant behavior, compared to 10/92 (10.87%) with malignant potential and 1/92(1.09%) with benign behavior, respectively. However, distant metastases occurred in less 6% patients, indicating that IMTs were not prone to metastases.
3.3. Treatment
There are three articles reporting the correlation between p62 expression and Beclin 1 and LC3. However, there is no correlation between the expression of p62 and these two factors in lung cancer patients (P values in both groups are greater than 0.05; Figure 5).
3.4. Publication bias
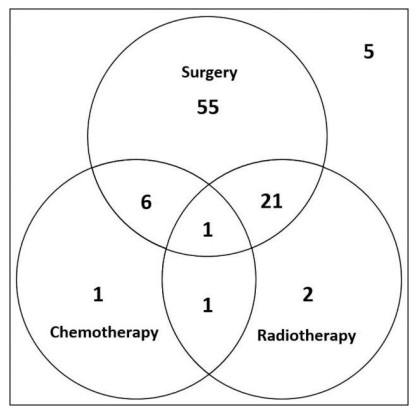
Except for 5 patients, 87 underwent surgery, chemotherapy or radiotherapy. These three main treatments are allocated as the following Figure 5. Surgical excision was the most common treatment method, accounting for 83/92 (90.22%). A total of 55 cases received surgery alone and 28 cases underwent surgery with chemotherapy or radiotherapy. The combination of surgery with radiotherapy included preoperation, intraoperative and postoperation radiotherapy and had 3, 1 and 17 cases, respectively. 9 cases underwent chemotherapy, in which only 1 case received chemotherapy alone. However, there was no substantial evidence to support any of these therapeutic methods separately or by the combined form because of the small case load and difficulties to control variables.
4.
Discussion
IMT was a mesenchymal neoplasm, and was usually recognized as intermediate biological potential, which shows a predilection for the abdominopelvic soft tissues of children and adolescents [1,10,11,12]. However, we drew some different and contradictory conclusions in this study.
We found that middle-aged patients (from 18y to 40y) took up the highest proportion of 1/3, compared to children and adolescents (no more than 17y), which accounted only for 10%. It was previously reported that females were more than males (female: male, 4:3) [2,6], totally different from the cohort in our study (female: male, 1:0.96). Notably, 7 patients were diagnosed as IMT before the age of 5, among whom the primary site of 3 patients was meninges, more frequent than the adult patients. The peak around 0-5 years in fig. 1 indicated that young children might be more susceptible to this malignance. IMT should be taken into account when a child is suffered from meninges disease.
The most common anatomical regions of IMT were different from other studies. In 2003, Janik et al reported 275 cases of children with IMT, in which approximately 1/3 of cases were pulmonary and 2/3 were extrapulmonary [13]. In 2006, Kovach SJ et al reviewed 44 cases with IMT in 2 major academic medical centers over 15 years old in America and found the most common sites were the lung and liver, 20.5% and 11.4%, respectively [7]. We found that connective, subcutaneous and other soft tissues of the limbs, hip or shoulder were the most common sites of involvement, accounting for about 1/3, which had not been shown in the previous studies.
The behavior of IMT was more invasive than what we previously knew. It was commonly recognized that IMT was a borderline biological potential tumor with a small risk of local recurrence and very low metastasis tendency. According to our data of 92 cases, however, most of the IMT (88%) were malignant neoplasms, which demonstrated that IMT might have more invasion ability and risk to metastasize.
Distant metastasis is widely thought to be rare (usually lower than 5%) [1]. In 2007, B C Gleason et al reviewed 21 IMT cases with distant metastasis and found that lung (33%), abdomen or mesentery (29%) and retroperitoneum (14%) were the most common primary sites. Moreover, it metastasized predominantly to lung, brain, liver and bone [1,14]. In our study, 5 cases with local and distant metastasis (5.4%), located in soft tissues of lower limb, thorax, pelvis and bowel. The rate was similar to previous studies.
It is widely accepted that complete surgical resection is the best available and recommended treatment [1,2,7,8]. In this paper, more than 90% cases perform surgery though detailed methods of operation is unknown. The curative effect of chemotherapy is unclear since the case load is too small to provide meaningful evidence. Some papers hold the view that chemotherapy should be based on surgical method, biological behavior and molecular features including chromosomal aberration [1,7]. Radiotherapy is also controversial and may have shown some benefit in some pulmonary IMT cases [4,7,15]. In 2006, Kovach SJ et al reviewed 44 cases with IMT, in which 3 local recurrence (8%) happened in patients with partial resection without chemotherapy or radiotherapy [7]. In 2003, Dishop et al reported a case with malignant transformation and found that no recurrence happened after 2-year follow-up when two partial resections and chemotherapy was performed [16].
5.
Conclusion
In this paper, however, no survival benefit is found among these therapeutic methods. The relationship between treatment and prognosis is still ambiguous and more study is needed to elucidate the best individual treatment in patients with various clinical features, pathological histology and so on.
Taking into together, this study summarized 92 real cases with pathologically confirmed IMT and there were several novel findings. First, most of the IMT (89%) were malignant or malignant potential, though the risk of local and distant metastasis was low (5%). Secondly, three predilection age peaks were 0–4y, 36–40y, more than 50y and the middle-aged group (from 18y to 40y), accounting for the highest proportion of 1/3. Thirdly, the predilection sites were the soft tissues of the limbs, hip, shoulder, head, face and neck, accounting for more than 40%. Last, tumor size and ages were predictive factors of survival time.
Funding sources
The National Natural Science Foundation of Zhejiang Province (LY18H160007) to H.J; The Zhejiang Provincial Science and Technology Project (2015C33172) to J. G; The Zhejiang Provincial Natural Science Foundation of China (Y18H160029) to H.J; the Education of Zhejiang Province (Y201534623) to H.J.
Author contributions
H.J. and X.H. conceived the concept and designed the study; G.F., C.X. and N.Y. performed the experiments; G.F. and J.G. analyzed the data and prepared the figures; G.F., C.X. and X.H. wrote the paper; all authors reviewed the manuscript.
Conflict of interest
The authors declare that they have no conflict of interest.









 DownLoad:
DownLoad: