Dynamics of an epidemic model with advection and free boundaries

1.   Introduction

Memristor, initiated by Chua in 1971, can simulate the human brain ^[1,2,3,4]. Owing to its advantages, it has been introduced into neural networks to elaborate their dynamical behavior ^[5,6,7,9]. In-depth research on the theory and the dynamical behavior gives rise to the inertial neural network (INN), which was discussed in 1986 by introducing inductance into the neural current to represent its inertial characteristics ^[9]. The application of inertial terms in neural networks, which has a strong biological background, not only improves the performance of neural networks in the disorder search, but also serves as an essential method to make the designed neural networks generate chaos and bifurcation behaviors. Additionally, second-order differential equations are employed to detail the dynamical models of the INNs ^[11]. The study on the dynamic behavior of INNs with memristors enjoys practical significance and theoretical value, as previous studies have proved second-order neural networks advantages over first-order counterparts in terms of complex dynamics and biological context ^{[12,13,14,15,16,17]}.

Synchronization refers to the dynamical behavior of a coupled system reaching an identical state simultaneously. The synchronization problems that are critical among the many behaviors vary in forms, such as finite-time synchronization, adaptive synchronization, preassigned-time synchronization, and exponential synchronization. Studies on such problems of INNs have been rich with fruitful results ^{[18,19,20,21,22,23]}, including the finite-time and fixed-time synchronization ^{[24,25,26,27,28,29]}, the quasi-synchronization ^[30], the passivity-based synchronization ^[31], and the exponential synchronization ^[32]. These papers share one commonality; namely, second-order neural networks are usually converted into first-order neural networks, which both enlarge the dimension of the model and complicate the theoretical analysis. Therefore, attempts are made in this paper to address the synchronization problems of INNs based on the direct analysis method.

The complex-valued neural networks (CVNNs) are proposed to generalize the real-valued neural networks (RVNNs). The CVNNs have been proven to outperform the RVNNs regarding computational power and processing speed, which justifies the wide application of the former. Physically speaking, CVNNs and memristors work together to fully use the advantages of memory. Meanwhile, the memristor-based neural networks (MNNs) are more capable of conveying genetic information and more correctly characterizing the physical systems seen in the real world. The study of complex-valued memristor-based neural networks (CVMNNs) is essential for more accurate modeling of dynamic processes based on the aforementioned qualities. A common practice for the study of CVNNs is to split them into two RVNNs, and then discuss them separately afterward ^{[33,34,35,36,37,38]}. However, such a move increases the model's dimension and the computation's difficulty, which naturally leads to exploration concerning the analysis of the synchronization problem of CVNNs, incorporating the non-separation approach grounded in complex functions theory and utilizing appropriate Lyapunov functions. Though the non-separation approach is simpler and more effective, existing papers that utilize the approach to address the synchronization problem of complex-valued INNs are few, which makes the topic more challenging.

The paper aims to elucidate the synchronization of inertial complex-valued memristor-based neural networks (ICVMNNs) with time-varying delays with previous works as reference. The following reveals the features of this paper:

(1) The model proposed in this paper takes into account factors such as memristors and inertial terms. This makes the model considered more versatile and practical.

(2) Compared with existing results, this paper delves into the synchronization problem of ICVMNNs by combining the non-separation method and complex functions theory. This approach complements and extends the synchronization issues observed in first-order CVNNs.

(3) Instead of the reduced-order approach frequently used before, the construction of an improved Lyapunov function is employed in this paper to investigate the synchronization problem of INNs.

(4) The paper considers exponential synchronization, which offers a faster convergence rate. Additionally, it explores the more implementation-friendly adaptive synchronization to ensure practical applicability.

The following explains the framework of this paper. Problems are formulated in Section 2. The exponential synchronization and adaptive synchronization are established in Sections 3 and 4, respectively. Section 5 presents a numerical example, while, Section 6 draws a conclusion.

$\mathbf{Notations}:$ Throughout this paper, $\Lambda = \{1, 2, \cdots, n\}$. Additionally, $\mathbb{R}$, $\mathbb{C}$, and $\mathbb{C}^{n}$ represent the set of real numbers, the set of complex numbers, and the set of $n$-dimensional complex-value vectors, respectively. For $u\in\mathbb{C}$, the norm is defined as $|u| = \sqrt{u\overline{u}}$, where $\overline{u}$ is the conjugate of $u$. For $u = (u_1, u_2, \cdots, u_n)^T\in\mathbb{C}^{n}$, the norm is denoted by $\|u\| = \sqrt{\sum_{l = 1}^n|u_l|^{2}}$.

2.   Problem description

A class of the ICVMNNs with time-varying delays is presented as follows:

where $l\in\Lambda$; $u_{l}(t)\in\mathbb{C}$ is the neural state variable of the $l$th neuron at time $t$; $\dot{u}_{l}(t)$ is the first-order derivative of $u_{l}(t)$; $\ddot{u}_{l}(t)$ is the second-order derivative representing the inertial term of system $(2.1)$. $c_{l} > 0$ and $a_{l} > 0$ are constants; $a_{l}$ denotes the rate at which the $l$th neuron will reset its potential to the resting state in isolation when disconnected from the network and external input; $f_q(\cdot)$ and $g_q(\cdot):\mathbb{C}\rightarrow \mathbb{C}$ are the activation functions; $\tau_{q}(t)$ is the real-valued time delay, which satisfies $0 < \pi_{q}(t) < \bar{\pi} = \max_{q\in\Lambda}\sup_{t\in\mathbb{R}}\{\pi_{q}(t)\}$, and $\pi_{q}'(t) < \hat{\pi} = \max_{q\in\Lambda}\sup_{t\in\mathbb{R}}\{\pi_{q}'(t)\} < 1$; $I_l(t)\in\mathbb{C}$ denotes the input; $\mathfrak{a}_{lq}(\cdot)$ and $\mathfrak{b}_{lq}(\cdot)$ denote real-valued memristive connection weights. According to the characteristics of memristor, in this paper we set

where $\Upsilon_{l} > 0$ is the switching jump, and $\hat{\mathfrak{a}_{lq}}$, $\check{\mathfrak{a}_{lq}}$, $\hat{\mathfrak{b}_{lq}}$, $\check{\mathfrak{b}_{lq}}$ are known constants. For the convenience of calculation, it may be useful to denote $\mathfrak{a}_{lq}^+ = \max\{|\hat{\mathfrak{a}_{lq}}|, |\check{\mathfrak{a}_{lq}}|\}$, $\mathfrak{b}_{lq}^+ = \max\{|\hat{\mathfrak{b}_{lq}}|, |\check{\mathfrak{b}_{lq}}|\}$, $\mathfrak{a}_{lq}^* = |\hat{\mathfrak{a}_{lq}}-\check{\mathfrak{a}_{lq}}|$, $\mathfrak{b}_{lq}^* = |\hat{\mathfrak{b}_{lq}}-\check{\mathfrak{b}_{lq}}|$, $l, q\in\Lambda$.

The initial condition of $(2.1)$ is defined as

where $\varphi_{l}(\cdot)$ and $\hat{\varphi}_{l}(\cdot)$ are bounded continuous functions, $l\in\Lambda$.

The corresponding response system is proposed by the following equation:

where $\omega_{l}(t)\in\mathbb{C}$ is the neural state variable and $W_l(t)$ denotes a controller that will be designed. The meanings of other notations are given the same as that presented in system $(2.1)$. The initial condition of $(2.2)$ is defined as

where $\psi_{l}(s)$ and $\hat{\psi}_{l}(s)$ are bounded continuous functions, $l\in\Lambda$.

Denote $æ_{l}(t) = \omega_{l}(t)-u_{l}(t)$, then

where $\tilde{f}_q(æ_q(t)) = f_q(\omega_{q}(t))-f_q(u_q(t))$ and $\tilde{g}_q(æ_q(t-\pi_{q}(t))) = g_q(\omega_{q}(t-\pi_{q}(t)))-g_q(u_q(t-\pi_{q}(t)))$, $l, q\in\Lambda$.

Definition 2.1. ICVMNNs $(2.1)$ and $(2.2)$ are said to be globally exponentially synchronized if there exist constants $\nu > 0$ and $L > 0$ such that

Assume that the following conditions hold:

$(H_{1})$ functions $f_{q}$ and $g_{q}$ are Lipschitz continuous. That is, there exist constants $F_{q} > 0$, $G_{q} > 0$, such that for all $u$, $\omega\in\mathbb{C}$,

and $|f_{q}(\cdot)|\leq M$, $|g_{q}(\cdot)|\leq N$, where $M$ and $N$ are positive constants, $q\in\Lambda$.

3.   The exponentially synchronization

To implement the exponential synchronization of the ICVMNNs $(2.1)$ and $(2.2)$, we design the controllers as follows:

where $b_{l} > 0$ and $p_{l} > 0$ denote control gains to be determined, $l\in\Lambda$.

Assume that the following conditions hold:

$(H_{2})$ for any $l\in \Lambda$, there exist nonzero constants $\alpha_{l}$, $\beta_{l}$, and $\alpha_{l}\beta_{l} > 0$ and positive constants $\gamma_{l}$, $\nu$ such that

where

Theorem 3.1. Let $(H_1)$ and $(H_2)$ hold, then the systems $(2.1)$ and $(2.2)$ can achieve exponential synchronization under the feedback controller $(3.1)$.

Proof. Consider the Lyapunov functional:

Calculating the derivative of $V(t)$:

By means of the theory of complex functions and $(H_{1})$,

Submit $(3.3)$–$(3.6)$ into $(3.2)$, and we have

Let $\triangle = \{l\in\Lambda:\Theta_l = 0\}$, and from $(H_{2})$, we have $\Pi_l = 0$ for $l\in\triangle$. Meanwhile, note that $\Theta_l\leq0$, $\Psi_l\leq0$, and $\Pi_l^{2}\leq4\Theta_l\Psi_l$, then

which implies that $V(t)\leq V(0)$, $t\geq 0$. Thus, one has

where $\gamma^{-} = \min_{l\in\Lambda}\{\gamma_{l}\}$. The proof is complete. □

Specifically, if $\alpha_{l} = \beta_{l}$ for all $l\in\Lambda$, $(H_{2})$ can be replaced as:

$(H_{3})$ for any $l\in \Lambda$, there exists nonzero constant $\alpha_{l}$ such that the control gains $b_{l}$ and $p_{l}$ in $(3.1)$ satisfy

Therefore, we can draw the following corollary:

Corollary 3.1. If $(H_1)$ and $(H_3)$ hold, the systems $(2.1)$ and $(2.2)$ are exponentially synchronized under the controller (3.1).

The proof is similar to the proof of the Corollary 1 in ^[39], and it is omitted here.

Remark 3.1. By simplifying the parameter settling of $\alpha_{l}$ and $\beta_{l}$, $l\in \Lambda$, Corollary 3.1 can be obtained based on Theorem 3.1, and thus, the conditions in Theorem 3.1 are more flexible and general. Meanwhile, $(H_{3})$ in Corollary 3.1 provides a more explicit gain control scheme that may be more applicable in practical situations.

Remark 3.2. In ^[40], the authors used a non-reduced order approach to study the global dissipativity of inertial RVNNs. Compared with the work, a more general class of inertial CVNNs is considered in this paper.

4.   The adaptive synchronization

From $(H_3)$, exponential synchronization is guaranteed as long as the feedback gains $b_l$ and $p_l$ in $(3.1)$ are large enough. In practice, however, this is not desirable from a cost control perspective. Therefore, the following adaptive control schemes are designed:

where $\lambda_{l} > 0$, $\rho_{l} > 0$, $l\in\Lambda$.

Theorem 4.1. Let $(H_1)$ holds, then the systems $(2.1)$ and $(2.2)$ can achieve adaptive synchronization under the feedback controller $(4.1)$.

Proof. Consider the Lyapunov functional:

where $\tilde{\alpha}_{l} > 0$. Constants $\tilde{b}_l$, $\tilde{p}_l$, and $\tilde{\gamma}_{l} > 0$ will be given later.

Calculating the derivative of $V_{1}(t)$:

Similarly, one has

For $l\in\Lambda$, choose

where $\mu > 0$. From $(4.2)$, it is easy to get that $\tilde{\gamma}_{l} > 0$, then one has

Thus

By virtue of the of Barbalat lemma, it yields

Hence, the dynamics of systems $(2.1)$ and $(2.2)$ are adaptively synchronized. The proof is complete. □

Remark 4.1. In neural networks, time delay is inevitable due to the finite transmission speed and signal propagation time (^[41,42,43]). According to Theorems 3.1 and 4.1, time delays affect the synchronization result.

Remark 4.2. Some results have been made in studying synchronization issues in ICVMNNs (^[44,45]). However, traditional techniques primarily rely on the reduced-order separation method, where the second order is reduced to the first, simultaneously converting the complex-valued neural network into real-valued neural networks. This process results in a doubling of dimensionality and computational effort. In contrast, the approach we adopt in this paper is to design controllers for the original system rather than the reduced-order and separation conversion system. This strategy is considered more practical and highly relevant to real-world applications.

5.   Numerical simulation

Consider the following ICVMNNs with time-varying delays:

and the response system is described as:

where $l$, $q\in\Lambda = \{1, 2\}$, $\pi_{1}(t) = \pi_{2}(t) = \frac{1+\sin 2t}{4}$, $c_1 = 0.8$, $c_2 = 1.5$, $a_1 = 0.8$, $a_2 = 1.2$, $I_{1} = \sin t+i\sin 2t$, $I_{2} = \cos t+i\sin t$, $f_q(\cdot) = g_q(\cdot) = \tanh(Re(\cdot))+i\sin(Im(\cdot))$, and

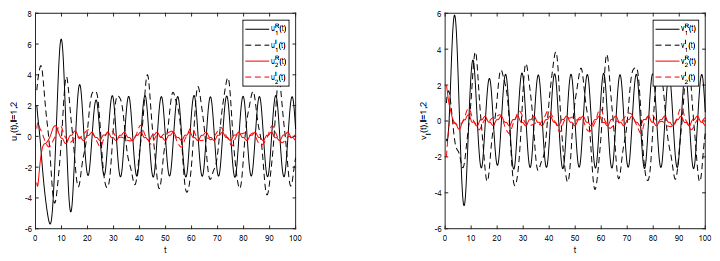
The initial values are selected as $\varphi_{1}(s) = 2+3i$, $\varphi_{2}(s) = -3+0.5i$, $\hat{\varphi}_{1}(s) = \hat{\varphi}_{2}(s) = -2+2i$, $\psi_{1}(s) = -1+i$, $\psi_{2}(s) = 2-2i$, $\hat{\psi}_{1}(s) = \hat{\psi}_{2}(s) = -1+2i$, $s\in[-0.5, 0]$, $l = 1, 2$. The state responses of systems (5.1) and (5.2) are shown in Figure 1. Figure 2 is the phase plot of the state real part and imaginary part of system (5.1). Figure 3 illustrates the evolutions of the synchronization errors without control.

Choose $\alpha_{1} = 1$, $\alpha_{2} = 1.2$. It follows from $(H_3)$ that $b_1 > 9.35$, $b_2 > 11.9$, $p_1 > 2.65$, and $p_2 > 4.2$. From Corollary 3.1, the ICVMNNs (5.1) and (5.2) with the controller (3.1) are exponentially synchronized by the controller gains $b_1 = 9.6$, $b_2 = 12$, $p_1 = 3$, and $p_2 = 4.5$, which is demonstrated by Figure 4.

Furthermore, considering the adaptive control scheme (4.1), let $\lambda_l = 0.3$, $\lambda_2 = 0.5$, $\rho_l = 0.4$, $\rho_2 = 0.6$. By using the Theorem 4.1, the adaptive synchronization is obtained, which is shown in Figure 5. Moreover, the trajectory of the controllers (3.1) and (4.1) are exhibited in Figure 6.

Remark 5.1. The aforementioned example illustrates that while exponential synchronization is quicker, the feedback control parameters needed to achieve it are considerably larger than those required for adaptive synchronization. Hence, adaptive synchronization proves to be more suitable for practical applications.

6.   Conclusions

The synchronization problem of ICVMNNs with time-varying delays is elucidated in the paper, given the practical significance and theoretical value of the dynamic behavior of INNs. A novel controller is developed based on the Lyapunov functions to realize the exponential synchronization of the studied system. An adaptive controller is also designed to accomplish asymptotical synchronization, which is simpler and better for practical engineering applications. The non-separation and nondecreasing order method are adopted in the paper, which has never been seen before. Furthermore, the settling time of fixed-time synchronization is proven to not depend on the system's initial conditions, which is more in line with the requirements in practical applications. However, studies on fixed-time synchronization of ICVMNNs are still rare, requiring further attention to these interesting and challenging issues.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

This work was funded by the National Natural Sciences Foundation of People's Republic of China, No. 12002297.

Conflict of interest

The authors declare there is no conflict of interest.