Effects of quantum noises on χ state-based quantum steganography protocol

1.   Introduction

Quantum information technology applies the basic principles of quantum mechanics to the fields of computation, communication, and cryptography, forming three branches of quantum computing ^[1,2,3], quantum communication ^[4], and quantum cryptography ^[5]. As an emerging frontier interdisciplinary subject, quantum secure communication is developed by the combination of quantum mechanics and information technologies ^[6,7,8,9,10]. As the rapid development of network ^[11,12], it becomes an urgent need for communication security. In 1993, C.H. Bennett proposed the concept of quantum communication. In the same year, a scheme ^[13] for realizing quantum teleportation using the combination of classical and quantum information was proposed. The scheme requires that two parties of the communication first share an EPR pair. The sender measures the single qubit state to be transmitted and his EPR particles under the Bell basis, and transmits the measurement result to the receiver through the classical channel. After that, the receiver can recover the target quantum state. Quantum teleportation is not only important in the field of physics to people's understanding and revealing the mysterious laws of nature, but also can use quantum state as information carrier ^[14,15] to realize the transmission of large-capacity information that cannot be deciphered in principle. Quantum secure communication uses quantum state as information carrier, such as quantum key distribution (QKD) ^[16], quantum secret sharing (QSS) ^[17], and quantum identity verification (QIV) ^[18]. Compared with the classical communication protocol, it shows a better performance in both security and efficiency.

As a member of quantum security communication, quantum information hiding plays a vital role. Based on the design idea of classical information hiding, quantum information hiding realizes the communication of secret message between communicators by establishing hidden quantum channels in quantum public channel. In 2001, Terhal et al. ^[19,20] proposed a quantum information hiding protocol based on the Bell state. The protocol was the first quantum information hiding protocol proposed at that time, which laid the foundation for the development of quantum information hiding technique later.

Quantum steganography is an important sub-discipline of quantum information hiding. The secret message is embedded in an ordinary quantum carrier, and the receiver can decode the secret message after the quantum carrier is transmitted. It can achieve the purpose of secretly transmitting the message. In recent years, a variety of quantum steganography protocols have emerged by using different carrier media ^{[21,22,23,24,25,26,27,28]}. In 2003, Guo et al. ^[29] proposed a steganographic method for encoding secret information into an extended Bell state set by using the uncertainty in the preparation of the Bell state. In 2007, K.Matin ^[30] proposed a new quantum steganography protocol based on the BB84 protocol, which sends secret messages by establishing a hidden channel during the process of key distribution. In 2010, we proposed a protocol for establishing hidden information to convey secret messages in the ordinary information transfer process using Bell state entanglement swapping ^[31]. In the following year, by using the characteristics of the entanglement swapping, we proposed a $\chi$ state based steganographic protocol ^[32], which has better imperceptibility and large capacity. In 2013, Wei et al. ^[33] proposed a quantum steganography protocol for embedding secrets into carrier data using POVM measurements, which has good secrecy and security. In 2015, Wei et al. ^[34] proposed a new quantum image steganography algorithm based on least significant bits. In 2017, Heidari et al. ^[35] proposed a color image steganography scheme based on Gray code. In 2017, we proposed a robust quantum watermarking algorithm based on the polar representation of quantum images ^[36]. In the same year, based on the quantum video-based MCQI representation, we proposed a new large-capacity quantum video steganography protocol ^[37].

Quantum noise has to be taken into consideration in actual communication, as it will seriously disturb a communicating quantum system. For channel noise, the channel coding method is a good way to resist the effects of noise. Channel coding has three types of coding methods: quantum error correcting code, error avoiding code and error proventing code. The quantum error correcting code ^[38] usually uses a specific encoding method, so that the error detection measurement can correctly detect what kind of error was occurred and recover it. The quantum error avoiding code ^[39] achieves the effect of overcoming quantum noise by encoding information in a quantum subspace that is unaffected by noise. The basic idea of quantum error-preventing code is to use the quantum Zeno effect ^[40] to make frequent measurements on the quantum system, so that the quantum system which has been affected by quantum noise can change to initial state after a small change. However, due to the high requirements on hardware facilities, it is still in the theoretical stage. In recent years, some new researches on resistance to quantum noise have emerged ^[41,42]. In 2010, Alexander N. Korotkov et al. ^[43] proposed a scheme to overcome decoherence by using quantum non-collapse measurements, which can effectively preserve quantum states. In 2014, Guan et al. ^[44] analyzed arbitrary two-qubit remote preparation schemes in noisy environments and calculated the effect of quantum noise on protocol efficiency. In 2015, Raphael Fortes et al. ^[45] analyzed the effects of noise on the quantum teleportation process in detail. The conclusion shows that the joint influence of two noises has some symmetry. In 2017, Wang et al. ^[46,47,48] performed quantum noise analysis on quantum remote preparation of single-particle, two-particle and multi-particle, and respectively gave the efficiency of the above protocol in noisy environment. In the same year, we studied the relationship between the effects of noise and the use of quantum channels for several arbitrary two-qubit state remote preparation protocols ^[49]. In addition, for the remote preparation of arbitrary three-qubit state, we analyze the efficiency of the protocol under noise, and propose a scheme to improve the remote preparation efficiency under noisy environment ^[50] by using the natural characteristic of the mutual restraint of the noise channel. In addition to the remote preparation scheme will be affected by noise, the steganographic protocol is also affected by noise and can seriously affect the usefulness of the protocol. Therefore, this paper will carry out noise analysis on the $\chi$ state-based steganography protocol ^[32] proposed in 2011, and further propose an optimization scheme, which makes it have better practicability in the real environment.

The rest of this paper consists of four parts. In Section 2, four kinds of quantum noises are introduced briefly, which are the four most possible quantum noises encountered in quantum channels. In Section 3, a brief review of the steganographic protocol based on the $\chi$ state is given. In Section 4, the noise environment that the steganographic protocol may be subjected to is analyzed, and the fidelity of information is calculated for all possible cases. The efficiency of the protocol is analyzed and the optimization scheme under noise is given. This article will be discussed and summarized in Section 5.

2.   Quantum noise channels

This section describes the four common types of quantum noises in quantum channels, namely amplitude damping, phase damping, bit flip, and depolarizing noises. Quantum noise can be regarded as one specific quantum operation, so that it can be described by the form of operator sum.

2.1.   Amplitude damping channel

Amplitude damping describes the dissipation process of energy, that is, the effect that energy is lost from the quantum system. The operands for amplitude damping are described below.

In which, the noise coefficient $p$ denotes the probability that a quantum system loses a photon.

2.2.   Phase damping channel

Phase damping is described as the interfere to quantum information without energy dissipation, often referred to as the "T2" (or spin-spin) relaxation process, the operator-sum formalism can be expressed as

where the noise coefficient $p$ can be understood as the probability of scattering from a photon without energy loss from the system, ${\sigma _z}$ is Pauli matrix.

2.3.   Bit flip channel

Bit flip channel flips the qubit state with a probability $p$ from $\left| 0 \right\rangle$ to $\left| {\rm{1}} \right\rangle$ (or vice versa), and the operands of its quantum operation are expressed as

where $p$ is noise coefficient, ${\sigma _x}$ is Pauli matrix.

2.4.   Depolarizing channel

Another noisy channel is a kind of important quantum noise. The depolarizing channel depolarizes the qubit with a probability of $p$, i, e, makes it becoming a maximum mixed state $I/2$. The operands of the depolarized channel can be expressed as

where $I, {\sigma _x}, {\sigma _y}, {\sigma _z}$ are four Pauli matrices.

3.   The steganography protocol based on $\chi$ state

In the original protocol ^[32], the sender Alice wanted to send a classical information sequence to Bob while carrying 4 bits of secret information. The specific steps of the protocol are as follows.

S1) The sender Alice and the receiver Bob know in advance how to encode the four Pauli operators, that is

S2) Alice prepares a series of entangled four-particle $\chi$ state, and the particles of the quantum states sequence is expressed as $\left[{P_1^1, P_2^1, P_3^1, P_4^1, P_1^2, P_2^2, ..., P_3^n, P_4^n} \right]$, the superscript indicates the sequence number of the nth entangled particle pair, and the subscript indicates the four particles of each $\chi$ state. All entangled particles are initially in the ${\left| {{\chi _{00}}} \right\rangle _{3214}}$ state. The $i$th particle of each entangled pair constitutes the sequence ${C_i} = \left[{P_i^1, P_i^2, ..., P_i^n} \right]$, Alice keeps particle sequence $C_1$ and $C_3$, sends the sequence $C_2$ and $C_4$ to Bob.

S3) Bob randomly selects the particles in the corresponding position from $C_2$ and $C_4$ to start the eavesdropping detection by measuring the particles under BM1 or BM2 basis. He will tell Alice the position and measurement basis of the particles through the classical channel. Then, Alice measures her particle at the corresponding position and at the corresponding basis AM1 or AM2. Finally, Alice and Bob public the measurement results to detect eavesdropping. Since the basis in this step is not used in our work, the corresponding measurement basis is not listed here.

S4) According to the information bit sequence, Alice performs corresponding unitary operation on her particles. Suppose Alice wants to pass the information ${i_1}{i_2}{i_3}{i_4}$, she will perform $\sigma _{\rm{3}}^{2{i_1} + {i_2}}$ and $\sigma _{\rm{1}}^{2{i_{\rm{3}}} +{i_{\rm{4}}}}$ on particle 3 and 1 to encode the information and then send the encoded particles to Bob. After receiving the particles, Bob measures the particle group $\left[{P_3^i, P_1^i, P_2^i, P_4^i} \right]$ under the FMB basis ($\chi$ state orthogonal basis) to decode information. In this step, Alice can choose whether to enter the secret information hiding mode S5).

S5) Secret information hiding mode: (a) Alice selects the location m of the hidden channel according to the secret information, and the secret information is in one-to-one correspondence with the entangled exchange result set of $\left| {{\chi ^{ij}}} \right\rangle _{3214}^{m - 1}$ and $\left| {{\chi ^{pq}}} \right\rangle _{3214}^m$, $m$ can be sent to Bob through a classical channel. (b) Alice copies the information carried by $\left| {{\chi ^{ij}}} \right\rangle _{3214}^{m - 1}$ to $\left| {{\chi ^{ij}}} \right\rangle _{3214}^{m + 1}$ by performing the same unitary operator $\sigma _{\rm{3}}^i\sigma _1^j$ on $P_3^{m + 1}P_1^{m + 1}$. Then $\left| {{\chi ^{ij}}} \right\rangle _{3214}^{m + 1}$ just as an auxiliary particle to hide the secret and no longer transmits classic messages. (c) Alice achieves entanglement swapping by exchanging the particles $\left[{{\rm{1}}, {\rm{4}}} \right]$ and $\left[{{\rm{1'}}, 4'} \right]$ of $\left| {{\chi ^{ij}}} \right\rangle _{3214}^m$ and $\left| {{\chi ^{ij}}} \right\rangle _{3'2'1'4'}^{m + 1}$.

S6) Secret decoding mode: (a) Bob first receives the $m$ sent by Alice. (b) He measures particle groups $\left[{P_3^m, P_2^m, P_1^m, P_4^m} \right]$ and $\left[{P_3^{m + 1}, P_2^{m + 1}, P_1^{m + 1}, P_4^{m + 1}} \right]$ respectively under the CMB basis (cat state measurement basis). (c) Based on the measurement results, Bob can decode the secret message sent by Alice.

Two types of messages are sent in the original protocol, one is a normal information sequence, and the other is a 4-bit secret message. Next, we will start to analyze the impact of these two types of messages after being affected by noise.

4.   The steganography protocol under noisy channels

From the previous section, it's easy to know that the transmitted information and secret information will be affected by quantum channel noise in the steganographic protocol. In this section, we will analyze the different noise effects on these two types of messages in detail.

4.1.   Effect on transmitted information

In order to facilitate the analysis, we will study the effect of noise on a single $\chi$ state that carries transmitted message.

In the step S2, Alice sends the particle sequences C2 and C4 to Bob. During the transmission process, each of the 2, 4 particles will be affected by the quantum noise channel. The initial quantum system can be written as

After the transmission is completed, the quantum system shared by Alice and Bob is affected by the quantum noise becomes

Among them, the superscript represents the quantum particle affected by the noise operator. For example, $E_j^2$ represents the noise operator acting on particle numbered 2 in the χ state. After the eavesdropping detection step S3, Alice performs corresponding unitary operations $\sigma _{\rm{3}}^{2{i_1} + {i_2}}$ and $\sigma _{\rm{1}}^{2{i_{\rm{3}}} + {i_{\rm{4}}}}$ on the 1, 3 particles according to the transmission information, and the encoded quantum system becomes

Alice then sends the particles 1, 3 to Bob via the quantum noise channel. After the transfer is completed, the quantum system composed of the particles owned by Bob becomes

while the state that Bob should obtain theoretically is

Fidelity can describe the distance between two quantum states, so fidelity can be used to describe the effect of noise on quantum states. Fidelity is defined as follows

The values of fidelity range from 0 to 1. The greater the fidelity, the more similar the two quantum states are. A fidelity of 1 indicates that the two quantum states are identical to each other.

For ease of analysis, it is supposed that the classic information transmitted is $0000$, then the unitary operation $\sigma _{\rm{3}}^{2{i_1} + {i_2}}\sigma _{\rm{1}}^{2{i_{\rm{3}}} + {i_{\rm{4}}}}$ Alice performs is $\sigma _{\rm{3}}^0\sigma _{\rm{1}}^0$.

Considering that Alice's first transmission quantum channel is affected by quantum noise (noise factor ${p_A} \ne 0$) and the second transmission quantum channel is not affected by noise (noise factor ${p_B}{\rm{ = }}0$). Thus, under the four noisy channels, the fidelity of the transmitted information is

${F_{\left({X, Y} \right)}}$ indicates the fidelity of the information in the case that Alice's first transmission quantum channel is subjected to $X\left({AD, Phs, BF, D} \right)$ noise and her second transmission quantum channel is subjected to $Y\left({AD, Phs, BF, D, \emptyset } \right)$ noise.

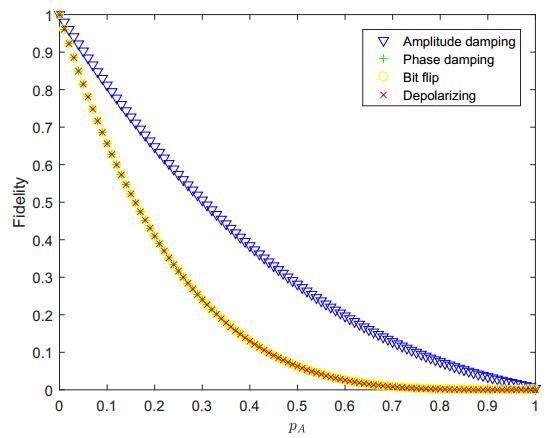
From the calculation results, we can see that phase damping, bit flip and depolarizing noise have the same effect on the transmitted information. In Figure 1, we plot the equations 4.7 to 4.10 for the fidelity as a function of the noise factor $p_A$. It can be seen that the amplitude damping noise has less influence on the transmitted information than the other three kinds of quantum noises. As the quantum noise factor $p_A$ increases, the fidelity of the information transmitted under the four kinds of noises respectively gradually decreases. When $p_A$ increases to 1, each fidelity under phase damping, bit flip or depolarizing noise is reduced to zero, the fidelity at amplitude damping reaches a minimum of 0.0625.

Next, we analyze the situation when both Alice's first and second transmission quantum channels are disturbed by quantum noise (${p_A} \ne 0$, ${p_B} \ne 0$).

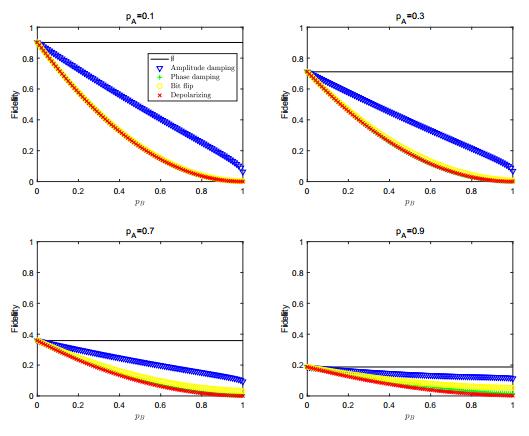
At first, let assume that Alice's first transmission quantum channel is subject to amplitude damping noise and her second transmission quantum channel is affected by four different quantum noises. The fidelities of the system in these cases are

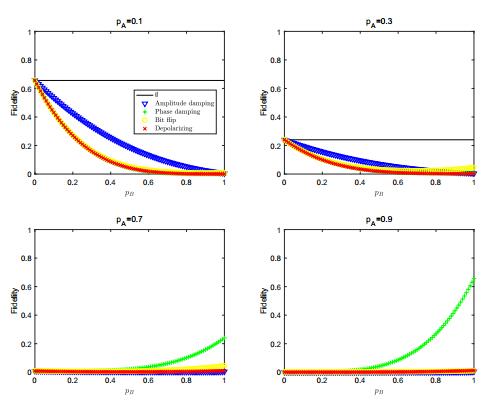
Figure 2 shows the variation of fidelities with noise factor ${p_B}$ in the four cases. For ease of analysis, only the images that the value of ${p_A}$ is 0.1, 0.3, 0.7, and 0.9 respectively are listed here. Through the calculation results, we found that when ${p_B}$ is less than about 0.6, as the ${p_A}$ value increases, the fidelity of the transmitted information gradually decreases. If ${p_B}$ is greater than about 0.6, with the increases of ${p_A}$ the fidelity of the transmission information will go down first and then increase, while the Alice's second transmission channel is subjected to phase damping noise, this change is especially obvious when ${p_B} = 1$. With the value of ${p_A}$ grows, ${F_{\left({AD, AD} \right)}}$ increases from 0.0625 to 0.1250, ${F_{\left({AD, Phs} \right)}}$ increases from 0 to 0.0625, ${F_{\left({AD, BF} \right)}}$ increases from 0 to 0.0625, ${F_{\left({AD, D} \right)}}$ increases from 0 to 0.0069. Although this change is very small, we can see that there is a kind of mutual restraint effect after the combination of quantum noise. And when ${p_A}$ is small (${p_A} \le 0.3$), the effects of phase damping, bit flip and depolarizing noise are almost the same. With the increase of ${p_A}$, the influence of bit flip noise is gradually less than phase damping, and the effect of phase flip noise is less than depolarizing noise. In addition, in this case, both Alice's first and second transmission channels are subject to noise fidelity less than if only Alice's first transmission channel is subject to noise.

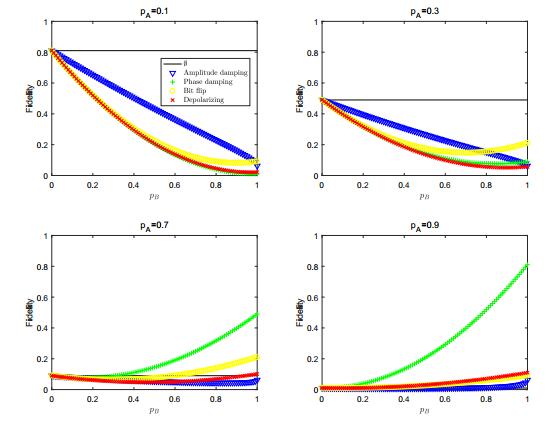
The other case is that Alice's first transmission quantum channel is subjected to phase damping and her second transmission quantum channel is affected by four different quantum noises. In this case, the fidelity of the system is

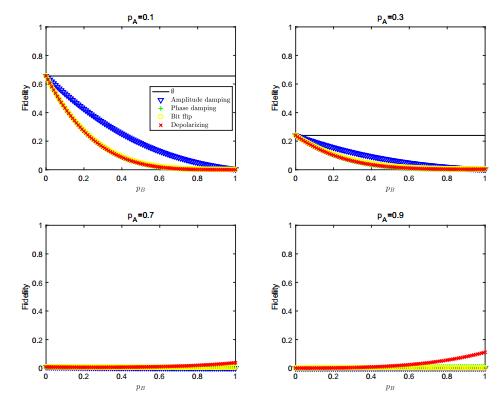
In this case, the fidelity as a function of the noise factor is shown in Figure 3. When ${p_B} = 0$, the fidelity of all the four quantum noises decreases with the increase of ${p_A}$. When ${p_A}$ is small, the influence of phase damping on the transmitted information is smaller than that of the other three kinds of quantum noises. With the increase of ${p_B}$, the fidelity under bit flip noise is gradually greater than the fidelity under amplitude damping. As the ${p_A}$ increases, the fidelity of the phase damping noise will gradually increase. When ${p_A}{\rm{ = 0}}{\rm{.5}}$, the fidelity of the phase damping noise will be equal to the fidelity under the bit flip. At the same time, the phase damping and bit flip noise will be the same when ${p_A}{\rm{ = 0}}{\rm{.5, }}{p_B}{\rm{ = 1}}$ and ${p_A}{\rm{ = 0}}{\rm{.5, }}{p_B}{\rm{ = 0}}$. As ${p_A}$ continues to increase, the fidelity of phase damping noise will gradually exceed the bit flip noise, and then the black solid line which represents that only Alice's channel is subject to noise. This means that we can increase the fidelity of the transmitted information by using the appropriate ${p_B}$ according to the value of ${p_A}$. When $p_A = 1$, ${p_B}$ is the intensity factor of the phase damping noise and the $p_B$ reach the value of 1 can maximize the fidelity of the transmitted information. This means that, in this case, the phase damping can be completely offset by the phase damping noise.

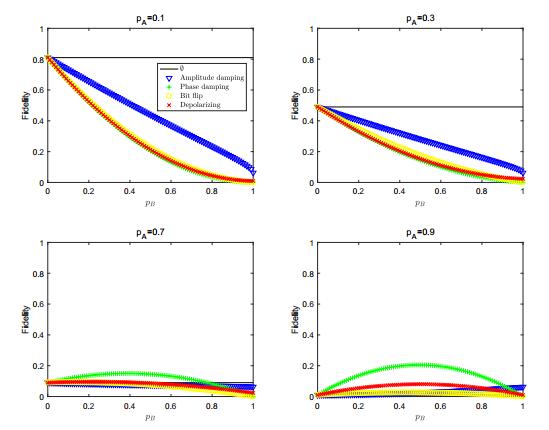
Next, Alice's first transmission quantum channel is affected by bit flip and the second transmission quantum channel is affected by four different quantum noises. The fidelity of quantum systems in these four cases is

In this case, the fidelities of the transmitted information under the four kinds of noises vary with ${p_A}$ and ${p_B}$ are shown in Figure 4. When ${p_A}$ is small, the fidelity under amplitude damping noise is greater than that of the other three quantum channels. As the ${p_A}$ increases, the fidelity under phase damping noise is gradually greater than that of the other three quantum noises. When ${p_A} \ge {\rm{0}}{\rm{.6}}$, the fidelity under phase damping will gradually be greater than that of noise-free in the second transmission channel. And in this case, the maximum fidelity is taken at ${p_A}{\rm{ = 1, }}{p_B}{\rm{ = 0}}{\rm{.5}}$, which is 0.25. It can be found that adding extra noise can increase the fidelity of the system when ${p_A}$ is large. When ${p_A}$ is small, the reduction effect is not obvious.

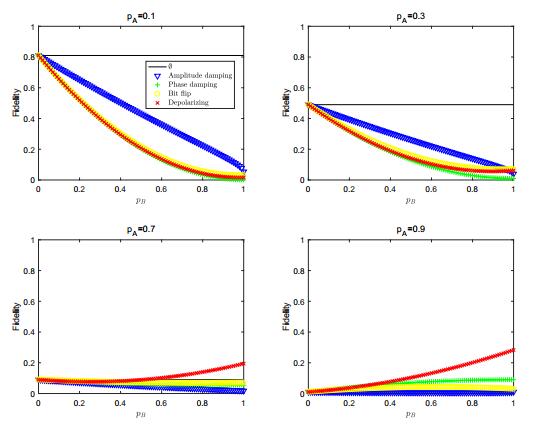
In another case, Alice's first transmission quantum channel is subject to the depolarizing noise, and the second transmission quantum channel is subject to four different quantum noises. The fidelity of the system is

Figure 5 plots the fidelities vary with $p_A$ and $p_B$ under the situation that Alice's first channel under depolarizing and her second channel under four different quantum noises. Similarly, when $p_A$ is small, the effect of amplitude damping is also small. As the $p_A$ increases, the fidelity that the second transmission channel under depolarizing noise gradually increases. When ${p_A} \approx 0.6$, the fidelity of the information that the second channel under depolarizing noise is gradually greater than that of noise-free channel. When ${p_A} \ge 0.6$, the maximum fidelity can be obtained at the situation that ${p_A}{\rm{ = 1, }}{p_B}{\rm{ = 1}}$, and the maximum fidelity was 0.33.

In addition, according to the equations 4.11 to 4.26, it can be found that the influence of quantum noise on the transmitted information has excellent symmetry. That is ${F_{\left({M, N} \right)}} = {F_{\left({N, M} \right)}}$ $\left({M, N \in \left\{ {AD, Phs, BF, D} \right\}} \right.$ $\cap \left. {M \ne N} \right)$. This symmetry effectively simplifies our analysis of the effects of noise and the connection between different noises.

4.2.   Effect on secret information

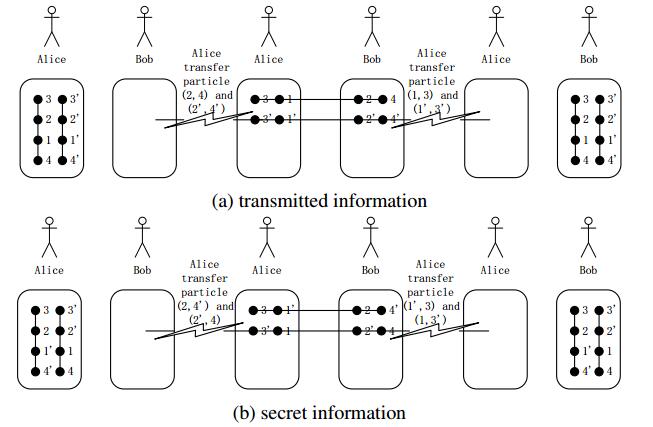
This section will analyze the impact of quantum noise on secret information. According to the protocol reviewed in Section 3, we know that the secret information is based on the transmission information carrier, two $\chi$ states. And the secret information is encoded by the characteristics of the transmitted information carriers, which is essentially the transmission of information carrier. It should be noted that Alice performs an entanglement swapping on the two $\chi$ states which carries the secret information. Taking the two $\chi$ states that is encoded secret information as an example, transmission process of the normal transmission information and the transmission process of secret information are shown in Figure 6.

As can be seen from Figure 6, when the secret information is transmitted, although the entanglement swapping operation is performed, the particles transmitted actually are exactly the same as the particles that carry transmission information. This means that the effect of quantum noise on the two $\chi$ states of secret information is the same as the effect of the same two $\chi$ states of ordinary transmission information. Therefore, the influence of quantum noise on secret information is the same as that of transmitted information, and has the same conclusion on how to improve the fidelity under noise.

To be specific, it's supposed that the secret message is $0000$, there are 16 possible two $\chi$ states combinations for Alice to choose. For the convenience of studying, assuming Alice chooses $\left| {{\chi ^{00}}} \right\rangle \otimes \left| {{\chi ^{00}}} \right\rangle$, the quantum system contains secret message will be

Alice performs the entanglement swapping by exchanging the particles $\left[{1, 4} \right]$ and $\left[{1', 4'} \right]$, then the system will become

Alice transmits the particles $\left[{2, 4'} \right]$ and $\left[{2', 4} \right]$ to Bob. It's supposed that Alice's every transmission using the same quantum channel, which means that the four particles will suffer the same quantum noise in the noisy channel. After the transmission is completed, the quantum system will be

Then Alice sends the particles $\left[{3, 1'} \right]$ and $\left[{3', 1} \right]$ to Bob, the quantum system that Bob receives is

Bob measures the particles $\left[{3, 1', 2, 4'} \right]$ and $\left[{3', 1, 2', 4} \right]$ on the CMB basis to deduce the two $\chi$ states which performed entanglement swapping, then Bob can decode the secret message. Let measure the effect of noise on the secret message by calculating the fidelity of quantum system Bob finally received. The fidelity can be defined as

in which,

A simple calculation shows that the fidelity of secret information is the square of the fidelity of an ordinary information.

Then, in the situation that Alice's first transmission suffers four types of noises (${p_A} \ne 0$) and her second transmission is not affected by noise (${p_B} = 0$), the fidelities are as follows, respectively.

The curves of fidelity as a function of noise factor are plotted in Figure 7. We can see that the effects of noise on secret message are similar to that on ordinary information, the difference is that the fidelity of a single secret message is lower than an ordinary information. The other possible situations will not be analyzed in detail due to it has the same conclusion as the ordinary information, the fidelity curves will be given in Supplementary.

5.   Conclusions

This paper analyzes the performance of a steganographic protocol based on the $\chi$ state under quantum noise. When Alice's first transmission channel is affected by quantum noise, and the second transmission channel is not affected by the quantum noise, the performance of the protocol under amplitude damping noise is better than that of the other three kinds of quantum noises. When Alice's first channel is affected by quantum noise and her second quantum channel is also affected by quantum noise, if Alice can adjust the corresponding second noise channel according to her first transmission channel noise intensity, then she can select the appropriate noise channel, so that the fidelity of the transmitted information can increase under the quantum noise, and the anti-noise performance of the protocol is improved. Specifically, when Alice's first channel is subjected to phase damping, bit flip, and depolarization noise, she can increase the fidelity of the transmitted information by adjusting the coefficient of the noise. When Alice's first channel is affected by amplitude damping noise, this method cannot be used to improve the performance of the protocol. Under some certain conditions, the fidelity of information under phase damping and bit flip can be increased by adding phase damping noise. The fidelity of information under depolarizing can be increased by adding depolarizing noise. The method proposed in this paper has a better improvement effect on strong noises especially. Therefore, when the protocol needs to work in a strong noise environment, the method proposed in this paper can be a good option to improve the performance of the protocol.

Acknowledgments

This work was supported by the National Natural Science Foundation of China (No. 61373131, 61601358, 61501247, 61672290, 61303039, 61232016), the Six Talent Peaks Project of Jiangsu Province (Grant No. 2015-XXRJ-013), Natural Science Foundation of Jiangsu Province (Grant No. BK20171458), the Natural Science Foundation of the Higher Education Institutions of Jiangsu Province (China under Grant No.16KJB520030), Sichuan Youth Science and Technique Foundation (No.2017JQ0048), NUIST Research Foundation for Talented Scholars (2015r014), PAPD and CICAEET funds.

Conflict of interest

The authors declare that there are no conflicts of interest.

Supplementary