1.
Introduction
Given a r-tuple \bmα=(α1,α2,…,αr) of positive integers with αr≥2, a multiple zeta value ζ(\bmα) (MZV) is defined to be [6,8,9]
We let {a}k be k repetitions of a such that ζ({1}3,3)=ζ(1,1,1,3) and ζ(2,{3}2,5)=ζ(2,3,3,5).
The generalized harmonic numbers are defined by
where s and n are positive integers. In particular, H(1)n=Hn is the classical harmonic number. The famous formula
was discovered by Euler. Series incorporating harmonic numbers find application in various mathematical disciplines and related fields [1,2,5]. It is well-known that binomial coefficients play a crucial role in various subjects, such as combinatorics, graph theory, number theory, and probability.
Sofo [16,17] discovered closed-form representations for sums involving harmonic numbers with reciprocal binomial coefficients of various forms:
In the literature, numerous papers explore infinite sums involving reciprocals of binomial and harmonic numbers [1,13,15,18]. Recently, Chu [7] investigated double series of the following forms:
Additionally, the first author [4] developed q-analogues of such series. It prompts us to explore this general type of series further. This paper focuses on determining the value of an infinite series given by the expression:
This series is evaluated as a finite sum of MZVs, with the parameters being positive integers a1,…,an,b1,…,bn,c1,…,ck. For example,
where a,b are positive integers. It is noted that for our convenience, we denote ζ(a1,…,an,{1}−1,c) as ζ(a1,…,an+c−1). Therefore, we have
In the next section, we introduce the algebraic structure for MZVs, as originally proposed by Hoffman [10]. Furthermore, we present a combinatorial generalization of the iterated integral associated with a 2-poset, represented by a Hasse diagram. These integrals are referred to as Yamamoto's integrals [19,20]. In Section 3, for given positive integers n and a1,a2,…,an, we determine the value of
in terms of MZVs. Then, in Section 4, we express the value of
using MZVs, where n and a1,…,an,b1,…,bn are positive integers. Finally, we present the conclusion of our main results in Section 5, along with additional concrete examples. For example,
2.
Algebraic settings and integrals associated with 2-posets
We review the algebraic setup of MZVs introduced by Hoffman [10]. Let Q⟨x,y⟩ be the Q-algebra of polynomials in two noncommutative variables which is graded by the degree (where each of the variables x and y is assumed to be of degree 1); we identify the algebra Q⟨x,y⟩ with the graded Q-vector space H spanned by the monomials in the variables x and y.
We also introduce the graded Q-vector spaces H0⊂H1⊂H by H1=Q1⨁xH, H0=Q1⨁xHy, where 1 denotes the unit (the empty word of weight 0 and length 0) of the algebra H. A word starts with x and ends with y, and we refer to such words as "admissible." In other words, the subalgebra H0 is generated by admissibile words. Let Z:H0→R be the Q-linear map that assigns to each word u1u2⋯uk in H0, where ui∈{x,y}, the multiple integral
Here, wx(t)=dt/(1−t), wy(t)=dt/t. As the word u1u2⋯uk is in H0, we always have wu1(t)=dt/(1−t) and wuk(t)=dt/t, so the integral converges. The space H1 can be regarded as the subalgebra of Q⟨x,y⟩ generated by the words zs=xys−1 (s=1,2,3,…).
Let us define the bilinear product Ш (the shuffle product) on H by the rules
for any word w, and
for any words w1, w2, any letters xi=x or y (i=1,2), and then extend the above rules to the whole algebra H and the whole subalgebra H1 by linearity. It is known that each of the above products is commutative and associative. We denote the algebras (H1,+,Ш) by H1Ш. By the standard shuffle product identity of iterated integrals, the evaluation map Z is again an algebra homomorphism for the multiplication Ш [11]:
Yamamoto [19] introduced a combinatorial generalization of the iterated integral, the integral associated with a 2-poset. We review the definitions and basic properties of 2-labeled posets (we will call them 2-posets for short in this paper) and the associated integrals first introduced by Yamamoto [19].
Definition 2.1. [12,Definition 3.1] A 2-poset is a pair (X,δX), where X=(X,≤) is a finite partially ordered set (poset for short) and δX is a map from X to {0,1}. We often omit δX and simply say "a 2-poset X." The δX is called the label map of X.
A 2-poset (X,δX) is called admissible if δX(x)=0 for all maximal elements x∈X and δX(x)=1 for all minimal elements x∈X.
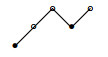
A 2-poset is depicted as a Hasse diagram in which an element x with δ(x)=0 (resp. δ(x)=1) is represented by ∘ (resp. ∙). For example, the diagram
represents the 2-poset X={x1,x2,x3,x4,x5} with order x1<x2<x3>x4<x5 and label (δX(x1),…,δX(x5))=(1,0,0,1,0).
Definition 2.2. [12,Definition 3.2] For an admissible 2-poset X, we define the associated integral
where
Note that the admissibility of a 2-poset corresponds to the convergence of the associated integral. We also recall an algebraic setup for 2-posets (cf. Remark at the end of §2 of [19]). Let P be the Q-algebra generated by the isomorphism classes of 2-posets, whose multiplication is given by the disjoint union of 2-posets. Then, the integral (2.5) defines a Q-algebra homomorphism I:P0→R from the subalgebra P0 of P generated by the classes of admissible 2-posets. We refer to this type of integral as Yamamoto's integral.
It is known that [20] there is a Q-linear map
which transforms a 2-poset into a finite sum of words in x and y. This transformation is characterized by the following two conditions: The first condition states that for a totally ordered X=x1<x2<⋯<xk, W(X)=zδ(x1)zδ(x2)⋯zδ(xk), and the second condition asserts that if a and b are noncomparable in X, then W(X) can be expressed as W(Xba)+W(Xab), where Xba represents the 2-poset obtained from X by adjoining a new relation a<b. This W sends P0 onto H0 and satisfies I=Z∘W:P0→R.
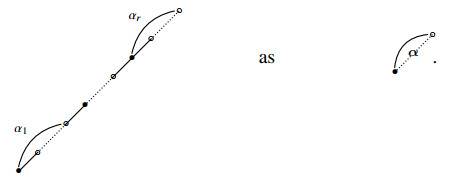
Here, we present three known examples in [19,20]: For an index \bmα=(α1,…,αr) (admissible or not), we write the 'totally ordered' diagram:
For an index \bmβ=(β1,β2,…,βm), we write the following diagram:
Then, if \bmα and \bmβ are admissible, we have [20,Propositions 2.4 and 2.7]
For example,
The last example is the MZV of Mordell-Tornheim type which is defined by the series
They have the following integral form [20,Proposition 2.8]
3.
Series with reciprocal of binomial coefficients
We first address the basic form of double series, employing primarily the approach of evaluating Yamamoto's integral using both integral and series modes separately.
Theorem 3.1. For integers a≥0 and b≥0, we have
Proof. Using Eq (2.7), we know that
On the other hand, we evaluate this Yamamoto's integral as follows.
We treat this integral as
where
We represent A(s1) and B(s1) as a power series:
Therefore,
Since the integral in the righthand side is the beta function, we obtain
Combing Eq (3.2), we conclude our result. □
Next, we generalize the result stated in the theorem above to a more general form.
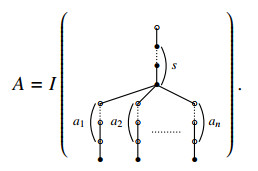
Theorem 3.2. Given n+1 nonnegative integers a1,a2,…,an and s with ∑ni=1ai=w, we have
where Sn is the symmetric group of n objects and σa is induced permutations of σ∈Sn on the set {a1,a2,…,an}.
Proof. Consider the following Yamamoto's integral
We use the map W which is defined in Eq (2.6) to transform the n legs in the 2-poset as (xya1,xya2,…,xyan). Since (see [3,Eq (4.6)])
where Sn is the symmetric group of n objects and σa is induced permutations of σ∈Sn on the set {a1,a2,…,an}. Then, we shuffle them together as a totally ordered diagram, and by Eq (2.7) we have
On the other hand, we write the Yamamoto's integral as follows.
where
We expand them into a power series:
Therefore,
Given that the integral on the righthand side is the beta function, we deduce that
Combining the two expressions for A given by Eqs (3.4) and (3.5), we obtain the desired formula. □
4.
Series involving harmonic numbers
In this section, we begin by computing the double series form where the numerator involves harmonic numbers.
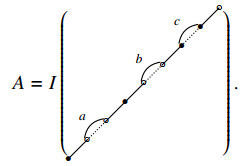
Theorem 4.1. Given three nonnegative integers a,b,c, we have
Proof. Consider the following Yamamoto's integral:
Using Eq (2.7), we know that
On the other hand, we evaluate this Yamamoto's integral as follows.
where Δ is a simplex in [0,1]a+b+c+3 with
We treat this integral as
where
We represent B(w1) and C(w1) as a power series:
Therefore,
Since the integral on the righthand side is the beta function, and by combining it with Eq (4.2), we arrive at our result. □ Next, we extend this to multiple series and sums where the numerator involves additional harmonic numbers. However, before proceeding further, we will introduce some basic concepts regarding the shuffle product identities that we will utilize.
The shuffle product can be described combinatorially. Utilizing the definition of the shuffle product Ш in H, we readily obtain
where ui∈{x,y} are letters, and
Therefore,
where C(d1,…,d2n) is a suitable constant depending on the variables d1,…,d2n.
Some explicit formulas can be found in [14].
Here, we express a formula of xyaxybШxycxyd as follows (ref. [14,Eq (2.11)]).
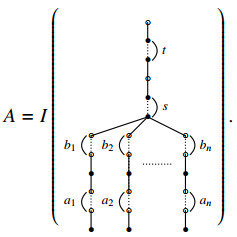
Theorem 4.2. Given 2n+2 nonnegative integers a1,…,an,b1,…,bn, and s,t with ∑ni=1ai+bi=w, we have
where C(d1,…,d2n) is defined in Eq (4.3).
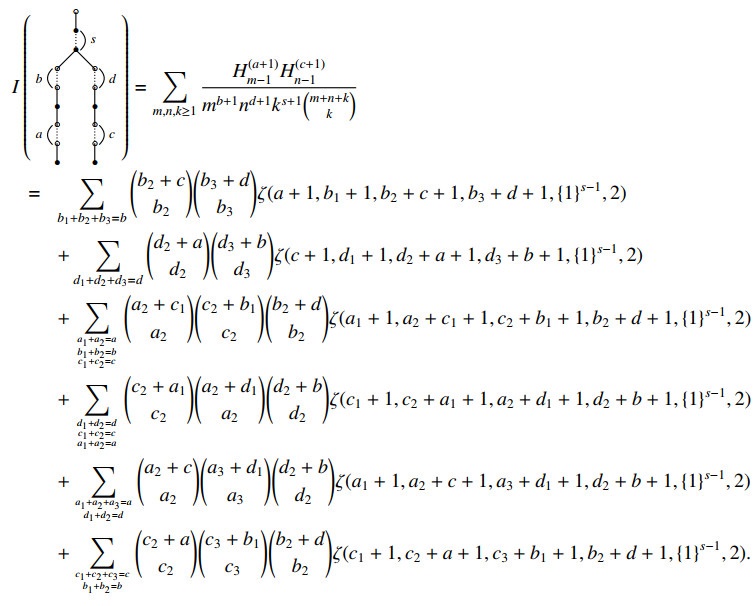
Proof. Consider the following Yamamoto's integral:
We use the map W which is defined in Eq (2.6) to transform the n legs in the 2-poset as (xya1xyb1,xya2xyb2,…,xyanxybn). Then, we shuffle them together (by Eq (4.3)) as a totally ordered diagram, and by Eq (2.7) we have
On the other hand, we write the Yamamoto's integral as follows.
where
We represent B(w1) and C(w1) as a power series:
Therefore,
Given that the integral on the righthand side is the beta function, we deduce that
Combining the two expressions for A given by Eqs (4.6) and (4.7), we obtain the desired formula. □
5.
Main results and conclusions
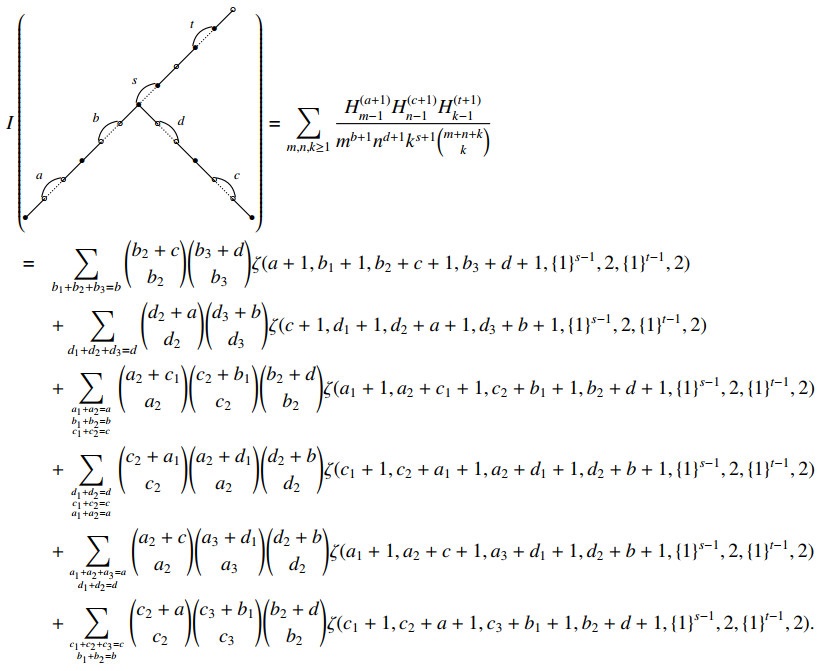
Now, we treat the main result with the following fact [14]:
where E(d1,…,d2n+m) is a suitable constant depending on the variables d1,…,d2n+m.
Given 2n+m+1 nonnegative integers a1,…,an,b1,…,bn,c1,…,cm,s, with ∑ni=1(ai+bi)+∑mk=1ck=w, we have
Since H(ai+1)ki=H(ai+1)ki−1+1kai+1i, in combination with Theorem 3.1, we conclude our main result.
Theorem 5.1. For any nonnegative integers n,m,a1,…,an,b1,…,bn,c1,…,cm,s, the following infinite series
can be expressed as a finite sum of MZVs.
In the following, we will provide concrete examples for clarification. Substitute b=0 in Theorem 3.1, and we have
This identity is appeared in [7, Proposition 5].
Since H(a+1)n=H(a+1)n−1+1na+1, utilizing Theorems 3.1 and 4.1, we deduce that
Letting a=b=c=0 in Eq (5.3), we have (see [4,7])
Let a=1, b=c=0, and we have
Let a=b=c=1, and we have
Let a=b=c=2, and we have
In general, we set a=b=c≥1, then
Following the similar method, we have
where a,b,c,d are nonnegative integers. Similarly, we use Eq (5.4), Theorems 3.1 and 4.1 to get
In particular, we get
If we consider the triple infinite series as examples, then we first apply Theorem 3.1 to get
Then we evaluate
Second, we consider the following Yamamoto's integral
Third, we evaluate
Therefore, we have the formulas for
As a final example, we list
In this paper, we present a method for assessing the form described in Eq (5.2). Specifically, we derive this assessment by evaluating a particular Yamamoto's integral linked to a 2-poset Hasse diagram in two distinct manners: one employs the shuffle relations with its corresponding Lyndon words, while the other utilizes the corresponding summation expansions. Although we do not provide an explicit closed formula for the multiple series in Eq (5.2), if all the parameters are provided, our method enables us to derive the corresponding explicit expression as a linear combination of MZVs.
6.
Author contributions
Kwang-Wu Chen: Conceptualization, Formal Analysis, Methodology, and Writing-original draft; Fu-Yao Yang: Conceptualization, Methodology, Validation, and Writing-review & editing. All authors have read and approved the final version of the manuscript for publication.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
This research was founded by the National Science and Technology Council, Taiwan, R. O. C., under Grant NSTC 112-2115-M-845-001.
Conflict of interest
The authors declare no conflicts of interest.









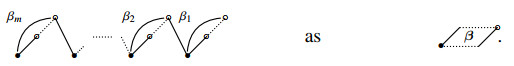
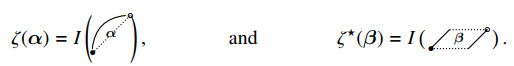
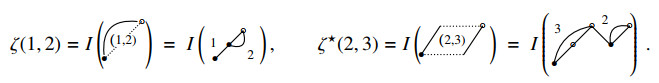
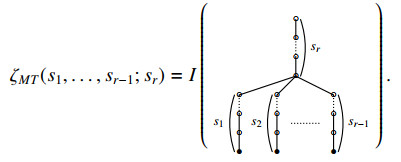
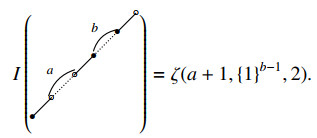
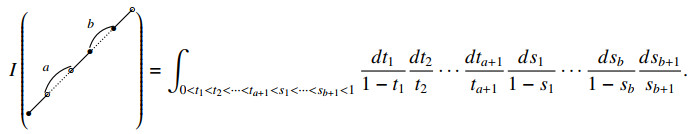
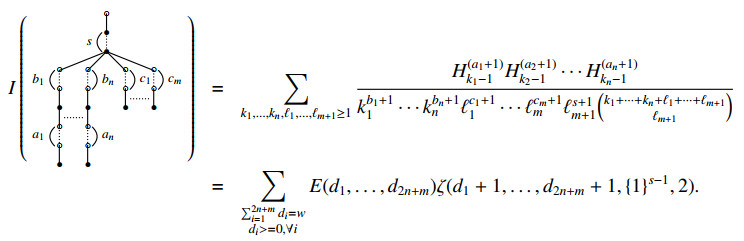

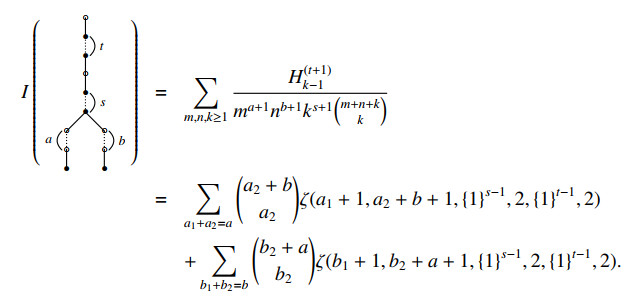




 DownLoad:
DownLoad: