1.
Introduction and preliminaries
The theory of soft set was pioneered by Molodtsov [1], which is a completely novel approach for modeling uncertainty and vagueness. He demonstrated some applications of this theory in solving some practical problems in engineering, economics, medical science, social science, etc. The concept of soft sets was used to define soft topological spaces in [2]. The study in [2] was particularly important in the development of the field of soft topology (for more details, see [3,4,5,6]). Generalizations of soft open sets play an effective role in soft topology through their use to improve on some known results or to open the door to redefine and investigate some of the soft topological concepts such as soft continuity [7], soft connectedness [8,9], soft separation axioms [10,11], etc. Akdag and Ozkan [12] initiated and studied the concept of soft α-open sets on soft topological spaces. The concept of soft β-open sets was studied by the authors of [13,14], and some properties of soft β-continuity were investigated. Also, the concepts of somewhere dense and Q-sets were defined and studied by the authors of [15,16]. Al-shami et al. [17] initiated the concept of weakly soft semi-open sets and studied its main properties. Also, Al-shami et al. [18] defined and studied the concept of weakly soft β-open sets. Kaur et al. [19] introduced a new approach to studying soft continuous mappings using an induced mapping based on soft sets. Al Ghour and Al-Mufarrij [20] defined new concepts of mappings over soft topological spaces: soft somewhat-r-continuity and soft somewhat-r-openness. Ameen et al. [21] explored more properties of soft somewhere dense continuity.
The notion of fuzzy soft sets was introduced by Maji et al. [22], which combines fuzzy sets [23] and soft sets [1]. Based on fuzzy topologies in the sense of Šostak [24], the notion of fuzzy soft topology is defined and some properties such as fuzzy soft continuity, fuzzy soft interior (closure) set, and fuzzy soft subspace are introduced in [25,26]. The notion of r-fuzzy soft regularly open sets was defined and studied by Çetkin and Aygün [27]. In addition, the notions of r-fuzzy soft β-open (resp., pre-open) sets were introduced by Taha [28]. A new approach to studying separation and regularity axioms via fuzzy soft sets was introduced by the author of [29,30] based on the paper by Aygünoǧlu et al. [25].
The main contribution of this study is arranged as follows:
● In Section 2, we are going to present the notions of fuzzy soft β-closure (β-interior) operators in fuzzy soft topological spaces based on the article by Aygünoǧlu et al. [25] and study some properties of them. Also, the concept of r-fuzzy soft β-connected sets was defined and studied.
● In Section 3, we investigate some properties of a fuzzy soft β-continuity. Moreover, we explore and study the notions of fuzzy soft almost (weakly) β-continuous functions, which are weaker forms of fuzzy soft β-continuous functions. Also, we show that fuzzy soft β-continuity ⇒ fuzzy soft almost β-continuity ⇒ fuzzy soft weakly β-continuity, but the converse may not be true.
● In Section 4, we introduce the notions of fuzzy soft β-irresolute (resp., strongly β-irresolute, β-irresolute open, β-irresolute closed, and β-irresolute homeomorphism) functions between two fuzzy soft topological spaces (V,ηF) and (U,τE) and investigate some properties of these functions. Additionally, the relationships between these classes of functions are considered with the help of some examples.
● In the end, we close this study with some conclusions and open a door to suggest some future papers in Section 5.
In this study, nonempty sets will be denoted by V, U, etc. F is the set of all parameters for V and B⊆F. The family of all fuzzy sets on V is denoted by IV (where I∘=(0,1],I=[0,1]), and for t∈I, t_(v)=t, for all v∈V. The following notions and results will be used in the next sections:
Definition 1.1. [25,31,32] A fuzzy soft set gB on V is a function from F to IV such that gB(k) is a fuzzy set on V, for each k∈B and gB(k)=0_, if k∉B. The family of all fuzzy soft sets on V is denoted by ~(V,F). In [33], the difference between two fuzzy soft sets gB and fA is a fuzzy soft set, defined as follows, for each k∈F:
(gB¯⊓fA)(k)={0_,ifgB(k)≤fA(k),gB(k)∧(fA(k))c,otherwise.
Definition 1.2. [34] A fuzzy soft point kvt on V is a fuzzy soft set, defined as follows:
where vt is a fuzzy point on V. kvt is said to belong to a fuzzy soft set gB, denoted by kvt˜∈gB, if t≤gB(k)(v). The family of all fuzzy soft points on V is denoted by ~Pt(V).
Definition 1.3. [35] A fuzzy soft point kvt∈~Pt(V) is called a soft quasi-coincident with gB∈~(V,F), denoted by kvt∇gB, if t+gB(k)(v)>1. A fuzzy soft set fA∈~(V,F) is called a soft quasi-coincident with gB∈~(V,F), denoted by fA∇gB, if there is k∈F and v∈V such that, fA(k)(v)+gB(k)(v)>1, if fA is not soft quasi-coincident with gB, fA¯∇gB.
Definition 1.4. [25] A function η:F⟶[0,1]~(V,F) is said to be a fuzzy soft topology on V if it satisfies the following, for each k∈F:
(1) ηk(Φ)=ηk(˜F)=1,
(2) ηk(gB⊓fA)≥ηk(gB)∧ηk(fA), for each gB,fA∈~(V,F),
(3) ηk(⊔δ∈Δ(gB)δ)≥∧δ∈Δηk((gB)δ), for each (gB)δ∈~(V,F),δ∈Δ.
Thus, (V,ηF) is said to be a fuzzy soft topological space (briefly, FSTS) based on the sense of Šostak [24].
Definition 1.5. [25] Let (V,ηF) and (U,τE) be an FSTSs. A fuzzy soft function φψ:~(V,F)⟶~(U,E) is said to be fuzzy soft continuous if ηk(φ−1ψ(gB))≥τe(gB) for each gB∈~(U,E), k∈F, and (e=ψ(k))∈E.
Definition 1.6. [26,27] In an FSTS (V,ηF), for each gB∈~(V,F), k∈F, and r∈I0, we define the fuzzy soft operators Cη and Iη :F×~(V,F)×I∘→~(V,F) as follows:
Cη(k,gB,r)=⊓{fA∈~(V,F):gB⊑fA,ηk(fcA)≥r},
Iη(k,gB,r)=⊔{fA∈~(V,F):fA⊑gB,ηk(fA)≥r}.
Definition 1.7. Let (V,ηF) be an FSTS and r∈I0. A fuzzy soft set gB∈~(V,F) is said to be r-fuzzy soft β-open [28] (resp., pre-open [28], semi-open [36], and regularly open [27]) if gB⊑Cη(k,Iη(k,Cη(k,gB,r),r),r) (resp., gB⊑Iη(k,Cη(k,gB,r),r), gB⊑Cη(k,Iη(k,gB,r),r), and gB=Iη(k,Cη(k,gB,r),r)) for each k∈F.
Definition 1.8. [27] Let (V,ηF) be an FSTS and r∈I0. A fuzzy soft set gB∈~(V,F) is said to be an r-fuzzy soft regularly closed if gB=Cη(k,Iη(k,gB,r),r) for each k∈F.
Remark 1.1. [28] From the previous definition, we can summarize the relationships among different types of fuzzy soft sets as in the next diagram.
Definition 1.9. [36] Let (V,ηF) and (U,τE) be an FSTSs. A fuzzy soft function φψ:~(V,F)⟶~(U,E) is said to be a fuzzy soft almost (resp., weakly) continuous if, for each kvt∈~Pt(V) and each gB∈~(U,E) with τe(gB)≥r containing φψ(kvt), there is fA∈~(V,F) with ηk(fA)≥r containing, kvt such that φψ(fA)⊑Iτ(e,Cτ(e,gB,r),r) (resp., φψ(fA)⊑Cτ(e,gB,r)).
Remark 1.2. [36] From Definitions 1.5 and 1.9, we have: fuzzy soft continuity ⇒ fuzzy soft almost continuity ⇒ fuzzy soft weakly continuity.
The basic results and definitions that we need in the next sections are found in [25,26].
2.
Some properties of r-fuzzy soft β-closed sets
Here, we introduce the concept of r-fuzzy soft β-closed sets in FSTSs based on the sense of Šostak [24] and investigate some properties of them. Also, we define and study the concepts of fuzzy soft β-closure (β-interior) operators. Moreover, the concept of r-fuzzy soft β-connected sets is defined and characterized.
Definition 2.1. Let (V,ηF) be an FSTS. A fuzzy soft set gB∈~(V,F) is said to be an r-fuzzy soft β-closed if Iη(k,Cη(k,Iη(k,gB,r),r),r)⊑gB for each k∈F and r∈I0.
Proposition 2.1. Let (V,ηF) be an FSTS, gB∈~(V,F), k∈F, and r∈I0, then we have
(1) gB is an r-fuzzy soft β-closed set if gcB is r-fuzzy soft β-open [28].
(2) Any intersection of r-fuzzy soft β-closed sets is r-fuzzy soft β-closed.
(3) Any union of r-fuzzy soft β-open sets is r-fuzzy soft β-open.
Proof. Follows from Definitions 1.7 and 2.1.
Proposition 2.2. Let (V,ηF) be an FSTS, gB,fA∈~(V,F), k∈F, and r∈I0. If gB is an r-fuzzy soft pre-open set such that gB⊑fA⊑Cη(k,Iη(k,gB,r),r), fA is r-fuzzy soft β-open.
Proof. Since gB is r-fuzzy soft pre-open and gB⊑fA, then gB⊑Iη(k,Cη(k,gB,r),r)⊑Iη(k,Cη(k,fA,r),r). Since fA⊑Cη(k,Iη(k,gB,r),r), then fA⊑Cη(k,Iη(k,Iη(k,Cη(k,fA,r),r),r),r)=Cη(k,Iη(k,Cη(k,fA,r),r),r), so fA is r-fuzzy soft β-open.
Proposition 2.3. Let (V,ηF) be an FSTS, gB,fA∈~(V,F), k∈F, and r∈I0. If gB is an r-fuzzy soft pre-closed set such that gB⊒fA⊒Iη(k,Cη(k,gB,r),r), fA is r-fuzzy soft β-closed.
Proof. Easily proved by a similar way in Proposition 2.2.
Definition 2.2. In an FSTS (V,ηF), for each gB∈~(V,F), k∈F, and r∈I0, we define a fuzzy soft operator βCη :F×~(V,F)×I∘→~(V,F) as follows: βCη(k,gB,r)=⊓{fA∈~(V,F):gB⊑fA,fAisr-fuzzy softβ-closed}.
Theorem 2.1. In an FSTS (V,ηF), for each fA,gB∈~(V,F), k∈F, and r∈I0, the operator βCη :F×~(V,F)×I∘→~(V,F) satisfies the following properties:
(1) βCη(k,Φ,r)=Φ.
(2) gB⊑βCη(k,gB,r)⊑Cη(k,gB,r).
(3) βCη(k,gB,r)⊑βCη(k,fA,r), if gB⊑fA.
(4) βCη(k,βCη(k,gB,r),r)=βCη(k,gB,r).
(5) βCη(k,gB⊔fA,r)⊒βCη(k,gB,r)⊔βCη(k,fA,r).
(6) gB is r-fuzzy soft β-closed if βCη(k,gB,r)=gB.
(7) βCη(k,Cη(k,gB,r),r)=Cη(k,gB,r).
Proof. (1), (2), (3), and (6) are easily proved from the definition of βCη.
(4) From (2) and (3), βCη(k,gB,r)⊑βCη(k,βCη(k,gB,r),r). Now, we show that βCη(k,gB,r)⊒βCη(k,βCη(k,gB,r),r). Suppose that βCη(k,gB,r) does not contain βCη(k,βCη(k,gB,r),r), then there is v∈V and t∈(0,1) such that βCη(k,gB,r)(k)(v)<t<βCη(k,βCη(k,gB,r),r)(k)(v).(A)
Since βCη(k,gB,r)(k)(v)<t, by the definition of βCη, there is hF as an r-fuzzy soft β-closed with gB⊑hF such that βCη(k,gB,r)(k)(v)≤hF(k)(v)<t. Since gB⊑hF, we have βCη(k,gB,r)⊑hF. Again, by the definition of βCη, βCη(k,βCη(k,gB,r),r)⊑hF. Hence, βCη(k,βCη(k,gB,r),r)(k)(v)≤hF(k)(v)<t, which is a contradiction for (A). Thus, βCη(k,gB,r)⊒βCη(k,βCη(k,gB,r),r), then βCη(k,βCη(k,gB,r),r)=βCη(k,gB,r).
(5) Since gB⊑gB⊔fA and fA⊑gB⊔fA, then by (3) we have βCη(k,gB,r)⊑βCη(k,gB⊔fA,r) and βCη(k,fA,r)⊑βCη(k,gB⊔fA,r). Thus, βCη(k,gB⊔fA,r)⊒βCη(k,gB,r)⊔βCη(k,fA,r).
(7) Since Cη(k,gB,r) is an r-fuzzy soft β-closed set, then by (6) we have βCη(k,Cη(k,gB,r),r)=Cη(k,gB,r).
Definition 2.3. Let (V,ηF) be an FSTS, r∈I0, and gB,fA∈~(V,F), then we have:
(1) Two fuzzy soft sets gB and fA are called r-fuzzy soft β-separated if gB¯∇βCη(k,fA,r) and fA¯∇βCη(k,gB,r) for each k∈F.
(2) Any fuzzy soft set which cannot be expressed as the union of two r-fuzzy soft β-separated sets is called an r-fuzzy soft β-connected.
Theorem 2.2. In an FSTS (V,ηF), we have:
(1) If fA and gB ∈~(V,F) are r-fuzzy soft β-separated and hC, tD ∈~(V,F) such that hC⊑fA and tD⊑gB, then hC and tD are r-fuzzy soft β-separated.
(2) If fA¯∇gB are either both r-fuzzy soft β-open or both are r-fuzzy soft β-closed, then fA and gB are r-fuzzy soft β-separated.
(3) If fA and gB are either both r-fuzzy soft β-open or both r-fuzzy soft β-closed, then fA⊓gcB and gB⊓fcA are r-fuzzy soft β-separated.
Proof. (1) and (2) are obvious.
(3) Let fA and gB be an r-fuzzy soft β-open. Since fA⊓gcB⊑gcB, βCη(k,fA⊓gcB,r)⊑gcB, and βCη(k,fA⊓gcB,r)¯∇gB, then βCη(k,fA⊓gcB,r)¯∇(gB⊓fcA). Again, since gB⊓fcA⊑fcA, βCη(k,gB⊓fcA,r)⊑fcA, and βCη(k,gB ⊓fcA,r)¯∇fA, then βCη(k,gB⊓fcA,r)¯∇(fA⊓gcB). Thus, fA⊓gcB and gB⊓fcA are r-fuzzy soft β-separated. The other case follows similar lines.
Theorem 2.3. In an FSTS (V,ηF), fA, gB ∈~(V,F) are r-fuzzy soft β-separated if there exist two r-fuzzy soft β-open sets hC and tD such that fA⊑hC, gB⊑tD, fA¯∇tD, and gB¯∇hC.
Proof. (⇒) Let fA and gB ∈~(V,F) be r-fuzzy soft β-separated, fA⊑(βCη(k,gB,r))c=hC and gB⊑(βCη(k,fA,r))c=tD, where tD and hC are r-fuzzy soft β-open, then tD¯∇βCη(k,fA,r) and hC¯∇βCη(k,gB,r). Thus, gB¯∇hC and fA¯∇tD. Hence, we obtain the required result.
(⇐) Let hC and tD be r-fuzzy soft β-open such that gB⊑tD, fA⊑hC, gB¯∇hC, and fA¯∇tD, then gB⊑hcC and fA⊑tcD. Hence, βCη(k,gB,r)⊑hcC and βCη(k,fA,r)⊑tcD, then βCη(k,gB,r)¯∇fA and βCη(k,fA,r)¯∇gB. Thus, gB and fA are r-fuzzy soft β-separated. Hence, we obtain the required result.
Theorem 2.4. In an FSTS (V,ηF), if gB∈~(V,F) is r-fuzzy soft β-connected such that gB⊑fA⊑βCη(k,gB,r), then fA is r-fuzzy soft β-connected.
Proof. Suppose that fA is not r-fuzzy soft β-connected, then there is r-fuzzy soft β-separated sets h∗C and t∗D ∈~(V,F) such that fA=h∗C⊔t∗D. Let hC=gB⊓h∗C and tD=gB⊓t∗D, then gB=tD⊔hC. Since hC⊑h∗C and tD⊑t∗D, by Theorem 2.2(1), hC and tD are r-fuzzy soft β-separated, which is a contradiction. Thus, fA is r-fuzzy soft β-connected, as required.
Theorem 2.5. In an FSTS (V,ηF), for each gB∈~(V,F), k∈F, and r∈I0, we define a fuzzy soft operator βIη :F×~(V,F)×I∘→~(V,F) as follows: βIη(k,gB,r)=⊔{fA∈~(V,F):fA⊑gB,andfAisr-fuzzy softβ-open}. For each fA,gB∈~(V,F), the operator βIη satisfies the following properties:
(1) βIη(k,˜F,r)=˜F.
(2) Iη(k,gB,r)⊑βIη(k,gB,r)⊑gB.
(3) βIη(k,gB,r)⊑βIη(k,fA,r), if gB⊑fA.
(4) βIη(k,βIη(k,gB,r),r)=βIη(k,gB,r).
(5) βIη(k,gB,r)⊓βIη(k,fA,r)⊒βIη(k,gB⊓fA,r).
(6) gB is r-fuzzy soft β-open if βIη(k,gB,r)=gB.
(7) βIη(k,gcB,r)=(βCη(k,gB,r))c.
Proof. (1), (2), (3), and (6) are easily proved from the definition of βIη.
(4) and (5) are easily proved by a similar way in Theorem 2.1.
(7) For each gB∈~(V,F), k∈F, and r∈I0, we have βIη(k,gcB,r)=⊔{fA∈~(V,F):fA⊑gcB,fAisr-fuzzy softβ-open} = [⊓{fcA∈~(V,F):gB⊑fcA,andfcAisr-fuzzy softβ-closed}]c = (βCη(k,gB,r))c.
3.
Weaker forms of fuzzy soft β-continuity
Here, we investigate some properties of fuzzy soft β-continuity. As a weaker form of fuzzy soft β-continuity, the concepts of fuzzy soft almost (weakly) β-continuous functions are introduced and some properties are given.
Definition 3.1. Let (V,ηF) and (U,τE) be FSTSs. A fuzzy soft function φψ:~(V,F)⟶~(U,E) is said to be a fuzzy soft β-continuous if φ−1ψ(gB) is an r-fuzzy soft β-closed set for each gB∈~(U,E) with τe(gcB)≥r, k∈F, (e=ψ(k))∈E, and r∈Io.
Theorem 3.1. Let (V,ηF) and (U,τE) be FSTSs and φψ:~(V,F)⟶~(U,E) be a fuzzy soft function. The following statements are equivalent for each gB∈~(U,E), k∈F, (e=ψ(k))∈E, and r∈I∘:
(1) φψ is fuzzy soft β-continuous.
(2) For each gB∈~(U,E) with τe(gB)≥r, φ−1ψ(gB) is r-fuzzy soft β-open.
(3) βCη(k,φ−1ψ(gB),r)⊑φ−1ψ(Cτ(e,gB,r)).
(4) φ−1ψ(Iτ(e,gB,r))⊑βIη(k,φ−1ψ(gB),r).
(5) Iη(k,Cη(k,Iη(k,φ−1ψ(gB),r),r),r)⊑φ−1ψ(Cτ(e,gB,r)).
Proof. (1) ⇔ (2) Follows from Proposition 2.1 and φ−1ψ(gcB)=(φ−1ψ(gB))c.
(1) ⇒ (3) Let gB∈~(U,E); hence, by (1), φ−1ψ(Cτ(e,gB,r)) is r-fuzzy soft β-closed, then we obtain βCη(k,φ−1ψ(gB),r)⊑φ−1ψ(Cτ(e,gB,r)).
(3) ⇔ (4) Follows from Theorem 2.5.
(3) ⇒ (5) Let gB∈~(U,E); hence, by (3), we obtain Iη(k,Cη(k,Iη(k,φ−1ψ(gB),r),r),r)⊑βCη(k,φ−1ψ(gB),r)⊑φ−1ψ(Cτ(e,gB,r)).
(5) ⇒ (2) Let gB∈~(U,E) with τe(gB)≥r. By (5), we obtain (φ−1ψ(gB))c=φ−1ψ(gcB)⊒Iη(k,Cη(k,Iη(k,φ−1ψ(gcB),r),r),r)=(Cη(k,Iη(k,Cη(k,φ−1ψ(gB),r),r),r))c, then φ−1ψ(gB)⊑Cη(k,Iη(k,Cη(k,φ−1ψ(gB),r),r),r), so φ−1ψ(gB) is r-fuzzy soft β-open.
Lemma 3.1. Every fuzzy soft continuous function [25] is fuzzy soft β-continuous.
Proof. Follows from Definition 2.5 and Theorem 3.1.
Remark 3.1. The converse of Lemma 3.1 is not true, as shown by Example 3.1.
Example 3.1. Let V={v1,v2,v3}, F={k1,k2}, and define gF,hF∈~(V,F) as follows: gF={(k1,{v10.2,v20.3,v30.2}),(k2,{v10.2,v20.3,v30.2})}, hF={(k1,{v10.3,v20.4,v30.8}),(k2,{v10.3,v20.4,v30.8})}. Define fuzzy soft topologies ηF,τF:F⟶[0,1]~(V,F) as follows: ∀k∈F,
ηk(nF)= {1,ifnF∈{Φ,˜F},12,ifnF=gF,0,otherwise,
τk(nF)= {1,ifnF∈{Φ,˜F},13,ifnF=hF,0,otherwise.
Thus, the identity fuzzy soft function φψ:(V,ηF)⟶(V,τF) is fuzzy soft β-continuous, but it is not fuzzy soft continuous.
Definition 3.2. Let (V,ηF) and (U,τE) be FSTSs. A fuzzy soft function φψ:~(V,F)⟶~(U,E) is said to be fuzzy soft almost (resp., weakly) β-continuous if for each kvt∈~Pt(V) and each fA∈~(U,E) with τe(fA)≥r containing φψ(kvt), there is gB∈~(V,F) that is an r-fuzzy soft β-open set containing kvt, such that φψ(gB)⊑Iτ(e,Cτ(e,fA,r),r) (resp., φψ(gB)⊑Cτ(e,fA,r)), k∈F, (e=ψ(k))∈E, and r∈I∘.
Lemma 3.2. (1) Every fuzzy soft β-continuous function is fuzzy soft almost β-continuous.
(2) Every fuzzy soft almost β-continuous function is fuzzy soft weakly β-continuous.
Proof. Follows from Definition 3.2 and Theorem 3.1.
Remark 3.2. The converse of Lemma 2 is not true, as shown by Examples 3.2 and 3.3.
Example 3.2. Let V={v1,v2,v3}, F={k1,k2}, and define fF,gF,hF ∈~(V,F) as follows: fF={(k1,{v10.5,v20.5,v30.5}),(k2,{v10.5,v20.5,v30.5})}, gF={(k1,{v10.4,v20.4,v30.4}),(k2,{v10.4,v20.4,v30.4})}, hF={(k1,{v10.5,v20.5,v30.6}),(k2,{v10.5,v20.5,v30.6})}. Define fuzzy soft topologies ηF,τF:F⟶[0,1]~(V,F) as follows: ∀k∈F,
ηk(nF)= {1,ifnF∈{Φ,˜F},23,ifnF=fF,12,ifnF=gF,0,otherwise,
τk(nF)= {1,ifnF∈{Φ,˜F},23,ifnF=fF,13,ifnF=hF,0,otherwise.
Thus, the identity fuzzy soft function φψ:(V,ηF)⟶(V,τF) is fuzzy soft almost β-continuous, but it is not fuzzy soft β-continuous.
Example 33. Let V={v1,v2,v3}, F={k1,k2}, and define gF,hF∈~(V,F) as follows: gF={(k1,{v10.6,v20.2,v30.4}),(k2,{v10.6,v20.2,v30.4})}, hF={(k1,{v10.3,v20.2,v30.5}),(k2,{v10.3,v20.2,v30.5})}. Define fuzzy soft topologies ηF,τF:F⟶[0,1]~(V,F) as follows: ∀k∈F,
ηk(nF)= {1,ifnF∈{Φ,˜F},12,ifnF=gF,0,otherwise,
τk(nF)= {1,ifnF∈{Φ,˜F},13,ifnF=hF,0,otherwise.
Thus, the identity fuzzy soft function φψ:(V,ηF)⟶(V,τF) is fuzzy soft weakly β-continuous, but it is not fuzzy soft almost β-continuous.
Lemma 3.3. (1) Every fuzzy soft almost continuous function is fuzzy soft almost β-continuous.
(2) Every fuzzy soft weakly continuous function is fuzzy soft weakly β-continuous.
Proof. Follows from Definitions 1.9 and 3.2.
Remark 3.3. From the previous definitions and results, we can summarize the relationships among different types of fuzzy soft continuity as in the next diagram.
Theorem 3.2. Let (V,ηF) and (U,τE) be FSTSs and φψ:~(V,F)⟶~(U,E) be a fuzzy soft function. The following statements are equivalent for each gB∈~(U,E), k∈F, (e=ψ(k))∈E, and r∈I∘:
(1) φψ is fuzzy soft almost β-continuous.
(2) φ−1ψ(gB) is r-fuzzy soft β-open, for each gB is r-fuzzy soft regularly open.
(3) φ−1ψ(gB) is r-fuzzy soft β-closed, for each gB is r-fuzzy soft regularly closed.
(4) βCη(k,φ−1ψ(gB),r)⊑φ−1ψ(Cτ(e,gB,r)), for each gB is r-fuzzy soft β-open.
(5) βCη(k,φ−1ψ(gB),r)⊑φ−1ψ(Cτ(e,gB,r)), for each gB is r-fuzzy soft semi-open.
(6) βIη(k,φ−1ψ(Iτ(e,Cτ(e,gB,r),r)),r)⊒φ−1ψ(gB), for each gB with τe(gB)≥r.
Proof. (1) ⇒ (2) Let kvt∈~Pt(V) and gB∈~(U,E) be an r-fuzzy soft regularly open set containing φψ(kvt). Hence, by (1), fA∈~(V,F) is an r-fuzzy soft β-open set containing kvt such that φψ(fA)⊑Iτ(e,Cτ(e,gB,r),r).
Thus, fA⊑φ−1ψ(Iτ(e,Cτ(e,gB,r),r))=φ−1ψ(gB) and kvt˜∈fA⊑φ−1ψ(gB), then kvt˜∈Cη(k,Iη(k,Cη(k,φ−1ψ(gB),r),r),r) and φ−1ψ(gB)⊑Cη(k,Iη(k,Cη(k,φ−1ψ(gB),r),r),r). Therefore, φ−1ψ(gB) is an r-fuzzy soft β-open set.
(2) ⇒ (3) Let gB be an r-fuzzy soft regularly closed set. Hence, by (2), φ−1ψ(gcB)=(φ−1ψ(gB))c is an r-fuzzy soft β-open set, then φ−1ψ(gB) is an r-fuzzy soft β-closed set.
(3) ⇒ (4) Let gB be an r-fuzzy soft β-open set. Since Cτ(e,gB,r) is an r-fuzzy soft regularly closed set, by (3), φ−1ψ(Cτ(e,gB,r)) is an r-fuzzy soft β-closed set. Since φ−1ψ(gB)⊑φ−1ψ(Cτ(e,gB,r)), then we have βCη(k,φ−1ψ(gB),r)⊑φ−1ψ(Cτ(e,gB,r)).
(4) ⇒ (5) This is obvious from every r-fuzzy soft semi-open set that is an r-fuzzy soft β-open set.
(5) ⇒ (3) Let gB be an r-fuzzy soft regularly closed set. Hence, gB is an r-fuzzy soft semi-open set, then by (5), βCη(k,φ−1ψ(gB),r)⊑φ−1ψ(Cτ(e,gB,r))=φ−1ψ(gB). Therefore, φ−1ψ(gB) is an r-fuzzy soft β-closed set.
(3) ⇒ (6) Let gB∈~(U,E) with τe(gB)≥r and kvt˜∈φ−1ψ(gB), then we have kvt˜∈φ−1ψ(Iτ(e,Cτ(e,gB,r),r)). Since [Iτ(e,Cτ(e,gB,r),r)]c is an r-fuzzy soft regularly closed set, by (3), φ−1ψ([Iτ(e,Cτ(e,gB,r),r)]c) is an r-fuzzy soft β-closed set. Thus, φ−1ψ(Iτ(e,Cτ(e,gB,r),r)) is an r-fuzzy soft β-open set and kvt˜∈βIη(k,φ−1ψ(Iτ(e,Cτ(e,gB,r),r)),r), then φ−1ψ(gB)⊑βIη(k,φ−1ψ(Iτ(e,Cτ(e,gB,r),r)),r).
(6) ⇒ (1) Let kvt∈~Pt(V) and gB∈~(U,E) with τe(gB)≥r containing φψ(kvt); hence, by (6), φ−1ψ(gB)⊑βIη(k,φ−1ψ(Iτ(e,Cτ(e,gB,r),r)),r).
Since kvt˜∈φ−1ψ(gB), then we obtain kvt˜∈βIη(k,φ−1ψ(Iτ(e,Cτ(e,gB,r),r)),r)=fA (say). Hence, fA∈~(V,F) is an r-fuzzy soft β-open set containing kvt such that φψ(fA)⊑Iτ(e,Cτ(e,gB,r),r). Therefore, φψ is fuzzy soft almost β-continuous.
In a similar way, we can prove the following theorem.
Theorem 3.3. Let (V,ηF) and (U,τE) be FSTSs and φψ:~(V,F)⟶~(U,E) be a fuzzy soft function. The following statements are equivalent for each gB∈~(U,E), k∈F, (e=ψ(k))∈E, and r∈I∘:
(1) φψ is fuzzy soft weakly β-continuous.
(2) Cη(k,Iη(k,Cη(k,φ−1ψ(Cτ(e,gB,r)),r),r),r)⊒φ−1ψ(gB), if τe(gB)≥r.
(3) Iη(k,Cη(k,Iη(k,φ−1ψ(Iτ(e,gB,r)),r),r),r)⊑φ−1ψ(gB), if τe(gcB)≥r.
(4) βCη(k,φ−1ψ(Iτ(e,gB,r)),r)⊑φ−1ψ(gB), if τe(gcB)≥r.
(5) βCη(k,φ−1ψ(Iτ(e,Cτ(e,gB,r),r)),r)⊑φ−1ψ(Cτ(e,gB,r)).
(6) βIη(k,φ−1ψ(Cτ(e,Iτ(e,gB,r),r)),r)⊒φ−1ψ(Iτ(e,gB,r)).
(7) φ−1ψ(gB)⊑βIη(k,φ−1ψ(Cτ(e,gB,r)),r), if τe(gB)≥r.
Let P and Q :F×~(V,F)×I∘→~(V,F) be operators on ~(V,F), and R and S :E×~(U,E)×I∘→~(U,E) be operators on ~(U,E).
Definition 3.3. [36] Let (V,ηF) and (U,τE) be FSTSs. φψ:~(V,F)⟶~(U,E) is said to be a fuzzy soft (P,Q,R,S)-continuous function if P[k,φ−1ψ(S(e,gB,r)),r]¯⊓Q[k,φ−1ψ(R(e,gB,r)),r] =Φ for each gB∈~(U,E) with τe(gB)≥r, k∈F, and (e=ψ(k))∈E.
In (2014), Aygünoǧlu et al. [25] defined the notion of fuzzy soft continuous functions: ηk(φ−1ψ(fA))≥τe(fA), for each fA∈~(U,E), k∈F, and (e=ψ(k))∈E. We can see that Definition 3.3 generalizes the concept of fuzzy soft continuous functions when we choose P = identity operator, Q = interior operator, R = identity operator, and S = identity operator.
A historical justification of Definition 3.3:
(1) In Section 3, we introduced the notion of fuzzy soft β-continuous functions: φ−1ψ(gB)⊑Cη(k,Iη(k,Cη(k,φ−1ψ(gB),r),r),r), for each gB∈~(U,E) with τe(gB)≥r. Here, P = identity operator, Q = closure interior closure operator, R = identity operator, and S = identity operator.
(2) In Section 3, we introduced the notion of fuzzy soft almost β-continuous functions: φ−1ψ(gB)⊑βIη(k,φ−1ψ(Iτ(e,Cτ(e,gB,r),r)),r), for each gB∈~(U,E) with τe(gB)≥r. Here, P = identity operator, Q = β-interior operator, R = interior closure operator, and S = identity operator.
(3) In Section 3, we introduced the notion of fuzzy soft weakly β-continuous functions: φ−1ψ(gB)⊑βIη(k,φ−1ψ(Cτ(e,gB,r)),r), for each gB∈~(U,E) with τe(gB)≥r. Here, P = identity operator, Q = β-interior operator, R = closure operator, and S = identity operator.
4.
Fuzzy soft β-irresoluteness
Here, we introduce the concepts of fuzzy soft β-irresolute (resp., strongly β-irresolute, β-irresolute open, β-irresolute closed, and β-irresolute homeomorphism) functions between two FSTSs (V,ηF) and (U,τE) and study some of its features. Also, we show that fuzzy soft strongly β-irresolute ⇒ fuzzy soft β-irresolute ⇒ fuzzy soft β-continuity, but the converse may not be true.
Definition 4.1. Let (V,ηF) and (U,τE) be FSTSs. A fuzzy soft function φψ:~(V,F)⟶~(U,E) is said to be a fuzzy soft β-irresolute (resp., strongly β-irresolute) if φ−1ψ(gB) is an r-fuzzy soft β-open (resp., semi-open) set for each gB∈~(U,E) r-fuzzy soft β-open set and r∈Io.
Lemma 4.1. (1) Every fuzzy soft strongly β-irresolute function is fuzzy soft β-irresolute.
(2) Every fuzzy soft β-irresolute function is fuzzy soft β-continuous.
Proof. Follows from Definition 4.1 and Theorem 3.1.
Remark 4.1. The converse of Lemma 4.1 is not true, as shown by Examples 4.1 and 4.2.
Example 4.1. Let V={v1,v2,v3}, F={k1,k2}, and define fF,gF∈~(V,F) as follows: fF={(k1,{v10.3,v20.3,v30.3}),(k2,{v10.3,v20.3,v30.3})}, gF={(k1,{v10.4,v20.4,v30.4}),(k2,{v10.4,v20.4,v30.4})}. Define fuzzy soft topologies ηF,τF:F⟶[0,1]~(V,F) as follows: ∀k∈F,
ηk(nF)= {1,ifnF∈{Φ,˜F},12,ifnF=gF,0,otherwise,
τk(nF)= {1,ifnF∈{Φ,˜F},12,ifnF=fF,0,otherwise.
Thus, the identity fuzzy soft function φψ:(V,ηF)⟶(V,τF) is fuzzy soft β-irresolute, but it is not fuzzy soft strongly β-irresolute.
Example 4.2. Let V={v1,v2,v3}, F={k1,k2}, and define fF,gF∈~(V,F) as follows: fF={(k1,{v10.5,v20.5,v30.5}),(k2,{v10.5,v20.5,v30.5})}, gF={(k1,{v10.4,v20.4,v30.4}),(k2,{v10.4,v20.4,v30.4})}. Define fuzzy soft topologies ηF,τF:F⟶[0,1]~(V,F) as follows: ∀k∈F,
ηk(nF)= {1,ifnF∈{Φ,˜F},13,ifnF=fF,12,ifnF=gF,0,otherwise,
τk(nF)= {1,ifnF∈{Φ,˜F},13,ifnF=fF,0,otherwise.
Thus, the identity fuzzy soft function φψ:(V,ηF)⟶(V,τF) is fuzzy soft β-continuous, but it is not fuzzy soft β-irresolute.
Remark 4.2. From the previous results, we have: fuzzy soft strongly β-irresolute ⇒ fuzzy soft β-irresolute ⇒ fuzzy soft β-continuity.
Theorem 4.1. Let (V,ηF) and (U,τE) be FSTSs and φψ:~(V,F)⟶~(U,E) be a fuzzy soft function. The following statements are equivalent for each gB∈~(U,E), k∈F, (e=ψ(k))∈E, and r∈I∘:
(1) φψ is fuzzy soft β-irresolute.
(2) For each gB r-fuzzy soft β-closed, φ−1ψ(gB) is r-fuzzy soft β-closed.
(3) βCη(k,φ−1ψ(gB),r)⊑φ−1ψ(βCτ(e,gB,r)).
(4) φ−1ψ(βIτ(e,gB,r))⊑βIη(k,φ−1ψ(gB),r).
(5) Iη(k,Cη(k,Iη(k,φ−1ψ(gB),r),r),r)⊑φ−1ψ(βCτ(e,gB,r)).
Proof. (1) ⇔ (2) Follows from Proposition 2.1 and φ−1ψ(gcB)=(φ−1ψ(gB))c.
(2) ⇒ (3) Let gB∈~(U,E). Hence, by (2), φ−1ψ(βCτ(e,gB,r)) is r-fuzzy soft β-closed, then we obtain βCη(k,φ−1ψ(gB),r)⊑φ−1ψ(βCτ(e,gB,r)).
(3) ⇔ (4) Follows from Theorem 2.5.
(3) ⇒ (5) Let gB∈~(U,E). Hence, by (3), we obtain Iη(k,Cη(k,Iη(k,φ−1ψ(gB),r),r),r)⊑βCη(k,φ−1ψ(gB),r)⊑φ−1ψ(βCτ(e,gB,r)).
(5) ⇒ (1) Let gB∈~(U,E) be an r-fuzzy soft β-open. Hence, by (5), we obtain (φ−1ψ(gB))c=φ−1ψ(gcB)⊒Iη(k,Cη(k,Iη(k,φ−1ψ(gcB),r),r),r)=(Cη(k,Iη(k,Cη(k,φ−1ψ(gB),r),r),r))c, then φ−1ψ(gB)⊑Cη(k,Iη(k,Cη(k,φ−1ψ(gB),r),r),r), so φ−1ψ(gB) is r-fuzzy soft β-open. Hence, φψ is fuzzy soft β-irresolute.
Theorem 4.2. Let (V,ηF) and (U,τE) be FSTSs and φψ:~(V,F)⟶~(U,E) be a fuzzy soft function. The following statements are equivalent for each gB∈~(U,E), k∈F, (e=ψ(k))∈E, and r∈I∘:
(1) φψ is fuzzy soft strongly β-irresolute.
(2) For each gB r-fuzzy soft β-closed, φ−1ψ(gB) is r-fuzzy soft semi-closed.
(3) Iη(k,Cη(k,φ−1ψ(gB),r),r)⊑φ−1ψ(βCτ(e,gB,r)).
Proof. (1) ⇔ (2) Follows from Proposition 2.1 and φ−1ψ(gcB)=(φ−1ψ(gB))c.
(2) ⇒ (3) Let gB∈~(U,E). Hence, by (2), φ−1ψ(βCτ(e,gB,r)) is r-fuzzy soft semi-closed, then we obtain Iη(k,Cη(k,φ−1ψ(gB),r),r)⊑φ−1ψ(βCτ(e,gB,r)).
(3) ⇒ (1) Let gB∈~(U,E) be an r-fuzzy soft β-open. Hence, by (3), we obtain (φ−1ψ(gB))c=φ−1ψ(gcB)⊒Iη(k,Cη(k,φ−1ψ(gcB),r),r)=(Cη(k,Iη(k,φ−1ψ(gB),r),r))c, then φ−1ψ(gB)⊑Cη(k,Iη(k,φ−1ψ(gB),r),r), so φ−1ψ(gB) is r-fuzzy soft semi-open. Hence, φψ is fuzzy soft strongly β-irresolute.
Proposition 4.1. Let (V,ηF), (U,τE), and (W,γH) be FSTSs and φψ:~(V,F)⟶~(U,E), φ∗ψ∗:~(U,E)⟶~(W,H) be two fuzzy soft functions, then the composition φ∗ψ∗∘φψ is fuzzy soft β-irresolute (resp., strongly β-irresolute and β-continuous) if φψ is fuzzy soft β-irresolute (resp., strongly β-irresolute and β-irresolute) and φ∗ψ∗ is fuzzy soft β-irresolute (resp., β-irresolute and β-continuous).
Proof. Follows from Definition 4.1 and Theorem 3.1.
Proposition 4.2. Let (V,ηF), (U,τE), and (W,γH) be FSTSs and φψ:~(V,F)⟶~(U,E), φ∗ψ∗:~(U,E)⟶~(W,H) be two fuzzy soft functions, then the composition φ∗ψ∗∘φψ is fuzzy soft almost β-continuous if φψ is fuzzy soft β-continuous (resp., β-irresolute and β-continuous) and φ∗ψ∗ is fuzzy soft almost continuous (resp., almost β-continuous and continuous).
Proof. Follows from the above definitions.
Definition 4.2. Let (V,ηF) and (U,τE) be FSTSs and r∈Io. A fuzzy soft function φψ:~(V,F)⟶~(U,E) is said to be a fuzzy soft β-irresolute open (resp., closed) if φψ(fA) is an r-fuzzy soft β-open (resp., β-closed) set for each fA∈~(V,F) which is an r-fuzzy soft β-open (resp., β-closed) set.
Theorem 4.3. Let (V,ηF) and (U,τE) be FSTSs and φψ:~(V,F)⟶~(U,E) be a fuzzy soft function. The following statements are equivalent for each fA∈~(V,F), gB∈~(U,E), k∈F, (e=ψ(k))∈E, and r∈I∘:
(1) φψ is fuzzy soft β-irresolute open.
(2) φψ(βIη(k,fA,r))⊑βIτ(e,φψ(fA),r).
(3) βIη(k,φ−1ψ(gB),r)⊑φ−1ψ(βIτ(e,gB,r)).
(4) For each gB and each fA r-fuzzy soft β-closed with φ−1ψ(gB)⊑fA, hC∈~(U,E) is r-fuzzy soft β-closed with gB⊑hC such that φ−1ψ(hC)⊑fA.
Proof. (1) ⇒ (2) Since φψ(βIη(k,fA,r))⊑φψ(fA), by (1), φψ(βIη(k,fA,r)) is r-fuzzy soft β-open. Hence, φψ(βIη(k,fA,r))⊑βIτ(e,φψ(fA),r).
(2) ⇒ (3) Put fA=φ−1ψ(gB). By (2), φψ(βIη(k,φ−1ψ(gB),r))⊑βIτ(e,φψ(φ−1ψ(gB)),r)⊑βIτ(e,gB,r), then βIη(k,φ−1ψ(gB),r)⊑φ−1ψ(βIτ(e,gB,r)).
(3) ⇒ (4) Let gB∈~(U,E) and fA∈~(V,F) be an r-fuzzy soft β-closed with φ−1ψ(gB)⊑fA. Since fcA⊑φ−1ψ(gcB), fcA=βIη(k,fcA,r)⊑βIη(k,φ−1ψ(gcB),r). Hence, by (3), fcA⊑βIη(k,φ−1ψ(gcB),r)⊑φ−1ψ(βIτ(e,gcB,r)). Thus, fA⊒(φ−1ψ(βIτ(e,gcB,r)))c=φ−1ψ(βCτ(e,gB,r)). Hence, βCτ(e,gB,r)∈~(U,E) is r-fuzzy soft β-closed with gB⊑βCτ(e,gB,r) such that φ−1ψ(βCτ(e,gB,r))⊑fA.
(4) ⇒ (1) Let wD∈~(V,F) be r-fuzzy soft β-open. Put gB=(φψ(wD))c and fA=wcD, φ−1ψ(gB)=φ−1ψ((φψ(wD))c)⊑fA. Hence, by (4), hC∈~(U,E) is r-fuzzy soft β-closed with gB⊑hC such that φ−1ψ(hC)⊑fA=wcD. Thus, φψ(wD)⊑φψ(φ−1ψ(hcC))⊑hcC. On the other hand, since gB⊑hC, φψ(wD)=gcB⊒hcC. Thus, φψ(wD)=hcC, so φψ(wD) is r-fuzzy soft β-open, then φψ is fuzzy soft β-irresolute open.
In a similar way, we can prove the following theorem.
Theorem 4.4. Let (V,ηF) and (U,τE) be FSTSs and φψ:~(V,F)⟶~(U,E) be a bijective fuzzy soft function. The following statements are equivalent for each fA∈~(V,F), gB∈~(U,E), k∈F, (e=ψ(k))∈E, and r∈I∘:
(1) φψ is fuzzy soft β-irresolute closed.
(2) βCτ(e,φψ(fA),r)⊑φψ(βCη(k,fA,r)).
(3) φ−1ψ(βCτ(e,gB,r))⊑βCη(k,φ−1ψ(gB),r).
(4) For each gB and each fA r-fuzzy soft β-open with φ−1ψ(gB)⊑fA, hC∈~(U,E) is r-fuzzy soft β-open with gB⊑hC such that φ−1ψ(hC)⊑fA.
Proposition 4.3. Let (V,ηF) and (U,τE) be FSTSs and φψ:~(V,F)⟶~(U,E) be a bijective fuzzy soft function. φψ is fuzzy soft β-irresolute closed if φψ is fuzzy soft β-irresolute open.
Proof. It is easily proved from:
φ−1ψ(βCτ(e,gB,r))⊑βCη(k,φ−1ψ(gB),r)
⟺φ−1ψ(βIτ(e,gcB,r))⊒βIη(k,φ−1ψ(gcB),r).
Definition 4.3. Let (V,ηF) and (U,τE) be FSTSs. A fuzzy soft function φψ:~(V,F)⟶~(U,E) is said to be a fuzzy soft β-irresolute homeomorphism if φψ is bijective and both of φψ and φ−1ψ are β-irresolute.
From the above theorems, we obtain the following corollary.
Corollary 4.1. Let (V,ηF) and (U,τE) be FSTSs and φψ:~(V,F)⟶~(U,E) be a bijective fuzzy soft function. The following statements are equivalent for each fA∈~(V,F), gB∈~(U,E), k∈F, (e=ψ(k))∈E, and r∈I∘:
(1) φψ is fuzzy soft β-irresolute homeomorphism.
(2) φψ is fuzzy soft β-irresolute and fuzzy soft β-irresolute open.
(3) φψ is fuzzy soft β-irresolute and fuzzy soft β-irresolute closed.
(4) φψ(βIη(k,fA,r))=βIτ(e,φψ(fA),r).
(5) φψ(βCη(k,fA,r))=βCτ(e,φψ(fA),r).
(6) βIη(k,φ−1ψ(gB),r)=φ−1ψ(βIτ(e,gB,r)).
(7) βCη(k,φ−1ψ(gB),r)=φ−1ψ(βCτ(e,gB,r)).
5.
Conclusions and future work
This article is laid out as follows:
(1) In Section 2, fuzzy soft β-closure (β-interior) operators are introduced and studied in FSTSs based on the article by Aygünoǧlu et al. [25]. Moreover, the concept of r-fuzzy soft β-connected sets is defined and characterized.
(2) In Section 3, some properties of a fuzzy soft β-continuity are investigated. As a weaker form of the notion of fuzzy soft β-continuous functions, the notions of fuzzy soft almost (weakly) β-continuous functions are introduced and some properties are obtained. Also, we show that fuzzy soft β-continuity ⇒ fuzzy soft almost β-continuity ⇒ fuzzy soft weakly β-continuity, but the converse may not be true. Furthermore, we have the following results:
● Fuzzy soft (idV,Cη(Iη(Cη)),idU,idU)-continuous function is fuzzy soft β-continuous.
● Fuzzy soft (idV,βIη,Iτ(Cτ),idU)-continuous function is fuzzy soft almost β-continuous.
● Fuzzy soft (idV,βIη,Cτ,idU)-continuous function is fuzzy soft weakly β-continuous.
(3) In Section 4, the notions of fuzzy soft β-irresolute (resp., strongly β-irresolute, β-irresolute open, β-irresolute closed, and β-irresolute homeomorphism) functions are introduced between two FSTSs (V,ηF) and (U,τE), and some properties of these functions are investigated. Additionally, we show that fuzzy soft strongly β-irresolute ⇒ fuzzy soft β-irresolute ⇒ fuzzy soft β-continuity, but the converse may not be true.
In upcoming manuscripts, we shall investigate the notions given here in the frames of fuzzy soft r-minimal structures [28]. Also, we will use the fuzzy soft β-closure operator to introduce some new separation axioms on FSTS based on the article by Aygünoǧlu et al. [25].
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Conflict of interest
The authors declare that they have no conflicts of interest.









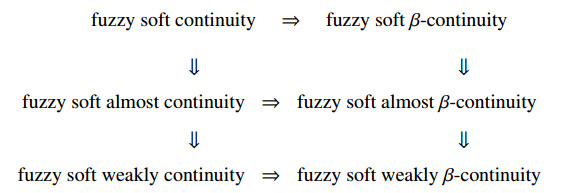




 DownLoad:
DownLoad: