Fractional tempered differential equations depending on arbitrary kernels

1.   Introduction

In this present paper, we consider the tempered fractional differential system (FDS) made of the fractional differential equation

subject to the initial conditions

where ${{^C\mathbb{D}_{a+}^{{\gamma}, {\lambda}, g}}}(\cdot)$ is the tempered Caputo fractional derivative of order $\gamma\in (n-1, n]$, for a given $n\in\mathbb N$, with respect to the smooth kernel $g$, where $\lambda\geq0$.

The study of fractional differential equations is an important topic and has attracted a large number of researchers to this field. By considering differential equations, where the differential operator has an arbitrary real positive order, we gain a more realist description of many life phenomena. For example, we mention applications in physics ^[1,2], control theory ^[3], finance ^[4], electrical engineering ^[5], optics ^[6], medicine ^[7,8], epidemiology ^[9], etc. Due to the complexity of many systems, different fractional operators are used in order to obtain a better description of the studied object. Thus, we believe it is important to present new results involving general forms of fractional derivatives, in a way that we may later consider particular cases of such operators depending on the given system.

Over the last few years, significant research on properties like the existence, uniqueness, stability, and attractivity of solutions of fractional differential equations has been done. This is due to the fact that fractional calculus is a consolidated field, where new formulations of fractional derivatives and fractional integrals are studied. This allows researchers to address new types of problems and even problems that have already been discussed via classical derivatives (ordinary and partial). What is expected when investigating a certain property of solutions of a fractional differential equation is that, in the end, new results are compared with the integer case and it is possible to highlight the novelties and advantages.

In 2019, Li et al. ^[10], motivated by biological questions and Jacobi's predictor-correction, investigated the well-posedness and numerical algorithm for a class of tempered fractional ordinary differential equations. At the time, the authors investigated such properties in the sense of tempered fractional derivatives of Caputo and Riemann-Liouville.

In 2020, Obeidat et al. ^[11] developed the theory, properties, and applications of a new technique in tempered fractional calculus called the tempered fractional natural transform method. In this work, they tackled a problem of tempered fractional linear and nonlinear ordinary and partial differential equations in Caputo and Riemann-Liouville senses. We can also mention the important work discussed by Sultana et al. ^[12], which deals with a class of tempered fractional integro-differential equations of the Caputo type, and a comparative study of three numerical schemes: linear, quadratic, and quadratic-linear schemes. There are numerous other works on fractional differential equations in the sense of tempered derivatives. However, the majority of them only address classical tempered fractional derivatives.

Motivated by the works above, in order to discuss and propose new results for fractional calculus and the theory of fractional differential equations in the "tempered" sense, in this paper we extend the notions of the tempered fractional derivatives in the Riemann-Liouville and Caputo senses to a new class of fractional operators, and investigate the existence and uniqueness of the solution for the fractional tempered differential system (1.1)–(1.2) in $[a, b]$. On the other hand, we also investigate the attractivity of solutions for (1.1) and (1.2) in $[0, \infty)$ through the Schauder fixed point theorem, Arzelà-Ascoli theorem, and Hausdorff measure of non-compactness.

A natural and expected consequence in the theory of fractional calculus is that the integer case is obtained when ${\gamma} = n\in\mathbb N$. In fact, with the new extensions of the Riemann-Liouville tempered fractional integral and the Caputo and Riemann-Liouville tempered fractional derivatives with respect to another function presented here, we can recover the integer case. On the other hand, it is worth noting that, from the particular choice of function $g$ and parameter $\lambda$ in the Caputo and Riemann-Liouville tempered fractional derivatives with respect to another function, it is possible to obtain numerous particular cases, according to ^{[10,13,14,15,16,17,18,19]} and the references therein.

The primary contributions of this work are outlined as follows:

$(1)$ We establish the existence and uniqueness of solutions for a system of fractional differential equations, subject to initial conditions, under the general form of a fractional derivative.

$(2)$ We illustrate the convergence of Picard's method, demonstrating its effectiveness in solving the aforementioned system.

$(3)$ We investigate the stability of the system, considering variations in the functions, initial conditions, or fractional order.

$(4)$ Under additional assumptions, we provide a proof of the existence of at least one attractive solution within the system.

The structure of the paper is as follows: in Section 2 we introduce the new notions of fractional tempered derivatives with respect to an arbitrary smooth kernel in the Riemann-Liouville and Caputo senses and establish some properties that are useful for the proofs of our results. In Section 3 we prove the results on the existence and uniqueness of the solution of a fractional differential system involving the Caputo fractional tempered derivative with respect to an arbitrary smooth kernel and we analyze the continuous data dependence of the Cauchy problem solution. Section 4 provides conditions that guarantee that the fractional problem under study admits at least one attractive solution. We finalize the paper with a conclusion section.

2.   Preliminaries

Motivated by the concept of fractional derivatives with respect to another function ^{[13,15,16,18,20]} and tempered fractional derivatives ^[10,14,17], we present new definitions of fractional operators combining these two ideas.

Let ${\lambda} \ge0$, $n\in\mathbb N$, $\gamma\in (n-1, n]$, and $g\in C^n([a, b], \mathbb R)$, such that $g'(t) > 0$ for all $t\in [a, b]$.

For a given integrable function $u\in L^1([a, b], \mathbb R)$, its tempered (left-sided) fractional integral of order ${\gamma}$ with respect to the function $g$ is defined as

where $\Gamma$ denotes the Gamma function and ${\mathbb{I}_{a+}^{{\gamma}, g}}$ is the fractional integral of order ${\gamma}$ with respect to the function $g$ (cf. ^[15]). In what follows, ${\mathbb{D}_{a+}^{{\gamma}, g}}$ and ${^C\mathbb{D}_{a+}^{{\gamma}, g}}$ represent the (left-sided) Riemann-Liouville and Caputo fractional derivatives of order ${\gamma}$ with respect to the kernel $g$, respectively (cf. ^[13]). The tempered (left-sided) Riemann-Liouville and Caputo fractional derivatives of order ${\gamma}$ with respect to the kernel $g$ are defined, respectively, as

where $n = [{\gamma}]+1,$ and

where $n = [{\gamma}]+1$ if ${\gamma}\notin\mathbb N$, and $n = {\gamma}$ if ${\gamma}\in\mathbb N$. Thus, given ${\gamma}\notin\mathbb N$,

and for ${\gamma}\in\mathbb N$,

Observe that

and that

By Lemmas 1 and 2 from ^[13], we may conclude that

and

where $E_{\gamma}$ denotes the one parameter Mittag-Leffler function

The class of functions that we will consider is the set of absolute continuous functions of order $n$ defined as

Starting with the formula (cf. ^[13, Theorem 3])

multiplying both members of the last equality by ${\mbox{e}}^{-{\lambda} t}$, we deduce that

and so, if

then

Theorem 2.1. If $u\in AC([a, b], \mathbb R)$, then

and if $u\in AC_g^n([a, b], \mathbb R)$, then

Proof. The formulae are a direct consequence of Theorems 4 and 5 from ^[13]. □

3.   Tempered fractional differential systems

Consider the tempered fractional differential system (FDS):

with

where $n = [{\gamma}]+1$ if ${\gamma}\notin\mathbb N$ and $n = {\gamma}$ if ${\gamma}\in\mathbb N$, $u\in AC_g^n([a, b], \mathbb R)$, $f:[a, b]\times \mathbb R\to\mathbb R$ is a continuous function, and $u^k$ are the initial values, for $k\in\{0, 1, \ldots, n-1\}$.

Theorem 3.1. Function $u$ is a solution of the fractional differential system (3.1)–(3.2) if and only if

Proof. The proof is a direct consequence of Theorem 2.1 and the formula

□

Next, we prove that under certain assumptions, the initial value problem (3.1)–(3.2) has a unique solution.

Theorem 3.2. Suppose that:

$(1)$ there exists a positive constant $M$ such that

$(2)$ there exists a positive real $\epsilon$ such that $[a, a+\epsilon]\subseteq [a, b]$ and

Then, problem (3.1)–(3.2) has a unique solution on the space $AC^n_g([a, a+\epsilon], \mathbb R)$.

Proof. Define $F:AC^n_g([a, a+\epsilon], \mathbb R)\to AC^n_g([a, a+\epsilon], \mathbb R)$ as

Function $F$ is well-defined since

Also, $F$ is a contraction map since, given two functions $u_1, u_2 \in AC^n_g([a, a+\epsilon], \mathbb R)$,

Then, the desired result follows from the Banach fixed point theorem. □

We remark that we can actually prove that there exists a global solution for the Cauchy problem (3.1)–(3.2). In fact, let $u_1^\star$ be the local solution on the interval $[a, a+\epsilon_1]$. Using the same ideas, we can prove the existence and uniqueness of a solution $u_2^\star$ on an interval $[a+\epsilon_1, a+\epsilon_2]\subseteq[a, b]$. Repeating the procedure, we obtain at the end the global solution $u:[a, b]\to\mathbb R$.

Theorem 3.3. Suppose that there exists a positive constant $M$ such that

Define the sequence of functions $u_m:[a, b]\to\mathbb R$ by

and

Then, the sequence $(u_m)_m$ converges uniformly to a function $u$ that is a solution of the Cauchy problem (3.1)–(3.2). Moreover,

Proof. First, we prove using mathematical induction that, for all $m\in\mathbb N_0$,

For $m = 0$ the proof is obvious. On the other hand, for each $m\in\mathbb N_0$, and recalling the following property of the Beta function:

we obtain

which ends the proof for the desired formula. Thus, for all $t\in[a, b]$ and for all $m\in\mathbb N_0$,

and so, applying the Weierstrass M-test, we conclude that the series $\sum_{m = 0}^\infty (u_{m+1}(t)-u_m(t))$ is uniformly convergent on the interval $[a, b]$. Let $u:[a, b]\to\mathbb R$ be the function

Observe that for each $j\in\mathbb N$,

Since

we conclude that the sequence of functions $(f(\cdot, u_j(\cdot)))_j$ also converges uniformly to $f(\cdot, u(\cdot))$. From (3.3), and doing $m\to\infty$, we conclude that $u$ is a solution for the Cauchy problem (3.1)–(3.2).

To prove the last formula, simply observe that

□

Example 3.1. Consider the following fractional differential system:

The solution is the function

The Picard's iterations are

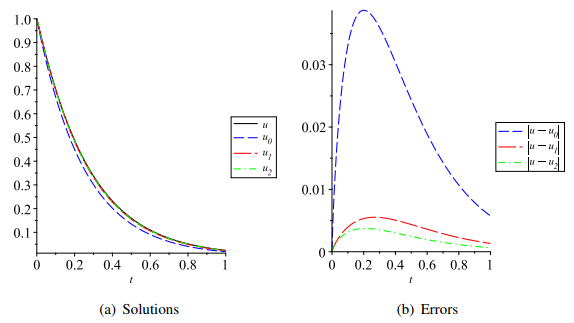
In Figure 1 we compare the exact solution with the first three Picard's iterations (Figure 1a) and the obtained error $|u(t)-u_j(t)|$, $j = 0, 1, 2$ (Figure 1b). The kernel is the function $g(t) = \sqrt{t+1}$, $t\in[0, 1]$.

The following two theorems show that, under certain assumptions, small perturbations in the initial data do not significantly affect the solution of the fractional tempered differential system (3.1)–(3.2).

Theorem 3.4. Let $f, h:[a, b] \times \mathbb{R} \to \mathbb{R}$ be two continuous functions and $u, v \in AC_g^n([a, b], \mathbb R)$ be two functions such that

and

where $u^k, v^k$, $k\in\{0, 1, \ldots, n-1\}$ are given real numbers. If

$(1)$ there exists a real $L > 0$ such that

$(2)$ there exists a continuous function $\eta: [a, b] \to \mathbb{R}^+_0$ such that

then

where

Proof. By Theorem 3.1 we conclude that

and

Observe that

Denoting $w(t): = |u(t)-v(t)|,$ we get

and, therefore,

Using the Gronwall inequality ^[21] we conclude that

proving the desired inequality:

□

Remark 3.1. We remark that:

$(1)$ If $\lambda = 0$, Theorem 3.4 reduces to Theorem 6 of ^[22].

$(2)$ Under the hypotheses of Theorem 3.4, we deduce the following two inequalities that are useful in the proof of Corollarys 3.1 and 3.2.

(a) Since

then

(b) Since

then

The following result shows that small changes in the initial conditions of the tempered fractional initial value problem (3.1)–(3.2) lead to small changes in the solution.

Corollary 3.1. Suppose that the assumptions of Theorem 3.4 hold. If $f = h$, then for all $t \in [a, b]$,

Proof. If $f = h$, then we may take $\eta(t) = 0$, for all $t\in [a, b]$. From inequalities (3.5) and (3.6) it follows that

proving the desired result. □

The next result shows that if the two systems of Theorem 3.4 have the same initial conditions and functions $f$ and $h$ are arbitrarily close, then the respective solutions are also arbitrarily close.

Corollary 3.2. Suppose that the assumptions of Theorem 3.4 hold. If $u^k = v^k$, $k = 0, 1, \ldots, n-1$, then for all $t \in [a, b]$,

Proof. From inequalities (3.5) and (3.6) it follows that

as desired. □

To finalize this section, we show the dependence of the solution of the fractional differential system (3.1)–(3.2) on the order of the fractional derivative.

Theorem 3.5. Let $f:[a, b] \times \mathbb{R} \to \mathbb{R}$ be a continuous function such that

Let $\epsilon\in (0, {\gamma}-n+1)$ and $u^k, v^k$, $k\in\{0, 1, \ldots, n-1\}$ be fixed real numbers. Suppose that $u \in AC^n_g([a, b], \mathbb R)$ is such that

and $v \in AC^n_g([a, b], \mathbb R)$ satisfies the system

Then, for all $t\in [a, b]$,

where

Proof. From Theorem 3.1 it follows that

Since

we can conclude that

and, therefore,

Applying the Gronwall inequality ^[21] we get

as desired. □

Now, we present an example that illustrates the usefulness of Theorem 3.5.

Example 3.2. Let ${\gamma} = n\in \mathbb N$ and $f(t, u) = u$, for all $t\in [0, 1]$. Clearly, $f$ satisfies the condition of Theorem 3.5. Let $\epsilon \in (0, 1)$ be fixed. For the following two fractional differential systems

one gets

It is clear that for each $t\in[0, 1]$, $\beta(t) \to 0$ when $\epsilon\to 0$. Therefore, by Theorem 3.5, we may conclude that $|u(t)-v(t)|\to 0$ when $\epsilon\to 0$, for all $t\in[0, 1]$, proving that for small parameter $\epsilon$ the solution of the ordinary differential system (3.7) approximates the solution to the fractional tempered differential system (3.8).

4.   Attractivity of solutions

In this section, we investigate the attractivity of solutions of the fractional tempered differential equation

subject to the initial conditions

where $n-1 < {\gamma} < n$, $u\in AC_{g}^{n}([0, \infty), \mathbb R)$, and $f:[0, \infty)\times \mathbb{R}\rightarrow \mathbb{R}$ is a continuous function. We will assume, from now on, that the function $g$ is unbounded from above and that

Definition 4.1. ^[23] A solution $u$ of the fractional tempered system (4.1)–(4.2) are called attractive if $u(t)\to0$ as $t\to\infty$.

Function $u$ is a solution of the fractional tempered differential system (4.1)–(4.2) if and only if

To investigate the attractivity of a solution, we will first present the following hypotheses, namely:

${\bf (H_{1})}$ there exist $L\geq0$, $\delta > 0$, and $\beta\in(\gamma, n)$ such that

for all $t\in \left(0, \infty \right)$ and for all $u\in \mathbb{R}$;

${\bf (H_{2})}$ there exists a constant $\kappa > 0$ such that for any bounded set $E\subset \mathbb{R}$,

for $t > 0$ and $\mu (\cdot)$ is the Hausdorff measure of non-compactness.

For any $u\in C([0, \infty), \mathbb{R})$ and given $m\in \mathbb{N},$ define the operator ${\bf A}$ as follows:

for $t\in \lbrack 0, \infty).$

Since $n-1 < \gamma < \beta < n$, we can choose a $\widetilde{\gamma } > 0$ sufficiently small and $\delta\in\mathbb R$ such that

Let $T > 0$ such that

for $t\geq T.$

Define the set $\mathbb{S}_{g}^{\widetilde{\gamma }}$ as follows:

Note that $\mathbb{S}_{g}^{\widetilde{\gamma }}\neq\emptyset$ and $\mathbb{S}_{g}^{\widetilde{\gamma }}$ is a closed, convex, and bounded subset of $C\left([0, \infty), \mathbb{R}\right)$.

Next we present some auxiliary lemmas, needed for our main result of this section. Let $\Omega$ be a Banach space.

Lemma 4.1. ^[24] The set $\mathcal{H}\subset C([0, \infty), \Omega)$ is relatively compact if and only if the following conditions hold:

(1) for any $K > 0$, the functions in $\mathcal{H}$ are equicontinuous on $[0, K]$;

(2) for any $t\in [0, \infty)$, $\mathcal{H}(t) = \left\{u(t):u\in \mathcal{H}\right\}$ is relatively compact in $\Omega$;

(3) $\lim_{t\rightarrow \infty}|u(t)| = 0$ uniformly for $u\in \mathcal{H}$.

Lemma 4.2. Assume that $({\bf H}_{1})$ holds. Then $\{{\bf A}u:u\in \mathbb{S}_{g}^{\widetilde{\gamma }}\}$ is equicontinuous and $\underset{t\rightarrow \infty }{\lim }|\left({\bf A}u\right) \left(t\right) | = 0$ uniformly for $u\in \mathbb{S}_{g}^{\widetilde{ \gamma }}.$

Proof. Let $\varepsilon > 0$. Since $\gamma -\beta -\widetilde{\gamma }\delta < 0$, there exists a sufficiently large $T_{1} > 0$ such that

and

for $t\geq T_{1}$. For any $u\in \mathbb{S}_{g}^{\widetilde{\gamma }}$ and $t_{1}, t_{2}\geq T_{1}$ yields

Then, we have

On the other hand, for $0\leq t_{1} < t_{2}\leq T_{1}$ yields

Clearly, as $t_2\to t_1$, the first modulus of the right hand side goes to zero, and $e^{-\lambda t_{2}} \to e^{-\lambda t_{1}}$. So, to prove the desired lemma, it is enough to prove that

as $t_2\to t_1$. Let

Since

which goes to zero as $t_{2}\rightarrow t_{1}$, it is enough to prove that, in the limit,

that is,

This fact can be proven observing that

Similarly, for $T_{1}\in(t_{1}, t_{2})$, we get

as $t_{2}\rightarrow t_{1}$.

Thus, $\left\{ {\bf A}u:u\in \mathbb{S}_{g}^{\widetilde{\gamma }}\right\}$ is equicontinuous. Now, we show $\underset{t\rightarrow \infty }{\lim }\left\vert ({\bf A}u)(t)\right\vert = 0$ uniformly for $u\in \mathbb{S}_{g}^{\widetilde{\gamma }}$. Indeed, we have

which goes to zero for $t\rightarrow \infty$.

Therefore, $\underset{t\rightarrow \infty }{\lim }\left\vert ({\bf A}u)(t)\right\vert = 0$ uniformly for $u\in \mathbb{S}_{g}^{\widetilde{\gamma }}$. □

Lemma 4.3. Suppose $({\bf H}_{1})$ holds. Then, ${\bf A}$ maps $\mathbb{S}_{g}^{\widetilde{\gamma }}$ into $\mathbb{S}_{g}^{\widetilde{\gamma }}$ and is continuous on $\mathbb{S}_{g}^{\widetilde{\gamma }}.$

Proof. The proof of this result will be presented in two steps.

Step 1. ${\bf A}$ takes $\mathbb{S}_{g}^{\widetilde{\gamma }}$ into $\mathbb{S}_{g}^{\widetilde{\gamma }}$.

For $u\in \mathbb{S}_{g}^{\widetilde{\gamma }}$ and using Lemma 4.1, it follows that ${\bf A}u\in C([0, \infty), \mathbb{R})$. Using the same argument as the inequality (4.5), we conclude that that

Then, choosing $T_1 > 0$ sufficiently large, from the previous inequality we conclude that

for $t\geq T_1$.

Thus, we have $|(g(t)-g(0))^{\widetilde{\gamma }}{\bf A}u(t)|\leq 1$, so ${\bf A}\mathbb{S}_{g}^{\widetilde{\gamma }}\subset \mathbb{S}_{g}^{\widetilde{\gamma }}$.

Step 2. $A$ is continuous on $\mathbb{S}_{g}^{\widetilde{\gamma }}$.

Now, for $u_{j}, u\in \mathbb{S}_{g}^{\widetilde{\gamma }}$, $j = 1, 2, 3, ...,$ with $\underset{i\rightarrow \infty }{\lim }u_{j} = u$ we prove ${\bf A}u_{j}\rightarrow {\bf A}u$ as $j\rightarrow \infty$. For all $\varepsilon > 0$, there exists $T_{2} > 0$ large enough such that, for all $t \geq T_2$,

and

Then, for $t > T_{2}$, the following relation holds:

For $0 < t\leq T_{2}$, we have that

Applying the limit with $j\rightarrow \infty$ on both sides of Eq (4.6) and using the Lebesgue dominated convergence theorem yields $|\left({\bf A}u_{j}(t)-{\bf A}u(t)\right) |\rightarrow 0.$ Thus $\left\Vert {\bf A}u_{j}-{\bf A}u\right\Vert\rightarrow 0$ as $j\rightarrow \infty$ so ${\bf A}$ is continuous. □

We are now able to present the main result of this section, which ensures that our problem has at least one attractive solution, under some conditions.

Theorem 4.1. Assume $({\bf H}_{1})$ and $({\bf H}_{2})$ hold. Then, the tempered fractional problem (4.1)–(4.2) admits at least one attractive solution.

Proof. First, note that ${\bf A}:\mathbb{S}_{g}^{\widetilde{\gamma }}\rightarrow \mathbb{S}_{g}^{\widetilde{\gamma }}$ is bounded and continuous (see Lemma 4.3). Furthermore, we know that $\left\{ {\bf A}u:u\in \mathbb{S}_{g}^{\widetilde{\gamma } }\right\}$ is equicontinuous and $\underset{t\rightarrow \infty }{\lim } |{\bf A}u(t)| = 0$ uniformly for $u\in \mathbb{S}_{g}^{\widetilde{\gamma }}$ (see Lemma 4.2). It remains to be verified that for any $t\in \lbrack 0, \infty)$, $\left\{{\bf A}u:u\in \mathbb{S}_{g}^{\widetilde{\gamma }}\right\}$ is relatively compact in $\mathbb{R}$ by using $({\bf H}_{2})$ (see ^[24]). Therefore, by Schauder fixed point theorem, the operator ${\bf A}$ has a fixed point $u_{m}\in \mathbb{S}_{g}^{\widetilde{\gamma }}$ with $u_{m}(t)\rightarrow 0$ as $t\rightarrow \infty$. Applying a similar method as the one given in ^[24], we can prove that $(u_{m}(t))_m$ is relatively compact. Using the Arzelà-Ascoli theorem ^[25], $(u_{m}(t))_m$ has a uniformly convergent subsequence $(u_{m_{p}})_p$. Moreover, $(u_{m_{p}})_p$ satisfies

Let $u^{\ast }(t) = \underset{p\rightarrow \infty }{\lim }u_{m_{p}}(t),$ $(t\neq 0)$. The Lebesgue dominated theorem with Eq (4.7) yields

with $t\in \lbrack 0, \infty)$, so $u^{\ast }$ is an attractive solution for the tempered fractional problem. □

5.   Conclusions

In this work we studied fractional differential equations in the presence of a generalized fractional derivative, which involves an arbitrary kernel $g$ and an exponential decay. Using the Banach fixed point theorem, an existence and uniqueness result is obtained. Moreover, a numerical procedure to determine approximations of the solution is given, based on Picard's iterations. The stability of solutions is considered, as an application of the considered fractional Gronwall inequality. We end with the study of the attractivity of solutions of the fractional differential system. After proving some auxiliary lemmas, we deduce the desired result as a consequence of the Schauder fixed point theorem and the Arzelà-Ascoli theorem.

As a potential direction for future research, it would be valuable to investigate numerical algorithms designed to handle such derivatives. While numerical methods exist to address problems involving fractional derivatives with respect to an arbitrary kernel $g$, to the best of our knowledge, a general procedure for dealing with this generalized tempered fractional derivative remains absent.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

R. Almeida and N. Martins are supported by Portuguese funds through the CIDMA - Center for Research and Development in Mathematics and Applications, and the Portuguese Foundation for Science and Technology (FCT-Funda\c{c}ão para a Ciência e a Tecnologia), within project UIDB/04106/2020, with DOI 10.54499/UIDB/04106/2020.

Conflict of interest

Prof. Ricardo Almeida and Prof. Natália Martins are the Guest Editors of special issue "Advances in Fractional Calculus: Theory and Applications" for AIMS Mathematics. Prof. Ricardo Almeida and Prof. Natália Martins were not involved in the editorial review and the decision to publish this article. All authors declare no conflicts of interest in this paper.