Some Tsallis entropy measures in concomitants of generalized order statistics under iterated FGM bivariate distribution

1.   Introduction and main results

A complex exponential system is defined as $E(\Lambda) : = \{e^{2\pi i \lambda (\cdot)} : \lambda\in\Lambda\}$ on a measurable set $S \subset \mathbb R$ called a domain, where $\Lambda \subset \mathbb R$ is a discrete set called a frequency set or a spectrum.

Despite the simple formulation of exponential systems, the existence of exponential Riesz bases for a given domain $S$ is a highly nontrivial problem. In 1995, Seip ^[26] proved that for any interval $S \subset [0, 1)$, there exists a set $\Lambda \subset \mathbb{Z}$ such that $E(\Lambda)$ is a Riesz basis for $L^2(S)$. Only recently in 2015, Kozma and Nitzan ^[12] showed that for any finite union $S$ of disjoint intervals in $[0, 1)$, there exists a set $\Lambda \subset \mathbb{Z}$ such that $E(\Lambda)$ is a Riesz basis for $L^2(S)$. To date, there are only a handful of classes of domains for which exponential Riesz bases are known to exist; see, e.g., ^{[2,3,6,8,11,12,16,17,18,20,25]}. Recently, Kozma, Nitzan, and Olevskii ^[13] constructed a bounded measurable set $S \subset \mathbb R$ such that no exponential system can be a Riesz basis for $L^2(S)$.

Aside from the question of existence of exponential Riesz bases, one may also ask whether frequency sets and domains of exponential Riesz bases can be combined or split in a canonical way.

Question 1 (Combining exponential Riesz bases). Let $S_1, S_2 \subset \mathbb R$ be disjoint measurable sets and let $\Lambda_1, \Lambda_2 \subset \mathbb R$ be discrete sets with $\mathrm{dist} (\Lambda_1, \Lambda_2) : = \inf \{ | \lambda_1 - \lambda_2 | : \lambda_1 \in \Lambda_1, \, \lambda_2 \in \Lambda_2 \} > 0$. Assume that for each $n = 1, 2$, the system $E(\Lambda_n)$ is a Riesz basis for $L^2(S_n)$. Is $E(\Lambda_1 \cup \Lambda_2)$ then a Riesz basis for $L^2(S_1 \cup S_2)$?

Note that we have excluded the case $\mathrm{dist} (\Lambda_1, \Lambda_2) = 0$ from consideration. This case can be divided into two sub-cases:

(ⅰ) $\Lambda_1 \cap \Lambda_2 \neq \emptyset$.

(ⅱ) $\Lambda_1 \cap \Lambda_2 = \emptyset$, and there exist sequences $\{ \lambda_{1, k} \}_{k = 1}^\infty \subset \Lambda_1$ and $\{ \lambda_{2, k} \}_{k = 1}^\infty \subset \Lambda_2$ such that $| \lambda_{1, k} - \lambda_{2, k} | \rightarrow 0$ as $k \rightarrow \infty$.

Certainly, we do not want the frequency sets $\Lambda_1$ and $\Lambda_2$ to intersect, since those elements lying in the intersection would be counted only once in $\Lambda_1 \cup \Lambda_2$. On the other hand, (ⅱ) would imply that $\Lambda_1 \cup \Lambda_2$ is a non-separated set, in which case $E(\Lambda_1 \cup \Lambda_2)$ is not even a Riesz sequence. This justifies the assumption $\mathrm{dist} (\Lambda_1, \Lambda_2) > 0$ in Question 1.

Question 2 (Splitting exponential Riesz bases). Let $S_1, S_2 \subset \mathbb R$ be disjoint measurable sets. If $\Lambda_1, \Lambda_2 \subset \mathbb R$ are disjoint sets such that $E(\Lambda_2)$ and $E(\Lambda_1 \cup \Lambda_2)$ are Riesz bases for $L^2(S_2)$ and $L^2(S_1 \cup S_2)$ respectively, then is the system $E(\Lambda_1)$ a Riesz basis for $L^2(S_1)$?

Unfortunately, both questions turn out negative in general.

For Question 1, note that $E(4 \mathbb{Z} \cup (4 \mathbb{Z}{+}2))$ is not a Riesz basis for $L^2([0, \frac{1}{4}) \cup [\frac{2}{4}, \frac{3}{4}))$, even though $E(4 \mathbb{Z})$ and $E(4 \mathbb{Z}{+}2)$ form Riesz bases for $L^2[0, \frac{1}{4})$ and $L^2[\frac{2}{4}, \frac{3}{4})$ respectively. However, it can be amended to form a Riesz basis by slightly shifting one of the frequency sets. Indeed, for every $0 < \delta < 2$, the system $E(4 \mathbb{Z} \cup (4 \mathbb{Z}{+}2{+}\delta))$ is a Riesz basis for $L^2([0, \frac{1}{4}) \cup [\frac{2}{4}, \frac{3}{4}))$ while each of $E(4 \mathbb{Z})$ and $E(4 \mathbb{Z}{+}2{+}\delta)$ forms a Riesz basis for $L^2[0, \frac{1}{4})$ and $L^2[\frac{2}{4}, \frac{3}{4})$ respectively. See Example 2.6 below for more details.

We also provide an example which cannot be amended by shifting the frequency sets as above. Fix any $0 < \epsilon < \frac{1}{4}$ and let

Then, $E(\Lambda^{(1)})$, $E(\Lambda^{(2)})$ and $E(\Lambda^{(1)}\cup \Lambda^{(2)})$ form Riesz bases for $L^2[0, \frac{1}{2})$, $L^2[\frac{1}{2}, 1)$ and $L^2[0, 1)$ respectively, despite the fact that $\Lambda^{(1)} \cap \Lambda^{(2)} = \{ 0 \}$. To separate the frequency sets apart, we consider a uniform shifting of $\Lambda^{(2)}$. For any $0 < \delta < \epsilon$, we have $\mathrm{dist} (\Lambda^{(1)}, \Lambda^{(2)} {+} \delta) = \delta > 0$, and each of $E(\Lambda^{(1)})$ and $E(\Lambda^{(2)} {+} \delta)$ forms a Riesz basis for $L^2[0, \frac{1}{2})$ and $L^2[\frac{1}{2}, 1)$ respectively. However, their union $E(\Lambda^{(1)} \cup (\Lambda^{(2)} {+} \delta))$ is overcomplete in $L^2[0, 1)$ and thus cannot be a Riesz basis for $L^2[0, 1)$. See Example 2.7 below for more details.

Concerning Question 2, the sets $\Lambda_1 : = \Lambda^{(1)} \backslash \{ 0 \}$ and $\Lambda_2 : = \Lambda^{(2)}$ are disjoint and satisfy that $E(\Lambda_2)$ and $E(\Lambda_1 \cup \Lambda_2)$ are Riesz bases for $L^2[\frac{1}{2}, 1)$ and $L^2[0, 1)$ respectively. However, $E(\Lambda_1)$ is incomplete in $L^2[0, \frac{1}{2})$ and therefore is not a Riesz basis for $L^2[0, \frac{1}{2})$.

These observations suggest that in order to derive some positive answers to Questions 1 and 2, we would need to impose some additional conditions on the sets $S_1, S_2 \subset \mathbb R$ and $\Lambda_1, \Lambda_2 \subset \mathbb R$.

Our motivation for Questions 1 and 2 comes from applications in OFDM (orthogonal frequency division multiplexing) based communications, such as 4G and 5G mobile communications. The essential idea of OFDM is to divide the available bandwidth into multiple narrowband subcarriers, each of which is modulated independently. In mathematical terms, this boils down to the problem of efficient design of exponential Riesz bases for unions of intervals, where an ideal solution would involve constructing, for given disjoint intervals $I_1, \ldots, I_N$, some corresponding frequency sets $\Lambda_1, \ldots, \Lambda_N$ such that $E\left(\bigcup_{n \in J} \Lambda_n \right)$ is a Riesz basis for $L^2\left(\bigcup_{n \in J} I_n \right)$ whenever $J \subset \{ 1, \ldots, N \}$. Questions 1 and 2 contribute to this problem from slightly different perspectives: Question 1 deals with the scenario where two existing exponential Riesz bases can be straightforwardly combined by taking unions, and Question 2 addresses the scenario where an existing exponential Riesz basis can be split into two individual Riesz bases. While these questions stand on their own as pure mathematical problems, they can be potentially used in practical applications, such as in communications, as discussed above.

1.1.   Related work on Questions 1 and 2

A seminal work related to Question 1 is by Seip ^[26], who proved the existence of exponential Riesz bases for single intervals and for unions of disjoint intervals with a strong restriction on the lengths of the intervals. Lyubarskii and Seip ^[21] showed the existence of exponential Riesz bases for a finite union of disjoint intervals with equal lengths, and Lev ^[19] extended their result to a finite union of disjoint intervals with lengths belonging to $\alpha \mathbb{Z} + \beta \mathbb{Z}$ for $\alpha, \beta > 0$. Further, the case of finite unions of disjoint cubes in $\mathbb R^d$ was treated by Marzo ^[22], and the case of finite unions of disjoint rectangles in $\mathbb R^d$ with rational vertices was treated by De Carli ^[5].

A significant breakthrough was made by Kozma and Nitzan ^[12], who proved that if $I_1, \ldots, I_N$ are disjoint intervals contained in $[0, 1)$, then there exists a set $\Lambda \subset \mathbb{Z}$ such that $E(\Lambda)$ is a Riesz basis for $L^2 (\cup_{n = 1}^N \, I_n)$.

Recently, Pfander, Revay, and Walnut ^[25] proved that for any partition of $[0, 1)$ into intervals $I_1, \ldots, I_N$, there exists a partition of $\mathbb{Z}$ into sets $\Lambda_1, \ldots, \Lambda_N$ such that for each $n$, the system $E(\Lambda_n)$ is a Riesz basis for $L^2 (I_n)$, and moreover $E(\cup_{n \in J} \, \Lambda_n)$ is a Riesz basis for $L^2 (\cup_{n \in J} \, I_n)$ whenever $J \subset \{ 1, \ldots, N \}$ is a consecutive index set (see [25, Theorems 1 and 2]). Moreover, Caragea and Lee ^[3] showed that if $I_1, \ldots, I_N$ are disjoint subintervals of $[0, 1)$ with the property that the numbers $1, a_1, \ldots, a_L, b_1, \ldots, b_L$ are linearly independent over $\mathbb Q$, then there exist pairwise disjoint subsets $\Lambda_1, \ldots, \Lambda_N$ of $\mathbb{Z}$ such that for every $J \subset \{ 1, \ldots, N \}$, the system $E(\cup_{n \in J} \, \Lambda_n)$ is a Riesz basis for $L^2 (\cup_{n \in J} \, I_n)$.

Another work related to Question 1 is by Frederick and Mayeli ^[7], who proved the following result. Let $S \subset [0, 1)$ be a measurable set and let $A \subset \mathbb{Z}_N : = \{ 0, 1, \ldots, N{-}1 \}$ with $N \in \mathbb{N}$. If there exist sets $\Lambda \subset \mathbb R$ and $J \subset \mathbb{Z}_N$ such that $E(\Lambda)$ and $E(J)$ are Riesz bases for $L^2(S)$ and $\ell_2 (A)$ respectively, and if

then $E(\Lambda{+}\frac{J}{N})$ is a Riesz basis for $L^2(S{+}A)$ (see [7, Theorem 2]). Here, $\delta_\lambda$ denotes the Dirac delta at $\lambda$, and the sums are understood in the Minkowski sense, that is, $\Lambda+\frac{J}{N} = \{ \lambda + \frac{j}{N} : \lambda \in \Lambda, \; j \in J \}$ and $S+A = \{ s + a : s \in S, \; a \in A \}$. Also, $\widehat{f}$ denotes the Fourier transform of $f$ defined by $\widehat{f} (\omega) = \int_{-\infty}^{\infty} f(t) \, e^{- 2 \pi i t \omega} \, dt$ for $f \in L^1(\mathbb R) \cap L^2(\mathbb R)$, which extends to functions in $L^2(\mathbb R)$ and even to tempered distributions. This result, however, relies heavily on the Fourier duality and the condition (1.2) which requires some implicit symmetry in $\Lambda$ and $A$.

Regarding Question 2, we would like to mention two related results. In [23, Proposition 2.1] (also see [1, Proposition 5.4]), it was shown that for any $\Lambda \subset \mathbb{Z}$ and any measurable set $S \subset [0, 1)$, the system $E(\Lambda)$ is a Riesz basis for $L^2(S)$ if and only if $E(\mathbb{Z} \backslash \Lambda)$ is a Riesz basis for $L^2 ([0, 1)\backslash S)$. Also, it was proved in ^[21] that if there exists a set $\Lambda \subset \mathbb R$ such that $E(\Lambda)$ is a Riesz basis for $L^2[0, 1)$, then for each $0 < a < 1$, there exists a subset $\Lambda' \subset \Lambda$ such that $E(\Lambda')$ and $E(\Lambda \backslash \Lambda')$ are Riesz bases for $L^2[0, a)$ and $L^2[a, 1)$, respectively. The former result provides a positive answer to Question 2 in the case that $\Lambda_1 ⊍ \Lambda_2 = \mathbb{Z}$ and $S_1 ⊍ S_2 = [0, 1)$, while the latter result only shows the existence of a particular splitting of $\Lambda$ into $\Lambda_1 : = \Lambda'$ and $\Lambda_2 : = \Lambda \backslash \Lambda'$ such that $E(\Lambda_1)$ and $E(\Lambda_2)$ are Riesz bases for $L^2[0, a)$ and $L^2[a, 1)$, respectively.

1.2.   Main results

In this paper, we develop new methods for combining exponential Riesz bases, which rely on taking unions of frequency sets and domains respectively. This is conceptually different from the key lemmas of ^[3] and ^[12] which combine the frequency sets for $A_{\geq n}$, $n = 1, \ldots, N$ (see Section 3 for more details). Also, our result requires only some mild conditions on the sets themselves and does not require any involved conditions like (1.2).

Theorem 1. Let $S_1, S_2 \subset \mathbb R$ be disjoint bounded measurable sets with $S_1 {+} a \subset S_2$ for some $a \in \mathbb R \backslash \{ 0 \}$. If there exist sets $\Lambda_1 \subset \mathbb R \backslash \frac{1}{a} \mathbb{Z}$ and $\Lambda_2 \subset \frac{1}{a} \mathbb{Z}$ with $\mathrm{dist} (\Lambda_1, \frac{1}{a} \mathbb{Z}) > 0$ such that for each $\ell = 1, 2$, the system $E(\Lambda_\ell)$ is a Riesz basis (resp., a complete sequence, a Riesz sequence) for $L^2(S_\ell)$, then $E (\Lambda_1 \cup \Lambda_2)$ is a Riesz basis (resp., a complete sequence, a Riesz sequence) for $L^2(S_1 \cup S_2)$.

As a direct generalization of Theorem 1, we have the following:

Theorem 2. Let $S_1, S_2 \subset \mathbb R$ be disjoint bounded measurable sets satisfying $S_1 {+} ka \subset S_2$ for $k = 1, \ldots, K$, with some $a \in \mathbb R \backslash \{ 0 \}$ and $K \in \mathbb{N}$. Let $c_1, \ldots, c_K \in [0, \frac{1}{a})$ be distinct real numbers, and assume that there exist sets $\Lambda_1 \subset \mathbb R \backslash \cup_{k = 1}^K (\frac{1}{a} \mathbb{Z}{+}c_k)$ and $\Lambda_2 \subset \cup_{k = 1}^K (\frac{1}{a} \mathbb{Z}{+}c_k)$ with $\mathrm{dist} (\Lambda_1, \cup_{k = 1}^K (\frac{1}{a} \mathbb{Z}{+}c_k)) > 0$, such that for each $\ell = 1, 2$, the system $E(\Lambda_\ell)$ is a Riesz basis (resp., a complete sequence, a Riesz sequence) for $L^2(S_\ell)$. Then, $E (\Lambda_1 \cup \Lambda_2)$ is a Riesz basis (resp., a complete sequence, a Riesz sequence) for $L^2(S_1 \cup S_2)$.

It should be noted that $\Lambda_1 \subset \mathbb R \backslash \cup_{k = 1}^K (\frac{1}{a} \mathbb{Z}{+}c_k)$ can be an arbitrary set which is not contained in a union of lattices.

We point out that the main advantage of Theorem 2 lies in the arbitrary choice of $c_1, \ldots, c_K \in [0, \frac{1}{a})$. For instance, if $S_1, S_2 \subset \mathbb R$ satisfy $S_1 {+} \frac{K}{N} \subset S_2$ for some $K, N \in \mathbb{N}$, then one needs $\Lambda_2 \subset \frac{N}{K} \mathbb{Z} = \cup_{k = 0}^{K-1} (N \mathbb{Z}{+}k\frac{N}{K})$ in order to apply Theorem 1. However, according to Theorem 2, such a requirement can be weakened to $\Lambda_2 \subset \cup_{k = 1}^K (N \mathbb{Z}{+}c_k)$ with arbitrary $c_1, \ldots, c_K \in [0, N)$, if the conditions $S_1 {+} \frac{k}{N} \subset S_2$, $k = 1, \ldots, K$, are satisfied.

Using Theorem 2, we can construct some exponential Riesz bases which cannot be obtained from the existing methods, such as the combining trick of Kozma and Nitzan (see Lemma 12 below).

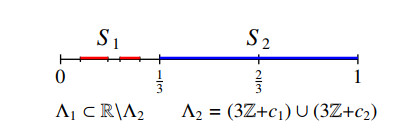
Example. Let $S_2 = [\frac{1}{3}, 1)$ and $\Lambda_2 = (3 \mathbb{Z}{+}c_1) \cup (3 \mathbb{Z}{+}c_2)$ with any $c_1, c_2 \in [0, 3)$ satisfying

Then, $E(\Lambda_2)$ is a Riesz basis for $L^2(S_2)$ by Proposition 9 below. Now, let $S_1 \subset [0, \frac{1}{3})$ be a measurable set and let $\Lambda_1 \subset \mathbb R \backslash \Lambda_2$ be a discrete set with $\mathrm{dist} (\Lambda_1, \Lambda_2) > 0$ such that $E(\Lambda_1)$ is a Riesz basis for $L^2(S_1)$. Then, the sets $S_1 {+} \frac{1}{3}$ and $S_1 {+} \frac{2}{3}$ are both contained in $S_2$, so the assumption of Theorem 2 is fulfilled with $a = \frac{1}{3}$ and $K = 2$. Therefore, the system $E (\Lambda_1 \cup \Lambda_2)$ is a Riesz basis for $L^2(S_1 \cup S_2)$ by Theorem 2.

Note that there is no requirement on the structure of $\Lambda_1$. Indeed, we only require $\Lambda_1 \subset \mathbb R \backslash \Lambda_2$ to be a discrete set with $\mathrm{dist} (\Lambda_1, \Lambda_2) > 0$ such that $E(\Lambda_1)$ is a Riesz basis for $L^2(S_1)$. In contrast, Lemma 12 requires all the frequency sets to be subsets of shifted copies of $N \mathbb{Z}$; hence, Lemma 12 is not applicable here.

As a partial converse of Theorems 1 and 2, we have the following.

Theorem 3. (a) Let $S_1, S_2 \subset \mathbb R$ be disjoint bounded measurable sets with $S_1 {+} a \subset S_2$ for some $a \in \mathbb R \backslash \{ 0 \}$. If there exist sets $\Lambda_1 \subset \mathbb R \backslash \frac{1}{a} \mathbb{Z}$ and $\Lambda_2 \subset \frac{1}{a} \mathbb{Z}$ such that $E (\Lambda_1 \cup \Lambda_2)$ is complete in $L^2(S_1 \cup S_2)$, then $E(\Lambda_1)$ is complete in $L^2(S_1)$.

(b) Let $S_1, S_2 \subset \mathbb R$ be disjoint bounded measurable sets satisfying $S_1 {+} ka \subset S_2$ for $k = 1, \ldots, K$, with some $a \in \mathbb R \backslash \{ 0 \}$ and $K \in \mathbb{N}$. Let $c_1, \ldots, c_K \in [0, \frac{1}{a})$ be distinct real numbers, and assume that there exist sets $\Lambda_1 \subset \mathbb R \backslash \cup_{k = 1}^K (\frac{1}{a} \mathbb{Z}{+}c_k)$ and $\Lambda_2 \subset \cup_{k = 1}^K (\frac{1}{a} \mathbb{Z}{+}c_k)$ such that $E (\Lambda_1 \cup \Lambda_2)$ is complete in $L^2(S_1 \cup S_2)$. Then $E(\Lambda_1)$ is complete in $L^2(S_1)$.

Using Theorem 3(a), one can deduce the following. Let $S_1 = [0, \frac{1}{2})$ and $S_2 = [\frac{1}{2}, 1)$. If there is a set $\Lambda_1 \subset \mathbb R \backslash 2 \mathbb{Z}$ such that $E (\Lambda_1 \cup 2 \mathbb{Z})$ is a Riesz basis for $L^2(S_1 \cup S_2)$, then $E(\Lambda_1)$ is necessarily complete in $L^2(S_1)$ by Theorem 3(a). This is in clear contrast with the counterexample for Question 2 discussed on p. 2, where the sets $\Lambda_1 : = \Lambda^{(1)} \backslash \{ 0 \}$ and $\Lambda_2 : = \Lambda^{(2)}$ are disjoint and satisfy that $E(\Lambda_2)$ and $E(\Lambda_1 \cup \Lambda_2)$ are Riesz bases for $L^2(S_2)$ and $L^2(S_1 \cup S_2)$ respectively, but $E(\Lambda_1)$ is incomplete in $L^2(S_1)$. Theorem 3(a) shows that such pathological examples cannot occur if $\Lambda_2 = 2 \mathbb{Z}$.

We point out that Theorems 1–3 can be easily generalized to higher dimensions. For instance, Theorem 1 can be generalized to dimension $d$ as follows.

For $(a_1, \ldots, a_d) \in \mathbb R^d$, we define the set

which is an additive closed subgroup of $\mathbb{R}^d$. For instance, in the $d = 2$ case,

is the full 2-dimensional plane,

is the union of all vertical lines whose $x$-intercept is a half-integer, and

is the union of all lines having slope $-2$ and integer $y$-intercept.

Theorem 4. Let $S_1, S_2 \subset \mathbb R^d$ be disjoint bounded measurable sets satisfying $S_1 {+} (a_1, \ldots, a_d) \subset S_2$ for some $(a_1, \ldots, a_d) \in \mathbb R^d \backslash \{ (0, \ldots, 0) \}$. If there exist sets $\Lambda_1 \subset \mathbb R^d \backslash \Gamma_{a_1, \ldots, a_d }$ and $\Lambda_2 \subset \Gamma_{a_1, \ldots, a_d }$ with $\mathrm{dist} (\Lambda_1, \Gamma_{a_1, \ldots, a_d }) > 0$ such that for each $\ell = 1, 2$, the system $E(\Lambda_\ell)$ is a Riesz basis (resp., a complete sequence, a Riesz sequence) for $L^2(S_\ell)$, then $E (\Lambda_1 \cup \Lambda_2)$ is a Riesz basis (resp., a complete sequence, a Riesz sequence) for $L^2(S_1 \cup S_2)$.

Clearly, Theorem 1 is a special case of Theorem 4 with $d = 1$.

Note that Theorems 1–4 can be extended to unions of two or more domains. This will be addressed as part of a paper currently in preparation.

Finally, it is worth mentioning that practical applications involving frames and Riesz bases often require information about frame/Riesz constants. However, our proofs for Theorems 1–4 do not readily provide a straightforward method for determining these constants. We leave the task of estimating frame/Riesz constants for future investigation.

1.3.   Organization of the paper

In Section 2, we review some necessary background on exponential systems and give some examples. In Section 3, we discuss some known methods for combining exponential Riesz bases and compare them with our results. Sections 4–7 are devoted to the proofs of Theorems 1–4, respectively. Section 8 provides a proof of Proposition 9 for self-containedness of the paper.

2.   Preliminaries

Definition 5. A sequence $\{ f_n \}_{n\in \mathbb{Z}}$ in a separable Hilbert space $\mathcal{H}$ is called

● a Bessel sequence in $\mathcal{H}$ (with a Bessel bound $B$) if there is a constant $B > 0$ such that $\sum_{n\in \mathbb{Z}} |\langle f, f_n\rangle|^{2} \leq B \, \| f \|^2$ for all $f \in \mathcal{H}$,

● a frame for $\mathcal{H}$ (with frame bounds $A$ and $B$) if there are constants $0 < A \leq B < \infty$ such that $A \, \| f \|^2 \leq \sum_{n\in \mathbb{Z}} |\langle f, f_n\rangle|^{2} \leq B \, \| f \|^2$ for all $f \in \mathcal{H}$,

● a Riesz sequence in $\mathcal{H}$ (with Riesz bounds $A$ and $B$) if there are constants $0 < A \leq B < \infty$ such that $A \, \| {\boldsymbol{c}} \|_{\ell_2}^2 \leq \| \sum_{n\in \mathbb{Z}} c_n \, f_n \|^2 \leq B \, \| {\boldsymbol{c}} \|_{\ell_2}^2$ for all ${\boldsymbol{c}} = \{c_n\}_{n\in \mathbb{Z}} \in \ell_2 (\mathbb Z)$,

● a Riesz basis for $\mathcal{H}$ if it is a complete Riesz sequence in $\mathcal{H}$.

It is well known that a sequence in $\mathcal{H}$ is a Riesz basis if and only if it is both a frame and a Riesz sequence (see, e.g., [4, Proposition 3.7.3, Theorems 5.4.1 and 7.1.1] or [12, Lemma 1]).

Let $\Lambda \subset \mathbb R$ be a discrete set and let $S \subset \mathbb R$ be a positive measure set. If $E(\Lambda) : = \{e^{2\pi i \lambda (\cdot)} : \lambda\in\Lambda\}$ is a Riesz sequence in $L^2(S)$, then $\Lambda$ is necessarily separated, i.e., $\inf\{ |\lambda - \lambda'| : \lambda \neq \lambda' \in \Lambda \} > 0$ (see, e.g., [15, Proposition 11]). Conversely, if $\Lambda \subset \mathbb R$ is separated and $S \subset \mathbb R$ is bounded, then $E(\Lambda)$ is a Bessel sequence in $L^2(S)$ (see [28, p. 135, Theorem 4]).

Proposition 6 (see, e.g., Lemma 8 in ^[15]). Let $\Lambda \subset \mathbb R$ be a discrete set and let $S \subset \mathbb R$ be a measurable set such that $E(\Lambda)$ is a Riesz basis for $L^2(S)$ with bounds $0 < A \leq B < \infty$. Then, for any $a, b \in \mathbb R$ and $c > 0$, the system $E(c \Lambda+a)$ is a Riesz basis for $L^2(\frac{1}{c} S +b)$ with bounds $\frac{A}{c}$ and $\frac{B}{c}$. Moreover, the system $E(- \Lambda)$ is a Riesz basis for $L^2(- S)$ with bounds $A$ and $B$.

Let us discuss an important property of exponential Riesz bases concerning the density of frequency sets and the measure of domains.

For a discrete set $\Lambda \subset \mathbb R^d$, we define the lower and upper density of $\Lambda$ respectively by (see, e.g., ^[9])

If $D^-(\Lambda) = D^+(\Lambda)$, we say that $\Lambda$ has uniform density $D(\Lambda) : = D^-(\Lambda) = D^+(\Lambda)$.

Proposition 7 (Landau's density theorem ^[14]). Let $\Lambda \subset \mathbb R^d$ be a discrete set and let $S \subset \mathbb R^d$ be a bounded set. If $E(\Lambda)$ is a Riesz basis for $L^2(S)$, then $\Lambda$ has uniform density, and $D(\Lambda) = |S|$.

Theorem 8 (Kadec's 1/4 theorem; see, e.g., p. 36 in ^[28]). Let $c > 0$ and $0 < \delta < \frac{1}{4}$. If a sequence $\Lambda = \{ \lambda_n \}_{n \in \mathbb{Z}} \subset \mathbb R$ satisfies $|\lambda_n - c n | \leq c \delta$ for all $n \in \mathbb{Z}$, then $E(\Lambda)$ is a Riesz basis for $L^2[0, 1/c)$.

Fix any $N \in \mathbb{N}$. For any set $\mathcal{K} = \{ c_1, \ldots, c_K \}$ consisting of $K$ distinct real numbers in $[0, N)$, and any set $\mathcal{L} \subset \mathbb{Z}_N : = \{ 0, 1, \ldots, N{-}1 \}$ of cardinality $L$, we define

Proposition 9. Fix any $N \in \mathbb{N}$. Let $\Omega \subset N \mathbb{Z}$ and $S \subset [0, \frac{1}{N})$ be such that $E(\Omega)$ is a Riesz basis for $L^2(S)$ with Riesz bounds $0 < A \leq B < \infty$. Let $\mathcal{K} = \{ c_1, \ldots, c_K \}$ be a set of $K$ distinct real numbers in $[0, N)$, and let $\mathcal{L} \subset \mathbb{Z}_N$ be a set of cardinality $L$. Then, $E(\cup_{k = 1}^K \Omega {+} c_k)$ is

(a) a frame for $L^2(\cup_{\ell \in \mathcal{L}} \, S{+}\frac{\ell}{N})$ if and only if $W_{\mathcal{K}, \mathcal{L}}$ is injective,

(b) a Riesz sequence in $L^2(\cup_{\ell \in \mathcal{L}} \, S{+}\frac{\ell}{N})$ if and only if $W_{\mathcal{K}, \mathcal{L}}$ is surjective,

(c) a Riesz basis for $L^2(\cup_{\ell \in \mathcal{L}} \, S{+}\frac{\ell}{N})$ if and only if $W_{\mathcal{K}, \mathcal{L}}$ is bijective.

Moreover, the corresponding lower frame/Riesz bounds are given by $A \, \sigma_{\min}^2 (W_{\mathcal{K}, \mathcal{L}})$ and $B \, \sigma_{\max}^2 (W_{\mathcal{K}, \mathcal{L}})$, where $\sigma_{\min} (W_{\mathcal{K}, \mathcal{L}})$ and $\sigma_{\max} (W_{\mathcal{K}, \mathcal{L}})$ are the smallest and the largest singular values of $W_{\mathcal{K}, \mathcal{L}}$, respectively.

Remark. If $\mathcal{K}$ or $\mathcal{L}$ consists of consecutive integers, then $W_{\mathcal{K}, \mathcal{L}}$ is essentially a Vandermonde matrix (due to the multi-linearity of determinant in the rows/columns; see (5.3) below). If $N \in \mathbb{N}$ is a prime and $\mathcal{K}, \mathcal{L} \subset \mathbb{Z}_N$, then $W_{\mathcal{K}, \mathcal{L}}$ has full rank by Chebotarëv's theorem on roots of unity, which asserts that every minor of the Fourier matrix in prime dimension is nonzero (see, e.g., ^[27]).

Proposition 9 is somewhat considered folklore; see, e.g., [5, Theorem 1.1] and [24, Lemma 1.13]. For self-containedness of the paper, we provide a proof of Proposition 9 in Section 8.

Now, let us discuss some examples of exponential Riesz bases. The first example is based on Proposition 9.

Example 10. (a) Let $\mathcal{K}, \mathcal{L} \subset \mathbb{Z}_4$ with $| \mathcal{K} | = | \mathcal{L} |$. If $\mathcal{K}$ or $\mathcal{L}$ is consecutive, then $W_{\mathcal{K}, \mathcal{L}}$ is essentially a Vandermonde matrix which is invertible, and hence $E(\cup_{k \in \mathcal{K}} \, 4 \mathbb{Z} {+} k)$ is a Riesz basis for $L^2(\cup_{\ell \in \mathcal{L}} \, [\frac{\ell}{4}, \frac{\ell+1}{4}))$. On the other hand, for $\mathcal{K} = \mathcal{L} = \{ 0, 2 \}$, the matrix

is singular, so that $E(2 \mathbb{Z}) = E(4 \mathbb{Z} \cup (4 \mathbb{Z} {+} 2))$ is not a frame nor a Riesz sequence for $L^2([0, \frac{1}{4}) \cup [\frac{2}{4}, \frac{3}{4}))$. In fact, since all functions in $E(2 \mathbb{Z})$ are $\frac{1}{2}$-periodic, the system $E(2 \mathbb{Z})$ is not complete in $L^2([0, \frac{1}{4}) \cup [\frac{2}{4}, \frac{3}{4}))$. However, this turns out to be a rare case. If $\mathcal{K}$ is replaced by $\{ 0, c \}$ for any real number $0 < c < 4$, $c \neq 2$, then

is invertible, so that $E(4 \mathbb{Z} \cup (4 \mathbb{Z} {+} c))$ is a Riesz basis for $L^2([0, \frac{1}{4}) \cup [\frac{2}{4}, \frac{3}{4}))$.

(b) Let $P \in \mathbb{N}$ be a prime. By Chebotarëv's theorem on roots of unity (see, e.g., ^[27]), the matrix $W_{\mathcal{K}, \mathcal{L}}$ is invertible for every $\mathcal{K}, \mathcal{L} \subset \mathbb{Z}_P$ with $| \mathcal{K} | = | \mathcal{L} |$. Hence, the system $E(\cup_{k \in \mathcal{K}} \, P \mathbb{Z} {+} k)$ is a Riesz basis for $L^2(\cup_{\ell \in \mathcal{L}} \, [\frac{\ell}{P}, \frac{\ell+1}{P}))$ whenever $\mathcal{K}, \mathcal{L} \subset \mathbb{Z}_P$ with $| \mathcal{K} | = | \mathcal{L} |$. By rescaling (Proposition 6), we obtain that $E(\cup_{k \in \mathcal{K}} \, 4 \mathbb{Z} {+} \frac{4}{P}k)$ is a Riesz basis for $L^2(\cup_{\ell \in \mathcal{L}} \, [\frac{\ell}{4}, \frac{\ell+1}{4}))$ whenever $\mathcal{K}, \mathcal{L} \subset \mathbb{Z}_P$ with $| \mathcal{K} | = | \mathcal{L} |$.

The next example is rather pathological and shows the delicate nature of exponential Riesz bases.

Example 11. Fix any $0 < \epsilon < \frac{1}{4}$ and set

Then, $\Lambda^{(1)} \cap \Lambda^{(2)} = \{ 0 \}$ and

By labeling the elements of $\Lambda^{(1)}$ by

and comparing them with elements of $2 \mathbb{Z} {-} \frac{1-\epsilon}{2}$, we see that

Then, Theorem 8 with $c = 2$ and $\delta = \frac{1-\epsilon}{4}$ implies that $E(\Lambda^{(1)})$ is a Riesz basis for $L^2[0, \frac{1}{2})$; and thus, $E(\Lambda^{(2)}) = E(-\Lambda^{(1)})$ is a Riesz basis for $L^2[-\frac{1}{2}, 0) \cong L^2[\frac{1}{2}, 1)$. Also, Theorem 8 with $c = 1$ implies that $E(\Lambda^{(1)}\cup \Lambda^{(2)})$ is a Riesz basis for $L^2[0, 1)$. Consequently, $E(\Lambda^{(1)})$, $E(\Lambda^{(2)})$, and $E(\Lambda^{(1)}\cup \Lambda^{(2)})$ form Riesz bases for $L^2[0, \frac{1}{2})$, $L^2[\frac{1}{2}, 1)$, and $L^2[0, 1)$ respectively, despite the fact that $\Lambda^{(1)}$ and $\Lambda^{(2)}$ have a nonempty intersection, namely, $\Lambda^{(1)} \cap \Lambda^{(2)} = \{ 0 \}$.

Now, let us consider a uniform shifting of $\Lambda^{(2)}$. For any $0 < \delta < \epsilon \; (<\frac{1}{4})$, we have $\Lambda^{(1)} \cap (\Lambda^{(2)} {+} \delta) = \emptyset$, $\mathrm{dist} (\Lambda^{(1)}, \Lambda^{(2)} {+} \delta) = \delta$, and

Note that removing the element $0$ from $\Lambda^{(1)} \cup (\Lambda^{(2)} {+} \delta)$ yields a pointwise perturbation of $\mathbb{Z}$ by at most $\epsilon \; (<\frac{1}{4})$, so the system $E((\Lambda^{(1)} \backslash \{ 0 \}) \cup (\Lambda^{(2)} {+} \delta))$ is a Riesz basis for $L^2[0, 1)$ by Theorem 8. This implies that $E(\Lambda^{(1)} \cup (\Lambda^{(2)} {+} \delta))$ is overcomplete and thus cannot be a Riesz basis for $L^2[0, 1)$, whereas $E(\Lambda^{(1)})$ and $E(\Lambda^{(2)} {+} \delta)$ form Riesz bases for $L^2[0, \frac{1}{2})$ and $L^2[\frac{1}{2}, 1)$ respectively.

3.   Comparison with related work

As mentioned in the introduction, Kozma and Nitzan ^[12] proved that for any finite union $S$ of disjoint intervals in $[0, 1)$, there exists a set $\Lambda \subset \mathbb{Z}$ such that $E(\Lambda)$ is a Riesz basis for $L^2(S)$. Their result is based on the following lemma which combines exponential Riesz bases in a certain way.

For any $N \in \mathbb{N}$, any measurable set $S \subset [0, 1)$, and $n = 1, \ldots, N$, let

Lemma 12 (Lemma 2 in ^[12]). Let $N \in \mathbb{N}$ and let $S \subset [0, 1)$ be a measurable set. If there exist sets $\Lambda_1, \ldots, \Lambda_N \subset N \mathbb{Z}$ such that $E(\Lambda_n)$ is a Riesz basis for $L^2(A_{\geq n})$, then $E(\cup_{n = 1}^N (\Lambda_n {+} n))$ is a Riesz basis for $L^2 (S)$.

Note that the sets $\Lambda_n$ are shifted by $n$ before taking their union, which prevents overlapping of frequency sets. When $N$ is prime, one can use arbitrary shift factors instead of $n$.

Lemma 13 (Lemma 6 in ^[3]). Let $N \in \mathbb{N}$ be a prime and let $S \subset [0, 1)$ be a measurable set. If there exist sets $\Lambda_1, \ldots, \Lambda_N \subset N \mathbb{Z}$ such that $E(\Lambda_n)$ is a Riesz basis for $L^2(A_{\geq n})$, then for every permutation $\{ k_n \}_{n = 1}^N$ of $\{ 1, \ldots, N \}$, the system $E(\cup_{n = 1}^N (\Lambda_n {+} k_n))$ is a Riesz basis for $L^2 (S)$.

Using Lemmas 12 and 13, we obtain the following proposition which is in the same spirit as our main results, that is, one directly takes unions of frequency sets and domains respectively with appropriate shifts.

Proposition 14. Let $S_M \subset \cdots \subset S_1 \subset [0, \frac{1}{N})$ be measurable sets, where $M, N \in \mathbb{N}$ with $M \leq N$. Assume that there exist sets $\Lambda_1, \ldots, \Lambda_M \subset N \mathbb{Z}$ such that for each $m = 1, \ldots, M$, the system $E(\Lambda_{m})$ is a Riesz basis for $L^2(S_{m})$.

(a) For any permutation $\{ \ell_n \}_{n = 1}^N$ of $\{ 1, \ldots, N \}$,

(b) If $N$ is prime, then for any permutations $\{ k_n \}_{n = 1}^N$ and $\{ \ell_n \}_{n = 1}^N$ of $\{ 1, \ldots, N \}$,

and moreover, for any subset $J \subset \{ 1, \ldots, M\}$,

Comparison with Proposition 9. When $a = \frac{1}{N}$, $S = S_1 = \ldots = S_M \subset [0, \frac{1}{N})$, and $\Omega = \Lambda_1 = \ldots = \Lambda_M \subset N \mathbb{Z}$, Proposition 14 can be deduced from Proposition 9. Indeed, the corresponding matrix $W_{\mathcal{K}, \mathcal{L}}$ in part (a) is a Vandermonde matrix, while that in part (b) is a Fourier submatrix which is invertible by Chebotarëv's theorem on roots of unity.

Comparison with Theorem 1. Let $N \geq 2$. If $S_1 \subset S_2 \subset [0, \frac{1}{N})$ and $\Lambda_1, \Lambda_2 \subset N \mathbb{Z}$ are such that $E(\Lambda_1)$ and $E(\Lambda_2)$ form Riesz bases for $L^2(S_1)$ and $L^2(S_2)$ respectively, then Proposition 14(a) implies that $E ((\Lambda_1 {+} 1) \cup \Lambda_2)$ is a Riesz basis for $L^2 (S_1 \cup (S_2 {+} \frac{K}{N}))$ with any $K \in \{ 1, \ldots, N{-}1 \}$. This result can also be deduced from Theorem 1. Indeed, by setting $S_1' : = S_1$, $S_2' : = S_2 {+} \frac{K}{N}$, $\Lambda_1' : = \Lambda_1 {+} 1$, and $\Lambda_2' : = \Lambda_2$, we have $S_1' {+} \frac{K}{N} \subset S_2'$, $\Lambda_1' \subset N \mathbb{Z}{+}1 \, (\subset \mathbb R \backslash \frac{N}{K} \mathbb{Z})$, and $\Lambda_2' \subset N \mathbb{Z} \, (\subset \frac{N}{K} \mathbb{Z})$. Hence, Theorem 1 with $a = \frac{K}{N}$ yields the same result. Note that Theorem 1 actually allows more flexibility in the choice of $\Lambda_1' \subset \mathbb R \backslash \frac{N}{K} \mathbb{Z}$.

Proof of Proposition 14. (a) For any permutation $\{ \ell_n \}_{n = 1}^N$ of $\{ 1, \ldots, N \}$, the set $S : = \bigcup_{m = 1}^M (S_{m} {+} \frac{\ell_m - 1}{N})$ is contained in $[0, 1)$, and

By Lemma 12, the system $E(\cup_{m = 1}^M (\Lambda_{m} {+} m))$ is a Riesz basis for $L^2 (S)$.

(b) If $N \in \mathbb{N}$ is prime, then Lemma 13 yields that for every permutation $\{ k_n \}_{n = 1}^N$ of $\{ 1, \ldots, N \}$, the system $E(\cup_{m = 1}^M (\Lambda_{m} {+} k_m))$ is a Riesz basis for $L^2 (S)$. Now, fix any subset $J \subset \{ 1, \ldots, M\}$, say, $J = \{ j_1, \ldots, j_R \}$ with $j_1 < \cdots < j_R$ and $1 \leq R \leq M$. Since $S_{j_R} \subset \cdots \subset S_{j_1} \subset [0, \frac{1}{N})$, we can deduce from (3.1) that $E(\cup_{r = 1}^R (\Lambda_{j_r} {+} k_{j_r}))$ is a Riesz basis for $L^2 (\cup_{r = 1}^R (S_{j_r} {+} \frac{\ell_{j_r} {-} 1}{N}))$, which is exactly (3.2). □

4.   Proof of Theorem 1

In the proofs of Theorems 1–4, we will need the following notions. The Fourier transform is defined as

so that $\mathcal{F}[\cdot]$ extends to a unitary operator from $L^{2}(\mathbb{R})$ onto $L^{2}(\mathbb{R})$. The Paley-Wiener space over a measurable set $S \subset \mathbb R$ is defined as

equipped with the norm $\| f \|_{PW(S)} : = \| f \|_{L^2(\mathbb R)} = \| \widehat{f} \|_{L^2(S)}$. If $S \subset \mathbb R$ has finite measure, then every $f \in PW(S)$ is continuous, and

Definition 15. Let $S \subset \mathbb R$ be a measurable set. A discrete set $\Lambda \subset \mathbb R$ is called

● a set of uniqueness for $PW(S)$ if the only function $f \in PW(S)$ satisfying $f(\lambda) = 0$ for all $\lambda \in \Lambda$ is the trivial function $f = 0$,

● a set of interpolation for $PW(S)$ if for each $\{ c_ \lambda \}_{\lambda \in \Lambda} \in \ell_2(\Lambda)$ there exists a function $f \in PW(S)$ satisfying $f(\lambda) = c_\lambda$ for all $\lambda \in \Lambda$.

We can easily deduce the following from (4.1).

(ⅰ) $\Lambda$ is a set of uniqueness for $PW(S)$ if and only if $E(-\Lambda) = \overline{E(\Lambda)}$ is complete in $L^2(S)$ if and only if $E(\Lambda)$ is complete in $L^2(S)$.

(ⅱ) If $E(\Lambda)$ is a Bessel sequence in $L^2(S)$, then $\Lambda$ is a set of interpolation for $PW(S)$ if and only if $E(\Lambda)$ is a Riesz sequence in $L^2(S)$ (see [15, Appendix A] for more details).

We are now ready to prove Theorem 1.

Completeness. Assume that $f \in PW (S_1 \cup S_2)$ satisfies $f(\lambda) = 0$ for $\lambda \in \Lambda_1 \cup \Lambda_2$. Define $f_1\in PW(S_1)$ by $\widehat{f}_1 = \widehat{f} \cdot \mathbb{1}_{S_1}$ and define $g\in PW(S_2)$ by

Since $S_2$ is a bounded set, it follows from (4.1) that $g(x) = f(x) - (1- e^{2\pi i a x}) \, f_1(x)$ for all $x \in \mathbb R$; in particular, we have $g(\lambda_2) = f(\lambda_2) = 0$ for all $\lambda_2 \in \Lambda_2 \; (\subset \frac{1}{a} \mathbb{Z})$. Since $g\in PW(S_2)$ and since $\Lambda_2$ is a set of uniqueness for $PW(S_2)$, we have $g = 0$ and thus $f(x) = (1-e^{2\pi i a x}) \, f_1(x)$ for all $x \in \mathbb R$. By the assumption, it holds that $0 = f(\lambda_1) = (1-e^{2\pi i a \lambda_1}) \, f_1(\lambda_1)$ for all $\lambda_1 \in \Lambda_1$. Note that for every $\lambda_1 \in \Lambda_1 \; (\subset \mathbb R \backslash \frac{1}{a} \mathbb{Z})$, we have $e^{2\pi i a \lambda_1} \neq 1$, and therefore $f_1(\lambda_1) = 0$. Since $f_1 \in PW(S_1)$ and since $\Lambda_1$ is a set of uniqueness for $PW(S_1)$, we get $f_1 = 0$ and thus arrive at $f = 0$. This shows that $\Lambda_1 \cup \Lambda_2$ is a set of uniqueness for $PW (S_1 \cup S_2)$; hence, the system $E(\Lambda_1 \cup \Lambda_2)$ is complete in $L^2 (S_1 \cup S_2)$.

Riesz sequence. Since $\Lambda_1 \cup \Lambda_2$ is separated and since $S_1 \cup S_2$ is bounded, the system $E (\Lambda_1 \cup \Lambda_2)$ is a Bessel sequence in $L^2 (S_1 \cup S_2)$. Thus, to prove that $E (\Lambda_1 \cup \Lambda_2)$ is a Riesz sequence in $L^2 (S_1 \cup S_2)$, it is enough to show that $\Lambda_1 \cup \Lambda_2$ is a set of interpolation for $PW (S_1 \cup S_2)$.

To show this, we will fix an arbitrary ${\boldsymbol{b}} = \{ b_{\lambda} \}_{\lambda \in \Lambda_1 \cup \Lambda_2} \in \ell_2 (\Lambda_1 \cup \Lambda_2)$ and construct a function $f \in PW (S_1 \cup S_2)$ satisfying $f(\lambda) = b_{\lambda}$ for all $\lambda \in \Lambda_1 \cup \Lambda_2$. Since $\Lambda_2$ is a set of interpolation for $PW(S_2)$, there exists a function $g \in PW(S_2)$ satisfying $g(\lambda_2) = b_{\lambda_2}$ for all $\lambda_2 \in \Lambda_2$. Note that for any $f_1 \in PW(S_1)$, the function

belongs in $PW (S_1 \cup S_2)$ and satisfies $f(\lambda_2) = g(\lambda_2) = b_{\lambda_2}$ for $\lambda_2 \in \Lambda_2 \; (\subset \frac{1}{a} \mathbb{Z})$. We will now choose a particular function $f_1 \in PW(S_1)$ as follows. Since $\Lambda_1$ is separated and since $S_2$ is bounded, the system $E(\Lambda_1)$ is a Bessel sequence in $L^2(S_2)$, so that the sequence $\{ g(\lambda_1) \}_{\lambda_1 \in \Lambda_1}$ is in $\ell_2(\Lambda_1)$, and so is the sequence $\big\{ \frac{1}{ 1-e^{2\pi i a \lambda_1} } \big(b_{\lambda_1}-g(\lambda_1) \big) \big\}_{\lambda_1 \in \Lambda_1}$. Here, it holds that $e^{2\pi i a \lambda_1} \neq 1$ for all $\lambda_1 \in \Lambda_1 \; (\subset \mathbb R \backslash \frac{1}{a} \mathbb{Z})$, and moreover, the condition $\mathrm{dist} (\Lambda_1, \frac{1}{a} \mathbb{Z}) > 0$ ensures that $\inf_{\lambda_1 \in \Lambda_1} | 1-e^{2\pi i a \lambda_1} | > 0$. Now, since $\Lambda_1$ is a set of interpolation for $PW(S_1)$, there exists a function $f_1 \in PW(S_1)$ satisfying $f_1(\lambda_1) = \frac{1}{ 1-e^{2\pi i a \lambda_1} } \big(b_{\lambda_1}-g(\lambda_1) \big)$ for all $\lambda_1 \in \Lambda_1$. We then have $f(\lambda_1) = b_{\lambda_1}$ for all $\lambda_1 \in \Lambda_1$. This shows that the function $f \in PW (S_1 \cup S_2)$ satisfies $f(\lambda) = b_{\lambda}$ for all $\lambda \in \Lambda_1 \cup \Lambda_2$, as desired.

Riesz basis. By definition, a Riesz basis is a complete Riesz sequence. Hence, this part follows immediately by combining the completeness and Riesz sequence parts. □

Remark. Fix any nonzero real number $a \neq 0$. Then, for $x \in \mathbb R$, the term $1-e^{2\pi i a x}$ vanishes if and only if $a x \in \mathbb{Z}$. Similarly, for any $M \in \mathbb{N}$ and $x \in \mathbb R$, the term

vanishes if and only if $e^{2\pi i a x}$ is an $M$-th root of unity (i.e., $e^{2\pi i a x}$ is one of $1, \, e^{2\pi i /M}, \, e^{2\pi i 2/M}, \, \ldots, \, e^{2\pi i (M-1)/M}$) if and only if $a x \in \frac{1}{M} \mathbb{Z}$. We will make use of the factorization (4.2) in the proof of Theorem 2.

5.   Proof of Theorem 2

By shifting the sets $\Lambda_1, \Lambda_2 \subset \mathbb R$ simultaneously by a constant (see Proposition 6), we may assume without loss of generality that $c_1 = 0$.

Completeness. Assume that $f \in PW (S_1 \cup S_2)$ satisfies $f(\lambda) = 0$ for $\lambda \in \Lambda_1 \cup \Lambda_2$. Define $f_1\in PW(S_1)$ by $\widehat{f}_1 = \widehat{f} \cdot \mathbb{1}_{S_1}$ and define $g\in PW(S_2)$ by

for some constants $a_1, \ldots, a_K \in \mathbb R$ to be chosen later. Requiring $a_1 + \ldots + a_K = 1$, one may rewrite the equation as

which is equivalent to

Using the factorization (4.2), this is in turn equivalent to

where $h(x) : = a_1 + a_2 \, (1+ e^{2\pi i a x}) + \cdots + a_K \, (1+ \cdots + e^{2\pi i (K-1) a x})$. Changing the variables from $a_1, \ldots, a_K$ to $a_k' : = \sum_{n = k}^K a_n$ for $k = 1, \ldots, K$ (which corresponds to a linear bijection between $(a_1, \ldots, a_K) \in \mathbb R^K$ and $(a_1', \ldots, a_K') \in \mathbb R^K$), the constraint $a_1 + \ldots + a_K = 1$ becomes $a_1' = 1$. Thus, we have

We will now find $a_2', \ldots, a_K' \in \mathbb R$ satisfying $h (c_k) = 0$ for $k = 2, \ldots, K$, which corresponds to solving the $(K{-}1){\times} (K{-}1)$ linear system

Note that the associated matrix has a nonzero determinant given by

where we used the multi-linearity of determinant in the rows and the Vandermonde determinant formula (see, e.g., ^[10]). Hence, the linear system is uniquely solvable in $a_2', \ldots, a_K' \in \mathbb R$, and in turn, $a_1, \ldots, a_K \in \mathbb R$ are uniquely determined from $a_2', \ldots, a_K'$ via the 1:1 correspondence. In fact, we must have

which is clearly of the form (5.2) and satisfies $h (c_k) = 0$ for $k = 2, \ldots, K$. Since $c_1 = 0$, it follows from (5.1) that

Now, observe that $g(\lambda_2) = f(\lambda_2) = 0$ for all $\lambda_2 \in \Lambda_2 \subset \cup_{k = 1}^K (\frac{1}{a} \mathbb{Z}{+}c_k)$. Since $g\in PW(S_2)$ and since $\Lambda_2$ is a set of uniqueness for $PW(S_2)$, we have $g = 0$, and thus $f(x) = f_1(x) \cdot \prod_{k = 1}^K (1- e^{2\pi i a (x-c_k)})$ for all $x \in \mathbb R$. By the assumption, it holds that $0 = f(\lambda) = f_1(\lambda) \cdot \prod_{k = 1}^K (1- e^{2\pi i a (\lambda-c_k)})$ for all $\lambda \in \Lambda_1$. Note that for every $\lambda \in \Lambda_1 \subset \mathbb R \backslash \cup_{k = 1}^K (\frac{1}{a} \mathbb{Z}{+}c_k)$, we have $\prod_{k = 1}^K (1- e^{2\pi i a (\lambda-c_k)}) \neq 0$, and therefore $f_1(\lambda) = 0$. Since $f_1 \in PW(S_1)$ and since $\Lambda_1$ is a set of uniqueness for $PW(S_1)$, we get $f_1 = 0$ and arrive at $f = 0$. Hence, $\Lambda_1 \cup \Lambda_2$ is a set of uniqueness for $PW (S_1 \cup S_2)$; equivalently, $E(\Lambda_1 \cup \Lambda_2)$ is complete in $L^2 (S_1 \cup S_2)$.

Riesz sequence. Since $\Lambda_1 \cup \Lambda_2$ is separated and since $S_1 \cup S_2$ is bounded, the system $E (\Lambda_1 \cup \Lambda_2)$ is a Bessel sequence in $L^2 (S_1 \cup S_2)$. Thus, to prove that $E (\Lambda_1 \cup \Lambda_2)$ is a Riesz sequence in $L^2 (S_1 \cup S_2)$, it is enough to show that $\Lambda_1 \cup \Lambda_2$ is a set of interpolation for $PW (S_1 \cup S_2)$.

To show this, we will fix an arbitrary ${\boldsymbol{b}} = \{ b_{\lambda} \}_{\lambda \in \Lambda_1 \cup \Lambda_2} \in \ell_2 (\Lambda_1 \cup \Lambda_2)$ and construct a function $f \in PW (S_1 \cup S_2)$ satisfying $f(\lambda) = b_{\lambda}$ for all $\lambda \in \Lambda_1 \cup \Lambda_2$. Since $\Lambda_2$ is a set of interpolation for $PW(S_2)$, there exists a function $g \in PW(S_2)$ satisfying $g(\lambda_2) = b_{\lambda_2}$ for all $\lambda_2 \in \Lambda_2$. Note that for any $f_1 \in PW(S_1)$, the function

belongs in $PW (S_1 \cup S_2)$ (this is seen by following the derivations (5.1)–(5.4) backwards) and satisfies $f(\lambda_2) = g(\lambda_2) = b_{\lambda_2}$ for $\lambda_2 \in \Lambda_2 \subset \cup_{k = 1}^K (\frac{1}{a} \mathbb{Z}{+}c_k)$. We will now choose a particular function $f_1 \in PW(S_1)$ as follows. Since $\Lambda_1$ is separated and since $S_2$ is bounded, the system $E(\Lambda_1)$ is a Bessel sequence in $L^2(S_2)$, so that the sequence $\{ g(\lambda_1) \}_{\lambda_1 \in \Lambda_1}$ is in $\ell_2(\Lambda_1)$, and so is the sequence $\big\{ \frac{1}{ \prod_{k = 1}^K (1- e^{2\pi i a (\lambda_1-c_k)}) } \big(b_{\lambda_1}-g(\lambda_1) \big) \big\}_{\lambda_1 \in \Lambda_1}$. Note that $\prod_{k = 1}^K (1- e^{2\pi i a (\lambda_1-c_k)}) \neq 0$ for all $\lambda_1 \in \Lambda_1 \subset \mathbb R \backslash \cup_{k = 1}^K (\frac{1}{a} \mathbb{Z}{+}c_k)$, and moreover, $\mathrm{dist} (\Lambda_1, \cup_{k = 1}^K (\frac{1}{a} \mathbb{Z}{+}c_k)) > 0$ implies that

Now, since $\Lambda_1$ is a set of interpolation for $PW(S_1)$, there exists a function $f_1 \in PW(S_1)$ satisfying $f_1(\lambda_1) = \frac{1}{ \prod_{k = 1}^K (1- e^{2\pi i a (\lambda_1-c_k)}) } \big(b_{\lambda_1}-g(\lambda_1) \big)$ for all $\lambda_1 \in \Lambda_1$. We then have $f(\lambda_1) = b_{\lambda_1}$ for all $\lambda_1 \in \Lambda_1$. Hence, the function $f \in PW (S_1 \cup S_2)$ satisfies $f(\lambda) = b_{\lambda}$ for all $\lambda \in \Lambda_1 \cup \Lambda_2$, as desired.

Riesz basis. This part follows immediately by combining the completeness and Riesz sequence parts.

6.   Proof of Theorem 3

(a) To prove that $E(\Lambda_1)$ is complete in $L^2(S_1)$, we will assume that $f \in PW (S_1)$ satisfies $f(\lambda) = 0$ for $\lambda \in \Lambda_1$ and show that $f = 0$. Define $g\in PW(S_1 \cup S_2)$ by

Then, $g(x) = (1- e^{2\pi i a x}) \, f(x)$ for $x \in \mathbb R$, so that $g(\lambda) = 0$ for all $\lambda \in \Lambda_1 \cup \Lambda_2$. Since $g\in PW(S_1 \cup S_2)$ and since $\Lambda_1 \cup \Lambda_2$ is a set of uniqueness for $PW(S_1 \cup S_2)$, we get $g = 0$, so that $f(x) = 0$ for all $x \in \mathbb R \backslash \frac{1}{a} \mathbb{Z}$. Since $f \in PW (S_1)$ is analytic, we obtain $f = 0$ by the uniqueness of analytic continuation. Hence, $\Lambda_1$ is a set of uniqueness for $PW (S_1)$; equivalently, $E(\Lambda_1)$ is complete in $L^2 (S_1)$.

(b) As in part (a), we will assume that $f \in PW (S_1)$ satisfies $f(\lambda) = 0$ for $\lambda \in \Lambda_1$ and show that $f = 0$. Define $g\in PW(S_1 \cup S_2)$ by

for some $a_1, \ldots, a_K \in \mathbb R$ satisfying $a_1 + \ldots + a_K = 1$, so that

By similar arguments as in the proof of Theorem 2, the constants $a_1, \ldots, a_K \in \mathbb R$ are uniquely determined by requiring $g (c_k) = 0$ for $k = 2, \ldots, K$. In fact, due to the uniqueness, we obtain

Now, observe that $g(\lambda) = 0$ for all $\lambda \in \Lambda_1 \cup \Lambda_2$. Since $g\in PW(S_1 \cup S_2)$ and since $\Lambda_1 \cup \Lambda_2$ is a set of uniqueness for $PW(S_1 \cup S_2)$, we get $g = 0$, so that $f(x) = 0$ for all $x \in \mathbb R \backslash \cup_{k = 1}^K (\frac{1}{a} \mathbb{Z}{+}c_k)$. Since $f \in PW (S_1)$ is analytic, we obtain $f = 0$ by the uniqueness of analytic continuation. Hence, $\Lambda_1$ is a set of uniqueness for $PW (S_1)$; equivalently, $E(\Lambda_1)$ is complete in $L^2 (S_1)$.

7.   Proof of Theorem 4

We will use similar arguments as in the proof of Theorem 1. The $d$-dimensional Fourier transform is defined as

so that $\mathcal{F}[\cdot]$ extends to a unitary operator from $L^{2}(\mathbb{R}^d)$ onto $L^{2}(\mathbb{R}^d)$. The Paley-Wiener space over a measurable set $S \subset \mathbb R^d$ is defined as

equipped with the norm $\| f \|_{PW(S)} : = \| f \|_{L^2(\mathbb R^d)} = \| \widehat{f} \|_{L^2(S)}$. If $S \subset \mathbb R^d$ has finite measure, then every $f \in PW(S)$ is continuous, and

The notion of a set of uniqueness/interpolation for $PW(S)$ with $S \subset \mathbb R^d$ is defined similarly as in Definition 15.

Completeness. Assume that $f \in PW (S_1 \cup S_2)$ satisfies $f(\lambda) = 0$ for $\lambda \in \Lambda_1 \cup \Lambda_2$. Define $f_1\in PW(S_1)$ by $\widehat{f}_1 = \widehat{f} \cdot \mathbb{1}_{S_1}$ and define $g\in PW(S_2)$ by

Since $S_2$ is a bounded set, it follows that $g$ is continuous, and $g(x_1, \ldots, x_d) = f(x_1, \ldots, x_d) - (1- e^{2\pi i (a_1 x_1 + \cdots + a_d x_d)}) \, f_1(x_1, \ldots, x_d)$ for all $(x_1, \ldots, x_d) \in \mathbb R^d$. In particular, we have $g(\lambda_2) = f(\lambda_2) = 0$ for all $\lambda_2 \in \Lambda_2 \; (\subset \Gamma_{a_1, \ldots, a_d })$. Since $g\in PW(S_2)$ and since $\Lambda_2$ is a set of uniqueness for $PW(S_2)$, we have $g = 0$, and thus $f(x_1, \ldots, x_d) = (1- e^{2\pi i (a_1 x_1 + \cdots + a_d x_d)}) \, f_1(x_1, \ldots, x_d)$ for all $(x_1, \ldots, x_d) \in \mathbb R^d$. By the assumption, it holds that $0 = f(\lambda_1) = (1-e^{2\pi i (a_1, \ldots, a_d) \cdot \lambda_1}) \, f_1(\lambda_1)$ for all $\lambda_1 \in \Lambda_1$. Note that for every $\lambda_1 \in \Lambda_1 \; (\subset \mathbb R^d \backslash \Gamma_{a_1, \ldots, a_d })$, we have $e^{2\pi i (a_1, \ldots, a_d) \cdot \lambda_1} \neq 1$, and therefore $f_1(\lambda_1) = 0$. Since $f_1 \in PW(S_1)$ and since $\Lambda_1$ is a set of uniqueness for $PW(S_1)$, we get $f_1 = 0$ and thus arrive at $f = 0$. This shows that $\Lambda_1 \cup \Lambda_2$ is a set of uniqueness for $PW (S_1 \cup S_2)$; hence, the system $E(\Lambda_1 \cup \Lambda_2)$ is complete in $L^2 (S_1 \cup S_2)$.

Riesz sequence. Since $\Lambda_1 \cup \Lambda_2$ is separated and since $S_1 \cup S_2$ is bounded, the system $E (\Lambda_1 \cup \Lambda_2)$ is a Bessel sequence in $L^2 (S_1 \cup S_2)$. Thus, to prove that $E (\Lambda_1 \cup \Lambda_2)$ is a Riesz sequence in $L^2 (S_1 \cup S_2)$, it is enough to show that $\Lambda_1 \cup \Lambda_2$ is a set of interpolation for $PW (S_1 \cup S_2)$.

To show this, we will fix an arbitrary ${\boldsymbol{b}} = \{ b_{\lambda} \}_{\lambda \in \Lambda_1 \cup \Lambda_2} \in \ell_2 (\Lambda_1 \cup \Lambda_2)$ and construct a function $f \in PW (S_1 \cup S_2)$ satisfying $f(\lambda) = b_{\lambda}$ for all $\lambda \in \Lambda_1 \cup \Lambda_2$. Since $\Lambda_2$ is a set of interpolation for $PW(S_2)$, there exists a function $g \in PW(S_2)$ satisfying $g(\lambda_2) = b_{\lambda_2}$ for all $\lambda_2 \in \Lambda_2$. Note that for any $f_1 \in PW(S_1)$, the function

belongs in $PW (S_1 \cup S_2)$ and satisfies $f(\lambda_2) = g(\lambda_2) = b_{\lambda_2}$ for $\lambda_2 \in \Lambda_2 \; (\subset \Gamma_{a_1, \ldots, a_d })$. We will now choose a particular function $f_1 \in PW(S_1)$ as follows. Since $\Lambda_1$ is separated and since $S_2$ is bounded, the system $E(\Lambda_1)$ is a Bessel sequence in $L^2(S_2)$, so that the sequence $\{ g(\lambda_1) \}_{\lambda_1 \in \Lambda_1}$ is in $\ell_2(\Lambda_1)$, and so is the sequence $\big\{ \frac{1}{ 1-e^{2\pi i (a_1, \ldots, a_d) \cdot \lambda_1} } \big(b_{\lambda_1}-g(\lambda_1) \big) \big\}_{\lambda_1 \in \Lambda_1}$. Here, it holds that $e^{2\pi i (a_1, \ldots, a_d) \cdot \lambda_1} \neq 1$ for all $\lambda_1 \in \Lambda_1 \; (\subset \mathbb R^d \backslash \Gamma_{a_1, \ldots, a_d })$, and moreover, the condition $\mathrm{dist} (\Lambda_1, \Gamma_{a_1, \ldots, a_d }) > 0$ ensures that $\inf_{\lambda_1 \in \Lambda_1} | 1-e^{2\pi i (a_1, \ldots, a_d) \cdot \lambda_1} | > 0$. Now, since $\Lambda_1$ is a set of interpolation for $PW(S_1)$, there exists a function $f_1 \in PW(S_1)$ satisfying $f_1(\lambda_1) = \frac{1}{ 1-e^{2\pi i (a_1, \ldots, a_d) \cdot \lambda_1} } \big(b_{\lambda_1}-g(\lambda_1) \big)$ for all $\lambda_1 \in \Lambda_1$. We then have $f(\lambda_1) = b_{\lambda_1}$ for all $\lambda_1 \in \Lambda_1$. This shows that the function $f \in PW (S_1 \cup S_2)$ satisfies $f(\lambda) = b_{\lambda}$ for all $\lambda \in \Lambda_1 \cup \Lambda_2$, as desired.

Riesz basis. By definition, a Riesz basis is a complete Riesz sequence. Hence, this part follows immediately by combining the completeness and Riesz sequence parts.

8.   Proof of Proposition 9

(a) Frame. Fix any $f \in L^2(\cup_{\ell \in \mathcal{L}} \, S{+}\frac{\ell}{N})$. Then, for any $\lambda \in \Omega {+} c_k \; (\subset N \mathbb{Z} {+} c_k)$ with $1 \leq k \leq K$,

where

Then,

and since $E(\Omega)$ is a frame for $L^2(S)$ with bounds $0 < A \leq B < \infty$, we use Proposition 6 to obtain

Collecting (8.1) for $k = 1, \ldots, K$, we get

(ⅰ) If $K < L$, then the kernel of $W_{\mathcal{K}, \mathcal{L}} \in \mathbb C^{K \times L}$ has dimension at least $L {-} K > 0$. Fix any nontrivial vector ${\boldsymbol{v}} \in \mathrm{ker} (W_{\mathcal{K}, \mathcal{L}}) \subset \mathbb C^{L}$ and set $f \in L^2(\cup_{\ell \in \mathcal{L}} \, S{+}\frac{\ell}{N})$ by $[f (t {+} \frac{\ell}{N})]_{\ell \in \mathcal{L}} = {\boldsymbol{v}}$ for $t \in S$, so that $\sum_{k = 1}^K \| F_{k} \|_{L^2(S)}^2 = 0$ by (8.2) while $\| f \|_{L^2(\cup_{\ell \in \mathcal{L}} \, S{+}\frac{\ell}{N})}^2 = |S| \cdot \| {\boldsymbol{v}} \|_2^2 \neq 0$. Hence, the system $E(\cup_{k = 1}^K \Omega {+} c_k)$ is not a frame for $L^2(\cup_{\ell \in \mathcal{L}} \, S{+}\frac{\ell}{N})$. Note that since $K < L$, the matrix $W_{\mathcal{K}, \mathcal{L}} \in \mathbb C^{K \times L}$ is not injective.

(ⅱ) If $K \geq L$, then

Integrating both sides with respect to $t$ gives

and therefore,

This shows that if $K \geq L$, then $E(\cup_{k = 1}^K \Omega {+} c_k)$ is a frame for $L^2(\cup_{\ell \in \mathcal{L}} \, S{+}\frac{\ell}{N})$ if and only if the (tall) matrix $W_{\mathcal{K}, \mathcal{L}} \in \mathbb C^{K \times L}$ is injective.

(b) Riesz sequence. For any ${\boldsymbol{b}} = \{ b_\lambda \} \in \ell_2 (\cup_{k = 1}^K \Omega {+} c_k)$, we set

Then, for $t \in [0, \frac{1}{N})$ and $\ell \in \{ 0, 1, \cdots, N{-}1 \}$, we have

Collecting this equation for $\ell \in \mathcal{L}$, we obtain

(ⅰ) If $K > L$, then the kernel of $(W_{\mathcal{K}, \mathcal{L}})^* \in \mathbb C^{L \times K}$ has dimension at least $K {-} L > 0$. Fix any nontrivial vector ${\boldsymbol{v}} = \{ v_k \}_{k = 1}^K \in \mathrm{ker} ((W_{\mathcal{K}, \mathcal{L}})^*) \subset \mathbb C^{K}$. For each $k = 1, \ldots, K$, the system $E(\Omega {+} c_k)$ is a Riesz basis for $L^2(S)$, so there exists a unique sequence $\{ b_\lambda \} \in \ell_2 (\Omega {+} c_k)$ such that $\sum_{\lambda \in \Omega + c_k} b_\lambda \, e^{2\pi i \lambda t} = v_k$ for a.e. $t \in S$. We thus obtain a nontrivial sequence ${\boldsymbol{b}} = \{ b_\lambda \} \in \ell_2 (\cup_{k = 1}^K \Omega {+} c_k)$ satisfying

The corresponding function $f$ satisfies $\| f \|_{L^2(\cup_{\ell \in \mathcal{L}} \, S{+}\frac{\ell}{N})}^2 = \int_S \sum_{\ell \in \mathcal{L}} | f (t {+} \frac{\ell}{N})|^2 \, dt = 0$ by (8.3) while $\| {\boldsymbol{b}} \|_2 \neq 0$. Hence, the system $E(\cup_{k = 1}^K \Omega {+} c_k)$ is not a Riesz sequence in $L^2(\cup_{\ell \in \mathcal{L}} \, S{+}\frac{\ell}{N})$. Note that since $K > L$, the matrix $W_{\mathcal{K}, \mathcal{L}} \in \mathbb C^{K \times L}$ is not surjective.

(ⅱ) If $K \leq L$, then

where we used that $\sigma_{\min}^2 ((W_{\mathcal{K}, \mathcal{L}})^*) = \sigma_{\min}^2 (W_{\mathcal{K}, \mathcal{L}})$. Further, integrating both sides with respect to $t$ gives

where we used that $E(\Omega)$ is a Riesz basis for $L^2(S)$ with lower Riesz bound $A > 0$. This shows that if $K \leq L$, then $E(\cup_{k = 1}^K \Omega {+} c_k)$ is a Riesz sequence in $L^2(\cup_{\ell \in \mathcal{L}} \, S{+}\frac{\ell}{N})$ if and only if the (short and fat) matrix $W_{\mathcal{K}, \mathcal{L}} \in \mathbb C^{K \times L}$ is surjective.

(c) Riesz basis. Recall that a sequence in a separable Hilbert space is a Riesz basis if and only if it is both a frame and a Riesz sequence. Hence, this part follows immediately by combining the frame and Riesz sequence parts.

9.   Conclusions

In this paper, we addressed the problem of constructing exponential Riesz bases by combining or splitting existing ones. Through carefully designed examples, we showed that combining or splitting does not generally yield new exponential Riesz bases, highlighting the delicate nature of exponential Riesz bases. As our main results, we proved some sufficient conditions under which two exponential Riesz bases can be combined by simply taking unions of their frequency sets and domains, respectively. In addition, we discussed some generalizations of these results to higher dimensions.

Use of AI tools declaration

The author declares that he has not used artificial intelligence (AI) tools in the creation of this article.

Acknowledgments

This work was supported by the National Research Foundation of Korea (NRF) grant funded by the Korean government (MSIT) (RS-2023-00275360). The proof of Theorem 1 is essentially credited to David Walnut. The author would like to thank David Walnut, Goetz E. Pfander, and Andrei Caragea for fruitful and stimulating discussions. Theorem 4 is inspired by a personal communication with Laura De Carli. The author would like to thank her for helpful comments.

Conflict of interest

The author declares no conflict of interest.