Energy utilization area under Complex q-rung orthopair fuzzy soft information

1.   Introduction

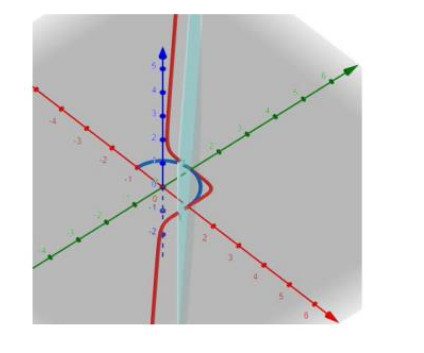
Uncertainty plays a significant role in the decision-making process for individuals. It can result in erroneous choices and impede the successful execution of plans. This hesitation arises when an individual is unable to accurately predict the outcomes of their actions with complete confidence. To reduce this uncertainty and ambiguines, in 1965, Zadeh ^[1] introduced a new fabulous concept named fuzzy set (FS) which is a historical invention in mathematics history. It gives a membership degree to each element whose values belong to a close interval [0, 1]. Fuzzy sets are used in various fields such as physics ^[2], medication ^[3], and computer science ^[4]. The fuzzy set theory leads the experts to handle and model uncertainty in different ways. Then, the concept of fuzzy relations (FRs) ^[5] was introduced that is not giving incomplete information about any relation. It explains the power and quality of good or bad relations. In 2016, a new idea called intuitionistic fuzzy set (IFS) ^[6] deliberates the two things i.e., membership as well as non-membership of any object. It is a bigger form of FS. Then, an innovative concept of intuitionistic fuzzy relations (IFRs) ^[7] was revealed which expounds on the effectiveness and ineffectiveness of any relation at the same time. A notion of the Pythagorean fuzzy set (PyFS) ^[8] revealed that increases the space of membership and non-membership by stately some new constraint i.e., the sum of the squares of membership and non-membership must be in the closed interval [0, 1]. After this, Pythagorean fuzzy relation (PyFR) is defined ^[9], which came after the CP of two PyFSs. It deals with problems better than IFRs. The concepts of q-rung orthopair fuzzy set (qROFSs) ^[10] are introduced, by eliminating the boundaries executed to the IFS and PyFS. This newly defined set permits specialists and scholars to easily give membership and non-membership degrees. The sum of the nth power of membership and non-membership degrees in qROFSs must be in the unit interval, with $n$ being a natural number. Then, the concept of q-rung orthopair fuzzy relation (qROFR) ^[11] was revealed. In this structure, everyone makes choices freely and the sum of the nth power of all choices belongs to the closed unit interval. Further, a review of qROF information ^[12] and information measures for qROFS ^[13] is explained. The comparison of the spaces of IFS, PyFS, and q-ROPFS is described in Figure 1 where the light turquoise color line represents the boundary space of IFS and the light blue semicircle shows the boundary space of PyFS while the red curve shows the space of q-ROPFS for q = 7.

After FS, a novel revolution in fuzzy algebra which is a broader form of fuzzy set announced known as complex fuzzy set (CFS) ^[14] that characterizes the membership degrees in the form of a complex number and involves two terms called amplitude term and phase term. It can solve multi-dimensional problems. Additionally, he also expresses complex fuzzy relations (CFRs). Additionally, In ^[15], a new and advanced computing method for time-series forecasting called the "complex neurofuzzy autoregressive integrated moving average (ARIMA)" is presented. This method integrates a complex neurofuzzy system (CNFS) and ARIMA models, resulting in the CNFS-ARIMA model. The use of CFSs in the CNFS further enhances the proposed approach. The work of Zhang et al. ^[16] added to the understanding of the method by examining its operational properties and proposing a distance measure for CFSs based on the difference in grades and phases of the two CFSs. Further, Complex intuitionistic fuzzy sets (CIFSs) ^[17] deal with which membership and non-membership indicate in the form of complex numbers. Complex intuitionistic fuzzy relation (CIFR) ^[18] was introduced with an application to examine cyber security and cybercrimes in the oil and gas sector. For example, CIFS is represented by quaternion numbers ^[19]. Then, a complex Pythagorean fuzzy set (CPyFS) ^[20] came into fuzzy algebra by generalizing the codomain of CIFS. Then, complex Pythagorean fuzzy relation (CPyFR) ^[21] is introduced as a broader form of CIFRs. It has more choices than CIFRs. Further, the expansion of the CPyFS made a complex q-rung orthopair fuzzy set (CqROFS) ^[22]. The complex-valued membership and non-membership degrees are allocated to the elements of a set in CqROFS, the sum of the modulus of membership and non-membership degrees must be within the unit interval, where $n$ is a natural number. After this, complex q-rung orthopair fuzzy relation (CqROFR) was revealed ^[23]. It has nth power of membership and non-membership. It is used in power combination operatives ^[24] and also in assemblage decision construction ^[25].

A novel concept of fuzzy soft set (FSS) ^[26] is given by mixing the FS and soft set. It decreases vagueness in daily life decisions and helps humans to make better choices. A note on SSs, rough SSs, and FSSs ^[27] is written comprehensively and made an adjustable approach to FSS decision-making ^[28]. After this, the novel concept of fuzzy soft relations (FSRs) ^[29] familiarized which is the combination of FRs and soft relations. An application of FSRs in decision-making ^[30] proposed and made approximations of FSSs by FSRs with image process application ^[31]. Then, an intuitionistic fuzzy soft set (IFSS) ^[32] was introduced which deals with both membership and non-membership of an element. After this, intuitionistic fuzzy soft relation (IFSR) ^[33] was introduced. It involves the effectiveness and ineffectiveness of a relation using simple values with a single dimensional. An extended form of IFSS is the Pythagorean fuzzy soft set (PyFSS) ^[34]. In this set, take the sum of the square of membership and non-membership. A new concept of q-rung orthopair fuzzy soft set (qROFSS) ^[35] was revealed as an extension of PyFSS and IFSS. It has nth power and a lot of options to make choices freely. A new idea of CFS set (CFSS) ^[36] defined which degree of membership is in the form of complex numbers and solves multi-dimensional problems. A systematic review of CFS and complex fuzzy logic ^[37] is being discussed. After this, an innovation named complex intuitionistic fuzzy soft set (CIFSS) ^[38] was introduced and explains both membership and non-membership.

The CqROFSS is a more comprehensive representation of the CIFSS and CPyFSS. The CqROFSS includes not only the amplitude component, which is absent in the qROFSS, but also the phase term. The versatility of the CqROFSS has led to its use in a variety of real-life applications, such as the economic modeling of electricity generation, as discussed by An et al ^[39] in their work using long short-term memory techniques. Mikhaylov et al. ^[40] analyzed the interdependence of oil prices and exchange rate movements of oil exporting countries using iteration-enhanced collaborative filtering, golden cut bipolar for analyzing the risk-based oil market spillovers. Mikhaylov et al. ^[41] extend the qROFSs in the form of bipolar qROFSs with the investigation of financial development and open invention-oriented fintech potential for emerging economies. The bipolar qROFSs also define both positive and negative degrees. Further, Candila et al. ^[42] discussed the relationship between oil and exchange rates of oil-exporting and oil-importing countries during the period of the Covid-19 era. Dinçer et al. ^[43] examination of renewable-friendly smart grid technologies by using a hybrid picture fuzzy rough decision-making method. Yang et al. ^[44] examine the influence factors of the Chinese electric vehicle market. Liu et al. ^[45] discussed the spatio-temporal distribution of Chinese cities' air quality and the impact of high-speed rail. Yang et al. ^[46] estimate alternative low-carbon fuel technologies using a stakeholder participation-based q-rung orthopair linguistic multi-criteria framework.

The motivation and contribution of the proposed work are as follows:

1) The CqROFS theory has demonstrated its ability to effectively model both uncertainty and periodicity in source data. However, it has limitations in handling situations involving graded parametrizations, as it is not equipped to address such complexities. To address this issue, we propose a new theory that is specifically designed to handle 2-dimensional vague data with parameterized fuzziness. This makes our proposed theory a more advanced solution compared to the CqROFS model.

2) The impact of CqROFSSs in the fields of uncertainty management, decision-making, and information processing is significant. These sets provide a flexible framework for modeling complex and ambiguous issues, with the use of complex numbers enhancing their ability to address such problems.

3) The implementation of CqROFSSs represents a significant advancement in the field of uncertainty management and decision-making. Their ability to handle complex and multi-dimensional difficulties, combined with their versatility, make them valuable assets for various applications and industries.

4) We have introduced the concept of CqROFSRs through the analysis of two CqROFSRs in CP.

5) We have outlined various types of CqROFSRs, including the CqROFS-reflexive relation, CqROFS-symmetric relation, CqROFS-transitive relation, CqROFS-equivalence relation, and CqROFS-partial order relation, among others. Each definition has been accompanied by relevant examples for clarity.

6) A multitude of results have been presented for the various types of CqROFSRs.

7) The CqROFSR concept represents a novel approach, offering superior advantages when compared to pre-existing structures such as SS, CFSS, CIFSS, and CPyFSS. The CqROFSRs discussed to provide a more comprehensive evaluation of both membership and non-membership degrees, with an increased dimensionality. Furthermore, they are capable of handling problems with multiple variables through the use of complex-valued mappings.

8) To verify the reliability of our proposed work, we have conducted a comparison with existing studies in the field.

Based on the information presented, it is evident that the proposed solution possesses immense value and practicality in managing ambiguous and uncertain information. Furthermore, it has been demonstrated that the proposed solution is capable of addressing problems that cannot be resolved by traditional techniques, such as FSs, IFSs, CFS, and CIFSs.

The sequential framework of the preceding paper is as follows: Section 1 introduces the paper and explains the history of the proposed concept. Section 2 completely describes the pre-existing frameworks of fuzzy algebra. Section 3 spells out the main results of the paper. Section 4 proposed an application to choose the best ES. Section 5 consists of the comparative study of the proposed concept with pre-existing concepts. Section 6 concludes the paper.

2.   Basic concepts

In the present section, we have established foundational concepts in fuzzy algebra, which will serve as a basis for further examination and exploration.

Definition 1: ^[1] A FS $F$ on a universe $Z$ is defined as:

Where $\mu \left(ῠ\right)$ is known as the membership degree of $ῠ$ and $0\le \mu \left(ῠ\right)\le 1$.

Definition 2: ^[14] A CFS $F$ on a universe $Z$ is defined as:

Where ${\mu }_{c}\left(ῠ\right)$ is known as the membership degree of $ῠ$ and $0\le \left|{\mu }_{c}\left(ῠ\right)\right|\le 1$. Also, ${\mu }_{c}\left(ῠ\right)$ can be written as ${\mu }_{c}\left(ῠ\right) = \alpha {e}^{i2\pi p}$, where $\alpha$ is known as amplitude term and $p$ is known as phase term of $ῠ$ with condition $0\le \alpha, p\le 1$.

Definition 3: ^[6] An IFS $F$ on a universe $Z$ is defined as:

Where ${\mu }_{c}\left(ῠ\right)$ is known as the membership degree of $ῠ$ and ${\tau }_{c}\left(ῠ\right)$ is known as the non-membership degree of $ῠ$ and $0\le \mu \left(ῠ\right)+\tau \left(ῠ\right)\le 1$.

Definition 4: ^[17] A CIFS $F$ on a universe $Z$ is defined as:

Where ${\mu }_{c}\left(ῠ\right)$ is known as membership degree of $ῠ$ and ${\tau }_{c}\left(ῠ\right)$ is known as non-membership degree of $ῠ$ and $0\le \left|{\mu }_{c}\left(ῠ\right)\right|+\left|{\tau }_{c}\left(ῠ\right)\right|\le 1$.

Definition 5: ^[20] A CPyFS $F$ on a universe $Z$ is defined as:

Where ${\mu }_{c}\left(ῠ\right)$ is known as membership degree of $ῠ$ and ${\tau }_{c}\left(ῠ\right)$ is known as the non-membership degree of $ῠ$. On a condition with $0\le {\left|{\mu }_{c}\left(ῠ\right)\right|}^{2}+{\left|{\tau }_{c}\left(ῠ\right)\right|}^{2}\le 1$.

Definition 6: ^[22] A CqROFS $F$ on a universe $Z$ is defined as:

Where ${\mu }_{c}\left(ῠ\right)$ is known as the membership degree of $ῠ$ and ${\tau }_{c}\left(ῠ\right)$ is known as the non-membership degree of $ῠ$ with a circumstance $0\le {\left|{\mu }_{c}\left(ῠ\right)\right|}^{n}+{\left|{\tau }_{c}\left(ῠ\right)\right|}^{n}\le 1$ and $n$ is a positive integer.

Definition 7: ^[26] Let $Z$ be the universe and $E$ be the set of parameters. Then, FSS $\left(\vartheta, F\right)$ and $F\subseteq E$ with a mapping $\vartheta :E\to Ᵽ\left(Z\right)$, (where $Ᵽ\left(Z\right)$ is the set of entirely possible subsets of $Z$) is defined as:

Definition 8: ^[36] Let $Z$ be the universe and $E$ be the set of parameters. Then, CFSS $\left(\vartheta, F\right)$ and $F\subseteq E$ with a mapping $\vartheta :E\to Ᵽ\left(Z\right)$, (where $Ᵽ\left(Z\right)$ is the set of entirely possible subsets of $Z$) is defined as:

Definition 9: ^[38] Let $Z$ be the universe and $E$ be the set of parameters. Then, CIFSS $\left(\vartheta, F\right)$ and $F\subseteq E$ with a mapping $\vartheta :E\to Ᵽ\left(Z\right)$, (where $Ᵽ\left(Z\right)$ is the set of entirely possible subsets of $Z$) is defined as:

With a condition $0\le {\alpha }_{\mu }\left(ῠ\right)+{\alpha }_{\tau }\left(ῠ\right)\le 1$ and $0\le {p}_{\mu }\left(ῠ\right)+{p}_{\tau }\left(ῠ\right)\le 1$.

Example 1: Consider the universal set consisting of three types of cellphones i.e., ${ẑ}_{1} = apple$, ${ẑ}_{2} = vivo$, ${ẑ}_{3} = oppo$. Then, $Z = \left\{{ẑ}_{1}, {ẑ}_{2}, {ẑ}_{3}\right\}$ and their parameter is ${ʈ}_{1} = batter~~yage$, ${ʈ}_{2} = processor$ and ${ʈ}_{3} = screen~~length$. Then, set of parameters $E = \left\{{ʈ}_{1}, {ʈ}_{2}, {ʈ}_{3}\right\}$. Let the observations given by experts is as follows:

Let their corresponding CIFSS as follows:

Definition 11: Let $\left(Ᵽ, Q\right)$ and $\left(Ṩ, Ṯ\right)$ be two CIFSSs on $Z$ and $E$ be the set of parameters. Then, the CP is $\left(Ᵽ, Q\right)\times \left(Ṩ, Ṯ\right) = \left(F, Q\times Ṯ\right)$ and $Q, Ṯ\subseteq E$ where $F:Q\times Ṯ\to C\left(Z\right)$ i.e., $Ᵽ = \left\{\left(ῠ, \left({\mu }_{c}\left(ῠ\right), {\tau }_{c}\left(ῠ\right)\right)\right), ῠ\in F\wedge {\mu }_{c}\left(ῠ\right), {\tau }_{c}\left(ῠ\right)\in Ᵽ\left(Z\right)\right\}$ and $Ṩ = \left\{\left(y, \left({\mu }_{c}\left(𝓎\right), {\tau }_{c}\left(𝓎\right)\right)\right), 𝓎\in F\wedge {\mu }_{c}\left(𝓎\right), {\tau }_{c}\left(𝓎\right)\in Ᵽ\left(Z\right)\right\}$

With a condition ${\mu }_{c}\left(ῠ, 𝓎\right) = min\left\{{\mu }_{c}\left(ῠ\right), {\mu }_{c}\left(𝓎\right)\right\}$ and ${\tau }_{c}\left(ῠ, 𝓎\right) = max\left\{{\tau }_{c}\left(ῠ\right), {\tau }_{c}\left(𝓎\right)\right\}$ and $0\le \left|{\mu }_{c}\left(ῠ\right)\right|+\left|{\tau }_{c}\left(ῠ\right)\right|\le 1$ and $0\le \left|{\mu }_{c}\left(ῠ\right)\right|+\left|{\tau }_{c}\left(ῠ\right)\right|\le 1$.

Any subset of CP of two CIFSSs is called CIFSR.

Example 2: From example 1, take CIFSS

Then, self CP of $Ṯ$ (CIFS relation) is given as,

3.   Main results

In this section, we explain the innovative concept of CP of two CqROFSSs, CqROFSRs, and different types of relations.

Definition 12: Let $Z$ be the universe and $E$ be the set of parameters. Then, CqROFSS $\left(\vartheta, F\right)$ and $F\subseteq E$ with a mapping $\vartheta :E\to Ᵽ\left(Z\right)$, (where $Ᵽ\left(Z\right)$ is the set of entirely possible subsets of $Z$) is defined as:

With a condition $0\le {\left({\alpha }_{\mu }\left(ῠ\right)\right)}^{n}+{\left({\alpha }_{\tau }\left(ῠ\right)\right)}^{n}\le 1$ and $0\le {\left({p}_{\mu }\left(ῠ\right)\right)}^{n}+{\left({p}_{\tau }\left(ῠ\right)\right)}^{n}\le 1$.

Example 3: Consider the universal set consists of three types of line coding schemes i.e., ${ẑ}_{1} = unipolar$, ${ẑ}_{2} = bipolar$, ${ẑ}_{3} = multilevel$. Then, $Z = \left\{{ẑ}_{1}, {ẑ}_{2}, {ẑ}_{3}\right\}$ and their parameter is ${ʈ}_{1} = bandwidth$, ${ʈ}_{2} = complexity$ and ${ʈ}_{3} = baseline~~wandering$. Then, a set of parameters $E = \left\{{ʈ}_{1}, {ʈ}_{2}, {ʈ}_{3}\right\}$. Let the observations given by experts be represented as follows:

Let their corresponding CqROFSS be stated as follows:

Definition 13: Let $\left(Ᵽ, Q\right)$ and $\left(Ṩ, Ṯ\right)$ be two CPyFSSs on $Z$ and $E$ be the set of parameters. Then, the CP is $\left(Ᵽ, Q\right)\times \left(Ṩ, Ṯ\right) = \left(F, Q\times Ṯ\right)$ and $Q, Ṯ\subseteq E$ where $F:Q\times Ṯ\to C\left(Z\right)$ i.e., $Ᵽ = \left\{\left(ῠ, \left({\mu }_{c}\left(ῠ\right), {\tau }_{c}\left(ῠ\right)\right)\right), ῠ\in F\wedge {\mu }_{c}\left(ῠ\right), {\tau }_{c}\left(ῠ\right)\in Ᵽ\left(Z\right)\right\}$ and $Ṩ = \left\{\left(𝓎, \left({\mu }_{c}\left(𝓎\right), {\tau }_{c}\left(𝓎\right)\right)\right), 𝓎\in F\wedge {\mu }_{c}\left(𝓎\right), {\tau }_{c}\left(𝓎\right)\in Ᵽ\left(Z\right)\right\}$

With a condition ${\mu }_{c}\left(ῠ, 𝓎\right) = min\left\{{\mu }_{c}\left(ῠ\right), {\mu }_{c}\left(𝓎\right)\right\}$ and ${\tau }_{c}\left(ῠ, 𝓎\right) = max\left\{{\tau }_{c}\left(ῠ\right), {\tau }_{c}\left(𝓎\right)\right\}$ and $0\le {\left|{\mu }_{c}\left(ῠ\right)\right|}^{n}+{\left|{\tau }_{c}\left(ῠ\right)\right|}^{n}\le 1$ and $0\le {\left|{\mu }_{c}\left(ῠ\right)\right|}^{n}+{\left|{\tau }_{c}\left(ῠ\right)\right|}^{n}\le 1$, where $n\ge 1$.

Example 4: Take set $Ṯ$ from example 3, then

Then, self CP of $Ṯ$ is given as,

Definition 14: Any subset of CP of two CqROFSSs is called CqROFSRs.

Example 5: The CqROFSR is as follows;

Definition 15: A CqROFS relation $R$ on $\left(\vartheta, F\right)$ with universe $Z$ is said to be CqROFS-reflexive- $R$ if

Then, $\forall \left(\left(ῠ, ῠ\right), \left(\left({\mu }_{c}\left(ῠ, ῠ\right)\right), \left({\tau }_{c}\left(ῠ, ῠ\right)\right)\right)\right)\in R$.

Definition 16: A CqROFS relation $R$ on $\left(\vartheta, F\right)$ with universe $Z$ is said to be CqROFS-symmetric- $R$ if

If $\forall \left(\left(ῠ, 𝓎\right), \left(\left({\mu }_{c}\left(ῠ, 𝓎\right)\right), \left({\tau }_{c}\left(ῠ, 𝓎\right)\right)\right)\right)\in R$. Then, $\left(\left(𝓎, ῠ\right), \left(\left({\mu }_{c}\left(𝓎, ῠ\right)\right), \left({\tau }_{c}\left(𝓎, ῠ\right)\right)\right)\right)\in R$.

Definition 17: A CqROFS relation $R$ on $\left(\vartheta, F\right)$ with universe $Z$ is said to be CqROFS-transitive- $R$ if

If $\forall \left(\left(ῠ, 𝓎\right), \left(\left({\mu }_{c}\left(ῠ, 𝓎\right)\right), \left({\tau }_{c}\left(ῠ, 𝓎\right)\right)\right)\right)\in R$ and $\left(\left(𝓎, ẑ\right), \left(\left({\mu }_{c}\left(𝓎, ẑ\right)\right), \left({\tau }_{c}\left(𝓎, ẑ\right)\right)\right)\right)$.

Then, $\left(\left(ῠ, ẑ\right), \left(\left({\mu }_{c}\left(ῠ, ẑ\right)\right), \left({\tau }_{c}\left(ῠ, ẑ\right)\right)\right)\right)\in R$.

Definition 18: A CqROFS relation $R$ on $\left(\vartheta, F\right)$ with universe $Z$ is assumed to be CqROFS-irreflexive- $R$ if

Then, $\forall \left(\left(ῠ, ῠ\right), \left(\left({\mu }_{c}\left(ῠ, ῠ\right)\right), \left({\tau }_{c}\left(ῠ, ῠ\right)\right)\right)\right)\notin R$.

Definition 19: A CqROFS relation $R$ on $\left(\vartheta, F\right)$ with universe $Z$ is assumed to be CqROFS-antisymmetric- $R$ if

If $\forall \left(\left(ῠ, 𝓎\right), \left(\left({\mu }_{c}\left(ῠ, 𝓎\right)\right), \left({\tau }_{c}\left(ῠ, 𝓎\right)\right)\right)\right)\in R$ and $\left(\left(𝓎, ῠ\right), \left(\left({\mu }_{c}\left(𝓎, ῠ\right)\right), \left({\tau }_{c}\left(𝓎, ῠ\right)\right)\right)\right)\in R$

Implies that $\left(\left(ῠ, 𝓎\right), \left(\left({\mu }_{c}\left(ῠ, 𝓎\right)\right), \left({\tau }_{c}\left(ῠ, 𝓎\right)\right)\right)\right) =$ $\left(\left(𝓎, ῠ\right), \left(\left({\mu }_{c}\left(𝓎, ῠ\right)\right), \left({\tau }_{c}\left(𝓎, ῠ\right)\right)\right)\right)$.

Definition 20: A CqROFS relation $R$ on $\left(\vartheta, F\right)$ with universe $Z$ is assumed to be CqROFS-equivalence- $R$ if

▪ Reflexive

▪ Symmetric

▪ Transitive

Example 6: Take a relation from example 4 as:

is a CqROFS-equivalence-R.

Definition 21: A CqROFS relation $R$ on $\left(\vartheta, F\right)$ with universe $Z$ is assumed to be CqROFS-partial order- $R$ if

▪ Reflexive

▪ Anti-symmetric

▪ Transitive

Example 7: Take a relation from example 4 as:

is a CqROFS-partial order-R.

Definition 22: A CqROFS relation $R$ on $\left(\vartheta, F\right)$ with universe $Z$ is assumed to be CqROFS-pre order- $R$ if

▪ Reflexive

▪ Transitive

Definition 23: A CqROFS relation $R$ on $\left(\vartheta, F\right)$ with universe $Z$ is assumed to be CqROFS-complete- $R$ if

Then, $\forall \left(\left(ῠ, 𝓎\right), \left(\left({\mu }_{c}\left(ῠ, 𝓎\right)\right), \left({\tau }_{c}\left(ῠ, 𝓎\right)\right)\right)\right)\in R$ or $\left(\left(𝓎, ῠ\right), \left(\left({\mu }_{c}\left(𝓎, ῠ\right)\right), \left({\tau }_{c}\left(𝓎, ῠ\right)\right)\right)\right)\in R$

Definition 24: A CqROFS relation $R$ on $\left(\vartheta, F\right)$ with universe $Z$ is assumed to be CqROFS-linear order- $R$ if

▪ Reflexive

▪ Anti-symmetric

▪ Transitive

▪ Complete

Definition 25: A CqROFS relation $R$ on $\left(\vartheta, F\right)$ with universe $Z$ is assumed to be CqROFS-strict order- $R$ if

▪ Reflexive

▪ Transitive

Example 8: Take a relation from example 4 as follows:

is CqROFS-strict order-R.

Definition 26: Let $R$ be a CqROFS $R$ on a CqROFSS $Z$. For $ῠ\in F$, a CqROFS equivalence class of $ῠ$ mod $R$ is defined as:

Example 9: Take an equivalence relation from example 6,

Now, equivalence class of

• ${ʈ}_{1}$ mod $R$

• ${ʈ}_{2}$ mod $R$

• ${ʈ}_{3}$ mod $R$

Definition 27:

Let ${R}_{1}$ and ${R}_{2}$ be two CqROFS relations on CqROFS $Z$. Then, composition ${R}_{1}\circ {R}_{2}$ can be defined as:

$\left(\left(ῠ, 𝓎\right), \left\{{u}_{c}\left(ῠ, 𝓎\right), {\tau }_{c}\left(ῠ, 𝓎\right)\right\}\right)\in {R}_{1}$ and $\left(\left(𝓎, ẑ\right), \left\{{u}_{c}\left(𝓎, ẑ\right), {\tau }_{c}\left(𝓎, ẑ\right)\right\}\right)\in {R}_{2}$

This implies $\left(\left(ῠ, ẑ\right), \left\{{u}_{c}\left(ῠ, ẑ\right), {\tau }_{c}\left(ῠ, ẑ\right)\right\}\right)\in {R}_{1}\circ {R}_{2}$, $\forall ῠ, 𝓎, ẑ\in F$.

Definition 28: The converse of a CqROFSR $R$ is defined as:

Example 10: Take a relation from example 4,

Then, converse of $R$ is

Theorem 1: A CqROFSRs $R$ are a CqROFS-symmetric-R on a CqROFSS $Z$ iff $R = {R}^{c}$.

Proof: Let $R = {R}^{c}$, then $\left(\left(ῠ, 𝓎\right), \left\{{u}_{c}\left(ῠ, 𝓎\right), {\tau }_{c}\left(ῠ, 𝓎\right)\right\}\right)\in R$ iff $\left(\left(𝓎, ῠ\right), \left\{{u}_{c}\left(𝓎, ῠ\right), {\tau }_{c}\left(𝓎, ῠ\right)\right\}\right)\in {R}^{c}$

This implies $\left(\left(𝓎, ῠ\right), \left\{{u}_{c}\left(𝓎, ῠ\right), {\tau }_{c}\left(𝓎, ῠ\right)\right\}\right)\in R$.

Hence, $R$ is a CqROFS-symmetric-R on a CqROFSS $Z$.

Now, let $R$ is a CqROFS-symmetric-R on a CqROFSS $Z$. Then,

$\left(\left(ῠ, 𝓎\right), \left\{{u}_{c}\left(ῠ, 𝓎\right), {\tau }_{c}\left(ῠ, 𝓎\right)\right\}\right)\in R$ iff $\left(\left(𝓎, ῠ\right), \left\{{u}_{c}\left(𝓎, ῠ\right), {\tau }_{c}\left(𝓎, ῠ\right)\right\}\right)\in R$

However, $\left(\left(𝓎, ῠ\right), \left\{{u}_{c}\left(𝓎, ῠ\right), {\tau }_{c}\left(𝓎, ῠ\right)\right\}\right)\in {R}^{c}$

Hence, proved.

Theorem 2: A CqROFSRs $R$ are a CqROFS-transitive-R on a CqROFSS $Z$ iff $R\circ R\subseteq R$.

Proof: Necessary condition:

Let $R$ is a CqROFS-transitive-R on a CqROFSS $Z$. Suppose that

Then, by transitive condition of $R$

$\left(\left(ῠ, 𝓎\right), \left\{{u}_{c}\left(ῠ, 𝓎\right), {\tau }_{c}\left(ῠ, 𝓎\right)\right\}\right)\in R$ and $\left(\left(𝓎, ẑ\right), \left\{{u}_{c}\left(𝓎, ẑ\right), {\tau }_{c}\left(𝓎, ẑ\right)\right\}\right)\in R$

This implies $\left(\left(ῠ, ẑ\right), \left\{{u}_{c}\left(ῠ, ẑ\right), {\tau }_{c}\left(ῠ, ẑ\right)\right\}\right)\in R$ $⟹$ $R\circ R\subseteq R$.

Sufficient condition:

Assume that $R\circ R\subseteq R$, then

$\left(\left(ῠ, 𝓎\right), \left\{{u}_{c}\left(ῠ, 𝓎\right), {\tau }_{c}\left(ῠ, 𝓎\right)\right\}\right)\in R$ and $\left(\left(𝓎, ẑ\right), \left\{{u}_{c}\left(𝓎, ẑ\right), {\tau }_{c}\left(𝓎, ẑ\right)\right\}\right)\in R$

This implies $\left(\left(ῠ, ẑ\right), \left\{{u}_{c}\left(ῠ, ẑ\right), {\tau }_{c}\left(ῠ, ẑ\right)\right\}\right)\in R\circ R\subseteq R$

Then, $\left(\left(ῠ, ẑ\right), \left\{{u}_{c}\left(ῠ, ẑ\right), {\tau }_{c}\left(ῠ, ẑ\right)\right\}\right)\in R$

Hence, $R$ is CqROFS-transitive-R on $Z$.

Theorem 3: If $R$ is a CqROFS-equivalence- $R$ on a CqROFSS $Z$, then $R\circ R = R$.

Proof: Let $\left(\left(ῠ, 𝓎\right), \left\{{u}_{c}\left(ῠ, 𝓎\right), {\tau }_{c}\left(ῠ, 𝓎\right)\right\}\right)\in R$

Now, by using symmetry condition, $\left(\left(𝓎, ῠ\right), \left\{{u}_{c}\left(𝓎, ῠ\right), {\tau }_{c}\left(𝓎, ῠ\right)\right\}\right)\in R$

Then, by using transitive property of $R$, $\left(\left(ῠ, ῠ\right), \left\{{u}_{c}\left(ῠ, ῠ\right), {\tau }_{c}\left(ῠ, ῠ\right)\right\}\right)\in R$

However, by definition of composite relation $\left(\left(ῠ, ῠ\right), \left\{{u}_{c}\left(ῠ, ῠ\right), {\tau }_{c}\left(ῠ, ῠ\right)\right\}\right)\in R\circ R$

Conversely, suppose that $\left(\left(ῠ, 𝓎\right), \left\{{u}_{c}\left(ῠ, 𝓎\right), {\tau }_{c}\left(ῠ, 𝓎\right)\right\}\right)\in R\circ R$,

then $\exists ẑ\in X\ni \left(ῠ, 𝓎\right)\in R$. However, since $R$ is a CqROFS-equivalence-R on $Z$, $R$ is also a CqROFS-transitive-R $R$. Thus,

From (1) and (2),

Theorem 4: The converse of a CqROFS-partial order-R ${R}^{c}$ on CqROFSS $Z$ is also a CqROFS-partial order-R $R$ on $Z$.

Proof: It is sufficient to show that the converse of a CqROFS-partial order-R ${R}^{c}$ satisfies three properties of a CqROFS-partial order-R $R$.

I. Since, $R$ is a CqROFS-reflexive- $R$. Thus, for any element in $X$,

So, ${R}^{c}$ is reflexive.

II. Let $\left(\left(ῠ, ῠ\right), \left\{{u}_{c}\left(ῠ, ῠ\right), {\tau }_{c}\left(ῠ, ῠ\right)\right\}\right)\in {R}^{c}$ and $\left(\left(𝓎, ῠ\right), \left\{{u}_{c}\left(𝓎, ῠ\right), {\tau }_{c}\left(𝓎, ῠ\right)\right\}\right)\in {R}^{c}$

Then, $\left(\left(ῠ, 𝓎\right), \left\{{u}_{c}\left(ῠ, 𝓎\right), {\tau }_{c}\left(ῠ, 𝓎\right)\right\}\right)\in R$ and $\left(\left(𝓎, ῠ\right), \left\{{u}_{c}\left(𝓎, ῠ\right), {\tau }_{c}\left(𝓎, ῠ\right)\right\}\right)\in R$

Since, $R$ is CqROFS-anti-symmetric-R. Thus,

So, ${R}^{c}$ is anti-symmetric.

III. Let $\left(\left(ῠ, 𝓎\right), \left\{{u}_{c}\left(ῠ, 𝓎\right), {\tau }_{c}\left(ῠ, 𝓎\right)\right\}\right)\in {R}^{c}$ and $\left(\left(𝓎, ῠ\right), \left\{{u}_{c}\left(𝓎, ῠ\right), {\tau }_{c}\left(𝓎, ῠ\right)\right\}\right)\in {R}^{c}$

Then, $\left(\left(ẑ, 𝓎\right), \left\{{u}_{c}\left(ẑ, 𝓎\right), {\tau }_{c}\left(ẑ, 𝓎\right)\right\}\right)\in R$ and $\left(\left(𝓎, ῠ\right), \left\{{u}_{c}\left(𝓎, ῠ\right), {\tau }_{c}\left(𝓎, ῠ\right)\right\}\right)\in R$

Since, $R$ is a CqROFS relation. Thus,

So, ${R}^{c}$ is transitive.

Hence, proved.

4.   Application

This section is presenting an application to pick an area with more energy utilization by using CqROFSRs and the following process made it easy to select or pick. Firstly, take four types of areas as a universe and also take some parameters. Then, calculate the CP of the set of parameters to make relations. Finally, calculate the score function for best results.

4.1.   Energy utilization (EU)

Energy utilization focuses on technology that could result in novel and possibly more effective ways to use electricity in settings such as homes, businesses, and industries, as well as in the transportation industry. Energy affects every aspect of current life. Energy demand is growing exponentially due to the exponential development of the world inhabitants. The algorithm of further application is shown in Figure 2.

Now, there are four basic types of ESs that are very important in decision-making:

• EU in construction area: $\left({ẑ}_{1} = EUCA\right)$ Energy is used in two simple ways in buildings. About 50% is used for heating, cooling, and hot water in buildings. The remaining 50% is used to power building lighting and all device and tools such as refrigerators, commercial freezers, televisions, washing machines, and ovens. About 55% of building energy is used in private homes and the rest in marketable buildings such as offices, schools, and hospitals.

• EU in transportation area: $\left({ẑ}_{2} = EUTA\right)$ The transport sector has the smallest amount of variation in energy sources and uses. The transport sector also consumes relatively little bioenergy, gas, and electricity. 57% of transport energy employed by 'light commercial vehicles' includes both passenger cars and light trucks, the latter now accounting for 47% of the latest car sales. In urban areas, alternative non-petroleum-based energy sources are increasingly getting used for transport.

• EU in industry area: $\left({ẑ}_{3} = EUIA\right)$ The industrial sector is the most diverse, both in terms of the kinds of energy services required and the mix of energy sources used to provide those services. Several cross-cutting energy services are of particular importance in this sector.

1) Motor-driven equipment, which accounts for 64% of commercial power consumption.

2) Steam systems for heating products and driving mechanical processes.

3) When compressed gas system. Industrial energy use is concentrated during a few 'heavy industries', which generally produce raw materials that need considerable energy to move, process, and shape. About 37% of the first energy in the gas sector Electricity 15% Electricity 14% Heat 4% Biomass 14% Oil 41% Coal biomass and other energy sources.

• EU in residential area: $\left({ẑ}_{4} = EURA\right)$ Households require energy to power numerous household uses, but on average, other than half (51% in 2015) of a household's annual energy consumption is for heating and air conditioning, which comes from one energy end-use. These primarily seasonal and energy-intensive uses depend on geographic location, home size and construction, and appliances and fuels used. Hot water, lighting and cooling are practically universal and use energy in homes all year round. In 2015, these three end-users mutually accounted for 27% of his household's total annual energy consumption. The remaining 21% of household energy use comes from devices such as televisions, cookware, washing machines and tumble dryers, and consumer products such as computers, tablets, smartphones, video game consoles, and internet streaming strategies. The list of appliances also continues to grow.

Then, the universe of different areas of the EU is:

The pictorial representation of universe $Z$ discussed in Figure 3.

Now, explaining the parameters of the universe $Z$ are as follows:

• Technological advancement: ${ʈ}_{1} = TA$ The impact of technological advances on energy consumption is complex. Newer technologies are often more efficient and tend to reduce increased energy consumption. Energy consumption, on the other hand, can increase with the introduction of new machines and systems. For the economy, technological progress acts as a development stimulant. Technological development is the main driver of productivity growth, which in turn is the main driver of economic growth, accompanied by increased energy consumption.

• Energy prices: ${ʈ}_{2} = EP$ Energy prices affect both the energy mix and overall energy consumption. The rise in the price of an energy carrier may reduce its consumption and increase demand for other energy carriers. Within the short term, however, available technologies limit what proportion of consumption can be reduced and whether switching to another energy carrier is possible. Relatively large price volatility must be sustained over an extended period of time in order to be economically viable in reducing energy usage or switching energy sources. Even as electricity prices rise, people don't immediately replace inefficient refrigerators or install heat pumps.

• Population projections: ${ʈ}_{3} = PP$ population arrangement and population scattering also affect energy consumption. Urbanization is intended to remain, and the number of individuals existing in cities is expected to increase. According to Statistics Norway, the population of the center is likely to increase by 1.5 million from 4.2 million to 5.7 million by 2040. In additional urban areas, the population merely increases by 80, 000 over the identical period. As a result of urbanization, more people tend to live in multi-family dwellings, which typically take up less space in each and therefore use less energy for heating.

• Economic growth: ${ʈ}_{4} = EG$ Economic growth in e-commerce leads to an increased claim for things and facilities. This will increase energy claims together in the production of things and facilities and in the transportation of persons and things. While economic development, population growth, and energy claim have become less related in current years, the level of activity in the Norwegian economy will remain to have a tough effect on the development of energy demand.

The set of parameters discussed in Figure 4 below:

Now, the human experts assign some degrees to parameters on the basis of their effectiveness and ineffectiveness as follows:

In the above set $Ṯ$, the value of the first column of each row is described as the degrees assigned by the expert to ${ẑ}_{1}$, the value of the second column of each row is described as the degrees assigned by the expert to ${ẑ}_{2}$, the value of third column of each row is described as the degrees assigned by the expert to ${ẑ}_{3}$, the value of forth column of each row is described as the degrees assign by expert to ${ẑ}_{4}$ and the value of last column of each row is described as the degrees assigned by the expert to the system as general belongingness $\mathrm{\lambda }.$ The last one value is used just to calculate the score function. Otherwise, this value is not required. Then, take self-product of $Ṯ$ $\left(Ṯ\times Ṯ = R\right)$ given in Table 1.

The above Table 1 presents a comprehensive illustration of the self-Cortisone production of $Ṯ$. It accurately portrays the interdependence of various parameters and highlights the impact of alterations in one parameter on the others.

4.2.   Score function

Firstly, convert the complex values in real values. Here, $\pi$ is just a symbol and show the half cycle of a circle. Now, apply score formula for CqROFSS $\frac{1}{2}\left({{\alpha }_{\mu }}^{2}+{{p}_{\mu }}^{2}-{{\alpha }_{\tau }}^{2}-{{p}_{\tau }}^{2}\right)$, get the results in Table 2 as follows:

Now, to choose the area that utilizes more energy than others, select the highest numerical value from each and every row of the table but the last column of general belongingness is omitted. Now, each highest numerical value is multiplied with $\lambda$ and EU score is obtained. Then, the greatest score of the system of EU is the best area for utilizing a great amount of energy and making the finest decision making. The same type of parameters does not include in this process because an object compared with itself is not a good idea. Now, calculate the score function's last step to get results:

Finally, calculate the values

It is indicated that the residential sector ${ẑ}_{4}$ exhibits optimal utilization of energy compared to other areas. In the residential sector, energy is consumed for various purposes, including space and water heating, cooling, cooking, lighting, and electrical usage. The illustration in Figure 5 presents a comprehensive depiction of energy usage in the residential sector in the EU.

5.   Comparative study

In this section, a comparison of the proposed concept with prior concepts is provided to assess the superiority and advancement of the new concept relative to its predecessors.

5.1.   CqROFSRs with FSRs and CFSRs

Firstly, FSRs indicate the membership degree of relation. In other words, only show the effectiveness of any pair exist in relation. It indicates the consequence of one parameter on another and has a single dimension. It gives limited information about relation. Then, CFSRs solve the problem with complex numbers i.e., membership degree is in form of complex numbers. It is used to solve multi-dimensional problems but only discusses the effectiveness of relation. So, it also gives limited information. Now, take a CFSS as:

Then, take the self-product of $Ṯ$ $\left(Ṯ\times Ṯ = R\right)$ given in Table 3. This Table only illustrates the degree of membership, whereas the proposed concept encompasses both membership and non-membership degrees in space 2, thereby resolving issues associated with periodicity and multivariable.

5.2.   CqROFSRs with IFSRs and CIFSRs

Now, IFSRs discuss the relation with both membership and non-membership but only in a single dimension. It discusses the effectiveness and ineffectiveness of any relationship comprehensively. It is not able to solve problems with multi-dimensional variables. CIFSRs solve the problems with both membership and non-membership degrees and uses multi-dimensional variables. But both IFSRs and CIFSRs work in space $N = 1$. These have a lower range of values. The sum of both degrees lies in a closed interval [0, 1].

5.3.   CqROFSRs with PyFSRs and CPyFSRs

PyFSRs and CPyFSRs both deal with effectiveness and ineffectiveness by using single and multi-dimensions with space $N = 2$. Finally, qROFSRs deals with relation with a single dimension and $N = n, n\ge 1$. The sum of squares of both degrees contains in closed unit interval. The proposed concept is broader a form of all these discussed frameworks. It has membership and non-membership degrees with space $N = n, n\ge 1$. It uses multi-dimensions to resolve the harms and tells the periodicity of relation also. In this framework, specialists freely made choices based on the characteristics of an object and discussed the relation in extended form in Table 4.

6.   Conclusion

This article introduces the innovative concept of CqROFSS and CqROFSR, which stem from the composition of two CqROFSS. It encompasses all types of relations, including CqROFS-symmetric-R, CqROFS-reflexive-R, CqROFS-transitive-R, CqROFS-equivalence-R, CqROFS-partial order-R, CqROFS-linear order-R, CqROFS-composite-R, among others. Each type of relation is exemplified with appropriate examples, and a number of reliable results have been established. While our developed model presents a number of advantages over existing approaches, it is important to note that it is not without limitations. One such limitation is its inability to fully capture the nuances of abstention and refusal in inexact human expressions. Additionally, the methods utilized to solve multi-criteria decision-making problems in this model may prove computationally intensive, requiring extensive and time-consuming calculations. In future endeavors, we plan to expand upon our work by incorporating the concepts of complex picture fuzzy soft sets and complex spherical fuzzy soft sets. We envision utilizing these ideas in a variety of fields, such as computer science, physics, social sciences, electronics and among others ^[47,48].

Conflicts of interests

The authors have no conflicts of interest.

Acknowledgments

This work was supported by the Korea Institute of Energy Technology Evaluation and Planning (KETEP) grant funded by the Korean government (MOTIE) (20224000000070, Human Resource Training for Smart energy new industry cluster) and the Brain Pool program funded by the Ministry of Science and ICT through the National Research Foundation of Korea (2022H1D3A2A02060097).