1.
Introduction
The hypothesis of no-arbitrage is a fundamental principle in the study of mathematical finance. The characterizations of no-arbitrage have been studied in discrete time [1] and in continuous time [2,3]. For frictionless markets, the arbitrage-free condition is equivalent to the existence of risk-neutral probabilities such that the security is priced as its expected payoffs under specially chosen risk-neutral probabilities [4]. In the financial markets with the proportional transaction costs, the implication of no-arbitrage is characterized as the existence of a state price vector, which derives a spread instead of a unique price [5].
In order to describe the asset prices in more detail, several new concepts of arbitrage have been introduced, such as good deals [6], approximate arbitrage [7] and statistical arbitrage [8]. In an exchange economy with exogenous collateral requirements, a statistical arbitrage is defined by requiring the expected payoff of a portfolio not less than zero instead of a non-negative almost surely random payoff, and a narrower spread is obtained with the absence of statistical arbitrage than the one with the absence of arbitrage [9].
However, we note that the statistical arbitrage ignores the fact of certain risk, as mentioned in [9]. In reality, there may exist relatively large errors between the real and the expected payoff of a portfolio. Especially, the prices of assets in the portfolio can have large fluctuations due to certain major events, such as a financial crisis and natural disaster. In this situation, the portfolio's payoff is not very stable, i.e., the real payoff may deviate from its expectation to a large extent. Thus, it is natural to consider the corresponding risk.
On the other hand, roughly speaking, the no-arbitrage principle is equivalent to "if you want to get a positive return in the future, you must have a positive input at present". In fact, as an investor, they are more concerned about "what is the reasonable investment in order to achieve a certain level of return". In other words, the no-arbitrage principle basically defines the fairness and rationality of asset prices. Therefore, in order to obtain the reasonable asset prices, we must first establish an effective no-arbitrage criterion, so that, under the established criterion, the asset pricing is fair.
Motivated by the above statements, we think that the following questions are interesting.
1) How to characterize the risk that has not been taken into account in statistical arbitrage in [9]?
2) For a given initial input, what is a reasonable and fair future return?
In the present paper, we introduce the concept of excess profit relative to the benchmark asset under the α-confidence level (α-REP). An α-REP is a portfolio with the expected return rate more than the one of the benchmark asset.
Our innovation of this study is to propose a new concept of arbitrage, that is α-REP, while considering the risk of statistical arbitrage and combining the problem of future return for a given initial input. Indeed, an investor in the real market is usually more concerned about the above questions instead of the classical arbitrage opportunity. Thus, the results of this paper may provide more practical guidance for the investors.
The paper is organized as follows. Section 2 introduces the model and some basic notions. Section 3 obtains a fundamental theorem of asset pricing. Moreover, we discuss the relationships between classical arbitrage opportunity, strong statistical arbitrage opportunity and α-REP. Section 4 illustrates the rationality of the results by some examples.
2.
Model and basic notions
We consider a single-period capital market with n assets. All assets are traded in the beginning of period and returns are delivered in the end of period. The number of states is m and the state j can occur with the probability pj, j∈{1,2,⋯,m}. Let us denote the expectation with respect to the family of probabilities (pj)1≤j≤m as E(⋅). The current price of an unit of asset i is Si where Si≥0, i=1,2,⋯,n. One must pay the transaction fees with the proportional coefficients λi and μi for purchasing and selling an unit of asset i where 0≤λi,μi<1, i=1,2,⋯,n. Let xi be the number of units invested in asset i. The investor will buy xi units of asset i if xi≥0 and sell −xi units of asset i otherwise. The random payoff of asset i is Ri and the value of it in state j is Rij. Some notations are formalized as follows:
● S=(S1,S2,⋯,Sn)T is the vector of current asset prices.
● λ=(λ1,λ2,⋯,λn)T and μ=(μ1,μ2,⋯,μn)T are the vectors of proportional transaction fees for purchasing and selling, respectively.
● R=(Rij)n×m is the payoff matrix.
● (S,R,λ,μ) represents our economy.
● x=(x1,x2,⋯,xn)T∈Rn is the portfolio vector.
● r0 is the risk-free rate.
● ri is the expectation of Ri, i.e., ri=E(Ri)=m∑j=1Rijpj.
● σi is the standard deviation of Ri, i.e., σ2i=m∑j=1(Rij−ri)2pj.
● Rk+={x∈Rk:x≥0} and Rk++={x∈Rk:x>0}.
The cost function of asset i is denoted as ci(⋅), where
and the total cost of portfolio x∈Rn is
Then, let us recall some concepts and properties about arbitrage opportunity.
Definition 2.1. [5] The market (S,R,λ,μ) has an arbitrage opportunity (AO) if there exists a portfolio x∈Rn such that
with at least one strict inequality.
proposition 2.1. [5] The market (S,R,λ,μ) exhibits no arbitrage if and only if there exists a state price vector q=(q1,q2,⋯,qm)T∈Rm+, such that
holds for every i=1,2,⋯,n.
Indeed, (2.1) implies the following spread interval
From the Definition 2.1, we can see that AO is risk-free. In detail, the net profit RTx−c(x)em of such an AO x is non-negative at each state j, where em is the unit vector of m×1. Let us recall the concept of strong statistical arbitrage opportunity in [9].
Definition 2.2. [9] The market (S,R,λ,μ) has a strong statistical arbitrage opportunity (SSAO) if there exists a portfolio x∈Rn such that
As mentioned in [9], SSAO is risky. Considering the risk of statistical arbitrage and combining the problem 2) in the Introduction, we introduce the concept of α-REP. Before giving the explicit definition of α-REP, we recall the concept of cost of capital. The cost of capital can be regarded as the market rate of capitalization for the expected value of the uncertain streams [10]. Assume that the cost of capital of asset i is ki. Without loss of generality, ki≥r0, ∀ i=1,2,⋯,n. Let us denote k0=max1≤i≤nki. Then, α-REP is defined as follows.
Definition 2.3. Let a,b∈R+ and k∈[r0,k0] such that a(1+k)=b, where a is the given initial input and k is the expected return rate of the benchmark asset. Then the market (S,R,λ,μ) has an excess profit relative to the benchmark asset under α-confidence level (α-REP) if there exists a portfolio x∈Rn such that
where ε≥0 is the average risk of all assets and α∈(0,1) is a confidence level.
Remark 2.1. Essentially, the left side hand of (2.5) is a probabilistic risk measure of the portfolio x by taking the risk level θ=1 [11]. In the numerical computation, ε can be calibrated by the arithmetic average, i.e., ε=1nn∑i=1σi
Remark 2.2. An α-REP is a portfolio with the expected return rate more than the one of the benchmark asset since E(RTx)−c(x)c(x)>b−aa=k. In particular, the benchmark asset is exactly the risk-free asset when k=r0. Then, we may claim that the asset pricing under the principle of no α-REP is relatively "fair". Because the higher expected return rate (relative to the benchmark asset) is impossible in such a market without α-REP.
3.
Main results
3.1. Fundamental theorem of asset pricing
Let us order the values of random variable |Ri−ri| such that
where {i1,i2,⋯,im} is the rearrangement of {1,2,⋯,m}. Define
We can see that if |Riil∗i−ri|=0, then P{|Ri−ri|=0}≥α. It implies that the asset i is risk-free under the confidence level α. We keep it out of our consideration and assume, without loss of generality, that the inequality P{|Ri−ri|=0}<α holds for every i=1,2,⋯,n.
Lemma 3.1. The inequality (2.5) can be equivalently written as
where Ui=ε|Riil∗i−ri|>0.
Proof. The inequality (2.5) says that min1≤i≤nP{|Rixi−rixi|≤ε}≥α. Equivalently, P{|Rixi−rixi|≤ε}≥α must hold for every i=1,2,⋯,n. The case where xi=0 is trivial since P{ε≥0}=1 and Ui>0. For the case where xi≠0, it can be deduce that P{|Rixi−rixi|≤ε}=P{|Ri−ri|≤ε|xi|}. Let us consider the series |Rii1−ri|,|Rii2−ri|,⋯,|Riim−rm|, which are reordered as (3.1). Then, the condition P{|Ri−ri|≤ε|xi|}≥α holds if and only if ε|xi|≥|Riil∗i−ri| according to the definition of l∗i as (3.2). Finally, we can obtain that |xi|≤ε|Riil∗i−ri|=Ui as |Riil∗i−ri|≠0 from the assumption that P{|Ri−ri|=0}<α for every i=1,2,⋯,n. □
Theorem 3.1. The market (S,R,λ,μ) exists no α-REP if and only if there exists β=(β0,β1,⋯,β3n)T∈R1+3n+ such that the family of asset prices (Si)i=1,2,⋯,n satisfies the following equalities:
Proof. Let x=(x1,x2,⋯,xn)T∈Rn be a portfolio. We can rewrite the cost function of asset i as ci(xi)=(1+λi)Six+i−(1−μi)Six−i where x+i=max{xi,0} and x−i=max{−xi,0}. Then, the total cost of x is c(x)=n∑i=1(1+λi)Six+i−n∑i=1(1−μi)Six−i. On the other hand, E(RTx)=n∑i=1rixi=n∑i=1ri(x+i−x−i) as xi=x+i−x−i. From the Lemma 3.1, we know that the condition (2.5) can be written as |xi|=x+i+x−i≤Ui,∀i=1,2,⋯,n. Let us denote ˉx=(x+1,x−1,⋯,x+n,x−n,1)T∈R2n+1, C=((1+λ1)S1,−(1−μ1)S1,⋯,(1+λn)Sn,−(1−μn)Sn,−a)T∈R2n+1, and
Then, the absence of α-REP if and only if ∄ˉx∈R2n+1 such that Aˉx≥0 and CTˉx<0. By virtue of Farkas' Lemma, it is equivalent to ∃β=(β0,β1,⋯,β3n)T∈R1+3n+, such that
Finally, we can directly derive the Eqs (3.4)–(3.6) from (3.7). □
Corollary 3.1. If the market (S,R,λ,μ) exists no α-REP, then, for every i=1,2,⋯,n,
where β0 and β2n+i satisfies the equality (3.6).
3.2. The relationships between AO, SSAO and α-REP
Theorem 3.2. The absence of α-REP is equivalent to no SSAO when a=b=0. Equivalently, there exists ˉβ=(ˉβ0,ˉβ1,⋯,ˉβ2n)T∈R1+2n+ such that the family of asset prices (Si)i=1,2,⋯,n satisfies the following equalities:
Moreover, the spread
holds for every i=1,2,⋯,n.
Proof. The equality (3.6) in the Theorem 3.1 is n∑i=1Uiβ2n+i=0 when a=b=0. As Uiβ2n+i≥0 and Ui>0, then, β2n+i=0 for every i=1,2,⋯,n. Thus, (3.4) and (3.5) can be respectively written as (3.9) and (3.10) by taking ˉβ0=β0 and ˉβ2i−1=β2i−1. The spread (3.11) is obvious as ri=m∑j=1Rijpj. □
Theorem 3.3. If the market (S,R,λ,μ) satisfies the equivalent conditions of no α-REP (3.4), (3.5) and (3.6) with the extra assumptions
then, the property of no SSAO holds. Furthermore, the market (S,R,λ,μ) exhibits the property of no-arbitrage.
Proof. From the Theorem 3.1, the absence of α-REP is equivalent to exist β=(β0,β1,⋯,β3n)T∈R1+3n+ such that (3.4), (3.5) and (3.6) hold. If β2i−1,β2i≥β2n+i, we may take ˉβ2i−1=β2i−1−β2n+i∈R+, ˉβ2i=β2i−β2n+i∈R+ and ˉβ0=β0 such that (3.9) and (3.10) hold in the Theorem 3.2. This implies that a SSAO is impossible in the market. Furthermore, take the state price deflator qj=pjˉβ0 for every j=1,2,⋯,m, such that
Thus, (1−μi)Si≤m∑j=1Rijqj≤(1+λi)Si from (3.11) in the Theorem 3.2. By the Proposition 2.1, we can conclude that the market (S,R,λ,μ) exhibits no-arbitrage. □
4.
Some examples
Example 4.1. Consider a single-step binomial market with one risky asset. The current price of the asset is S=100. In the end of the period, the price S will go up to R1=105 with the probability p1=5455 and go down to R2=50 with the probability p2=155. Assume that λ=μ=0.25%, the risk-free rate r0=3% and the cost of capital of this risky asset k0=4%.
It can be proved that there is no AO in this market since we can find q1,q2∈R+ such that (2.1) in the Proposition 2.1 holds. That is
Indeed, we can take q1=p11+k0 and q2=p21+k0 such that R1q1+R2q2=100. As (1−μ)S=99.75 and (1+λ)S=100.25, it is obvious that (4.1) holds. Thus, we can conclude that this model satisfies the condition of no-arbitrage.
On the other hand, consider the α-REP with k=r0=3% and α=98%. The expected payoff of the asset is r=R1p1+R2p2=104 and ε=σ=√(R1−r)2p1+(R2−r)2p2=10√3155. Thus, it is easy to compute that U=2√3155≈1.5. Let us consider an investment behavior of buying one unit of this risky asset. The cost of it is c(x)=(1+λ)S=100.25. Let b=104, then a=b1+k=100.97>c(x). As |x|=1<U, we can conclude from the Definition 2.3 that x=1 is an α-REP.
The first example compares the difference between the classical arbitrage opportunity and the new proposed concept in this paper, α-REP. In detail, we show that an α-REP, especially the risk-free asset, is chosen as the benchmark asset, which is possible in a no-arbitragr market. As we can see, an α-REP is constructed by investing one unit of risky asset in this example.
Example 4.2. Consider a single-step binomial model with two risky assets. The current prices of assets are S1=60 and S2=80. In the end of the period, there are two states (up and down) occurring with the probabilities p1=p2=12. Assume that the price S1 will go up to R11=100 in the first state and go down to R12=50 in the second state. Similarly, for the second asset, R21=90 and R21=60, respectively. Furthermore, the proportional transaction costs are λ1=λ2=μ1=μ2=0.2%.
The expected payoffs of the assets are r1=R11p1+R12p2=75 and r2=R21p1+R22p2=75. Thus, the average risk ε=12(σ1+σ2)=20 since the standard deviations are respectively
and
Thereby, we can compute that U1=45 and U2=43.
Let us consider the α-REP with k=4%. If we take b=1040, then, a=b1+k=1000. Now we can write the equalities of (3.4), (3.5) and (3.6) as follows:
By the simple computation, we can deduce that
is a solution of the family of equalities (4.2). According to the Theorem 3.1, there is no α-REP (k=4%) in this market.
The second example is a numerical application of the Theorem 3.1. In detail, we give a market model satisfying the condition of no α-REP when the expected return rate of the benchmark asset is k=4%. From this point of view, no α-REP can be expected to become an effective and realizable no-arbitrage criterion for the asset pricing.
Example 4.3. Let us continue to consider the market model in the Example 4.2. The assumptions (3.12) can be written as β1,β2≥β5 and β3,β4≥β6. Then, it is easy to prove that there is no β=(β0,β1,β2,β3,β4,β5,β6)T∈R7+, such that
On the other hand, we can prove that there may exist SSAO and AO in this market. Indeed, the Eqs (3.9) and (3.10) in the Theorem 3.2 can not be satisfied simultaneously. That is to say, there is no ˉβ=(ˉβ0,ˉβ1,ˉβ2,ˉβ3,ˉβ4)T∈R5+, such that
Thus, the market does not satisfy the equivalent conditions of no SSAO in the Theorem 3.2.
Moreover, there is no state price vector q=(q1,q2)T∈R2+ such that (2.1) in the Proposition 2.1 holds. That is, the family of inequalities
has no solution. Thus, the market does not satisfy the equivalent conditions of no AO in the Proposition 2.1.
The third example builds the relationship from the practical point of view between SSAO, AO and α-REP. It shows that the extra assumptions (3.12) in the Theorem 3.3 are necessary. Indeed, we illustrate that a SSAO and an AO are both possible if the extra assumptions (3.12) fail, even though the market satisfies the condition of no α-REP.
5.
Conclusions
In this paper, a generalized concept of arbitrage, α-REP, is introduced. We establish a fundamental theorem of asset pricing with the absence of α-REP. The asset price relationships are given as a family of equalities. By comparing three different concepts of arbitrage mentioned in this paper, we find that with some extra assumptions, no α-REP is stronger than no SSAO and no-arbitrage.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
This study was supported by the Natural Science Basic Research Program of Shaanxi Province, China under Grant [number 2022JQ-071], and the Shanghai Planning Project of Philosophy and Social Science [number 2021EGL006]. The authors are grateful to the responsible editor and the anonymous referees for their valuable comments and suggestions.
Conflict of interest
All authors declare no conflicts of interest in this paper.











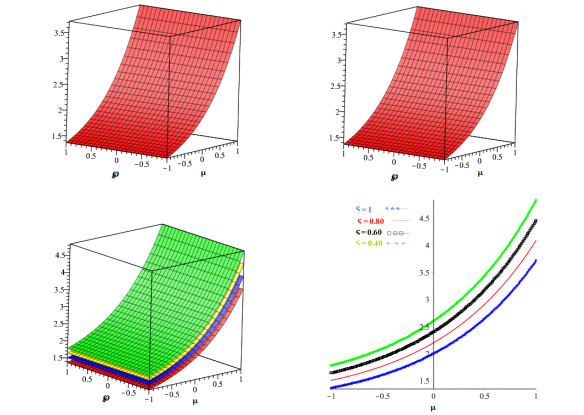
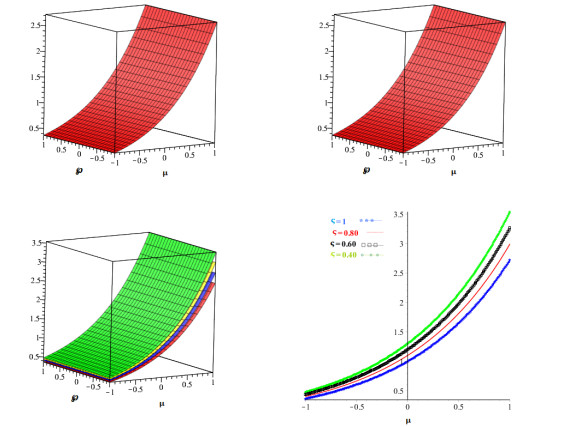
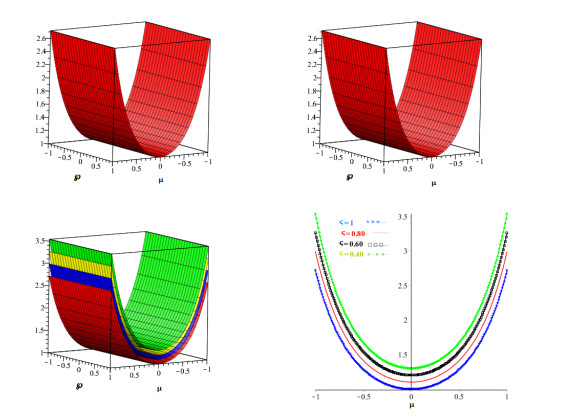


 DownLoad:
DownLoad: