A real representation method for special least squares solutions of the quaternion matrix equation $(AXB, DXE) = (C, F)$

1.   Introduction

In this article, we apply the following notations. $\mathbb{R}$ is the real number field. $\mathbb{R}^{m\times n}$ is the set of all $m\times n$ real matrices, and the set $\mathbb{R}^{m\times 1}$ is also denoted as $\mathbb{R}^m$. $\mathbb{R}_{CS}^{p\times q}$, $\mathbb{R}_{ACS}^{p\times q}$ are the sets of all $p\times q$ real centrosymmetric and anti centrosymmetric matrices, respectively. $\mathbb{Q}$ is the quaternion skew-field, and $\mathbb{Q}^{m\times n}$ is the set of all $m\times n$ quaternion matrices. $\mathbb{Q}_{CH}^{p\times q}$, $\mathbb{Q}_{SCH}^{p\times q}$ are the sets of all $p\times q$ quaternion centrohermitian and skew centrohermitian matrices, respectively. $C^{T}$, $\overline{C}$, $C^{†}$ and $R(C)$ denote the transpose, the conjugate, the Moore-Penrose inverse and the rank of the matrix $C$, respectively. $I_{k}$ denotes the $k\times k$ identity matrix. $J_k = (e_{k}, e_{k-1}, \cdots, e_{2}, e_{1})$, in which $e_i$ is the $i$ -th column of $I_k$. Let $A = (\alpha_{1}, \alpha_{2}, \cdots, \alpha_{n})\in \mathbb{R}^{m\times n}$, $vec(A) = (\alpha_{1}^{T}, \alpha_{2}^{T}, \cdots, \alpha_{n}^{T})^T$ is the vec operator. $\|\cdot\|$ stands for the matrix Frobenius norm. Let $B = (b_{ij})\in \mathbb{R}^{m\times n}, C\in \mathbb{R}^{p\times q}$, and then $B\otimes C = (b_{ij}C)\in \mathbb{R}^{mp\times nq}$ means the Kronecker product of $B$ and $C$. The $rand(m, n)$ is a function, which can generate a $m\times n$ random matrix in MATLAB.

Matrix equations are the main content of numerical algebra. They are widely used in computational mathematics, control theory, quantum physics, quantum mechanics, color image processing and so on. With the rapid development of these fields, matrix equations are widely studied ^{[1,2,3,4,5,6,7,8,9,10,11,12,13]}. In this article, our interest is the following matrix equation

in which $X$ is an unknown matrix, and others are given matrices with proper sizes. In recent years, this matrix equation has attracted much attention for its important role in pole assignment ^[14], measurement feedback ^[15], matrix programming problem ^[16] and so on. The main methods of solving (1.1) include the iterative method and the direct method. In this article, we are concerned with the latter.

Some significant results of the matrix equation (1.1) have been obtained by direct method. Navarra et al. ^[17] derived a representation of the general solution for the complex matrix equation (1.1). Liao et al. ^[18] gave an analytical expression of the minimal norm least squares solution for the real matrix equation (1.1) by the the generalized singular value decomposition. Yuan et al. ^[19,20] considered the minimal norm Hermitian least squares solution, $\eta$ -bi-Hermitian least squares solution and $\eta$ -anti-bi-Hermitian least squares solution for the quaternion matrix equation (1.1) by the complex representation method. Wang et al. ^[21] studied the minimal norm Hermitian least squares solution of the complex matrix equation (1.1) by a product of matrices and vectors. Zhang et al. ^[22] studied the same problems as ^[21] by the real representation matrices of quaternion matrices. Şimşek et al. ^[23] purposed the precise solutions on the minimum residual and matrix nearness problems of the quaternion matrix equation (1.1) for centrohermitian and skew centrohermitian matrices.

In addition, centrohermitian and skew centrohermitian matrices have wide applications in linear system theory, information theory, numerical analysis and linear estimate theory ^[24,25]. Therefore, for the quaternion matrix equation (1.1), it is valuable of the research of centrohermitian and skew centrohermitian solutions. It is generally known that the quaternion skew-filed $\mathbb{Q}$ is a non-commutative but associate algebra. Thus, many conclusions on real number field are invalid on the quaternion algebra. For example, the result $vec(AXB) = (B^{T}\otimes A)vec(X)$ does not hold on the quaternion algebra. Based on these, in the present paper, we will convert the quaternion least squares problems with constrained variables into the corresponding real least squares problems with free variables by using the real representations of quaternion matrices and relative properties, and give the solutions of corresponding problems.

We will consider the following two problems.

Problem I. Let $A\in \mathbb{Q}^{m\times p}$, $B\in \mathbb{Q}^{q\times s}, C\in \mathbb{Q}^{m\times s}, D\in \mathbb{Q}^{m\times p}$, $E\in \mathbb{Q}^{q\times t}, F\in \mathbb{Q}^{m\times t}$ and

Find $X_{CH}\in \mathbf{Q}_{CH}$ such that $\|X_{CH}\| = \min\limits_{X\in \mathbf{Q}_{CH}} \|X\|$.

Problem II. Let $A\in \mathbb{Q}^{m\times p}$, $B\in \mathbb{Q}^{q\times s}, C\in \mathbb{Q}^{m\times s}, D\in \mathbb{Q}^{m\times p}$, $E\in \mathbb{Q}^{q\times t}, F\in \mathbb{Q}^{m\times t}$ and

Find $X_{SCH}\in \mathbf{Q}_{SCH}$ such that $\|X_{SCH}\| = \min\limits_{X\in \mathbf{Q}_{SCH}} \|X\|$.

The solution $X_{CH}$ is also named the minimal norm centrohermitian least squares solution of (1.1), and $X_{SCH}$ is also named the minimal norm skew centrohermitian least squares solution of (1.1).

The rest of this article is arranged as follows. In Section 2, we provide some preliminary results. In Section 3, by using the real representations of quaternion matrices and relative properties, we convert the quaternion least squares problems with constrained variables into the corresponding real least squares problems with free variables, and then we obtain the solutions of Problems I and II. In Section 4, we first purpose two numerical algorithms for Problems I and II, respectively, and then we give two examples to verify our algorithms. Finally, in Section 5, we offer some brief comments.

2.   Preliminaries

A quaternion $q\in\mathbb{Q}$ can be written as

where $q_1, q_2, q_3, q_4\in \mathbb{R}$ and $\mathbf{i}^2 = \mathbf{j}^2 = \mathbf{k}^2 = \mathbf{ijk} = -1$. The conjugate of $q$ is defined as

Similarly, a quaternion matrix $Q \in \mathbb{Q}^{m\times n}$ can be written as

where $Q_1, Q_2, Q_3, Q_4\in \mathbb{R}^{m\times n}$, and its conjugate matrix is $\overline{Q}\equiv Q_{1}-Q_{2}\mathbf{i}-Q_{3}\mathbf{j}-Q_{4}\mathbf{k}.$ The real representation matrix of $Q$ can be defined as

The Frobenius norm of $Q$ is defined as

Obviously, $Q^\mathbf{R}$ has specific structure. Now, the $k$ -th row block of $Q^\mathbf{R}$ is denoted by $Q^\mathbf{R}_{r_{k}}$, and the $k$ -th column block of $Q^\mathbf{R}$ is denoted by $Q^\mathbf{R}_{c_{k}}$. $Q^\mathbf{R}$, $Q^\mathbf{R}_{r_{k}}$, $Q^\mathbf{R}_{c_{k}}$ have the properties as below.

Lemma 2.1 (^[26]). Let $A, B\in \mathbb{Q}^{m\times n}, C\in \mathbb{Q}^{n\times t}, l\in \mathbb{R}$. Then

${\rm (1)}$ $A = B\Leftrightarrow A^{\mathbf{R}} = B^{\mathbf{R}}\Leftrightarrow A^{\mathbf{R}}_{r_{k}} = B^{\mathbf{R}}_{r_{k}}\Leftrightarrow A^{\mathbf{R}}_{c_{k}} = B^{\mathbf{R}}_{c_{k}},$

${\rm (2)}$ $(A+B)^{\mathbf{R}}_{r_{k}} = A^{\mathbf{R}}_{r_{k}}+B^{\mathbf{R}}_{r_{k}}, (A+B)^{\mathbf{R}}_{c_{k}} = A^{\mathbf{R}}_{c_{k}}+B^{\mathbf{R}}_{c_{k}},$

${\rm (3)}$ $(lA)^{\mathbf{R}}_{r_{k}} = lA^{\mathbf{R}}_{r_{k}}, \quad (lA)^{\mathbf{R}}_{c_{k}} = lA^{\mathbf{R}}_{c_{k}},$

${\rm (4)}$ $(AC)^{\mathbf{R}}_{r_{k}} = A^{\mathbf{R}}_{r_{k}} C^{\mathbf{R}}, \quad (AC)^{\mathbf{R}}_{c_{k}} = A^{\mathbf{R}} C^{\mathbf{R}}_{c_{k}},$

${\rm (5)}$ $\|A\| = \|A^\mathbf{R}_{r_{k}}\| = \|A^\mathbf{R}_{c_{k}}\|,$

where $k = 1, 2, 3, 4.$

For simplicity, we use the first column block of $Q^\mathbf{R}$ to solve Problems I and II, and which is denoted as $Q^\mathbf{R}_c$, i.e.,

The following lemmas are useful for solving Problems I and II.

Lemma 2.2 (^[27]). Let $X\in \mathbb{Q}^{p\times q}$. Then $vec(X^\mathbf{R}) = \mathcal{F}vec(X^\mathbf{R}_c),$ where

and

Lemma 2.3. Let $X\in \mathbb{R}^{p\times q}$. Then $vec(X^\mathbf{R}_c) = \mathcal{M}\left(\begin{array}{c}vec(X_1)\\vec(X_2)\\vec(X_3)\\vec(X_4)\end{array}\right),$ where

and $\mathcal{I}_1 = (I_p, 0, \cdots, 0), \mathcal{I}_2 = (0, I_p, \cdots, 0), \cdots, \mathcal{I}_q = (0, 0, \cdots, I_p)\in\mathbb{R}^{p\times pq}.$

For a real matrix $X = (x_{ij})\in \mathbb{R}^{p\times q}$, if $x_{ij} = x_{(p-i+1)(q-j+1)}$, then $X$ is called centrosymmetric matrix. If $x_{ij} = -x_{(p-i+1)(q-j+1)}$, then $X$ is called anti centrosymmetric matrix. They can also be described equivalently as

Similarly, for a quaternion matrix $X = (x_{ij})\in \mathbb{Q}^{p\times q}$, if $x_{ij} = \overline{x}_{(p-i+1)(q-j+1)}$, then $X$ is called centrohermitian matrix. If $x_{ij} = -\overline{x}_{(p-i+1)(q-j+1)}$, then $X$ is called skew centrohermitian matrix. And it is not difficult to get the following conclusions:

Furthermore, we can get the following Lemma 2.4.

Lemma 2.4. Let $X = X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in \mathbb{Q}^{p\times q}$. Then

${\rm (1)}$ $X\in \mathbb{Q}_{CH}^{p\times q}\Longleftrightarrow X_1\in \mathbb{R}_{CS}^{p\times q}, X_2, X_3, X_4\in \mathbb{R}_{ACS}^{p\times q},$

${\rm (2)}$ $X\in \mathbb{Q}_{SCH}^{p\times q}\Longleftrightarrow X_1\in \mathbb{R}_{ACS}^{p\times q}, X_2, X_3, X_4\in \mathbb{R}_{CS}^{p\times q}.$

Proof. By the definition of centrohermitian matrix, we have

Thus (1) holds. Similarly, we can show that (2) holds.

Lemma 2.5. Suppose $X = (x_{ij})\in \mathbb{R}^{p\times q}$.

(1) If $p = 2m+1, q = 2n+1$, let

and $vec^{CS}_{oo}(X) = (\alpha_1, \alpha_2, \cdots, \alpha_q)^T,$ then

where

(2) If $p = 2m+1, q = 2n$, let

and $vec^{CS}_{oe}(X) = (\alpha_1, \alpha_2, \cdots, \alpha_q)^T,$ then

where

(3) If $p = 2m, q = 2n+1$, let

and $vec^{CS}_{eo}(X) = (\alpha_1, \alpha_2, \cdots, \alpha_q)^T,$ then

where

$(4)$ If $p = 2m, q = 2n$, let

and $vec_{ee}(X) = (\alpha_1, \alpha_2, \cdots, \alpha_q)^T,$ then

where

Lemma 2.6. Suppose $X = (x_{ij})\in \mathbb{R}^{p\times q}$.

(1) If $p = 2m+1, q = 2n+1$, let

and $vec^{ACS}_{oo}(X) = (\alpha_1, \alpha_2, \cdots, \alpha_q)^T,$ then

where

(2) If $p = 2m+1, q = 2n$, let

and $vec^{ACS}_{oe}(X) = (\alpha_1, \alpha_2, \cdots, \alpha_q)^T,$ then

where

(3) If $p = 2m, q = 2n+1$, let

and $vec^{ACS}_{eo}(X) = (\alpha_1, \alpha_2, \cdots, \alpha_q)^T,$ then

where

$(4)$ If $p = 2m, q = 2n$, let

and $vec^{ACS}_{ee}(X) = (\alpha_1, \alpha_2, \cdots, \alpha_q)^T,$ then

where

Lemma 2.7. Let $X = X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in \mathbb{Q}^{p\times q}$. Then

$(1)$ $X\in \mathbb{Q}^{p\times q}_{CH}\Longleftrightarrow \left(\begin{array}{c} vec(X_1) \\ vec(X_2) \\ vec(X_3)\\ vec(X_4)\\ \end{array} \right) = \mathcal{G}^{CH}_{\mu\nu} \left(\begin{array}{c} vec_{\mu\nu}^{CS}(X_1) \\ vec_{\mu\nu}^{ACS}(X_2) \\ vec_{\mu\nu}^{ACS}(X_3)\\ vec_{\mu\nu}^{ACS}(X_4)\\ \end{array} \right),$

$(2)$ $X\in \mathbb{Q}^{p\times q}_{SCH}\Longleftrightarrow \left(\begin{array}{c} vec(X_1) \\ vec(X_2) \\ vec(X_3)\\ vec(X_4)\\ \end{array} \right) = \mathcal{G}^{SCH}_{\mu\nu} \left(\begin{array}{c} vec_{\mu\nu}^{ACS}(X_1) \\ vec_{\mu\nu}^{CS}(X_2) \\ vec_{\mu\nu}^{CS}(X_3)\\ vec_{\mu\nu}^{CS}(X_4)\\ \end{array} \right),$

where

Here, $p, q$ are odd numbers, and then $\mu = \nu = o$. $p$ is odd number and $q$ is even number, and then $\mu = o, \nu = e$. $p$ is even number and $q$ is odd number, and then $\mu = e, \nu = o$. $p, q$ are even numbers, and then $\mu = \nu = e$.

Lemmas 2.3, 2.5 and 2.6 can be obtained by direct calculation. According to Lemmas 2.4–2.6, we can easily get Lemma 2.7, so we omit the details.

3.   The solutions of Problems I and II

In this section, we first convert the quaternion least squares problems with constrained variables into the corresponding real least squares problems with free variables by applying the real representations of quaternion matrices and the relative properties, and then obtain the solutions of Problems I and II.

Theorem 3.1. Suppose $A\in \mathbb{Q}^{m\times p}$, $B\in \mathbb{Q}^{q\times s}, C\in \mathbb{Q}^{m\times s}, D\in \mathbb{Q}^{m\times p}$, $E\in \mathbb{Q}^{q\times t}, F\in \mathbb{Q}^{m\times t}$ and $X = X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in \mathbb{Q}_{CH}^{p\times q}$, and denote $\mathcal{K} = \left(\!\! \begin{array}{c} (B^{\mathbf{R}}_c)^T\otimes A^{\mathbf{R}}\\ (E^{\mathbf{R}}_c)^T\otimes D^{\mathbf{R}}\\ \end{array} \!\!\right)$.Then the set $\mathbf{Q}_{CH}$ in Problem I can be represented as

where $y\in \mathbb{R}^r$ is an arbitrary vector. The unique solution $X_{CH} = X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in \mathbf{Q}_{CH}$ of Problem I satisfies

Here, if $p = 2m+1, q = 2n+1$, then $\mu = \nu = o$ and $r = 8mn+4m+4n+1$. If $p = 2m+1$, $q = 2n$, then $\mu = o, \nu = e$ and $r = 8mn+4n$. If $p = 2m, q = 2n+1$, then $\mu = e, \nu = o$ and $r = 8mn+4m$. If $p = 2m, q = 2n$, then $\mu = \nu = e$ and $r = 8mn$.

Proof. Because of

we get

Therefore, the set $\mathbf{Q}_{CH}$ of Problem I can also be represented as

According to Lemmas 2.1–2.3, we obtain

For $X = X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in \mathbb{Q}^{p\times q}_{CH}$, according to Lemma 2.7, we get

Consequently

For the real matrix equation

its least squares solutions can be represented as

where $y\in\mathbb{R}^r$ is an arbitrary vector. Then

Here, if $p = 2m+1, q = 2n+1$, then $\mu = \nu = o$ and $r = 8mn+4m+4n+1$. If $p = 2m+1$, $q = 2n$, then $\mu = o, \nu = e$ and $r = 8mn+4n$. If $p = 2m, q = 2n+1$, then $\mu = e, \nu = o$ and $r = 8mn+4m$. If $p = 2m, q = 2n$, then $\mu = \nu = e$ and $r = 8mn$.

Thus, we obtain the set $\mathbf{Q}_{CH}$ in (3.1), and the unique solution $X_{CH} = X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in \mathbf{Q}_{CH}$ of Problem I satisfies (3.2).

By Theorem 3.1, we can get the condition that there is a centrohermitian solution and the centrohermitian solution set of the quaternion matrix equation (1.1).

Corollary 3.2. Suppose $A\in \mathbb{Q}^{m\times p}$, $B\in \mathbb{Q}^{q\times s}, C\in \mathbb{Q}^{m\times s}, D\in \mathbb{Q}^{m\times p}$, $E\in \mathbb{Q}^{q\times t}, F\in \mathbb{Q}^{m\times t}$ and $X = X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in \mathbb{Q}_{CH}^{p\times q}$, and denote $\mathcal{K} = \left(\!\! \begin{array}{c} (B^{\mathbf{R}}_c)^T\otimes A^{\mathbf{R}}\\ (E^{\mathbf{R}}_c)^T\otimes D^{\mathbf{R}}\\ \end{array} \!\!\right)$.Then the quaternion matrix equation (1.1) has a centrohermitian solution $X\in \mathbb{Q}_{CH}^{p\times q}$ if and only if

When (1.1) has a centrohermitian solution, the centrohermitian solution set of (1.1) is

where $y\in\mathbb{R}^r$ is an arbitrary vector. In addition, if (1.1) has a centrohermitian solution, then (1.1) has a unique centrohermitian solution $X\in \mathbf{S}_{CH}$ if and only if

And the unique centrohermitian solution $X = X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in\mathbf{S}_{CH}$ satisfies

Here, if $p = 2m+1, q = 2n+1$, then $\mu = \nu = o$ and $r = 8mn+4m+4n+1$. If $p = 2m+1$, $q = 2n$, then $\mu = o, \nu = e$ and $r = 8mn+4n$. If $p = 2m, q = 2n+1$, then $\mu = e, \nu = o$ and $r = 8mn+4m$. If $p = 2m, q = 2n$, then $\mu = \nu = e$ and $r = 8mn$.

Proof. The quaternion matrix equation (1.1) has a centrohermitian solution $X$ if and only if $X$ satisfies $(AXB, DXE)\! = \!(C, F).$ Notice that

In addition,

So, (1.1) has a centrohermitian solution if and only if

Thus (3.3) holds. When (1.1) has a centrohermitian solution, the centrohermitian solution of (1.1) is the general solution of the following real matrix equation

So we obtain the formula in (3.4). In addition, (1.1) has a unique centrohermitian solution if and only if

And the unique centrohermitian solution $X = X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in\mathbf{S}_{CH}$ satisfies (3.5).

The method of solving Problem II is similar to that of Problem I, so we only give the conclusions and omit the details.

Theorem 3.3. Suppose $A\in \mathbb{Q}^{m\times p}$, $B\in \mathbb{Q}^{q\times s}, C\in \mathbb{Q}^{m\times s}, D\in \mathbb{Q}^{m\times p}$, $E\in \mathbb{Q}^{q\times t}, F\in \mathbb{Q}^{m\times t}$ and $X = X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in \mathbb{Q}_{SCH}^{p\times q}$, and denote $\mathcal{K} = \left(\!\! \begin{array}{c} (B^{\mathbf{R}}_c)^T\otimes A^{\mathbf{R}}\\ (E^{\mathbf{R}}_c)^T\otimes D^{\mathbf{R}}\\ \end{array} \!\!\right)$.Then the set $\mathbf{Q}_{SCH}$ in Problem II can be represented as

where $y\in \mathbb{R}^r$ is an arbitrary vector. The unique solution $X_{SCH}\! = \!X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in \mathbf{Q}_{SCH}$ of Problem II satisfies

Here, if $p = 2m+1, q = 2n+1$, then $\mu = \nu = o$ and $r = 8mn+4m+4n+3$. If $p = 2m+1$, $q = 2n$, then $\mu = o, \nu = e$ and $r = 8mn+4n$. If $p = 2m, q = 2n+1$, then $\mu = e, \nu = o$ and $r = 8mn+4m$. If $p = 2m, q = 2n$, then $\mu = \nu = e$ and $r = 8mn$.

Corollary 3.4. Suppose $A\in \mathbb{Q}^{m\times p}$, $B\in \mathbb{Q}^{q\times s}, C\in \mathbb{Q}^{m\times s}, D\in \mathbb{Q}^{m\times p}$, $E\in \mathbb{Q}^{q\times t}, F\in \mathbb{Q}^{m\times t}$ and $X = X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in \mathbb{Q}_{SCH}^{p\times q}$, and denote $\mathcal{K} = \left(\!\! \begin{array}{c} (B^{\mathbf{R}}_c)^T\otimes A^{\mathbf{R}}\\ (E^{\mathbf{R}}_c)^T\otimes D^{\mathbf{R}}\\ \end{array} \!\!\right)$.Then (1.1) has a skew centrohermitian solution $X\in \mathbb{Q}_{SCH}^{p\times q}$ if and only if

When (1.1) has a skew centrohermitian solution, the skew centrohermitian solution set of (1.1) is

where $y\in\mathbb{R}^r$ is arbitrary vector. In addition, if (1.1) has a skew centrohermitian solution, then (1.1) has a unique skew centrohermitian solution $X\in \mathbf{S}_{SCH}$ if and only if

And the unique skew centrohermitian solution $X = X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in\mathbf{S}_{SCH}$ satisfies

Here, if $p = 2m+1, q = 2n+1$, then $\mu = \nu = o$ and $r = 8mn+4m+4n+3$. If $p = 2m+1$, $q = 2n$, then $\mu = o, \nu = e$ and $r = 8mn+4n$. If $p = 2m, q = 2n+1$, then $\mu = e, \nu = o$ and $r = 8mn+4m$. If $p = 2m, q = 2n$, then $\mu = \nu = e$ and $r = 8mn$.

Remark 1. It is generally known that quaternion operations are more complex than real operations. In the present paper, we convert the quaternion least squares problems into the corresponding real least squares problems. The final results are expressed only by real matrices and real vectors, and thus they are portable and convenient.

Remark 2. In ^[23], the authors studied the matrix nearness problem of the quaternion matrix equation (1.1) by the complex representation matrices of quaternion matrices. In fact, if $X_0 = 0$ of Problem 2 in ^[23], then the solution of Problem 2 is also the solution of Problem I in this paper. We will compare the accuracy of the solution of Problem I computed by these two methods in Section 4.

4.   Numerical algorithms and examples

In this section, we first purpose two numerical algorithms for Problems I and II according to the results in Sections 3, and then give two examples to verify our purposed algorithms.

Algorithm 4.1. (For Problem I)

(1) Input $A_{1}, A_{2}, A_{3}, A_{4}\in \mathbb{R}^{m\times p}$, $B_{1}, B_{2}, B_{3}, B_{4}\in \mathbb{R}^{q\times s}$, $C_{1}, C_{2}, C_{3}, C_{4}\in \mathbb{R}^{m\times s}$, $D_{1}, D_{2}, D_{3}, D_{4}\in \mathbb{R}^{m\times p}$, $E_{1}, E_{2}, E_{3}, E_{4}\in \mathbb{R}^{q\times t}$, $F_{1}, F_{2}, F_{3}, F_{4}\in \mathbb{R}^{m\times t}$, and $\mathcal{F}, \mathcal{M}$, $G_{\mu\nu}^{CS}$, $G_{\mu\nu}^{ACS}$.

$(2)$ Form $A^{\mathbf{R}}$, $D^{\mathbf{R}}$, $B^{\mathbf{R}}_c$, $E^{\mathbf{R}}_c$, $C^{\mathbf{R}}_c$, $F^{\mathbf{R}}_c$. Then we calculate $\mathcal{G}_{\mu\nu}^{CH}$ and $\mathcal{K}$ according to Lemma 2.7 and Theorem 3.1, respectively.

$(3)$ Compute the unique solution $X_{CH}$ of Problem I on the base of (3.2).

Algorithm 4.2. (For Problem II)

$(1)$ Input $A_{1}, A_{2}, A_{3}, A_{4}\in \mathbb{R}^{m\times p}$, $B_{1}, B_{2}, B_{3}, B_{4}\in \mathbb{R}^{q\times s}$, $C_{1}, C_{2}, C_{3}, C_{4}\in \mathbb{R}^{m\times s}$, $D_{1}, D_{2}, D_{3}, D_{4}\in \mathbb{R}^{m\times p}$, $E_{1}, E_{2}, E_{3}, E_{4}\in \mathbb{R}^{q\times t}$, $F_{1}, F_{2}, F_{3}, F_{4}\in \mathbb{R}^{m\times t}$, and $\mathcal{F}, \mathcal{M}$, $G_{\mu\nu}^{CS}$, $G_{\mu\nu}^{ACS}$.

$(2)$ Form $A^{\mathbf{R}}$, $D^{\mathbf{R}}$, $B^{\mathbf{R}}_c$, $E^{\mathbf{R}}_c$, $C^{\mathbf{R}}_c$, $F^{\mathbf{R}}_c$. Then we calculate $\mathcal{G}_{\mu\nu}^{SCH}$ and $\mathcal{K}$ according to Lemma 2.7 and Theorem 3.3, respectively.

$(3)$ Compute the unique solution $X_{SCH}$ of Problem II on the base of (3.7).

Now, in order to verify the effectiveness of Algorithms 4.1–4.2, we give two examples. In the first example, we compute the errors between the actual solutions and the solutions obtained by purposed algorithms for Problems I and II. In the second example, we compare the error of the solution of Problem I computed by Algorithm 4.1 and the algorithm in ^[23].

Example 4.1. Let the quaternion matrix equation $(AXB, DXE) = (C, F)$, where

and

${\rm (1)}$ Let $X_{CH} = X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in\mathbb{Q}^{p\times q}$, in which $X_1$ is a real centrosymmetric matrix, and $X_2, X_3, X_4$ are real anti centrosymmetric matrices. Thus $X_{CH}\in \mathbb{Q}_{CH}^{p\times q}$. Let

Therefore (1.1) has a unique centrohermitian solution $X_{CH}$, and $X_{CH}$ is also the unique solution of Problem I. According to Algorithm 4.1, we compute $X_{CH}^*$. Let $m = k, s = t = 2k$. It is noticed that the centrosymmetric matrix and anti centrosymmetric matrix have different forms with the change of matrix order. The order of $X_{CH}$ can be divided into the following four cases:

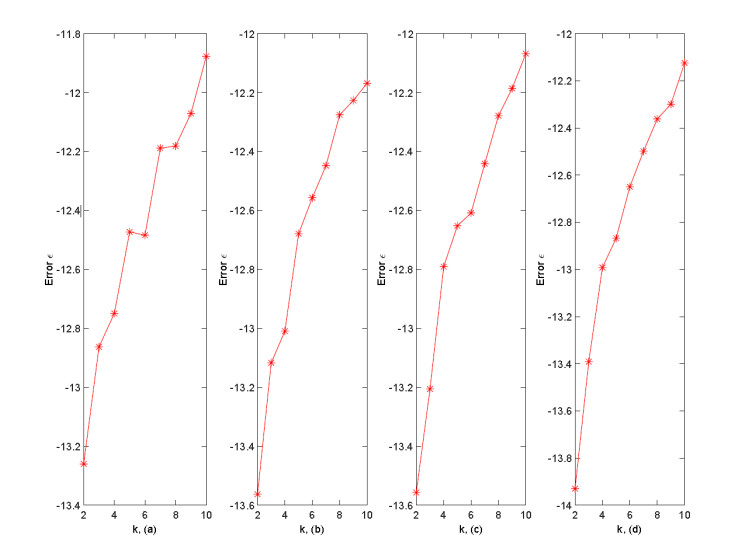
Let $k = 1:10$ and the error $\varepsilon = log10(\|X_{CH}^*-X_{CH}\|)$. For the above four cases, the errors are shown in Figure 1.

${\rm (2)}$ Let $X_{SCH} = X_1+X_2\mathbf{i}+X_3\mathbf{j}+X_4\mathbf{k}\in\mathbb{Q}^{p\times q}$, in which $X_1$ is a real anti centrosymmetric matrix, and $X_2, X_3, X_4$ are real centrosymmetric matrices. Thus, $X_{SCH}\in \mathbb{Q}_{SCH}^{p\times q}$. Let

Therefore (1.1) has a unique skew centrohermitian solution $X_{SCH}$, and $X_{SCH}$ is the unique solution of Problem II. According to Algorithm 4.2, we compute the solution $X_{SCH}^*$. Let $m = k, s = t = 2k$, and the values of $p, q$ has the following four cases:

Let $k = 1:10$ and the error $\varepsilon = log10(\|X_{SCH}^*-X_{SCH}\|)$. The errors are shown in Figure 2.

From Figure 1, we know that the errors $\varepsilon$ are no more than $-11.8$, which illustrate Algorithm 4.1 is effective. For the same number $k$, when $p = 2k+1, q = 2k+1$, the order of quaternion matrix is largest. So the corresponding norm is largest. When $p = 2k, q = 2k$, the order of quaternion matrix is smallest. Thus the corresponding norm is smallest. Figure 1 is consistent with theoretical analysis. Figure 2 also reflects Algorithm 4.2 is effective.

Example 4.2. Consider the solution of Problem I, and the orders of the matrices are the same as (1) in Example 4.1. We compute the errors by Algorithm 4.1 and the algorithm of ^[23]. Let $m = k, s = t = 2k, p = 2k, q = 2k+1$ and the error $\varepsilon = log10(\|X_{CH}^*-X_{CH}\|)$. The errors are shown in Figure 3.

From Figure 3, we can know that the errors obtained by Algorithm 4.1 are smaller than those obtained by the algorithm in ^[23], thus our purposed algorithms are more effective.

5.   Conclusions

In this article, we study the minimal norm centrohermitian least squares solution and skew centrohermitian least squares solution of quaternion matrix equation $(AXB, DXE) = (C, F)$ by using of the real representation matrices of quaternion matrices, and give the corresponding algorithms. This method is effective and convenient to analyze the problems of solution with special structures of quaternion matrix equation.

Acknowledgments

The authors would like to thank the handling editor and three anonymous referees for their valuable comments and suggestions. The study is supported by the Natural Science Foundation of Shandong Province of China (No. ZR2020MA053), and the Scientifc Research Foundation of Liaocheng University (No. 318011921).

Conflict of interest

The authors declare that there is no conflict of interest.