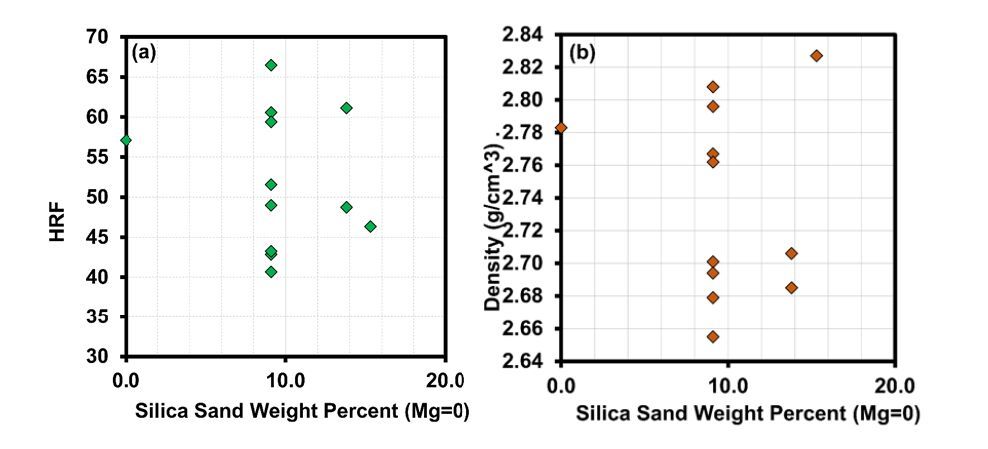
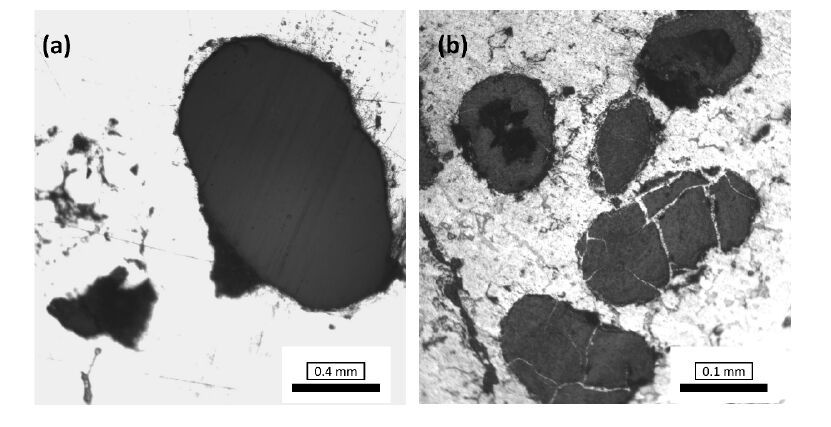
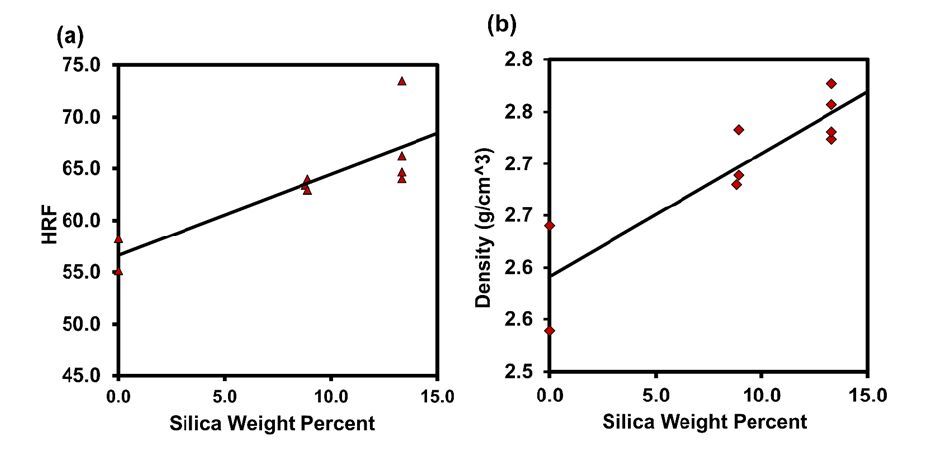
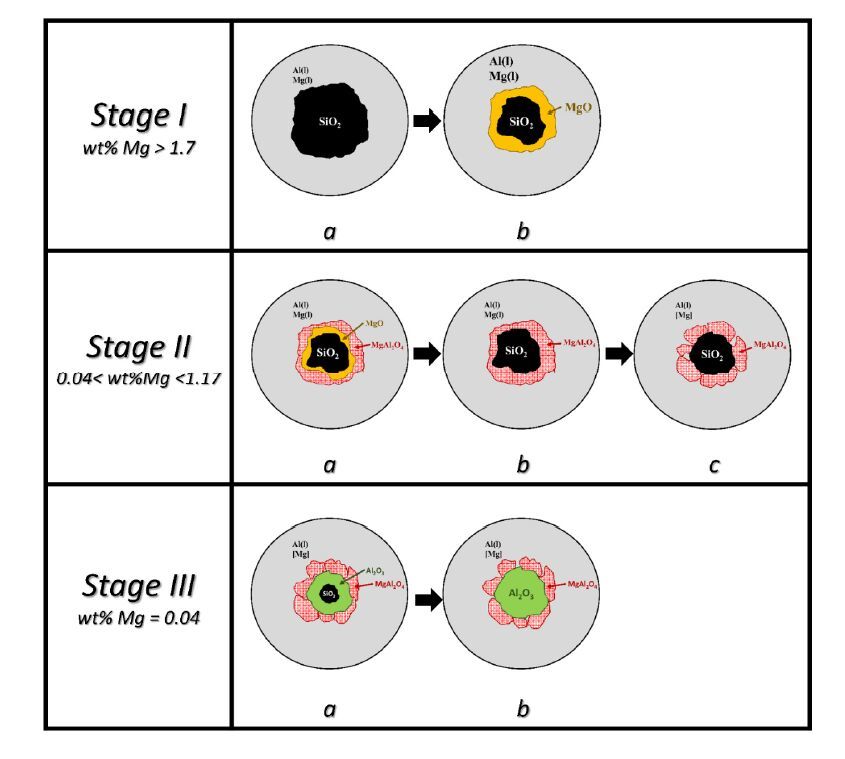
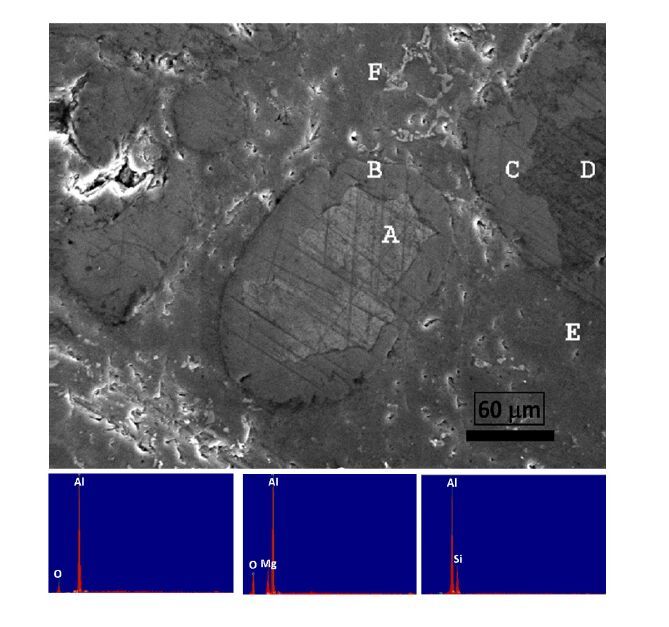
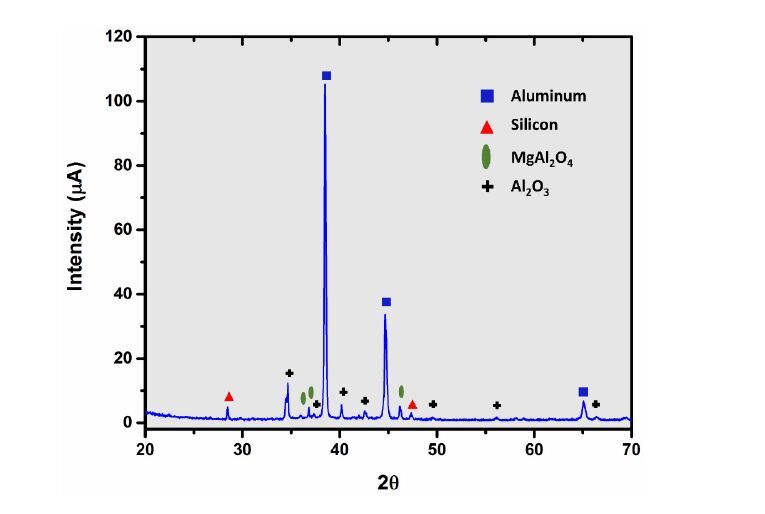
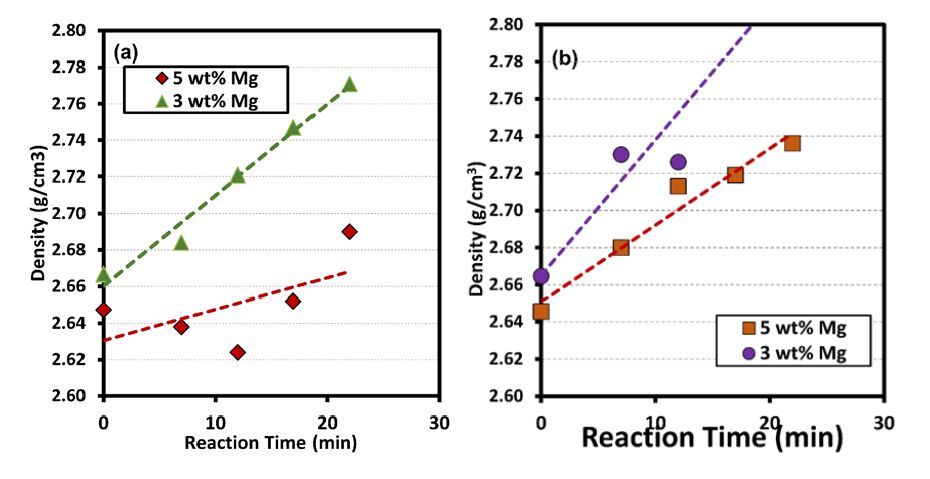
In order to gain a better understanding of the reactions and strengthening behavior in cast aluminum alloy/silica composites synthesized by stir mixing, experiments were conducted to incorporate low cost foundry silica sand into aluminum composites with the use of Mg as a wetting agent. SEM and XRD results show the conversion of SiO2 to MgAl2O4 and some Al2O3 with an accompanying increase in matrix Si content. A three-stage reaction mechanism proposed to account for these changes indicates that properties can be controlled by controlling the base Alloy/SiO2/Mg chemistry and reaction times. Experimental data on changes of composite density with increasing reaction time and SiO2 content support the three-stage reaction model. The change in mechanical properties with composition and time is also described.
1.
Introduction
In this paper, we are looking for solutions to the following Choquard equation
where N≥3, α∈(0,N), F(s):=∫s0f(t)dt, μ>0, 2<q≤¯q:=2+4N, a>0 is a given mass, and λ∈R appears as an unknown Lagrange multiplier. Iα:RN∖{0}↦R is the Riesz potential defined by
The Choquard equation
has been studied extensively for its profound physical backgrounds. In particular, when N=3, α=2, and F(s)=s22, Eq (1.2) turns into
which was introduced by Pekar [1] to describe the quantum theory of a polaron at rest and then used by Choquard [2] to study steady states of the one one-component plasma approximation to the Hartree-Fock theory. Also, Eq (1.3) reemerged as a model of self-gravitating matter [3,4], and in that context it is known as the Schrödinger-Newton equation.
The pioneering mathematical research dates back to Lieb [2], in which the author proved the existence and uniqueness for Eq (1.3) by variational methods. Later, Lions [5] obtained the existence of normalized solutions. In the homogeneous nonlinearity case of Eq (1.2) with F(s)=1p|s|p, Moroz and Van Schaftingen in [6] established the existence of ground states to Eq (1.2) with an optimal range N+αN<p<N+αN−2. Moreover, Ghimenti and Van Schaftingen in [7] obtained solutions which are odd with respect to a hyperplane of RN. The existence of saddle type nodal solution for the Choquard equation was proven in the study [8]. For a more general nonlinearity, Moroz and Van Schaftingen [9] proved Eq (1.2) has a ground solution when the nonlinearity satisfies Berestycki-Lions type condition and N≥3, see also [10] for the case N=2. For the Choquard equation with a local nonlinear perturbation
When N=3, 0<α<1, p=2, and 4≤q<6, Chen and Gao in [11] obtained the existence of solutions of Eq (1.4). For q=2∗ in Eq (1.4), Seok in [12] constructed a family of nontrivial solutions. In [13], the authors studied Eq (1.4) with a general local nonlinearity f(x,u) subcritical type instead of |u|q−2u.
From a physical point of view, it is interesting to find solutions with prescribed L2− norm, since there is a conservation of mass. Solutions of this type are often referred to as normalized solutions. In recent years, normalized solutions to nonlinear elliptic problems have attracted much attention from researchers. In [14], Jeanjean using a mountain pass structure for a stretched functional to consider the equation
He proved the existence of at least one normalized solution of Eq (1.5) in a purely L2− supercritical case. Later, Bartsch and Valeriola [15] obtained the existence of infinitely many normalized solutions by a new linking geometry for the stretched functional. For more general nonlinearity f(s) has L2− subcritical {growth, Shibata [16] obtained the existence and nonexistence of normalized ground state solution of} Eq (1.5) via minimizing method, Jeanjean and Lu in [17] showed the existence of nonradial normalized solutions for any N≥4. For more results on normalized solutions for Schrödinger equations by variational methods, we would like to refer [18,19,20,21,22,23]. Also, for the evolution equations with the singular potentials whose the steady state equations are the nonlinear elliptic equations, see [24].
Concerning the normalized solutions of Choquard equations, Li and Ye in [25] considered the existence of normalized solutions to the following equation
under a set of assumptions on f, where f takes the special case f(s)=C1|s|r−2s+C2|s|p−2s requires that N+2+αN<r≤p<N+αN−2. Later, Yuan et al. [26] generalized the results in [25] to more general f∈C(R,R). In [27], Bartsch et al. obtained the existence of infinitely many normalized solutions of (1.6). For F(u)=|u|pp, Ye in [28] studied the qualitative properties including existence and nonexistence of minimizers of the functional associated to the Eq (1.6). Yao et al. in [29] considered normalized solutions for the following problems
Under different assumptions on γ,μ,p, and q, they proved several existence, multiplicity, and nonexistence results. For more related topics, we refer the reader to [30,31,32,33].
Motivated by the above papers, it is natural to ask if the nonlinearity f(u) in Eq (1.1) satisfies general growth assumptions, and if the normalized ground states still exist. In the present paper, we attempt to study this kind of problem. In order to prove the existence of normalized ground state solutions to Eq (1.1), assuming that nonlinearity f∈C(R,R) satisfies the growth assumptions:
(f1) lims→0f(s)|s|αN=0,
(f2) lim|s|→+∞f(s)|s|2+αN=0,
(f3) f is odd and f does not change sign on (0,+∞),
(f4) lims→0F(s)|s|N+2+αN=+∞,
(f5) lim sups→0F(s)|s|N+2+αN<+∞.
To find solutions of Eq (1.1), we define functional Iμ(u):H1(RN)→R by
For a>0, set
Since f∈C(R,R) satisfies (f1) and (f2), using the Hardy-Littlewood-Sobolev inequality, we see that Iμ∈C1(H1(RN),R). Normalized solutions of Eq (1.1) can be obtained by looking for critical points of Iμ on the constraint S(a). It is standard that each critical point u∈S(a) of Iμ|S(a), corresponds a Lagrangian multiplier λ∈R such that (u,λ) solves Eq (1.1). We will be interested in ground state solutions, and following [34], we say that u∈S(a) is a normalized ground state solution to Eq (1.1) if (Iμ|S(a))′(u)=0 and
In particular, if u∈S(a) is a minimizer of the minimization problem
then u is a critical point of Iμ|S(a) as well as a normalized ground state to Eq (1.1).
Our main results dealing with problem (1.1) are the following:
Theorem 1.1. Assume that (f1)–(f3) hold. Let μ>0 and 2<q<¯q. Then, for any a>0, there exists a global minimizer ˜u with respect to m(a), which solves Eq (1.1) for some ˜λ<0. Moreover, ˜u is a ground state solution of Eq (1.1) which has constant sign, is radially symmetric with respect to some point in RN, and is decreasing.
Theorem 1.2. Assume that (f1)–(f3) hold and q=¯q. For any μ>0 small enough, there exist a a0=a0(μ)>0 and a∗∈[0,a0) such that:
(i) for any a∈(0,a∗), there is no global minimizer with respect to m(a).
(ii) for any a∈(a∗,a0), there exists a global minimizer u with respect to m(a), which solves Eq (1.1) for some λ<0. Moreover, u is a ground state solution of Eq (1.1) which has constant sign, is radially symmetric with respect to some point in RN, and is decreasing.
(iii) if (f4) holds, then a∗=0, and if (f5) holds, then a∗>0.
Remark 1. The value a0=a0(μ)>0 is explicit and is given in Lemma 4.1. In particular, a0>0 can be taken arbitrary large by taking μ>0 small enough.
The following result positively answers the existence of global minimizer with respect to m(a∗) for the sharp threshold a∗>0.
Theorem 1.3. Assume that (f1)–(f3) and
(f6) lim sups→0F(s)|s|N+2+αN=0 holds.
Let q=¯q. For a=a∗, there exists a global minimizer v with respect to m(a∗)=0, which solves Eq (1.1) for some λ<0. In particular, v is a ground state solution of Eq (1.1) which has constant sign and is radially symmetric with respect to some point in RN.
To the best of our knowledge, the main results in this paper are new. This is a complement of the results for Choquard equations about the existence of normalized solutions. Our main theorems can be viewed as an extension of some results in [25,29] to more general cases. In our setting, we only consider the situation when the constraint functional Iμ|S(a) is bounded from below and is coercive. As we will see, the existence of normalized states of Eq (1.1) are strongly effected by further assumptions on the exponent q. We are first devoted to prove the existence of ground state solutions of Eq (1.1) with 2<q<2+4N by application of the concentration-compactness principle [35]. In this case, m(a)<0 for any a>0 and the strict subadditivity inequality
holds, which permits us to exclude the dichotomy of the minimizing sequence. However, in the case of q=¯q:=2+4N, compare to [29], for a general f, the strict subadditivity inequality (1.8) does not hold, and m(a)<0 for all a>0 may not be satisfied. This prevents us from using the concentration-compactness principle in a standard way. In the proof, we adopt some ideas in [16,19] to recover the compactness of minimizing sequence with respect to m(a).
The paper is organized as follows. In Section 2, we introduce the variational framework and give some preliminary results. In Section 3, we discuss the case of 2<q<¯q and prove Theorem 1.1. In Section 4, we deal with the case of q=¯q and prove Theorems 1.2 and 1.3.
In this paper, we will use the following notations:
∙ H1(RN) is the usual Sobolev space endowed with the norm ‖u‖2=∫RN(|∇u|2+|u|2)dx.
∙ Ls(RN), for 1≤s<∞, denotes the Lebesgue space with the norm |u|ss=∫RN|u|sdx.
∙ For any r>0 and x∈RN, Br(x) denotes the ball of radius of r centered at the x.
∙ The letters C, C0,C′,C″,C1,C2⋅⋅⋅ are positive (possibly different) constants.
∙ on(1) denotes the vanishing quantities as n→∞.
2.
Preliminaries
In this section, we give some results which will be useful in forthcoming sections. First, let us recall the following Hardy-Littlewood-Sobolev inequality which will be frequently used throughout the paper.
Lemma 2.1. (See [[36], Theorem4.3]). Suppose α∈(0,N), and s,r>1 with 1r+1s=1+αN. Let f∈Ls(RN) and g∈Lr(RN). Then, there exists a constant C(N,α,s,r)>0 such that
In particular, if r=s=2NN+α, then
Let N≥1 and N+αN<p<2∗α:=N+αN−2. Then, we introduce the following Gagliardo-Nirenberg inequality of Hartree type ([28])
We also recall the following Gagliardo-Nirenberg inequality. For p∈(2,2∗) and u∈H1(RN),
Lemma 2.2. Assume that (f1)-(f2) hold. Let {un}⊂H1(RN) be a bounded sequence. If either limn→∞|un|2=0 or limn→∞|un|2(N+α+2)N+α=0 holds, then
Proof. By (f1) and (f2), for any ε>0, there exists Cε>0 such that
Then, using Lemma 2.1, for u∈H1(RN) we obtain
By Eq (2.2), we have
If limn→∞|un|2=0, by Eqs (2.3), (2.4), and the boundedness of {un}, we get
If limn→∞|un|2(N+α+2)N+α=0, by Eq (2.3), we have
Since {un} is bounded in H1(RN) and ε>0 is arbitrary, the conclusion holds. The proof is completed.
In our subsequent arguments, we will use the following nonlocal version of the Brezis-Lieb lemma.
Lemma 2.3. (See [[37], Lemma 2.2]). Assume α∈(0,N) and there exists a constant C>0 such that
Let {un}⊂H1(RN) be such that un⇀u weakly in H1(RN) and almost everywhere in RN as n→∞. Then,
Using a similar argument as the proof of ([9] Theorem 3), we have the following Pohoˇzaev of Eq (1.1).
Lemma 2.4. Assume that N≥3 and α∈(0,N). If f∈C(R,R) satisfies (f1) and (f2), and if (u,λ)∈H1(RN)×R solves problem (1.1), then
For each u∈S(a) and t>0, we define the scaling function
It is clear that ut∈S(a) and
3.
L2-subcritical perturbation
In this section, we deal with the case 2<q<¯q and prove Theorem 1.1.
Lemma 3.1. Assume that (f1) and (f2) hold. For any 2<q<¯q and a,μ>0, we have
Proof. By (f1) and (f2), for any ε>0, there exists Cε>0 such that
Using Lemma 2.1 and Eq (2.2), we obtain, for u∈H1(RN)
Then, for any u∈S(a), by Eqs (2.2) and (3.1), we get
Choosing ε=(4C4a2+αN)−12, it follows from Eq (3.2) that
for every u∈S(a). Since 2<q<¯q, we see that 0<qγq<2, and hence Iμ is coercive on S(a), which provides that m(a)>−∞.
On the other hand, for u∈S(a)
Noticing that 2<q<¯q, we have 2−N(q−2)2>0, and hence Iμ(ut)<0 for every u∈S(a) with t>0 small enough. Therefore, we have that m(a)<0 for any a>0.
Since m(a)<0 for any a>0, we can give the following strict sub-additivity.
Lemma 3.2. Let a1,a2>0 be such that a1+a2=a. Then,
Proof. For u∈S(a) and θ>1, we set ¯u(x)=u(θ−1Nx). Then, ¯u(x)∈S(θa). Let {un}⊂S(a) be a minimizing sequence for m(a). Since θ>1, we have
As a consequence,
with equality if and only if
But this is can not occur. Otherwise, by Eq (3.3), we find
By Lemma 3.1, Iμ is coercive on S(a) and we have {un} is bounded in H1(RN). It follows from θ>1 that
Combining Eq (2.2) we get limn→∞|un|qq=0. Then, by Lemma 3.1, we obtain
a contradiction. Thus, we have the strict inequality
Next, we show that m(a)<m(a1)+m(a2). We may assume that a1≥a2, by Eq (3.4) we have
The proof is completed.
Lemma 3.3. Assume that (f1) and (f2) hold and a,μ>0. Let {un}⊂H1(RN) be a sequence such that
Then, taking a subsequence if necessary, there exist ˜u∈S(a) and a family {yn}⊂RN such that un(⋅+yn)→˜u in H1(RN) as n→∞. Specifically, ˜u is a global minimizer.
Proof. Since {un}⊂H1(RN) satisfies Eq (3.5), it is easy to see that {un} is bounded in H1(RN). From the concentration-compactness lemma [35], there exists a subsequence of {un} (denoted in the same way) satisfying one of the three following possibilities:
vanishing: for all R>0
dichotomy: there exists a constant b∈(0,a), sequences {u(1)n}, {u(2)n} bounded in H1(RN) such that as n→∞
compactness: there exists a sequence {yn}⊂RN with the following property: for any ε>0, there exists R>0 such that
Claim 1. Vanishing does not occur.
Otherwise, by Lemma I.1 of [38], we get un→0 strongly in Lp(RN) for 2<p<2∗. Since 2<2(N+2+α)N+α<2∗, it follows from Lemma 2.2 that
Then, by Lemma 3.1 and Eq (3.5) we have
This contradiction proves Claim 1.
Claim 2. Dichotomy does not occur.
Otherwise, if dichotomy occurs, there exist b∈(0,a) and sequences {u(1)n}, {u(2)n} satisfying Eq (3.6). Furthermore, we may assume
Then, we have
and
By (f1), (f2), and Lemma 2.1, we obtain
By the definition of vn and Eq (3.6), we obtain that |vn|p→0 for 2≤p<2∗. It follows from the above inequality and the boundedness of {un} that
Using Eq (3.6) and Lemma 2.2, we get
Moreover, by the Young's inequality ([36] Theorem 4.2) and dist(suppu(1)n,suppu(2)n)→+∞, we infer that
Thus, Eqs (3.6) and (3.8)–(3.12) imply that
which contradicts to Lemma 3.2, and proves Claim 2.
Hence, the compactness holds, namely, there exists a subsequence {yn}⊂RN such that ˜un=un(x+yn)→˜u in L2(RN), ˜un⇀˜u in H1(RN) and ˜u∈S(a). It follows from interpolation inequality and Sobolev inequality that
Then, Lemma 2.2 implies
Hence,
Thus, we have Iμ(˜u)=m(a) and ‖˜un‖→‖˜u‖ as n→∞. Moreover, un(x+yn)→˜u in H1(RN).
Proof. [Proof of Theorem 1.1] By Lemmas 3.1 and 3.3, there exists a global minimizer ˜u for Iμ on S(a) with m(a)=Iμ(˜u)<0. Furthermore, ˜u is a ground state solution of Eq (1.1) for some ˜λ∈R. Then, by Lemma 2.4, we have
Then,
Since ∫RN(Iα∗F(˜u))F(˜u)dx>0, by Eq (3.13) we have ˜λ<0.
By (f3), without loss of generality, we may assume that f≥0 on (0,+∞). Since f is odd, then F is even and thus for every u∈H1(RN), Iμ(|u|)=Iμ(u). From this one easily obtain that the function |˜u| is also a ground state solution of Eq (1.1). By regularity properties of [9], |˜u| is continuous, we can apply the strong maximum principle get |˜u|>0 on RN and thus ˜u has constant sign.
Finally, we prove the symmetry of ˜u. Assume that H⊂RN is a closed half-space and that σH denotes the reflection with respect to ∂H. The polarization ˜uH(x):RN→R of ˜u is defined for x∈RN by
By the properties of polarization ([9] Lemma 5.4), we observe that
and then
Moreover, since F is nondecreasing on (0,+∞), we have F(˜uH)=F(˜u)H. Therefore, by Lemma 5.5 of [9], we get
with equality if and only if
On the other hand, since ˜u∈S(a) and |˜u|22=|˜uH|22, we have Iμ(˜uH)≥m(a). It follows from Eq (3.14) that Iμ(˜uH)=Iμ(˜u), and thus Eq (3.15) holds. If F(˜u)H=F(˜u), for every x∈H,
Since F is nondecreasing on (0,+∞), we get ˜u(σH(x))≤˜u(x). In particular, f(˜uH)=f(˜u) on RN, hence ˜uH=˜u. If F(˜u(σH))=F(˜u)H, we similarly get ˜uH=˜u(σH). Since the hyperplane H is arbitrary, in either case we conclude that the function ˜u is radially symmetric with respect to some point x0∈RN, and is radially decreasing.
4.
L2-critical perturbation
In this section, we consider the case q=¯q and prove Theorems 1.2 and 1.3. Set ¯aN:=¯q2C¯qN,¯q>0.
Lemma 4.1. Assume that (f1) and (f2) hold. For any μ>0, there exists a0(μ)=(¯aNμ−1)N2, such that for any a∈(0,a0), we have
Proof. For every u∈S(a), by Eqs (2.2) and (3.1), we get
Since a<a0, we have 12−μC¯qN,¯q¯qa2N>0. We choose ε>0 small such that C4ε2aα+2N=12(12−μC¯qN,¯q¯qa2N), it follows that
This implies m(a)>−∞.
In addition, for u∈S(a) we have
Noticing that a<a0, by Eq (2.2), we have 12|∇u|22−μ¯q|u|¯q¯q>0, and
Moreover, |ut|2(N+2+α)N+α2(N+2+α)N+α=t2NN+α|u|2(N+2+α)N+α2(N+2+α)N+α→0 as t→0. By Lemma 2.2, we have
Then, by Eq (4.1) we infer that
Thus, m(a)≤0. The proof is completed.
Next, we give some properties of m(a).
Lemma 4.2. It holds that
(i) Let a∈(0,a0). Then, we have for all b∈(0,a)
and if m(b) or m(a−b) is reached, then the inequality is strict.
(ii) Taking μ>0 small enough, there exists b0>0 such that 0<b0<a0(μ). Then, for any a∈(b0,a0), we have m(a)<0.
(iii) a∈(0,a0)↦m(a) is continuous.
Proof. (i) Fix b∈(0,a), we first show that
and that if m(b) is reached, the inequality is strict. By the definition of m(b), for any ε>0 sufficiently small, there exists a u∈S(b) such that
Now set ¯u(x)=u(θ−1Nx). Note that ¯u(x)∈S(θb). It follows from Eq (4.3) that
Since ε>0 is arbitrary, we have that m(θb)≤θm(b). If m(b) is reached, we can let ε=0 in Eq (4.3), and thus Eq (4.4) implies m(θb)<θm(b).
Then, by Eq (4.2) we have
with a strict inequality if m(b) is reached.
(ii) By (f3), there exists v∈H1(RN) such that
For any b>0, set vb(x)=v(b−1N|v|2N2x). Obviously vb∈S(b). Then, we have
Since g(b)→−∞ as b→+∞ and by choosing b0>0 large such that Iμ(vb0)<0, it follows that
Now, taking μ>0 small enough such that b0<a0(μ). For any a∈(b0,a0), by Lemma 4.1 and (i), we obtain
(iii) Let a∈(0,a0) be arbitrary and {an}⊂(0,a0) be such that an→a as n→∞. By the definition of m(an), for every n there exists un∈S(an) such that
Since m(an)≤0, by the proof of Lemma 4.1, the sequence {un} is bounded in H1(RN). Set vn=√aanun. It is clear that vn∈S(a). Then, we can write
where
By the boundedness of {un}, we have {|un|¯q¯q} is bounded. Thus, we have
Moreover,
Since an→a as n→∞, then {√aan} is bounded. It follows from (f1) and (f2) that
Thus, using Lemma 2.1 and the Sobolev imbedding inequality, we get
Thus,
Similarly,
Then, in view of Eq (4.9), we have
By Eqs (4.7), (4.8), and (4.10), we have
It follows from Eqs (4.5) and (4.6) that
On the other hand, for any ε>0, there exists u∈S(a) such that
Set ˜un(x)=√anau(x). Then, ˜un∈S(an). Similar to Eq (4.11), we have
It follows from Eq (4.12) that
Since ε>0 is arbitrary, we get
Thus, we infer that m(an)→m(a) as n→∞.
Now, for any fixed μ>0 small enough, we define
By Lemma 4.1, a∗∈[0,a0(μ)) is well defined and satisfying
Lemma 4.3. Assume that (f1)–(f3) hold. For any a∈(0,a0), if (f4) holds, then a∗=0; if (f5) holds, then a∗>0.
Proof. (i) Let a∈(0,a0), we choose u∈S(a)∩C∞0(RN) and set
By the assumption (f4), there exists δ>0 such that
Since |ut|∞≤δ for sufficiently small t>0, we have
Thus, m(a)≤Iμ(ut)<0 for any a∈(0,a0). By the definition of a∗, we get a∗=0.
(ii) By (f2) and (f5), there exists C′>0 such that
For u∈S(a), by Eqs (2.1) and (4.14), we get
It follows from Eq (2.2) that
Taking a>0 small enough such that C″2a2+αN+μ¯qC¯qN,¯qa2N≤12, we get Iμ(u)≥0. This implies m(a)≥0 for a small a>0. From Lemma 4.1 that m(a)=0 for a small a>0. Hence, we have a∗>0.
Lemma 4.4. Assume that (f1)–(f3) hold. Let μ>0 and a∈(0,a0). Let {un}⊂S(a) be a minimizing sequence for m(a). Then, one of the following holds:
(i).
(ii). Taking a subsequence if necessary, there exist u∈S(a) and a family {yn}⊂RN such that un(⋅+yn)→u in H1(RN) as n→∞. Specifically, u is a global minimizer.
Proof. Suppose that {un}⊂S(a) is a minimizing sequence which does not satisfy (i). Then, we have
Taking a subsequence if necessary, we may assume there exists a family {yn}⊂RN such that
Let us consider un(⋅+yn). Since {un}⊂S(a) is a minimizing sequence of m(a), by the proof of Lemma 4.1, {un} is bounded in H1(RN), Hence, {un(⋅+yn)} is bounded in H1(RN). Then, there exists u∈H1(RN) such that un(⋅+yn)⇀u in H1(RN), un(⋅+yn)→u in Lploc(RN) for 2≤p<2∗, and un(⋅+yn)→u almost everywhere in RN. It follows that
Then, by un(⋅+yn)→u in L2loc(RN), we obtain that u≠0 and |u|22>0. Set vn(x)=un(x+yn)−u. Then, vn⇀0 in H1(RN), and we have
Moreover, by Lemma 2.3, we have
Since Iμ(un)=Iμ(un(⋅+yn))=Iμ(vn+u), by Eqs (4.16)–(4.18), we obtain
Claim. limn→∞|vn|22=0.
Denote b=|u|22>0. By Eq (4.15), we see that b≤a, and if we prove that b=a, then the claim holds. We suppose by contradiction that b<a. Since vn∈S(|vn|22), then
It follows from Iμ(un)→m(a) and Eq (4.19) that
By Lemma 4.2 (iii) and Eq (4.15), we obtain
Note that b=|u|22, we have u∈S(b) and Iμ(u)≥m(b). If Iμ(u)>m(b), then Lemma 4.2 (i) and Eq (4.20) imply
which is impossible. Hence, Iμ(u)=m(b), that is u is a minimizer of m(b) on S(b). It follows from Lemma 4.2 (i) and Eq (4.20) that
which is a contradiction. Thus, the claim holds and Eq (4.15) implies |u|22=a.
Finally, we show that limn→∞|∇vn|22=0. Since u∈S(a), we have Iμ(u)≥m(a). It follows from Eq (4.19) that
this implies
On the other hand, by Lemma 2.2, Eq (2.2), and |vn|22→0, we infer that
In view of Eq (4.21), we get
Then, we have limn→∞|∇vn|22=0. It follows from |vn|22→0 that vn→0 in H1(RN). Hence, un(⋅+yn)→u in H1(RN) as n→∞.
Proof. [Proof of Theorem 1.2] (i) For the case 0<a<a∗, we assume by contradiction that there exists a global minimizer with respect to m(a). By Eq (4.13), we know m(a)=0. By Lemma 4.2 (i) with the strict inequality, we infer that
which is a contradiction.
(ii) For the case a∗<a<a0. By Eq (4.13), we have m(a)<0. Let {un}⊂S(a) satisfying limn→∞Iμ(un)=m(a). It is easy to see that {un} is bounded in H1(RN). Then, one of the two alternatives in Lemma 4.4 occurs. Let assume that (i) of Lemma 4.4 take place. The Lemma 1.1 of [38] implies un→0 in Lt(RN) for 2<t<2∗. Moreover, since 2<2(N+2+α)N+2<2∗, by Lemma 2.2 we have
From this, we infer that
which contradicts to m(a)<0. Thus, Lemma 4.4 (ii) holds, namely, there exist u∈S(a) and a family {yn}⊂RN such that un(⋅+yn)→u in H1(RN) as n→∞, and u is a global minimizer to m(a). Moreover, u is a ground state solution to Eq (1.1) for some λ∈R. By Lemma 2.4, Eq (3.13), and m(a)<0, we have λ<0. Similar as the proof of Theorem 1.1, u has constant sign and is radially symmetric about a point in RN.
Proof. [Proof of Theorem 1.4] By (f6) and (iii) of Theorem 1.2, we have a∗>0. Let an=a∗+ln for any n∈N, where 0<l<a0−a∗. By Eq (4.13) and Theorem 1.2 (ii), for every n, there exists a global minimizer un∈S(an) such that
Then, {un} is bounded in H1(RN). Since an→a∗, we have limn→∞|un|22=a∗. Set vn=√a∗|un|2⋅un. Clearly, vn∈S(a∗) and √a∗|un|2→1 as n→∞. By the similar proof of Lemma 4.2 (iii), we obtain
Thus, {vn} is a minimizing sequence with respect to m(a∗).
Next, we prove that {vn} is non-vanishing, that is
Otherwise, δ=0. By the definition of vn and √a∗|un|2→1, we get
By (ii) of Theorem 1.2, un satisfies Eq (1.1) and we may assume that un is radially symmetric with respect to the origin and decreasing for any n∈N. Using the elliptic regularity theory, we see that {un} is bounded in C1(B(0,1)). Thus, by Eq (4.23) we get
It follows from (f6) that for any ε>0 and sufficiently large n,
Choosing ε>0 small enough such that Cε2(a∗+l)2+αN≤12−μ¯qC¯qN,¯q(a∗+l)2N, then by Eq (2.2) we have
which contradicts Eq (4.22), and hence δ>0.
Then Lemma 4.4 (ii) holds, that is, up to a subsequence, there exist v∈S(a∗) and a family {yn}⊂RN such that vn(⋅+yn)→v in H1(RN) as n→∞. Then, v is a global minimizer to m(a∗). Moreover, v is a ground state solution to (1.1) for some λ∈R. By Lemma 2.4 and Eq (3.13), we have
which implies λ<0. By the same arguments of Theorem 1.1, we obtain the symmetric of v.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
The authors would like to express sincere thanks to the anonymous referees for their carefully reading this paper and valuable useful comments. This work was supported by Qinglan Project of Jiangsu Province (2022).
Conflict of interest
The authors declare there is no conflicts of interest.













 DownLoad:
DownLoad: