Plastics waste management: A review of pyrolysis technology

1.   Introduction

Fractional calculus (FC) is a discipline of mathematics concerned with the study of derivatives and integrals of non-integer orders. It was invented in September 1695 by L'Hospital. In a letter to L'-Hospital ^[1], who discussed the differentiation of product functions of order $\dfrac{1}{2}$, which laid the groundwork for FC ^[2,3,4]. It provides a great tool for characterizing memory and inherited qualities of different materials and procedures ^[4,5,6]. FC has grown in interest in recent decades as a result of the intensive development of fractional calculus theory and its applications in diverse sectors of science and engineering due to its high precision and applicability, for example, fractional control theory, image processing, signal processing, bio-engineering, groundwater problems, heat conduction, and behavior of viscoelastic and visco-plastic materials, see ^[7,8,9]. In addition, the electrical RLC circuit's performance has been determined using the fractional model ^[10].

In the last few decades, numerical and analytical solutions of fractional partial differential equations (FPDEs) have drawn a lot of attention among researchers ^{[11,12,13,14,15]}. The qualitative behavior of these mathematical models is significantly influenced by the fractional derivatives that are employed in FPDEs. This has numerous applications in the fields of solid-state physics, plasma physics, mathematical biology, electrochemistry, diffusion processes, turbulent flow, and materials science ^[16,17,18].

However, solving PDEs is not an easy task. A lot of mathematicians have put their effort into formulating analytical and numerical methods to solve fractional partial differential equations. The widely recognized methods for the solution of (FPDEs) are the Adomian decomposition method ^[19], homotopy analysis method ^[20,21], q-homotopy analysis transform method ^[22], homotopy perturbation method ^[23], variation iteration method ^[24], differential transform method ^[25], projected differential transform method ^[26], meshless method ^[27], backlund transformation method ^[28], Haar wavelet method ^[29], G'/G expansion method ^[30], residual power series method ^[31], Adam Bashforth's moulton technique ^[32], operational matrix method ^[33].

The nonlinear partial differential Navier-Stokes (N-S) equation, which expresses viscous fluid motion, was first developed by Claude Louis and Gabriel Stokes in 1822 ^[34]. This equation describes the conservation of mass and conservation of momentum for Newtonian and is referred to as the Newton's second law for fluids. The N-S equation has wide applications in engineering science, for example, examining liquid flow, studying wind current around wings, climate estimation, and blood flow ^[35,36]. Furthermore, along with Maxwell's equations the (N-S) equation can be applied to study and model magnetohydrodynamics, plasma physics, geophysics, etc. Also, fluid-solid interaction problems have been modeled and investigated by the N-S equation ^[37].

The multi-dimensional Navier-Stokes equation (MDNSE) stands as a fundamental cornerstone in fluid dynamics, providing a comprehensive mathematical framework to describe the motion of fluid substances in multiple dimensions. Derived from the Navier-Stokes equation, which govern the conservation of momentum for incompressible fluids, the MDNSE extends these principles to encompass the complexities of fluid flow in more than one spatial dimension. The equation accounts for the conservation of mass and the interplay of viscous and inertial forces, offering a powerful tool to model and analyze fluid behavior in diverse physical scenarios. The application of the multi-dimensional Navier-Stokes equation spans a wide range of scientific and engineering disciplines, playing a crucial role in understanding fluid dynamics across various contexts. In the field of aerospace engineering, MDNSE is employed to simulate the airflow around aircraft, aiding in the design and optimization of aerodynamic profiles. In marine engineering, it finds application in predicting the behavior of water currents around ships and offshore structures. Additionally, MDNSE is instrumental in weather modeling, allowing meteorologists to simulate and analyze atmospheric conditions in multiple dimensions for more accurate weather predictions. In the realm of biomedical engineering, it contributes to the study of blood flow in arteries and the behavior of biological fluids. Overall, the multi-dimensional Navier-Stokes equation serves as a versatile and indispensable tool for gaining insights into the intricate dynamics of fluid motion in diverse scientific and engineering.

In literature, many researchers have used numerous techniques to analyze the N-S equation. First of all, the authors of ^[38] solved the fractional-order N-S equation by using the Laplace transform, Fourier sine transform, and Hankel transform. The authors of ^[39,40,41] investigated the time-fractional N-S equation by using the homotopy perturbation method. Biraider ^[42] used the Adomian decomposition method to find a numerical solution. Recently, many researchers have focused on examining the multi-dimensional time-fractional N-S equation, by combining a variety of techniques with different transforms, see ^{[43,44,45,46]}.

Motivated by the mentioned work, in the present article the new iterative transform method (NITM) and homotopy perturbation transform method (HPTM) combined with natural transform are implemented to analyze the solution of the time-fractional multi-dimensional Navier-Stokes equation in the sense of Caputo-Fabrizio operator. The article is structured in the following way: In Section 2, some basic definitions and properties are explained. In Section 3, the interpretation of the NITM is explained for the solution of fractional PDEs. In Section 4, the above-mentioned method's convergence analysis is also presented. In Section 5, the outcome of the suggested method is illustrated by examples, and validated graphically. In Section 6, the HPTM is explicated. In Section 7, similar examples are presented to elucidate the HPTM.

2.   Basic concepts

Definition 1 (^[47]). The Caputo fractional derivative of $f(\ell)$ is defined as

where, $m\in \mathbb{Z}^{+}, \; \theta\in \mathbb{R}^{+}.$

Definition 2 (^[48]). The Caputo-Fabrizio fractional derivative of $f(\ell)$ is defined as

where $\theta\in [0, 1]$, and $\mathcal{B}(\theta)$ is a normalization function and satisfies the condition $\mathcal{B}(0) = \mathcal{B}(1) = 1$.

Definition 3 (^[49]). The fractional integral of function $f(\ell)$ of order $\theta$, is defined as

From Eq (2.3), the following results hold:

which gives,

Thus, Losada and Nieto ^[49] redefined the Caputo-Fabrizio fractional derivative as

Definition 4 (^[50]). The natural transform of $\mho(\ell)$ is given by

For $\ell\in (0, \infty)$, the natural transform of $\mho(\ell)$ is given by

where $\mathcal{H}$ is the Heaviside function.

The inverse of natural transform of $\mathcal{U}(s, v)$ is defined as

Definition 5 (^[51]). The natural transform of the fractional Caputo differential operator $^{C}_{0}D_{\ell}^{\theta} \mho(\ell)$ is ${\; \; \; \; \; }$defined as

Definition 6 (^[52]). The natural transform of the fractional Caputo-Fabrizio differential operator $^{\; CF}_{\; \; 0}D_{\ell}^{\theta} \mho(\ell)$ is defined as

3.   The procedure of NITM

This section considers, NITM with the CF fractional derivative operator in order to evaluate the multi-dimensional (N-S) problem. This iterative method is a combination of the new iterative method introduced in ^[53] and the natural transform ^[50].

Consider the fractional PDE of the form

with respect to the initial condition

$^{CF}_{\; \; \; \; 0}\mathcal{D}_{\ell}^{\theta}$ is the Caputo-Fabrizio fractional differential operator of order $\theta, \; \mathcal{R}$ and $\mathcal{N}$ are linear and non-linear terms, and $\mathcal{P}$ is the source term.

By employing the natural transform on both sides of Eq (3.1), we get

By using the inverse natural transform, Eq (3.4) can reduced to the form

The nonlinear operator $\mathcal{N}$ as in ^[53], can be decomposed as

Now, define an $\it{m}$th-order approximate series

Consider the solution of Eq (3.1) in a series form as

By substituting Eqs (3.6) and (3.7) into Eq (3.5), we get

From Eq (3.9), the following iterations are obtained.

4.   Convergence analysis

In this section, we demonstrate the uniqueness and convergence of the ${NITM}_{CF}$.

Theorem 1. The solution derived with the aid of the ${NITM}_{CF}$ of Eq (3.1) is unique whenever $0 < (\wp_1, \wp_2)[1-\theta+\theta\ell] < 1$.

Proof. Let $\mathrm{X} = (C[J], \parallel.\parallel)$ be the Banach space for all continuous functions over the interval $J = [0, T]$, with the norm $\parallel\phi (\ell) = max_{\ell\in J}|\phi(\ell)|$.

Define the mapping $\mathcal{F}:\mathrm{X}\rightarrow \mathrm{X}$, where

Now, assume that $\mathcal{R}(\mho)$ and $\mathcal{N}(\mho)$ satisfy the Lipschitz conditions with Lipschitz constants $\wp_1, \wp_2$ and $|\mathcal{R}(\mho)-\mathcal{R}(\bar{\mho})| < \wp_1|\mho-\bar{\mho}|$, $|\mathcal{N}(\mho)-\mathcal{N}(\bar{\mho})| < \wp_2|\mho-\bar{\mho}|$, where $\mho = \mho(\varphi, \varrho, \ell)$ and $\bar{\mho} = \mho(\varphi, \varrho, \ell)$ are the values of two distinct functions.

$\mathcal{F}$ is contraction as $0 < (\wp_1+\wp_2)[1-\theta+\theta\ell] < 1$. Thus, the result of (3.1) is unique with the aid of the Banach fixed-point theorem.

Theorem 2. The solution derived from Eq (3.1) using the ${NITM}_{CF}$ converges if $0 < \mho < 1$ and $\parallel \mho_{i}\parallel < \infty$, where $\mho = (\wp_1+\wp_2)[1-\theta+\theta\ell]$.

Proof. Let $\mho_{n} = \sum_{r = 0}^{n}\mho_{r}(\varphi, \varrho, \ell)$ be a partial sum of series. To prove that $\{\mho_n\}$ is a Cauchy sequence in the Banach space $\mathrm{X}$, we consider

If $m = n+1$, then

where $\wp = (\wp_1+\wp_2)[1-\theta+\theta\ell]$. In a similar way

We see that, $1-\wp^{m-n} < 1$, as $0 < \wp < 1$. Thus,

Since $\parallel \mho_{1}\parallel < \infty$, $\parallel\mho_{m}-\mho_{n}\parallel\rightarrow 0$ as $n\rightarrow \infty$. Hence, $\mho_m$ is a Cauchy sequence in $\mathrm{X}$. So, the series $\mho_m$ is convergent.

5.   Numerical examples

In this section, we demonstrate the effectiveness of the NITM with the natural transformation for the Caputo-Fabrizio fractional derivative to solve the two-dimensional fractional N-S equation.

5.1.   Example 1

Consider the two-dimensional fractional N-S equation

with initial conditions

From Eqs (5.1) and (5.2), we set the following

Using the iteration process outlined in Section 3, we have

In a general way,

With the addition of all $\mu$ and $\nu$,

The exact solution of Eq (5.1) at $\theta = 1$ and $q = 0$ is given by

5.2.   Example 2

Consider the two-dimensional fractional N-S equation

with the initial conditions

From Eqs (5.9) and (5.10), we set the following:

Using the iteration process outlined in Section 3, we have

In a general way,

With the addition of all $\mu$ and $\nu$,

The exact solution of Eq (5.9) at $\theta = 1$ and $q = 0$ is given by

6.   The procedure of HPTM

Consider the following non-linear fractional PDEs

subject to the initial condition

$^{CF}_{\; \; 0}\mathcal{D}_{\ell}^{\theta}$ is the Caputo-Fabrizio fractional differential operator of order $\theta, \mathcal{R}$ and $\mathcal{N}$ are linear and non-linear terms, and $\mathcal{P}$ is the source term.

By using the natural transform on both sides of Eq (6.1), we get

where

By applying the inverse natural transform, Eq (6.4) is reduced to the form

where $\varpi(\mho(\varphi, \varrho, \ell))$ represents the term arising from the source term. Now, applying the HPTM to find the solution of Eq (6.5), we get

and the non-linear tern can be decomposed as

Cnsider some He's polynomials ^[54], given as

By substituting Eqs (6.6) and (6.7) into Eq (6.5), we get

Comparing the coefficients of like powers of $z$, the following approximations are obtained:

6.1.   Example 3

Consider the two-dimensional fractional N-S equation

with initial conditions

Applying the natural transform and inversion in Eq (6.13), we obtain

By implementing HPTM in Eq (6.15), we get

where $\mathcal{H}_r(\varphi, \varrho) = \mu \dfrac{\partial{\mu}}{\partial{\varphi}}+\nu \dfrac{\partial{\mu}}{\partial{\varrho}}$ and $\mathcal{I}_r(\varphi, \varrho) = \mu \dfrac{\partial{\nu}}{\partial{\varphi}}+\nu \dfrac{\partial{\nu}}{\partial{\varrho}}$, represent the nonlinear term.

From Eq (6.16), comparing the powers of $z$, we get

where $\mathcal{H}_{0}(\varphi, \varrho) = \mu_{0}\dfrac{\partial{\mu_{0}}}{\partial{\varphi}}+\nu_{0} \dfrac{\partial{\mu_{0}}}{\partial{\varrho}}$ and $\mathcal{I}_{0}(\varphi, \varrho) = \mu_{0} \dfrac{\partial{\nu_{0}}}{\partial{\varphi}}+\nu_{0}\dfrac{\partial{\nu_{0}}}{\partial{\varrho}}$.

where $\mathcal{H}_{1}(\varphi, \varrho) = \left(\mu_{0}\dfrac{\partial{\mu_{1}}}{\partial{\varphi}}+\mu_{1}\dfrac{\partial{\mu_{0}}}{\partial{\varphi}}\right) +\left(\nu_{0} \dfrac{\partial{\mu_{1}}}{\partial{\varrho}}+\nu_{1} \dfrac{\partial{\mu_{0}}}{\partial{\varrho}}\right),$

and $\mathcal{I}_{1}(\varphi, \varrho) = \left(\mu_{0} \dfrac{\partial{\nu_{1}}}{\partial{\varphi}}+\mu_{1} \dfrac{\partial{\nu_{0}}}{\partial{\varphi}}\right)+\left(\nu_{0}\dfrac{\partial{\nu_{1}}}{\partial{\varrho}}+\nu_{1}\dfrac{\partial{\nu_{0}}}{\partial{\varrho}}\right)$.

where $\mathcal{H}_{2}(\varphi, \varrho) = \left(\mu_{0}\dfrac{\partial{\mu_{2}}}{\partial{\varphi}}+\mu_{1}\dfrac{\partial{\mu_{1}}}{\partial{\varphi}}+\mu_{2}\dfrac{\partial{\mu_{0}}}{\partial{\varphi}}\right) +\left(\nu_{0} \dfrac{\partial{\mu_{2}}}{\partial{\varrho}}+\nu_{1} \dfrac{\partial{\mu_{1}}}{\partial{\varrho}}+\nu_{2} \dfrac{\partial{\mu_{0}}}{\partial{\varrho}}\right),$

and $\mathcal{I}_{2}(\varphi, \varrho) = \left(\mu_{0}\dfrac{\partial{\nu_{2}}}{\partial{\varphi}}+\mu_{1}\dfrac{\partial{\nu_{1}}}{\partial{\varphi}}+\mu_{2}\dfrac{\partial{\nu_{0}}}{\partial{\varphi}}\right) +\left(\nu_{0} \dfrac{\partial{\nu_{2}}}{\partial{\varrho}}+\nu_{1} \dfrac{\partial{\nu_{1}}}{\partial{\varrho}}+\nu_{2} \dfrac{\partial{\nu_{0}}}{\partial{\varrho}}\right)$.

    $\vdots$

In a general way,

With the addition of all $\mu$ and $\nu$,

The exact solution of Eq (6.13) at $\theta = 1$ and $q = 0$ is given by

6.2.   Example 4

Consider the two-dimensional fractional order N-S equation

with initial conditions

Applying the natural transform and inversion in Eq (6.22), we obtain

By implementing HPTM in Eq (6.24), we get

where $\mathcal{H}_r(\varphi, \varrho) = \mu \dfrac{\partial{\mu}}{\partial{\varphi}}+\nu \dfrac{\partial{\mu}}{\partial{\varrho}}$ and $\mathcal{I}_r(\varphi, \varrho) = \mu \dfrac{\partial{\nu}}{\partial{\varphi}}+\nu \dfrac{\partial{\nu}}{\partial{\varrho}}$ represent the nonlinear terms.

From Eq (6.25), comparing the powers of $z$, we get

where $\mathcal{H}_{0}(\varphi, \varrho) = \mu_{0}\dfrac{\partial{\mu_{0}}}{\partial{\varphi}}+\nu_{0} \dfrac{\partial{\mu_{0}}}{\partial{\varrho}}$ and $\mathcal{I}_{0}(\varphi, \varrho) = \mu_{0} \dfrac{\partial{\nu_{0}}}{\partial{\varphi}}+\nu_{0}\dfrac{\partial{\nu_{0}}}{\partial{\varrho}}$.

where $\mathcal{H}_{1}(\varphi, \varrho) = \left(\mu_{0}\dfrac{\partial{\mu_{1}}}{\partial{\varphi}}+\mu_{1}\dfrac{\partial{\mu_{0}}}{\partial{\varphi}}\right) +\left(\nu_{0} \dfrac{\partial{\mu_{1}}}{\partial{\varrho}}+\nu_{1} \dfrac{\partial{\mu_{0}}}{\partial{\varrho}}\right),$

and $\mathcal{I}_{1}(\varphi, \varrho) = \left(\mu_{0} \dfrac{\partial{\nu_{1}}}{\partial{\varphi}}+\mu_{1} \dfrac{\partial{\nu_{0}}}{\partial{\varphi}}\right)+\left(\nu_{0}\dfrac{\partial{\nu_{1}}}{\partial{\varrho}}+\nu_{1}\dfrac{\partial{\nu_{0}}}{\partial{\varrho}}\right)$.

where $\mathcal{H}_{2}(\varphi, \varrho) = \left(\mu_{0}\dfrac{\partial{\mu_{2}}}{\partial{\varphi}}+\mu_{1}\dfrac{\partial{\mu_{1}}}{\partial{\varphi}}+\mu_{2}\dfrac{\partial{\mu_{0}}}{\partial{\varphi}}\right) +\left(\nu_{0} \dfrac{\partial{\mu_{2}}}{\partial{\varrho}}+\nu_{1} \dfrac{\partial{\mu_{1}}}{\partial{\varrho}}+\nu_{2} \dfrac{\partial{\mu_{0}}}{\partial{\varrho}}\right),$

and $\mathcal{I}_{2}(\varphi, \varrho) = \left(\mu_{0}\dfrac{\partial{\nu_{2}}}{\partial{\varphi}}+\mu_{1}\dfrac{\partial{\nu_{1}}}{\partial{\varphi}}+\mu_{2}\dfrac{\partial{\nu_{0}}}{\partial{\varphi}}\right) +\left(\nu_{0} \dfrac{\partial{\nu_{2}}}{\partial{\varrho}}+\nu_{1} \dfrac{\partial{\nu_{1}}}{\partial{\varrho}}+\nu_{2} \dfrac{\partial{\nu_{0}}}{\partial{\varrho}}\right)$.

    $\vdots$

In a general way,

With the addition of all $\mu$ and $\nu$,

The exact solution of Eq (6.22) at $\theta = 1$ and $q = 0$ is given by

7.   Result and discussion

Effective analytical techniques were used to analyze the solution of the time-fractional multi-dimensional N-S equation. The fractional derivatives are defined in the form of Caputo-Fabrizio, and are examined by the NITM and HPTM, along with NT. To verify that the suggested approaches are accurate and applicable, the graphical interpretation is illustrated for both fractional and integer orders for some examples.

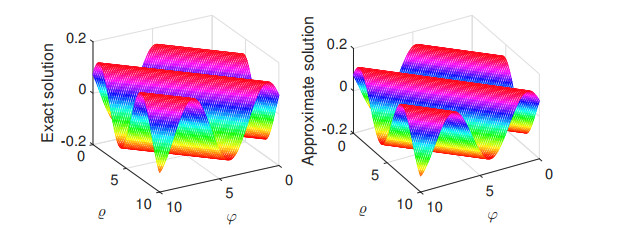
Figures 1 and 2 demonstrate the behavior of the exact and analytical solutions of Example 1 for $\mu(\varphi, \varrho, \ell)$ and $\nu(\varphi, \varrho, \ell)$ at $\theta = 1$, and demonstrate that the NITM solution figures are identical and in close contact with the exact solution of the example.

The physical attributes of $\mu(\varphi, \varrho, \ell)$ corresponding to the various fractional-orders $\theta = 0.2, \; 0.4, \; 0.6, \; 0.8$ of Example 1 are plotted in Figures 3 and 4.

Similarly, the graphical solutions of $\nu(\varphi, \varrho, \ell)$ for various fractional-orders $\theta = 0.2, \; 0.4, \; 0.6, \; 0.8$ of Example 1 are examined in Figures 5 and 6. It is shown that the NITM solutions are in strong agreement with the exact solutions and show a high rate of convergence.

Figures 7 and 8 represent the analytical and exact solutions of Examples 2 and 4 for $\mu(\varphi, \varrho, \ell)$ and $\nu(\varphi, \varrho, \ell)$ at $\theta = 1$.

It can be seen that the NITM solution figures are identical and in close contact with the exact solution of the example. Furthermore, in Figures 9 and 10, Examples 2 and 4 are calculated by the NITM method, and the value of $\mu(\varphi, \varrho, \ell)$ is examined corresponding to the various fractional orders $\theta = 0.2, \; 0.4, \; 0.6, \; 0.8$ by graphical interpretation.

Similarly, the graphical solution of $\nu(\varphi, \varrho, \ell)$ for various fractional orders $\theta = 0.2, \; 0.4, \; 0.6, \; 0.8$ of Example 2 is analyzed in Figures 11 and 12.

It is observed that the outcome of the NITM method and its graphical interpretation demonstrate the accuracy and applicability of the suggested techniques, and it is noted that the fractional-order solution exhibits the same convergence trends as that of integer-order solutions.

8.   Conclusions

This article presents the successful implementation of NITM and HPTM to evaluate the solution of the time-fractional multi-dimensional N-S equation analytically. The efficacy and accuracy of the proposed methods are examined with the support of four examples, and the outcomes show how effective, precise, and easy the methods are to use. The graphical interpretation of different values of the fractional-order $\theta$ on the solution profile is displayed in Figures 2–6 and in Figures 9–12, which demonstrate some interesting dynamics of the model. The results obtained by these methods are in a series form, and close agreement with those solutions is given by ^[44,45]. It is noted that there is a high rate of convergence between the series solutions obtained towards the solutions of integer order. Furthermore, the suggested methods are simple to use, and they may be used to solve additional fractional PDEs that arise in applied research.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The authors extend their appreciation to the Deputyship for Research and Innovation, Ministry of Education in Saudi Arabia for funding their research work through project number ISP-2024.

Conflict of interest

There is no competing interest among the authors regarding the publication of the article.