1.
Introduction
Menopause is a physiological process marked by the date of a woman's last menstruation that indicates definitive ovarian failure. Menopause is diagnosed retrospectively after 12 consecutive months of amenorrhea without any other pathological or physiological cause [1]. The age at which menopause occurs, generally between 45 and 55 years old, is primarily determined by genetics but also susceptible to the influence of environmental factors such as obesity, multiparity, physical activity, tobacco use, and alcoholism [2].
For women, entering into menopause can be associated with an increased prevalence of obesity, metabolic syndrome, cardiovascular disease, and osteoporosis [3]. Against those risks, diet is particularly relevant given its impact on quality of life and longevity and its modifiability [4]. Because menopause is also viewed as a time in women's lives when they are more susceptible to changing habits and acquiring healthier behaviors [1], it presents an excellent opportunity for health interventions as well.
Of all components of lifestyle, nutrition exerts some of the most significant impacts on postmenopausal women's quality of life and morbidity. Higher adherence to the Mediterranean diet (MD) has been shown to improve the prevention and management of age-associated non-communicable diseases, including cardiovascular and metabolic diseases, neurodegenerative diseases, cancer, depression, respiratory diseases, and fragility fractures [5]. In turn, the MD may also reduce total mortality in the population [6] and lengthen lifespans [7]. Therefore, the MD, currently recognized as one of the healthiest dietary models worldwide [8], may offer many benefits to women in the climacteric phase of life. The MD is characterized by a relatively high intake of vegetable products (i.e., vegetables, fruit, low-refined cereals, legumes, nuts, olives, and olive oil); the moderate consumption of fish, white meat, eggs, and dairy products (preferably in the form of cheese and yogurt); and the relatively low consumption of red and processed meat and wine with meals [9]. Given those characteristics, individuals with better adherence to the dietary pattern also have a better nutritional intake profile, including a high intake of fiber, vitamins, minerals, and phytochemicals (e.g., flavonoids, polyphenols, and carotenoids), along with a low glycemic index, a high monounsaturated: saturated fat intake ratio, and low omega-6: omega-3 fatty acid intake ratio [10].
Considering all of the above, the aim of this systematic review was to examine current evidence about the effectiveness of studies on MD-based interventions conducted among menopausal women.
2.
Materials and methods
2.1. Search strategy
A systematic search of peer-reviewed studies published up to July 2023 was conducted following the PRISMA guidelines [11], however, the study protocol had not been previously published. The search used the following databases: Scopus, PubMed, and Web of Science. The query question was (“intervention” or “food and nutrition education” or “trial” or “pilot study” or “program”) AND (“mediterranean diet”) AND (“menopause” or “menopausal”). Only studies published in the last 15 years were included. Reference lists of eligible studies were scanned to identify additional pertinent publications, and the bibliographic reference management software EndNote™ 20 software (Clarivate, Philadelphia, USA) was used.
2.2. Inclusion and exclusion criteria
All studies that provided the description of interventions with MD in menopausal women published in the last 15 years (2008–2023) were included. This encompassed randomized trials, pilot intervention without a control arm or experimental study. Exclusion criteria included studies not exclusively targeted on dietary intervention but involving various health behaviors (e.g., physical activity), as well as protocols, commentaries, and opinion papers. Articles were not excluded because of age, sample size, sampling methodology, or geographical location. The titles and abstracts of retrieved articles were independently evaluated by two researchers (CG and RS), not blinded to authorships.
2.3. Data extraction and analysis
Relevant information was extracted regarding the country, participants (number, age, and inclusion and exclusion criteria), study design, enrolment (start and end date), length of participant intervention and follow-up, as well as recruitment and sampling procedures, intervention description, and outcomes. When extracting data related to outcomes, the authors opted to focus on the parameters most mentioned in the literature regarding anthropometric/body composition parameters (weight, body mass index (BMI), total fat mass, visceral fat, fat-free mass, and waist circumference), blood pressure (systolic blood pressure (SBP) and diastolic blood pressure (DBP)), dietary (MD adherence, energy, fat, saturated fat, polyunsaturated fatty acids (PUFA), monosaturated fatty acids (MUFA), ω6: ω3 ratio, carbohydrates and fiber), and blood outcomes (ω6: ω3 ratio, triglycerides, total cholesterol, high density lipoproteins (HDL), low density lipoproteins (LDL), fasting glucose, homocysteine, C-reactive protein (CRP), and nitric oxide level (NO)). Data extraction was carried out by one researcher, CG, in consultation with a second researcher, RS, when required.
2.4. Studies quality assessment
The quality of randomized intervention studies were assessed by the revised Cochrane risk-of-bias tool for randomized trials (RoB 2 tool) [12]. This tool evaluates bias in five domains: (1) randomization process, (2) deviations from intended interventions, (3) missing outcome data, (4) measurement of the outcome, and (5) selection of the reported results. The possible risk-of-bias judgements in each domain are low risk of bias, some concerns, or high risk of bias. Furthermore, an overall risk-of-bias judgement of the study was done giving the evaluation of each domain. Studies were considered to have a high risk of bias if they were judged to be at least in one domain with high risk of bias. They were considered to have some concerns of bias if they were judged to raise some concerns for at least one domain. They were considered low risk of bias only if they were judged to be at low risk of bias for all domains.
The quality of non-randomised intervention studies were assessed by “Risk Of Bias In Non-randomised Studies - of Interventions” (ROBINS-I tool) [13]. This tool considered the assessment of bias in seven domains: (1) confounding, (2) selection of participants, (3) classification of interventions, (4) deviations from intended interventions, (5) missing data, (6) measurement of outcomes, and (7) selection of the reported result. The categories for risk of bias judgements are low, moderate, serious, and critical risk of bias. An overall risk-of-bias judgement was determined to be low risk of bias if the study was low risk for all domains, moderate risk of bias if the study was of low or moderate risk for all domains, serious risk of bias if the study was at serious risk in at least one domain, and critical risk of bias if the study was at critical risk in at least one domain. The risk-of-bias plots were generated using the app robvis [14].
3.
Results
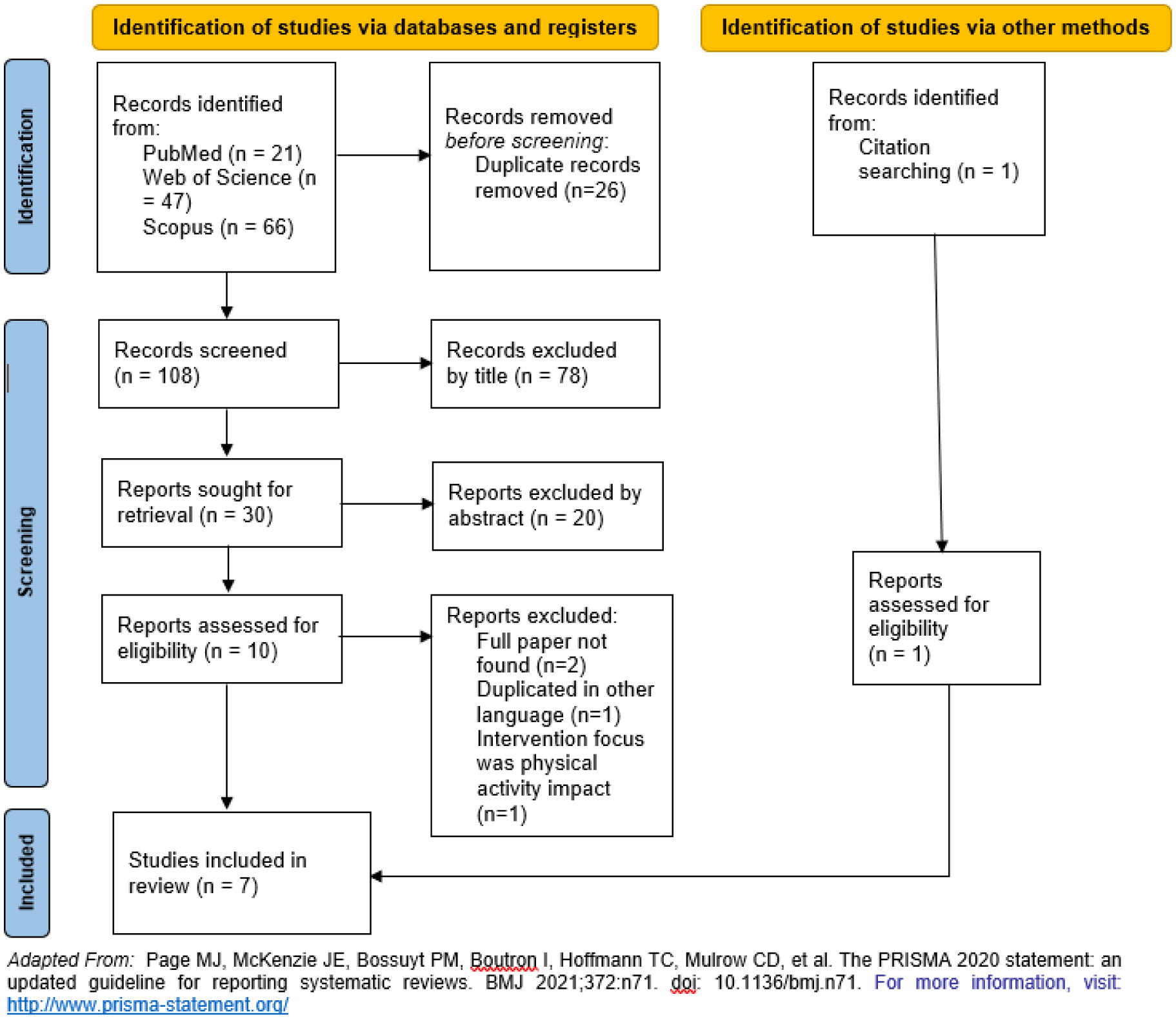
The first search yielded 134 potentially relevant publications (Figure 1). Following the removal of duplicates and title and abstract screening, 10 publications were retrieved for full-text screening, and at the end six were included in the systematic review. One study was included resulting from the reference list of eligible studies.
From the seven intervention studies, three were conducted in countries bordering the Mediterranean (Spain [15] and Italy [16],[17]), three were from of Northern Europe (Poland [18]–[20]) and one was from USA [21]. The number of participants varies between 16 and 230, and all studies included menopausal women with mean ages ranging from 49–77 years old (Table 1). The supplementary table provide details in inclusion and exclusion criteria for participants selection.
Two studies were non-randomized intervention studies. One was a controlled before-and-after study involving 16 menopausal women following the MD [21] and the other was a case-control study, comparing menopausal women (case) with fertile women over 45 years old (control) intervein with MD [16]. Five studies were randomized trials, randomizing participants to the MD or usual diet [15], or placebo [17], or Central European diet (CED) [18]–[20] (Table 2). The follow-up ranged from 2 months to 1 year, and the intervention spanned from 1 week to 1 year. The intervention designs are diverse, and included education workshops [15], prescription of the MD by a nutritionist [16],[21], oral supplementation with enriched extra virgin olive oil [17], and the provision of pre-packaged main meals according to MD plus dietary advice [18]–[20].
The included studies aimed to assess the effect of the MD on various parameters, including the reduction of weight [15],[16],[20] and changes in body composition [15], cardiovascular risk factors [17],[19]–[21], atherosclerosis prevention [18], and bone health [17]. To assess these effects, studies evaluated several outcomes that authors summarize in Table 3 (anthropometric and blood pressure outcomes), Table 4 (dietary outcomes), and Table 5 (blood outcomes). One study also evaluates the association between genetic polymorphism of FADS1 and FADS2 genes and fatty acids concentrations after MD intervention period [19].
In general, MD was modestly efficacious at reducing body weight (ranging from -0.2 to -7.7 kg)[16],[20],[21], with significant changes only in one study [20]. No significant effects on BMI were found. Regarding body composition, one study reported a significant reduction in total fat mass (mean change -6.6 kg) [20], discrepant changes were found in visceral fat between studies (one with significant increase [15] and another with significant decrease [20]). A reduction on fat free mass (ranging from -0.6 to -1.1 kg) [16],[20] was noted, with only in one study achieving significant changes [20]. Waist circumference (ranging from -0.1 to -7.4 cm), SBP (ranging from -9 to -10.2 mmHg) and DBP (ranging from -6.7 to -7 mmHg) decreases after MD intervention [15],[16],[20], however significant changes only found in one study [20] (Table 3).
Three studies evaluated dietary outcomes (Table 4) [15],[20],[21]. One of these studies present MD adherence score from MEDAS-14 questionnaire [22], while the others reported the MD adherence score from MedSD questionnaire [23] and nutritional analysis from multiple 3-day diet records [21] or from daily food records [20]. In both studies [15],[21] MD interventions significantly increased the adherence to the MD, increased the intake of fat, PUFA, MUFA, and decreased the intake of saturated fat, ω6: ω3 ratio and carbohydrates. However, there was discrepancies in the findings related to energy intake. Bihuniak et al. [21] reported that energy intake increased and Bajerska et al. [20] found a significant decrease.
Changes in lipid levels were verified in five studies [16],[17],[19]–[21] (Table 5). It was verified that MD led to a decrease in triglycerides (ranging from -33.9 to -5.8 mg/dL), total cholesterol (ranging from -22.8 to -2.3 mg/dL), LDL (ranging from -28.2 to -4.0 mg/dL), and ω6: ω3 ratio (ranging from -1.5 to -0.2). The effect of MD on HDL, yielded different results, with two studies reporting an increase in HDL values [16],[17] and the other two showing a decrease in HDL values [20],[21]. Bajerska et al. [20] verified a significant increase in homocysteine levels (mean change +0.7 µM, p = 0.002), this could be relevant since some authors correlates homocysteine with cardiovascular problems [24]. MD also showed significant and beneficial impact on C-reactive protein (CRP) (mean change -1.2 mg/L) [18] and on nitric oxide level (NO) (mean change -6.6 nmol NO/mg protein) [17], both indicators of CVD risk, due to the development of atherosclerosis that is associated with inflammation within the vessel walls.
The MD produced similar effects on glycemic control (Table 5), since all of them showed reduction on fasting glucose levels (ranging from -6.4 to -0.8 mg/dL) [16],[17],[20]. Notably, the study from Bajerska et al. showed significant decrease on fasting glucose levels and in homeostatic model assessment of insulin resistance (HOMA2-IR) in women submitted to MD (mean change -0.46, p < 0.0001) [20].
The five randomized studies included exhibited degrees of bias of low concern [17], some concerns [18]–[20], and one with high concern [15] (Figure 2). The domain with poorest performance in the analysis was the bias related to deviations from intended interventions.
The two non-randomized studies showed a serious risk of bias [16],[21], mainly due to confounding variables (e.g., physical activity level), deviations from intended interventions, and missing data (Figure 3).
4.
Discussion
In 2020, the United Nations estimated that, worldwide, 985 million women were 50 years old or older, and that number is projected to increase to 1.65 billion by 2050 [25]. Therefore, women are living longer, and the phase of their life lived in menopause is increasing as well. In that context, the immediate and long-term impacts of estrogen depletion and aging on health pose a significant challenge to health care systems around the world.
Optimizing diet is increasingly recognized as being crucial in strategies aimed to promote women's health during the menopause period [4]. The MD, characterized by the high intake of plan-based foods, is considered by several authors to be a possible explanation for improved bone metabolism, enhanced muscle performance, and reduced oxidative stress, inflammation, and insulin resistance [4],[26]. Therefore, gaining insights into the impacts associated with adhering to the MD on menopausal women's health is essential for the appropriate and specific design of public health interventions.
This systematic review, addressing the effectiveness of MD-based interventions conducted among menopausal women, suggests that adhering to the MD can have beneficial impacts during the climacteric phase of life, including the reduction of weight, blood pressure, blood ω6: ω3 ratio, triglycerides, total cholesterol, and LDL levels.
Menopause has been associated with an increase in body weight and changes in body composition such as an increase in fat mass and a reduction in fat-free mass. That shift is attributed to the reduction of basal metabolic rate, diminished physical activity, and the loss of estrogen's influences on lipoprotein lipase activity and lipolysis [27],[28]. Moreover, changes in body composition reflect the significant decline in muscle mass, with a sharp annual decline after the age of 50 years, ranging between 0.6% and 2%, most prominently in the first 3 years after the onset of permanent amenorrhea [29],[30]. Those changes can be critical for the risk of insulin resistance, diabetes, and cardiovascular diseases. The studies included in this systematic review suggest that following the MD is associated with reduced body weight but cannot reduce BMI and can exert conflicting impacts on body composition (e.g., total and visceral fat levels). The observed discrepancies in results regarding BMI may be partially explained by the reduction in women's height, often linked to worsened bone condition in the spine, such that osteoporotic vertebral fractures are common in the postmenopause period but are seldom clinically identified [31]. Other authors have found that the MD can result in greater weight loss than a low-fat diet [32] and that a higher adherence to the diet is associated with increased likelihood of maintained weight loss [33] and reduced abdominal adiposity, particularly visceral fat levels [34],[35]. However, the particularities of menopausal women can be complex, and total energy intake and physical activity level can be important confounders that should be more rigorously controlled in future studies.
The accelerated loss of fat-free mass, also reported to occur during menopause, increases the risk of sarcopenia, a condition that can lead to functional impairment and physical disabilities [36]. The accelerated loss of fat-free mass reflects a decline in both muscle mass and bone mass, the latter of which intensifies after menopause [37]. The results of this review generally show the reduction of fat-free mass in menopausal women following a MD-based dietary intervention. Moreover, although no clinical trials were found that examined adherence to the MD and sarcopenia, a systematic review of observational studies has shown no evidence of the MD's positive effect on sarcopenia other than its general positive role in improving muscle mass and muscle function [38].
During the reproductive period, the production of oestrogens exerts a protective effect on endothelial function and lipid metabolism, with a decrease in LDL and the ratio of total cholesterol and HDL. Estrogen, along with changes in the lipid profile, contributes to improved vasodilation and reduced levels of homocysteine and fibrinogen [39]. Conversely, the drop in estrogen levels in the bloodstream may potentially contribute to an increase in blood pressure through various mechanisms, such as influencing the arterial wall, activating the renin–angiotensin system, and stimulating the sympathetic nervous system [40]. Those physiological changes are associated with and elevated risk of cardiovascular diseases and the higher prevalence of metabolic syndrome [4]. The results of this review suggest that menopausal women who follow the MD may experience benefits such as reduced blood pressure, triglycerides, total cholesterol, and LDL levels. Those results align with the findings of other authors [32],[41],[42] and can be partly explained by components of the MD such as a high prevalence of unsaturated fats in sources of fiber and protein, a scarcity of saturated fats, and a richness of fruits, vegetables, whole grains, nuts, and legumes, all of which contribute to the dietary pattern [41]. Other authors have also found that supplementation of fish oil rich in omega-3 significantly improves endothelial function and reduces pro-inflammatory markers among patients with diabetes due to its antioxidant and anti-inflammatory properties [43].
Increased blood pressure with aging can be also explained by increased sensitivity to salt with aging, which contributes to the development of age-related cardiovascular disease [44]. The MD, however, makes no prescriptions regarding salt intake, and Viroli et al. [45] did not detect any differences in sodium intake between a lower versus higher adherence to the MD. Another recent systematic review concluded that among individuals more than 65 years old, high potassium intake (i.e., >3510 mg/d) and a low sodium: potassium ratio (i.e., <1) have a beneficial effect on reducing the risk of cardiovascular disease. That positive outcome is largely because the potassium present in fruits and vegetables can mitigate the harmful effects of excess sodium intake [46].
A systematic review encompassing all meta-analyses and randomised controlled trials that compared the MD with a control diet on the treatment of type 2 diabetes and prediabetic states found that MD was associated with better glycemic control and improved cardiovascular risk factors than control diets, including those characterized by lower fat content [47]. That association was attributed to components of the MD that are considered to be anti-inflammatory and antioxidative (e.g., fiber, vitamins, and minerals, as well as antioxidants and polyphenols), coupled with a lower intake of proinflammatory foods and nutrients (e.g., saturated and trans fatty acids, refined sugars, and starches). Those explanations align with the findings of the present review, which all showed a reduction in fasting glucose levels among menopausal women [16],[17],[20].
It may be surprising that the present systematic review focused on the effectiveness of dietary interventions but only three studies assessed dietary outcomes [15],[20],[21], primarily to control adherence to the intervention. That point is significant because most health-related outcomes can be directly influenced by differences in the daily total energy and nutritional intake of participants. Two of the studies evaluated adherence to the MD using two different scoring systems [15],[21], which could also be viewed as a limitation. After all, using a score to estimate a dietary pattern is limited by subjectivity, which can cause considerable variability in the interpretation of the results [48].
Another limitation of the systematic review was that most studies did not include assessments of physical activity or total energy intake, both of which could be significant confounders affecting the outcomes. Thus, the interpretation of the results should be approached with caution. Beyond that, the relatively short duration of interventions (e.g., 1 week) could be insufficient to achieve meaningful results.
5.
Conclusions
In conclusion, this systematic review suggests that adhering to a MD can have beneficial impacts on menopausal women's health, including the reduction of weight, blood pressure, blood ω6: ω3 ratio, triglycerides, total cholesterol, and LDL levels. Those findings appear to be relevant in the context of public health interventions to aimed to enhancing the quality of life for menopausal women.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.









 DownLoad:
DownLoad:




