1.
Introduction
Stochastic volatility models driven by fractional Brownian motion have been gaining attention in the area of mathematical finance, since many empirical studies find that the decay in the autocorrelation function of the volatility is better modeled by a power function than an exponential function and fractional stochastic volatility models generate better fits to the observed market implied volatility surface. Refer to, for example, Comte and Renault [9], Alˊos et al. [2], Bayer et al. [4], Garnier and Solna [17], Forde and Zhang [12], Gatheral et al. [19], Guennoun et al. [20], Kim et al. [21], Bennedsen et al. [5], Fukasawa and Gatheral [16], Shi and Yu [30], and Cont and Das [10], among others. While Comte and Renault [9] stressed that fractional Brownian motion was relevant to capture long memory in stochastic volatility, Gatheral et al. [19] showed that log-volatility behaves essentially as a fractional Brownian motion with a Hurst exponent of order 0.1, at any reasonable time scale. A recent paper by Wang et al. [33] also found that the logarithmic daily realized volatility series of various financial assets have rough sample paths. However, fractional Brownian motion is not a semimartingale unless the Hurst exponent is 1/2, as shown in Rogers [28], leading to a possible arbitrage opportunity (a free lunch with vanishing risk, as a general term). "Arbitrage" means profiting from a price gap between a derivative and a portfolio of assets that replicates the derivative's cash flows. So, to get around this problem while taking into account the long or short memory property, there have been studies using the mixed fractional Brownian motion of Cheridito [8], the approximate fractional Brownian motion of Thao [32], or the generalized fractional Brownian motion introduced by Pang and Taqqu [24].
We are interested in the approximate fractional Brownian motion introduced in Thao [32] in the context of modeling stochastic volatility for pricing derivatives. This process is a semimartingale even if the Hurst exponent is not 1/2 (contrary to the original fractional Brownian motion) and so the no-arbitrage theory can be applied to obtain a partial differential equation (PDE) for the option price. There are several works which have used this process for underlying asset price models or stochastic volatility models. Refer to, for instance, Dung [11] for the Black-Scholes model, Sattayatham and Intarasit [29] for a jump-diffusion model, Pospisil and Sobotka [26] for the Heston stochastic volatility model, and Chang et al. [7] for the double Heston stochastic volatility model. In the present paper, we apply approximate fractional Brownian motions to the multiscale stochastic volatility model of Fouque et al. [14]. The merit of this stochastic volatility model is that the resulting option price approximation is independent of the particular details of the volatility model and leads to more flexibility in the parametrization of the implied volatility surface. We take two approximate fractional Brownian motions with two Hurst exponents (instead of two standard Brownian motions) corresponding to two characteristic (fast and slow) time scales of the multiscale volatility model, respectively. As far as we know, this approach does not exist in the literature. However, this type of volatility formulation is consistent with some of the previous works related to fractional Brownian motion. According to Xiao and Yu [35,36], the asymptotic distribution for the estimator of the persistence parameter is different when the Hurst exponent is less than 1/2 from that when it is larger than 1/2 in the fractional Vasicek model. Alˊos and Leon [3] found, based on the Clark-Ocone-Haussman formula for the integrated variance, that the volatility can be composed of terms with a Hurst index less than 1/2 being more relevant at short scales and terms with Hurst index greater than 1/2 being more relevant at long scales. Also, Bennedsen et al. [5] discovered evidence consistent with the hypothesis that time series of realized volatility are both rough and very persistent. On the other hand, Cont and Das [10] observed interestingly that even when the instantaneous volatility has the same roughness as Brownian motion, the realized volatility exhibits behavior corresponding to a Hurst exponent significantly smaller than 1/2. This observation supports our use of approximate fractional Brownian motion instead of fractional Brownian motion. The approximate fractional Brownian motion is thought of as between the standard Brownian motion and the fractional Brownian motion. It is a stochastic process equipped with a Hurst parameter, i.e., a measure of long-term memory of the time series. It is, however, a semimartingale in the form of a Brownian motion plus a time (Riemann) integral of an adapted process. So, the arbitrage opportunity can be excluded from the fundamental theorem of asset pricing and we are allowed to use the replicating portfolio method to obtain the corresponding PDEs for European vanilla and exotic options. The contribution of this work is as follows. We obtain approximate closed-form formulas for the prices of European vanilla and two exotic options. Our results cover both long- and short-memory properties of volatilities and control the skew slope by selecting appropriate Hurst exponents of the fast and slow scale volatility movements. It unifies two previously known results regarding the Hurst parameter dependence of the blow-up and slow-flattening behavior of skews and smiles of implied volatility surfaces. Consequently, the implied volatility surfaces can be calibrated over a wide range of time-to-maturities. Also, we provide a calibration method by representing the observed SPX option prices in terms of the term structure of the implied volatility formula. Based on the calibration result, we find that the implied volatility becomes higher when the fast-scale motion of the (spot) volatility becomes "rougher" and the slow-scale motion of the volatility becomes "smoother", which in turn supports the necessity of a multiscale modeling framework for stochastic volatility.
The paper is organized as follows. In Section 2, we use approximate fractional Brownian motions to establish a stochastic volatility model. In Section 3, we apply the replicating portfolio method to obtain the corresponding PDE formula for the price of a European vanilla option and derive explicitly a closed-form formula for the approximate option price using the combination of singular and regular perturbations and the Mellin transform method. Subsequently, a closed-form formula for the implied volatility corresponding to a European call option is obtained in Section 4. We check the accuracy of the pricing formula, show how to calibrate the pricing parameters, and investigate the sensitivity of the implied volatilities to the Hurst exponents in Section 5. We extend the vanilla option price formula to two exotic-option cases in Section 6. Finally, Section 7 provides some concluding remarks.
2.
Model formulation
A fractional Brownian motion BHt with a Hurst exponent H, 0<H<1, is defined by a centered Gaussian process satisfying the covariance function
The process BHt is a self-similar process but is neither a semimartingale nor a Markov process except in the case where H=1/2. Mandelbrot and van Ness [22] gave an integral representation of the general fractional Brownian motion as follows:
where Wt is a standard Brownian motion. The last integral part of (2.1) is a self-similar Gaussian process, which becomes a Brownian motion for H=1/2 and has non-stationary increments for H≠1/2. It is a truncated version of the general fractional Brownian motion and is usually called Riemann-Liouville fractional Brownian motion with a Hurst index H. This type of fractional Brownian motion has been widely used in the modeling of volatilities. See, for example, Comte and Renault [9], Alˊos et al. [2], Bayer et al. [4], and Gatheral et al. [19], among others. It has a simple representation but it is not a semimartingale for H≠1/2. Thus Thao [32] used a perturbation parameter, say γ, to introduce an approximate fractional Brownian motion defined as
where Wt is a standard Brownian motion, and proved that Bγ,Ht is a semimartingale and converges to the last integral of (2.1) in L2(Ω) sense and uniformly with respect to t∈[0,T] for any fixed positive number T when γ goes to 0.
In this paper, we use the process Bγ,Ht as a random source of the volatility of the underlying risky asset return to introduce a new model given by
under a risk-neutral probability measure Q. The model (2.2) is the same as the multiscale stochastic volatility model in Fouque et al. [14] except that the standard Brownian motions driving the two stochastic volatility factors Yt and Zt are now replaced by the approximate fractional Brownian motions. Since, by the Itˆo formula (see Oksendal [23], for example), the differential of the approximate fractional Brownian motion is
the initial model (2.2) becomes
where ϕ1,t and ϕ2,t are defined by
where (t−s+γ)H−32 does not blow up at any s∈[0,t] and H∈(0,1). In the model (2.3), we assume that 0<δ≪ϵ≪√δ<1, Wxt, Wyt, and Wzt are standard Brownian motions defined on a filtered probability space (Ω,F,Ft,Q), they have a correlation structure given by d⟨Wx,Wy⟩t=ρxydt, d⟨Wx,Wz⟩t=ρxzdt, and d⟨Wy,Wz⟩t=ρyzdt, and H1∈(0,12) and H2∈(12,1). If Yt is a mean-reverting process, its mean itself is also mean-reverting slowly, and it is driven by Zt, then this situation is called double-mean-reverting (cf. Gatheral [18]). Wy and Wz are assumed to be correlated here so that the model can somewhat capture the double-mean-reverting property of stochastic volatility even if the dependence of Yt on Zt is not explicitly specified. The functions f, α, β, g, and h are assumed to satisfy necessary smooth and boundedness conditions for the stochastic differential equation for Xt to have a unique solution. The volatility factor Yt in (2.3) reflects rapid variation (for example, rapid mean reversion) while the volatility factor Zt represents slow variation because those processes correspond to the solutions of stochastic differential equations in which time t is replaced by t/ϵ (sped up) and δt (slowed down), respectively. Particularly, the process Yt is assumed to be ergodic and have an invariant distribution, denoted by Φ, which allows us to use averaging principles in Fouque et al. [15] to approximate the option price. Of course, the model (2.3) is reduced to the model of Fouque et al. [14] if both H1 and H2 are equal to 1/2.
While fractional Brownian motion was stressed to capture long memory in the early age of fractional stochastic volatility model development such as the study of Comte and Renault [9], it has been discovered empirically since then that those models are valid only for long-term behavior of volatility, while some rough volatility models are more appropriate in the short run (see, in particular, Gatheral et al. [19]). This has led several authors to introduce volatility models incorporating both roughness, meaning exponentially decaying autocorrelation, and long memory, meaning non-integrable autocorrelation, corresponding to two different Hurst exponents. Refer to Alˊos and Leon [3] and Bennedsen et al. [5], for instance. This paper seeks to relate two characteristic (fast and slow) time scales of the multiscale stochastic volatility model of Fouque et al. [15] to the roughness and the long memory, respectively. This approach allows us to obtain an option pricing formula that can be calculated easily starting from the Black-Scholes price.
3.
Option price formula
3.1. Singular perturbation problem
In this paper, the following lemmas are useful for asymptotic analysis. They are the solvability condition of a Poisson equation and the growth condition related to the infinitesimal generator of the ergodic process Yt, 1ϵA0, where A0 is a differential operator defined as
Lemma 3.1. The Poisson equation
has a solution p(t,x,y,z) if and only if the function q is centered with respect to the invariant distribution Φ of the process Y, i.e.,
Proof. This is a version of the Fredholm alternative. Refer to Section 3.2 in Fouque et al. [15]. □
Lemma 3.2. Assume that equation A0p(t,x,y,z)=0 admits only solutions that do not grow as fast as
Then the solution p does not depend on y.
Proof. Solving the equation A0p=0 directly leads to this result. □
Since the approximate fractional Brownian motion Bγ,Ht is a semimartingale, the no-arbitrage theory is allowed for the model (2.3) by the fundamental theorem of asset pricing (see Pascucchi [25], for example). So, one can use the replicating portfolio approach to value the options. If Pϵ,δ(t,x,y,z;ϕ1,ϕ2) denotes the option price with a payoff function H(x) under the model (2.3) when Xt=x, Yt=y, Zt=z, ϕ1,t=ϕ1, and ϕ2,t=ϕ2, then the no-arbitrage argument with the self-financing assumption and the Itˆo formula leads to a final value problem expressed by
where the multiscale operator Aϵ,δ is
where the operator symbol Dn is defined by
3.2. Approximation
From now on, the dependence of Pϵ,δ on ϕ1 and ϕ2 is omitted for notational simplicity. Since the PDE problem (3.1) is a singular perturbation problem, we are interested in an asymptotic solution of the form
Following the multiscale asymptotic analysis of Fouque et al. [15] and using the operator A BS defined by
one can find that P00, P01, and P10 are independent of the variable y and they satisfy the PDE problems
respectively, where the functions AH11, AH12, BH21, and BH22 are
respectively. Here, ψ(y,z) is a function defined by the solution to
Note that AH11(z) and AH12(z) are related to the fast variation of volatility and a Hurst exponent less than 12 while BH21 and BH22 are connected with the slow-scale variation of volatility and a Hurst exponent larger than 12.
Since A BS is the differential operator ∂t plus the infinitesimal generator of a geometric Brownian motion solving the stochastic differential equation
as its notation suggests, the PDE problem A BSP00(t,x,z)=0 with the final condition P00(T,x,z)=H(x) gives us that P00 is the Black-Scholes option price (cf. Black and Scholes [6]) with constant volatility replaced by z-dependent volatility, and subsequently we use notation P BS(t,x,z) instead of P00(t,x,z) from now on.
By solving the PDE problems in (3.4) for P BS, P01, and P10, we obtain the following European option price formula.
Proposition 3.1. Under the dynamics of (2.3) of the underlying asset price, the option price Pϵ,δ is approximated by ¨Pϵ,δ:=PBS+√ϵP01+√δP10, that is
where Dn1:=(D1)n=(x∂∂x)n and Aϵ,H11, Aϵ,H12, Bδ,H21, and Bδ,H22 are given by
respectively.
Proof. To solve the PDE problems in (3.4) for P BS, P01, and P10, we use the Mellin transform and its inverse transform defined by
respectively, where a is a real number, and obtain the ODE problems for ˆP01 and ˆP10 as follows.
where ˆh(ω) is the Mellin transform of h(x) and the functions λ(ω,z), η1(ω,z), and η2(ω,z) are
respectively. The solutions of (3.8) are given by
Substituting (3.9) into (3.10), we obtain the following Mellin transform of ¨Pϵ,δ explicitly:
The pricing formula (3.11) is given by a linear combination of terms that are in the form of a product of ˆP BS and a polynomial function of ω. So, we can calculate the formula explicitly through the following property of the Mellin transform: M((D1)nf)(ω)=(−ω)nˆf(ω). Using an inverse Mellin transform on (3.11), we obtain a closed-form formula given in the proposition. □
Therefore, once the Black-Scholes option price P BS is given, we can calculate the approximation ¨Pϵ,δ by computing the derivatives of P BS in the formula (3.7) and plugging the estimated group parameters Aϵ,H11, Aϵ,H12, Bδ,H21, and Bδ,H22 into (3.7). Note that the original model parameters and functions such as γ, ϵ, δ, α, β, g, and h are not required to be directly chosen for the purpose of the option price approximation. The group parameters are required to be chosen for calculating ¨Pϵ,δ. We use the implied volatility term structure of SPX call options to estimate those four group parameters. See Section 5.2 for details.
Remark: One can obtain the second-order terms P02, P11, and P20 further and a subsequent formula for the approximation ⃛Pϵ,δ:=P BS+√δP10+√ϵP01+δP02+√δϵP11+ϵP20 as shown in Appendix A, where the approximation error is also given. We note that the same approach (approximation to the Black-Scholes price) used in this section and the following section are also used in the more simple framework of the Heston model driven by the standard Brownian motion in Alˊos et al. [1].
4.
Implied volatility
All of the original model parameters given in the model (2.3) are not required to price derivatives. In fact, from Proposition 3.1, we notice that ˉσf, Aϵ,H11, Aϵ,H12, Bδ,H21, and Bδ,H22 are the ones required to be estimated. In order to estimate those pricing parameters, we can utilize calibration from near-the-money European call option implied volatilities. The volatility Iϵ,δ implied by the pricing formula (3.7) is defined by the solution to the equation P BS(t,x;Iϵ,δ)=¨Pϵ,δ(t,x,z), where P BS(t,x;σ) stands for the classical Black-Scholes call option price formula with volatility σ. Then the two correction terms I01 and I10 of the asymptotic expansion
are given by I01=(∂σPBS)−1P01 and I10=(∂σPBS)−1P10, respectively, and the leading term I00 is defined as
Using the vega-gamma and speed-gamma relationships, i.e.,
we can obtain an approximate implied volatility surface ¨Iϵ,δ:=I00+√ϵI01+√δI10 given by
We are interested in the slope behavior of the implied volatility skew with respect to time-to-maturity and the Hurst exponent. The slope of the implied volatility skew is given by
where k:=logK.
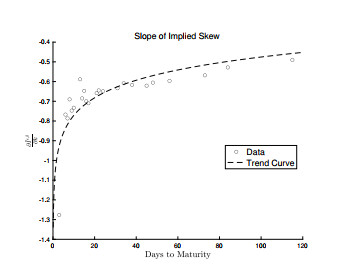
Based on the calibration result shown in Section 5.2 for SPX options, we do a numerical experiment to show how the skew slope behaves against time-to-maturity. Figure 1 presents the experimental result for the skew slope term structure. It shows that the slope tends to blow up as time-to-maturity becomes shorter.
Comte and Renault [9] considered a Hurst exponent with H>12 to explain the slow flattening of skews and smiles of the implied volatility surface when time-to-maturity increases, while Alòs et al. [2] gave a better description of the short time-to-maturity blow-up of the implied volatility surface with a Hurst exponent H<12. So, our result is consistent with these two results in that the skew slope becomes small when time-to-maturity increases while it becomes large when time-to-maturity decreases as seen in Figure 1. Our result unifies these two separate previous results. This is desirable in practice as the market volatility has both long- and short-memory properties depending on the situation. As described by (4.2), the skew slope ∂¨Iϵ,δ∂k can behave flexibly depending on the appropriate Hurt parameters H1 and H2 in the range of (0,12) and (12,1), respectively. As a consequence, the implied volatility surface can be calibrated over a wide range of time-to-maturities.
5.
Performance, calibration, sensitivity, and accuracy
In this section, we check the accuracy and performance of the price formula given by (3.7) in Proposition 3.1 for European call options via Monte Carlo simulation. We give an example of calibrating the pricing parameters for three different time-to-maturities. We also investigate the sensitivity of the implied volatility to the Hurst exponents H1 and H2.
Using the well-known Greeks in the Black-Scholes model, one can verify easily that the derivative price ¨P(t,x,z) given by (3.7) satisfies the following identity for a European call option with strike K:
where N, d1, d2, and φ are given by
respectively.
5.1. Performance of the formula
In this section, we calculate option prices under three different models and a Monte Carlo simulation result (with 1 million simulations) and compare them with real market data, that is, the SPX option data observed on December 7, 2022. Notations Pmarket, PMC, PfMSV, PMSV, and PBS stand for the market option price, the Monte Carlo (MC) simulation result, the option price computed by the formula (5.1) corresponding to the fractional multiscale stochastic volatility (fMSV) model (2.3), the option price computed under a multiscale stochastic volatility (MSV) model corresponding to the case of H1=H2=12 in the formula (5.1), and the Black-Scholes option price, respectively.
For Monte Carlo simulation, we need random numbers generated by the stochastic processes ϕ1,t and ϕ2,t in (2.4). The time interval [0,T] is discretized into t0(=0), t1, t2, ⋯, tn(=T) satisfying t0<t1<⋯<tn with Δt=ti−ti−1, i=1,2,⋯,n. Omitting the subscript number and superscript letter of ϕ1,t, ϕ2,t, H1, H2, Wys, and Wzs (and so using ϕt, H, and Ws), the random source ϕt satisfies the following recursive equation by the Itˆo calculus:
where the notation d= denotes distributional equality and the Zi's are independent and identically (standard normal) distributed. Thus, we generate the random sources ϕti, i=1,2,⋯,n, recursively, by the following algorithm:
Applying this algorithm to (2.3), we can obtain a Monte Carlo simulation result PMC for the European options. Table 1 represents the setting of the related parameters and functions for finding the price PMC.
We calculate Pmarket, PMC, PfMSV, PMSV, and PBS for a European call option with two time-to-maturities, where PMC is obtained based on the setting in Table 1. Using these results, we compute the square norms ∥Pmarket−PMC∥, ∥Pmarket−PfMSV∥, ∥Pmarket−PMSV∥, and ∥Pmarket−PBS∥ for the purpose of comparing the Monte Carlo simulation and the three different volatility models. Table 2 presents the result. It shows that the fMSV model outperforms the other models including the Monte Carlo simulation. This tends to be the case more conspicuously when time-to-maturity becomes shorter. Note that shorter time-to-maturity options tend to have higher trading volume in general. Therefore, using the approximate fractional Brownian motion instead of the standard Brownian motion for volatility seems to provide a great advantage in option pricing. On the other hand, Table 3 provides the elapsed time of option pricing based on the Monte Carlo simulation (reputation number = 1,000,000 and dt = 1/25200), and the three different volatility models. The computer used for the computation is specified as Intel(R) Core(TM)-i9-10900 CPU, Windows 10 Pro O/S and 64GB RAM. Moreover, the program used for the computation is MATLAB R2022b. The Monte Carlo simulation method takes much more time than the analytic methods based on the three different volatility models while the three different models are relatively similar to each other in terms of the elapsed pricing time.
Among those parameters required to be estimated, i.e., ˉσf, Aϵ,H11, Aϵ,H12, Bδ,H21, and Bδ,H22, the parameter ˉσf is first estimated from historical SPX data over a period of time in the near past, where the slow-scale variable (z) dependence of ˉσf accounts for updating the long-run average from time to time. To estimate the group parameters Aϵ,H11, Aϵ,H12, Bδ,H21, and Bδ,H22, we rewrite the implied volatility surface (4.1) as
where the parameters aϵ, bϵ, cδ, and dδ are related to the pricing parameters Aϵ,H11, Aϵ,H12, Bδ,H21, and Bδ,H22 through the relationship
So, once aϵ, bϵ, cδ, and dδ are estimated from calibration to the implied volatility term structure of SPX call options, one can use the relationship (5.3) to estimate the pricing group parameters Aϵ,H11, Aϵ,H12, Bδ,H21, and Bδ,H22 and calculate the derivative price ¨Pϵ,δ(t,x,z) obtained in Proposition 3.1.
More concretely, the averaged volatility ˉσf is first estimated using the 10-day historical volatilities calculated from the SPX data obtained from the site http://www.investing.com and then the parameters aϵ, bϵ, cδ, and dδ are estimated using the SPX call option data obtained from the site http://www.barchart.com. Figure 2 shows the implied volatilities of the SPX option in the real market and the curve ¨Iϵ,δ fitted to the market data. From this fit, aϵ, bϵ, cδ, and dδ are estimated and then the pricing group parameters Aϵ,H11, Aϵ,H12, Bδ,H21, and Bδ,H22 are determined. Figure 3 demonstrates the corresponding result observed at one day.
5.2. Sensitivity to H1 and H2
The Hurst exponents H1 and H2 related to the fast- and slow-scale variations of the volatility, respectively, are important parameters in our underlying asset price model (2.3). To investigate the dependence of the implied volatility (4.1) on the Hurst exponents, we rewrite (4.1) as follows:
where aϵ1(H1), aϵ2(H1), bδ1(H2), and bδ2(H2) are
respectively.
In Figure 4, we demonstrate the behavior of the implied volatility ¨I with respect to the Hurst parameters H1 and H2. Figure 4(a) shows ¨I against H1 for a variety of H2 while Figure 4(b) shows ¨I against H2 for a variety of H1. The figures indicate that ¨I increases as H1 decreases or H2 increases for any fixed H2 or H1, respectively. So, the implied volatility becomes higher when the fast-scale motion of the volatility becomes "rougher" and the slow-scale motion of the volatility becomes "smoother". This is an interesting result in view of modeling stochastic volatility. This provides us with one of the reasons why we need a multiscale framework for stochastic volatility.
5.3. Accuracy
In this section, we check the accuracy of the approximation PfMSV with respect to the parameters γ, ϵ, and δ. We choose a very small value, close to zero, of each of the parameters and show that the approximations converge to the Monte Carlo simulation result, denoted by PMC*, with the small parameter(s).
Table 4 shows how PfMSV converges to PMC*=41.2643 as γ goes to 0.0001. On the other hand, Table 5 shows how the approximations PfMSV move to the Monte Carlo simulation result PMC*=41.2576 when ϵ and δ go to 1.0000×10−6 and 1.0000×10−9, respectively. We note that the correlation term ρyz does not appear in the first-order approximation of our interest in this article but it would appear in higher order approximation. We assume ρyz=0 in the numerical experiment in order to match the situation given by our first-order approximation.
6.
Extension to exotic options
In general, the prices of exotic options can be determined after option pricing models are calibrated to market data of plain vanilla options. In this section, we extend the pricing result for European vanilla options under the fractional multiscale stochastic volatility model (2.3) to two types of path-dependent exotic options, i.e., barrier and lookback options.
6.1. Barrier option
Barrier options are similar to vanilla options but they only become activated or extinguished when the underlying asset hits a specific price level (the so-called "barrier"). So, the value of barrier options can jump up or down greatly. This type of option is commonly traded in the foreign exchange and equity markets.
Given the model (2.3), let Uϵ,δ(t,x,y,z) be the price of a down-and-out (D/O) barrier option, where a payoff function is given by
with a strike price K, a barrier level B, and an expiration time T. From the no-arbitrage theory with the self-financing condition and the Itˆo formula, Uϵ,δ(t,x,y,z) satisfies the PDE problem
where the multiscale operator Aϵ,δ is defined by (3.2).
We are going to derive a solution of the form
as an approximate solution of the PDE problem (6.2). Substituting the series expansion (6.3) into the PDE problem (6.2) and using the same methodology as used for Proposition 3.1, one can have the following PDE problems for the terms Uij(t,x,y,z), (i,j)∈{(0,0),(0,1),(1,0)}:
where AH11(z), AH12(z), BH21(z), and BH22(z) are given by (3.5).
The following lemma is useful to solve the PDE problems for the terms U01 and U10.
Lemma 6.1. Consider the PDE problems
If ξ satisfies the equation ABSξ(t,x,z)=0, then the solution u(t,x,z) can be decomposed into
where u1 and u2 are solutions to the PDE problems given by
respectively. Furthermore, the solutions u1 and u2 are given by
respectively.
Proof. This lemma is related to the Black-Scholes framework with volatility ˉσf. Refer to Section 6.2 in Fouque et al. [15] for a proof. □
Based on Lemma 6.1, we obtain the following semi-closed form formula for an approximate value of Uϵ,δ(t,x,y,z).
Proposition 6.1. Under the dynamics of (2.3), the option price Uϵ,δ(t,x,y,z) is approximated by ¨Uϵ,δ:=UBS+√ϵU01+√δU10, that is
where UBS(t,x,z) is defined by
and Hϵ,H101 and Hδ,H210 are differential operators defined by
respectively. Here, PBS(t,x,z;ϖ) is the Black-Scholes call option price given by
where di(s,z;ϖ) (i=1,2) are defined in (5.1). The group parameters Aϵ,H11(z), Aϵ,H12(z), Bδ,H21(z), and Bδ,H22(z) are defined in Proposition 3.1.
Proof. First of all, since U00 satisfies a PDE problem for a D/O barrier option under the Black-Scholes model with volatility ˉσf as seen in (6.4), it becomes U BS defined in the proposition.
On the other hand, from (6.4), we have the following PDE problems for ¨U01:=√ϵU01 and ¨U10:=√δU10:
respectively. Applying Lemma 6.1 to (6.7) directly, one can obtain solutions for ¨U01 and ¨U10 which lead to ¨Uϵ,δ(t,x,z) given in the proposition when they are added to U00. □
6.2. Lookback option
A lookback option is an exotic option that allows the holder to exercise an option at the most favorable (minimum or maximum) price of the underlying asset over the life of the option. The floating strike lookback option eliminates the risk associated with the market entry time. In this section, we obtain a pricing formula for the floating strike lookback call option under the model (2.3). In terms of a stochastic process defined by
(the minimum value from the contract time 0 until the current time t), we let Vϵ,δ(t,m,x,y,z) denote the price of the lookback call option, where the payoff function H(x,m) is given by
From the no-arbitrage theory with the self-financing condition and the Itˆo formula, Vϵ,δ(t,m,x,y,z) satisfies the PDE problem
We solve the PDE problem (6.9) for Vϵ,δ(t,x,m,y,z) using the asymptotic series expansion
Plugging (6.10) into the PDE in (6.9) and applying the same methodology as used for Proposition 3.1 yield that Vij(t,x,m,y,z), (i,j)∈{(0,0),(0,1),(1,0)}, satisfy the PDE problems
respectively.
The following lemma is useful to solve the PDE problems for the terms V01 and V10.
Lemma 6.2. Consider a PDE problem given by
Then the solution v(t,x,z) is given by
where G(τ,w,x,z) is given by
Here, the function φ is defined in (5.2).
Proof. As the PDE in (6.12) is a non-homogeneous linear PDE with a Neumann boundary condition, by the change of variables, it can become a non-homogeneous heat equation whose solution is well-known. Refer to Polyanin and Nazaiknskii [27] for details. □
Based on Lemma 6.2, we obtain the following formula for an approximate value of Vϵ,δ(t,x,m,z).
Proposition 6.2. Under the dynamics of (2.3), the option price Vϵ,δ(t,x,m,z) is approximated by ¨Vϵ,δ:=VBS+√ϵV01+√δV10, that is
where VBS(t,x,m,z) is defined as
Hϵ,H101 and Hδ,H210 are the differential operators defined by (6.5), and G is the function defined in Lemma 6.2. Here, PBS(t,x,z;ϖ), φ(ϖ,μ,σ), and d1(x,z;ϖ) are defined in (6.6) and (5.2), respectively.
Proof. Above all, as the solution V00 of the first PDE problem in (6.11) is exactly the price of the floating strike lookback call option under the Black-Scholes model whose volatility is ˉσf(z), it is the same as V BS given by (6.14) which can be found in, for instance, Wilmott [34].
To derive approximate closed-form formulas for Vϵ,δ, we let ¨V01:=√ϵV01 and ¨V10:=√δV10, and apply the reduction method of the dimension used in Shreve [31] to the PDE problems in (6.11). Letting w:=xm and ¨Vij(t,x,m,z)=m¨Vij(t,xm,1,z)=:mWij(t,w,z), (i,j)={(0,1),(1,0)}, (6.11) becomes
where
Applying Lemma 6.2 to the PDE problems (6.15) for W10 and W01 directly, one can obtain the solutions corresponding to the formula (6.13). □
7.
Conclusions
In this paper, we have introduced a semimartingale approximation of fractional stochastic volatility in terms of two approximate fractional Brownian motions corresponding to two characteristic time scales. Based on the semimartingale property, we make use of the replicating portfolio method to obtain the parabolic PDE problems for European vanilla, barrier, and lookback options, and then solve those problems explicitly and derive approximate closed-form formulas for the option prices. The mixture of the Hurst parameters and the multiple time scales of volatility can unify effectively the previously known separate results about the time-to-maturity dependence of the blow-up or flattening behavior of the skews of the implied volatility. So, knowing that stochastic volatility models driven by fractional Brownian motions can generate better fits to implied volatility surfaces, our uniform approximation result can contribute to the situation that the volatility parameters including the Hurst exponent should be calibrated over a wide range of time-to-maturities.
Author contributions
Min-Ku Lee: Resources, software, data curation, methodology, validation, visualization, and writing-original draft; Jeong-Hoon Kim: Conceptualization, formal analysis, writing-original draft, writing-review & editing, supervision, project administration, and funding acquisition. All authors have read and agreed to the published version of the manuscript.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Data and code availability
Datasets analyzed during the current study are available through the sites http://www.investing.com and http://www.barchart.com. Code for parameter calibration is provided as part of the replication package and is available at https://sites.google.com/view/two-scale-sv-fbms.
Acknowledgments
The authors deeply thank the four anonymous reviewers for their valuable comments and suggestions to improve this paper.
The author J.-H. Kim gratefully acknowledges the financial support from the National Research Foundation of Korea grant NRF2021R1A2C1004080.
Conflict of interest
The authors certify that there is no actual or potential conflict of interest in relation to this article.
Appendix
A. Second-order approximation
In this section, we obtain the second-order terms P02, P11, and P20 in the asymptotic series expansion (3.3). The corresponding PDE problems have the terminal conditions ⟨P02(T,x,⋅,z)⟩=0, P11(T,x,y,z)=0, and P20(T,x,y,z)=0, respectively. Here, the averaged terminal condition ⟨P02(T,x,⋅,z)⟩=0 and the condition
are imposed based on the terminal layer analysis given by Fouque et al. [13].
We first obtain the following lemma for the terms P02, P12, and P03 in (3.3).
Lemma A.1. The second-order terms P02, P12, and P03 in (3.3) can be expressed as
for some functions F02, F12, and F03 independent of the variable y, where η, ξ, and ζ are the solutions of
respectively.
Proof. Putting (3.3) into (3.1), Lemmas 3.1 and 3.2 draw forth Poisson equations given by
Using the solutions η, ξ, and ζ of (A.3), we can derive the solutions P02, P12, and P30 in the form of (A.2) for some y-independent functions F02, F12, and F03. □
Next, we obtain PDEs for F02 (and so P02), P11, and P20.
Proposition A.1. The second-order solutions F02 in (A.2), P11, and P20 are independent on the variable y and satisfy the following PDEs
with the boundary conditions F02(T,x,z)=P11(T,x,z)=P20(T,x,z)=0.
Proof. First of all, similarly with the proof of Lemma A.1, we can obtain the PDEs given by
which yields that P11, P20, and P21 are independent on the variable y. Additionally, we can get the PDE given by
Applying Lemma 3.1 and the y-independence of P11, P20, and P21 into (A.6), we can have the PDEs
and
Thus, we can obtain the PDEs for P11 and P02 in (A.5).
On the other hand, putting P02 in (A.2) into (A.7), we can obtain
where the assumption (A.1) has been used. Thus the first equation for F02 in (A.5) has been derived.
□
Finally, the second-order approximation ⃛P(t,x,y,z):=P BS+√δP10+√ϵP01+δP02+√δϵP11+ϵP20 and its accuracy are obtained as follows by solving the PDEs (A.5) in Proposition A.1.
Proposition A.2. Under the dynamics of (2.3) of the underlying asset price, the option price Pϵ,δ is approximated by ⃛P(t,x,y,z):=PBS+√δP10+√ϵP01+δP02+√δϵP11+ϵP20, where the first approximation part ¨P(t,x,y,z):=PBS+√δP10+√ϵP01 is given by Proposition 3.1 and P02, P11, and P20 are given by
where Akij, Bkij, Ckij, and Dkij are set aside in Appendix B for comfortable readability. Moreover, the approximation has the accuracy
for any l<1.
Proof. First of all, we can rewrite the solutions P01 and P10 in Proposition 3.1 as
where B and C are the operators defined by
respectively. Putting the solutions (A.10) for P01 and P10 and (A.2) for P02, P12, and P03 into (A.5), we have the following PDEs
where G, H, M, and N are the differential operators defined by
Here, DH1,H2i, DH1i, DH2i, and EH2i are defined in Appendix B. By applying the following results (motivational equations) in Fouque et al. [13] to the PDEs (A.11), we solve the PDEs (A.11) to obtain the solutions (A.9).
The proof of the error estimate should be similar to the proof in Fouque et al. [13] and so we omit the proof. □
B. The constants in the solutions F02, P11, and P20









 DownLoad:
DownLoad:





