On an extension of KU-algebras

1.   Introduction

Prabpayak and Leerawat introduced KU-algebras in ^[9], basic properties of KU-algebras and its ideals are discussed in ^[9,10]. After that many authors widely studied KU-algebras in different directions e.g. in fuzzy, in neutrosophic and in intuitionistic context ^[17], soft and rough sense etc. Naveed et al. ^[15] introduced the concept of cubic KU-ideals of KU-algebras whereas Mostafa et al. ^[7] defined fuzzy ideals of KU-algebras. Further Mostafa et al. ^[8] studied Interval valued fuzzy KU-ideals in KU-algebras. Recently Moin and Ali introduced roughness in KU-algebras ^[1]. Ali et al. ^[4] introduced pseudo-metric on KU-algebras. Senapati and Shum ^[16] defined Atanassovs intuitionistic fuzzy bi-normed KU-ideals of a KU-algebra. The study on n-ary block codes on KU-algebras are discussed in ^[3]. Moreover, $(\alpha, \beta)$ soft sets are explored on KU-algebras in ^[2].

Imai and Iseki ^[14] introduced two classes of abstract algebras namely BCK/BCI algebras as an extension of the concept of set-theoretic difference and proportional calculi. Then onwards many works been done based on this logical algebras. Subrahmanya defined and shown results based on Commutative extended BCK-algebra. Farag and Babiker ^[5] studied Quasi-ideals and Extensions of BCK-algebras.

Extensions of different algebraic structures whether in classical or logical algebras are intensively studied by many researchers in recent years. Motivated by works based on extension, we have studied an extension of KU-algebras. Some recent work based on extension and generalization of logical algebras can be seen in ^[11,12,13].

In this article, definitions, examples and basic properties of KU-algebras are given in Section 2. In section 3, extended KU-algebras are defined with examples and related results. In section 4, ideals of extended KU-algebras are studied and section 5 concludes the whole work.

2.   Preliminaries

In this section, we shall give definitions and related terminologies on KU-algebras, KU-subalgebras, KU-ideals with examples and some results based on them.

Definition 1. ^[9] By a KU-algebra we mean an algebra $(X, \circ, 1)$ of type $(2, 0)$ with a single binary operation $\circ$ that satisfies the following propoerties: for any $x, y, z\in X,$

${\rm(ku1)} \; (x\circ y)\circ \lbrack (y\circ z)\circ (x\circ z)] = 1,$

${\rm(ku2)} \; x\circ 1 = 1,$

${\rm(ku3)} \; 1\circ x = x,$

${\rm(ku4)} \; x\circ y = y\circ x = 1$ implies $x = y.$

In what follows, let $(X, \circ, 1)$ denote a KU-algebra unless otherwise specified. For brevity we also call $X$ a KU-algebra. The element $1$ of $X$ is called constant which is the fixed element of $X.$ Partial order “$\leq$” in $X$ is denoted by the condition $x\leq y$ if and only if $y\circ x = 1.$

Lemma 1. ^[9] $(X, \circ, 1)$ is a KU-algebra if and only if it satisfies:

${\rm (ku5)} \; x\circ y\leq (y\circ z)\circ (x\circ z),$

${\rm (ku6)} \; x\leq 1,$

${\rm (ku7)} \; x\leq y, \; y\leq x$ implies $x = y,$

Lemma 2. In a KU-algebra, the following properties are true:

${\rm (1)} \; \ z\circ z = 1,$

${\rm (2)} \; \ z\circ (x\circ z) = 1,$

${\rm (3)} \; \ z\circ (y\circ x) = y\circ (z\circ x),$ for all $x, y, z\in X,$

${\rm (4)} \; \ y\circ \lbrack (y\circ x)\circ x] = 1.$

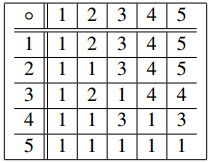
Example 1. ^[7] Let $X = \{1, 2, 3, 4, 5\}$ in which $\circ$ is defined by the following table

It is easy to see that $X$ is a KU-algebra.

Definition 2. A non-empty subset $K$ of a KU-algebra $X$ is called a KU-ideal of $X$ if it satisfies the following conditions:

${\rm(1)} \; 1\in K,$

${\rm(2)} \; x\in K$ and $x\circ y\in K$ implies $y\in K,$ for all $x, y\in X.$

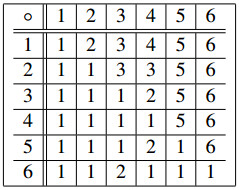
Example 2. ^[1] Let $X = \{1, 2, 3, 4, 5, 6\}$ in which $\circ$ is defined by the following table:

Clearly $(X, \circ, 1)$ is a KU-algebra. It is easy to show that $K_1 = \{1, 2\}$ and $K_2 = \{1, 2, 3, 4, 5\}$ are KU-ideals of $X.$

3.   Extended KU-Algebras

In this section, we give a definition of an extension of KU-algebras and related results. In the whole text by ${\rm(kue)}$ we mean an extended KU-algebras as defined below.

Definition 3. For a non-empty set $X,$ we define an extended KU-algebra corresponding to a non-empty subset $K$ of $X$ as an algebra $(X_K; \circ, K)$ such that $\circ$ is a binary operation on $X_K$ satisfies the following axioms:

${\rm(kue1)} \; (x\circ y)\circ \left[(y\circ z)\circ (x\circ z)\right]\in K,$

${\rm(kue2)} \; x\circ K = \{x\circ k:\; k\in K\}\subseteq K,$

${\rm(kue3)} \; K\circ x = \{k\circ x:\; k\in K\} = \{x\},$

${\rm(kue4)} \; x\circ y\in K$ and $y\circ x\in K$ implies $x = y$ or $x, y\in K$ for any $x, y, z\in X$.

For simplicity we will denote simply $X_K$ as an extended KU-algebra $(X_K, \circ, K)$ in the later text.

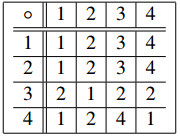
Example 3. Let $X = \{1, 2, 3, 4\}$ and $K = \{1, 2\}.$ Then we can see in the following table that $X_K$ is an extended KU-algebra.

Example 4. Let $X = \{1, 2, 3, 4, 5\}$ and $K = \{1, 2\}.$ Then we can see in the following table that $X_K$ is an extended KU-algebra.

Now we have the following properties and basic results of an extended KU-algebra $X_K$.

Theorem 1. Every KU algebra is an extended KU-algebra and converse holds if and only if $K$ is a singleton set.

Proof. Clearly, any KU-algebra $(X, \circ, 1)$ is an extended KU-algebra $X_K$ by considering $K = \{1\}.$

If $X_K$ is an extended KU-algebra with $K = \{k\},$ then $(X_K, \circ, 1: = k)$ is a KU-algebra.

Conversely, we suppose that an extended KU-algebra $X_K$ is a KU-algebra. Take $k_1, k_2\in K,$ then by (kue3) $k_1\circ k_1 = k_1$ and $k_2\circ k_2 = k_2.$ Also, by considering $X_K$ as a KU-algebra, we get that $k_1\circ k_1 = k_2\circ k_2 = 1$ using Lemma 2(1). We conclude that $k_1 = k_2 = 1$ and hence $K = \{1\}.$

Lemma 3. Each extended KU-algebra $X_K,$ satisfies the following properties for all $x, y, z\in X:$

${\rm (i)} \; \ z\circ z\in K,$

${\rm (ii)} \; \ z\circ (x\circ z)\in K,$

${\rm (iii)} \; \ y\circ [(y\circ z)\circ z]\in K,$

${\rm (iv)} \; \ z\circ (y\circ x) = y\circ (z\circ x),$

${\rm (v)} \; \ (z\circ x)\circ [(y\circ z)\circ (y\circ x)]\in K$ for all $x, y, z\in X.$

Proof. ${\rm(i)}$, ${\rm(ii)}$ and ${\rm(iii)}$ directly follow from the Definition 4.

${\rm(iv)}$ Taking $x: = z, \; y: = (z\circ x)\circ x$ and $z: = y\circ x$ in (kue1) we get,

Since $z\circ ((z\circ x)\circ x)\in K$ by part (3) and using (kue3) in above equation we get,

Considering (kue1) with $x: = y, \; y: = z\circ x$ and $z: = x$ we obtain,

Again put $x: = y\circ (z\circ x), \; y: = ((z\circ x)\circ x)\circ (y\circ x)$ and $z: = z\circ (y\circ x)$ in (kue1) we get,

$\left[(y\circ (z\circ x))\circ (((z\circ x)\circ x)\circ (y\circ x))\right]$

$\circ \left[((((z\circ x)\circ x)\circ (y\circ x))\circ (z\circ (y\circ x)))\circ ((y\circ (z\circ x))\circ (z\circ (y\circ x)))\right]\in K.$

Using Eqs (3.1) and (3.2) with (kue3) in above relation we get,

Interchange $y$ and $z$ in Eq (3.3), we get that,

Combining Eqs (3.3) and (3.4) and using (kue4) we obtain,

${\rm(v)}$ It follows from (kue1) and part ${\rm(4)}$.

Definition 4. We define a binary relation $\leq$ on an extended KU-algebra $X_K$ as, $x\leq y$ if and only if either $x = y$ or $y\circ x\in K$ and $y\not \in K.$

Note that if $y\in K$ and $y\circ x\in K$ for any $x\in X,$ then by (kue3) we get, $x = y\circ x\in K$ and $x\circ y = y\in K \Rightarrow x = y.$

Definition 5. A non-empty subset $K$ of a KU-algebra $X$ is called the minimal set in $(X_K, \leq)$ if $x\leq k$ implies $x = k,$ for any $x, y, z\in X$ and $k\in K.$

Lemma 4. An extended KU-algebra $X_K$ with binary relation $\leq$ is a partial ordered set with a minimal set $K.$

Proof. It follows from the definition of $\leq$ and Lemma 3 (i) that $x\leq x$.

Let $x\leq y$ and $y\leq x.$ If $x = y,$ then we are done, otherwise by the definition of $\leq$ we get, $y\circ x\in K$ and $x\circ y\in K$ which implies $x = y$ by (kue4).

Moreover, if $x = y$ or $y = z,$ then $x\leq z$. Otherwise by the definition of $\leq$ we get, $y\circ x\in K$ and $z\circ y\in K.$

Now,

Since $x\leq k\in K,$ therefore it directly follows from the Definition 4 that $x = k$ and hence $K$ is a minimal set.

Taking $(X_K, \leq)$ as a partial ordered set we obtain the following properties:

Theorem 2. Let $X_K$ be an extended KU-algebra with partial order $\leq.$ Then

${\rm (i)} \; x\leq y$ implies $z\circ x\leq z\circ y$ or $z\circ x, z\circ y\in K,$

${\rm (ii)} \; x\leq y$ implies $y\circ z\leq x\circ z$ or $y\circ z, x\circ z\in K,$

${\rm (iii)}$ either $x\circ k\in K$ for all $k\in K$ or $x\circ k_1 = x\circ k_2,$ for all $k_1, k_2\in K,$

${\rm (iv)} \; ((x\circ y)\circ y)\circ y = x\circ y$ or $x\circ y\in K,$

${\rm (v)} \; (y\circ x)\circ k = (y\circ k)\circ (x\circ k)$ or $(y\circ x)\circ k\in K,$

${\rm (vi)} \; x\circ k\in K$ and $y\circ k\in K$ implies $(y\circ x)\circ k\in K$ and $(x\circ y)\circ k\in K,$

${\rm (vii)} \; x\circ (y\circ x)\in K,$

${\rm (viii)}$ if $x, y\not \in K,$ then $(y\circ x)\circ x\leq x$ and $(y\circ x)\circ x\leq y$ for all $x, y, z\in X$ and $k\in K.$

Proof. ${\rm(i)}$ Let $x\leq y.$ If $x = y,$ then the proof is clear. Otherwise $y\circ x\in K$ and then by Lemma 3(v) and (kue3), $(z\circ y)\circ (z\circ x) = (y\circ x)\circ ((z\circ y)\circ (z\circ x))\in K$ implies $z\circ x\leq z\circ y$ if $z\circ y\not\in K$ or if $z\circ y\in K,$ then $(z\circ y)\circ (z\circ x) = z\circ x\in K.$

${\rm(ii)}$ Similar to ${\rm(i)}.$

${\rm(iii)}$ Let $k_1, k_2\in K$ and $x\in X.$ Then by Lemma 3(v) and (kue3), we get $(x\circ k_2)\circ (x\circ k_1) = (k_2\circ k_1)\circ ((x\circ k_2)\circ (x\circ k_1))\in K.$ Similarly $(x\circ k_1)\circ (x\circ k_2) = (k_1\circ k_2)\circ ((x\circ k_1)\circ (x\circ k_2))\in K.$ Now by (kue4), $x\circ k_1; x\circ k_2\in K$ or $x\circ k_1 = x\circ k_2$ for all $k_1, k_2\in K.$

${\rm(iv)}$ Since $(x\circ y)\circ (((x\circ y)\circ y)\circ y) = ((x\circ y)\circ y)\circ ((x\circ y)\circ y)\in K$ by Lemma 3.

Taking (kue1) with $x: = x, \; y: = (x\circ y)\circ y$ and $z: = y$ we get that, $(x\circ ((x\circ y)\circ y))\circ [(((x\circ y)\circ y)\circ y)\circ (x\circ y)]\in K$ and so $((x\circ y)\circ (x\circ y))\circ [(((x\circ y)\circ y)\circ y)\circ (x\circ y)]\in K.$ Hence $(((x\circ y)\circ y)\circ y)\circ (x\circ y)\in K$ by Lemma 3.

Thus by (kue4), either $(((x\circ y)\circ y)\circ y) = x\circ y$ or $x\circ y\in K$ and $(((x\circ y)\circ y)\circ y)\in K.$

${\rm(v)}$ If $x\circ k\not \in K,$ then by Lemma 3(i) and part (iii), we get $x\circ k = x\circ ((y\circ x)\circ (y\circ x)).$ By Lemma 3(iv) and (kue2),

Now by part (iv) either $(y\circ x)\circ k'' = (y\circ x)\circ k$ or $(y\circ x)\circ k\in K$ which implies either $(y\circ k)\circ (x\circ k) = (y\circ x)\circ k$ or $(y\circ x)\circ k\in K.$

${\rm(vi)}$ Let $x\circ k\in K$ and $y\circ k\in K.$ By (kue3), $(y\circ k)\circ (x\circ k)\in K.$ Hence $(y\circ k)\circ (x\circ k) = k_1,$ for some $k_1\in K.$ By (ku1), $(x\circ y)\circ k_1 = (x\circ y)\circ ((y\circ k)\circ (x\circ k))\in K.$

Similarly we can prove that, $(x\circ y)\circ k_2\in K.$ By part (iv), $(x\circ y)\circ K\subseteq K$ and $(y\circ x)\circ K\subseteq K.$ Thus $(y\circ x)\circ k\in K$ and $(x\circ y)\circ k\in K.$

${\rm(vii)}$ and ${\rm(viii)}$ follow from Lemma 3(iv).

Theorem 3. Let $X_{K_1}$ and $X_{K_2}$ be two extended KU-algebras with same operation $\circ.$ Then $K_1 = K_2.$

Proof. Let $x\in K_1.$ Then by (kue3) $x = x\circ x$ but by Lemma 3(i) $x = x\circ x\in K_2$ implies $K_1\subseteq K_2.$ Similarly we can show that $K_2\subseteq K_1.$ Hence $K_1 = K_2.$

Definition 6. A set $(Y; \circ; L)$ is called extended sub-algebra of an extended KU-algebra $X_K$ if $Y\subseteq X, \; L\subseteq K,$ and $Y_L$ is also an extended KU-algebra.

Example 5. From Example 3 if we take $Y = \{1, 2, 3\}$ with $K = \{1, 2\},$ then $Y_K$ is a sub-algebra of $X_K.$

The following result derived from the definition of extended KU-algebras.

Proposition 1. If $(X_i, \circ, K),$ for $i\in \Lambda,$ is a family of extended KU-subalgebras of an extended KU-algebra $(X_K, \circ, K),$ then $\bigcap_{i\in \Lambda} (X_i; \circ, K)$ is also an extended KU-subalgebra.

Theorem 4. Let $X_K$ be an extended KU-algebra. Then $Y_L$ is a sub-algebra of $X_K$ if and only if $x\circ y\in Y,$ for all $x, y\in Y,$ and $L = K\cap Y.$

Proof. Let $Y_L$ be a sub-algebra of an extended KU-algebra $X_K.$ Then clearly $x\circ y\in Y,$ for all $x, y\in Y$ and let $M = K\cap Y.$ Since $M\subseteq K,$ therefore it is easy to see that $Y_M$ is a subalgebra of $X_K.$ By Theorem 3, $M = L = K\cap Y.$ Converse is obvious.

Corollary 1. If $X_L$ is a sub-algebra of $X_K,$ then $L = K.$

4.   Ideals on extended KU-algebras

In this section we will discuss ideals and some properties of ideals related to extended KU-algebras.

Definition 7. A subset $I$ of an extended KU-algebra $X_K$ is called an ideal of $X_K$ if $K\subseteq I$ and $x\in I, \; x\circ y\in I\Rightarrow y\in I.$

Clearly $X_K$ itself and $K$ are trivial ideals of $X_K.$

Example 6. In Example 4 we can see that the subset $I = \{1, 2, 3, 4\}$ is an ideal of the extended KU-algebra $X_K.$

Proposition 2. For any ideal $I$ of extended KU-algebra, $X_K.$ If $x\in I$ and $y\leq x,$ then $y\in I.$

Proof. Proof follows from the Definitions 4 and 7.

Proposition 3. Let $\{I_\lambda :\lambda\in\Lambda\}$ be a family of ideals of $X_K$. Then $\bigcap_{\lambda\in\Lambda} I_\lambda$ is also ideal of $X_K$.

Proof. Since, $K\subseteq I_\lambda,$ for all $\lambda\in\Lambda,$ we have $K\subseteq \bigcap_{\lambda\in\Lambda} I_\lambda.$ Let $x, x\circ y\in \bigcap_{\lambda\in\Lambda} I_\lambda.$ Then $x, x\circ y\in I_\lambda,$ for all $\lambda\in\Lambda.$ Since $I_\lambda$ is an ideal, we have $x\in I_\lambda,$ for all $\lambda\in \Lambda.$ Implies $x\in \bigcap_{\lambda\in\Lambda} I_\lambda.$

Theorem 5. For an extended KU-algebra $(X, \circ, K),$ let $(X', \circ, 1)$ be a KU-algebra, where $X' = (X\setminus K)\cup \{1\}.$ Then for any ideal $I$ of an extended KU-algebra $X_K,$ the set $J = (I\setminus K) \cup \{1\}$ is an ideal of KU-algebra $X'.$

Proof. Clearly $1\in J.$ Let $x\in J$ and $x\circ y\in J$ for $x, y\in X'.$ If $x = 1,$ then $1\circ y = y\in J.$ Also if $x\neq 1$ but $y = 1,$ then $y\in J$ and we are done.

Therefore we suppose that both $x, y\neq 1,$ hence $x\in I\setminus K$ and $y\in X\setminus K.$ If $x\circ y = 1,$ then by Lemma 3(iii) and (ku3) we get $x\circ ((x\circ y)\circ y) = x\circ (1\circ y) = x\circ y\in K$ which is a contradiction, implies $x\circ y\in I\setminus K.$ As $I$ is an ideal of $X_K$ and $x, x\circ y\in I\setminus K$ gives $y\in I\setminus K\subseteq J.$ Hence $J$ is an ideal of $Y.$

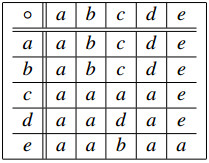
Example 7. Let $X = \{a, b, c, d, e\}$ and $K = \{a, b\}.$ By the following table, $X_K$ is an extended KU-algebra.

Take $X' = \{1, c, d, e\}$ with the following table.

which is a KU-algebra. We can see that $I = \{a, b, c, d\}$ is an ideal of $X_K$ and $J = (I\setminus K)\cup \{1\} = \{1, c, d\}$ is an ideal of $X'.$

Definition 8. We call a map $f:(X, \circ_1, K)\rightarrow (Y, \circ_2, L)$ between two extended KU-algebras an isomorphism if $f$ is bijective and $f(x_1\circ_1 y_1) = f(x_1)\circ_2 f(x_2),$ for all $x_1, x_2\in X.$

If $f$ is an isomorphism, then we say that $X_K$ is isomorphic to $Y_L$ and write it as, $X_K \simeq Y_L.$

Theorem 6. Let $f:(X, \circ_1, K)\rightarrow (Y, \circ_2, L)$ be an isomorphism between two extended KU-algebras. Then $f(K) = L.$

Proof. By Definition 8, the $(f(X) = Y, \circ_1, f(K))$ is an extended KU-algebra and hence by Theorem 3 we get, $f(K) = L$.

Theorem 7. Let $f:(X, \circ_1, K)\rightarrow (Y, \circ_2, L)$ be an isomorphism and $I$ be an ideal of $X_K = (X, \circ_1, K).$ Then $J = f(I)$ is also an ideal of $Y_L = (Y, \circ_2, L).$

Proof. Since $f$ is a bijective function and $I$ is an ideal of $X_K$, therefore $K\subseteq I$ and hence $f(K)\subseteq f(I).$ By Theorem 6, $f(K) = L\subseteq J = f(I),$ the rest follows by the fact that $f$ is an isomorphism.

5.   Conclusions

In this paper, an extension for KU-algebras is given as extended KU algebras $X_{K}$ depending on a non-empty subset $K$ of $X.$ We see that every KU-algebra is an extended KU-algebra and extended KU-algebras $X_{K}$ is a KU-algebra $X$ if and only if $K$ is a singleton set. Several properties including extended KU-algebras were explored. We also discuss ideals and isomorphisms related properties on extended KU-algebras.

As a future work one can consider such extensions on other logical algebras. Moreover, several identities such as fuzzification, roughness, codes, soft sets and other related work can be seen on extended KU-algebras.

Acknowledgments

The authors are thankful to the anonymous referees for their valuable comments and suggestions which improved the final version of this article.

Conflict of interest

The authors declare no conflict of interest.