Processing math: 32%
Search Advanced

AIMS Mathematics

2020, Volume 5, Issue 4: 2813-2842. doi: 10.3934/math.2020181
Previous Article Next Article
Research article Special Issues

A mathematical model of tuberculosis (TB) transmission with children and adults groups: A fractional model

  • 1.
    Department of Mathematics, Faculty of Science and Technology, Universitas Airlangga Surabaya 60115, Indonesia
  • 2.
    Faculty of Natural and Agricultural Sciences, University of the Free State, South Africa
  • 3.
    Department of Mathematics Education, University of Education Winneba, Kumasi Campus, Kumasi, Ghana
  • 4.
    Departement de Mathématiques, Faculté des Sciences et Techniques Errachidia, Université Moulay Ismail, Morocco
  • Received: 01 December 2019 Accepted: 19 February 2020 Published: 17 March 2020

  • MSC : 34A34

  • We consider a novel fractional model to investigates the (tuberculosis) TB model dynamics with two age groups of human, that is, the children and the adults. First, we formulate the model and present the basic results associated to the model. Then, using the fractional operators, Caputo and the Atangana-Baleanu and obtain a generalized model. Further, we give a novel numerical approach for the solution of the fractional model and obtain their approximate solution. We show graphical results with various values of the fractional order. A comparison of the two operators are shown graphically. The results obtained through Atangana-Baleanu operator is flexible than that of Caputo derivative. The infection in tuberculosis (TB) infected people decreases fast when decreasing the fractional order.

    Citation: Fatmawati, Muhammad Altaf Khan, Ebenezer Bonyah, Zakia Hammouch, Endrik Mifta Shaiful. A mathematical model of tuberculosis (TB) transmission with children and adults groups: A fractional model[J]. AIMS Mathematics, 2020, 5(4): 2813-2842. doi: 10.3934/math.2020181

    Related Papers:

    [1] Mashail M. Al Sobhi . The modified Kies-Fréchet distribution: Properties, inference and application. AIMS Mathematics, 2021, 6(5): 4691-4714. doi: 10.3934/math.2021276
    [2] M. G. M. Ghazal, Yusra A. Tashkandy, Oluwafemi Samson Balogun, M. E. Bakr . Exponentiated extended extreme value distribution: Properties, estimation, and applications in applied fields. AIMS Mathematics, 2024, 9(7): 17634-17656. doi: 10.3934/math.2024857
    [3] Dawlah Alsulami . A new extension of the Rayleigh distribution: Properties, different methods of estimation, and an application to medical data. AIMS Mathematics, 2025, 10(4): 7636-7663. doi: 10.3934/math.2025350
    [4] Ahmed Sedky Eldeeb, Muhammad Ahsan-ul-Haq, Mohamed S. Eliwa . A discrete Ramos-Louzada distribution for asymmetric and over-dispersed data with leptokurtic-shaped: Properties and various estimation techniques with inference. AIMS Mathematics, 2022, 7(2): 1726-1741. doi: 10.3934/math.2022099
    [5] Fiaz Ahmad Bhatti, Azeem Ali, G. G. Hamedani, Mustafa Ç. Korkmaz, Munir Ahmad . The unit generalized log Burr XII distribution: properties and application. AIMS Mathematics, 2021, 6(9): 10222-10252. doi: 10.3934/math.2021592
    [6] Naif Alotaibi, A. S. Al-Moisheer, Ibrahim Elbatal, Salem A. Alyami, Ahmed M. Gemeay, Ehab M. Almetwally . Bivariate step-stress accelerated life test for a new three-parameter model under progressive censored schemes with application in medical. AIMS Mathematics, 2024, 9(2): 3521-3558. doi: 10.3934/math.2024173
    [7] Khaled M. Alqahtani, Mahmoud El-Morshedy, Hend S. Shahen, Mohamed S. Eliwa . A discrete extension of the Burr-Hatke distribution: Generalized hypergeometric functions, different inference techniques, simulation ranking with modeling and analysis of sustainable count data. AIMS Mathematics, 2024, 9(4): 9394-9418. doi: 10.3934/math.2024458
    [8] Ayed. R. A. Alanzi, Muhammad Imran, M. H. Tahir, Christophe Chesneau, Farrukh Jamal, Saima Shakoor, Waqas Sami . Simulation analysis, properties and applications on a new Burr XII model based on the Bell-X functionalities. AIMS Mathematics, 2023, 8(3): 6970-7004. doi: 10.3934/math.2023352
    [9] Nuran M. Hassan, M. Nagy, Subhankar Dutta . Statistical inference for the bathtub-shaped distribution using balanced and unbalanced sampling techniques. AIMS Mathematics, 2024, 9(9): 25049-25069. doi: 10.3934/math.20241221
    [10] SidAhmed Benchiha, Amer Ibrahim Al-Omari, Naif Alotaibi, Mansour Shrahili . Weighted generalized Quasi Lindley distribution: Different methods of estimation, applications for Covid-19 and engineering data. AIMS Mathematics, 2021, 6(11): 11850-11878. doi: 10.3934/math.2021688
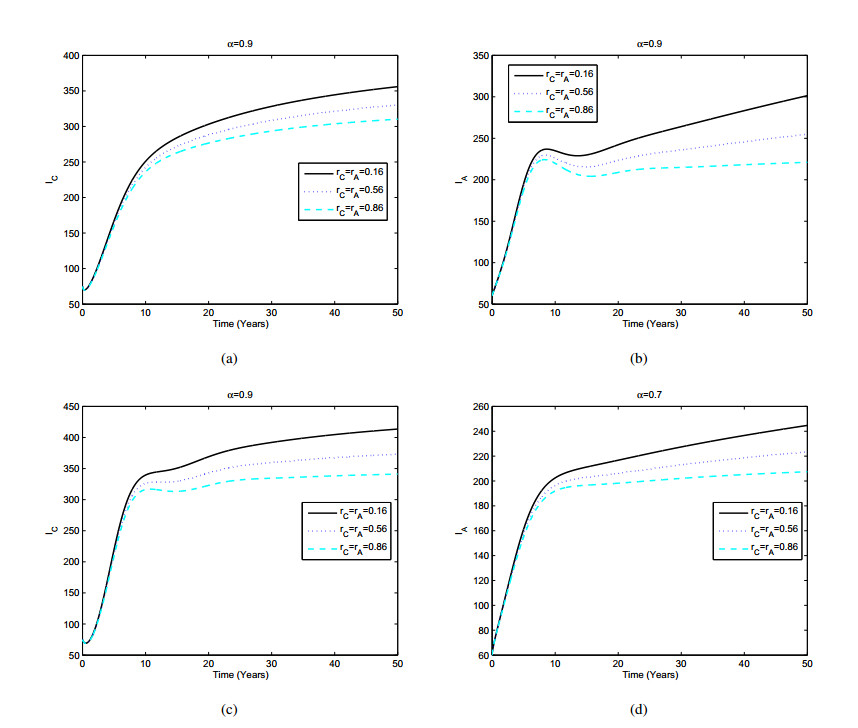
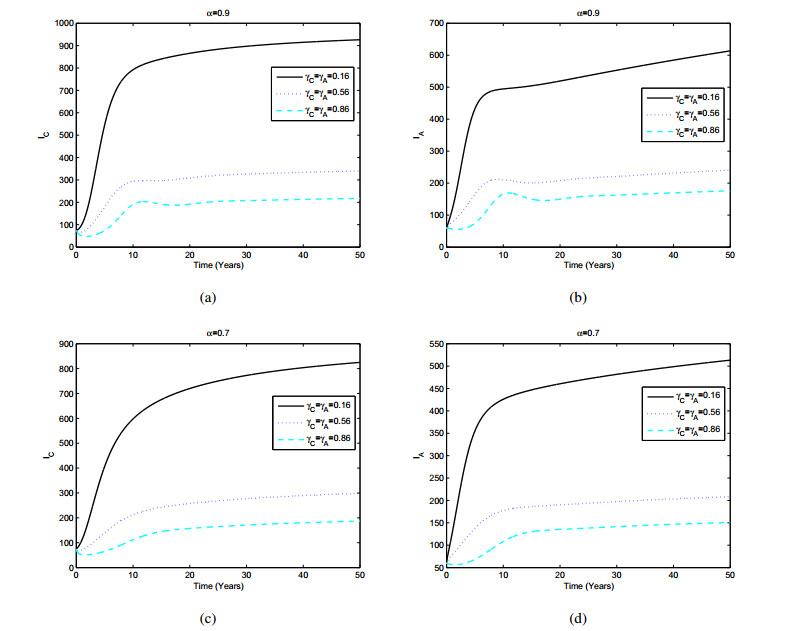
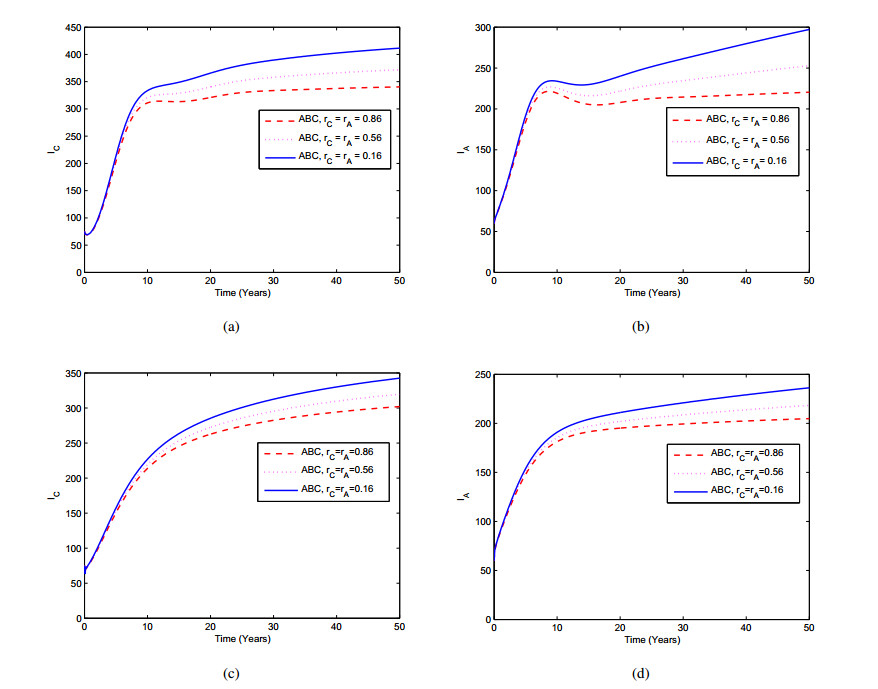
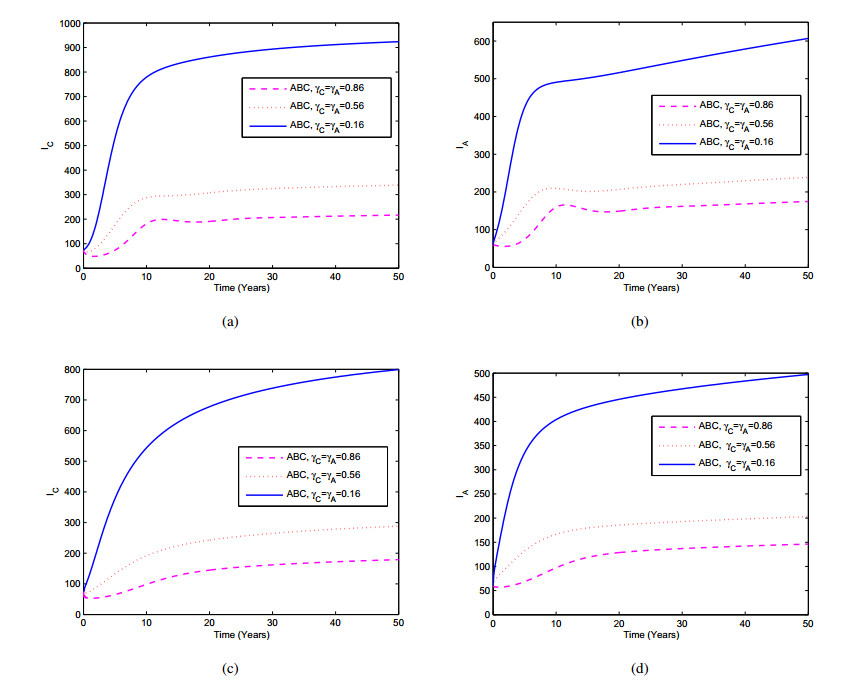
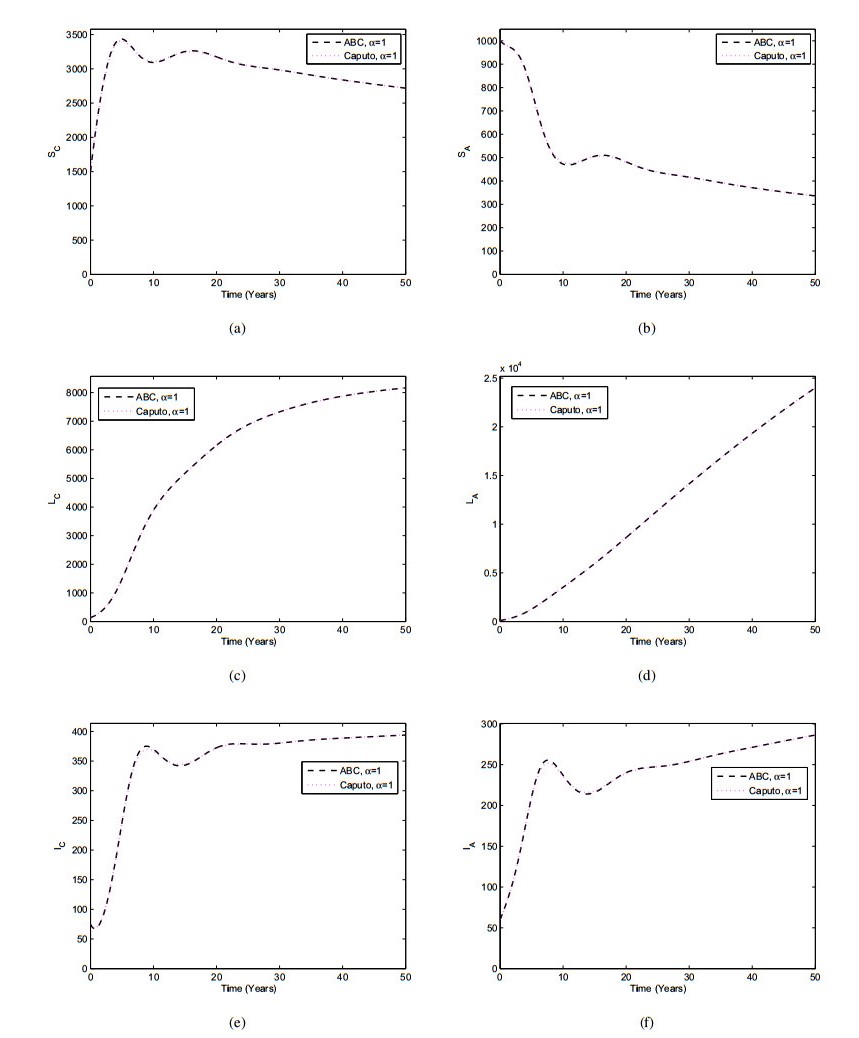
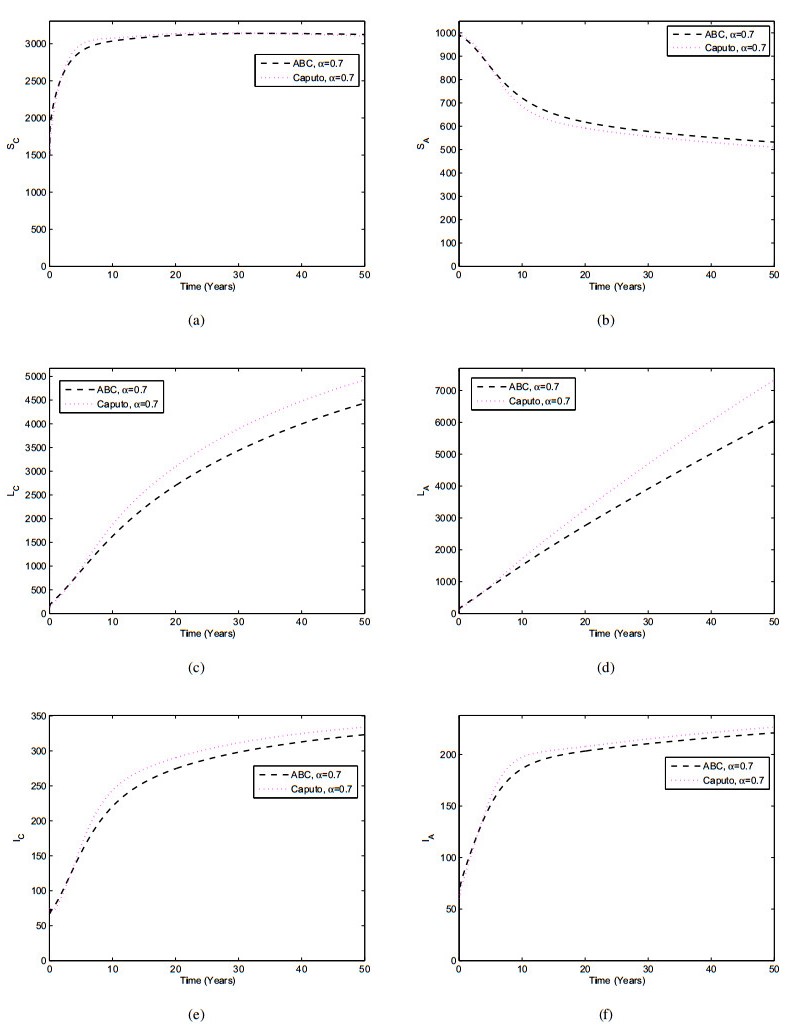
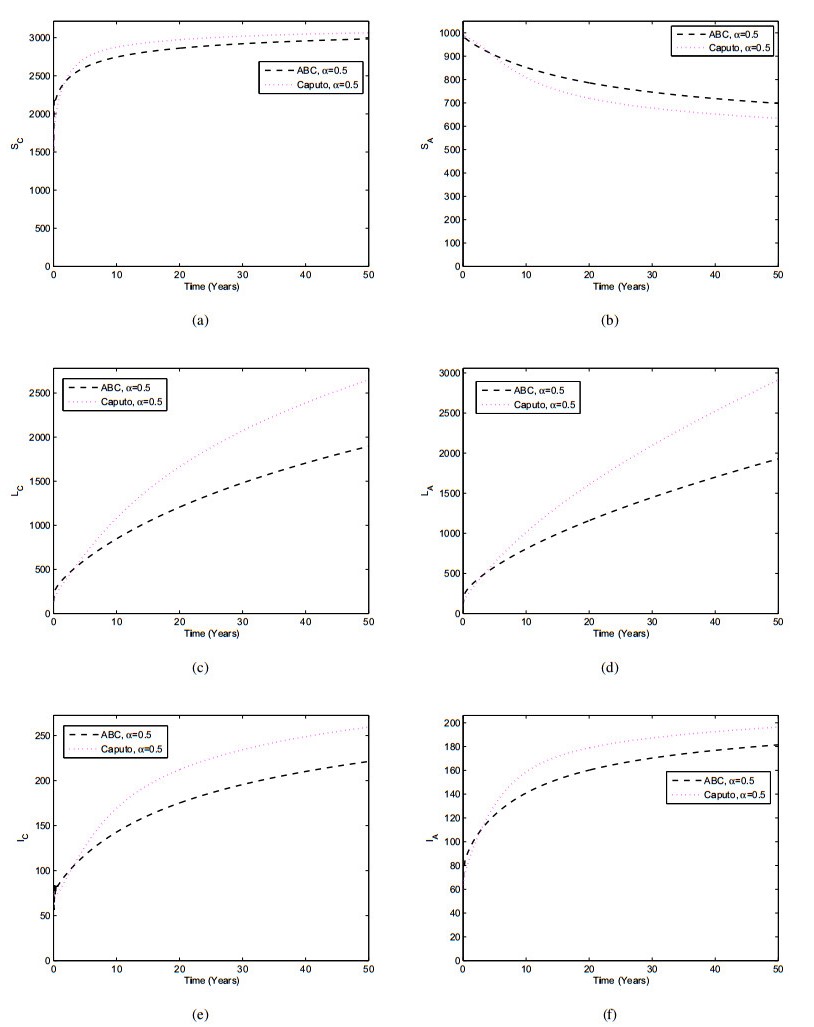
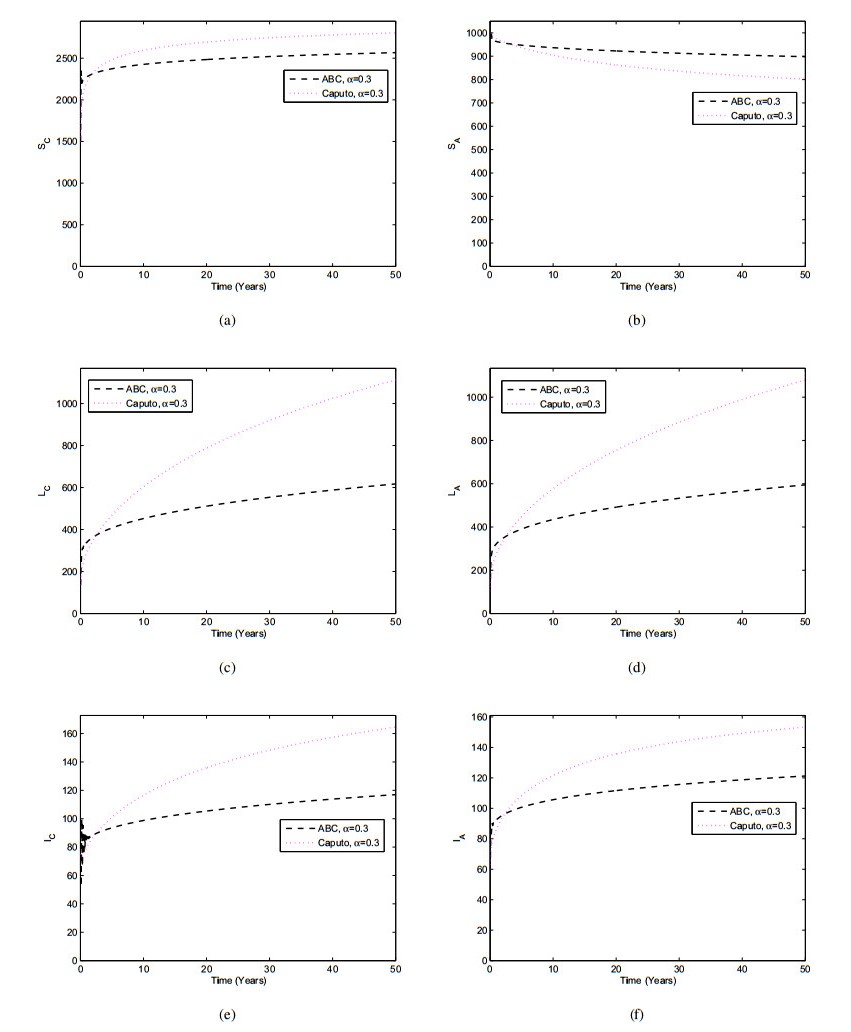
  • We consider a novel fractional model to investigates the (tuberculosis) TB model dynamics with two age groups of human, that is, the children and the adults. First, we formulate the model and present the basic results associated to the model. Then, using the fractional operators, Caputo and the Atangana-Baleanu and obtain a generalized model. Further, we give a novel numerical approach for the solution of the fractional model and obtain their approximate solution. We show graphical results with various values of the fractional order. A comparison of the two operators are shown graphically. The results obtained through Atangana-Baleanu operator is flexible than that of Caputo derivative. The infection in tuberculosis (TB) infected people decreases fast when decreasing the fractional order.


    1.   Introduction

    Data analysis has been received a great interest in several applied fields such as medicine, reliability analysis, engineering, environmental studies, and economics. Several authors have proposed more flexible statistical distributions to model and predict various experimental and phenomenal data encountered in applied fields.

    The Fréchet (Fr) distribution is also known as the inverse-Weibull distribution and it is one of the useful distributions in extreme value theory. The Fr model has some important applications in life testing, floods, earthquakes, and wind speeds, among others. Further information about the applications of the Fr distribution can be explored in [1,2,3,4,5].

    The Fr distribution is specified by the following cumulative distribution function (CDF)

    F(x;θ,λ)=e−λx−θ,θ,λ>0,x>0. (1.1)

    Its probability density function (PDF) reduces to

    f(x;θ,λ)=θλx−(θ+1)e−λx−θ,θ,λ>0,x>0, (1.2)

    where θ and λ are, respectively, the shape and scale parameters. The PDF (1.2) exhibits a unimodal shape or a decreasing shape depending on θ while its hazard rate function (HRF) is always unimodal.

    There is a clear need to define and develop more flexible versions of the Fr model using the well-known families to model several datasets encountered in many applied fields such as medicine, geology, engineering, and economics, among others. Hence, many authors have proposed several generalized forms of the Fr distribution to improve its flexibility and capability in modeling real-life data. Some notable extensions are the following: the exponentiated-Fr [6], beta-Fr [7], Marshall–Olkin Fr [8], transmuted Marshall–Olkin Fr [9], Weibull–Fr [10], beta exponential-Fr [11], Burr-X Fr [12], odd Lindley–Fr [13], logarithmic-transformed Fr [14], and modified Kies–Fr distributions [15].

    This article introduces a new flexible extension of the Fr distribution called the extended Weibull–Fréchet (EWFr) distribution, which provides more flexibility to model real-life data than other competing distributions. Then, the first motivation to this article is devoted to introducing the EWFr distribution as a new extension of the Fr distribution via the extended Weibull-G (EW-G) family [16]. The useful characteristics of the EWFr distribution can be summarized as follows: The EWFr distribution is a more flexible version for the Fr distribution, and it improves the fitting of real-life data; it produces more flexible kurtosis than the baseline Fr model. The HRF of the EWFr distribution can exhibit an upside-down bathtub shape, an increasing shape, and a decreasing shape. Its density function can exhibit a symmetrical shape, a unimodal shape, an asymmetrical shape, a J shape, and a reversed-J shape. Furthermore, the EWFr distribution can be adopted to model various data in the medicine and engineering sciences. This fact was illustrated by modeling two real datasets from both fields, showing its superiority fit over other competing distributions.

    Another motivation to this article is to show how several classical estimators of the EWFr distribution perform for different parameter combinations and several sample sizes. Hence, the EWFr parameters are estimated using different estimation approaches including: the maximum product of spacings estimators (MPSEs), least-squares estimators (LSEs), the right-tail Anderson-Darling estimators (RADEs), the maximum likelihood estimators (MLEs), the weighted least-squares estimators (WLSEs), the percentiles estimators (PCEs), the Cramér–von Mises estimators (CRVMEs), and the Anderson–Darling estimators (ADEs). Extensive simulation results were introduced to explore the performance of these estimators. Furthermore, these estimators are compared using partial and overall ranks to determine the best method for estimation the parameters of the EWFr distribution.

    The paper is organized in six sections as follows: Section 2 introduces the EWFr distribution and its related functions. The distribution properties are determined in Section 3. Section 4 presents some classical estimators of the EWFr parameters. The simulation results for the classical methods are provided in the same section. Two real-life datasets are fitted using the EWFr distribution in Section 5. Some final remarks are presented in Section 6.

    2.   The EWFr distribution

    The EWFr distribution is constructed based on the EW-G family [16] which is specified, for any baseline CDF G(x;ζ), by the CDF

    F(x;ϑ,φ,ζ)=1−{1+φ[G(x;ζ)1−G(x;ζ)]ϑ}−1φ,ϑ,φ>0,x∈ℜ. (2.1)

    The corresponding PDF of (2.1) takes the form

    f(x;ϑ,φ,ζ)=ϑg(x;ζ)G(x;ζ)ϑ−1[1−G(x;ζ)]ϑ+1{1+φ[G(x;ζ)1−G(x;ζ)]ϑ}−1φ−1. (2.2)

    where g(x;ζ)=dG(x;ζ)/dx refers to the baseline density with parameter vector ζ.

    To this end, by inserting Eq (1.1) in (2.1), the CDF of the EWFr model follows as

    F(x;η)=1−{1+φ(eλx−θ−1)−ϑ}−1φ,ϑ,φ,λ,θ>0,x>0, (2.3)

    where η=(ϑ,φ,λ,θ)⊺. The PDF of the EWFr model reduces to

    f(x;η)=ϑθλx−(θ+1)eλx−θ(eλx−θ−1)−(ϑ+1){1+φ(eλx−θ−1)−ϑ}−(1φ+1), (2.4)

    where ϑ,φ and θ are shape parameters whereas λ is a scale parameter.

    2.1.   Some Useful Functions and Shapes

    The survival function (SF) of the EWFr distribution is given as

    S(x;η)={1+φ(eλx−θ−1)−ϑ}−1φ.

    Long-term SF (LT-SF) is a useful feature in the modeling process, because a portion of the population may no longer be eligible to the event of interest with probability p (see [17,18]).

    The general form of the LT-SF is SLT(x;p,η)=p+(1−p)S(x;η), where S(x;η) denotes the SF of any distribution and p denotes the probability of being cured. Hence, the PDF of the LT-SF can be derived as

    fLT(x;p,η)=−∂∂xSLT(x;p,η)=(1−p)f(x;p,η),p∈(0,1).

    Using Eq (2.4), the PDF of the LT-SF of the EWFr distribution takes the form

    fLT(x;p,η)=ϑθλ(1−p)eλx−θxθ+1(eλx−θ−1)−(ϑ+1){1+φ(eλx−θ−1)−ϑ}−(1φ+1).

    The HRF of the EWFr distribution takes the form

    h(x;η)=ϑθλx−(θ+1)eλx−θ(eλx−θ−1)−(ϑ+1)1+φ(eλx−θ−1)−ϑ.

    Its reversed HRF has the form

    r(x;η)=ϑθλx−(θ+1)eλx−θ(eλx−θ−1)−(ϑ+1){1+φ(eλx−θ−1)−ϑ}−(1φ+1)1−{1+φ(eλx−θ−1)−ϑ}−1φ.

    The odd ratio of the EWFr model is derived as

    O(x;η)=F(x|η)S(x|η)={1+φ(eλx−θ−1)−ϑ}1φ−1.

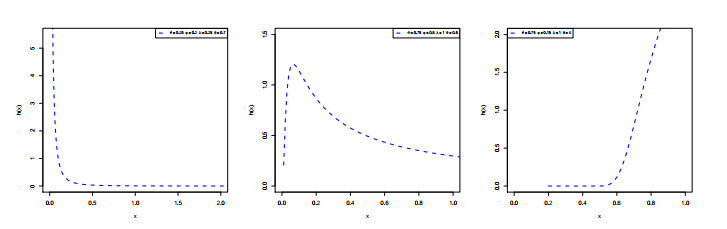
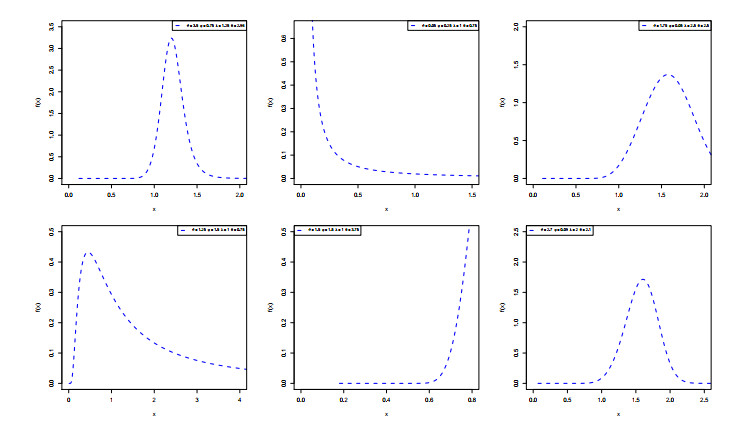
    Figure 1 presents some possible shapes of the EWFr PDF for different values of its parameters. The EWFr PDF can be a symmetrical shape, a unimodal shape, an asymmetrical shape, a J shape, and a reversed-J shape. The hazard rate plots of the EWFr model are depicted in Figure 2. The EWFr HRF can be an increasing shape, a unimodal shape, and a decreasing shape.

    Figure 1.  Possible shapes of the EWFr PDF for several values of ϑ, φ, λ and θ.
    DownLoad: Full-Size Img PowerPoint
    Figure 2.  Possible shapes of the EWFr HRF for several values of ϑ, φ, λ and θ.
    DownLoad: Full-Size Img PowerPoint

    3.   The EWFr characteristics

    3.1.   Quantile function and median

    The quantile function (QF) of the EWFr distribution, say, Q(u), can be calculated by solving F(x)=p in (2.3) in terms of p. Then, the EWFr QF follows as

    Q(p)=λ1θ{ln([(1−p)−φ−1]1ϑφ1ϑ+[(1−p)−φ−1]1ϑ)}1θ,0<p<1. (3.1)

    The median of the EWFr distribution follows by substituting p=0.5 in Eq (3.1).

    3.2.   Linear representation

    A useful linear representation for the PDF of the EWFr model is provided based on [16]. Alizadeh et al. [16] introduced a simple representation for the density of the EW-G class as follows

    f(x)=∞∑w,u=0ψw,uhϑw+u(x), (3.2)

    where ψw,u=−φwΓ(ϑw+u)(−1φ)w/[w!u!Γ(ϑw)] and

    hϑw+u(x)=(ϑw+u)g(x)G(x)ϑw+u−1,

    is the exponentiated-G PDF with a power parameter (ϑw+u)>0. Using Eqs (1.1) and (1.2) of the Fr distribution, Eq (3.2) can be expressed as

    f(x)=∞∑w,u=0ψw,u(ϑw+u)θλx−(θ+1)e−(ϑw+u)λx−θ. (3.3)

    Equation (3.3) can be rewritten as

    f(x)=∞∑w,u=0ψw,ug(ϑw+u)(x), (3.4)

    where g(ϑw+u)(x) denotes the Fr PDF with two-parameter θ and (ϑw+u)λ. Then, the density function of the EWFr model is expressed as a linear representation of Fr densities. Let Y be a random variable having the Fr distribution in (1.1). Hence, the r−th ordinary, μ′r,Y, and incomplete moments, ϕ(r,Y)(t), of Y are, respectively, given (for r<λ, ) by

    μ′r,Y=λrθΓ(1−rθ)andϕ(r,Y)(t)=λrθγ(1−rθ,λt−θ),

    where Γ(s)=∫∞0ws−1e−wdw is the complete gamma function (GF) and γ(s,z)=∫z0ws−1e−wdw is the lower incomplete GF.

    3.3.   Moments and incomplete moments

    This section was devoted to deriving the r−th ordinary moment and incomplete moments of the EWFr distribution.

    Proposition: Based on (3.4), the r−th moment of the EWFr distribution is defined by

    μ′r=∞∑w,u=0ψw,u∫∞0xrgϑw+u(x)dxforr∈N.
    μ′r=∞∑w,u=0ψw,u(ϑw+u)rθΓ(1−rθ). (3.5)

    Setting r=1 in Eq (3.5), we get the mean of x.

    The s−th incomplete moment, say ϕs(x), of the EWFr distribution takes the form

    ϕs(t)=∫t0xsf(x)dx=∞∑w,u=0ψw,u∫t0xsg(ϑw+u)(x)dx.

    Then, we obtain (fors<θ)

    ϕs(t)=∞∑w,u=0ψw,u(ϑw+u)sθγ(1−sθ,(ϑw+u)λt−θ).

    The mean (μ), variance (σ2), skewness (ξ1(X)), and kurtosis (ξ2(X)) of the EWFr distribution are calculated numerically with λ=1 and different values of ϑ, φ and θ. Table 1 displays these numerical results. Table 1 shows that the EWFr model can be right-skewed and it can be leptokurtic (i.e., ξ2(X)>3).

    Table 1.  Some numerical values for μ, σ2, ξ1(X), and ξ2(X) of the EWFr distribution with λ=1 and different values of ϑ, φ and θ.
    η⊺ μ σ2 ξ1(X) ξ2(X)
    (ϑ=1.50,φ=0.50,θ=1.50) 1.3511 0.4752 3.1421 56.2021
    (ϑ=2.50,φ=0.75,θ=1.75) 1.2701 0.1329 1.9395 16.1410
    (ϑ=2.25,φ=1.00,θ=2.75) 1.1904 0.0780 2.2648 19.2907
    (ϑ=2.25,φ=0.25,θ=0.75) 1.6154 0.7600 1.6132 9.0569
    (ϑ=2.25,φ=1.25,θ=3.25) 1.1831 0.0747 2.8068 27.8064
    (ϑ=5.50,φ=1.50,θ=2.50) 1.1927 0.0223 1.7507 11.7806
     | Show Table
    DownLoad: CSV

    3.4.   Order statistics

    Let X1,X2,…Xn be a random sample from the EWFr (2.4) and X1:n≤X2:n≤⋯≤Xn:n be their corresponding order statistics (OS). The PDF and the CDF of the of r−th OS, say, Xr:n and 1≤r≤n are, respectively, defined by

    fr:n(x)=n!(n−r)!(r−1)![F(x)]r−1[1−F(x)]n−rf(x)=n!(n−r)!(r−1)!n−r∑u=0(−1)u(n−ru)[F(x)]r−1+uf(x) (3.6)

    and (for k=1,2,…,n)

    Fr:n(x)=n∑l=k(nl)[F(x)]l[1−F(x)]n−l=n∑l=kn−r∑u=0(−1)u(nl)(n−ru)[F(x)]l+u. (3.7)

    Using Eqs (3.6) and (3.7), the PDF and CDF of the r−th OS of the EWFr reduce to

    fr:n(x)=ϑθλx−(θ+1)n!(r−1)!(n−r)!eλt−θ(eλx−θ−1)−(ϑ+1){1+φ(eλx−θ−1)−ϑ}−(1φ+1)n−r∑u=0(−1)u(n−ru)[1−{1+φ(eλx−θ−1)−ϑ}−1φ]r+u−1

    and

    Fr:n(x)=n∑l=kn−r∑u=0(−1)u(nl)(n−ru)[1−{1+φ(eλx−θ−1)−ϑ}−1φ]l+u.

    4.   Estimation and simulations

    In this section, the EWFr parameters ϑ, λ, φ and θ are estimated using different frequentist approaches. We also provide detailed simulation results to compare and order their performances using partial and overall ranks.

    4.1.   Maximum likelihood estimators

    The MLEs of the parameters ϑ, λ, φ and θ of the EWFr distribution are introduced in this sub-section. Let x1,…,xn be a sample from the EWFr distribution in (2.4). Hence, the log-likelihood function of η=(ϑ,φ,λ,θ)⊺ takes the form

    l(η;x)=nlog(ϑ)+nlog(θ)+nlog(λ)−(θ+1)n∑i=1log(xi)−(ϑ+1)n∑i=1log(eλxi−θ−1)+λn∑i=1xi−θ−(1φ+1)n∑i=1log{1+φ(eλxi−θ−1)−ϑ}.

    The MLEs follow by maximizing the above equation by several programs such as {SAS} ({PROC NLMIXED}) or {R} ({optim} function).

    4.2.   Ordinary and weighted least-squares estimators

    Let x(1), x(2), ⋯, x(n) be the OS of a random sample from the PDF (2.4), then the LSEs [19] of the EWFr parameters are obtained by minimizing the function:

    S(η)=n∑i=1[F(x(i))−in+1]2.

    Similarly, these estimators are also obtained by solving the following equation (for k=1,2,3,4)

    n∑i=1({1+φ(eλxi−θ−1)−ϑ}−1φ−in+1)Ωk(x(i)|η)=0,

    where

    Ω1(x(i)|η)=∂∂ϑF(x(i)|η)=1φ(1+φw−ϑi)−(1φ+1)−φw−ϑilnwi,
    Ω2(x(i)|η)=∂∂φF(x(i)|η)=(1+φw−ϑi)−1φln(1+φw−ϑi),
    Ω3(x(i)|η)=∂∂λF(x(i)|η)=1φ(1+φw−ϑi)−(1φ+1)−ϑφx−θiw−(ϑ+1)ieλxi−θ

    and

    Ω4(x(i)|η)=∂∂θF(x(i)|η)=ϑλw−(ϑ+1)ieλxi−θx−θi(1+φw−ϑi)−(1φ+1)lnxi,

    where wi=eλxi−θ−1. The solution of Ωk for k=1,2,3,4 may be obtained numerically.

    The WLSEs of the EWFr parameters can be determined by minimizing the equation (see [19]):

    W(η)=n∑i=1(n+1)2(n+2)i(n−i+1)[F(x(i)|η)−in+1]2.

    4.3.   Maximum product of spacing and Cramér–von Mises estimators

    The uniform spacings of a random sample from the EWFr distribution are defined (for i=1,2,…,n+1) by {Di(η)=F(x(i)|η)−F(x(i−1)|η)}, where F(x(0)|η)=0, F(x(n+1)|η)=1 and ∑n+1i=1Di(η)=1. The MPSEs of the EWFr parameters can be determined by maximizing the following geometric mean (GM) of spacings

    G(η)=[n+1∏i=1Di(η)]1n+1

    or by maximizing the logarithm of the GM of sample spacings

    H(η)=1n+1n+1∑i=1logDi(η),

    The CRVMEs can be obtained using the difference between the estimated and empirical CDFs. The CRVMEs [20] of the EWFr parameters are determined by minimizing

    C(η)=112n+n∑i=1[F(x(i)|η)−2i−12n]2.

    4.4.   Anderson–Darling and right-tail Anderson–Darling estimators

    The ADEs [21] of the EWFr parameters are calculated by minimizing

    A(η)=−n−1nn∑i=1(2i−1)[logF(x(i)|η)+logS(x(i)|η)].

    The RADEs of the EWFr parameters are calculated by minimizing

    R(η)=n2−2n∑i=1F(xi:n|η)−1nn∑i=1(2i−1)logS(xn+1−i:n|η).

    4.5.   Percentile estimators

    Consider the unbiased estimator of F(x(i)|η) which is defined by ui=i/(n+1). Hence, the PCEs of the EWFr parameters can be calculated by minimizing

    P(η)=n∑i=1(x(i)−{ln([(1−ui)−φ−1]1ϑφ1ϑ+[(1−ui)−φ−1]1ϑ)λ}1θ)2.

    4.6.   Simulation analysis

    To compare and explore the behavior of different estimators of the EWFr parameters, we presented the numerical simulation results and ranked them with respect to their: average of absolute biases (|Bias(ˆη)|), |Bias(ˆη)|= 1N∑Ni=1|ˆη−η|, average of mean relative errors (MREs), MREs=1N∑Ni=1|ˆη−η|/η, and average mean square errors (MSEs), MSEs=1N∑Ni=1(ˆη−η)2.

    The following algorithm can be adopted to explore the behavior of different estimators of the EWFr parameters:

    Step 1: A random sample x1,x2,…,xn of sizes n=20, 80, 200, and 500 are generated from the QF (3.1).

    Step 2: The required results are obtained based on eight combinations of the parameters ϑ={0.25,0.75,1.75,3.50,3.50}, φ={0.50,0.75,2.00,2.50,3.50}, λ={0.25,0.50,1.50,3.00,4.25} and θ={0.25,1.25,2.50}.

    Step 3: Each sample is replicated N=5,000 times.

    Step 4: Results of the biases, MSEs, and MREs are computed for the eight combinations, and to save more space, we present just the result of 5 combinations in Tables 2–6.

    Table 2.  Simulation results for η=(ϑ=1.75,φ=0.5,λ=0.25,θ=1.25)⊺.
    n Est. Est. Par. MLEs LSEs WLSEs CRVMEs MPSEs PCEs ADEs RADEs
    20 |BIAS| ˆϑ 0.58150 {3} 0.59477 {4} 0.53422 {1} 0.54541 {2} 0.60555 {5} 0.71685 {8} 0.67445 {7} 0.61466 {6}
    ˆφ 0.47550 {4} 0.54812 {7} 0.46820 {2} 0.53093 {6} 0.19120 {1} 0.55104 {8} 0.46906 {3} 0.51894 {5}
    ˆλ 0.08930 {2} 0.10351 {7} 0.08402 {1} 0.10057 {5} 0.10077 {6} 0.11204 {8} 0.09758 {3} 0.09866 {4}
    ˆθ 0.49749 {7} 0.47497 {4} 0.46884 {3} 0.48364 {5} 0.45238 {1} 0.53285 {8} 0.46505 {2} 0.48608 {6}
    MSEs ˆϑ 0.47425 {2} 0.52401 {4} 0.52337 {3} 0.46463 {1} 0.54588 {6} 0.64689 {8} 0.59726 {7} 0.53428 {5}
    ˆφ 0.27624 {1} 0.38734 {7} 0.29294 {2} 0.36212 {6} 0.31849 {4} 0.39577 {8} 0.30160 {3} 0.35513 {5}
    ˆλ 0.01107 {2} 0.01423 {7} 0.00983 {1} 0.01356 {5} 0.01357 {6} 0.01651 {8} 0.01289 {3} 0.01296 {4}
    ˆθ 0.37764 {7} 0.33320 {3} 0.35778 {4} 0.36101 {5} 0.29381 {1} 0.38887 {8} 0.32193 {2} 0.36311 {6}
    MREs ˆϑ 0.33229 {3} 0.33987 {4} 0.17807 {1} 0.31166 {2} 0.34603 {5} 0.40963 {8} 0.38540 {7} 0.35123 {6}
    ˆφ 0.95101 {3} 1.09624 {7} 0.93641 {1} 1.06186 {6} 0.97231 {4} 1.10208 {8} 0.93811 {2} 1.03788 {5}
    ˆλ 0.35719 {2} 0.41405 {7} 0.33607 {1} 0.40230 {5} 0.40307 {6} 0.44817 {8} 0.39034 {3} 0.39464 {4}
    ˆθ 0.39799 {7} 0.37998 {4} 0.37507 {3} 0.38691 {5} 0.36190 {1} 0.42628 {8} 0.37204 {2} 0.38886 {6}
    ∑Ranks 43 {2} 65 {7} 23 {1} 53 {5} 46 {4} 96 {8} 44 {3} 62 {6}
    80 |BIAS| ˆϑ 0.65720 {2} 0.73260 {6} 0.64523 {1} 0.71780 {4} 0.67014 {3} 0.78730 {8} 0.72979 {5} 0.78285 {7}
    ˆφ 0.29918 {4} 0.36951 {7} 0.27270 {1} 0.36572 {6} 0.28461 {2} 0.43485 {8} 0.29440 {3} 0.31911 {5}
    ˆλ 0.06946 {2} 0.08089 {7} 0.06755 {1} 0.07955 {6} 0.07162 {4} 0.07099 {3} 0.07301 {5} 0.08347 {8}
    ˆθ 0.41705 {6} 0.41605 {5} 0.41277 {4} 0.43216 {7} 0.34179 {1} 0.38656 {3} 0.37952 {2} 0.45183 {8}
    MSEs ˆϑ 0.53699 {1} 0.65792 {5} 0.71214 {7} 0.63722 {3} 0.62257 {2} 0.71659 {8} 0.64185 {4} 0.70948 {6}
    ˆφ 0.12478 {3} 0.19502 {7} 0.11097 {1} 0.18881 {6} 0.12320 {2} 0.28084 {8} 0.13150 {4} 0.15268 {5}
    ˆλ 0.00672 {1} 0.00884 {7} 0.00693 {2} 0.00853 {6} 0.00712 {4} 0.00711 {3} 0.00740 {5} 0.00932 {8}
    ˆθ 0.26049 {5} 0.25434 {4} 0.30456 {8} 0.27845 {6} 0.16695 {1} 0.24032 {3} 0.20942 {2} 0.29980 {7}
    MREs ˆϑ 0.37554 {2} 0.41863 {6} 0.21508 {1} 0.41017 {4} 0.38294 {3} 0.44989 {8} 0.41702 {5} 0.44734 {7}
    ˆφ 0.59835 {4} 0.73902 {7} 0.54540 {1} 0.73144 {6} 0.56922 {2} 0.86970 {8} 0.58881 {3} 0.63822 {5}
    ˆλ 0.27786 {2} 0.32356 {7} 0.27019 {1} 0.31820 {6} 0.28647 {4} 0.28396 {3} 0.29205 {5} 0.33388 {8}
    ˆθ 0.33364 {6} 0.33284 {5} 0.33022 {4} 0.34573 {7} 0.27343 {1} 0.30924 {3} 0.30361 {2} 0.36147 {8}
    ∑Ranks 38 {3} 73 {7} 32 {2} 67 {6} 29 {1} 66 {5} 45 {4} 82 {8}
    200 |BIAS| ˆϑ 0.54244 {1} 0.74060 {6} 0.66029 {4} 0.72065 {5} 0.56860 {2} 0.78528 {8} 0.64417 {3} 0.74318 {7}
    ˆφ 0.20343 {3} 0.27714 {7} 0.18758 {1} 0.27628 {6} 0.19120 {2} 0.33648 {8} 0.21264 {4} 0.21990 {5}
    ˆλ 0.05329 {1} 0.07033 {7} 0.05985 {3} 0.06898 {6} 0.05669 {2} 0.05992 {4} 0.06059 {5} 0.07617 {8}
    ˆθ 0.30910 {2} 0.38666 {6} 0.37834 {5} 0.39240 {7} 0.26754 {1} 0.34697 {4} 0.32167 {3} 0.42492 {8}
    MSEs ˆϑ 0.39682 {1} 0.65105 {6} 0.72704 {8} 0.62292 {4} 0.51529 {2} 0.70871 {7} 0.53035 {3} 0.65051 {5}
    ˆφ 0.06226 {3} 0.11401 {7} 0.05424 {1} 0.11214 {6} 0.05643 {2} 0.18593 {8} 0.06927 {4} 0.07447 {5}
    ˆλ 0.00405 {1} 0.00665 {7} 0.00571 {5} 0.00644 {6} 0.00452 {2} 0.00513 {4} 0.00507 {3} 0.00753 {8}
    ˆθ 0.14395 {2} 0.20851 {5} 0.26568 {8} 0.22075 {6} 0.10322 {1} 0.19203 {4} 0.14560 {3} 0.25309 {7}
    MREs ˆϑ 0.30997 {2} 0.42320 {6} 0.22010 {1} 0.41180 {5} 0.32491 {3} 0.44873 {8} 0.36810 {4} 0.42468 {7}
    ˆφ 0.40685 {3} 0.55429 {7} 0.37516 {1} 0.55256 {6} 0.38240 {2} 0.67295 {8} 0.42528 {4} 0.43980 {5}
    ˆλ 0.21316 {1} 0.28134 {7} 0.23940 {3} 0.27593 {6} 0.22675 {2} 0.23969 {4} 0.24238 {5} 0.30470 {8}
    ˆθ 0.24728 {2} 0.30933 {6} 0.30267 {5} 0.31392 {7} 0.21403 {1} 0.27758 {4} 0.25734 {3} 0.33994 {8}
    ∑Ranks 22 {1.5} 77 {7} 45 {4} 70 {5} 22 {1.5} 71 {6} 44 {3} 81 {8}
    500 |BIAS| ˆϑ 0.40816 {2} 0.63822 {6} 0.59712 {4} 0.62303 {5} 0.38723 {1} 0.72351 {8} 0.50869 {3} 0.64537 {7}
    ˆφ 0.13392 {3} 0.19775 {6} 0.12778 {1} 0.19966 {7} 0.13208 {2} 0.26446 {8} 0.14927 {4} 0.15660 {5}
    ˆλ 0.03902 {1} 0.05985 {7} 0.04987 {4} 0.05913 {6} 0.04014 {2} 0.05408 {5} 0.04806 {3} 0.06618 {8}
    ˆθ 0.21255 {2} 0.32416 {6} 0.31285 {5} 0.33146 {7} 0.18308 {1} 0.30762 {4} 0.24975 {3} 0.35803 {8}
    MSEs ˆϑ 0.24842 {1} 0.52025 {5} 0.58302 {7} 0.50041 {4} 0.33081 {2} 0.63280 {8} 0.37071 {3} 0.52827 {6}
    ˆφ 0.02762 {3} 0.05937 {6} 0.02573 {1} 0.06052 {7} 0.02727 {2} 0.12045 {8} 0.03399 {4} 0.03724 {5}
    ˆλ 0.00224 {1} 0.00478 {7} 0.00422 {5} 0.00470 {6} 0.00257 {2} 0.00402 {4} 0.00325 {3} 0.00569 {8}
    ˆθ 0.06777 {2} 0.14391 {5} 0.19157 {8} 0.15413 {6} 0.05743 {1} 0.14128 {4} 0.08776 {3} 0.17743 {7}
    MREs ˆϑ 0.23323 {3} 0.36470 {6} 0.19904 {1} 0.35602 {5} 0.22127 {2} 0.41343 {8} 0.29068 {4} 0.36879 {7}
    ˆφ 0.26784 {3} 0.39550 {6} 0.25556 {1} 0.39932 {7} 0.26417 {2} 0.52891 {8} 0.29853 {4} 0.31320 {5}
    ˆλ 0.15609 {1} 0.23939 {7} 0.19949 {4} 0.23651 {6} 0.16056 {2} 0.21632 {5} 0.19222 {3} 0.26474 {8}
    ˆθ 0.17004 {2} 0.25933 {6} 0.25028 {5} 0.26517 {7} 0.14646 {1} 0.24610 {4} 0.19980 {3} 0.28642 {8}
    ∑Ranks 24 {2} 73 {5.5} 46 {4} 73 {5.5} 20 {1} 74 {7} 40 {3} 82 {8}
     | Show Table
    DownLoad: CSV
    Table 3.  Simulation results for η=(ϑ=1.75,φ=2,λ=1.5,θ=2.5)⊺.
    n Est. Est. Par. MLEs LSEs WLSEs CRVMEs MPSEs PCEs ADEs RADEs
    20 |BIAS| ˆϑ 0.58249 {3} 0.53932 {1} 0.56241 {2} 0.61397 {5} 0.60739 {4} 0.82341 {8} 0.68123 {7} 0.66592 {6}
    ˆφ 0.70201 {2} 0.74992 {4} 0.71005 {3} 0.99334 {7} 0.41044 {1} 1.11769 {8} 0.88784 {5} 0.97457 {6}
    ˆλ 0.26246 {1} 0.28717 {3} 0.28400 {2} 0.42881 {5} 0.40138 {4} 0.64545 {8} 0.43589 {7} 0.43050 {6}
    ˆθ 0.83485 {6} 0.91523 {7} 0.92408 {8} 0.76222 {3} 0.77841 {4} 0.78092 {5} 0.74535 {1} 0.74828 {2}
    MSEs ˆϑ 0.45340 {3} 0.42087 {1} 0.44427 {2} 0.51220 {4} 0.51720 {5} 0.77654 {8} 0.58720 {7} 0.56968 {6}
    ˆφ 0.65182 {1} 0.75540 {3} 0.66110 {2} 1.25282 {7} 0.95641 {4} 1.49737 {8} 1.01553 {5} 1.19476 {6}
    ˆλ 0.07059 {1} 0.08751 {3} 0.08543 {2} 0.29909 {7} 0.24742 {4} 0.52940 {8} 0.29252 {6} 0.29154 {5}
    ˆθ 0.84612 {6} 1.00860 {8} 1.00532 {7} 0.71906 {3} 0.75325 {4} 0.82231 {5} 0.69565 {1} 0.70768 {2}
    MREs ˆϑ 0.33285 {3} 0.30818 {1} 0.32138 {2} 0.35084 {5} 0.34708 {4} 0.47052 {8} 0.38927 {7} 0.38052 {6}
    ˆφ 0.35100 {1} 0.37496 {3} 0.35503 {2} 0.49667 {7} 0.42863 {4} 0.55884 {8} 0.44392 {5} 0.48728 {6}
    ˆλ 0.17497 {1} 0.19145 {3} 0.18933 {2} 0.28587 {5} 0.26758 {4} 0.43030 {8} 0.29059 {7} 0.28700 {6}
    ˆθ 0.33394 {6} 0.36609 {7} 0.36963 {8} 0.30489 {3} 0.31136 {4} 0.31237 {5} 0.29814 {1} 0.29931 {2}
    ∑Ranks 34 {1} 44 {3} 42 {2} 61 {7} 46 {4} 87 {8} 59 {5.5} 59 {5.5}
    80 |BIAS| ˆϑ 0.49925 {2} 0.47135 {1} 0.52766 {3} 0.66329 {5} 0.55966 {4} 0.89224 {8} 0.67228 {6} 0.69447 {7}
    ˆφ 0.52063 {1} 0.55025 {3} 0.52909 {2} 0.70683 {7} 0.56354 {4} 0.93582 {8} 0.63998 {5} 0.69954 {6}
    ˆλ 0.25198 {1} 0.25883 {3} 0.25641 {2} 0.43311 {7} 0.30034 {4} 0.66563 {8} 0.40607 {5} 0.43206 {6}
    ˆθ 0.78480 {6} 0.82532 {7} 0.83697 {8} 0.69063 {3} 0.58559 {1} 0.72122 {5} 0.63842 {2} 0.69164 {4}
    MSEs ˆϑ 0.31719 {2} 0.30026 {1} 0.34946 {3} 0.55815 {5} 0.49857 {4} 0.86629 {8} 0.56483 {6} 0.59417 {7}
    ˆφ 0.27881 {1} 0.32553 {3} 0.29109 {2} 0.66883 {7} 0.44978 {4} 1.03760 {8} 0.55511 {5} 0.64550 {6}
    ˆλ 0.06369 {1} 0.06795 {3} 0.06642 {2} 0.29117 {7} 0.15725 {4} 0.54984 {8} 0.25250 {5} 0.28331 {6}
    ˆθ 0.66344 {5} 0.73666 {7} 0.74889 {8} 0.58730 {3} 0.45144 {1} 0.66717 {6} 0.51377 {2} 0.59201 {4}
    MREs ˆϑ 0.28529 {2} 0.26934 {1} 0.30152 {3} 0.37902 {5} 0.31981 {4} 0.50985 {8} 0.38416 {6} 0.39684 {7}
    ˆφ 0.26032 {1} 0.27512 {3} 0.26454 {2} 0.35341 {7} 0.28177 {4} 0.46791 {8} 0.31999 {5} 0.34977 {6}
    ˆλ 0.16798 {1} 0.17255 {3} 0.17094 {2} 0.28874 {7} 0.20023 {4} 0.44375 {8} 0.27071 {5} 0.28804 {6}
    ˆθ 0.31392 {6} 0.33013 {7} 0.33479 {8} 0.27625 {3} 0.23423 {1} 0.28849 {5} 0.25537 {2} 0.27666 {4}
    ∑Ranks 29 {1} 42 {3} 45 {4} 66 {6} 39 {2} 88 {8} 54 {5} 69 {7}
    200 |BIAS| ˆϑ 0.47555 {3} 0.44985 {2} 0.51341 {4} 0.67707 {6} 0.42436 {1} 0.89805 {8} 0.66134 {5} 0.69895 {7}
    ˆφ 0.50115 {2} 0.50837 {4} 0.50279 {3} 0.56296 {7} 0.41044 {1} 0.81679 {8} 0.51451 {5} 0.55936 {6}
    ˆλ 0.25019 {2} 0.25187 {4} 0.25076 {3} 0.41917 {7} 0.21499 {1} 0.65544 {8} 0.37297 {5} 0.41435 {6}
    ˆθ 0.79159 {6} 0.80107 {7} 0.82123 {8} 0.64647 {3} 0.43028 {1} 0.75308 {5} 0.57181 {2} 0.66234 {4}
    MSEs ˆϑ 0.25888 {2} 0.23847 {1} 0.29413 {3} 0.56097 {6} 0.37531 {4} 0.86783 {8} 0.54545 {5} 0.59107 {7}
    ˆφ 0.25134 {1} 0.26082 {4} 0.25349 {2} 0.43952 {7} 0.25968 {3} 0.80523 {8} 0.37160 {5} 0.43084 {6}
    ˆλ 0.06261 {1} 0.06361 {3} 0.06295 {2} 0.25891 {7} 0.09970 {4} 0.52619 {8} 0.20829 {5} 0.25203 {6}
    ˆθ 0.64670 {5} 0.66161 {6} 0.69240 {8} 0.51309 {3} 0.27527 {1} 0.68347 {7} 0.41304 {2} 0.53268 {4}
    MREs ˆϑ 0.27175 {3} 0.25705 {2} 0.29338 {4} 0.38690 {6} 0.24249 {1} 0.51317 {8} 0.37791 {5} 0.39940 {7}
    ˆφ 0.25057 {2} 0.25419 {4} 0.25139 {3} 0.28148 {7} 0.20522 {1} 0.40839 {8} 0.25725 {5} 0.27968 {6}
    ˆλ 0.16679 {2} 0.16791 {4} 0.16717 {3} 0.27945 {7} 0.14333 {1} 0.43696 {8} 0.24865 {5} 0.27623 {6}
    ˆθ 0.31664 {6} 0.32043 {7} 0.32849 {8} 0.25859 {3} 0.17211 {1} 0.30123 {5} 0.22872 {2} 0.26494 {4}
    ∑Ranks 35 {2} 48 {3} 51 {4.5} 69 {6.5} 20 {1} 89 {8} 51 {4.5} 69 {6.5}
    500 |BIAS| ˆϑ 0.47314 {3} 0.44590 {2} 0.51215 {4} 0.64549 {6} 0.23589 {1} 0.89642 {8} 0.57765 {5} 0.66728 {7}
    ˆφ 0.50000 {5} 0.50031 {7} 0.50001 {6} 0.44580 {4} 0.25497 {1} 0.66140 {8} 0.39329 {2} 0.42269 {3}
    ˆλ 0.25000 {2} 0.25003 {4} 0.25001 {3} 0.38068 {6} 0.11500 {1} 0.63857 {8} 0.31593 {5} 0.38454 {7}
    ˆθ 0.79445 {5} 0.80134 {6} 0.82604 {8} 0.59243 {3} 0.25280 {1} 0.80505 {7} 0.49356 {2} 0.61821 {4}
    MSEs ˆϑ 0.23703 {3} 0.21187 {2} 0.27432 {4} 0.51809 {6} 0.19898 {1} 0.86166 {8} 0.44538 {5} 0.54549 {7}
    ˆφ 0.25000 {3} 0.25034 {5} 0.25001 {4} 0.28548 {7} 0.11705 {1} 0.56366 {8} 0.22462 {2} 0.25796 {6}
    ˆλ 0.06250 {2.5} 0.06252 {4} 0.06250 {2.5} 0.20888 {6} 0.03935 {1} 0.50179 {8} 0.14873 {5} 0.20922 {7}
    ˆθ 0.63903 {5} 0.64949 {6} 0.68943 {7} 0.43597 {3} 0.12546 {1} 0.74499 {8} 0.31912 {2} 0.46804 {4}
    MREs ˆϑ 0.27037 {3} 0.25480 {2} 0.29266 {4} 0.36885 {6} 0.13479 {1} 0.51224 {8} 0.33008 {5} 0.38130 {7}
    ˆφ 0.25000 {5.5} 0.25015 {7} 0.25000 {5.5} 0.22290 {4} 0.12748 {1} 0.33070 {8} 0.19665 {2} 0.21134 {3}
    ˆλ 0.16667 {2.5} 0.16669 {4} 0.16667 {2.5} 0.25379 {6} 0.07667 {1} 0.42571 {8} 0.21062 {5} 0.25636 {7}
    ˆθ 0.31778 {5} 0.32053 {6} 0.33042 {8} 0.23697 {3} 0.10112 {1} 0.32202 {7} 0.19742 {2} 0.24728 {4}
    ∑Ranks 44.5 {3} 55 {4} 58.5 {5} 60 {6} 12 {1} 94 {8} 42 {2} 66 {7}
     | Show Table
    DownLoad: CSV
    Table 4.  Simulation results for η=(ϑ=3.5,φ=0.75,λ=0.5,θ=1.25)⊺.
    n Est. Est. Par. MLEs LSEs WLSEs CRVMEs MPSEs PCEs ADEs RADEs
    20 |BIAS| ˆϑ 1.05821 {8} 0.62985 {3} 0.96882 {6} 0.61879 {2} 0.39728 {1} 1.00062 {7} 0.93184 {5} 0.74851 {4}
    ˆφ 0.58239 {4} 0.61540 {8} 0.57588 {3} 0.61442 {7} 0.16810 {1} 0.59648 {6} 0.54321 {2} 0.58344 {5}
    ˆλ 0.09205 {8} 0.08984 {5} 0.08749 {3} 0.09161 {6} 0.07490 {1} 0.09181 {7} 0.08360 {2} 0.08890 {4}
    ˆθ 0.65193 {8} 0.48317 {2} 0.52978 {5} 0.51841 {4} 0.37874 {1} 0.57738 {7} 0.51808 {3} 0.53685 {6}
    MSEs ˆϑ 1.62863 {8} 0.80009 {2} 1.26747 {6} 0.83422 {3} 0.44242 {1} 1.52407 {7} 1.26323 {5} 1.02485 {4}
    ˆφ 0.42299 {3} 0.47351 {8} 0.43072 {4} 0.47310 {7} 0.39842 {2} 0.45399 {6} 0.39008 {1} 0.43912 {5}
    ˆλ 0.01191 {6} 0.01157 {5} 0.01104 {3} 0.01199 {7} 0.00839 {1} 0.01226 {8} 0.01037 {2} 0.01130 {4}
    ˆθ 0.56633 {8} 0.35145 {2} 0.39993 {3} 0.40530 {5} 0.22597 {1} 0.46238 {7} 0.39998 {4} 0.42492 {6}
    MREs ˆϑ 0.30235 {8} 0.17996 {3} 0.27681 {6} 0.17680 {2} 0.11351 {1} 0.28589 {7} 0.26624 {5} 0.21386 {4}
    ˆφ 0.77652 {4} 0.82054 {8} 0.76784 {3} 0.81923 {7} 0.73001 {2} 0.79531 {6} 0.72428 {1} 0.77792 {5}
    ˆλ 0.18410 {8} 0.17968 {5} 0.17498 {3} 0.18321 {6} 0.14981 {1} 0.18363 {7} 0.16720 {2} 0.17780 {4}
    ˆθ 0.52155 {8} 0.38654 {2} 0.42382 {5} 0.41473 {4} 0.30299 {1} 0.46190 {7} 0.41446 {3} 0.42948 {6}
    ∑Ranks 81 {7} 53 {4} 50 {3} 60 {6} 14 {1} 82 {8} 35 {2} 57 {5}
    80 |BIAS| ˆϑ 1.30458 {8} 0.99944 {2} 1.19014 {7} 1.02885 {3} 0.28553 {1} 1.10670 {4} 1.14568 {6} 1.11457 {5}
    ˆφ 0.32983 {3} 0.40620 {6} 0.34267 {4} 0.40746 {7} 0.27616 {1} 0.42471 {8} 0.32604 {2} 0.35934 {5}
    ˆλ 0.06496 {7} 0.06255 {5} 0.05976 {3} 0.06558 {8} 0.03810 {1} 0.06030 {4} 0.05724 {2} 0.06303 {6}
    ˆθ 0.60157 {8} 0.45112 {2} 0.47825 {5} 0.49541 {6} 0.19099 {1} 0.45370 {3} 0.45453 {4} 0.50033 {7}
    MSEs ˆϑ 1.98067 {8} 1.42246 {2} 1.62721 {7} 1.51028 {3} 0.28600 {1} 1.54773 {5} 1.54350 {4} 1.62460 {6}
    ˆφ 0.16347 {2} 0.24145 {7} 0.17842 {4} 0.24070 {6} 0.12275 {1} 0.27309 {8} 0.16427 {3} 0.19753 {5}
    ˆλ 0.00614 {7} 0.00590 {5} 0.00549 {3} 0.00646 {8} 0.00224 {1} 0.00582 {4} 0.00513 {2} 0.00600 {6}
    ˆθ 0.50150 {8} 0.33442 {3} 0.35764 {5} 0.39078 {6} 0.06108 {1} 0.33595 {4} 0.33240 {2} 0.39877 {7}
    MREs ˆϑ 0.37274 {8} 0.28555 {2} 0.34004 {7} 0.29396 {3} 0.08158 {1} 0.31620 {4} 0.32734 {6} 0.31845 {5}
    ˆφ 0.43977 {3} 0.54161 {6} 0.45690 {4} 0.54328 {7} 0.36821 {1} 0.56628 {8} 0.43472 {2} 0.47912 {5}
    ˆλ 0.12992 {7} 0.12509 {5} 0.11952 {3} 0.13116 {8} 0.07620 {1} 0.12061 {4} 0.11448 {2} 0.12607 {6}
    ˆθ 0.48126 {8} 0.36089 {2} 0.38260 {5} 0.39633 {6} 0.15279 {1} 0.36296 {3} 0.36362 {4} 0.40027 {7}
    ∑Ranks 77 {8} 47 {3} 57 {4} 71 {7} 12 {1} 59 {5} 39 {2} 70 {6}
    200 |BIAS| ˆϑ 1.18071 {8} 1.13329 {4} 1.15680 {6} 1.15101 {5} 0.12881 {1} 1.09310 {2} 1.11947 {3} 1.17399 {7}
    ˆφ 0.21288 {2} 0.27282 {6} 0.22827 {4} 0.28207 {7} 0.16810 {1} 0.31936 {8} 0.21687 {3} 0.23691 {5}
    ˆλ 0.05455 {5} 0.05483 {6} 0.05139 {4} 0.05648 {8} 0.02373 {1} 0.04586 {2} 0.05000 {3} 0.05607 {7}
    ˆθ 0.50995 {8} 0.46810 {5} 0.45161 {4} 0.48892 {7} 0.11088 {1} 0.36948 {2} 0.42847 {3} 0.48519 {6}
    MSEs ˆϑ 1.65210 {6} 1.59933 {5} 1.54359 {4} 1.65457 {7} 0.11230 {1} 1.41356 {2} 1.45126 {3} 1.65710 {8}
    ˆφ 0.06995 {2} 0.11564 {6} 0.08167 {4} 0.12209 {7} 0.04607 {1} 0.16683 {8} 0.07397 {3} 0.08772 {5}
    ˆλ 0.00441 {5} 0.00457 {6} 0.00403 {4} 0.00483 {8} 0.00088 {1} 0.00341 {2} 0.00386 {3} 0.00477 {7}
    ˆθ 0.38578 {8} 0.35447 {5} 0.32071 {4} 0.38130 {7} 0.02076 {1} 0.24604 {2} 0.29346 {3} 0.38069 {6}
    MREs ˆϑ 0.33734 {8} 0.32380 {4} 0.33052 {6} 0.32886 {5} 0.03680 {1} 0.31232 {2} 0.31985 {3} 0.33543 {7}
    ˆφ 0.28384 {2} 0.36376 {6} 0.30437 {4} 0.37610 {7} 0.22413 {1} 0.42582 {8} 0.28917 {3} 0.31587 {5}
    ˆλ 0.10910 {5} 0.10967 {6} 0.10278 {4} 0.11295 {8} 0.04745 {1} 0.09173 {2} 0.10000 {3} 0.11214 {7}
    ˆθ 0.40796 {8} 0.37448 {5} 0.36129 {4} 0.39114 {7} 0.08871 {1} 0.29559 {2} 0.34277 {3} 0.38815 {6}
    ∑Ranks 67 {6} 64 {5} 52 {4} 83 {8} 12 {1} 42 {3} 36 {2} 76 {7}
    500 |BIAS| ˆϑ 0.99609 {2} 1.12481 {6} 1.05940 {5} 1.12860 {7} 0.04256 {1} 1.04542 {4} 1.03887 {3} 1.14079 {8}
    ˆφ 0.14496 {2} 0.18941 {6} 0.15844 {4} 0.19306 {7} 0.10411 {1} 0.23525 {8} 0.15349 {3} 0.16453 {5}
    ˆλ 0.04309 {3} 0.05010 {7} 0.04425 {5} 0.04998 {6} 0.01474 {1} 0.03603 {2} 0.04334 {4} 0.05109 {8}
    ˆθ 0.38900 {4} 0.44670 {6} 0.39875 {5} 0.45266 {7} 0.06374 {1} 0.31824 {2} 0.38560 {3} 0.45867 {8}
    MSEs ˆϑ 1.21294 {2} 1.50352 {6} 1.31440 {5} 1.52047 {8} 0.02893 {1} 1.25338 {3} 1.26686 {4} 1.51668 {7}
    ˆφ 0.03263 {2} 0.05612 {6} 0.03902 {4} 0.05818 {7} 0.01736 {1} 0.09221 {8} 0.03639 {3} 0.04120 {5}
    ˆλ 0.00278 {3} 0.00378 {6.5} 0.00295 {5} 0.00378 {6.5} 0.00034 {1} 0.00220 {2} 0.00283 {4} 0.00400 {8}
    ˆθ 0.23678 {4} 0.31580 {6} 0.25000 {5} 0.32430 {7} 0.00703 {1} 0.18536 {2} 0.23280 {3} 0.33477 {8}
    MREs ˆϑ 0.28460 {2} 0.32137 {6} 0.30269 {5} 0.32246 {7} 0.01216 {1} 0.29869 {4} 0.29682 {3} 0.32594 {8}
    ˆφ 0.19328 {2} 0.25255 {6} 0.21126 {4} 0.25741 {7} 0.13881 {1} 0.31367 {8} 0.20466 {3} 0.21938 {5}
    ˆλ 0.08617 {3} 0.10020 {7} 0.08850 {5} 0.09997 {6} 0.02948 {1} 0.07207 {2} 0.08669 {4} 0.10217 {8}
    ˆθ 0.31120 {4} 0.35736 {6} 0.31900 {5} 0.36213 {7} 0.05100 {1} 0.25459 {2} 0.30848 {3} 0.36694 {8}
    ∑Ranks 33 {2} 74.5 {6} 57 {5} 82.5 {7} 12 {1} 47 {4} 40 {3} 86 {8}
     | Show Table
    DownLoad: CSV
    Table 5.  Simulation results for η=(ϑ=3.5,φ=2,λ=1.5,θ=2.5)⊺.
    n Est. Est. Par. MLEs LSEs WLSEs CRVMEs MPSEs PCEs ADEs RADEs
    20 |BIAS| ˆϑ 0.98923 {7} 0.64331 {3} 0.95672 {6} 0.63413 {2} 0.44818 {1} 1.04755 {8} 0.90857 {5} 0.78916 {4}
    ˆφ 0.95367 {6} 0.90318 {4} 0.88553 {3} 0.95450 {7} 0.31253 {1} 0.97463 {8} 0.85825 {2} 0.93652 {5}
    ˆλ 0.50387 {8} 0.29370 {2} 0.38814 {5} 0.31558 {3} 0.23477 {1} 0.41787 {7} 0.38863 {6} 0.36144 {4}
    ˆθ 0.83605 {8} 0.71444 {3} 0.81238 {7} 0.68991 {2} 0.62151 {1} 0.74404 {5} 0.75604 {6} 0.71561 {4}
    MSEs ˆϑ 1.35323 {7} 0.71389 {2} 1.18225 {6} 0.73718 {3} 0.37586 {1} 1.57224 {8} 1.13154 {5} 1.02701 {4}
    ˆφ 1.18857 {8} 1.06788 {4} 1.02750 {3} 1.18599 {7} 0.87512 {1} 1.15778 {6} 0.96462 {2} 1.13400 {5}
    ˆλ 0.36432 {8} 0.14714 {2} 0.22539 {5} 0.17712 {3} 0.09172 {1} 0.28638 {7} 0.23923 {6} 0.22267 {4}
    ˆθ 0.77865 {8} 0.64926 {4} 0.77561 {7} 0.60519 {2} 0.52925 {1} 0.67762 {5} 0.68866 {6} 0.64046 {3}
    MREs ˆϑ 0.28264 {7} 0.18380 {3} 0.27335 {6} 0.18118 {2} 0.12805 {1} 0.29930 {8} 0.25959 {5} 0.22547 {4}
    ˆφ 0.47683 {6} 0.45159 {4} 0.44276 {3} 0.47725 {7} 0.40539 {1} 0.48731 {8} 0.42912 {2} 0.46826 {5}
    ˆλ 0.33591 {8} 0.19580 {2} 0.25876 {5} 0.21038 {3} 0.15651 {1} 0.27858 {7} 0.25908 {6} 0.24096 {4}
    ˆθ 0.33442 {8} 0.28578 {3} 0.32495 {7} 0.27596 {2} 0.24860 {1} 0.29762 {5} 0.30242 {6} 0.28624 {4}
    ∑Ranks 89 {8} 36 {2} 63 {6} 43 {3} 12 {1} 82 {7} 57 {5} 50 {4}
    80 |BIAS| ˆϑ 1.00512 {8} 0.76116 {3} 0.98814 {7} 0.76068 {2} 0.22490 {1} 0.94177 {5} 0.95845 {6} 0.83406 {4}
    ˆφ 0.56247 {3} 0.64453 {6} 0.57353 {4} 0.64522 {7} 0.46925 {1} 0.76277 {8} 0.55915 {2} 0.63949 {5}
    ˆλ 0.45485 {8} 0.31115 {2} 0.36895 {6} 0.33000 {3} 0.11306 {1} 0.39112 {7} 0.36093 {5} 0.34405 {4}
    ˆθ 0.76702 {8} 0.62063 {3} 0.68635 {7} 0.62598 {4} 0.33066 {1} 0.60384 {2} 0.66063 {6} 0.63130 {5}
    MSEs ˆϑ 1.24539 {7} 0.92842 {2} 1.16588 {6} 0.94580 {3} 0.13919 {1} 1.25646 {8} 1.12647 {5} 1.05555 {4}
    ˆφ 0.45689 {3} 0.57098 {6} 0.46410 {4} 0.57244 {7} 0.32482 {1} 0.71835 {8} 0.44181 {2} 0.55971 {5}
    ˆλ 0.28629 {8} 0.17260 {2} 0.20504 {5} 0.19582 {3} 0.02220 {1} 0.25122 {7} 0.20232 {4} 0.20735 {6}
    ˆθ 0.68706 {8} 0.51170 {3} 0.58072 {7} 0.52071 {4} 0.16747 {1} 0.49400 {2} 0.55280 {6} 0.52733 {5}
    MREs ˆϑ 0.28718 {8} 0.21747 {3} 0.28233 {7} 0.21734 {2} 0.06426 {1} 0.26908 {5} 0.27384 {6} 0.23830 {4}
    ˆφ 0.28123 {3} 0.32226 {6} 0.28677 {4} 0.32261 {7} 0.23462 {1} 0.38138 {8} 0.27957 {2} 0.31974 {5}
    ˆλ 0.30323 {8} 0.20744 {2} 0.24597 {6} 0.22000 {3} 0.07538 {1} 0.26075 {7} 0.24062 {5} 0.22937 {4}
    ˆθ 0.30681 {8} 0.24825 {3} 0.27454 {7} 0.25039 {4} 0.13226 {1} 0.24153 {2} 0.26425 {6} 0.25252 {5}
    ∑Ranks 80 {8} 41 {2} 70 {7} 49 {3} 12 {1} 69 {6} 55 {4} 56 {5}
    200 |BIAS| ˆϑ 0.98460 {6} 0.86500 {3} 1.00674 {7} 0.86298 {2} 0.12154 {1} 1.05269 {8} 0.98185 {5} 0.89235 {4}
    ˆφ 0.38859 {2} 0.45911 {5} 0.41129 {4} 0.46722 {6} 0.31253 {1} 0.67732 {8} 0.40329 {3} 0.46920 {7}
    ˆλ 0.39873 {8} 0.31023 {2} 0.35370 {6} 0.32546 {3} 0.06881 {1} 0.37938 {7} 0.34126 {5} 0.32900 {4}
    ˆθ 0.68985 {8} 0.57483 {3} 0.64129 {7} 0.59868 {5} 0.21029 {1} 0.55195 {2} 0.61763 {6} 0.59808 {4}
    MSEs ˆϑ 1.11626 {6} 1.01791 {2} 1.13048 {7} 1.02064 {3} 0.05498 {1} 1.30905 {8} 1.09868 {5} 1.05550 {4}
    ˆφ 0.23053 {2} 0.31234 {5} 0.25573 {4} 0.32276 {6} 0.15678 {1} 0.58268 {8} 0.24649 {3} 0.32421 {7}
    ˆλ 0.22103 {7} 0.16583 {2} 0.18036 {5} 0.17985 {4} 0.00837 {1} 0.22479 {8} 0.17270 {3} 0.18259 {6}
    ˆθ 0.58585 {8} 0.45832 {3} 0.51809 {7} 0.48976 {5} 0.07171 {1} 0.44322 {2} 0.49209 {6} 0.48968 {4}
    MREs ˆϑ 0.28132 {6} 0.24714 {3} 0.28764 {7} 0.24657 {2} 0.03472 {1} 0.30077 {8} 0.28053 {5} 0.25496 {4}
    ˆφ 0.19429 {2} 0.22956 {5} 0.20564 {4} 0.23361 {6} 0.15627 {1} 0.33866 {8} 0.20165 {3} 0.23460 {7}
    ˆλ 0.26582 {8} 0.20682 {2} 0.23580 {6} 0.21697 {3} 0.04587 {1} 0.25292 {7} 0.22751 {5} 0.21933 {4}
    ˆθ 0.27594 {8} 0.22993 {3} 0.25652 {7} 0.23947 {5} 0.08412 {1} 0.22078 {2} 0.24705 {6} 0.23923 {4}
    ∑Ranks 71 {6.5} 38 {2} 71 {6.5} 50 {3} 12 {1} 76 {8} 55 {4} 59 {5}
    500 |BIAS| ˆϑ 0.93304 {5} 0.93263 {4} 0.97539 {7} 0.92961 {3} 0.05348 {1} 1.08240 {8} 0.95522 {6} 0.92527 {2}
    ˆφ 0.27572 {2} 0.32936 {5} 0.29210 {4} 0.33160 {6} 0.19113 {1} 0.58412 {8} 0.28766 {3} 0.33820 {7}
    ˆλ 0.34882 {7} 0.31976 {2} 0.33838 {6} 0.33117 {5} 0.03908 {1} 0.35124 {8} 0.32736 {3} 0.32969 {4}
    ˆθ 0.61892 {8} 0.57580 {3} 0.60923 {7} 0.59341 {6} 0.12272 {1} 0.51040 {2} 0.59246 {5} 0.58416 {4}
    MSEs ˆϑ 0.99386 {2} 1.04383 {6} 1.04849 {7} 1.04352 {5} 0.00732 {1} 1.28318 {8} 1.01861 {3} 1.03056 {4}
    ˆφ 0.11591 {2} 0.16651 {5} 0.13079 {4} 0.16807 {6} 0.06394 {1} 0.45248 {8} 0.12739 {3} 0.17250 {7}
    ˆλ 0.17000 {7} 0.15789 {3} 0.15993 {4} 0.16663 {5} 0.00250 {1} 0.18698 {8} 0.15186 {2} 0.16915 {6}
    ˆθ 0.48714 {8} 0.45146 {4} 0.47250 {6} 0.47497 {7} 0.02504 {1} 0.39915 {2} 0.45105 {3} 0.47078 {5}
    MREs ˆϑ 0.26658 {5} 0.26647 {4} 0.27868 {7} 0.26560 {3} 0.01528 {1} 0.30926 {8} 0.27292 {6} 0.26436 {2}
    ˆφ 0.13786 {2} 0.16468 {5} 0.14605 {4} 0.16580 {6} 0.09557 {1} 0.29206 {8} 0.14383 {3} 0.16910 {7}
    ˆλ 0.23255 {7} 0.21318 {2} 0.22559 {6} 0.22078 {5} 0.02606 {1} 0.23416 {8} 0.21824 {3} 0.21980 {4}
    ˆθ 0.24757 {8} 0.23032 {3} 0.24369 {7} 0.23736 {6} 0.04909 {1} 0.20416 {2} 0.23698 {5} 0.23367 {4}
    ∑Ranks 63 {5.5} 46 {3} 69 {7} 63 {5.5} 12 {1} 78 {8} 45 {2} 56 {4}
     | Show Table
    DownLoad: CSV
    Table 6.  Simulation results for η=(ϑ=4.5,φ=0.75,λ=0.5,θ=1.25)⊺.
    n Est. Est. Par. MLEs LSEs WLSEs CRVMEs MPSEs PCEs ADEs RADEs
    20 |BIAS| ˆϑ 1.25197 {8} 0.45438 {3} 1.06521 {7} 0.44888 {2} 0.20680 {1} 0.83805 {5} 1.02528 {6} 0.62513 {4}
    ˆφ 0.58123 {5} 0.60203 {7} 0.57995 {4} 0.61278 {8} 0.16575 {1} 0.57749 {3} 0.53508 {2} 0.58165 {6}
    ˆλ 0.08854 {8} 0.07652 {2} 0.08227 {7} 0.07831 {3} 0.06474 {1} 0.08065 {6} 0.07939 {4} 0.07980 {5}
    ˆθ 0.66759 {8} 0.42262 {2} 0.53041 {7} 0.44839 {3} 0.32466 {1} 0.51024 {5} 0.52797 {6} 0.48959 {4}
    MSEs ˆϑ 2.41625 {8} 0.60512 {2} 1.67131 {6} 0.67908 {3} 0.18285 {1} 1.58289 {5} 1.74298 {7} 1.01407 {4}
    ˆφ 0.42148 {3} 0.46192 {7} 0.43565 {5} 0.46874 {8} 0.38218 {1} 0.43432 {4} 0.38271 {2} 0.43679 {6}
    ˆλ 0.01072 {8} 0.00870 {2} 0.00963 {7} 0.00906 {3} 0.00620 {1} 0.00950 {6} 0.00923 {5} 0.00919 {4}
    ˆθ 0.58894 {8} 0.28693 {2} 0.40885 {6} 0.32249 {3} 0.16767 {1} 0.38646 {5} 0.41599 {7} 0.36863 {4}
    MREs ˆϑ 0.27821 {8} 0.10097 {3} 0.23671 {7} 0.09975 {2} 0.04596 {1} 0.18623 {5} 0.22784 {6} 0.13892 {4}
    ˆφ 0.77497 {5} 0.80271 {7} 0.77327 {4} 0.81704 {8} 0.70903 {1} 0.76998 {3} 0.71344 {2} 0.77554 {6}
    ˆλ 0.17707 {8} 0.15303 {2} 0.16453 {7} 0.15662 {3} 0.12948 {1} 0.16130 {6} 0.15879 {4} 0.15960 {5}
    ˆθ 0.53407 {8} 0.33809 {2} 0.42432 {7} 0.35871 {3} 0.25973 {1} 0.40819 {5} 0.42237 {6} 0.39167 {4}
    ∑Ranks 85 {8} 41 {2} 74 {7} 49 {3} 12 {1} 58 {6} 57 {5} 56 {4}
    80 |BIAS| ˆϑ 1.62748 {8} 0.91367 {2} 1.37823 {7} 0.98139 {3} 0.11224 {1} 1.13720 {5} 1.31803 {6} 1.11805 {4}
    ˆφ 0.31837 {3} 0.39968 {7} 0.34474 {4} 0.40380 {8} 0.27352 {1} 0.37466 {6} 0.31821 {2} 0.35079 {5}
    ˆλ 0.07169 {8} 0.05804 {3} 0.06253 {7} 0.06113 {6} 0.03286 {1} 0.05699 {2} 0.05980 {5} 0.05870 {4}
    ˆθ 0.64853 {8} 0.39956 {2} 0.49396 {7} 0.44278 {4} 0.16292 {1} 0.43570 {3} 0.47512 {6} 0.45276 {5}
    MSEs ˆϑ 3.14585 {8} 1.58782 {2} 2.33956 {7} 1.79431 {3} 0.08679 {1} 1.96177 {4} 2.22845 {6} 2.05141 {5}
    ˆφ 0.15254 {2} 0.23761 {7} 0.18156 {4} 0.24031 {8} 0.12335 {1} 0.22013 {6} 0.15798 {3} 0.19064 {5}
    ˆλ 0.00707 {8} 0.00520 {4} 0.00579 {7} 0.00566 {6} 0.00171 {1} 0.00514 {2} 0.00541 {5} 0.00519 {3}
    ˆθ 0.56658 {8} 0.28713 {2} 0.38674 {7} 0.34041 {4} 0.04197 {1} 0.32550 {3} 0.36722 {6} 0.35156 {5}
    MREs ˆϑ 0.36166 {8} 0.20304 {2} 0.30627 {7} 0.21809 {3} 0.02494 {1} 0.25271 {5} 0.29289 {6} 0.24846 {4}
    ˆφ 0.42449 {3} 0.53291 {7} 0.45966 {4} 0.53840 {8} 0.36469 {1} 0.49955 {6} 0.42429 {2} 0.46771 {5}
    ˆλ 0.14337 {8} 0.11609 {3} 0.12506 {7} 0.12226 {6} 0.06572 {1} 0.11399 {2} 0.11961 {5} 0.11740 {4}
    ˆθ 0.51882 {8} 0.31965 {2} 0.39517 {7} 0.35422 {4} 0.13034 {1} 0.34856 {3} 0.38010 {6} 0.36221 {5}
    ∑Ranks 80 {8} 43 {2} 75 {7} 63 {6} 12 {1} 47 {3} 58 {5} 54 {4}
    200 |BIAS| ˆϑ 1.48139 {8} 1.17284 {2} 1.40391 {7} 1.21294 {4} 0.04307 {1} 1.17950 {3} 1.33404 {6} 1.29174 {5}
    ˆφ 0.20186 {2} 0.26830 {7} 0.21385 {4} 0.26575 {6} 0.16575 {1} 0.27408 {8} 0.20558 {3} 0.23166 {5}
    ˆλ 0.06056 {8} 0.05260 {3} 0.05573 {7} 0.05571 {6} 0.02038 {1} 0.04542 {2} 0.05309 {4} 0.05470 {5}
    ˆθ 0.54563 {8} 0.42847 {3} 0.47838 {7} 0.45810 {5} 0.09746 {1} 0.36816 {2} 0.44966 {4} 0.46232 {6}
    MSEs ˆϑ 2.63270 {8} 2.08923 {3} 2.37558 {7} 2.20468 {5} 0.02535 {1} 1.86391 {2} 2.18628 {4} 2.32684 {6}
    ˆφ 0.06343 {2} 0.11259 {7} 0.07126 {4} 0.11064 {6} 0.04499 {1} 0.12626 {8} 0.06649 {3} 0.08397 {5}
    ˆλ 0.00531 {8} 0.00437 {3} 0.00472 {6} 0.00486 {7} 0.00066 {1} 0.00347 {2} 0.00441 {4} 0.00470 {5}
    ˆθ 0.44122 {8} 0.33416 {3} 0.37078 {5} 0.37139 {6} 0.01519 {1} 0.25838 {2} 0.33754 {4} 0.37704 {7}
    MREs ˆϑ 0.32920 {8} 0.26063 {2} 0.31198 {7} 0.26954 {4} 0.00957 {1} 0.26211 {3} 0.29645 {6} 0.28705 {5}
    ˆφ 0.26915 {2} 0.35774 {7} 0.28513 {4} 0.35434 {6} 0.22100 {1} 0.36544 {8} 0.27411 {3} 0.30888 {5}
    ˆλ 0.12113 {8} 0.10520 {3} 0.11146 {7} 0.11142 {6} 0.04075 {1} 0.09084 {2} 0.10618 {4} 0.10941 {5}
    ˆθ 0.43650 {8} 0.34278 {3} 0.38270 {7} 0.36648 {5} 0.07797 {1} 0.29453 {2} 0.35973 {4} 0.36986 {6}
    ∑Ranks 78 {8} 46 {3} 72 {7} 66 {6} 12 {1} 44 {2} 49 {4} 65 {5}
    500 |BIAS| ˆϑ 1.29672 {5} 1.27235 {3} 1.31237 {6} 1.32513 {7} 0.01519 {1} 1.15120 {2} 1.28994 {4} 1.34189 {8}
    ˆφ 0.13744 {2} 0.17802 {6} 0.14815 {4} 0.18664 {7} 0.10227 {1} 0.19392 {8} 0.14640 {3} 0.15865 {5}
    ˆλ 0.04970 {5} 0.05107 {6} 0.04913 {4} 0.05400 {8} 0.01264 {1} 0.03665 {2} 0.04825 {3} 0.05335 {7}
    ˆθ 0.44102 {5} 0.44509 {6} 0.43050 {4} 0.47518 {8} 0.05962 {1} 0.31796 {2} 0.42160 {3} 0.46945 {7}
    MSEs ˆϑ 2.07266 {4} 2.19148 {6} 2.07839 {5} 2.34226 {8} 0.00344 {1} 1.67247 {2} 2.02330 {3} 2.31957 {7}
    ˆφ 0.02955 {2} 0.05030 ^{\left\{6 \right\}} 0.03417 ^{\left\{4 \right\}} 0.05288 ^{\left\{7 \right\}} 0.01679 ^{\left\{1 \right\}} 0.06276 ^{\left\{8 \right\}} 0.03295 ^{\left\{3 \right\}} 0.03874 ^{\left\{5 \right\}}
    \hat{\lambda} 0.00377 ^{\left\{4 \right\}} 0.00418 ^{\left\{6 \right\}} 0.00378 ^{\left\{5 \right\}} 0.00452 ^{\left\{7 \right\}} 0.00025 ^{\left\{1 \right\}} 0.00242 ^{\left\{2 \right\}} 0.00363 ^{\left\{3 \right\}} 0.00454 ^{\left\{8 \right\}}
    \hat{\theta} 0.30987 ^{\left\{5 \right\}} 0.34423 ^{\left\{6 \right\}} 0.30505 ^{\left\{4 \right\}} 0.37795 ^{\left\{8 \right\}} 0.00564 ^{\left\{1 \right\}} 0.19875 ^{\left\{2 \right\}} 0.29242 ^{\left\{3 \right\}} 0.37470 ^{\left\{7 \right\}}
    MREs \hat{\vartheta} 0.28816 ^{\left\{5 \right\}} 0.28275 ^{\left\{3 \right\}} 0.29164 ^{\left\{6 \right\}} 0.29447 ^{\left\{7 \right\}} 0.00337 ^{\left\{1 \right\}} 0.25582 ^{\left\{2 \right\}} 0.28665 ^{\left\{4 \right\}} 0.29820 ^{\left\{8 \right\}}
    \hat{\varphi} 0.18326 ^{\left\{2 \right\}} 0.23736 ^{\left\{6 \right\}} 0.19754 ^{\left\{4 \right\}} 0.24885 ^{\left\{7 \right\}} 0.13636 ^{\left\{1 \right\}} 0.25856 ^{\left\{8 \right\}} 0.19520 ^{\left\{3 \right\}} 0.21153 ^{\left\{5 \right\}}
    \hat{\lambda} 0.09940 ^{\left\{5 \right\}} 0.10214 ^{\left\{6 \right\}} 0.09825 ^{\left\{4 \right\}} 0.10801 ^{\left\{8 \right\}} 0.02528 ^{\left\{1 \right\}} 0.07330 ^{\left\{2 \right\}} 0.09651 ^{\left\{3 \right\}} 0.10671 ^{\left\{7 \right\}}
    \hat{\theta} 0.35281 ^{\left\{5 \right\}} 0.35607 ^{\left\{6 \right\}} 0.34440 ^{\left\{4 \right\}} 0.38014 ^{\left\{8 \right\}} 0.04770 ^{\left\{1 \right\}} 0.25437 ^{\left\{2 \right\}} 0.33728 ^{\left\{3 \right\}} 0.37556 ^{\left\{7 \right\}}
    \sum{Ranks} {\textbf{49}} ^{\left\{4 \right\}} {\textbf{66}} ^{\left\{6 \right\}} {\textbf{54}} ^{\left\{5 \right\}} {\textbf{90}} ^{\left\{8 \right\}} {\textbf{12}} ^{\left\{1 \right\}} {\textbf{42}} ^{\left\{3 \right\}} {\textbf{38}} ^{\left\{2 \right\}} {\textbf{81}} ^{\left\{7 \right\}}
     | Show Table
    DownLoad: CSV

    All computations are obtained using R software (version 4.0.2) [22].

    For each sample and each parametric combination, the EWFr parameters \vartheta , \varphi , \lambda and \theta are estimated using the eight estimators called MLEs, LSEs, WLSEs, MPSEs, CRVMEs, ADEs, PCEs, and RADEs. Simulated results are listed in Tables 2–6 which also indicate the ranks of each of the proposed estimators in each row, where the superscripts show the indicators, and the \sum Ranks illustrates the partial sum of ranks in each column for a particular sample size.

    Table 7 lists the partial and overall ranks for all parametric combinations. From Tables 2–6, one can conclude that all methods of estimation illustrate the consistency property, that is, the MREs and MSEs decrease as the sample size increases, for all parametric combinations. Table 7 shows that the MPS approach has an overall score of 54, hence it outperforms other estimation methods. Therefore, we conclude and confirm the superiority of MPSEs for estimating the EWFr parameters. However, the ADEs and MLEs are approximately have a similar performance, where their overall scores are, respectively, 130.5 and 133.5.

    Table 7.  Partial and overall ranks of the classical estimation methods for several parametric values.
    \mathit{\boldsymbol{\eta}}^{\intercal} n MLEs LSEs WLSEs CRVMEs MPSEs PCEs ADEs RADEs
    (\hat{\vartheta}=1.75, \hat{\varphi}=0.5, \hat{\lambda}=0.25, \hat{\theta}=1.25) 20 2 7 1 5 4 8 3 6
    80 3 7 2 6 1 5 4 8
    200 1.5 7 4 5 1.5 6 3 8
    500 2 5.5 4 5.5 1 7 3 8
    (\hat{\vartheta}=1.75, \hat{\varphi}=2, \hat{\lambda}=1.5, \hat{\theta}=2.5) 20 1 3 2 7 4 8 5.5 5.5
    80 1 3 4 6 2 8 5 7
    200 2 3 4.5 6.5 1 8 4.5 6.5
    500 3 4 5 6 1 8 2 7
    (\hat{\vartheta}=3.5, \hat{\varphi}=0.75, \hat{\lambda}=0.5, \hat{\theta}=1.25) 20 7 4 3 6 1 8 2 5
    80 8 3 4 7 1 5 2 6
    200 6 5 4 8 1 3 2 7
    500 2 6 5 7 1 4 3 8
    (\hat{\vartheta}=3.5, \hat{\varphi}=2, \hat{\lambda}=1.5, \hat{\theta}=2.5) 20 8 2 6 3 1 7 5 4
    80 8 2 7 3 1 6 4 5
    200 6.5 2 6.5 3 1 8 4 5
    500 5.5 3 7 5.5 1 8 2 4
    (\hat{\vartheta}=4.5, \hat{\varphi}=0.75, \hat{\lambda}=0.5, \hat{\theta}=1.25) 20 8 2 7 3 1 6 5 4
    80 8 2 7 6 1 3 5 4
    200 8 3 7 6 1 2 4 5
    500 4 6 5 8 1 3 2 7
    (\hat{\vartheta}=4.5, \hat{\varphi}=0.75, \hat{\lambda}=0.5, \hat{\theta}=1.25) 20 8 2 5 3 1 7 6 4
    80 6 2 4 3 1 8 7 5
    200 6 2 4 3 1 7 8 5
    500 6 2 5 3 1 8 7 4
    (\hat{\vartheta}=0.25, \hat{\varphi}=3.5, \hat{\lambda}=3, \hat{\theta}=0.25) 20 1 6 8 2 7 5 4 3
    80 1.5 5 8 1.5 6 4 3 7
    200 1 7 4 6 2 3 5 8
    500 2 8 6 7 1 3 4.5 4.5
    (\hat{\vartheta}=0.75, \hat{\varphi}=2.5, \hat{\lambda}=4.25, \hat{\theta}=0.25) 20 2 8 6 5 3 1 4 7
    80 1.5 7 5 6 1.5 3 4 8
    200 2 7 5 6 1 3 4 8
    500 2 8 5 6 1 3 4 7
    \sum Ranks \textbf{133.5} \textbf{143.5}\textbf{160}\textbf{164}\textbf{54}\textbf{176}\textbf{130.5}\textbf{190.5}
    {\textbf{Overall Rank}}\textbf{3}\textbf{4}\textbf{5}\textbf{6}\textbf{1}\textbf{7}\textbf{2}\textbf{8}
     | Show Table
    DownLoad: CSV

    5.   Modeling medicine and engineering data

    In this section, the flexibility and importance of the EWFr distribution in modeling real-life data are illustrated using two datasets from the medicine and engineering fields. The first dataset contains 128 observations and it refers to remission times (in months) for bladder cancer patients [23]. The second dataset contains 63 observations and it represents strengths for single carbon fibers of 10 mm of gauge lengths [24]. The fits of the EWFr distribution is checked and compared with some important extensions of the Fr distribution called the exponentiated-Fr (EFr) [6], beta-Fr (BFr) [7], odd Lomax-Fr (OLxFr) [25], Kumaraswamy Marshall–Olkin Fr (KMOFr) [26], gamma extended-Fr (GExFr) [27] and transmuted exponentiated-Fr (TEFr) [28] and Fr distributions.

    We checked the competing distributions using some goodness-of-fit analytical measures such as AIC (Akaike information criterion), CAIC (consistent Akaike information criterion), HQIC (Hannan–Quinn information criterion), BIC (Bayesian information criterion), W^{\ast} (Cramér–Von Mises), A^{\ast} (Anderson–Darling), and KS (Kolmogorov–Smirnov) statistics with its PV (p-value).

    The maximum likelihood (ML) estimates of the parameters of the fitted distributions, their standard errors (SEs), and the analytical measures are reported in Tables 8 and 9 for the cancer and gauge lengths data, respectively. The numbers in Tables 8 and 9 indicate that the EWFr distribution gives a superior fit over other competing models, since it has the lowest values for all measures and the largest PV.

    Table 8.  The parameters estimates of the competing distributions and goodness-of-fit measures for cancer data.
    Model Par.. Estimates (SEs) AIC CAIC BIC HQIC W^{*} A^{*} KS (PV)
    EWFr \hat{\vartheta} 56.93662 (38.42845) 827.52150 827.84670 838.92960 832.15660 0.01991 0.13402 0.03757 0.99713
    \hat{\varphi} 0.46697 (0.22382)
    \hat{\lambda} 0.71825 (0.01713)
    \hat{\theta} 0.01794 (0.01207)
    OLxFr \hat{\vartheta} 2.18260 (1.04763) 827.70270 828.02790 839.11080 832.33780 0.02380 0.16520 0.04060 0.98433
    \hat{\varphi} 625.38000 (622.71234)
    \hat{a} 0.12210 (0.08023)
    \hat{b} 1.38560 (0.17273)
    KMOFr \hat{\vartheta} 43.87750 (128.52731) 831.05330 831.54510 845.31340 836.84730 0.26278 0.34950 0.04457 0.96120
    \hat{\varphi} 0.52028 (0.41388)
    \hat{\delta} 0.01314 (0.00808)
    \hat{a} 3.27359 (2.90929)
    \hat{b} 19.93782 (47.58906)
    EFr \hat{a} 1426.40000 (1440.19245) 830.85880 831.05240 839.41490 834.33520 0.06720 0.46640 0.04910 0.91754
    \hat{b} 0.26240 (0.02850)
    \hat{\theta} 46.24800 (22.87321)
    BFr \hat{\vartheta} 0.60970 (0.32261) 833.09830 833.42350 844.50640 837.73350 0.06900 0.47650 0.05540 0.82683
    \hat{\varphi} 36.60200 (19.48341)
    \hat{a} 739.38700 (629.24021)
    \hat{b} 0.32240 (0.06044)
    GExFr \hat{\vartheta} 226.67000 (267.96324) 840.21630 840.54150 851.62450 844.85150 0.14320 0.95970 0.06640 0.62593
    \hat{\varphi} 91.93900 (133.94324)
    \hat{a} 22.27100 (119.19139)
    \hat{b} 0.07090 (0.04132)
    TEFr \hat{\vartheta} 3.25820 (0.40714) 892.00150 892.09750 897.70560 894.31910 0.74430 4.54640 0.14080 0.01254
    \hat{\varphi} 0.75210 (0.04224)
    \hat{a} 39.38721 (40.24321)
    \hat{b} 0.25243 (0.76041)
    Fr \hat{\theta} 0.75208 (0.04242) 892.00150 892.09750 897.70560 894.31910 0.74432 4.54642 0.14079 0.01250
    \hat{\lambda} 2.43109 (0.21928)
     | Show Table
    DownLoad: CSV
    Table 9.  The parameters estimates of the competing distributions and goodness-of-fit measures for gauge lengths data.
    Model Par.. Estimates (SEs) AIC CAIC BIC HQIC W^{*} A^{*} KS (PV)
    EWFr \hat{\vartheta} 0.76563 (0.3765631) 119.68850 120.37820 128.26110 123.06020 0.04181 0.23988 0.06847 0.92920
    \hat{\varphi} 0.04349 (0.3074545)
    \hat{\lambda} 145.67068 (208.851634)
    \hat{\theta} 4.60846 (1.2033681)
    OLxFr \hat{\vartheta} 5.14301 (9.9790719) 119.94170 120.63140 128.51430 123.31340 0.04741 0.26530 0.07631 0.85664
    \hat{\varphi} 5.82568 (7.6204045)
    \hat{a} 4.17374 (2.671917)
    \hat{b} 2.82338 (0.9308868)
    KMOFr \hat{\vartheta} 31946.70000 (837.7074) 121.90480 122.95750 132.62050 126.11940 0.04616 0.26108 0.07450 0.87551
    \hat{\varphi} 3.40405 (1.493079)
    \hat{\delta} 3.35291 (0.9682264)
    \hat{a} 0.85381 (0.1934628)
    \hat{b} 13724.50000 (32837.29)
    EFr \hat{a} 2.58672 (2.2847576) 122.19730 122.88700 130.76990 125.56900 0.07082 0.40707 0.08894 0.70136
    \hat{b} 0.50304 (0.4780356)
    \hat{\theta} 6.25000 (10.0300775)
    BFr \hat{\vartheta} 2.38748 (5.65075) 120.58420 121.27380 129.15670 123.95580 0.06008 0.32203 0.07953 0.82045
    \hat{\varphi} 1.00168 (5.322252)
    \hat{a} 22.38231 (162.860747)
    \hat{b} 27.37096 (295.790324)
    GExFr \hat{\vartheta} 2.41716 (8.509608) 120.57980 121.26950 129.15240 123.95150 0.06064 0.32329 0.07962 0.81934
    \hat{\varphi} 1.20859 (2.106142)
    \hat{a} 24.77784 (82.649275)
    \hat{b} 15.60163 (85.339807)
    TEFr \hat{\vartheta} 4.04638 (1.0119058) 121.04420 121.73390 129.61670 124.41580 0.06547 0.34378 0.07500 0.87047
    \hat{\varphi} 2.50000 (0.5973595)
    \hat{a} 5.25000 (2.6300897)
    \hat{b} 0.09255 (1.2750272)
    Fr \hat{\theta} 5.43392 (0.50788) 121.80430 122.00430 126.09060 123.49010 0.11497 0.64202 0.10013 0.55274
    \hat{\lambda} 230.48644 (110.91778)
     | Show Table
    DownLoad: CSV

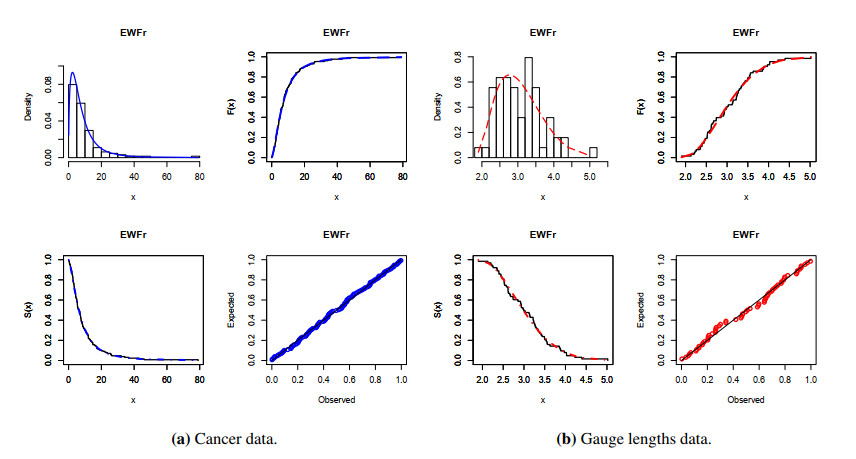
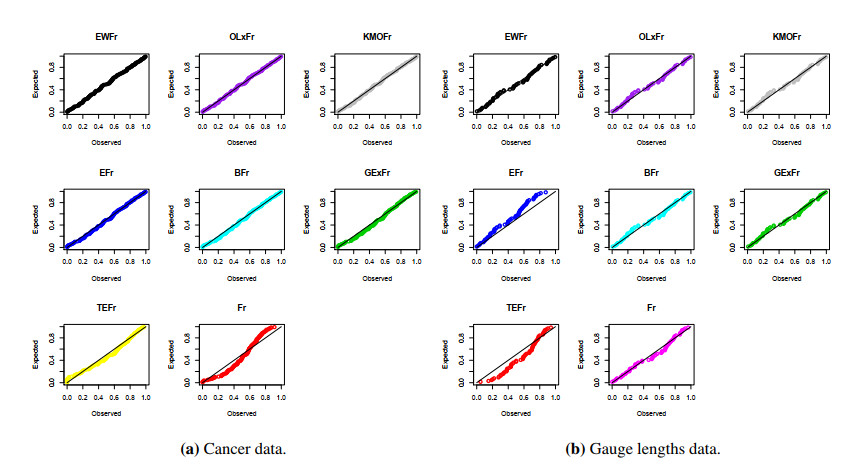
    Some plots including the PDF, CDF, and SF along with the PP plots of the EWFr model are displayed in Figure 3 for both datasets. The PP plots of all studied distributions are displayed in Figure 4 for the two datasets, respectively.

    Figure 3.  The fitted EWFr PDF, CDF, SF, and P-P plots of the EWFr distribution.
    DownLoad: Full-Size Img PowerPoint
    Figure 4.  The PP plots of the EWFr distribution and other distributions.
    DownLoad: Full-Size Img PowerPoint

    The estimates of the EWFr parameters under several estimation approaches and the goodness-of-fit measures for both datasets are listed in Tables 10 and 11, respectively. Based on the values of PV in Tables 10 and 11, the Anderson-Darling approach is recommended to estimate the EWFr parameters for cancer data, while the least-squares approach is recommended for gauge lengths data.

    Table 10.  The estimates of the EWFr parameters under several methods of estimation and analytical measures for cancer data.
    Methods \hat{\vartheta} \hat{\varphi} \hat{\lambda} \hat{\theta} W^{*} A^{*} KS PV
    MLEs 56.93661 0.46697 0.71825 0.01794 0.01991 0.13402 0.03757 0.99713
    LSEs 56.93660 0.55502 0.71932 0.01871 0.01850 0.12986 0.03334 0.99918
    MPSEs 56.93660 0.46698 0.71750 0.01717 0.01916 0.13069 0.03617 0.99708
    WLSEs 56.94615 0.51962 0.71884 0.01825 0.01815 0.12600 0.03251 0.99947
    CRVMEs 56.93659 0.55064 0.71962 0.01891 0.01795 0.12584 0.03352 0.99910
    ADEs 56.93668 0.47772 0.71887 0.01815 0.01763 0.12016 0.03167 0.99968
    PCEs 57.47389 0.81500 0.72219 0.02155 0.03320 0.23254 0.03830 0.99367
    RADEs 56.93805 0.56319 0.71967 0.01899 0.01830 0.12878 0.03385 0.99895
     | Show Table
    DownLoad: CSV
    Table 11.  The estimates of the EWFr parameters under several methods of estimation and analytical measures for gauge lengths data.
    \textbf{Methods} \hat{\vartheta} \hat{\varphi} \hat{\lambda} \hat{\theta} W^{*} A^{*} \textbf{KS} \textbf{PV}
    \textbf{MLEs} 0.76563 0.04349 145.67068 4.60846 0.04181 0.23988 0.06847 0.92920
    \textbf{LSEs} 0.69421 0.08110 145.67010 4.56661 0.04850 0.26973 0.06486 0.94154
    \textbf{MPSEs} 0.75534 0.13947 145.66920 4.65631 0.05531 0.29551 0.07780 0.81897
    \textbf{WLSEs} 0.71596 0.01530 145.66740 4.57637 0.04747 0.26675 0.06895 0.90999
    \textbf{CRVMEs} 0.71398 0.00100 145.67030 4.57259 0.04761 0.26713 0.06733 0.92336
    \textbf{ADEs} 0.72963 0.00010 145.67980 4.57988 0.04696 0.26562 0.07047 0.89642
    \textbf{PCEs} 0.75075 0.02596 145.67020 4.59649 0.04775 0.26737 0.07311 0.87060
    \textbf{RADEs} 0.74062 0.00010 145.66970 4.57722 0.04668 0.26516 0.07058 0.89538
     | Show Table
    DownLoad: CSV

    6.   Conclusions

    In this paper, we introduced a more flexible four-parameter model called the extended Weibull–Fréchet (EWFr) distribution. Its basic mathematical properties are explored. The EWFr parameters are estimated using eight classical estimation methods. The simulation results showed that the maximum product of spacings approach outperforms other considered methods based on overall ranks. The importance and flexibility of the EWFr distribution over some competing extensions of the Fréchet distribution are addressed by analyzing two real-life datasets from the medicine and engineering fields. The analytical measures showed that our EWFr model returned an adequate fit in comparison with other distributions.

    Acknowledgments

    This study was funded by Taif University Researchers Supporting Project number (TURSP-2020/279), Taif University, Taif, Saudi Arabia. The authors would like to thank the editorial board and the three referees for their constructive comments that improved the final version of the paper.

    Conflict of interest

    The authors declare no conflict of interest.



    [1] World Health Organization, Anti-tuberculosis Drug Resistance in the World: Third Global Report, No. WHO / HTM / TB / 2004. 343, World Health Organization, 2004.
    [2] J. Cohen, Extensively drug-resistant TB gets foothold in South Africa, Science 313 (2006), 1554.
    [3] L. B. Reichman, J. H. Tanne, Timebomb: The Global Epidemic of Multi-Drug Resistant Tuberculosis, 2002.
    [4] Y. Zhou, K. Khan, Z. Feng, et al. Projection of tuberculosis incidence with increasing immigration trends, J. Theor. Biol., 254 (2008), 215–228.
    [5] P. Rodrigues, M. G. M. Gomes, C. Rebelo, Drug resistance in tuberculosis: a reinfection model, Theor. Popul. Biol., 71 (2007), 196–212.
    [6] N. Blaser, C. Zahnd, S. Hermans, et al. Tuberculosis in Cape Town: an age-structured transmission model, Epidemics, 14 (2016), 54–61.
    [7] C. P. Bhunu, W. Garira, Z. Mukandavire, et al. Tuberculosis transmission model with chemoprophylaxis and treatment, B. Math. Biol., 70 (2008), 1163–1191.
    [8] Centers for Disease Control and Prevention, TB in Children in the United States, CDC, 2014. Available from: https://www.cdc.gov/tb/topic/populations/tbinchildren/default.htm.
    [9] S. M. Blower, P. M. Small, P. C. Hopewell, Control strategies for tuberculosis epidemics: new models for old problems, Science, 273 (1996), 497–500.
    [10] Fatmawati, H. Tasman, An optimal treatment control of TB-HIV coinfection, International Journal of Mathematics and Mathematical Sciences, 2016 (2016).
    [11] R. I. Hickson, G. N. Mercer, K. M. Lokuge, A metapopulation model of tuberculosis transmission with a case study from high to low burden areas, PLoS One, 7 (2012).
    [12] R. M. G. J. Houben, T. Sumner, A. D. Grant, et al. Ability of preventive therapy to cure latent Mycobacterium tuberculosis infection in HIV-infected individuals in high-burden settings, P. Natl. A. Sci., 111 (2014), 5325–5330.
    [13] R. Kaplan, J. Caldwell, K. Middelkoop, et al. Impact of ART on TB case fatality stratified by CD4 count for HIV-positive TB patients in Cape Town, South Africa (2009-2011), J. Acq. Imm. Def., 66 (2014), 487–494.
    [14] Fatmawati, U. D. Purwati, F. Riyudha, et al. Optimal control of a discrete age-structured model for tuberculosis transmission, Heliyon, 6 (2020).
    [15] S. G, Samko, A. A, Kilbas, O. I. Marichev, Fractional Integrals and Derivatives: Theory and Applications, Gordon and Breach Science Publishers, 1993.
    [16] I. Podlubny, Fractional Differential Equations, Academic Press, San Diego, California, USA, 1999.
    [17] T. Sardar, S. Rana, J. Chattopadhyay, A mathematical model of dengue transmission with memory, Commun. Nonlinear Sci., 22 (2015), 511–525.
    [18] J. Huo, H. Zhao, L. Zhu, The effect on backward bifurcation in a fractional orde HIV model, Nonlinear Analysis: Real World Applications, 26 (2015), 289–305.
    [19] M. Saeedian, M. Khalighi, N. Azimi-Tafreshi, et al. Memory effects on epidemic evolution: the susceptible-infected-recovered epidemic model, Phys. Rev. E., 95 (2017).
    [20] C. M. A. Pinto, A. R. M. Carvalho, The HIV/TB coinfection severity in the presence of TB multidrug resistant strains, Ecol. Complex., 32 (2017), 1–20.
    [21] Fatmawati, E. M. Shaiful, M. I. Utoyo, A fractional order model for HIV dynamics in a two-sex population, International Journal of Mathematics and Mathematical Sciences, 2018 (2018).
    [22] G. C. Wu, Z. G. Deng, D. Baleanu, et al. New variable-order fractional chaotic systems for fast image encryption, Chaos: An Interdisciplinary Journal of Nonlinear Science, 29 (2019).
    [23] G. C. Wu, T. Abdeljawad, J. Liu, et al. Mittag-Leffler stability analysis of fractional discrete-time neural networks via fixed point technique, Nonlinear Analysis: Modelling and Control, 24 (2019), 919–936.
    [24] G. C. Wu, D. Q. Zeng, D. Baleanu, Fractional impulisve differential equations: Exact solutions, integral equations and short memory case, Frac. Calc. Appl. Anal., 22 (2019), 180–192.
    [25] M. Itik, S. P. Banks, Chaos in a three-dimensional cancer model, Int. J. Bifurcat. Chaos, 20 (2010), 71–79.
    [26] M. Caputo, M. Fabrizio, A new definition of fractional derivative without singular kernel, Progr. Fract. Differ. Appl., 1 (2015), 1–13.
    [27] M. Caputo, M. Fabrizio, On the notion of fractional derivative and applications to the hysteresis phenomena, Meccanica, 52 (2017), 3043–3052.
    [28] T. Zhang, L. Xiong, Periodic motion for impulsive fractional functional differential equations with piecewise Caputo derivative, Appl. Math. Lett., 101 (2020), 106072.
    [29] A. Atangana, D. Baleanu, New fractional derivatives with nonlocal and non-singular kernel: theory and application to heat transfer model, Therm. Sci., 20 (2016).
    [30] A. Atangana, I. Koca, Chaos in a simple nonlinear system with Atangana-Baleanu derivatives with fractional order, Chaos, Solitons & Fractals, 89 (2016), 447–454.
    [31] A. Atangana, K. M. Owolabi, New numerical approach for fractional differential equations, Math. Model. Nat. Pheno., 13 (2018).
    [32] A. Atangana, J. F. Gomez-Aguilar, Decolonisation of fractional calculus rules: Breaking commutativity and associativity to capture more natural phenomena, The European Physical Journal Plus, 133 (2018).
    [33] M. A. Khan, S. Ullah, M. Farooq, A new fractional model for tuberculosis with relapse via Atangana-Baleanu derivative, Chaos, Solitons & Fractals, 116 (2018), 227–238.
    [34] E. Bonyah, Chaos in a 5-D hyperchaotic system with four wings in the light of non-local and non-singular fractional derivatives, Chaos, Solitons & Fractals, 116 (2018), 316–331.
    [35] K. Muhammad Altaf, A. Atangana, Dynamics of Ebola disease in the framework of different fractional derivatives, Entropy, 21 (2019).
    [36] R. Jan, M. A. Khan, P. Kumam, et al, Modeling the transmission of dengue infection through fractional derivatives, Chaos, Solitons & Fractals, 127 (2019), 189–216.
    [37] W. Wang, M. A. Khan, P. Kumam, et al. A comparison study of bank data in fractional calculus, Chaos, Solitons & Fractals, 126 (2019), 369–384.
    [38] M. A. Khan, The dynamics of a new chaotic system through the Caputo-Fabrizio and AtanaganBaleanu fractional operators, Adv. Mech. Eng., 11 (2019).
    [39] Fatmawati, M. A. Khan, M. Azizah, et al. A fractional model for the dynamics of competition between commercial and rural banks in Indonesia, Chaos, Solitons & Fractals, 122 (2019), 32–46.
    [40] S. Ullah, M. A. Khan, M. Farooq, et al. A fractional model for the dynamics of tuberculosis (TB) using Atangana-Baleanu derivative, Discrete Cont. Dyn. S, 13 (2019).
    [41] A. A. Velayati, Tuberculosis in children, International Journal of Mycobacteriology, 5 (2016).
    [42] C. Castillo-Chaves, B. Song, Dynamic models of tuberculosis and their applications, Math. Biosci. Eng., 1 (2004), 361–404.
    [43] Z. M. Odibat, N. T. Shawagfeh, Generalized Taylors formula, Appl. Math. Comput., 186 (2007), 286–293.
    [44] W. Lin, Global existence theory and chaos control of fractional differential equations, J. Math. Anal. Appl., 332 (2007), 709–726.
    [45] P. van den Driessche, J. Watmough, Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission, Math. Biosci., 180 (2002), 29–48.
    [46] K. Diethelm, N. J. Ford, A. D. Freed, A predictor-corrector approach for the numerical solution of fractional differential equations, Nonlinear Dynamics, 29 (2002), 3–22.
    [47] K. Diethelm, N. J. Ford, A. D. Freed, Detailed error analysis for a fractional Adams method, Numerical Algorithms, 36 (2004), 31–52.
    [48] C. P. Bhunu, Mathematical analysis of a three-strain tuberculosis transmission model, Appl. Math. Model., 35 (2011), 4647–4660.
    [49] S. Athithan, M. Ghosh, Optimal control of tuberculosis with case detection and treatment, World Journal of Modelling and Simulation, 11 (2015), 111–122.
    [50] Word Health Organization, Factsheet on the World Tuberculosis Report 2017, WHO, 2017. Available from: https://www.who.int/en/news-room/fact-sheets/detail/tuberculosis.
    [51] J. J. Tewa, S. Bowong, B. Mewoli, Mathematical analysis of two-patch model for the dynamical transmission of tuberculosis, Appl. Math. Model., 36 (2012), 2466–2485.
    [52] M. Toufik, A. Atangana, New numerical approximation of fractional derivative with non-local and non-singular kernel: application to chaotic models, The European Physical Journal Plus, 132 (2017), 444.
    [53] L. Xu, H. Hub, F. Qinc, Ultimate boundedness of impulsive fractional differential equations, Appl. Math. Lett., 62 (2016), 110–117.
    [54] L. Xu, J. Li, S. S. Ge, Impuls ivestabilization of fractional differential systems, ISA T., 70 (2017), 125–131.
    [55] L. Xu, X. Chu, H. Hu, Exponential ultimate boundedness of non-autonomous fractional differential systems with time delay and impulses, Appl. Math. Lett., 99 (2020), 106000.

  • This article has been cited by:

    1. Selasi Kwaku Ocloo, Lewis Brew, Suleman Nasiru, Benjamin Odoi, Jewgeni Dshalalow, Harmonic Mixture Fréchet Distribution: Properties and Applications to Lifetime Data, 2022, 2022, 1687-0425, 1, 10.1155/2022/6460362
    2. Xueyu Wu, Zubair Ahmad, Eslam Hussam, Marwan H. Alhelali, Ramy Aldallal, Muqrin A. Almuqrin, Fathy H. Riad, A new Cosine-Weibull model: Distributional properties with applications to basketball and medical sectors, 2023, 66, 11100168, 751, 10.1016/j.aej.2022.10.068
    3. Abdul Ghaniyyu Abubakari, Chin-Chia Wu, Actuarial Measures, Regression, and Applications of Exponentiated Fréchet Loss Distribution, 2022, 2022, 1687-0425, 1, 10.1155/2022/3155188
    4. Adam Braima S. Mastor, Oscar Ngesa, Joseph Mung’atu, Nada M. Alfaer, Ahmed Z. Afify, M. De Aguiar, The Extended Exponential Weibull Distribution: Properties, Inference, and Applications to Real-Life Data, 2022, 2022, 1099-0526, 1, 10.1155/2022/4068842
    5. Otieno Kevin Okumu, Omondi Joseph Ouno, Anthony Nyutu Karanjah, Samuel Nganga Muthiga, Three-parameters Gumbel Distribution: Formulation and Parameter Estimation, 2024, 10, 2472-3509, 25, 10.11648/j.ijsd.20241002.12
    6. Fatimah E. Almuhayfith, Talha Arslan, Hassan S. Bakouch, Aminh M. Alnaghmosh, A versatile model for lifetime of a component under stress, 2023, 13, 2045-2322, 10.1038/s41598-023-47313-3
    7. Hassan M. Aljohani, The single and double inverse Pareto–Burr XII distribution: Properties, estimation methods, and real-life data applications, 2024, 14, 2158-3226, 10.1063/5.0203919
    8. M. G. M. Ghazal, Yusra A. Tashkandy, Oluwafemi Samson Balogun, M. E. Bakr, Exponentiated extended extreme value distribution: Properties, estimation, and applications in applied fields, 2024, 9, 2473-6988, 17634, 10.3934/math.2024857
    9. Ahmed Z. Afify, Ekramy A. Hussein, Hazem Al-Mofleh, Rehab Alsultan, Hassan M. Aljohani, Nian-Sheng Tang, The Flexible Fréchet Distribution: Properties, Inference, and Medical Applications, 2024, 2024, 2314-4629, 10.1155/jom/2600560
    10. Hatem Semary, Ahmad Abubakar Suleiman, Aliyu Ismail Ishaq, Jamilu Yunusa Falgore, Umar Kabir Abdullahi, Hanita Daud, Mohamed A. Abd Elgawad, Mohammad Elgarhy, A new modified Sine-Weibull distribution for modeling medical data with dynamic structures, 2025, 18, 16878507, 101427, 10.1016/j.jrras.2025.101427
    11. Dawlah Alsulami, A new extension of the Rayleigh distribution: Properties, different methods of estimation, and an application to medical data, 2025, 10, 2473-6988, 7636, 10.3934/math.2025350
  • Reader Comments
  • © 2020 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)
通讯作者: 陈斌, bchen63@163.com
  • 1. 

    沈阳化工大学材料科学与工程学院 沈阳 110142

  1. 本站搜索
  2. 百度学术搜索
  3. 万方数据库搜索
  4. CNKI搜索
AIMS Mathematics
1.8 3.4

Metrics

Article views(7653) PDF downloads(954) Cited by(54)

Preview PDF
Download XML
Export Citation
Article outline
Abstract
   Introduction
   The EWFr distribution
   The EWFr characteristics
   Estimation and simulations
   Modeling medicine and engineering data
   Conclusions
Acknowledgments
Conflict of interest
References

Figures and Tables

Figures(11)  /  Tables(2)

Other Articles By Authors

Related pages

Tools

Copyright © AIMS Press

/

DownLoad:  Full-Size Img  PowerPoint
Return
Return

Catalog