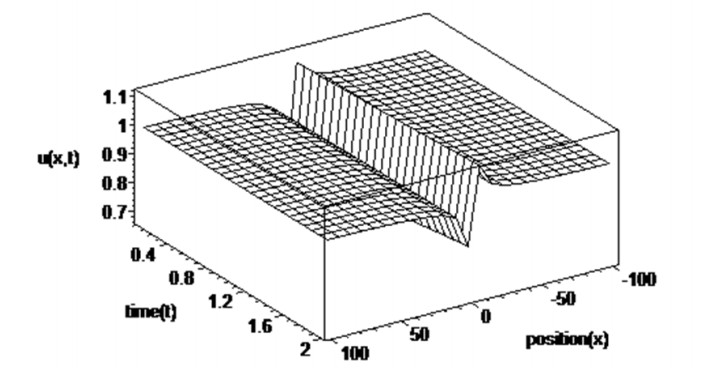
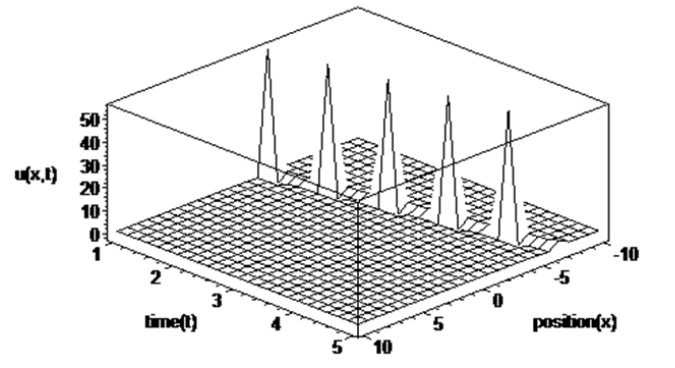
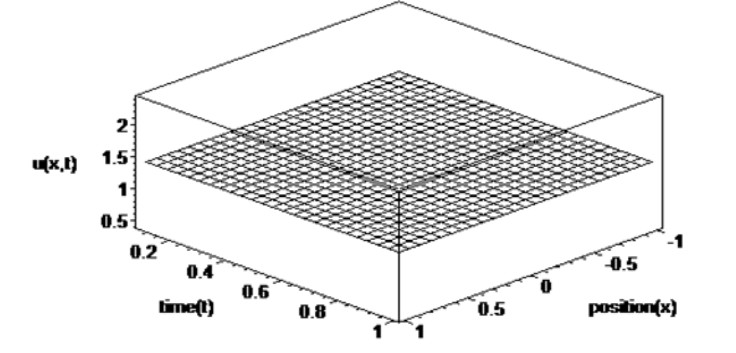
In this work, two algorithms namely, the generalized exp(-w(ξ)) and rational (G'/G2)-expansion methods are suggested for constructing new optical solitons solutions for the perturbed fractional nonlinear Schrodinger equation. The solutions include hyperbolic, trigonometric or rational function. Our results indicate that, group of new solutions are obtained with much reliability, accuracy and efficiency of the proposed methods. Eventually, our pending may become of wide relevance in addition to realize the main features and even propagation of the nonlinear waves in fractal medium.
1.
Introduction
Very recently, there are many models that describe the telecommunications industry, namely, nonlinear Schrodinger's equations, Manakov model (GLL) equation, Gerdjikov-Ivanov model (LPD) equation and many other models of special interest in nonlinear optics [1,2,3,4,5,6,7,8,9,10,11,12,13,14]. On the other hand, fractional calculus has a wide array of various applications in nonlinear science [15,16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32,33,34,35,36,37,38,39,40,41,42,43,44,45]. Schrodinger equation one of the important equations in the last century and widely used in the area of quantum mechanics and quantum optics. Several methods are used in solving Schrodinger equation including approximate, exact and numerical solutions, starting from the perturbation, variational and WKB methods [46,47,48,49,50,51]. Recently, there are many methods were introduced to solve this important equation. On the other hand, the Schrodinger equation and its solutions in the fractional from is studied in many papers [28,29,30,31,32,33]. This paper studies the possibility to find solutions for perturbed fractional time nonlinear Schrodinger equation. In this study, two algorithms schemes are used for constructing the optical solitons solutions to the mentioned model. They are generalized exp(-w(ξ)) method and rational (G'/G2) expansion method.
The outlines of this work are given as: Section 2 presents the fractional calculus of conformable fractional derivatives and some properties; section 3.A, 3.B introduce the two integrations schemes; sections 4.A, 4.B present the new solitons solutions of Eq (13); and the last section is the conclusion.
2.
Preliminaries
The derivative used in this paper is, the conformable derivative of order α and can be defined as: Defintion: Let g:(0, ∞) → R, flowing are definition, properties and theorem about used conformable derivatives [22]:
If g is differentiable, then U_{α} (g)(t) = t1-α (dg/dh).
Theorem: Let, g:(0, ∞) → R be differentiable and α differentiable function, then
The time fractional nonlinear Schrodinger equation with temporal evolution is given in its dimensionless as follows [9].
In Eq (7) γ1 represents dispersion term, γ2 is nonlinear dispersion andγ3 is the nonlinear dispersion term.
To gain the optical soliton solutions of the Eq (7). With the aid of the hypothesis as [23,24,25,26,27,28].
Then Eq (7) reads
Where V is a complex function defines as
Where L is a constant, and U(ξ) is a real function. Making use Eq (10), then Eq (9) yields
Equation (12), can be rewritten as:
3.
A quick of two algorithms schemes
Given the general fractional nonlinear evolution equation as follows:
Making use of e fractional complex transformation as:
Where k and c are to be determined later, with
Where σx' = σt' = l, where l is a constant. Then Eq (14) becomes
3.1. The exp(-wξ)-expansion method
In view of this method [24], we express the solution of Eq (17) as:
Where αi,βj are constants to be specified later, and w(ξ) satisfies:
Where λ and μ are constants to be evaluated later. The general solutions of Eq (19) reads
Case Ⅰ: when δ=λ2−4μ>0 and μ≠0, gain the hyperbolic function solution is
Case Ⅱ: If δ=λ2−4μ<0 and, μ≠0, then the trigonometric function solution is:
Case Ⅲ: If λ2−4μ>0,μ=0 and λ≠0, then the hyperbolic function solution
Case Ⅳ: If λ2−4μ=0,μ≠0 and λ≠0 gain the rational function solution is
Case Ⅴ: If λ2−4μ=0,μ≠0 and λ≠0, implies to the rational function solution
Where c1 is constant of integration, ξ=kxβΓ(1+β)+ctαΓ(1+α).
With the aid of Eq (18) and Eq (19) into Eq (13), yields the coefficients ofexp(−w(ξ)) to zero, gives a set of algebraic equations which can be solved to find αi, βi μ, λ, c, k. Inserting these values in Eq (18), then the new optical soliton solutions of Eq (14) are obtained.
3.2. The extended rational (G'/G2) expansion method
In view of the (G′G2)-expansion method [36,37,38,39]. The quick gain of this method the solution of Eq (17) can be expressed as
Where ψ(ξ)=(G′(ξ)G2(ξ)) satisfies
Where λ≠0 and μ≠1 and a0, ai, bj to be determined. In terms of the general solutions of Eq (19) which can be classified as:
Case Ⅰ: Trigonometric function solution for λμ > 0
Case Ⅱ: Hyperbolic function solution for λμ < 0, then
Case Ⅲ: Rational function solution for λ≠0 and μ = 0, then
Where k1 and k2 are constants.
Step 2: The integer N and M in Eq (25) and Eq (18) are determined by balancing between the highest order derivative via
Where α, q, β are real constant.
Step 3: Making use Eq (25) with Eq (26) in Eq (13), collecting the same power ψi(ξ), and to zero, we gain a system of algebraic equations, solved it for values of ai, bj, c. Then optical soliton solutions of Eq (24) are given.
4.
Optical soliton solutions of perturbed time FNSE
4.1. Optical soliton solutions using the generalized exp(-w(ξ)) method
Consequently, to solve Eq (13) usingexp(-wξ) method [30], considering the balance principle to Eq (13), we obtain N = M + 1. By taking M = 1, then N = 2. Then the solution of Eq (13) admits to:
Inserting Eq (32) into Eq (13) with Eq (19), collecting all power of exp(-φ(ξ)), and using symbolic computation program gives,
Case Ⅰ:
Case Ⅱ:
Case Ⅲ:
In view of Eq (33) with Eqs (20-24) into Eq (32), admits to the following solutions as
By using Eq (34) with Eqs (20-24) into Eq (32), we gain the following exact solutions:
According to (Case Ⅲ) with Eqs (20-24) into Eq (32), admits to solutions of Eq (7) as
Where wi(ξ), i = 1, …, 5 are given in Eqs (20-24),
It is worth noting that all explored solutions Eqs (36-40) presents optical soliton solutions to Eq (7).
4.2. Optical soliton solutions via rational (G′G2) expansion method
This section, is devoted for obtaining the optical soliton solutions of Eq (13) via the rational (G′G2) method [34,35]. Consider the balance between U'', and U3, we get N = M + 1. For M = 1, we have N = 2. Therefore the solutions of Eq.(25) becomes
Eqs (41) is employed to Eqs (13) having the same power of ψ(ξ) and equating to zero, we have a set of algebraic equations. By solving it, we obtain
Case Ⅰ:
Subsequently, in view of Eq (42) with Eqs (27-29) into Eq (41), admits to the solutions of Eq. (7) as
ψi(ξ)=(G′i(ξ)G2i(ξ)),ξ=ax+btαΓ(1+α),(i=1,2,3) are given in Eqs (27-29).
Case Ⅱ:
In view of Eq (45) with (27-29) into Eq (41), we gain the new solutions for Eq (7) as
ψi(ξ)=(G′i(ξ)G2i(ξ)),ξ=ax+btαΓ(1+α),(i=1,2,3) are given in Eqs (27-29).
Case Ⅲ:
Making use Eq (48) with Eqs (27-29) into Eq (41), we write down explicitly the following solutions of Eq (7) as
ψi(ξ)=(G′i(ξ)G2i(ξ)),ξ=ax+btαΓ(1+α),(i=1,2,3) are given in Eqs (27-29).
Case Ⅳ:
In view of Eq (51) with Eqs (27-29) into Eq (41), we obtain
ψi(ξ)=(G′i(ξ)G2i(ξ))ξ=ax+btαΓ(1+α),(i=1,2,3) are given in Eqs (27-29).
Thus, the optical solutions of Eq (7) can be captured by Eq (49-53), under the constraints Eq (10) via V(ξ) = eiLξU(ξ).
5.
Conclusions
In this work, the generalized exp(-w(ξ)) and rational (G′G2) expansion methods have been applied for finding the new optical soliton solutions for the perturbed time fractional nonlinear Schrodinger equation with the help of conformable fractional derivative. The results are group of new solutions of Schrodinger equation, where the proposed methods proved better reliability, accuracy and efficiency. The obtained results and figures (1-4) conclude that, the fractional parameter α plays the main rule in the solutions, for example if we substituted by α = 1, the solutions become the same with the obtained by the normal derivative.
Conflict of interest
The authors declare that there is no conflict of interest in this paper.
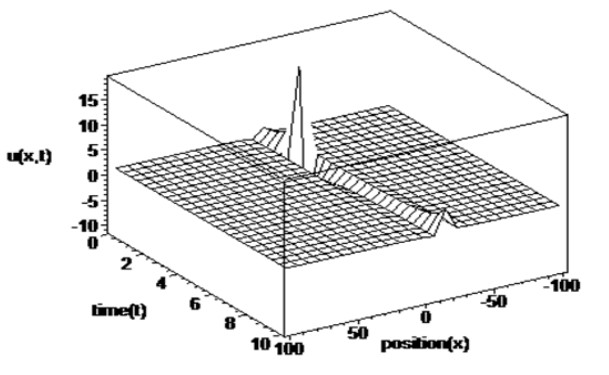









 DownLoad:
DownLoad: