Citation: Muhsin Incesu. LS (3)-equivalence conditions of control points and application to spatial Bézier curves and surfaces[J]. AIMS Mathematics, 2020, 5(2): 1216-1246. doi: 10.3934/math.2020084
| [1] | Xuanyi Zhao, Jinggai Li, Shiqi He, Chungang Zhu . Geometric conditions for injectivity of 3D Bézier volumes. AIMS Mathematics, 2021, 6(11): 11974-11988. doi: 10.3934/math.2021694 |
| [2] | Joseph Lifton, Tong Liu, John McBride . Non-linear least squares fitting of Bézier surfaces to unstructured point clouds. AIMS Mathematics, 2021, 6(4): 3142-3159. doi: 10.3934/math.2021190 |
| [3] | Qi Xie, Yiting Huang . A class of generalized quadratic B-splines with local controlling functions. AIMS Mathematics, 2023, 8(10): 23472-23499. doi: 10.3934/math.20231193 |
| [4] | Yujun Li . Characteristics of planar sextic indirect-PH curves. AIMS Mathematics, 2024, 9(1): 2215-2231. doi: 10.3934/math.2024110 |
| [5] | Syed Ahmad Aidil Adha Said Mad Zain, Md Yushalify Misro . A novel technique on flexibility and adjustability of generalized fractional Bézier surface patch. AIMS Mathematics, 2023, 8(1): 550-589. doi: 10.3934/math.2023026 |
| [6] | Salwa Syazwani Mahzir, Md Yushalify Misro, Kenjiro T. Miura . Preserving monotone or convex data using quintic trigonometric Bézier curves. AIMS Mathematics, 2024, 9(3): 5971-5994. doi: 10.3934/math.2024292 |
| [7] | Samia BiBi, Md Yushalify Misro, Muhammad Abbas . Smooth path planning via cubic GHT-Bézier spiral curves based on shortest distance, bending energy and curvature variation energy. AIMS Mathematics, 2021, 6(8): 8625-8641. doi: 10.3934/math.2021501 |
| [8] | Haiming Liu, Jiajing Miao . Geometric invariants and focal surfaces of spacelike curves in de Sitter space from a caustic viewpoint. AIMS Mathematics, 2021, 6(4): 3177-3204. doi: 10.3934/math.2021192 |
| [9] | Justin Tzou, Brian Wetton . Optimal covering points and curves. AIMS Mathematics, 2019, 4(6): 1796-1804. doi: 10.3934/math.2019.6.1796 |
| [10] | Ayman Elsharkawy, Clemente Cesarano, Abdelrhman Tawfiq, Abdul Aziz Ismail . The non-linear Schrödinger equation associated with the soliton surfaces in Minkowski 3-space. AIMS Mathematics, 2022, 7(10): 17879-17893. doi: 10.3934/math.2022985 |
In mechanics the concept of similarity is mostly used in development of dimensional analysis. Dimensional analysis arose from an attempt to extend to physics some concepts like similarity, ratio, and proportion [1,2]. It was first applied by Galileo in 1638 to predict the strength of beams of given material as a function of linear dimensions [1]. Other applications were given by Mariotte in 1679 and Newton in 1686 [1]. However, Fourier stated for the first time in 1822 that all physical quantities are expressed in a number of fundamental units and that the conversion of physical systems to other physical systems is possible by the conversion of these fundamental units to each other [1,2].
Developments in invariant theory at the end of the 19th century have affected different areas of mathematics. The basis of invariant theory studies is the problem of examining whether the invariant polynomial ring R[x]G has finite generator or not [3]. After David Hilbert M. Nagata showed that the ring R[x]G has finite generators if G is linear reductive [4]. In case G is not a linear reductive group, how conditions of that the ring R[x]G has finite generators are given in [5,6].
Until F. Klein, only certain geometries were known. In 1872, Klein showed that groups are important building blocks of geometry in his Erlangen Programme. According to Klein two elements A and B are equivalent if and only if there exist a transformation f such that B=f(A) is satisfied [7]. In the 20th century Bridgman [8], Sedov [9], and Langhaar [10] are some contributors in this area. In 1946, Herman Weyl gave the complete invariant system of points for real n dimensional orthogonal group O(n) in [11].
Some applications of invariant theory for various groups to points systems and the differential curves are given in [12,13,14,15,16,17,18,19,20] recently.
Computer aided design (CAD) is based on parametric curves and parametric surfaces. The most important tools in computer aided geometric design (CAGD) are Bézier Curves and surfaces, Coons patches and then B-spline methods [21]. The CAGD has gained discipline in itself under the leadership of Barnhill and Riesenfeld, following a conference at the University of Utah in 1974 in the United States [22].
The best examples of points systems are Bézier curves and Bézier surfaces. The invariants of these curves and surfaces under an affine transformation have the same meaning as the invariants of the control points of these curves and surfaces. Therefore it is necessary that the invariant properties of modelling of mechanism allow durable and precision, and reliable results under transformation groups.In this sense, knowing of complete of invariants of Bézier and B-spline curves and surfaces which gives most stable solutions within CAD systems [23] is important to apply it to different areas.
Bézier curves and surfaces have been used in a wide range of applications from automotive industry to aircraft industry. Many example i.e. on offset surfaces, G. Farin [24], R. Farouki [25], J. Hoschek [26], W. Tiller [27], H. Potmann [28]; on different surfaces and conics, R. Farouki and Carla Manni [29], F. Chen [30], H. Pottmann [31], R. Krasauskas [32]; on the properties of trigonometric functions, Reyes [33]; on kinematics, B. Jüttler [34], Q.J. Ge [35], B. Roth [36] and on the application of heat transfer by the limited element method R. Cholewa et al. [37] can be given as examples of using Bézier curves and surfaces.
Recently, many of studies have been studied on Bézier curves and surfaces and their CAD CAM applications. There are some examples in [38,39,40,41,42,43,44,45,46,47,48,49,50,51,52].
Let G be a transformation group and act on X. Any elements x,y∈X are G−equivalent elements if there exist a transformation g∈G such that y=gx is satisfied. Similarly Let A={x1,x2,...,xn} and B={y1,y2,...,yn} be any two subspaces of X with n−elements. In this case the subspaces A and B are G−equivalent if there exist a transformation g∈G such that yi=gxi is satisfied for every i=1,2,...,n.
When planar points systems be given, for the group G=LS(2), the generators of G−invariant rational functions and the G−equivalence conditions of these points systems were given in terms of the generators invartiants of these systems in R2 in [13].
This paper presents the G−equivalence conditions of the subspaces A and B with m−elements in 3−dimensional Euclidean space E3 under the transformation group G=LS(3) which is the linear similarity transformation group in E3 in section 3. So while in [13] only coplanar points are discussed, but in this paper, the G=LS(3)-equivalence conditions are given in terms of their rational G=LS(3) invariants for non-coplanar points.
In section 4 and section 5, the G=LS(3)−equivalence conditions of spatial Bézier curves and Bézier surfaces in terms of their rational G=LS(3) invariants of the control points of these curves and surfaces as the application of the G=LS(3)−equivalence conditions of points sets to curves and surfaces which are geometric structures are given.
In addition in section 6, the font design, which is an important application of Bézier curves and surfaces in CAD systems, is discussed. By using quadratic Bézier curves, a simple letter s is designed and two different shadow curves of this letter (composite curves) are obtained. As well It is emphasized that the only requirement that the obtained curves are shadow or other type of the designed letter should be G=LS(3)−equivalent.
As well known in linear algebra, a linear transformation between two vector spaces V and W is a map T:V→W such that the following hold:
T(v1+v2)=T(v1)+T(v2) and T(αv)=αT(v)
for any vectors v1 and v2 in V and for any scalar α∈F.
Let E be a three dimensional Euclidean space then the transformation F:E↦E is called a similarity transformation if there exist a positive real number λ such that ‖F(x)−F(y)‖=λ‖x−y‖ is satisfied for every x,y∈E.
The homotethy transformation in three dimensional Euclidean space E is defined by F:E↦E such that F(x)=a+λ(x−a) for every x∈E and for λ∈R, where a is called the center of the homotethy F.
The group of all the orthogonal transformations defined in 3-dimensional Euclidean space E is denoted by O(3,E). For shortness this group is denoted by O(3). The group of all the rotations is denoted by SO(3). Accordingly the linear homotethy transformation F in three dimensional Euclidean space E is defined by F(x)=λx for every x∈E.
Since a linear similarity transformation is composed of multiplication of two transformations: A linear homothety and a linear isometry or an orthogonal transformation, any linear similarity transformation F:E↦E in three dimensional Euclidean space E can be stated as
| F(x)=λgx | (2.1) |
where g∈O(3) [13].
Let G be any transformation group. Then, the function f is called G− invariant function, if
| f(gx)=f(x) | (2.2) |
for every x∈E and every g∈G.
Let R[x(1),x(2),...,x(m)] be a ring of polynomials for m vector variables x(1),x(2),...,x(m) in 3- dimensional Euclidean space R3 over the field R and a transformation group G be given. Then the algebra of G−invariant polynomials for m vector variables x(1),x(2),...,x(m) in 3-dimensional Euclidean space R3 over the field R is denoted by R[x(1),x(2),...,x(m)]G.
Theorem 2.1. Let x(1),x(2),...,x(m) be m vector variables in 3- dimensional Euclidean space R3. Then, the system of functions
| ⟨x(i),x(j)⟩;i,j=1,2,...,m;i≤j | (2.3) |
is the generator system of the algebra R[x(1),x(2),...,x(m)]O(3).
Proof: See, [11].
Theorem 2.2. Let x(1),x(2),...,x(m) be m vector variables in 3- dimensional Euclidean space R3. If m>3 then, for i,j=1,2,3 and p=4,...,m the generator system of the field of the O(3)-invariant rational functions R(x(1),x(2),...,x(m))O(3) is as follows
| ⟨x(i),x(j)⟩;i≤j,⟨x(i),x(p)⟩; | (2.4) |
If m≤3 then, the generator system of the field R(x(1),x(2),...,x(m))O(3) is the same as the system (2.3).
Proof: See, [11].
The generators of any G-invariant polynomial ring or the generators of any field of G-invariant rational functions are also the generator invariants. So any G-invariant function is stated as a function of these generators.
Any LS(3)−invariant polynomial function is constant [3]. So it is stated here only LS(3)−invariant rational functions.
Theorem 2.3. Let x(1),x(2),...,x(m) be m vector variables different from zero in 3-dimensional Euclidean space R3. If m>3 then, for i,j=1,2,3 and p=4,...,m the generator system of the field R(x(1),x(2),...,x(m))LS(3) is as follows
| ⟨x(i),x(j)⟩⟨x(1),x(1)⟩;i≤j,⟨x(i),x(p)⟩⟨x(1),x(1)⟩; | (2.5) |
If m≤3 then, the generator system of the field R(x(1),x(2),...,x(m))LS(3) is the system
| ⟨x(i),x(j)⟩⟨x(1),x(1)⟩,i≤j, | (2.6) |
Proof: See, [53].
Let G be a transformation group and E be a three dimensional Euclidean space. Then, the points x,y∈E are called G-equivalent points if there exist a transformation g∈G such that y=gx satisfies. If x and y are G-equivalent points then the notation xG≅y is used.
Let G be a transformation group and E be a three dimensional Euclidean space and two points systems {x(1),x(2),...,x(m)} and {y(1),y(2),...,y(m)} in E be given. Then, these systems are called G-equivalent if there exist a transformation g∈G such that y(i)=gx(i) satisfies for every i∈{1,2,...,m}. If these points systems {x(1),x(2),...,x(m)} and {y(1),y(2),...,y(m)} are G-equivalent systems then the notation {x(1),x(2),...,x(m)}G≅{y(1),y(2),...,y(m)} is used.
Theorem 3.1. Let two vectors x=(x1,x2,x3) and y=(y1,y2,y3) be given in R3. Then,
1. if x=0 and y≠0 or x≠0 and y=0 then x and y are not LS(3)-equivalent. ie. x
2. if x = 0 and y = 0 or x\neq 0 and y\neq 0 then x \overset{LS(3)}{\cong } y is always satisfied.
Proof.
1. Let x\neq 0 and y = 0 be given and x \overset{LS(3)}{ \cong } y be supposed. In this case there exist a transformation h \in LS(3) such that y = hx is satisfied. It means there exist g \in O(3) and \lambda > 0 such that y = \lambda gx is satisfied. Since the orthogonal transformations save the inner product, \left\langle y, y\right\rangle = \lambda^{2}\left\langle x, x\right\rangle is obtained. So because of x\neq 0 and \lambda > 0 , the inner product \left\langle y, y\right\rangle must be different zero and y can not be 0 vector. This is a contradiction. So x and y are not LS(3) -equivalent vectors. similarly in case x = 0 and y\neq 0 the statement can be reduced first case since the relationship \overset{G}{\cong } is an equivalence relation and has symmetry property.
2. Let x = (0, 0, 0) and y = (0, 0, 0) be given. In case gx = 0 for every g \in O(3) and y = \lambda gx can be stated since \lambda gx = 0 Thus from (2.2) x \overset{LS(3)}{ \cong } y is proved.
Let x\neq 0 and y\neq 0 be given. Then \left\langle x, x\right\rangle and \left\langle y, y\right\rangle are different from zero. Thus, the positive real number \lambda can be chosen as \lambda = \frac{\left\langle y, y\right\rangle}{\left\langle x, x\right\rangle} . So \left\|y\right\| = \left\| \lambda x \right\| is obtained. In this case the vectors y and \lambda x are on the same O(3) -orbit. It means there exist an orthogonal transformation g \in O(3) such that y = g (\lambda x) = \lambda gx satisfies. From this, x \overset{LS(3)}{ \cong } y is proved.
Theorem 3.2. Let two points systems \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} and \left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} in E be given. So,
1. if x^{(i)} = 0 and y^{(i)}\neq 0 or x^{(i)}\neq 0 and y^{(i)} = 0 for any i = 1, 2, ..., m , then these systems are not LS(3) - equivalent. ie. \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \ncong } \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}
2. if x^{(i)} = 0 and y^{(i)} = 0 for any i = 1, 2, ..., m , then the equivalence conditions of the systems with m vectors is reduced to the equivalence conditions with m-1 vectors.
3. if x^{(i)}\neq 0 and y^{(i)}\neq 0 for every i = 1, 2, ..., m , then
(a) if the ranks of the matrices \left[x^{(1)} x^{(2)}... x^{(m)} \right] and \left[y^{(1)} y^{(2)}... y^{(m)} \right] are equal to 3 then \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \cong } \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} if and only if \frac{ \left\langle x^{(i)}, x^{(j)} \right\rangle}{\left\langle x^{(1)}, x^{(1)}\right\rangle} = \frac{\left\langle y^{(i)}, y^{(j)} \right\rangle}{ \left\langle y^{(1)}, y^{(1)} \right\rangle} and \frac{\left\langle x^{(i)}, x^{(p)} \right\rangle}{\left\langle x^{(1)}, x^{(1)} \right\rangle} = \frac{\left\langle y^{(i)}, y^{(p)} \right\rangle}{\left\langle y^{(1)}, y^{(1)} \right\rangle} where i, j = 1, 2, 3 and i\leq j and p = 4, ..., m .
(b) if the ranks of the matrices \left[x^{(1)} x^{(2)}... x^{(m)} \right] and \left[y^{(1)} y^{(2)}... y^{(m)} \right] is equal to 2 then \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \cong } \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} if and only if \frac{ \left\langle x^{(i)}, x^{(j)} \right\rangle}{\left\langle x^{(1)}, x^{(1)} \right\rangle} = \frac{\left\langle y^{(i)}, y^{(j)} \right\rangle}{ \left\langle y^{(1)}, y^{(1)} \right\rangle} where i = 1, 2 and j = 2, 3, ..., m
(c) if the ranks of the matrices \left[x^{(1)} x^{(2)}... x^{(m)} \right] and \left[y^{(1)} y^{(2)}... y^{(m)} \right] is equal to 1 then \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \cong }\left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} if and only if \frac{\left\langle x^{(1)}, x^{(j)} \right\rangle}{\left\langle x^{(1)}, x^{(1)} \right\rangle} = \frac{\left\langle y^{(1)}, y^{(j)} \right\rangle}{ \left\langle y^{(1)}, y^{(1)} \right\rangle} where j = 2, 3, ..., m is satisfied.
(d) if the rank of the matrix \left[x^{(1)} x^{(2)}... x^{(m)} \right] is different from the rank of the matrix \left[y^{(1)} y^{(2)}... y^{(m)} \right] then these system can not be LS(3) -equivalent.
Proof.
1. Let x^{(i)} = 0 and y^{(i)}\neq 0 be given for any i = 1, 2, ..., m and \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\}\overset{LS(3)}{ \cong } \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} be supposed. Then there exist a scalar \lambda > 0 and an orthogonal transformation g \in O(3) such that y^{(j)} = \lambda gx^{(j)} for every j = 1, 2, ..., m is satisfied. So y^{(i)} must be 0 since x^{(i)} = 0 . This is a contradiction. Thus these systems \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} and \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} are not LS(3) - equivalent. Similarly in case x^{(i)}\neq 0 and y^{(i)} = 0 for any i = 1, 2, ..., m , then we will prove that these systems \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} and \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} are not LS(3) - equivalent. Suppose that these systems are LS(3) - equivalent. Then, there exist a \lambda > 0 and g \in O(3) such that y^{(j)} = \lambda gx^{(j)} for every j = 1, 2, ..., m is satisfied. From this equation for the integer i , x^{(i)} = \frac{1}{\lambda } g^{T}y^{(i)} can be written. Then x^{(i)} must be 0 Since y^{(i)} = 0 . This is a contradiction. So these systems \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} and \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} are not LS(3) -equivalent.
2. Let x^{(i)} = 0 and y^{(i)} = 0 for any i = 1, 2, ..., m be given and \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \cong } \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} be supposed. Then, y^{(j)} = \lambda gx^{(j)} for every j = 1, 2, ..., m is satisfied. Excluding the i th elements of these systems y^{(j)} = \lambda gx^{(j)} for every j = 1, 2, ..., i-1, i+1, ..., m is satisfied. So \left\{x^{(1)}, ..., x^{(i-1)}, x^{(i+1)}, ..., x^{(m)}\right\}\overset{LS(3)}{\cong }\left\{y^{(1)}, ..., y^{(i-1)}, y^{(i+1)}, ..., y^{(m)}\right\} can be written. Conversely let \left\{x^{(1)}, x^{(2)}, ..., x^{(i-1)}, x^{(i+1)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \cong }\left\{y^{(1)}, y^{(2)}, ..., y^{(i-1)}, y^{(i+1)}, ..., y^{(m)}\right\} be supposed. Then, there exist a scalar \lambda > 0 and g \in O(3) such that y^{(j)} = \lambda gx^{(j)} for every j = 1, 2, ..., i-1, i+1, ..., m . The i th elements of these systems can be added here since x^{(i)} = 0 and y^{(i)} = 0 So y^{(j)} = \lambda gx^{(j)} for every j = 1, 2, ..., m is satisfied then \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \cong } \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} is obtained.
3. (a) Let x^{(i)}\neq 0 and y^{(i)}\neq 0 for every i = 1, 2, ..., m and the ranks of the matrices \left[x^{(1)}x^{(2)}...x^{(m)}\right] and \left[y^{(1)}y^{(2)}...y^{(m)}\right] are equal to 3 be given and \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} be supposed. In this case there exist a scalar \lambda > 0 and g\in O(3) such that y^{(j)} = \lambda gx^{(j)} satisfies for every j = 1, 2, ..., m . Because the orthogonal transformation g\in O(3) save inner product, this equality
| \begin{equation*} \frac{\left\langle y^{(i)}, y^{(j)}\right\rangle }{\left\langle y^{(1)}, y^{(1)}\right\rangle } = \frac{\left\langle \lambda gx^{(i)}, \lambda gx^{(j)}\right\rangle }{\left\langle \lambda gx^{(1)}, \lambda gx^{(1)}\right\rangle } = \frac{\left\langle x^{(i)}, x^{(j)}\right\rangle }{ \left\langle x^{(1)}, x^{(1)}\right\rangle } \end{equation*} |
is obtained.
Conversely for every i, j = 1, 2, 3 , p = 4, ..., m . and i\leq j let this equalities \frac{\left\langle x^{(i)}, x^{(j)}\right\rangle }{\left\langle x^{(1)}, x^{(1)}\right\rangle } = \frac{\left\langle y^{(i)}, y^{(j)}\right\rangle }{\left\langle y^{(1)}, y^{(1)}\right\rangle } and \frac{\left\langle x^{(i)}, x^{(p)}\right\rangle }{\left\langle x^{(1)}, x^{(1)}\right\rangle } = \frac{\left\langle y^{(i)}, y^{(p)}\right\rangle }{\left\langle y^{(1)}, y^{(1)}\right\rangle } be given. It will be proved that \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} . Now from hyphothesis for every i, j = 1, 2, 3 , p = 4, ..., m
| \begin{eqnarray*} \left\langle y^{(i)}, y^{(j)}\right\rangle & = &\frac{\left\langle y^{(1)}, y^{(1)}\right\rangle }{\left\langle x^{(1)}, x^{(1)}\right\rangle } \left\langle x^{(i)}, x^{(j)}\right\rangle \\ \left\langle y^{(i)}, y^{(p)}\right\rangle & = &\frac{\left\langle y^{(1)}, y^{(1)}\right\rangle }{\left\langle x^{(1)}, x^{(1)}\right\rangle } \left\langle x^{(i)}, x^{(p)}\right\rangle \end{eqnarray*} |
can be written. So if the multiple \frac{\left\langle y^{(1)}, y^{(1)}\right\rangle }{\left\langle x^{(1)}, x^{(1)}\right\rangle } > 0 is denoted by \lambda ^{2} , then
| \begin{eqnarray} \left\langle y^{(i)}, y^{(j)}\right\rangle & = &\left\langle \lambda x^{(i)}, \lambda x^{(j)}\right\rangle \end{eqnarray} | (3.1) |
| \begin{eqnarray} \left\langle y^{(i)}, y^{(p)}\right\rangle & = &\left\langle \lambda x^{(i)}, \lambda x^{(p)}\right\rangle \end{eqnarray} | (3.2) |
is obtained for every i, j = 1, 2, 3 , p = 4, ..., m and i\leq j . Since the the ranks of the matrices \left[x^{(1)}x^{(2)}...x^{(m)}\right] and \left[y^{(1)}y^{(2)}...y^{(m)}\right] are equal to 3, m\geq 3 must be chosen. if m = 3 then since the vector systems \left\{ x^{(1)}, x^{(2)}, x^{(3)}\right\} and \left\{ y^{(1)}, y^{(2)}, y^{(3)}\right\} in three dimensional Euclidean space are linearly independent, the Gram determinant of the vectors \lambda x^{(1)} , \lambda x^{(2)} and \lambda x^{(3)}
| \begin{eqnarray*} \left\vert Gr\left( \lambda x^{(1)}, \lambda x^{(2)}, \lambda x^{(3)}\right) \right\vert & = &\left\vert \begin{array}{lll} \left\langle \lambda x^{(1)}, \lambda x^{(1)}\right\rangle & \left\langle \lambda x^{(1)}, \lambda x^{(2)}\right\rangle & \left\langle \lambda x^{(1)}, \lambda x^{(3)}\right\rangle \\ \left\langle \lambda x^{(2)}, \lambda x^{(1)}\right\rangle & \left\langle \lambda x^{(2)}, \lambda x^{(2)}\right\rangle & \left\langle \lambda x^{(2)}, \lambda x^{(3)}\right\rangle \\ \left\langle \lambda x^{(3)}, \lambda x^{(1)}\right\rangle & \left\langle \lambda x^{(3)}, \lambda x^{(2)}\right\rangle & \left\langle \lambda x^{(3)}, \lambda x^{(3)}\right\rangle \end{array} \right\vert \\ & = &\left\vert Gr\left( y^{(1)}, y^{(2)}, y^{(3)}\right) \right\vert \end{eqnarray*} |
is different from zero. Since
| \begin{equation} \left[ y^{(1)}y^{(2)}y^{(3)}\right] ^{T}\left[ y^{(1)}y^{(2)}y^{(3)}\right] = Gr\left( y^{(1)}, y^{(2)}, y^{(3)}\right) \end{equation} | (3.3) |
From (3.1) the equality of the matrices
| \begin{equation} \left[ \lambda x^{(1)}\lambda x^{(2)}\lambda x^{(3)}\right] ^{T}\left[ \lambda x^{(1)}\lambda x^{(2)}\lambda x^{(3)}\right] = \left[ y^{(1)}y^{(2)}y^{(3)}\right] ^{T}\left[ y^{(1)}y^{(2)}y^{(3)}\right] \end{equation} | (3.4) |
is obtained. Since the matrices \left[\lambda x^{(1)}\lambda x^{(2)}\lambda x^{(3)}\right] and \left[y^{(1)}y^{(2)}y^{(3)}\right] are regular and have inverses, there exist a regular matrix g such that
| \begin{equation} \left[ y^{(1)}y^{(2)}y^{(3)}\right] = g\left[ \lambda x^{(1)}\lambda x^{(2)}\lambda x^{(3)}\right] \end{equation} | (3.5) |
satisfies. If the equality (3.5) Substitutes to (3.4) and multiply both sides of (3.4) by \left(\left[\lambda x^{(1)}\lambda x^{(2)}\lambda x^{(3)}\right] ^{T}\right) ^{-1} at left firstly and by \left[\lambda x^{(1)}\lambda x^{(2)}\lambda x^{(3)}\right] ^{-1} at right secondly, then I = g^{T}g is obtained. This means that g is orthogonal i.e. there exist \widetilde{g}\in O\left(3\right) . From (3.5),
| \begin{equation*} y^{(i)} = \lambda \widetilde{g}x^{(i)} \end{equation*} |
can be written for i = 1, 2, 3 . So \left\{ x^{(1)}, x^{(2)}, x^{(3)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, y^{(3)}\right\} are obtained.
If m > 3 then, Since the rank of the matrix \left[x^{(1)}x^{(2)}...x^{(m)} \right] is equal to 3, the vector systems \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} and \left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} in three dimensional Euclidean space are linearly dependent. Suppose that \exists k, l, z\in \left\{ 1, ..., m\right\} such that the vectors x^{(k)} x^{(l)} and x^{(z)} are linearly independent. In this case the vectors y^{(k)} y^{(l)} and y^{(z)} are also linearly independent since the Gram determinant of the vectors \lambda x^{(k)} , \lambda x^{(l)} and \lambda x^{(z)} is different from zero. So the determinants
| \begin{eqnarray*} \left\vert Gr\left( \lambda x^{(k)}, \lambda x^{(l)}, \lambda x^{(z)}\right) \right\vert & = &\left\vert \begin{array}{lll} \left\langle \lambda x^{(k)}, \lambda x^{(k)}\right\rangle & \left\langle \lambda x^{(k)}, \lambda x^{(l)}\right\rangle & \left\langle \lambda x^{(k)}, \lambda x^{(z)}\right\rangle \\ \left\langle \lambda x^{(l)}, \lambda x^{(k)}\right\rangle & \left\langle \lambda x^{(l)}, \lambda x^{(l)}\right\rangle & \left\langle \lambda x^{(l)}, \lambda x^{(z)}\right\rangle \\ \left\langle \lambda x^{(z)}, \lambda x^{(k)}\right\rangle & \left\langle \lambda x^{(z)}, \lambda x^{(l)}\right\rangle & \left\langle \lambda x^{(z)}, \lambda x^{(z)}\right\rangle \end{array} \right\vert \\ & = &\left\vert Gr\left( \lambda y^{(k)}, \lambda y^{(l)}, \lambda y^{(z)}\right) \right\vert \label{Eq4.23} \end{eqnarray*} |
is different from zero. Now any vector in the systems of \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} and \left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} can be stated as in terms of linearly independent vectors as follow
| \begin{equation} x^{(i)} = \alpha _{ik}x^{(k)}+\alpha _{il}x^{(l)}+\alpha _{iz}x^{(z)} \end{equation} | (3.6) |
| \begin{equation} y^{(i)} = \beta_{ik} y^{(k)}+ \beta_{il} y^{(l)} + \beta_{iz} y^{(z)} \end{equation} | (3.7) |
for every integer i = 1, 2, ...m are obtained. Since
| \begin{equation*} \left[y^{(k)} y^{(l)} y^{(z)} \right]^{T} \left[y^{(k)} y^{(l)} y^{(z)} \right] = Gr\left(y^{(k)}, y^{(l)}, y^{(z)} \right) \end{equation*} |
From (3.6) the equality of the matrices
| \begin{equation} \left[\lambda x^{(k)} \lambda x^{(l)} \lambda x^{(z)} \right]^{T} \left[ \lambda x^{(k)} \lambda x^{(l)} \lambda x^{(z)} \right] = \left[y^{(k)} y^{(l)} y^{(z)} \right]^{T} \left[y^{(k)} y^{(l)} y^{(z)} \right] \end{equation} | (3.8) |
is obtained. Because the matrices \left[\lambda x^{(k)} \lambda x^{(l)} \lambda x^{(z)}\right] and \left[y^{(k)} y^{(l)} y^{(z)} \right] are regular and have inverses, there exist a regular matrix g such that
| \begin{equation} \left[ y^{(k)}y^{(l)}y^{(z)}\right] = g\left[ \lambda x^{(k)}\lambda x^{(l)}\lambda x^{(z)}\right] \end{equation} | (3.9) |
satisfies. If the equality (3.9) Substitutes to (3.8) and multiply both sides of (3.8) by \left(\left[\lambda x^{(k)}\lambda x^{(l)}\lambda x^{(z)}\right] ^{T}\right) ^{-1} at left firstly and by \left[\lambda x^{(k)}\lambda x^{(l)}\lambda x^{(z)}\right] ^{-1} at right secondly, then
| \begin{equation*} I = g^{T}g \end{equation*} |
is obtained. This means that g is orthogonal i.e. there exist \widetilde{g}\in O\left(3\right) . From (3.9),
| \begin{equation*} y^{(k)} = \lambda \widetilde{g}x^{(k)} \end{equation*} |
| \begin{equation*} y^{(l)} = \lambda \widetilde{g}x^{(l)} \end{equation*} |
| \begin{equation*} y^{(z)} = \lambda \widetilde{g}x^{(z)} \end{equation*} |
are obtained. From this and (3.6)
| \begin{equation} \widetilde{g}(\lambda x^{(i)}) = \alpha _{ik}y^{(k)}+\alpha _{il}y^{(l)}+\alpha _{iz}y^{(l)} \end{equation} | (3.10) |
for every i = 1, 2, ..., m are obtained.Now we must prove the cofficients of the vectors y^{(k)}, y^{(l)}, y^{(z)} in equations (3.7) and (3.10) are the same. i.e.
\beta _{ik} = \alpha _{ik} , \beta _{il} = \alpha _{il} and \beta _{iz} = \alpha _{iz} . From (3.6) and (3.7)
| \begin{equation*} \left\langle \lambda x^{(k)}, \lambda x^{(i)}\right\rangle = \alpha _{ik}\left\langle \lambda x^{(k)}, \lambda x^{(k)}\right\rangle +\alpha _{il}\left\langle \lambda x^{(k)}, \lambda x^{(l)}\right\rangle +\alpha _{iz}\left\langle \lambda x^{(k)}, \lambda x^{(z)}\right\rangle \end{equation*} |
| \begin{equation*} \left\langle \lambda x^{(l)}, \lambda x^{(i)}\right\rangle = \alpha _{ik}\left\langle \lambda x^{(l)}, \lambda x^{(k)}\right\rangle +\alpha _{il}\left\langle \lambda x^{(l)}, \lambda x^{(l)}\right\rangle +\alpha _{iz}\left\langle \lambda x^{(l)}, \lambda x^{(z)}\right\rangle \end{equation*} |
| \begin{equation*} \left\langle \lambda x^{(z)}, \lambda x^{(i)} \right\rangle = \alpha_{ik} \left\langle \lambda x^{(z)}, \lambda x^{(k)} \right\rangle + \alpha_{il} \left\langle \lambda x^{(z)}, \lambda x^{(l)} \right\rangle + \alpha_{iz} \left\langle \lambda x^{(z)}, \lambda x^{(z)} \right\rangle \end{equation*} |
and
| \begin{equation*} \left\langle y^{(k)}, y^{(i)} \right\rangle = \beta_{ik} \left\langle y^{(k)}, y^{(k)} \right\rangle + \beta_{il} \left\langle y^{(k)}, y^{(l)} \right\rangle + \beta_{iz} \left\langle y^{(k)}, y^{(z)} \right\rangle \end{equation*} |
| \begin{equation*} \left\langle y^{(l)}, y^{(i)} \right\rangle = \beta_{ik} \left\langle y^{(l)}, y^{(k)} \right\rangle + \beta_{il} \left\langle y^{(l)}, y^{(l)} \right\rangle + \beta_{iz} \left\langle y^{(l)}, y^{(z)} \right\rangle \end{equation*} |
| \begin{equation*} \left\langle y^{(z)}, y^{(i)} \right\rangle = \beta_{ik} \left\langle y^{(z)}, y^{(k)} \right\rangle + \beta_{il} \left\langle y^{(z)}, y^{(l)} \right\rangle + \beta_{iz} \left\langle y^{(z)}, y^{(z)} \right\rangle \end{equation*} |
can be wriiten. Solving these linear equations system
| \begin{equation*} \alpha _{ik} = \frac{\left\vert \begin{array}{lll} \left\langle \lambda x^{(k)}, \lambda x^{(i)}\right\rangle & \left\langle \lambda x^{(k)}, \lambda x^{(l)}\right\rangle & \left\langle \lambda x^{(k)}, \lambda x^{(z)}\right\rangle \\ \left\langle \lambda x^{(l)}, \lambda x^{(i)}\right\rangle & \left\langle \lambda x^{(l)}, \lambda x^{(l)}\right\rangle & \left\langle \lambda x^{(l)}, \lambda x^{(z)}\right\rangle \\ \left\langle \lambda x^{(z)}, \lambda x^{(i)}\right\rangle & \left\langle \lambda x^{(z)}, \lambda x^{(l)}\right\rangle & \left\langle \lambda x^{(z)}, \lambda x^{(z)}\right\rangle \end{array} \right\vert }{\det Gr\left( \lambda x^{(k)}, \lambda x^{(l)}, \lambda x^{(z)}\right) } \end{equation*} |
| \begin{equation*} \alpha _{il} = \frac{\left\vert \begin{array}{lll} \left\langle \lambda x^{(k)}, \lambda x^{(k)}\right\rangle & \left\langle \lambda x^{(k)}, \lambda x^{(i)}\right\rangle & \left\langle \lambda x^{(k)}, \lambda x^{(z)}\right\rangle \\ \left\langle \lambda x^{(l)}, \lambda x^{(k)}\right\rangle & \left\langle \lambda x^{(l)}, \lambda x^{(i)}\right\rangle & \left\langle \lambda x^{(l)}, \lambda x^{(z)}\right\rangle \\ \left\langle \lambda x^{(z)}, \lambda x^{(k)}\right\rangle & \left\langle \lambda x^{(z)}, \lambda x^{(i)}\right\rangle & \left\langle \lambda x^{(z)}, \lambda x^{(z)}\right\rangle \end{array} \right\vert }{\det Gr\left( \lambda x^{(k)}, \lambda x^{(l)}, \lambda x^{(z)}\right) } \end{equation*} |
| \begin{equation*} \alpha _{iz} = \frac{\left\vert \begin{array}{lll} \left\langle \lambda x^{(k)}, \lambda x^{(k)}\right\rangle & \left\langle \lambda x^{(k)}, \lambda x^{(l)}\right\rangle & \left\langle \lambda x^{(k)}, \lambda x^{(i)}\right\rangle \\ \left\langle \lambda x^{(l)}, \lambda x^{(k)}\right\rangle & \left\langle \lambda x^{(l)}, \lambda x^{(l)}\right\rangle & \left\langle \lambda x^{(l)}, \lambda x^{(i)}\right\rangle \\ \left\langle \lambda x^{(z)}, \lambda x^{(k)}\right\rangle & \left\langle \lambda x^{(z)}, \lambda x^{(l)}\right\rangle & \left\langle \lambda x^{(z)}, \lambda x^{(i)}\right\rangle \end{array} \right\vert }{\det Gr\left( \lambda x^{(k)}, \lambda x^{(l)}, \lambda x^{(z)}\right) } \end{equation*} |
and
| \begin{equation*} \beta _{ik} = \frac{\left\vert \begin{array}{lll} \left\langle y^{(k)}, y^{(i)}\right\rangle & \left\langle y^{(k)}, y^{(l)}\right\rangle & \left\langle y^{(k)}, y^{(z)}\right\rangle \\ \left\langle y^{(l)}, y^{(i)}\right\rangle & \left\langle y^{(l)}, y^{(l)}\right\rangle & \left\langle y^{(l)}, y^{(z)}\right\rangle \\ \left\langle y^{(z)}, y^{(i)}\right\rangle & \left\langle y^{(z)}, y^{(l)}\right\rangle & \left\langle y^{(z)}, y^{(z)}\right\rangle \end{array} \right\vert }{\det Gr\left( y^{(k)}, y^{(l)}, y^{(z)}\right) } \end{equation*} |
| \begin{equation*} \beta _{il} = \frac{\left\vert \begin{array}{lll} \left\langle y^{(k)}, y^{(k)}\right\rangle & \left\langle y^{(k)}, y^{(i)}\right\rangle & \left\langle y^{(k)}, y^{(z)}\right\rangle \\ \left\langle y^{(l)}, y^{(k)}\right\rangle & \left\langle y^{(l)}, y^{(i)}\right\rangle & \left\langle y^{(l)}, y^{(z)}\right\rangle \\ \left\langle y^{(z)}, y^{(k)}\right\rangle & \left\langle y^{(z)}, y^{(i)}\right\rangle & \left\langle y^{(z)}, y^{(z)}\right\rangle \end{array} \right\vert }{\det Gr\left( y^{(k)}, y^{(l)}, y^{(z)}\right) } \end{equation*} |
| \begin{equation*} \beta _{iz} = \frac{\left\vert \begin{array}{lll} \left\langle y^{(k)}, y^{(k)}\right\rangle & \left\langle y^{(k)}, y^{(l)}\right\rangle & \left\langle y^{(k)}, y^{(i)}\right\rangle \\ \left\langle y^{(l)}, y^{(k)}\right\rangle & \left\langle y^{(l)}, y^{(l)}\right\rangle & \left\langle y^{(l)}, y^{(i)}\right\rangle \\ \left\langle y^{(z)}, y^{(k)}\right\rangle & \left\langle y^{(z)}, y^{(l)}\right\rangle & \left\langle y^{(z)}, y^{(i)}\right\rangle \end{array} \right\vert }{\det Gr\left( y^{(k)}, y^{(l)}, y^{(z)}\right) } \end{equation*} |
is obtained for every i = 1, 2, ...m . Using (3.1) and (3.2) \beta _{ik} = \alpha _{ik} , \beta _{il} = \alpha _{il} and \beta _{iz} = \alpha _{iz} are obtained. So from (3.10) y^{(i)} = \lambda \widetilde{g}x^{(i)} for every i = 1, 2, ...m are obtained and \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} is proved.
(b) Let x^{(i)}\neq 0 and y^{(i)}\neq 0 for every i = 1, 2, ..., m and the rank of the matrices \left[x^{(1)}x^{(2)}...x^{(m)}\right] and \left[y^{(1)}y^{(2)}...y^{(m)}\right] are equal to 2 be given and \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} be supposed. In this case there exist a scalar \lambda > 0 and g\in O(3) such that y^{(j)} = \lambda gx^{(j)} satisfies for every j = 1, 2, ..., m . Then because the orthogonal transformation g\in O(3) save inner product.
| \begin{equation*} \frac{\left\langle y^{(i)}, y^{(j)}\right\rangle }{\left\langle y^{(1)}, y^{(1)}\right\rangle } = \frac{\left\langle \lambda gx^{(i)}, \lambda gx^{(j)}\right\rangle }{\left\langle \lambda gx^{(1)}, \lambda gx^{(1)}\right\rangle } = \frac{\left\langle x^{(i)}, x^{(j)}\right\rangle }{\left\langle x^{(1)}, x^{(1)}\right\rangle } \end{equation*} |
is obtained for i = 1, 2 and j = 3, ..., m .
Conversely for every i = 1, 2 and j = 2, 3, ..., m let this equality \frac{ \left\langle x^{(i)}, x^{(j)}\right\rangle }{\left\langle x^{(1)}, x^{(1)}\right\rangle } = \frac{\left\langle y^{(i)}, y^{(j)}\right\rangle }{\left\langle y^{(1)}, y^{(1)}\right\rangle } be given. It will be proved that
| \begin{equation*} \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} . \end{equation*} |
Now from hyphothesis for every i = 1, 2 and j = 2, 3, ..., m ,
| \begin{equation*} \left\langle y^{(i)}, y^{(j)}\right\rangle = \frac{\left\langle y^{(1)}, y^{(1)}\right\rangle }{\left\langle x^{(1)}, x^{(1)}\right\rangle } \left\langle x^{(i)}, x^{(j)}\right\rangle \end{equation*} |
can be written. So if the multiple \frac{\left\langle y^{(1)}, y^{(1)}\right\rangle }{\left\langle x^{(1)}, x^{(1)}\right\rangle } > 0 is denoted by \lambda ^{2} , then
| \begin{equation} \left\langle y^{(i)}, y^{(j)}\right\rangle = \left\langle \lambda x^{(i)}, \lambda x^{(j)}\right\rangle \end{equation} | (3.11) |
is obtained for every i = 1, 2 and j = 2, 3, ..., m . Since the ranks of thematrices \left[x^{(1)}x^{(2)}...x^{(m)}\right] and \left[y^{(1)}y^{(2)}...y^{(m)}\right] are equal to 2, m\geq 2 must be chosen. The vector systems \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} and \left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} in three dimensional Euclidean space are linearly dependent. Suppose that the vectors x^{(1)} and x^{(2)} are linearly independent. In this case the vectors y^{(1)} and y^{(2)} are also linearly independent since the Gram determinant of the vectors \lambda x^{(1)} and \lambda x^{(2)}
| \begin{equation*} \left\vert Gr\left( \lambda x^{(1)}, \lambda x^{(2)}\right) \right\vert = \left\vert \begin{array}{ll} \left\langle \lambda x^{(1)}, \lambda x^{(1)}\right\rangle & \left\langle \lambda x^{(1)}, \lambda x^{(2)}\right\rangle \\ \left\langle \lambda x^{(2)}, \lambda x^{(1)}\right\rangle & \left\langle \lambda x^{(2)}, \lambda x^{(2)}\right\rangle \end{array} \right\vert = \left\vert Gr\left( \lambda y^{(1)}, \lambda y^{(2)}\right) \right\vert \end{equation*} |
is different from zero. Now any vector in the systems of \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} and \left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} can be stated as in terms of linearly independent vectors as follow
| \begin{equation} x^{(i)} = \alpha _{i1}x^{(1)}+\alpha _{i2}x^{(2)} \end{equation} | (3.12) |
| \begin{equation} y^{(i)} = \beta _{i1}y^{(1)}+\beta _{i2}y^{(2)} \end{equation} | (3.13) |
for every integer i = 1, 2, ...m are obtained. Since
| \begin{equation*} \left[ y^{(1)}y^{(2)}\right] ^{T}\left[ y^{(1)}y^{(2)}\right] = Gr\left( y^{(1)}, y^{(2)}\right) \end{equation*} |
From (3.12) the equality of the matrices
| \begin{equation} \left[\lambda x^{(1)} \lambda x^{(2)} \right]^{T} \left[\lambda x^{(1)} \lambda x^{(2)} \right] = \left[y^{(1)} y^{(2)} \right]^{T} \left[y^{(1)} y^{(2)} \right] \end{equation} | (3.14) |
is obtained. Because the matrices \left[\lambda x^{(1)} \lambda x^{(2)} \right] and \left[y^{(1)} y^{(2)} \right] are regular and have inverses, there exist a regular matrix g such that
| \begin{equation} \left[y^{(1)} y^{(2)} \right] = g \left[\lambda x^{(1)} \lambda x^{(2)} \right] \end{equation} | (3.15) |
satisfies. If the equality (3.15) substitutes to (3.14) and multiply both sides of (3.14) by \left(\left[\lambda x^{(1)}\lambda x^{(2)} \right]^{T} \right)^{-1} at left firstly and by \left[\lambda x^{(1)} \lambda x^{(2)} \right]^{-1} at right secondly, then
| \begin{equation*} I = g^{T}g \end{equation*} |
is obtained. this means that g is orthogonal i.e. there exist \widetilde{g} \in O\left(3\right) . From (3.15),
| \begin{equation*} y^{(1)} = \lambda \widetilde{g} x^{(1)} \end{equation*} |
| \begin{equation*} y^{(2)} = \lambda \widetilde{g} x^{(2)} \end{equation*} |
are obtained. From this and (3.13)
| \begin{equation} \widetilde{g} (\lambda x^{(i)}) = \alpha_{ik} y^{(k)} + \alpha_{il} y^{(l)} \end{equation} | (3.16) |
for every i = 1, 2, ..., m are obtained.Now it must proved that the cofficients of the vectors y^{(k)}, y^{(l)} in equations (3.13) and (3.16) are the same. i.e.
\beta_{ik} = \alpha_{ik} and \beta_{il} = \alpha_{il} . From (3.12) and (3.13)
| \begin{equation*} \left\langle \lambda x^{(k)}, \lambda x^{(i)} \right\rangle = \alpha_{ik} \left\langle \lambda x^{(k)}, \lambda x^{(k)} \right\rangle + \alpha_{il} \left\langle \lambda x^{(k)}, \lambda x^{(l)} \right\rangle \end{equation*} |
| \begin{equation*} \left\langle \lambda x^{(l)}, \lambda x^{(i)} \right\rangle = \alpha_{ik} \left\langle \lambda x^{(l)}, \lambda x^{(k)} \right\rangle + \alpha_{il} \left\langle \lambda x^{(l)}, \lambda x^{(l)} \right\rangle \end{equation*} |
and
| \begin{equation*} \left\langle y^{(k)}, y^{(i)} \right\rangle = \beta_{ik} \left\langle y^{(k)}, y^{(k)} \right\rangle + \beta_{il} \left\langle y^{(k)}, y^{(l)} \right\rangle \end{equation*} |
| \begin{equation*} \left\langle y^{(l)}, y^{(i)} \right\rangle = \beta_{ik} \left\langle y^{(l)}, y^{(k)} \right\rangle + \beta_{il} \left\langle y^{(l)}, y^{(l)} \right\rangle \end{equation*} |
can be wriiten. Solving these linear equations system
| \begin{equation*} \alpha _{ik} = \frac{\left\vert \begin{array}{ll} \left\langle \lambda x^{(k)}, \lambda x^{(i)}\right\rangle & \left\langle \lambda x^{(k)}, \lambda x^{(l)}\right\rangle \\ \left\langle \lambda x^{(l)}, \lambda x^{(i)}\right\rangle & \left\langle \lambda x^{(l)}, \lambda x^{(l)}\right\rangle \end{array} \right\vert }{\det Gr\left( \lambda x^{(k)}, \lambda x^{(l)}\right) } \end{equation*} |
| \begin{equation*} \alpha _{il} = \frac{\left\vert \begin{array}{ll} \left\langle \lambda x^{(k)}, \lambda x^{(k)}\right\rangle & \left\langle \lambda x^{(k)}, \lambda x^{(i)}\right\rangle \\ \left\langle \lambda x^{(l)}, \lambda x^{(k)}\right\rangle & \left\langle \lambda x^{(l)}, \lambda x^{(i)}\right\rangle \end{array} \right\vert }{\det Gr\left( \lambda x^{(k)}, \lambda x^{(l)}\right) } \end{equation*} |
and
| \begin{equation*} \beta _{ik} = \frac{\left\vert \begin{array}{ll} \left\langle y^{(k)}, y^{(i)}\right\rangle & \left\langle y^{(k)}, y^{(l)}\right\rangle \\ \left\langle y^{(l)}, y^{(i)}\right\rangle & \left\langle y^{(l)}, y^{(l)}\right\rangle \end{array} \right\vert }{\det Gr\left( y^{(k)}, y^{(l)}\right) } \end{equation*} |
| \begin{equation*} \beta _{il} = \frac{\left\vert \begin{array}{ll} \left\langle y^{(k)}, y^{(k)}\right\rangle & \left\langle y^{(k)}, y^{(i)}\right\rangle \\ \left\langle y^{(l)}, y^{(k)}\right\rangle & \left\langle y^{(l)}, y^{(i)}\right\rangle \end{array} \right\vert }{\det Gr\left( y^{(k)}, y^{(l)}, y^{(z)}\right) } \end{equation*} |
is obtained for every i = 1, 2, ...m . Using (3.11) \beta_{ik} = \alpha_{ik} and \beta_{il} = \alpha_{il} are obtained. So \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{ \cong } \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} is proved.
(c) Let x^{(i)}\neq 0 and y^{(i)}\neq 0 for every i = 1, 2, ..., m and the rank of the matrices \left[x^{(1)} x^{(2)}... x^{(m)} \right] and \left[y^{(1)} y^{(2)}... y^{(m)} \right] are equal to 1 be given and \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} be supposed. In this case there exist a scalar \lambda > 0 and g\in O(3) such that y^{(i)} = \lambda gx^{(i)} is satisfied for every i = 1, 2, ..., m . Since the rank of above matrices are 1, there exist one linear independent vector in each system \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} and \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} . Let these linear independent vectors be denoted x^{(s)} and y^{(s)} for any s \in \left\{1, ...m\right\} . For every i = 1, ..., m
| \begin{equation} x^{(i)} = l_{i} x^{(s)} \ ; \ y^{(i)} = m_{i} y^{(s)} \end{equation} | (3.17) |
can be written.
So from (3.17)
| \frac{m_{i}}{m_{1}} = \frac{\left\langle y^{(1)}, y^{(i)} \right\rangle}{\left\langle y^{(1)}, y^{(1)} \right\rangle} = \frac{\left\langle \lambda g x^{(l)}, \lambda g x^{(i)} \right\rangle}{\left\langle \lambda g x^{(l)}, \lambda g x^{(l)} \right\rangle} = \frac{\left\langle \lambda g l_{1} x^{(s)}, \lambda g l_{i}x^{(s)} \right\rangle}{\left\langle \lambda g l_{1} x^{(s)}, \lambda g l_{1} x^{(s)} \right\rangle} = \frac{l_{i}}{l_{1}} = \frac{m_{i}}{m_{1}} = \frac{\left\langle x^{(1)}, x^{(i)} \right\rangle}{\left\langle x^{(1)}, x^{(1)}\right\rangle} |
is obtained for every i = 2, ..., m .
Conversely let this equality \frac{\left\langle y^{(1)}, y^{(i)} \right\rangle}{\left\langle y^{(1)}, y^{(1)} \right\rangle} = \frac{\left\langle x^{(1)}, x^{(i)} \right\rangle}{\left\langle x^{(1)}, x^{(1)}\right\rangle} be given for every j = 2, ..., m .It will be proved that
| \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\}. |
From Theorem 3.1 x^{(s)}\overset{LS(3)}{\cong }y^{(s)} always satisfies and then there exist a scalar \lambda > 0 and g\in O(3) such that y^{(s)} = \lambda gx^{(s)} satisfies. Then from (3.17)
| \begin{equation*} x^{(s)} = \frac{1}{l_{i}}x^{(i)}\text{ and } y^{(s)} = \frac{1}{m_{i}}y^{(i)} \end{equation*} |
is obtained and since y^{(s)} = \lambda gx^{(s)} then, \frac{1}{m_{i}} y^{(i)} = \lambda g\frac{1}{l_{i}}x^{(i)} can be written. So for every i , y^{(i)} = \lambda \frac{m_{i}}{l_{i}}gx^{(i)} is obtained. From hypotthesis \frac{m_{i}}{l_{i}} = \frac{m_{1}}{1_{1}} = \sqrt{ \frac{\left\langle y^{(1)}, y^{(1)} \right\rangle}{\left\langle x^{(1)}, x^{(1)}\right\rangle}} is always positive. If the real number \frac{m_{1}}{l_{1}}\lambda is denoted by \overline{\lambda } , then it is seen that \overline{\lambda } > 0 .So for every j = 1, ..., m ;
| \begin{equation*} y^{(j)} = m_{j}y^{(s)} = \frac{m_{1}}{l_{1}} l_{j}(\lambda gx^{(s)}) = \overline{\lambda} gx^{(j)} \end{equation*} |
can be written. So
| \begin{equation*} \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} . \end{equation*} |
is proved.
(d) Let x^{(i)}\neq 0 and y^{(i)}\neq 0 for every i = 1, 2, ..., m and the ranks of the matrices \left[x^{(1)}x^{(2)}...x^{(m)}\right] and \left[y^{(1)}y^{(2)}...y^{(m)}\right] be different from each other. Firstly each rank of given matrices must be different from zero. Otherwise it means all of the vectors in these systems are zero. It is mentioned above. Just let rank\left[x^{(1)}x^{(2)}...x^{(m)}\right] = 1 and rank\left[y^{(1)}y^{(2)}...y^{(m)}\right] = 2 be given. In this case there exist the integers i, j, s\in \left\{ 1, 2, ..., m\right\} such that the vector x^{(i)} is linearly independent in the system \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} and these vectors y^{(j)}, y^{(s)} are linearly independent in the system \left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} . So
| \begin{equation*} x^{(k)} = a_{k}x^{(i)} \end{equation*} |
| \begin{equation*} y^{(k)} = b_{kj}y^{(j)}+b_{ks}y^{(s)} \end{equation*} |
can be written for every k = 1, 2, ..., m . It is clear that a_{i} = b_{jj} = b_{ss} = 1 and b_{js} = b_{sj} = 0 . Let \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} be supposed. In this case there exist a \lambda > 0 and g\in O(3) such that y^{(k)} = \lambda gx^{(k)} for every k = 1, ...m . Then it follows
| \begin{equation*} y^{(k)} = \lambda g\left( a_{k}x^{(i)}\right) = a_{k}y^{(i)} \end{equation*} |
for every k = 1, ...m . It means each vectors {y^{(1)}, y^{(2)}, ..., y^{(m)}} can be expressed by the vector x^{(i)} . This is a contradiction and so \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\ncong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} . Similarly in case rank\left[x^{(1)}x^{(2)}...x^{(m)}\right] = 1 and rank \left[y^{(1)}y^{(2)}...y^{(m)}\right] = 3 these systems are not equivalent. i.e. \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\ncong } \left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} .
Let rank\left[x^{(1)}x^{(2)}...x^{(m)}\right] = 2 and rank \left[x^{(1)}x^{(2)}...x^{(m)}\right] = 1 be given. In this case there exist the integers i, j, s\in \left\{ 1, 2, ..., m\right\} such that these vectors x^{(i)}, x^{(j)} are linearly independent in the system \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} and the vector y^{(s)} is linearly independent in the system \left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} begiven. So,
| \begin{equation*} y^{(k)} = a_{k}y^{(s)} \end{equation*} |
can be written for every k = 1, ...m . Let \left\{x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\cong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} be supposed. In this case there exist a \lambda > 0 and g\in O(3) such that y^{(k)} = \lambda gx^{(k)} for every k = 1, ...m . This statement can be written as matrix form as
| \begin{equation*} \left[ y^{(1)}y^{(2)}...y^{(m)}\right] = g\left[ \lambda x^{(1)}\lambda x^{(2)}...\lambda x^{(m)}\right] \end{equation*} |
It follows
| \begin{equation*} \left[ \lambda x^{(1)}\lambda x^{(2)}...\lambda x^{(m)}\right] = g^{T}\left[ y^{(1)}y^{(2)}...y^{(m)}\right] \end{equation*} |
It means for every k = 1, ..., m
| \begin{equation*} \lambda x^{(k)} = g^{T}\left( a_{k}y^{(s)}\right) = a_{k}\lambda x^{(s)} \end{equation*} |
can be written. And every vectors \left\{ \lambda x^{(1)}, \lambda x^{(2)}, ..., \lambda x^{(m)}\right\} can be stated by the vector \lambda x^{(s)} and it is a contradiction. So the assumption is wrong. As a result of this
| \begin{equation*} \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\ncong }\left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} \end{equation*} |
Similarly in case rank\left[x^{(1)}x^{(2)}...x^{(m)}\right] = 3 and rank\left[y^{(1)}y^{(2)}...y^{(m)}\right] = 1 these systems are not equivalent. i.e. \left\{ x^{(1)}, x^{(2)}, ..., x^{(m)}\right\} \overset{LS(3)}{\ncong } \left\{ y^{(1)}, y^{(2)}, ..., y^{(m)}\right\} .
The best examples of points system are Bézier curves and Bézier surfaces. The invariants of these curves and surfaces under an affine transformation have the same meaning as the invariants of the control points of these curves and surfaces. Therefore it is necessary that the invariant properties of modelling of mechanism allow durable and precision, and reliable results under transformation groups. In this sense, knowing of complete of invariants of Bézier and B-spline curves and surfaces which gives most stable solutions within CAD systems [23] is important.
A general Bézier curve X(t) of degree n with control points b_{0}, b_{1}, b_{2}, ..., b_{n} is defined by
| \begin{equation*} X(t) = \sum\limits_{i = 0}^{n}B_{i}^{n}(t)b_{i} \end{equation*} |
where t\in \lbrack 0, 1] and B_{i}^{n}(t) are Bernstein basis polynomials of degree n defined by [21].
| \begin{equation*} B_{i}^{n}(t) = \left( \overset{n}{i}\right) (1-t)^{n-i}t^{i} \end{equation*} |
Theorem 4.1. (Invariance under Affine Transformations property)Let X(t) be given a general Bézier curves of degree n with control points {b_{0}, b_{1}, b_{2}, ..., b_{n}} in R^{3} . So, X satisfies as follow:
Let T be an (affine) transformation (for example, a rotation, reflection, translation, or scaling or similarity transformation). Then
| T\left( X(t)\right) = T\left( \sum\limits_{i = 0}^{n}B_{i}^{n}(t)b_{i}\right) = \sum\limits_{i = 0}^{n}B_{i}^{n}(t)T\left( b_{i}\right) |
is satisfied. [54]
Definition: Let X(t) and Y(t) be given two general Bézier curves of degree n with control points {b_{0}, b_{1}, b_{2}, ..., b_{n}} and {q_{0}, q_{1}, q_{2}, ..., q_{n}} in R^{3} respectively. Then these Bézier curves are called LS(3)- equivalent if there exist a ransformation g \in LS(3)- such that q_{i} = gb_{i} for every i = 1, ..., n .
A linear Bézier curve X(t) with control points b_{0}, b_{1} is defined by
| \begin{equation*} X(t) = (1-t)b_{0}+tb_{1} \end{equation*} |
where t\in \lbrack 0, 1] [54].
Theorem 4.2. Let X, Y be given two linear Bézier curves with control points {b_{0}, b_{1}} and {p_{0}, p_{1}} in R^{3} respectively. So,
1. if any b_{i} = (0, 0, 0) and any p_{i} = (0, 0, 0) for i = 0, 1 then these curves are always LS(3)- equivalent.
2. if any b_{i} = (0, 0, 0) and each p_{j}\neq (0, 0, 0) or each b_{i}\neq (0, 0, 0) and any p_{j} = (0, 0, 0) for i, j = 0, 1 then these curves are not LS(3)- equivalent.
3. if every b_{i}\neq (0, 0, 0) and p_{j}\neq (0, 0, 0) for i, j = 0, 1 then
(a) if rank\left[b_{0}\text{ }b_{1}\right] = rank\left[p_{0}\text{ }p_{1}\right] = 2 then these Bézier curves are LS(3)- equivalent if and only if
\frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{0}, p_{1}\right\rangle }{\left\langle b_{0}, b_{1}\right\rangle } = \frac{\left\langle p_{1}, p_{1}\right\rangle }{\left\langle b_{1}, b_{1}\right\rangle } is satisfied.
(b) if rank\left[b_{0}\text{ }b_{1}\right] = rank\left[p_{0}\text{ }p_{1} \right] = 1 then these Bézier curves are LS(3)- equivalent if and only if
\frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{0}, p_{1}\right\rangle }{\left\langle b_{0}, b_{1}\right\rangle } is satisfied.
Proof. This theorem is a conclusion of Theorem 3.2. In third case the ranks of matrice of control points of each Bézier curves X and Y are equal to 2 or 1. If the rank of matrices is 2, then according to theorem 3.2, these equality \frac{\left\langle b_{0}, b_{1}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{ \left\langle p_{0}, p_{1}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle } and \frac{\left\langle b_{1}, b_{1}\right\rangle}{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{1}, p_{1}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle } must be satisfied. So \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{0}, p_{1}\right\rangle }{\left\langle b_{0}, b_{1}\right\rangle } = \frac{\left\langle p_{1}, p_{1}\right\rangle }{\left\langle b_{1}, b_{1}\right\rangle } is true.
If the rank of matrices is 1 then from Theorem 3.2. \frac{\left\langle b_{0}, b_{1}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{ \left\langle p_{0}, p_{1}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle } must be satisfied. So \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{0}, p_{1}\right\rangle }{\left\langle b_{0}, b_{1}\right\rangle } is satisfied.
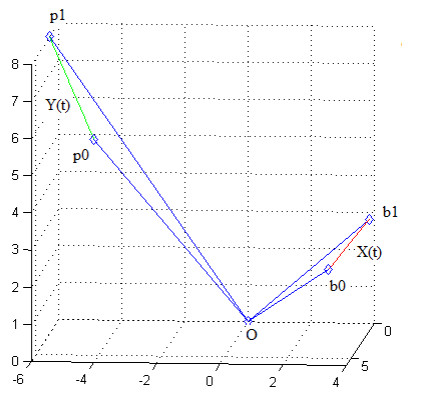
In Figure 1 LS(3)- equivalent linear Bézier curves X(t) and Y(t) are illustrated.
A quadratic Bézier curve X(t) with control points b_{0}, b_{1}, b_{2} is defined by
| \begin{equation*} X(t) = (1-t)^{2}b_{0}+2(1-t)tb_{1}+t^{2}b_{2} \end{equation*} |
where t\in \lbrack 0, 1] [54].
Theorem 4.3. Let X, Y be given two quadratic Bézier curves with control points {b_{0}, b_{1}, b_{2}} and {p_{0}, p_{1}, p_{2}} in R^{3} respectively. So,
1. if any b_{i} = (0, 0, 0) and p_{i} = (0, 0, 0) for i = 0, 1, 2 then these curves are LS(3)- equivalent if and only if the condition of third case of Theorem 3.2 is satisfied excluding b_{i} and p_{i} .
2. if any b_{i} = (0, 0, 0) and each p_{j}\neq (0, 0, 0) or each b_{i}\neq (0, 0, 0) and any p_{j} = (0, 0, 0) for i, j = 0, 1, 2 then these curves are not LS(3)- equivalent.
3. if every b_{i}\neq (0, 0, 0) and p_{j}\neq (0, 0, 0) for i, j = 0, 1, 2 and
(a) the ranks of matrices of control points of each Bézier curves X and Y are equal to 3 then these Bézier curves are LS(3)- equivalent if and only if all of these ratio must be same for i, j = 1, 2 as follows:
\frac{\left\langle p_{i}, p_{0}\right\rangle }{\left\langle b_{i}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{1}\right\rangle }{\left\langle b_{i}, b_{1}\right\rangle } = \frac{\left\langle p_{2}, p_{2}\right\rangle }{\left\langle b_{2}, b_{2}\right\rangle } = \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle }
are satisfied.
(b) the ranks of matrices of control points of each Bézier curves X and Y are equal to 2 then, these Bézier curves are LS(3)- equivalent if and only if for i = 1, 2
\frac{\left\langle p_{i}, p_{0}\right\rangle }{\left\langle b_{i}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{1}\right\rangle }{\left\langle b_{i}, b_{1}\right\rangle } = \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle }
are satisfied.
(c) the ranks of matrice of control points of each Bézier curves X and Y are not equal to each other then, these Bézier curves are not LS(3)- equivalent.
Proof. This theorem is also a conclusion of Theorem 3.2. Since the Bézier Curves are quadratic in this theorem, the ranks of matrices of control points of each Bézier curves X and Y can be equal to 2 (in case the control points of these Bézier Curves lie the plane consisted of the origin point and two of control points) or 3 (other case).
1. If the ranks of matrices of control points of each Bézier curves X and Y can be equal to 3 then in this case from Theorem 3.2. for i = 0, 1, 2 and i\leq j , \frac{\left\langle b_{i}, b_{j}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{j}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle } ; must be satisfied to be X equivalent to Y . Then from this equalities
| \frac{\left\langle p_{i}, p_{0}\right\rangle}{\left\langle b_{i}, b_{0}\right\rangle} = \frac{\left\langle p_{i}, p_{1}\right\rangle }{\left\langle b_{i}, b_{1}\right\rangle} = \frac{\left\langle p_{2}, p_{2}\right\rangle }{\left\langle b_{2}, b_{2}\right\rangle} = \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle} |
are obtained for every i = 1, 2 .
2. If ranks of matrices of control points of each Bézier curves X and Y can be equal to 2 then
| \frac{\left\langle b_{i}, b_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{0}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle } \text{;} \frac{\left\langle b_{i}, b_{1}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{1}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle } |
are satisfied. From these equalities, the conclusion that all of these ratios for i = 1, 2 must be equal are obtained as follows:
| \frac{\left\langle p_{i}, p_{0}\right\rangle }{\left\langle b_{i}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{1}\right\rangle }{\left\langle b_{i}, b_{1}\right\rangle } = \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } |
In Figure 2 LS(3)- equivalent quadratic Bézier curves X(t) and Y(t) are plotted.
A Cubic Bézier curve X(t) with control points b_{0}, b_{1}, b_{2}, b_{3} is defined by
| \begin{equation*} X(t) = (1-t)^{3}b_{0}+3(1-t)^{2}tb_{1}+3(1-t)t^{2}b_{2}+t^{3}b_{3} \end{equation*} |
where t\in \lbrack 0, 1] [54].
Theorem 4.4. Let X, Y be given two Cubic Bézier curves with control points {b_{0}, b_{1}, b_{2}, b_{3}} and {p_{0}, p_{1}, p_{2}, p_{3}} in R^{3} respectively. So,
1. if any b_{i} = (0, 0, 0) and p_{i} = (0, 0, 0) for i, j = 0, 1, 2, 3 then these curves are LS(3)- equivalent if and only if the condition of third case of theorem 3.2 is satisfied excluding b_{i} and p_{i} .
2. if any b_{i} = (0, 0, 0) and each p_{j}\neq (0, 0, 0) or each b_{i}\neq (0, 0, 0) and any p_{j} = (0, 0, 0) for i, j = 0, 1, 2, 3 then these curves are not LS(3)- equivalent.
3. if every b_{i}\neq (0, 0, 0) and p_{j}\neq (0, 0, 0) for i, j = 0, 1, 2, 3 and
(a) If the ranks of matrices of control points of each Bézier curves X and Y are equal to 3 then these Bézier curves are LS(3)- equivalent if and only if for i = 1, 2, 3 for j = 2, 3
\frac{\left\langle p_{i}, p_{0}\right\rangle }{\left\langle b_{i}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{1}\right\rangle }{\left\langle b_{i}, b_{1}\right\rangle } = \frac{\left\langle p_{j}, p_{2}\right\rangle }{\left\langle b_{j}, b_{2}\right\rangle } = \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle }
are satisfied.
(b) If the ranks of matrices of control points of each Bézier curves X and Y are equal to 2 then, these Bézier curves are LS(3)- equivalent if and only if for j = 1, 2, 3
\frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{0}, p_{j}\right\rangle }{\left\langle b_{0}, b_{j} \right\rangle} = \frac{\left\langle p_{1}, p_{j}\right\rangle }{\left\langle b_{1}, b_{j}\right\rangle}
must be same.
(c) If the ranks of matrices of control points of each Bézier curves X and Y are not equal to each other then, these Bézier curves are not LS(3)- equivalent.
Proof. This theorem is also a conclusion of Theorem 3.2. Since the Bézier Curves are Cubic in this theorem, the ranks of matrices of control points of each Bézier curves X and Y can be equal to 2 or 3 like Theorem 4.3. If these ranks are equal to 3 then from Theorem 3.2. for i = 1, 2, 3 and j = 2, 3
| \frac{\left\langle b_{0}, b_{i}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{0}, p_{i}\right\rangle }{ \left\langle p_{0}, p_{0}\right\rangle } \text{ ; }\frac{\left\langle b_{i}, b_{1}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{ \left\langle p_{i}, p_{1}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle }\text{ ; }\frac{\left\langle b_{j}, b_{2}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{j}, p_{2}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle } |
can be written. Then from this,
| \frac{\left\langle p_{i}, p_{0}\right\rangle }{\left\langle b_{i}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{1}\right\rangle }{\left\langle b_{i}, b_{1}\right\rangle } = \frac{\left\langle p_{j}, p_{2}\right\rangle }{\left\langle b_{j}, b_{2}\right\rangle } = \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } |
are obtained.
If these ranks are equal to 2 then from Theorem 3.2. for i = 0, 1 and j = 1, 2, 3
| \frac{\left\langle b_{i}, b_{j}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{j}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle } |
is satisfied. So from this for j = 1, 2, 3
| \frac{\left\langle b_{0}, b_{j}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{0}, p_{j}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle }\text{ and }\frac{\left\langle b_{1}, b_{j}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{1}, p_{j}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle } |
can be written. And so for j = 1, 2, 3 these equalities of ratios
| \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{0}, p_{j}\right\rangle }{\left\langle b_{0}, b_{j} \right\rangle} = \frac{\left\langle p_{1}, p_{j}\right\rangle }{\left\langle b_{1}, b_{j} \right\rangle} |
are obtained.
In Figure 3 LS(3)- equivalent Cubic Bézier curves X(t) and Y(t) are graphed.
Theorem 4.5. Let X, Y be given two general Bézier curves of degree n with control points {b_{0}, b_{1}, b_{2}, ..., b_{n}} and {p_{0}, p_{1}, p_{2}, ..., p_{n}} in R^{3} respectively. So,
1. if any b_{i} = (0, 0, 0) and any p_{i} = (0, 0, 0) for i = 0, 1, 2, ..., n then these curves are LS(3)- equivalent if and only if the condition of third case of theorem 3.2 is satisfied excluding b_{i} and p_{i} .
2. if any b_{i} = (0, 0, 0) and each p_{j}\neq (0, 0, 0) or each b_{i}\neq(0, 0, 0) and any p_{j} = (0, 0, 0) for i, j = 0, 1, 2, ..., n then these curves are not LS(3)- equivalent.
3. if every b_{i}\neq (0, 0, 0) and p_{j}\neq (0, 0, 0) for i, j = 0, 1, 2, ..., n then these Bézier curves are LS(3)- equivalent if and only if
(a) for i, j = 0, 1, 2 ; k = 3, ..., n and i\leq j .
| \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{j}\right\rangle }{\left\langle b_{i}, b_{j} \right\rangle } = \frac{\left\langle p_{i}, p_{k} \right\rangle }{\left\langle b_{i}, b_{k}\right\rangle } |
must be same where i, j = 0, 1, 2 ; k = 3, ..., n and i\leq j if the ranks of matrices of control points of each Bézier curves X and Y are equal to 3.
(b)
| \begin{eqnarray*} \frac{\left\langle b_{i}, b_{j}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } & = &\frac{\left\langle p_{i}, p_{j}\right\rangle }{ \left\langle p_{0}, p_{0}\right\rangle } \\ \frac{\left\langle b_{i}, b_{k}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } & = &\frac{\left\langle p_{i}, p_{k}\right\rangle }{ \left\langle p_{0}, p_{0}\right\rangle } \end{eqnarray*} |
it is satisfied if the ranks of matrices of control points of each Bézier curves X and Y are equal to 2 where i, j = 0, 1 ; k = 2, ..., n and i\leq j .
Proof. This theorem is also a conclusion of Theorem 3.2. The ranks of matrices of control points of each Bézier curves X and Y can be equal to 2 or 3 similarly.
If these raks are equal to 3 then from Theorem 3.2. for i, j = 0, 1, 2 ; k = 3, ..., n and i\leq j .
| \begin{align*} \frac{\left\langle b_{i}, b_{j}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } & = \frac{\left\langle p_{i}, p_{j}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle } \\ \frac{\left\langle b_{i}, b_{k}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } & = \frac{\left\langle p_{i}, p_{k}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle } \end{align*} |
can be written. So from this for i, j = 0, 1, 2 ; k = 3, ..., n and i\leq j .
| \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{j}\right\rangle }{\left\langle b_{i}, b_{j} \right\rangle } = \frac{\left\langle p_{i}, p_{k} \right\rangle }{\left\langle b_{i}, b_{k}\right\rangle } |
must be same.
If these raks are equal to 2 then from Theorem 3.2. for i, j = 0, 1 ; k = 2, ..., n and i\leq j .
| \begin{align*} \frac{\left\langle b_{i}, b_{j}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } & = \frac{\left\langle p_{i}, p_{j}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle } \\ \frac{\left\langle b_{i}, b_{k}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } & = \frac{\left\langle p_{i}, p_{k}\right\rangle }{\left\langle p_{0}, p_{0}\right\rangle } \end{align*} |
can be written. So from this for i, j = 0, 1 ; k = 2, ..., n and i\leq j it is obtained that
| \frac{\left\langle p_{0}, p_{0}\right\rangle }{\left\langle b_{0}, b_{0}\right\rangle } = \frac{\left\langle p_{i}, p_{j}\right\rangle }{\left\langle b_{i}, b_{j} \right\rangle } = \frac{\left\langle p_{i}, p_{k} \right\rangle }{\left\langle b_{i}, b_{k}\right\rangle } |
must be same.
A general (n, m)- Typed Bézier Surface X(t, u) with control points b_{ij} in R^{3} for i = 0, 1, ..., n and j = 0, 1, ..., m is defined by
| \begin{equation*} X(t, u) =\sum\limits_{i = 1} ^n\sum\limits_{j = 1} ^m B_{i}^{n}\left( t\right) B_{j}^{m}\left( u\right) b_{ij} \end{equation*} |
where B_{i}^{n}\left(u\right), B_{j}^{m}\left(v\right) are the Bernstein basis functions and t, u\in \lbrack 0, 1] [54].
The parameter curves of a Bézier surface are spatial Bézier curves. In particular, the parameter curves X(t, 0), X(t, 1), X(0, u), X(1, u), are Bézier curves which form the four edges of the Bézier surface. A number of the properties of Bézier and B-spline surfaces can be deduced in a similar manner to the corresponding properties for curves. The details are omitted.
Theorem 5.1. (Invariance under Affine Transformations property) Let X(t, u) be given a general (n, m) Typed Bézier Surfaces with control points b_{ij} for i = 0, 1, ..., n and j = 0, 1, ..., m in R^{3} . So The Bézier surface X(t, u) satisfies as follow:
Let T be a affine transformation. Then [54].
| \begin{equation*} T\left( X(t, u)\right) = T\left( \sum\limits_{i = 1} ^n \sum\limits_{j = 1}^mB_{i}^{n}\left( t\right) B_{j}^{m}\left( u\right)b_{ij}\right) = \sum\limits_{i = 1} ^n \sum\limits_{j = 1}^mB_{i}^{n}\left( t\right) B_{j}^{m}\left( u\right) T\left(b_{ij}\right) \end{equation*} |
Definition: Let X(t, u) and Y(t, u) be given two general (n, m) Typed Bézier Surfaces with control points b_{ij} and q_{ij} for i = 0, 1, ..., n and j = 0, 1, ..., m in R^{3} . Then these Bézier Surfaces are called LS(3)- equivalent if there exist a transformation g \in LS(3) such that q_{ij} = g b_{ij} for every i = 0, 1, ..., n and j = 0, 1, ..., m are satisfied.
A (1, 1) Typed Bézier Surface X(u, v) with control points b_{00}, b_{01}, b_{10}, b_{11} is defined by
| \begin{equation*} X(u, v) = (1-u)(1-v)b_{00}+(1-u)vb_{01}+u(1-v)b_{10}+uvb_{11} \end{equation*} |
where t, u\in \lbrack 0, 1] [54].
Theorem 5.1. Let X(t, u) and Y(t, u) be given two (1, 1) Typed Bézier Surfaces with control points {b_{00}, b_{01}}, {b_{10}, b_{11}} and {p_{00}, p_{01}, {p_{10}, p_{11}}} in R^{3} respectively. So,
1. if any b_{ij} = (0, 0, 0) and each p_{ij}\neq (0, 0, 0) or each b_{ij}\neq (0, 0, 0) and any p_{ij} = (0, 0, 0) for i, j = 0, 1 then these surfaces are not LS(3)- equivalent.
2. if every b_{ij}\neq (0, 0, 0) and p_{ij}\neq (0, 0, 0) for i, j = 0, 1 then these Bézier surfaces are LS(3)- equivalent if and only if the proportions \frac{\left\langle p_{_{_{ij}}}, p_{_{_{sk}}}\right\rangle }{ \left\langle b_{_{ij}}, b_{_{sk}}\right\rangle } are the same for i, j = 0, 1 and for s, k = 0, 1
Proof. The coordinat curves X(0, u), X(1, u), X(t, 0), X(t, 1) and Y(0, u), Y(1, u), Y(t, 0), Y(t, 1) of every Bézier surfaces are Bézier curves. So to be LS(3)- equivalent of two Bézier surfaces X(t, u) and Y(t, u) , the Bézier curves with control points \left\{ {b_{00}, b_{01}}\right\} , \left\{ {b_{00}, b_{10}}\right\}, \left\{ {b_{01}, b_{11}}\right\}, \left\{ {b_{10}, b_{11}}\right\} and \left\{ {p_{00}, p_{01}}\right\} , \left\{ { p_{00}, p_{10}}\right\}, \left\{ {p_{01}, p_{11}}\right\}, \left\{ {p_{10}, p_{11}}\right\} must be mutually LS(3)- equivalent. This means that \left\{ {b_{00}, b_{01}}\right\} \overset{LS(3)}{\cong }\ \left\{ {p_{00}, p_{01}}\right\} , \left\{ {b_{00}, b_{10}}\right\} \overset{LS(3)}{\cong }\ \left\{ {p_{00}, p_{10}}\right\} , \left\{ {b_{01}, b_{11}}\right\} \overset{LS(3)}{\cong }\left\{ {p_{01}, p_{11}}\right\} , \left\{ {b_{10}, b_{11}}\right\} \overset{LS(3)}{\cong } \left\{ {p_{10}, p_{11}}\right\} must be separately satisfied. So \frac{\left\langle p_{00}, p_{00}\right\rangle }{\left\langle b_{00}, b_{00}\right\rangle } = \frac{\left\langle p_{00}, p_{01}\right\rangle }{\left\langle b_{00}, b_{01}\right\rangle } = \frac{\left\langle p_{01}, p_{01}\right\rangle }{\left\langle b_{01}, b_{01}\right\rangle } = \frac{\left\langle p_{00}, p_{10}\right\rangle }{\left\langle b_{00}, b_{10}\right\rangle } = \frac{\left\langle p_{10}, p_{10}\right\rangle }{\left\langle b_{10}, b_{10}\right\rangle } = \frac{\left\langle p_{10}, p_{11}\right\rangle }{\left\langle b_{10}, b_{11}\right\rangle } = \frac{\left\langle p_{01}, p_{11}\right\rangle }{\left\langle b_{01}, b_{11}\right\rangle } = \frac{\left\langle p_{11}, p_{11}\right\rangle }{\left\langle b_{11}, b_{11}\right\rangle } is obtained.
In Figure 4 LS(3)- equivalent (1, 1) Typed Bézier surfaces X(t, u) and Y(t, u) are plotted.
A (1, 2)-Typed Bézier surface X(u, v) with control points b_{00}, b_{01}, b_{02}, b_{10}, b_{11}, b_{12} is defined by
| \begin{equation*} X(u, v) = (1-u)(1-v)^{2}b_{00}+2(1-u)v(1-v)b_{01}+(1-u)v^{2}b_{02}+u(1-v)^{2}b_{10}+2uv(1-v)b_{11}+uv^{2}b_{12} \end{equation*} |
where u, v\in \lbrack 0, 1] [54].
A (2, 1)-Typed Bézier Surface X(u, v) with control points b_{00}, b_{01}, b_{10}, b_{11}, b_{20}, b_{21} is defined by
| \begin{equation*} X(u, v) = (1-u)^{2}(1-v)b_{00}+(1-u)^{2}vb_{01}+2u(1-u)(1-v)b_{10}+2u(1-u)vb_{11}+u^{2}(1-v)b_{20}+u^{2}vb_{21} \end{equation*} |
where u, v\in \lbrack 0, 1] [54].
Theorem 5.2. Let X(t, u) and Y(t, u) be given two (2, 1) Typed Bézier Surfaces with control points b_{00}, b_{01}, b_{10}, b_{11}, b_{20}, b_{21} and p_{00}, p_{01}, p_{10}, p_{11}, p_{20}, p_{21} in R^{3} respectively. So,
1. if any b_{ij} = (0, 0, 0) and each p_{ij}\neq (0, 0, 0) or each b_{ij}\neq (0, 0, 0) and any p_{ij} = (0, 0, 0) for i = 0, 1, 2 and j = 0, 1 then these surfaces are not LS(3)- equivalent.
2. if every b_{ij}\neq (0, 0, 0) and p_{ij}\neq (0, 0, 0) for i = 0, 1, 2 and j = 0, 1 then these Bézier surfaces are LS(3)- equivalent if and only if these ratios or proportions \frac{\left\langle p_{i0}, p_{j0}\right\rangle }{\left\langle b_{i0}, b_{j0}\right\rangle }, \frac{\left\langle p_{i1}, p_{j1}\right\rangle }{\left\langle b_{i1}, b_{j1}\right\rangle } are the same for i, j = 0, 1, 2 .
Proof. Let X(t, u) and Y(t, u) be given two (2, 1) Typed Bézier Surfaces with control points b_{00}, b_{01}, b_{10}, b_{11}, b_{20}, b_{21} and p_{00}, p_{01}, p_{10}, p_{11}, p_{20}, p_{21} in R^{3} respectively. So the coordinat curves X(0, u), X(1, u), X(t, 0), X(t, 1) and Y(0, u), Y(1, u), Y(t, 0), Y(t, 1) of every Bézier surfaces must be mutually LS(3)- equivalent. i.e. \left\{ {b_{00}, b_{01}}\right\} \overset{LS(3)}{\cong }\ \left\{ {p_{00}, p_{01}}\right\} , \left\{ {b_{00}, b_{10}, b_{20}}\right\} \overset{LS(3)}{\cong }\ \left\{ {p_{00}, p_{10}, p_{20}}\right\} , \left\{ {b_{01}, b_{11}, b_{21}}\right\} \overset{LS(3)}{\cong }\ \left\{ {p_{01}, p_{11}, p_{21}}\right\} , \left\{ {b_{20}, b_{21}}\right\} \overset{LS(3)}{\cong } \left\{ {p_{20}, p_{21}}\right\} must be satisfied. From Theorem 4.2 and Theorem 4.3, these equalities \frac{\left\langle p_{00}, p_{00}\right\rangle }{\left\langle b_{00}, b_{00}\right\rangle } = \frac{\left\langle p_{00}, p_{01}\right\rangle }{\left\langle b_{00}, b_{01}\right\rangle } = \frac{\left\langle p_{01}, p_{01}\right\rangle }{\left\langle b_{01}, b_{01}\right\rangle } ; \frac{\left\langle p_{00}, p_{00}\right\rangle }{\left\langle b_{00}, b_{00}\right\rangle } = \frac{\left\langle p_{00}, p_{10}\right\rangle }{\left\langle b_{00}, b_{10}\right\rangle } = \frac{\left\langle p_{00}, p_{20}\right\rangle }{\left\langle b_{00}, b_{20}\right\rangle } = \frac{\left\langle p_{10}, p_{10}\right\rangle }{\left\langle b_{10}, b_{10}\right\rangle } = \frac{\left\langle p_{10}, p_{20}\right\rangle }{\left\langle b_{10}, b_{20}\right\rangle } = \frac{\left\langle p_{20}, p_{20}\right\rangle }{ \left\langle b_{20}, b_{20}\right\rangle } ; \frac{\left\langle p_{01}, p_{01}\right\rangle }{\left\langle b_{01}, b_{01}\right\rangle } = \frac{\left\langle p_{01}, p_{11}\right\rangle }{\left\langle b_{01}, b_{11}\right\rangle } = \frac{\left\langle p_{01}, p_{21}\right\rangle }{\left\langle b_{01}, b_{21}\right\rangle } = \frac{\left\langle p_{11}, p_{11}\right\rangle }{\left\langle b_{11}, b_{11}\right\rangle } = \frac{\left\langle p_{11}, p_{21}\right\rangle }{\left\langle b_{11}, b_{21}\right\rangle } = \frac{\left\langle p_{21}, p_{21}\right\rangle }{\left\langle b_{21}, b_{21}\right\rangle } ; \frac{\left\langle p_{20}, p_{20}\right\rangle }{\left\langle b_{20}, b_{20}\right\rangle } = \frac{\left\langle p_{20}, p_{21}\right\rangle }{\left\langle b_{20}, b_{21}\right\rangle } = \frac{\left\langle p_{21}, p_{21}\right\rangle }{\left\langle b_{21}, b_{21}\right\rangle } can be obtained. So the minimal equivalent conditions of these surfaces is these ratios or proportions \frac{\left\langle p_{i0}, p_{j0}\right\rangle }{\left\langle b_{i0}, b_{j0}\right\rangle }, \frac{\left\langle p_{i1}, p_{j1}\right\rangle }{\left\langle b_{i1}, b_{j1}\right\rangle } are the same for i, j = 0, 1, 2 .
Theorem 5.3. Let X(t, u) and Y(t, u) be given two (1, 2) Typed Bézier Surfaces with control points b_{00}, b_{01}, b_{02}, b_{10}, b_{11}, b_{12} and p_{00}, p_{01}, p_{02}, p_{10}, p_{11}, p_{12} in R^{3} respectively. So,
1. if any b_{ij} = (0, 0, 0) and each p_{ij}\neq (0, 0, 0) or each b_{ij}\neq (0, 0, 0) and any p_{ij} = (0, 0, 0) for i = 0, 1 and j = 0, 1, 2 then these surfaces are not LS(3)- equivalent.
2. if every b_{ij}\neq (0, 0, 0) and p_{ij}\neq (0, 0, 0) for i = 0, 1 and j = 0, 1, 2 then these Bézier surfaces are LS(3)- equivalent if and only if these ratios or proportions \frac{\left\langle p_{0i}, p_{0j}\right\rangle }{\left\langle b_{0i}, b_{0j}\right\rangle }, \frac{\left\langle p_{1i}, p_{1j}\right\rangle }{\left\langle b_{1i}, b_{1j}\right\rangle } are the same for i, j = 0, 1, 2 .
Proof. As Theorem 5.2., let X(t, u) and Y(t, u) be given two (1, 2) Typed Bézier Surfaces with control points b_{00}, b_{01}, b_{02}, b_{10}, b_{11}, b_{12} and p_{00}, p_{01}, p_{02}, p_{10}, p_{11}, p_{12} in R^{3} respectively. So the coordinat curves X(0, u), X(1, u), X(t, 0), X(t, 1) and Y(0, u), Y(1, u), Y(t, 0), Y(t, 1) of every Bézier surfaces must be mutually LS(3)- equivalent. i.e. \left\{ {b_{00}, b_{10}}\right\} \overset{LS(3)}{ \cong }\ \left\{ {p_{00}, p_{10}}\right\} , \left\{ {b_{00}, b_{01}, b_{02}}\right\} \overset{LS(3)}{\cong }\ \left\{ {p_{00}, p_{01}, p_{02}}\right\} , \left\{ {b_{10}, b_{11}, b_{12}}\right\} \overset{LS(3)}{\cong }\ \left\{ { p_{10}, p_{11}, p_{12}}\right\} , \left\{ {b_{02}, b_{12}}\right\} \overset{LS(3)}{\cong } \left\{ {p_{02}, p_{12}}\right\} must be satisfied. From Theorem 4.2 and Theorem 4.3, these ratios or proportions \frac{\left\langle p_{i0}, p_{j0}\right\rangle }{\left\langle b_{i0}, b_{j0}\right\rangle }, \frac{ \left\langle p_{i1}, p_{j1}\right\rangle }{\left\langle b_{i1}, b_{j1}\right\rangle } are the same for i, j = 0, 1, 2 . can be obtained similarly with Theorem 5.2.
In Figures 5 and 6 LS(3)- equivalent (1, 2) Typed and (2, 1) Typed Bézier surfaces X(t, u) and Y(t, u) are plotted respectively.
A general (n, m)-Typed Bézier Surface X(u, v) with control points b_{ij} for i = 0, 1, ..., n and j = 0, 1, ..., m in R^{3} is defined by
| \begin{equation*} X(u, v) = \sum\limits_{i = 1} ^n \sum\limits_{j = 1}^mB_{i}^{n}\left( u\right) B_{j}^{m}\left( v\right) b_{ij} \end{equation*} |
where B_{i}^{n}\left(u\right), B_{j}^{m}\left(v\right) are the Bernstein basis functions and t, u\in \lbrack 0, 1] [54].
Theorem 5.4. Let X(t, u) and Y(t, u) be given two general (n, m) Typed Bézier Surfaces with control points b_{ij} and p_{ij} for i = 0, 1, ..., n and j = 0, 1, ..., m in R^{3} respectively. So,
1. if any b_{ij} = (0, 0, 0) and each p_{ij}\neq (0, 0, 0) or each b_{ij}\neq (0, 0, 0) and any p_{ij} = (0, 0, 0) then these surfaces are not LS(3)- equivalent.
2. if every b_{ij}\neq (0, 0, 0) and p_{ij}\neq (0, 0, 0) then these Bézier surfaces are LS(3)- equivalent if and only if
\frac{\left\langle p_{00}, p_{u0}\right\rangle }{\left\langle b_{00}, b_{u0}\right\rangle } , \frac{\left\langle p_{10}, p_{v0}\right\rangle }{\left\langle b_{10}, b_{v0}\right\rangle } , \frac{\left\langle p_{20}, p_{w0}\right\rangle }{\left\langle b_{20}, b_{w0}\right\rangle } ; \frac{\left\langle p_{00}, p_{0i}\right\rangle }{\left\langle b_{00}, b_{0i}\right\rangle } , \frac{\left\langle p_{01}, p_{0j}\right\rangle }{\left\langle b_{01}, b_{0j}\right\rangle } , \frac{\left\langle p_{02}, p_{0k}\right\rangle }{\left\langle b_{02}, b_{0k}\right\rangle } ; \frac{\left\langle p_{0m}, p_{im}\right\rangle }{\left\langle b_{0m}, b_{im}\right\rangle } , \frac{\left\langle p_{1m}, p_{jm}\right\rangle }{\left\langle b_{1m}, b_{jm}\right\rangle } , \frac{\left\langle p_{2m}, p_{km}\right\rangle }{\left\langle b_{2m}, b_{km}\right\rangle } ; \frac{\left\langle p_{n0}, p_{ni}\right\rangle }{\left\langle b_{n0}, b_{ni}\right\rangle } , \frac{\left\langle p_{n1}, p_{nj}\right\rangle }{\left\langle b_{n1}, b_{nj}\right\rangle } , \frac{\left\langle p_{n2}, p_{nk}\right\rangle }{\left\langle b_{n2}, b_{nk}\right\rangle } for u = 0, 1, ..., n , v = 1, ..., n ; w = 2, ..., n , i = 0, 1, ..., m , j = 1, ..., m ; k = 2, ..., m must be same.
Proof. As Theorem 5.2. and Theorem 5.3., let X(t, u) and Y(t, u) be given two (n, m) Typed Bézier Surfaces with control points b_{ij} and p_{ij} for i = 0, 1, ..., n and j = 0, 1, ..., m in R^{3} respectively. So the coordinat curves X(0, u), X(1, u), X(t, 0), X(t, 1) and Y(0, u), Y(1, u), Y(t, 0), Y(t, 1) of each Bézier surfaces must be mutually LS(3)- equivalent. i.e. \left\{ {b_{00}, b_{10}, ..., b}_{n0}\right\} \overset{ LS(3)}{\cong }\ \left\{ {p_{00}, p_{10}, ..., p}_{n0}\right\} , \left\{ { b_{00}, b_{01}, ..., b_{0m}}\right\} \overset{LS(3)}{\cong }\ \left\{ { p_{00}, p_{01}, ..., p_{0m}}\right\} , \left\{ {b_{n0}, b_{n1}, ..., b_{nm}} \right\} \overset{LS(3)}{\cong }\ \left\{ {p_{n0}, p_{n1}, ..., p_{nm}}\right\} , \left\{ {b_{0m}, b_{1m}, ..., b}_{nm}\right\} \overset{LS(3)}{\cong } \left\{ {p_{0m}, p_{1m}, ..., p}_{nm}\right\} must be satisfied. Then from Theorem 4.5. these proportions \frac{\left\langle p_{00}, p_{u0}\right\rangle }{\left\langle b_{00}, b_{u0}\right\rangle } , \frac{\left\langle p_{10}, p_{v0}\right\rangle }{\left\langle b_{10}, b_{v0}\right\rangle } , \frac{\left\langle p_{20}, p_{w0}\right\rangle }{\left\langle b_{20}, b_{w0}\right\rangle } ; \frac{\left\langle p_{00}, p_{0i}\right\rangle }{\left\langle b_{00}, b_{0i}\right\rangle } , \frac{\left\langle p_{01}, p_{0j}\right\rangle }{\left\langle b_{01}, b_{0j}\right\rangle } , \frac{\left\langle p_{02}, p_{0k}\right\rangle }{\left\langle b_{02}, b_{0k}\right\rangle } ; \frac{\left\langle p_{0m}, p_{im}\right\rangle }{\left\langle b_{0m}, b_{im}\right\rangle } , \frac{\left\langle p_{1m}, p_{jm}\right\rangle }{\left\langle b_{1m}, b_{jm}\right\rangle } , \frac{\left\langle p_{2m}, p_{km}\right\rangle }{\left\langle b_{2m}, b_{km}\right\rangle } ; \frac{\left\langle p_{n0}, p_{ni}\right\rangle }{\left\langle b_{n0}, b_{ni}\right\rangle } , \frac{\left\langle p_{n1}, p_{nj}\right\rangle }{\left\langle b_{n1}, b_{nj}\right\rangle } , \frac{\left\langle p_{n2}, p_{nk}\right\rangle }{\left\langle b_{n2}, b_{nk}\right\rangle } for u = 0, 1, ..., n , v = 1, ..., n ; w = 2, ..., n , i = 0, 1, ..., m , j = 1, ..., m ; k = 2, ..., m must be same.
One of the most important applicaitons of Bézier curves in CAD systems is font design. Mostly quadratic and Cubic Bézier curves are used in this area. Higher degree curves are more computationally expensive to evaluate. When more complex shapes are needed, low order Bézier curves are patched together, producing a composite Bézier curve. A composite Bézier curve is commonly referred to as a "path" in vector graphics languages (like PostScript), vector graphics standards (like SVG) and vector graphics programs (like Artline, Timeworks Publisher, Adobe Illustrator, CorelDraw and Inkscape) [55].
TrueType fonts use composite Bézier curves composed of quadratic Bézier curves. Other languages and imaging tools (such as PostScript, Asymptote, Metafont, and SVG) use composite Béziers composed of Cubic Bézier curves for drawing curved shapes. OpenType fonts can use either kind, depending on the flavor of the font. [55,56]. Some other studies can be seen in [38,57,58]. An example to design a typeface of letter 's' can be given as follows:
To design a typeface of letter S the anchor and other control points are determined firstly. The anchor points lie on the curve "s", and other control points are outside of this curve. Let's use composite Bézier curves composed of quadratic Bézier curves even though it has disadvantages such as the need to add more anchor nodes and big file sizes. This typeface have 7 anchor points and 6 other control points (see in Figure 7). These points can be choose as follows:
The anchor points: A = (-1, 1) , C = (0, 0) , E = (1, 1) , G = (0, 2) , I = (-1, 3) , L = (0, 4) , and N = (1, 3) . The other control points B = (-0.5, 0) , D = (0.5, 0) , F = (0.5, 2) , H = (-0.5, 2) , K = (-0.5, 4) , M = (0.5, 4) .
If these curve segments on the letter "S" are denoted as follows:
From the point N to the point L as \alpha_{1} , from the point L to the point I as \alpha_{2} , from the point I to the point G as \alpha_{3} , from the point G to the point E as \alpha_{4} , from the point E to the point C as \alpha_{5} and from the point C to the point A as \alpha_{6} then these quadratic Bézier curves \alpha_{1} , \alpha_{2} , \alpha_{3} , \alpha_{4} , \alpha_{5} , and \alpha_{6} form the composite Bézier curve \alpha .
So these points N, M, L; L, K, I; I, H, G; G, F, E; E, D, C; C, B, A are the control points of these quadratic Bézier curves \alpha_{i} respectively for i = 1, ..., 6 .
Considering the first coordinates as 0, these points A to N can be recognized as in 3 dimensional points. Now let's apply any g \in LS(3) transform to this created letter S. It can be used to obtain different font shadows.Let the transform g be choosen as g = \left(\begin{array}{ccc} 0 & 0 & -1\\ 0 & 1 & 0 \\ 1 & 0 & 0 \\ \end{array} \right)
which rotates all points with 90 degree in the xz- plane.
The points A to N transform the points A' to N' with this transformation g . Thus, the anchor points transform to these points: A' = (-1, -1, 0) , C' = (0, 0, 0) , E' = (-1, 1, 0) , G' = (-2, 0, 0) , I' = (-3, -1, 0) , L' = (-4, 0, 0) , and N' = (-3, 1, 0) . The other control points transform to points B' = (0, -0.5, 0) , D' = (0, 0.5, 0) , F' = (-2, 0.5, 0) , H' = (-2, -0.5, 0) , K' = (-4, -0.5, 0) , M' = (-4, 0.5, 0) . From Theorem 4.1 the composite Bézier curve \alpha transform the composite Bézier curve \beta consisted of \beta_{i} for i = 1, ..., 6 with this transformation g . From Theorem 4.1 all of these curves \beta_{i} are also quadratic Bézier curves which control points N', M', L'; L', K', I'; I', H', G'; G', F', E'; E', D', C'; C', B', A' respectively for i = 1, ..., 6 . According to this the control points A to N are LS(3) -equivalent to the points A' to N'. So the composite Bézier curve \alpha is LS(3) -equivalent to the composite Bézier curve \beta . In Figure 8 the composite Bézier curves \alpha and \beta is given together.
Let the transform g be choosen as g = \left(\begin{array}{ccc} 0 & -\sqrt{2} & -\sqrt{2} \\ 0 & \sqrt{2} & -\sqrt{2} \\ 2 & 0 & 0 \\ \end{array} \right)
which rotates all points with 90 degree in the xz- plane and rotates all points with 45 degree in the xy- plane with scale \lambda = 2 .
The points A to N transform the points A" to N" with this transformation g . Thus, the anchor points transform to these points: A'' = (0, -2\sqrt{2}, 0) , C'' = (0, 0, 0) , E'' = (-2\sqrt{2}, 0, 0) , G'' = (-2\sqrt{2}, -2\sqrt{2}, 0) , I'' = (-2\sqrt{2}, -4\sqrt{2}, 0) , L'' = (-4\sqrt{2}, -4\sqrt{2}, 0) , and N'' = (-4\sqrt{2}, -2\sqrt{2}, 0) . The other control points transform to points B'' = (\frac{\sqrt{2}}{2}, -\frac{\sqrt{2}}{2}, 0)) , D'' = (-\frac{\sqrt{2}}{2}, \frac{\sqrt{2}}{2}, 0) , F'' = (-5\frac{\sqrt{2}}{2}, -3\frac{\sqrt{2}}{2}, 0) , H'' = (-3\frac{\sqrt{2}}{2}, -5\frac{\sqrt{2}}{2}, 0) , K'' = (-7\frac{\sqrt{2}}{2}, -9\frac{\sqrt{2}}{2}, 0) , M'' = (-9\frac{\sqrt{2}}{2}, -7\frac{\sqrt{2}}{2}, 0) . Also From Theorem 4.1 the composite Bézier curve \alpha transform the composite Bézier curve \gamma consisted of \gamma_{i} for i = 1, ..., 6 with this transformation g . From Theorem 4.1 all of these curves \gamma_{i} are also quadratic Bézier curves which control points N'', M'', L'' , L'', K'', I'' , I'', H'', G'' , G'', F'', E'' , E'', D'', C'' , C'', B'', A'' respectively for i = 1, ..., 6 . According to this the control points A to N are LS(3) -equivalent to the points A'' to N'' . So the composite Bézier curve \alpha is LS(3) -equivalent to the composite Bézier curve \gamma . In Figure 9 the composite Bézier curves \alpha and \gamma is given together.
In the other view if a shadow of a typeface could not read clearly then the operation process mentioned above can be apply by reversed to obtain more readable typefaces.
The authors are grateful to the editors and referees for giving every useful piece of information they have checked and developed.
The authors declare no conflict of interest.
| [1] | G. Birkhoff, Hydrodynamics, 2Eds., New Jersey: Princeton Univ. Press, 1960. |
| [2] | J. B. J. Fourier, Theoric Analytique de la Chaleur, 1822, English Transl. By A. Freeman, The Analytical Theory of Heat, Cambridge University Press, 1878. |
| [3] | M. Incesu, The Complete System of Point Invariants in the Similarity Geometry, Phd. Thesis, Karadeniz Technical University, Trabzon, 2008. |
| [4] | M. Nagata, Local Rings, Interscience, NY, 1962. |
| [5] |
D. Hadjiev, Some questions in the theory of vector invariants, Math. USSR-Sbornic, 1 (1967), 383-396. doi: 10.1070/SM1967v001n03ABEH001988

|
| [6] |
F. Grosshans, Obsevable groups and Hilbert's problem, Am. J. Math., 95 (1973), 229-253. doi: 10.2307/2373655

|
| [7] |
F. Klein, A comperative review of recent researches in geometry (translated by Dr. M.W. Haskell), Bull. New York Math. Soc., 2 (1893), 215-249. doi: 10.1090/S0002-9904-1893-00147-X

|
| [8] | P. W. Bridgman, Dimensional Analysis, 2Eds., Yale University Press, New Heaven, 1931. |
| [9] | L. I. Sedov, Similarity and Dimensional Method in Mechanics, English Tr. By V. Kisin, Mir Publishers, USSR, 1982. |
| [10] | H. L. Langhaar, Dimensional Analysis and Theory of Models, Wiley, 1951. |
| [11] | H. Weyl, The Classical Groups, Their Invariants and Representations, 2Eds., with suppl., Princeton, Princeton University Press, 1946. |
| [12] | D. Khadjiev, An Application of the Invariant Theory to the Differential Geometry of Curves, (in Russian) Fan, Tashkent, 1988. |
| [13] | M. Incesu, O. Gursoy, LS (2)-Equivalence conditions of control points and application to planar Bézier curves, New Trends Math. Sci., 5 (2017), 70-84. |
| [14] | Y. Sagiroglu, The equivalence problem for parametric curves in one-dimensional affine space, Int. Math. Forum, 6 (2011), 177-184. |
| [15] | Y. Sagiroglu, Equi-affine differential invariants of a pair of curves, TWMS J. Pure Appl. Math., 6 (2015), 238-245. |
| [16] | Y. Sagiroglu, O. Peksen, The equivalence Of Centro-Equiaffine curves, Turk. J. Math., 34 (2010), 95-104. |
| [17] |
I. Oren, Complete system of invariants of subspaces of Lorentzian space, Iran. J. Sci. Technol. A, 40 (2016),1-8. doi: 10.1007/s40995-016-0003-1

|
| [18] | I. Oren, On invariants of m-vector in Lorentzian geometry, Int. Electron. J. Geom., 9 (2016), 38-44. |
| [19] | D. Khadjiev, I. Oren, O. Peksen, Generating systems of differential invariants and the theorem on existence for curves in the pseudo-Euclidean geometry, Turk. J. Math., 37 (2013), 80-94. |
| [20] | M. Incesu, O. Gürsoy, Düzlemsel Bézier Eǧrilerinin S(2)-Denklik Şartları, MSU. J. Sci., 5 (2017), 471-477. |
| [21] | G. Farin, Curves and Surfaces for Computer Aided Geometric Design A Practical Guide, 2Eds., Academic Press Inc., San Diago, 1990. |
| [22] | R. Barnhill, R. F. Riesenfeld, Computer aided Geometric Design, Academic Press, 1974. |
| [23] |
R. Farouki, V. T. Rajan, On the numerical condition of polynomials in Bernstein form, Comput. Aided Geom. D., 4 (1987), 191-216. doi: 10.1016/0167-8396(87)90012-4

|
| [24] |
G. Farin, Curvature continuity and offsets for piecewise conics, ACM T. Graphic, 8 (1989), 89-99. doi: 10.1145/62054.62056

|
| [25] |
R. Farouki, Exact offsets procedures for simple solids, Comput. Aided. Geom. D., 2 (1985), 257-279. doi: 10.1016/S0167-8396(85)80002-9

|
| [26] |
J. Hoschek, Offset curves in the plane, Comput. Aided. Des., 17 (1985), 77-82. doi: 10.1016/0010-4485(85)90249-0

|
| [27] | W. Tiller, E. Hanson, Offsets of two-dimentional profiles, IEEE Comput. Graph., 4 (1984), 36-46. |
| [28] |
H. Potmann, Rational curves and surfaces with rational offsets, Comput. Aided. Geom. D., 12 (1995), 175-192. doi: 10.1016/0167-8396(94)00008-G

|
| [29] |
R. Farouki, C. Manni, A. Sestini, Real-time CNC interpolators for Bézier conics, Comput. Aided. Geom. D., 18 (2001), 639-655. doi: 10.1016/S0167-8396(01)00058-9

|
| [30] |
F. Chen, J. Zheng, T. W. Sederberg, The mu-basis of rational ruled surface, Comput. Aided Geom. D., 18 (2001), 61-72. doi: 10.1016/S0167-8396(01)00012-7

|
| [31] |
H. Pottmann, P. Paukowitsch, Inflections of planar surface curves, Comput. Aided Geom. D., 14 (1997), 293-297. doi: 10.1016/S0167-8396(96)00052-0

|
| [32] |
R. Krasauskas, Toric surface patches, Adv. Comput. Math., 17 (2002), 89-113. doi: 10.1023/A:1015289823859

|
| [33] |
J. S. Reyes, Harmonic rational Bézier curves, p-Bézier curves and trigonometric polynomials, Comput. Aided Geom. D., 15 (1998), 909-923. doi: 10.1016/S0167-8396(98)00031-4

|
| [34] |
B. Jüttler, M. G. Wagner, Computer-aided design with spatial rational B-Spline motions, J. Mech. Des-T. ASME, 118 (1996), 193-201. doi: 10.1115/1.2826869

|
| [35] |
Q. J. Ge, M. Sirchia, Computer aided geometric design of two-parameter freeform motions, J. Mech. Des-T. ASME, 121 (1999), 502-506. doi: 10.1115/1.2829489

|
| [36] |
C. Mavroidis, B. Roth, On the geometry of spatial polygons and screw polygons, J. Mech. Des.-T. ASME, 119 (1997), 246-252. doi: 10.1115/1.2826243

|
| [37] |
R. Cholewa, A. J. Nowak, R. A. Bialecki, et al., Cubic Bézier Splines for BEM heat transfer analysis of the 2-D continuous casting problems, Comput. Mech., 28 (2002), 282-290. doi: 10.1007/s00466-001-0288-5

|
| [38] |
M. Sarfraz, M. A. Khan, Automatic outline capture of Arabic fonts, Inform. Sci., 140 (2002), 269-281. doi: 10.1016/S0020-0255(01)00176-1

|
| [39] | X. Xu, Integrating Advanced Computer-Aided Design, Manufacturing and Numerical Control: Principles and Implementations, IGI Global, Hershey, London, 2009. |
| [40] | H. K. Samanci, S. Celik, M. Incesu, The Bishop Frame of Bézier Curves, Life Sci. J., 12 (2015), 175-180. |
| [41] |
V. Bulut, A. Caliskan, The Exchange Variations Between Bézier Directrix Curves of Two Developable Ruled Surfaces, J. Dynamical Sys. Geom. Theories, 13 (2015), 103-114. doi: 10.1080/1726037X.2015.1076225

|
| [42] | H. K. Samanci, Generalized dual-variable Bernstein polynomials, Konuralp J. Math., 5 (2016), 56-67. |
| [43] | H. K. Samanci, Some geometric properties of the spacelike Bézier curve with a timelike principal normal in Minkowski 3-space, Cumhuriyet Sci. J., 39, (2018), 71-79. |
| [44] |
E. Erkan, S. Yuce, Serret-Frenet frame and curvatures of Bézier curves, Mathematics, 6 (2018), 321-351. doi: 10.3390/math6120321

|
| [45] |
G. Hu, J. L. Wu, X. Q. Qin, A newapproach in designing of local controlled developable H-Bézier surfaces, Adv. Eng. Soft., 121 (2018), 26-38. doi: 10.1016/j.advengsoft.2018.03.003

|
| [46] |
G. Hu, J. L. Wu, X. Q. Qin, A novel extension of the Bézier model and its applications to surface modeling, Adv. Eng. Soft., 125 (2018), 27-54. doi: 10.1016/j.advengsoft.2018.09.002

|
| [47] | H. K. Samanci, O. Kalkan, S. Celik, The timelike Bézier spline in Minkowski 3-space, J. Sci. Arts, 19 (2019), 357-374. |
| [48] | G. Hu, J. L. Wu, Generalized quartic H-Bézier curves: Construction and application to developable surfaces, Adv. Eng. Soft., 138 (2019), 102723. |
| [49] |
P. Y. Gousenbourger, E. Massart, P. A. Absil, Data fitting on manifolds with composite Bézier-like curves and blinded cubic splines, J. Math. Imaging Vis., 61 (2019), 645-671. doi: 10.1007/s10851-018-0865-2

|
| [50] |
F. AlMachot, M. Ali, A. H. Mosa, et al., Real-time raindrop detection based on cellular neural networks for ADAS, J. Real-Time Image Pr., 16 (2019), 931-943. doi: 10.1007/s11554-016-0569-z

|
| [51] |
S. Baydas, B. Karakas, Detecting a curve as a Bézier curve, J. Taibah Univ. Sci., 13 (2019), 522-528. doi: 10.1080/16583655.2019.1601913

|
| [52] |
N. Ausheva, V. Olevskyi, Y. Olevska, Modelling of minimal surface based on an isotropic Bézier curve of fifth Order, J. Geom. Symmetry Phys., 52 (2019), 1-15. doi: 10.7546/jgsp-52-2019-1-15

|
| [53] | M. Incesu, O. Gursoy, The similarity invariants of Bézier curves and surfaces, Nat. Math. Symp., Ataturk University, Erzurum, Turkey, 2007. |
| [54] | D. Marsh, Applied geometry for computer graphics and CAD, Springer-Verlag London Berlin Heidelberg, London, 1999. |
| [55] | Wikipedia, the free encyclopedia. (2019) Available from: https://en.wikipedia.org/wiki/B/ezier_curve. |
| [56] | LÝnoType, Font Technology, Monotype GmbH. (2017) Available from: https://web.archive.org/web/20170703232935/https://www.linotype.com/8120/the-difference-between-cff-and-ttf.html. |
| [57] | The Mathematics behind Font Shapes, Bézier Curves and More, jdhao's blog. (2018) Available from: https://jdhao.github.io/2018/11/27/font_shape_mathematics_bezier_curves/. |
| [58] | TrueType fundamentals, Microsoft truetype font documentation. (2019) Available from: https://docs.microsoft.com/en-us/typography/opentype/spec/ttch01#from-design-to-font-file. |
| 1. | Muhsin İNCESU, Osman GÜRSOY, R3 de Açık B-Spline Eğrilerinin G-Benzerlikler, 2020, 2147-7930, 10.18586/msufbd.807153 | |
| 2. | HATİCE KUŞAK SAMANCI, SERKAN ÇELİK, MUHSİN İNCESU, THE SHAPE OPERATOR OF THE BEZIER SURFACES IN MINKOWSKI-3 SPACE, 2020, 20, 2068-3049, 865, 10.46939/J.Sci.Arts-20.4-a08 | |
| 3. | Muhsin Incesu, Sara Yilmaz Evren, Osman Gursoy, 2021, Chapter 11, 978-981-16-8176-9, 167, 10.1007/978-981-16-8177-6_11 | |
| 4. | HATİCE KUŞAK SAMANCI, THE SERRET-FRENET FRAME OF THE RATIONAL BEZIER CURVES IN THE EUCLIDEAN-3 SPACE BY ALGORITHM METHOD, 2021, 21, 2068-3049, 721, 10.46939/J.Sci.Arts-21.3-a12 | |
| 5. | Şeyda KILIÇOGLU, Süleyman ŞENYURT, On the matrix representation of 5th order B\'{e}zier Curve and derivatives in E$^{3}$, 2022, 71, 1303-5991, 133, 10.31801/cfsuasmas.895598 | |
| 6. | Hüsnü Anıl Çoban, Detecting similarities of rational plane curves using complex differential invariants, 2024, 34, 0218-1967, 35, 10.1142/S0218196723500686 | |
| 7. | Hatice Kuşak Samancı, Algorithmic computation of the bishop frame of the rational Bezier curves with augmented reality applications, 2025, 0264-4401, 10.1108/EC-06-2024-0499 |