1.
Introduction
In this paper, we consider the following two-dimensional multi-term time fractional diffusion equation:
where f∈C(ˉQ), Ω⊂R2. In Eq(1.1a), Dαtu denotes the multi-term fractional derivative, which is defined by
where J is a positive integer, bm≥0, α:=(α1,⋯,αJ) with 0<αJ<⋯<α1<1, and the fractional Caputo derivative Dαtu(0<α<1) in Eq (1.2) is defined by
For existence and uniqueness of the exact solution for problem (1.1), one can refer to [15,17]. The initial weak singularity of the solution for problem (1.1) is given in [9]. In recent years, many authors have investigated single-term time-fractional diffusion equations, see, e.g., [16,28,30]. At the same time, multi-term fractional equations [12,22,26] which have been successfully used in application of real life have attracted more and more attention. Among many types of fractional derivatives, some researchers have used Riemann-Liouville derivative [7,25], while others, including this article, choose to use Caputo derivative [13,27,30]. However, most of the previous work assumes that the exact solutions in temporal direction are smooth enough, whereas the solutions typically exhibit some weak singularities at initial time. Alternating direction implicit (ADI) method was first introduced in [18], the advantage of the ADI method is that it can reduce the computational cost by transforming a multi-dimensional problem into sets of 1D problems. Nowadays, there are many researchers using ADI method to solve various types of fractional derivative problems, such as [2,6,14,19,20,21,23,31]. Recently, Huang et al. [11] have presented an ADI scheme for 2D multi-term time-space fractional nonlinear diffusion-wave equations under reasonable solution regularity assumption. Cao and Chen [1] have used ADI difference method on uniform mesh to solve a 2D multi-term time-fractional subdiffusion equation with initial singularity. However, the global accuracy in time direction of [1] is low. It is only O(τα). So, our current work is on graded mesh to improve the temporal accuracy. More precisely, we investigate a fully discrete ADI method for solving the problem (1.1) with weakly singular solution, where the temporal discretization is based L1 approximation on graded mesh, and finite difference method is used for spatial discretization. We establish the stability and convergence of the fully discrete L1-ADI scheme, both L2-norm and H1-norm error estimates are obtained, and the final error bounds do not blow up when α1→1−.
The rest of the paper is organized as follows. In Section 2, we construct a fully discrete L1-ADI scheme for problem (1.1). In Section 3, we establish the stability and convergence L1-ADI scheme in discrete L2-norm. Then, the sharp H1-norm convergence of L1-ADI scheme is presented in Section 4. Some numerical experiments are given in Section 5. The final part is the conclusion.
Notation. Throughout the paper, we denote by C a generic positive constant, which may change its value at different occurrences, but is always independent of the mesh sizes. We call a constant C α-robust, if it doesn't blow up when α1→1−.
2.
Fully discrete L1-ADI scheme
In the whole paper, to simplify the analysis, let us choose Ω=(0,L)×(0,L), where L>0 is constant. We use positive integers N1, N2 and M respectively to define the spatial and temporal partition parameters. Consider the graded temporal grid in [0,T]:tj=T(j/M)r, j=0,1,…,M and r≥1. Denote time step τn:=tn−tn−1 for n=1,⋯,M. Then, we use xm=mh1 for m=0,1,…,N1 and yn=nh2 for n=0,1,…,N2 to denote the spatial grids, where h1=L/N1, h2=L/N2. Set Ω∗h={(xm,yn)|0≤m≤N1,0≤n≤N2}, Ωh=Ω∗h∩Ω, and ∂Ωh=Ω∗h∩∂Ω.
We approximate the Caputo fractional derivative Dαmtu(⋅,⋅,tn) with 0<αm<1 and 1≤n≤M on graded mesh by using well-known L1 scheme as follows:
where
Then, we denote
Thus one can approximate the multi-term fractional derivative Dαtu in Eq (1.2) by
Given a mesh function{vj}Mj=0, and for 0≤n≤M, we define
The notations δyvni,j−12, δ2yvni,j and δxδyvni−12,j−12 can be defined similarly. We define the discrete Laplace operator Δh:=δ2x+δ2y which is a second-order approximation of Δ. Let uni,j be the numerical approximation of the exact solution u(xi,yj,tn), so the discrete problem of (1.1) is as follows
To solve 2D problem, we want to solve 1D problem at first, after that we solve another 1D problem. If a small term γ2nδ2xδ2yδαtuni,j for n=1,…,M, where γn=z−1n,1, is added to the left side of Eq (2.5a), one gets
Thus the purpose is achieved as Eq (2.6) can be rewritten by
We set u∗i,j=(1−γnδ2y)ui,j. Then, the first 1D problem we need to solve is, for 1≤j≤N2−1,
and the second 1D problem we need to solve is, for 1≤i≤N1−1,
Thus, we have the following fully discrete ADI scheme for the problem (1.1):
3.
Analysis of stability and convergence
We define the convolution multipliers σn,j, which is positive for n=1,2,…,M and j=1,2,…,n−1 by
We have the following two lemmas on the properties of the convolution multipliers σn,j.
Lemma 1. ([10, Corollary 1]) One has
Lemma 2. ([10, Corollary 2]) Set lM=1/lnM. Assume that M≥3 so 0<lM<1. Then
To investigate the stability and convergence of the fully discrete L1-ADI scheme (2.9), we need the following fractional discrete Gronwall inequality.
Lemma 3. [8, Lemma 5.3] Suppose that the sequences {εn}∞n=1,{ηn}∞n=1 are nonnegative and assume the grid function {Vn:n=0,1,…,M} satisfies V0≥0 and
Then,
Set
For any mesh functions u,v∈Vh, define the discrete L2 inner product (u,v)=h1h2∑N1−1i=1∑N2−1j=1ui,jvi,j, and the norm ‖v‖=√(v,v). We also define a new inner product (u,v)γn:=(u,v)+ γ2n(u,v)xy, where
and set ‖u‖γn=√(u,u)γn.
Lemma 4. [1, Lemma 3] The inner product (u,v)γn satisfies
Lemma 5. For n=1,2,…,M, the solution of ADI scheme (2.9) satisfies
Proof. On both sides of Eq (2.9a), we take the discrete inner product with un. Then, one has
Then, by linear property of the inner product and discrete Green formula, the Eq (3.2) becomes
Using Lemma 4, one has
which is equivalent to
Then, setting all ηn=0 in Lemma 3, and applying it to Eq (3.3), the result is proved. □
From [3, Lemmas 2.2 and 2.3], one can easily get that
Lemma 6. Set σ∈(0,1). Suppose that |u(k)(t)|≤C(1+tσ−k) where k=0,1,2. Then, for 1≤n≤M, one has
Now we come to the convergence of the fully discrete ADI scheme (2.9).
Theorem 1. Suppose that {|u(l)(t)|≤C(1+tσ−l)} for l=0,1,2 with σ∈(0,1). Then the computed solution errors eni,j:=u(xi,yj,tn)−uni,j satisfy:
where C is α-robust.
Proof. From Eqs (2.9a) and (1.1a), we get the following error equation:
where ϕni,j is the truncation error. By the well-known simple bound |Δu−Δhu|≤C(h21+h22), |γ2n|≤CM−2α1, and Lemma 6, the truncation error ϕni,j satisfies
From Lemma 5, and noting that e0=0, we have
Then, using Lemmas 1 and 2, we can get
where C is α-robust. Finally, according to the definition of the norm ‖⋅‖γn, the proof is completed. □
4.
H1-norm convergence of L1-ADI scheme
In order to prove the stability and convergence of the fully discrete ADI scheme (2.9) in H1-norm sense in this section, we first define some norms. For any grid functions U,V∈Vh, define
The corresponding seminorms are
For any function V∈Vh, define
According to the definition of ‖∇hVn‖ and ‖Vn‖A, we can see that ‖∇hVn‖≤‖Vn‖A. From [31, Lemma 2.2], one has ‖Vn‖≤C‖∇hVn‖ for all functions V∈Vh, then, we have ‖Vn‖H1≤C‖∇hVn‖, hence, ‖Vn‖H1≤C‖Vn‖A.
Lemma 7. [29, Lemma 3.2] For any grid functions U,V∈Vh, one has
where the equality holds when U=V.
Next, we denote Rtuni,j=(1+γ2nδ2xδ2y)δαtu(xi,yj,tn)−Dαtu(xi,yj,tn), Rsuni,j=Δu(xi,yj,tn)−Δhu(xi,yj,tn). Then the error equation (3.4) can be written as
Besides, we have
and
Theorem 2. Suppose that |u(l)(t)|≤C(1+tσ−l) for l=0,1,2 with σ∈(0,1). Then, the computed solution error eni,j:=u(xi,yj,tn)−uni,j satisfy:
where C is α-robust.
Proof. Taking discrete L2 inner product with −Δhen on both sides of Eq (4.1) and noting that e0=0, we get
Using Lemma 7, one has
From Eqs (4.2) and (4.3), we obtain
that is
Thus, by Lemma 3, from Eq (4.5) we have
Then, using Lemmas 1 and 2, we come to the conclusion
by noting that
and
Finally, the results follow from ‖en‖H1≤C‖en‖A for n=1,2,…,M. The proof is completed. □
5.
Numerical experiments
In this section, some numerical examples are reported to support our theoretical analysis. In this section, we present some 2D numerical examples to illustrate the result of our error analysis and convergence order on the temporal graded mesh. We define max1≤n≤M‖u(tn)−un‖ as temporal global L2-norm error and global H1-norm error is defined similarly.
Example 1. In Eq (1.1) take Ω=[0,π]×[0,π], T=1, J=2, b1=b2=1. Choose u(x,y,t)=tα1sinxsiny as an exact solution. The force term f(x,y,t)=b1Γ(1+α1)+b2Γ(1+α1)tα1−α2Γ(1−α2+α1)sinxsiny+2tα1sinxsiny.
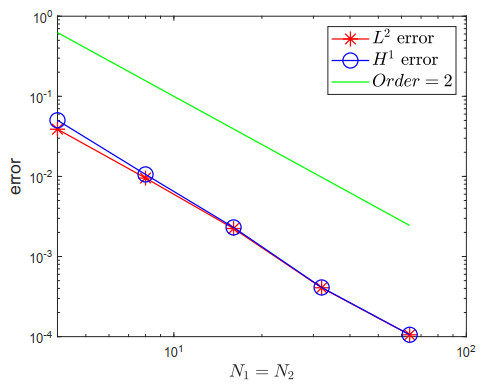
For this example, the regularity parameter σ in Lemma 6 is σ=α1. Thus, both the temporal convergence orders in Theorems 1 and 2 are O(M−min{2α1,rα1,2−α1}). We take N1=N2=1000 to eliminate the contamination of spatial errors. Tables 1–3 present L2-norm errors and orders of convergence for Example 1 with different α1, α2 and r. And Tables 4, 6 and 8 present H1-norm errors and convergence orders. From these tables, we find that the temporal convergence orders are consistent with our theoretical results. As in [1], we also present the local H1-norm errors and orders of convergence at t=1 for Example 1 in Tables 5, 7 and 9, which have better convergence rates than the global errors. We next test the spatial accuracy of the fully discrete ADI scheme (2.9). Take M=1000 such that the spatial errors are dominant. We using α1=0.6, α2=0.4 and r=2 as an example and the results are presented in Figure 1. The results show that the spatial convergence orders are second order in L2 and H1-norm sense as is indicated in Theorems 1 and 2.
Example 2. In Eq (1.1) take Ω=[0,π]×[0,π], T=1, J=2, b1=b2=1. Let u0(x,y)=sinxsiny, the force term f(x,y,t)=0.
The exact solution u(x,y,t) of Example 2 is unknown. We use the two-mesh principle in [4, p107] to calculate the convergence order of the numerical solution. Let uni,j with 0≤i≤N1, 0≤j≤N2 and 0≤n≤M be the solution computed by our L1-ADI Scheme 2.6. Then, consider a spatial mesh and the temporal mesh which is defined by 0≤i≤N1, 0≤j≤N2 and tn=T(n/(2M)) for 0≤n≤2M. We denote Wnh with 0≤n≤2M as the computed solution on this mesh. Then, we define Dnh=‖unh−W2nh‖H1 as the H1-norm of the two-mesh differences and the estimated rate of convergence is computed by log2(Dnh/D2nh).
To test the temporal convergence of our scheme we set spatial partition parameters N1=N2=1000. Tables 10, 12 and 14 present H1-norm errors and convergence orders for Example 2 in the case of α1=0.4,0.6,0.8 with α2=0.2,0.4,0.6. The results show that the temporal convergence rates are O(M−min{2α1,rα1,2−α1}), which, once again, confirm the sharpness of our theoretical analysis. We have also present the local H1-norm errors and convergence orders at t=1 for Example 2 in Tables 11, 13 and 15, which have better convergence rates than global errors.
6.
Conclusion
A fully discrete L1-ADI scheme is investigated for the initial-boundary problem of a multi-term time-fractional diffusion equation. Stability and convergence of the fully discrete L1-ADI scheme are rigorously established. Both L2-norm and H1-norm error estimates of the fully discrete L1-ADI scheme are obtained, and they are α-robust. Numerical experiments are given to illustrate the sharpness of the theoretical analysis. It should be noted that since the computational cost of numerical methods for time-fractional PDEs will be very time-consuming. One can also use the fast and parallel numerical methods such as [5,24,32] for accelerating the proposed method, which will be the focus of our future work.
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
The research is supported in part by the National Natural Science Foundation of China under Grant 11801026, and Fundamental Research Funds for the Central Universities (No. 202264006).
Conflict of interest
The authors declare there is no conflict of interest.









 DownLoad:
DownLoad: