A periodic homogenization problem with defects rare at infinity

1.   Introduction

1.1.   Motivation

The purpose of this paper is to address the homogenization problem for a second order elliptic equation in divergence form with a certain class of oscillating coefficients:

where $\Omega$ is a bounded domain of $\mathbb{R}^d$ ($d \geq 1$) sufficiently regular (the regularity will be made precise later on) and $f$ is a function in $L^2(\Omega)$. The class of (matrix-valued) coefficients $a$ considered is that of the form

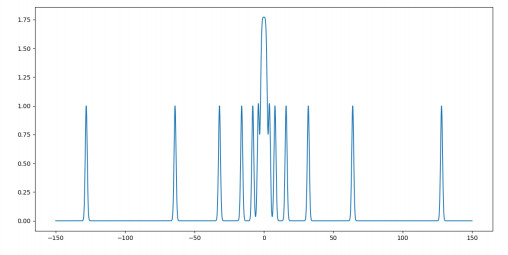
which describes a periodic geometry encoded in the coefficient $a_{per}$ and perturbed by a coefficient ${\tilde a}$ that represents a non-local perturbation (a "defect") that, although it does not vanish at infinity, becomes rare at infinity. More specifically, we consider coefficients ${\tilde a}$ that locally behave like $L^2(\mathbb{R}^d)$ functions in the neighborhood of a set of points localized at an exponentially increasing distance from the origin. Formally, the coefficient ${\tilde a}$ is an infinite sum of localized perturbations, increasingly distant from one another. A prototypical one-dimensional example of such a defect reads as $\sum\limits_{k \in \mathbb{Z}} \phi(x - \operatorname{sign}(k)2^{|k|})$ for some fixed $\phi \in \mathcal{D}(\mathbb{R})$, where $|k|$ denotes the absolute value of $k$ and $\operatorname{sign}(k)$ denotes its sign. It is depicted in Figure 1.

Homogenization theory for the unperturbed periodic problem (1)-(2) when ${\tilde a} = 0$ is well-known (see for instance [5,19]). The solution $u^{\varepsilon}$ converges strongly in $L^2(\Omega)$ and weakly in $H^1(\Omega)$ to $u^*$, solution to the homogenized problem:

where $a^*$ is a constant matrix. The convergence in the $H^1(\Omega)$ norm is obtained upon introducing a corrector $w_{per,p}$ defined for all $p$ in $\mathbb{R}^d$ as the periodic solution (unique up to the addition of a constant) to:

This corrector allows to both make explicit the homogenized coefficient

(where $Q$ denotes the d-dimensional unit cube, $(e_i)$ the canonical basis of $\mathbb{R}^d$) and define the approximation

such that $u^{\varepsilon,1} - u^{\varepsilon}$ strongly converges to $0$ in $H^1(\Omega)$ (see [1] for more details). In addition, convergence rates can be made precise, with in particular:

for some constants independent of $f$.

Our purpose here is to extend the above results to the setting of the perturbed problem (1)-(2). The main difficulty is that the corrector equation

(formally obtained by a two-scale expansion (see again [1] for the details) and analogous to (4) in the periodic case) is defined on the whole space $\mathbb{R}^d$ and cannot be reduced to an equation posed on a bounded domain, as is the case in periodic context in particular. This prevents us from using classical techniques. The present work follows up on some previous works [6,8,9,10] where the authors have developed an homogenization theory in the case where ${\tilde a} \in L^p(\mathbb{R}^d)$ for $p \in ]1, \infty[$. The existence and uniqueness (again up to an additive constant) of a corrector, the gradient of which shares the same structure "periodic + $L^p$" as the coefficient $a$, is established. Convergence rates are also made precise. Similarly to [6,8,9,10], we aim to show here, in a context of a perturbation rare at infinity, there also exists a corrector (unique up to the addition of a constant), and such that its gradient has the structure (2) of the diffusion coefficient: it can be decomposed as a sum of the gradient of a periodic corrector and a gradient that becomes rare at infinity (in a sense similar to that for ${\tilde a}$, and made precise below).

1.2.   Functional setting

We introduce here a suitable functional setting to describe the class of defects we consider.

In order to formalize our mathematical setting, we first define a generic infinite discrete set of points denoted by $\mathcal{G} = \left\{x_p \right\}_{p \in \mathbb{Z}^d}$. In the sequel, each point $x_p$ actually models the presence of a defect in the periodic background modeled by $a_{per}$ and our aim is to ensure these defects are sufficiently rare at infinity.

We next introduce the Voronoi diagram associated with our set of points. For $x_p \in \mathcal{G}$, we denote by $V_{x_p}$ the Voronoi cell containing the point $x_p$ and defined by

We now consider three geometric assumptions that ensure an appropriate distribution of the points in the space. The set $\mathcal{G}$ is required to satisfy the following three conditions :

where $|A|$ denotes the volume of a subset $A \subset \mathbb{R}^d$, $Diam(A)$ the diameter of $A$ and $D(.,.)$ the euclidean distance.

Assumption (H2) is the most significant assumption in our case since it implies that the points are increasingly distant from one another far from the origin. It in particular implies

More precisely, it ensures the distance between a point $x_p$ and the others has the same growth as the norm $|x_p|$ and, therefore, requires the Voronoi cell $V_{x_p}$ (which contains a ball of radius $\frac{D\left(x_p, \mathcal{G}\setminus{\{x_p\}}\right)}{2}$ as a consequence of its definition) to be sufficiently large. This assumption actually ensures that the defects modeled by the points $x_p$ are sufficiently rare at infinity. In particular, we show in Section 2 that Assumption (H2) implies that the number of points $x_p$ contained in a ball $B_R$ of radius $R>0$ is bounded by the logarithm of $R$. This property is an essential element for the methods used in the proof of this article.

In contrast to (H2), Assumptions (H1) and (H3) are only technical and not very restrictive. They limit the size of the Voronoi cells. In the case where these assumptions are not satisfied, our main results of Theorems 1.1 and 1.2 stated below still hold. Their proofs have to be adapted, upon splitting the Voronoi cells in several subsets such that each subset satisfies geometric constraints similar to (H1), (H2) and (H3). To some extent, our assumptions (H1) and (H3) ensure we consider the worst case scenario, where the set $\mathcal{G}$ contains as many points as possible while satisfying (H2).

In addition, although we establish in Section 2 all the geometric properties satisfied by the Voronoi cells $V_{x_p}$ which are required in our approach to study the homogenization problem (1) with the whole generality of Assumptions (H1), (H2) and (H3), we choose, for the sake of illustration and for pedagogic purposes, to work with a particular set of points (for which the coordinates are powers of 2) and to establish our main results of homogenization in this specific setting. There are, of course, many alternative sets that satisfy (H1), (H2) and (H3) but our specific choice is convenient. To define our specific set of points, we first introduce a constant $C_0>1$ and a set of indices $\mathcal{P}_{C_0}$ defined by:

Our specific set of points (see Figure 2) is then defined by:

We use here the convention $\operatorname{sign}(0) = 0$. The set of indices (8) contains only the points with integer coordinates on the axes $\operatorname{Span}\left(e_i\right)$ and the points close to each diagonal of the form $\operatorname{Span}\left( e_{i_1} + ... + e_{i_k} \right)$ for $k \in \left\{2,..,d \right\}$ and $\left(i_1,...,i_k \right) \in \left\{1,...,d\right\}^k$. In this way, the points of $\mathcal{G}_{C_0}$ are exponentially distant from each other with respect to the norm of $p$. In Section 2, we show that the set $\mathcal{G}_{C_0}$ defined by (9) indeed satisfies Assumptions (H1), (H2) and (H3).

In the sequel, we use the following notation:

$\bullet$ $B_R$: the ball of radius $R>0$ centered at the origin; $B_R(x)$: the ball of radius $R>0$ and center $x\in \mathbb{R}^d$; $A_{R,R'}$: the set $B_R \setminus{B_{R'}}$ for $R>R'>0$.

$\bullet$ $Q_R(x)$: the set $\left\{ y \in \mathbb{R}^d \ \middle| \ \max\limits_i |y_i - x_i| \leq R \right\}$ for $R>0$ and $x\in \mathbb{R}^d$; $Q_R$: the set $Q_R(0)$.

$\bullet$ $\sharp B$: the cardinality of a discrete set $B$.

$\bullet$ $2^p$: the point $x_p \in \mathcal{G}_{C_0}$ for $p\in \mathcal{P}_{C_0}$; $\tau_p$: the translation $\tau_{2^p}$ where we denote $\tau_x f = f(.+x)$ for $x\in \mathbb{R}^d$; $V_p$: the Voronoi cell $V_{2^p}$.

$\bullet$ $|p|$: the norm defined by $\max\limits_{i \in \{1,...,d\}} |p_i|$ for $p \in \mathcal{P}_{C_0}$.

In addition, for a normed vector space $(X,\|.\|_X)$ and a matrix-valued function $f \in X^n$, $n\in \mathbb{N}$, we use the notation $\|f\|_{X} \equiv \|f \|_{X^n}$ when the context is clear.

We associate to (8)-(9) the following functional space:

equipped with the norm

In (10), (11) we have denoted by:

and

Intuitively, a function in $\mathcal{B}^2(\mathbb{R}^d)$ behaves, locally at the "vicinity" of each point $x_p$, as a fixed $L^2$ function truncated over the domain $V_p$. We show several properties of the functional space $\mathcal{B}^2(\mathbb{R}^d)$ in Section 3.

As specified above, in the sequel we focus on homogenization problem (1) with non-local perturbations induced by the particular setting (8)-(9)-(10). We note, however, that the definition of $\mathcal{B}^2(\mathbb{R}^d)$ can be naturally adapted to the generality of Assumptions (H1)-(H2)-(H3) and the homogenization results established in the present study can of course be extended to this general setting. More precisely, most of our proofs only involve the general structure of the functional space $\mathcal{B}^2(\mathbb{R}^d)$ and several geometric properties related to the rarity of the points $x_p$ that are established under our general assumptions in Section 2. The specific geometric properties of the set (9) are only explicitly used to study the equation $-\operatorname{div} (a_{per} \nabla u) = \operatorname{div}(f)$ when $f \in \mathcal{B}^2(\mathbb{R}^d)$, particularly to establish the convergence of several sums involving the asymptotic behavior of the Green function of the Laplacian operator (see Lemmas 4.3, 4.4 and 5.2). However, these results are not specific to the set (9). We explain how to adapt their proofs under our general assumptions in Remarks 4 and 8.

1.3.   Main results

We henceforth assume that the ambient dimension $d$ is equal to or larger than 3. The one-dimensional and two-dimensional contexts are specific. Some results or proofs must be adapted in these particular cases but we will not proceed in that direction in all details. This is due to the asymptotic behavior of the Green function of the Laplacian operator in these two dimensions. In these two particular cases, we claim that it is still possible to show the existence of the corrector defined by Theorem 1.1 below. However, the method used in Lemmas 4.3 and 4.4, both useful for the proof of Theorem 1.1, need to be adapted. The one-dimensional context can be addressed easily because the solution to (14) is explicit. The two-dimensional case requires more work. We explain how to adapt our proof in Remark 5. In contrast, in dimensions $d = 1$ and $d = 2$, the convergence rates of Theorem 1.2 no longer hold. Indeed, the corrector $w_p$ is then not necessarily bounded (see Lemma 5.2 for details). We are only able to prove weaker results in these cases. Additional details about these cases may be found in Remarks 5, 7, and 9.

For $\alpha \in ]0,1[$, we denote by $\mathcal{C}^{0,\alpha}(\mathbb{R}^d)$ the space of uniformly Hölder continuous and bounded functions with exponent $\alpha$, that is:

where

We consider a matrix-valued coefficient of the form (2) with $a_{per} \in L^2_{per}(\mathbb{R}^d)^{d \times d}$ and ${\tilde a} \in \mathcal{B}^2(\mathbb{R}^d)^{d \times d}$. We denote by ${\tilde a}_{\infty}$ the matrix-valued limit $L^2$-function associated with ${\tilde a}$, where each coefficient $({\tilde a}_{\infty})_{i,j}$ is the limit $L^2$-function associated with $({\tilde a})_{i,j}\in \mathcal{B}^2(\mathbb{R}^d)$ and defined in (10). We assume that $a_{per}$, ${\tilde a}$ and ${\tilde a}_{\infty}$ satisfy:

and

The coercivity (12) and the $L^{\infty}$ bound on $a$ ensure that the sequence of solutions $\left(u^{\varepsilon}\right)_{\varepsilon>0}$ to (1) converges in $weak-H^1(\Omega)$ and $strong-L^2(\Omega)$ up to an extraction when $\varepsilon \rightarrow 0$. Classical results of homogenization show the limit $u^*$ is a solution to a diffusion equation of the form (3) for some matrix-valued coefficient $a^*$ to be determined. The questions that we examine in this paper are: What is the diffusion coefficient $a^*$ of the homogenized equation? Is it possible to define an approximate sequence of solutions $u^{\varepsilon,1}$ as in (6)? For which topologies does this approximation correctly describe the behavior of $u^{\varepsilon}$? What is the convergence rate?

In answer to our first question, we prove in Proposition 13 that the homogenized coefficient $a^*$ is constant and is the same as in the periodic case. This result is a direct consequence of Proposition 10 which ensures that the perturbations of $\mathcal{B}^2(\mathbb{R}^d)$ have a zero average in a strong sense. Consequently, our perturbations are "small" at the macroscopic scale and do not affect the homogenization that occurs in the periodic case associated with the periodic coefficient $a_{per}$. In reply to the other questions, our main results are contained in the following two theorems:

Theorem 1.1. For every $p \in \mathbb{R}^d$, there exists a unique (up to an additive constant)function $w_p \in H^1_{loc}(\mathbb{R}^d)$ such that $\nabla w_p \in \left(L^2_{per}(\mathbb{R}^d) + \mathcal{B}^2(\mathbb{R}^d)\right)^d\cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)^d$, solution to:

Theorem 1.2. Assume $\Omega$ is a ${C}^{2,1}$-bounded domain. Let $\Omega_1 \subset \subset \Omega$. We define $u^{\varepsilon,1} = u^* + \varepsilon \sum\limits_{i = 1}^{d}\partial_i u^{*} w_{e_i}(./\varepsilon)$ where $w_{e_i}$ is defined by Theorem 1.1 for $p = e_i$ and $u^*$ is the solution to (3). Then $R^{\varepsilon} = u^{\varepsilon} - u^{\varepsilon,1}$ satisfies the following estimates:

where $C_1$ and $C_2$ are two positive constants independent of $f$ and $\varepsilon$.

Our article is organized as follows. In Section 2 we prove some geometric properties satisfied by our set of points $\mathcal{G}_{C_0}$, in particular we show that it satisfies Assumptions (H1), (H2) and (H3). In section 3 we study the properties of $\mathcal{B}^2(\mathbb{R}^d)$ and its elements. In Section 4 we prove Theorem 1.1. Finally, in Section 5 we obtain the expected homogenization convergences stated in Theorem 1.2. We conclude this introduction section with some comments.

1.4.   Extensions and perspectives

A first possible extension of the above results, which is studied in [16,Appendix A], consists in considering the functional spaces $\mathcal{B}^r$ for $r\neq 2$, $1<r<\infty$, defined similarly to $\mathcal{B}^2$, but using the $L^r$ topology. In this case the convergence rates of Theorem 1.2 are modified and depend upon the value of $r$ and the ambient dimension $d$. Indeed, some results related to the strict sub-linearity of the corrector allow to show that the convergence rate of $R^{\varepsilon}$ is $\varepsilon^{\frac{d}{r}}|\log(\varepsilon)|^{\frac{1}{r}}$ if $r> d$ and $\varepsilon$ else.

In addition, although we have not pursued in these directions, we believe it is possible to extend the above results in several other manners.

$1)$ First, under additional assumptions satisfied by the function $f$, we expect the estimates of Theorem 1.2 to hold, with possibly different rates, in other norms than $L^2$ such as $L^q$, for $1<q< \infty$ or $\mathcal{C}^{0,\alpha}$, for $\alpha \in ]0,1[$. It seems that such questions could be addressed by adapting the proofs of Section 5 and consider the methods employed in [6] using the behavior of the Green function associated with problem (1).

$2)$ We also believe that it is possible to show results analogous to that of Theorems 1.1 and 1.2 in the case of equations not in divergence form, instead of (1),

where $a$ is a periodic coefficients perturbed by a defect in $\mathcal{B}^2(\mathbb{R}^d)$ of the form (2). One way to address this question could be to adapt the methods of [8,Section 3] in the case of local perturbations, that is, to show the existence of an invariant measure $m = m_{per} + \tilde{m}$ in $L ^2_{per}+ \mathcal{B} ^2(\mathbb{R}^d)$ solution to:

such that $\inf m >0$. Indeed, using the method presented in [3], this study could be then reduced to a problem of divergence form operator as soon as such a measure $m$ exists and the results established in this article could allow to conclude.

$3)$ In the same way, another possible generalization concerns advection-diffusion equation in the form:

where $a$ and $b$ are two periodic coefficients perturbed by a defect in $\mathcal{B}^2(\mathbb{R}^d)$. The method [7] is likely to be adapted to this case, showing the existence of an invariant measure $m$ in $L ^2_{per}+ \mathcal{B} ^2(\mathbb{R}^d)$ solution to

2.   Geometric properties of the Voronoi cells

We start by studying the geometric properties of the Voronoi cells associated to every sets of points $\mathcal{G}$ satisfying the general Assumptions (H1), (H2) and (H3). In particular, we show these assumptions ensure the rarity of the points $x_{p}$ in the space proving, in Proposition 3 and Corollary 1, that the number of points of $\mathcal{G}$ contained in a ball of radius $R>0$ is bounded by the logarithm of $R$. In Propositions 2 and 4, we also show two technical properties regarding the size and the structure of the cells. All these properties are actually fundamental for the rest of our work since they allow us to prove several results regarding the existence and uniqueness of solutions to the class (31) of diffusion equations $-\operatorname{div}(a\nabla u) = \operatorname{div}(f)$ studied in Section 4. In particular, as we shall see in the proof of Lemma 4.3, we use these geometric properties to bound several integrals in order to define a solution to equation (35), that is (31) with $a = a_{per}$, using the associated Green function. To conclude this section, we also show that our specific set of points $\mathcal{G}_{C_0}$, defined by (9), satisfies (H1), (H2) and (H3).

2.1.   General properties

In this subsection only, we proceed with the whole generality of Assumptions (H1), (H2) and (H3) and we introduce several useful geometric properties satisfied by every sets of points $\mathcal{G}$ satisfying these assumptions. These properties relate to the size of the Voronoi cells, their volume and their distribution in the space $\mathbb{R}^d$.

To start with, we show two properties regarding the volume of the Voronoi cells.

Proposition 1. There exist $C_1>0$ and $C_2>0$ such that for every $x \in \mathcal{G}$, we have the following bounds:

Proof. For every $x\in \mathcal{G}$, using the definition of the Voronoï diagram, we have the following inclusion:

Therefore, there exists a constant $C(d)>0$ such that:

We conclude using (H2) and (H3).

Proposition 2. There exists a sequence $(x_{n})_{n\in \mathbb{N}} \in \mathcal{G}^{\mathbb{N}}$ such that $\left(V_{x_n} - x_n\right)$ is an increasing sequence of sets and:

Proof. We consider a sequence $(x_{n})_{n\in \mathbb{N}} \in \mathcal{G}^{\mathbb{N}}$ such that the sequence $|x_n|$ is increasing and $\lim\limits_{n \rightarrow \infty}|x_n| = \infty$ (such a choice is always possible according to Assumptions (H1) and (H2)). Since we have assumed that $\mathcal{G}$ satisfies (H2), there exists $C>0$ such that for all $n \in \mathbb{N}$:

Therefore, as a consequence of the definition of the Voronoi cells, the ball $B_{C|x_n|/2} (x_n)$ is included in $V_{x_n}$ and, by translation, the ball $B_{C|x_n|/2}$ is included in $V_{x_n}-x_n$. Since $(x_n)_{n\in \mathbb{N}}$ is an increasing sequence such that $\lim\limits_{n \rightarrow \infty}|x_n| = \infty$, we use (H1) and we obtain, up to an extraction, that $V_{x_n}$ is included in $B_{C|x_{n+1}|/2}(x_{n})$. Thus

The sequence $(V_{x_n} - x_n)$ is therefore an increasing sequence of sets and, in addition,

We directly deduce that $\mathbb{R}^d = \bigcup\limits_{n \in \mathbb{N}} \left(V_{x_{n}} - x_n \right)$.

The next results ensure a certain distribution of the Voronoi cells in the space. In particular, we prove that the number of cells contained in a ball of radius $R>0$ increases at most as the logarithm of this radius. This property reflects the rarity of our points far from the origin and is essential in our approach.

Proposition 3. There exists a constant $C(d)>0$ that depends only of the ambient dimension $d$ such that:

Proof. Let $x\in \mathcal{G}$ such that $x \in A_{2^n,2^{n+1}}$. The definition of the Voronoi cells ensures that the distance $D(x,\partial V_x)$ is equal to $\frac{D\left(x, \mathcal{G} \setminus \left\{x \right\}\right)}{2}$. Property (H2) gives the existence of a constant $C_1>0$ independent of $x$ such that:

Then, the ball $B_{C_12^{n-1}}(x)$ is contained in $V_x$, that is $x$ is the only element of $\mathcal{G}$ in this ball. In addition, since $|x| \leq 2^{n+1}$, we obtain the following inclusion using a triangle inequality :

Since this inclusion is valid for every $x \in \mathcal{G}\cap A_{2^n,2^{n+1}}$ we obtain:

Therefore, there exists $C_2(d)>0$ such that:

Next, we know that the Voronoi cells are disjoint and, therefore, the collection of balls $\left(B_{C_1 2^{n-1}}(x)\right)_{x \in \mathcal{G}\cap A_{2^n,2^{n+1}}}$ is also disjoint. Thus, there exists $C_3(d)>0$ such that:

With (17) and (18), we conclude that:

Corollary 1. There exists $C>0$ such that for every $R>0$ and $x_0 \in \mathbb{R}^d$:

Proof. We start by proving the result if $R = 2^n$ for $n \in \mathbb{N}^*$. Without loss of generality, we can assume that $n$ is sufficiently large to ensure there exists $x$ in $\mathcal{G}\cap B_{2^n}(x_0)$. Using a triangle inequality, we remark that if $y\in B_{2^n}(x_0)$ we have:

That is, if $\tilde{x} \in \mathcal{G}$ is such that $\tilde{x} \notin B_{2^{n+3}}(x_0)$, every point $y \in B_{2^n}(x_0)$ is closer to $x$ than to $\tilde{x}$, that is $V_{\tilde{x}} \cap B_{2^n}(x_0) = \emptyset$. Therefore, we have

Next, if $|x_0|\leq 2^{n+4}$, we have $B_{2^{n+3}(x_0)} \subset B_{2^{2n+7}}$ and we use Proposition 3 to obtain the existence of a constant $C>0$ independent of $n$ such that:

If $|x_0|>2^{n+4}$, we denote by $m\geq n+4$ the unique integer such that $2^m\; <\; |x_0|$ and $|x_0|\leq\; 2^{m+1}$. In this case, we use a triangle inequality and we have

Proposition 3 gives the existence of $C>0$ independent of $x_0$ and $n$ such that:

Finally, we have estimate (19) in the particular case $R = 2^n$.

Next, for any $R>0$, we have:

where $[.]$ denotes the integer part. Thus, we obtain the following upper bound:

and we can conclude.

To conclude this section, we now introduce a particular set (denoted by $W_x$ in the proposition below) containing a point $x\in \mathcal{G}$ which is both bigger than the cell $V_x$ and far from all the others points of $\mathcal{G}$. As we shall see in Lemmas 4.3 and 4.4, this set is actually a technical tool that allows us to show the existence of the corrector stated in Theorem 1.1.

Proposition 4. For every $x \in \mathcal{G}$, there exists a convex open set $W_x$ of $\mathbb{R}^d$ and $C_1$, $C_2$, $C_3$, $C_4$ and $C_5$ five positive constants independent of $x$ such that:

Proof. Let $x$ be in $\mathcal{G}$. In the sequel, we denote $I_{x,y} = \left\{z \in \mathbb{R}^d \ \middle| \ |z-x| \leq |z - y| \right\}$ and $\varphi_x$ the homothety of center $x$ and ratio $\frac{3}{2}$. For $y \in \mathcal{G}\setminus{\{x\}}$, we denote by $H_{x,y}$ the set defined by:

The set $H_{x,y}$ can be easily determined, it is the half-space defined by:

We finally consider:

which is actually the image of the cell $V_x$ by the homothety $\varphi_x$ (see figure 3).

We next prove that $W_x$ satisfies (ⅰ), (ⅱ), (ⅲ), (ⅳ) and (ⅴ).

$\rm (i):$ For every $y \in \mathcal{G}\setminus{\{x\}}$ we have $I_{x,y} \subset H_{x,y}$ and therefore, we obtain using definition (7) of $V_x$:

and we have the first inclusion.

$\rm(ii):$ $W_x$ is a $\frac{3}{2}$-dilation of $V_x$, thus we have $Diam(W_x) = \frac{3}{2}Diam(V_x)$. We use (H2) and (H3) to obtain the first estimate. Next, the definitions of the sets $H_{x,y}$ and $W_x$ give:

We conclude using (H2).

$\rm(iii):$ Let $y$ be in $\mathcal{G}\setminus{\{x\}}$. By definition, for every $v\in W_x$, there exists $u \in I_{x,y}$ such that $v = u + \frac{1}{4}\overrightarrow{xy}$. Therefore, we use the triangle inequality and we have:

Taking the infimum over all $v \in W_x$ in the above inequality and using (H2), we finally obtain:

where $C>0$ is independent of $x$ and $y$.

$\rm (iv):$ First, we have proved there exists a constant $C_1\geq1$ independent of $x$ such that $Diam\left(W_x\right)\leq C_1 |x|$. Second, using Assumption (H2), we know there exists a constant $C_2>0$ such that for every $y \in \mathcal{G}$ we have $D\left(y, \mathcal{G}\setminus{\{y\}}\right)\geq C_2 |y|$. Let $k>2$ be an integer such that:

We denote $n \in \mathbb{N}$, the unique integer such that $x \in A_{2^n,2^{n+1}}$. Here, it is sufficient to establish a bound for $x$ sufficiently large, thus without loss of generality, we can assume that $n>k$. We next show that if $y \in \mathcal{G}$ satisfies $|y|\leq 2^{-k-1}|x| \leq 2^{n-k}$ or $|y|\geq 2^k |x| \geq 2^{n+k}$, then $W_x \cap V_y = \emptyset$.

We start by assuming that $y \in \mathcal{G}\cap \left(\mathbb{R}^d \setminus{B_{2^{n+k}}}\right)$. Since

we have $W_x \subset B_{C_12^{n+1}}(x)$. Therefore, using a triangle inequality we obtain $W_x \subset B_{C_12^{n+2}}$. Our aim here is to prove that $I_{y,x} \cap B_{C_12^{n+2}} = \emptyset$ in order to deduce $I_{y,x} \cap W_x = \emptyset$. For every $z \in I_{y,x}$:

In addition, for every $y \in \mathcal{G} \setminus{\{y\}}$, we have $D \left(x,I_{x,y} \right) = \frac{1}{2}|x-y|\geq \frac{1}{2} D\left(y,\mathcal{G}\setminus{\{y\}}\right)$ and we deduce that:

Therefore, $I_{x,y} \subset \left(\mathbb{R}^d \setminus{ B_{C_1 2^{n+3}}}\right)$ and we obtain $W_x \cap I_{x,y} = \emptyset$. Since, $V_y = \bigcap\limits_{z \in \mathcal{G}\setminus{\{y\}}} I_{z,y}$, we deduce that $V_y \cap W_x = \emptyset$.

Next we assume that $y \in B_{2^{n-k}}$ and we want to prove that $V_y \cap H_{x,y} = \emptyset$. As above, we can show that $V_y \subset B_{C_1 2^{n-k+1}}$ and for every $z \in H_{x,y}$:

Therefore $H_{x,y} \subset{B_{C_1 2^{n-k+2}}}$ and we have $V_y \cap H_{x,y} = \emptyset$. We deduce that $V_y \cap W_{x} = \emptyset$.

To conclude, we use Proposition 3 and we obtain the existence of a constant $C_3>0$ independent of $n$ such that:

and therefore:

We have finally proved (ⅳ).

$\rm(v):$ Let $y$ be in $\mathcal{G}\setminus{\{x\}}$. We first assume that $2^{-k-1}|y|> |x|$, where $k$ is defined as in (20) and is independent of $x$. In the proof of (ⅳ) above, we have shown that $W_y\cap V_x = \emptyset$. Therefore, using Properties (ⅰ) and (ⅱ) of $W_y$ we easily obtain that there exists a constant $M_1>0$ independent of $x$ and $y$ such that $D(V_x,V_y)>M_1|y|$ and we can conclude. Next, we assume that $2^{-k-1}|y| \leq |x|$. Using again Properties (ⅰ) and (ⅱ) of $W_x$, we obtain the existence of $M_2>0$ independent of $x$ and $y$ such that $D\left(V_x, V_y\setminus{W_x}\right)\geq M_2 |x| \geq M_2 2 ^{-k-1} |y|$. Finally, we have proved (ⅴ) with $C_5 = \min(M_1, M_22 ^{-k-1})$.

2.2.   The particular case of the "$2^p$"

We next prove that the set $\mathcal{G}_{C_0}$ defined by (9) satisfies Assumptions (H1), (H2) and (H3). In order to avoid many unnecessary technical details, we study here the Voronoi diagram only for $d = 3$ and, in the sequel, we admit that these properties still hold in higher dimension. We also consider the cell $V_p$ only for $p = (p_1,p_2,p_3) \in \left(\mathbb{R}^{+*}\right)^3$. Since the distribution of the points $2^p$ is symmetric with respect to the origin, the other cases are similar and we omit them.

Proof of (H1). Let $p = (p_1,p_2,p_3)$ be in $\mathcal{P}_{C_0}\cap \left(\mathbb{R}^{+*}\right)^3$. We first prove the following inclusion:

To this aim, we want to show that if $(x,y,z)\notin \prod\limits_{i = 1}^3 \left[2^{p_i-1},2^{|p|+3}\right]$, then there exists $x_q\in \mathcal{P}_{C_0}\setminus{\{x_p\}}$ such that the point $(x,y,z)$ is closer to $x_q$ than to $x_p$ and therefore $(x,y,z) \notin V_p$. We consider $(x,y,z)\in \left(\mathbb{R}^+\right)^3$ and we start by assuming that $x<\; 2^{p_1-1}$. We have

and

Since $x<2^{p_1-1}$, we use a triangle inequality and

We obtain that $D((x,y,z) , x_p)^2 > D((x,y,z), (0,2^{p_2},2^{p_3}))^2$. That is, $(x,y,z)$ is closer to $(0,2^{p_2},2^{p_3})\in \mathcal{G}_{C_0}$ than to $x_p$ and we deduce that $(x,y,z) \notin V_{p}$. We can therefore conclude that $V_p$ is included in $\left\{ (x,y,z) \in \mathbb{R}^3 \ \middle| \ 2^{p_1 - 1} \leq x \right\}$.

We next assume that $x>2^{|p|+3}$. Since $|p|\geq p_1$, we have:

Using $x>2^{|p|+3}$, it follows:

On the other hand, we have

We obtain

Similarly, we can show that $|z-2^{p_3}|^2\geq |z-2^{p_3+1}|^2 - 3 \times 2^{|p|}$ and, using (22), we have

Now we claim that $(2^{|p|+1}, 2^{p_2+1},2^{p_3 +1}) \in \mathcal{G}_{C_0}$. Indeed, since $\left(p_1, p_2 ,p_3 \right)\in \mathcal{P}_{C_0}$, we have using (8) :

Since $D((x,y,z) , x_p)^2 > D((x,y,z) , (2^{|p|+1}, 2^{p_2+1},2^{p_3 +1}))^2$, we therefore conclude that $(x,y,z)$ is closer to $(2^{|p|+1}, 2^{p_2+1},2^{p_3 +1})$ than to $x_p$ and that $(x,y,z) \notin V_{p}$.

Using the symmetry of the distribution, we can use exactly the same argumentation to treat the cases $y<2^{p_2-1}$, $y>2^{|p|+3}$, $z<2^{p_3-1}$ and $z>2^{|p|+3}$. We have finally established inclusion (21). Since the volume of the cube $\prod\limits_{i = 1}^3 \left[2^{p_i-1},2^{|p|+3}\right]$ is bounded by $8^3.2^{3|p|}$, we can deduce that:

(H1) is proved.

Proof of (H2). Let $p$ be in $\mathcal{P}_{C_0}\cap \left(\mathbb{R}^{+*}\right)^3$. We have:

and therefore:

To show the upper bound, we consider $x_q \in \mathcal{G}_{C_0}\setminus{\{x_p\}}$. Without loss of generality, we can assume $|p_1| = |p|$ and there are three cases:

● If $|q_1|\neq |p_1|$, then:

● If $p_1 = q_1$, since $p\in \mathcal{P}_{C_0}$, we have $\max(|p_2|,|p_3|)\geq |p| - C_0$. Since $x_q \neq x_p$, we obtain as above:

● If $p_1 = - q_1$, we have:

In the three cases we conclude there exists $C>0$ independent of $q$ such that $D(x_p,x_q) \geq C 2^{|p|}$. Finally, since $|x_p| = \left(2^{2p_1} + 2^{2p_2} + 2^{2p_3}\right)^{1/2}\leq \sqrt{3}.2^{|p|}$, we obtain the existence of a constant $C_1>0$ independent of $p$ such that:

Proof of (H3). Let $p = (p_1,p_2,p_3)$ be in $\mathcal{P}_{C_0}\cap \left(\mathbb{R}^{+*}\right)^3$. We use (21) to bound the diameter of $V_{p}$ by the diameter of the cube $[0, 2^{|p|+3}]^3$, that is:

In addition, (H2) shows the existence of $C>0$ such that for every $x_p \in \mathcal{G}$, we have :

and we obtain (H3).

We finally conclude this section establishing an estimate regarding the norm of each element $x_p$ of $\mathcal{G}_{C_0}$. Using Proposition 1, the next property shall be useful to estimate the volume of the Voronoi cells in our particular case.

Proposition 5. There exists $C_1>0$ and $C_2>0$ such that for every $p$ in $\mathcal{P}_{C_0}$, we have:

Proof. For $p \in \mathcal{P}_{C_0}$, we have:

We first use the inequality $|p_i|\leq |p|$ to obtain the upper bound. That is:

For the lower bound, we denote $j = \operatorname{argmax}_{i \in \{1,...,d\}} |p_i|$ and we have:

We have established the norm estimate (23).

In the sequel of this work, we only consider the specific set $\mathcal{G}_{C_0}$, defined by (9), for a fixed arbitrary constant $C_0>1$. Therefore, for the sake of clarity and without loss of generality, we will denote $\mathcal{G}$ and $\mathcal{P}$ instead of $\mathcal{G}_{C_0}$ and $\mathcal{P}_{C_0}$.

3.   Properties of the functional space $\mathcal{B}^2(\mathbb{R}^d)$

In this section we prove some properties satisfied by the functional space $\mathcal{B}^2(\mathbb{R}^d)$. The following results are heavily based upon the geometric distribution of the $x_p$. They are key for the understanding of the structure of $\mathcal{B}^2$ and to establish the homogenization of problem (1).

To start with, we show the uniqueness of a limit $L^2$-function $f_{\infty}$ in $L^2(\mathbb{R}^d)$ defined in (10) and characterizing each element of $\mathcal{B}^2(\mathbb{R}^d)$. This result ensures that the definition of the function space $\mathcal{B}^2(\mathbb{R}^d)$ is consistent.

Proposition 6. Let $f$ be a function of $\mathcal{B}^2(\mathbb{R}^d)$. Then, the limit function $f_{\infty}$ of $L^2(\mathbb{R}^d)$ defined in (10) is unique.

Proof. We assume there exist two functions $f_{\infty}$ and $g_{\infty}$ in $L^2(\mathbb{R}^d)$ such that

By a triangle inequality, we obtain for every $p \in \mathcal{P}$:

In addition, we have $\|\tau_{-p}f_{\infty} - \tau_{-p}g_{\infty}\|_{L^2(V_p)} = \|f_{\infty} - g_{\infty}\|_{L^2(V_p-2^p)}$. According to Proposition 2, we can find a sequence $(p_n)_{n \in \mathbb{N}} \in \mathcal{P}$ such that $\lim\limits_{n \rightarrow \infty}|p_n| = \infty$ and:

We can finally conclude that $\|f_{\infty} - g_{\infty}\|_{L^2(\mathbb{R}^d)} = 0$, that is $f_{\infty} = g_{\infty}$ in $L^2(\mathbb{R}^d)$.

We next study the structure of the space $\mathcal{B}^2(\mathbb{R}^d)$ showing two essential properties that shall allow us to establish the existence of the corrector in Section 4. In particular, we prove in Proposition 7 that $\mathcal{B}^2(\mathbb{R}^d)$ is a Banach space.

Proposition 7. The space $\mathcal{B}^2(\mathbb{R}^d)$ equipped with the norm defined by (11), is a Banach space.

Proof. Let $(f_n)_{n \in \mathbb{N}}$ be a Cauchy sequence in $\mathcal{B}^2(\mathbb{R}^d)$. Definitions (10) and (11) ensure the existence of a Cauchy sequence $f_{n, \infty}$ in $L^2(\mathbb{R}^d)$ such that for every $n \in \mathbb{N}$,

Then, for any $\varepsilon>0$, there exists $N\in \mathbb{N}$ such that for all $n>N$, $k>0$:

Since $L^2$ and $L^2_{unif}$ are Banach spaces, there exist $f \in L^2_{unif}(\mathbb{R}^d)$ and $f_{\infty} \in L^2(\mathbb{R}^d)$ such that $f_n \underset{n\to+\infty}{\longrightarrow} f$ in $L^2_{unif}(\mathbb{R}^d)$ and $f_{n, \infty} \underset{n\to+\infty}{\longrightarrow} f_{\infty}$ in $L^2(\mathbb{R}^d)$. We consider the limit in (24) when $k \rightarrow \infty$ and we obtain:

Since $\varepsilon$ can be chosen arbitrary small, we deduce:

The function $f$ is therefore the limit of $f_n$ for the norm (11). We just have to show that $f \in \mathcal{B}^2(\mathbb{R}^d)$ to conclude. Indeed, for a fixed $n>N$ and for $p$ sufficiently large, we have:

Using a triangle inequality, it follows:

Finally, we obtain $\lim\limits_{|p| \rightarrow \infty} \|f - \tau_{-p} f_{\infty} \|_{L^2(V_p)} = 0$.

Proposition 8. Let $\alpha \in ]0,1[$, then $\mathcal{C}^{0,\alpha}(\mathbb{R}^d)\cap \mathcal{B}^2(\mathbb{R}^d)$ is dense in $\left(\mathcal{B}^2(\mathbb{R}^d), \|.\|_{\mathcal{B}^2(\mathbb{R}^d)}\right).$

Proof. We consider $f \in \mathcal{B}^2(\mathbb{R}^d)$ and $f_{\infty} \in L^2(\mathbb{R}^d)$ the associated limit function defined by (10). First, for any $\varepsilon >0$, there exists $\phi \in \mathcal{D}(\mathbb{R}^d)$ such that $\| \phi - f_{\infty} \|_{L^2(\mathbb{R}^d)} < \dfrac{\varepsilon}{3}$, thus $\| \tau_{-p} \phi - \tau_{-p} f_{\infty}\|_{L^2(V_p)} \leq \dfrac{\varepsilon}{3}$ for all $p \in \mathcal{P}$. Second, since $f \in \mathcal{B}^2(\mathbb{R}^d)$:

Since $\phi$ is compactly supported there also exists $P$, which we can always assume larger than $P^*$, such that for every $|p|>P$ and for all $q\neq p$, we have $(\tau_{-q} \phi)_{|V_p} = 0$.

The finite sum $\sum\limits_{|q|\leq P} 1_{V_q} f$ (where $1_A$ denotes the indicator function of $A$) is compactly supported and then belongs to $L^2(\mathbb{R}^d)$. Again, we can find $\psi \in \mathcal{D}(\mathbb{R}^d)$ such that $\left\|\psi -\; \sum\limits_{|q|\leq P} 1_{V_q} f\right\|_{L^2(\mathbb{R}^d)}\leq \dfrac{\varepsilon}{3}$. We fix $g = \psi + \sum\limits_{|p|>P} \tau_{-p}\phi$ and we want to show that $g$ is a good approximation of $f$ in $\mathcal{B}^2(\mathbb{R}^d) \cap \mathcal{C}^{0, \alpha}(\mathbb{R}^d)$, that is $g$ is close to $f$ on each $V_p$, uniformly in $p$. First, we have:

Therefore $g$ is bounded and we can easily prove that $g \in \mathcal{B}^2(\mathbb{R}^d)\cap \mathcal{C}^{\infty}(\mathbb{R}^d)$ where the associated limit function in $L^2(\mathbb{R}^d)$ is given by $g_{\infty} = \phi$. Furthermore, $g$ is in $\mathcal{C}^{0,\alpha}(\mathbb{R}^d)$ since it is a $\mathcal{C}^{\infty}$ function and all of its derivatives are bounded. Indeed, for every $k$ in $\mathbb{N}^d$, we denote $\partial_k = \partial_{x_1}^{k_1}\partial_{x_2}^{k_2}...\partial_{x_d}^{k_d}$ and we have:

and $\partial_k g$ is clearly bounded.

Let $p \in \mathcal{P}$, we consider two cases. If $|p|\leq P$, then:

Else, if $|p|>P$, using that $\sum\limits_{|q|\leq P} 1_{V_q} f$ has support in $\bigcup\limits_{|q| \leq P} V_q$ we have:

We obtain:

and we can conclude.

We now establish a property regarding multiplication of elements of $\mathcal{B}^2(\mathbb{R}^d)$.

Proposition 9. Let $g$ and $h$ be in $\mathcal{B}^2(\mathbb{R}^d) \cap L^{\infty}(\mathbb{R}^d)$. We assume the associated $L^2$ function of $g$, denoted by $g_{\infty}$, is in $L^{\infty}(\mathbb{R}^d)$, then $hg \in \mathcal{B}^2(\mathbb{R}^d)$.

Proof. Since $g_{\infty} \in L^{\infty}(\mathbb{R}^d)$, we clearly have $g_{\infty} h_{\infty} \in L^2(\mathbb{R}^d)$. Using that for all $p \in \mathcal{P}$:

We have by the triangle inequality:

It follows, taking the limit for $|p| \rightarrow \infty$, that $gh \in \mathcal{B}^2(\mathbb{R}^d)$ and that $\left(gh\right)_{\infty} = g_{\infty}h_{\infty}$.

Our next result is one of the most important properties for the sequel. As we shall see in section 5, it first implies that the homogenized coefficient in our setting is the same as the homogenized coefficient in the periodic case, that is, without perturbation. In addition, it gives some information about the growth of the corrector defined in Theorem 1.1 (in particular, we give a proof in Proposition 11 of the strict sublinearity of the corrector). We will use all of these properties to prove the convergence stated in Theorem 1.2 in our case.

Proposition 10. Let $u \in \mathcal{B}^2(\mathbb{R}^d)$. Then, for every $x_0 \in \mathbb{R}^d$:

with the following convergence rate:

where $C>0$ is independent of $R$ and $x_0$.

Proof. We fix $R>0$. Using the Cauchy-Schwarz inequality, we have:

Since the number of $V_p$ such that $B_R(x_0)\cap V_p \neq \emptyset$ is bounded by $\log(R)$ according to Corollary 1, we obtain:

Here, $C(d)$ depends only on the ambient dimension $d$. The last inequality yields (26) and conclude the proof.

Corollary 2. Let $u \in \mathcal{B}^2(\mathbb{R}^d) \cap L^{\infty}(\mathbb{R}^d)$, then $|u(./\varepsilon)|$ is convergent to 0 in the weak*-$L^{\infty}$ topology when $\varepsilon \rightarrow 0$.

Proof. We fix $R>0$ and we first consider $\varphi = 1_{B_R}$. For any $\varepsilon>0$, we have:

We next use (26) in the right-hand term and we obtain the existence of $C>0$ independent of $\varepsilon$ and $\varphi$ such that:

We conclude using the density of simple functions in $L^1(\mathbb{R}^d)$.

We next introduce the notion of sub-linearity which is actually a fundamental property in homogenization. Indeed, in order to precise the convergence of the approximated sequence of solutions (6), we have to study the behavior of the sequences $\varepsilon w_{e_i}(./\varepsilon)$ when $\varepsilon \rightarrow 0$. The convergence to zero of these sequences and the understanding of the rate of convergence are key for establishing estimates (15) and (16) stated in Theorem 1.2. In the sequel, we therefore study this phenomenon for the functions with a gradient in $\mathcal{B} ^2(\mathbb{R}^d)$.

Definition 3.1. A function $u$ is strictly sub-linear at infinity if:

In the next proposition we prove the sub-linearity of all the functions $u$ such that $\nabla u \in \left(\mathcal{B}^2(\mathbb{R}^d) \cap L^{\infty}(\mathbb{R}^d)\right)^d$. We assume, for this general property only, that $d \geq 2$.

Proposition 11. Assume $d\geq 2$. Let $u \in H^1_{loc}(\mathbb{R}^d)$ with $\nabla u \in \left(\mathcal{B}^2(\mathbb{R}^d) \cap L^{\infty}(\mathbb{R}^d)\right)^d$. Then $u$ is strictly sub-linear at infinity and for all $s >d$, there exists $C>0$ such that for every $x,y \in \mathbb{R}^d$ with $x \neq y$:

Proof. Let $x,y \in \mathbb{R}^d$ with $x \neq y$ and fix $r = |x-y|$. Since $\nabla u \in L^{\infty}(\mathbb{R}^d)^d$, we have $\nabla u \in L^s_{loc}(\mathbb{R}^d)^d$ for every $s \geq 1$. We next fix $s>d$. We know there exists a constant $C>0$, depending only on $d$, such that:

This estimate is established for instance in [13,Remark p.268] as corollary of the Morrey's inequality ([13,Theorem 4 p.266]). Since $s>d\geq 2$, we use the boundedness of $\nabla u$ to obtain:

We next split the integral of (28) on each $V_p$ such that $V_p\cap B_r(x)\neq \emptyset$ and we have :

We finally use Corollary 1 and we obtain the existence of a constant $C_1 >0$ such that:

This inequality is true for all $s>d$, which allows us to conclude. In addition, the sub-linearity of $u$ is obtained fixing $y = 0$ and letting $|x|$ go to the infinity in estimate (27).

Remark 1. In the case $d = 1$, since $s\geq 2$, the above proof gives:

The last proposition of this section gives an uniform estimate of the integral remainders of the functions of $\mathcal{B}^2(\mathbb{R}^d)$. The idea here is that the functions of $\mathcal{B}^2(\mathbb{R}^d)$ behave like a fixed $L^2$-functions at the vicinity of the points of $\mathcal{G}$ and therefore, have to be small in a $L^2$ sense far from these points. This property will be used in the proof of Lemma 4.3 in next section to establish an estimate in $\mathcal{B}^2(\mathbb{R}^d)$ satisfied by the solutions to diffusion equation (35).

Proposition 12. Let $f$ be in $\mathcal{B}^2(\mathbb{R}^d)$ and $f_{\infty}$ the associated limit function in $L^2(\mathbb{R}^d)$. For any $\varepsilon >0$, there exists $R^*>0$ such that for every $R>R^*$ and every $p,q \in \mathcal{P}$:

where $B_R(2^q)^c$ denotes the set $\mathbb{R}^d \setminus{B_R(2^q)}$. Therefore, we have the following limit:

Proof. Let $\varepsilon>0$. First, for every $R>0$, $p, q \in \mathcal{P}$ we use a triangle inequality and we obtain the following upper bound:

We want to bound the three terms $I^{p,q}_{1}(R)$, $I^{p,q}_{2}(R)$ and $I^{p,q}_{3}(R)$ by $\varepsilon$ uniformly in $p,q$.

We start by considering $I^{p,q}_{1}$. We have assumed that $f \in \mathcal{B}^2(\mathbb{R}^d)$, then, by definition, there exists $P>0$ such that for every $q \in \mathcal{P}$ satisfying $|q|>P$, we have:

In addition, since the volume of each $V_q$ is finite according to assumption (H1), there exists $R_1>0$ such that for every $|q|\leq P$, $B_{R_1}(2^q)^c \cap V_q = \emptyset$. Therefore, as soon as $|q|\leq P$ and $R\geq R_1$, we have $I_{p,q}(R) = 0$. Finally, considering successively the case $|q|\leq P$ and the case $|q|>P$, we obtain for every $q,p\in \mathcal{P}$ and $R\geq R_1$:

We next study the second term $I^{p,q}_{2}$. Since $f_{\infty}$ is in $L^2(\mathbb{R}^d)$, there exists $R_2>0$, which we can always assume larger than $R_1$, such that for every $q \in \mathcal{P}$:

And we directly obtain, for every $R \geq R_2$:

Finally, in order to bound the last term, we know that $\lim\limits_{|l| \rightarrow \infty} D\left(2^l, \mathcal{G}\setminus{\{2^l\}}\right) = +\infty$ as a consequence of Assumption (H2). Therefore, there exists a finite number of indices $l$ such that:

Thus, we deduce the existence of a positive radius $R_3$ independent of $q,p$ such that for every $l$ satisfying (30) we have $B_{R_3}(2^l)^c \cap V_l = \emptyset$. Again we can always assume $R_3$ larger than $R_2$. There are two cases depending on the value of q:

$1)$ If $q$ satisfies (30), we have $B_{R_3}(2^q)^c \cap V_q = \emptyset$ and we obtain $I^{p,q}_{3}(R_3) = 0$

$2)$ Else, for every $y \in V_q$, we have $|y - 2^p| > R_2$. Therefore:

Using (29), we have for every $R \geq R_3$, $I^{p,q}_{3}(R) \leq \frac{\varepsilon}{3}$.

In the two cases, we obtain for $R\geq R_3$:

Since the values of $R_3$ is independent of $p$ and $q$ we can conclude the proof for $R^* = R_3$.

4.   Existence result for the corrector equation

This section is devoted to the proof of Theorem 1.1. Equation (14) being posed on the whole space $\mathbb{R}^d$, we need to use here the geometric distribution of the $2^p$ and introduce some constructive techniques involving the fundamental solution of the operator $-\operatorname{div}(a \nabla.)$ to solve it. To start with, we establish some general results on equations

for coercive coefficients $a$ of the form (2) and right hand side $f$ in $\mathcal{B}^2(\mathbb{R}^d)^d$ in order to deduce the existence of the corrector stated in Theorem 1.1. For this purpose, we consider the following strategy adapted from [8]: we first study diffusion problem (35) in the periodic context, that is, when the diffusion coefficient $a = a_{per}$ is periodic. Secondly we show in Lemma 4.5 the continuity of the associated reciprocal linear operator $\nabla\left(-\operatorname{div}a\nabla\right)^{-1}\operatorname{div}$ from $\mathcal{B}^2(\mathbb{R}^d)$ to $\mathcal{B}^2(\mathbb{R}^d)$. Finally, we use this continuity in order to generalize the existence results of the periodic context to the general context when $a$ is a perturbed coefficient of the form (2). To this end, we apply a method based on the connexity of the set $\mathcal{I} = [0,1]$ as we shall see in the proof of Lemma 4.6.

4.1.   Preliminary uniqueness results

We begin by establishing the uniqueness of a solution $u$ to (31) such that $\nabla u \in \mathcal{B}^2(\mathbb{R}^d)^d$. This result is actually essential in the proof of Theorem 1.1 since it both ensures the uniqueness of the corrector solution to (14) and also allows us to establish the continuity estimate of Lemma 4.5 which is key in our approach to show the existence of a solution to (31).

Lemma 4.1. Let $a$ be an elliptic and bounded coefficient, and $u \in H^1_{loc}(\mathbb{R}^d)$, such that $\sup\limits_{p \in \mathcal{P}} \int_{V_p}|\nabla u|^2 < \infty$, be a solution to:

in the sense of distribution. Then $\nabla u$ = 0.

Proof. we consider $u\in H^1_{loc}(\mathbb{R}^d)$ solution to (32). Since $u$ is a solution to (32), there exists $C>0$ such that for every $R >0$, we have the following estimate (for details see for instance [14,Proposition 2.1 p.76] and [14,Remark 2.1 p.77]):

where:

We use the Poincaré-Wirtinger inequality on the right-hand side and we obtain:

Furthermore, we can write this inequality in the following form:

In addition, using Corollary (1), we know there exists a constant $C_1>0$ independent of $R$ such that:

Next, we define $F(R) = \int_{ B_{R}} |\nabla u|^2$. The inequalities (33) and (34) yield for all $R > 0$ and for every $n \in \mathbb{N}^{*}$, we obtain

Since $\dfrac{C}{1+C}<1$, we have:

and it therefore follows, letting $n$ go to infinity, that $F(R) = 0$ for all $R>0$, thus $\nabla u = 0$.

Corollary 3. Let $f \in \mathcal{B}^2(\mathbb{R}^d)^d$, then a solution $u$ to (31) with $\nabla u \in \mathcal{B}^2(\mathbb{R}^d)^d$ is unique up to an additive constant.

Remark 2. Here the restriction made on the dimension is actually not necessary. The result and the proof of Lemma 4.1 of uniqueness still hold if we assume $d = 1$ or $d = 2$.

Remark 3. We remark that Assumptions (2) and (13) regarding the structure and the regularity of the coefficient $a$ are not required to establish the uniqueness result of Lemma 4.1. In the proof, we only use the "Hilbert" structure of $L^2$, induced by the assumptions satisfied by $u$, and the fact that $a$ is elliptic and bounded.

4.2.   Existence results in the periodic problem

Now that uniqueness has been dealt with, we turn to the existence of the solution to (31). We need to first establish it for a periodic coefficient considering the equation:

We start by introducing the Green function $G_{per}: \mathbb{R}^d \times \mathbb{R}^d \rightarrow \mathbb{R}$ associated with the operator $-\operatorname{div}\left(a_{per}\nabla. \right)$ on $\mathbb{R}^d$. That is, the unique solution to

According to the results established in [4,Section 2] about the asymptotic growth of the Green function (see also [2,Theorem 13,proof of Lemma 17] and [18] for bounded domain or [11, Proposition 8] for additional details), there exists $C_1>0$, $C_2>0$ and $C_3>0$ such that for every $x,y \in \mathbb{R}^d$ with $x\neq y$:

We first introduce a result of existence in the $L^2(\mathbb{R}^d)$ case. The following lemma allows us to define a solution to (35) using the Green function when $f$ belongs to $L^2(\mathbb{R}^d)^d$. The proof of this result is established in [4].

Lemma 4.2. Let $f$ be in $L^2(\mathbb{R}^d)^d$, then the function:

is a solution in $H^1_{loc}(\mathbb{R}^d)$ to (35) such that $\nabla u \in L^2(\mathbb{R}^d)^d$.

Our aim is now to generalize the above result to our case and, in particular, to give a sense to the function $u$ define by (39) when $f\in \mathcal{B}^2(\mathbb{R}^d)^d$. The idea here is to split the function $f$ into a sum of $L^2$-functions $f_p$ compactly supported in each $V_p$ for $p\in \mathcal{P}$. Using Lemma 4.2, we shall obtain the existence of a collection $u_p$ of solution to (35) when $f = f_p$. The main difficulty here is to show that the function $u$ defined as the sum of the $u_p$ is bounded.

Lemma 4.3. Let $f\in L^2_{loc}(\mathbb{R}^d)^d$ such that $\sup\limits_{p \in \mathcal{P}} \|f\|_{L^2(V_p)} < \infty$, then the function $u$ defined by

is a solution in $H^1_{loc}(\mathbb{R}^d)$ to (35).In addition, $u$ is the unique solution to (35) which satisfies $\sup\limits_{p \in \mathcal{P}} \|\nabla u \|_{L^2(V_p)} < \infty$ and there exists $C>0$ independent of $f$ and $u$ such that we have the following estimate:

Proof. Step 1: $u$ is well defined

We start by proving that definition (40) makes sense and, in particular, that the above integral defines a function $u$ solution to (35) in $H^1_{loc}(\mathbb{R}^d)$. In the sequel the letter $C$ denotes a generic constant that may change for one line to another. For every $q \in \mathcal{P}$, we first introduce a set $W_q$ and five constants $C_1$, $C_2$, $C_3$, $C_4$ and $C_5$ independent of $q$ and defined by Proposition 4 such that:

To start with, we define for each $q \in \mathcal{P}$ the function:

Lemma 4.2 ensures this function is a solution in $H^1_{loc}(\mathbb{R}^d)$ to:

Considering the gradient of (42), we have for every $x \in \mathbb{R}^d\setminus{V_q}$:

Next, for every $N \in \mathbb{N^*}$, we define:

and

We next show that the two series $U_N$ ans $S_N$ are convergent in $L^2_{loc}(\mathbb{R}^d)$. To this aim, since the collection $\left(V_p\right)_{p\in \mathcal{P}}$ is a partition of $\mathbb{R}^d$, it is sufficient to prove that they normally converge in $L^2(V_p)$ for every $p \in \mathcal{P}$. We fix $p \in \mathcal{P}$ and for every $q \in \mathcal{P}$ such that $V_q \cap W_p = \emptyset$, we use the Cauchy-Schwarz inequality to obtain:

Next, estimate (36) gives:

Since $V_q \cap W_p = \emptyset$, Property (ⅴ) gives the existence of $C>0$ such that for every $x\in V_p$ and $y\in V_q$, we have $|x-y|\geq C 2^{|q|}$. We next use Propositions 1 and 5 to obtain the existence of a constant $C>0$ independent of $p$ and $q$ such that $\left|V_q\right| \leq C 2^{d|q|}$. Finally:

We thus obtain the following upper bound:

The first sum is finite according to Property (ⅳ) and we only have to prove the convergence of the second one. We have assumed $d>2$ and consequently $d/2-1 >0$. In addition, since the number of $q\in \mathcal{P}$ such that $|q| = n\in \mathbb{N}$ is bounded independently of $n$ (as a consequence of Proposition 3), we have:

Therefore, for every $p \in \mathcal{P}$, the absolute convergence of $U_N$ to $u$ in $L^2(V_p)$ is proved. That is, since the sequence of the sets $V_q$ defines a partition of $\mathbb{R}^d$, $U_N$ converges to $u$ in $L^2_{loc}(\mathbb{R}^d)$. Using asymptotic estimate (38) for $\nabla_x \nabla_y G_{per}$ we can conclude with the same arguments to prove the convergence of $S_N$ in $L^2_{loc}(\mathbb{R}^d)$. In addition, the gradient operator being continuous in $\mathcal{D}'(\mathbb{R}^d)$, we have:

To complete the proof, we have to show that $u$ is a solution to (35). Let $N$ be in $\mathbb{N}$. By linearity of the operator $\operatorname{div(a_{per} \nabla.)}$, $U_N$ is a solution in $H^1_{loc}(\mathbb{R}^d)$ to:

We take the $L^2_{loc}$-limit when $N \rightarrow \infty$ in (46) and we obtain:

Therefore, $u$ is a solution to (35) in $\mathcal{D}'(\mathbb{R}^d)$.

Step 2: Proof of estimate (41)

Let $p$ be in $\mathcal{P}$, we want to split $u$ in two parts. For every $x \in V_p$, we write:

$I_{1,p}$ and $I_{2,p}$ are two distributions (they are in $L^2_{loc}(\mathbb{R}^d$)), so we can consider their gradients in a distribution sense. In addition, $I_{2,p}$ is a differentiable function on $V_p$ and

We start by establishing a bound for $\|\nabla I_{1,p}\|_{L^2(V_p)}$. First, we use estimate (36) for $\nabla_y G_{per}$ and we obtain:

We next apply the Cauchy-Schwarz inequality:

Property (ⅱ) implies that $W_p \subset Q_{C_1 2^{|p|}}(2^p)$. Therefore, for every $x \in W_p$ and $y \in W_p$, we have by a triangle inequality that $x-y \in Q_{C 2^{|p|+1}}$ and then:

Using (47) and the Fubini theorem, we finally obtain:

Lemma 4.2 ensures that $I_{1,p}$ is a solution in $\mathcal{D}'(\mathbb{R}^d)$ to:

Since Property (ⅱ) ensures $D(\partial V_p, W_p) \geq C2^{|p|}$, we can apply a classical inequality of elliptic regularity (see for instance [15,Theorem 4.4 p.63]) to equation (49) in order to establish the following estimate:

and we deduce from previous inequalities (48) and (50) that:

In addition, we have:

Next, we use a triangle inequality and Property (ⅳ) of $W_p$ to obtain:

We apply this inequality in (51) and we finally obtain:

where $C>0$ is independent of $p$.

We next prove a similar bound for $\| \nabla I_{2,p}\|_{L^2(V_p)}$. To start with, we want to show there exists a constant $C>0$ such that:

To this aim, we fix $x \in V_p$ and we use estimate (38) for $\nabla_x \nabla_y G_{per}$ to obtain:

Next, using Property (ⅱ) of $W_p$, there exists $C>0$ such that for every $q \neq p$,

and it follows:

The last inequality is actually a direct consequence of Propositions 1 and 5. In addition, we have proved in Proposition 3 there exists a constant $C>0$ such that for every $n\in \mathbb{N}$, the number of $q\in \mathcal{P}$ such that $|q| = n$ is bounded by $C$. Therefore we have:

And finally :

Furthermore, we have with similar arguments:

And we obtain again:

That is:

Using estimates (54) and (55), we have finally proved (53) and it follows:

Therefore we have the existence of a constant $C>0$ independent of $p$ such that:

For every $p \in \mathcal{P}$, using estimates (52) and (56) and a triangle inequality, we conclude that:

We finally obtain expected estimate (41) taking the supremum over all $p \in \mathcal{P}$ in the above inequality.

To conclude the study of problem (35) with a periodic coefficient, we next show that the solution to (40) given in Lemma 4.3 has a gradient in $\mathcal{B}^2(\mathbb{R}^d)$.

Lemma 4.4. Let $f\in \mathcal{B}^2(\mathbb{R}^d)^d$, then the function $u$ defined by (40) is the unique solution to (35) such that $\nabla u \in \mathcal{B}^2(\mathbb{R}^d)^d$.

Proof. We want to prove there exists a function $g \in L^2(\mathbb{R}^d)^d$ such that

In this proof, the letter C also denotes a generic constant independent of $p$, $u$ and $f$ that may change from one line to another. Using the result of Lemma 4.2, we can define a function $u_{\infty} \in L^2_{loc}(\mathbb{R}^d)$ by:

solution in $\mathcal{D}'(\mathbb{R}^d)$ to:

such that $\nabla u_{\infty} \in L^2(\mathbb{R}^d)^d$. For every $p\in \mathcal{P}$, by subtracting a $2^p$-translation of (57) from (35), the periodicity of $a_{per}$ implies:

For every $p \in \mathcal{P}$, in the sequel we denote $u_p = u-\tau_{-p}u_{\infty}$ and $f_p = f-\tau_{-p}f_{\infty}$. In order to prove $\nabla u \in \mathcal{B}^2(\mathbb{R}^d)^d$, the idea is to show that $\lim\limits_{|p| \rightarrow \infty} \int_{V_p} |\nabla u_p|^2 dx = 0$. We start by fixing $\varepsilon>0$. Since $f\in \mathcal{B}^2(\mathbb{R}^d)^d$, Proposition 12 gives the existence of a radius $R>0$, such that for every $p,q \in \mathcal{P}$,

In the sequel, the idea is to repeat step by step the method used in the proof of Lemma 4.3. For $p\in \mathcal{P}$, we thus introduce the set $W_p$ as in the previous proof and we split $u_p$ in two parts. For every $x \in V_p$, we can write:

In the sequel, we denote $A_p$ the set $W_p \setminus V_p$. As in the previous proof (see the details of the proof of estimate (51)) we can show that:

and we next prove that $\lim\limits_{|p| \rightarrow \infty} \|f_p\|^2_{L^2(W_p)} = 0$. First, since $f\in \mathcal{B}(\mathbb{R}^d)^d$, we already know that $\lim\limits_{|p| \rightarrow \infty} \int_{V_{p}} |f_p|^2 = 0$ and we only have to treat the integration term on $A_p$. Using Property (ⅲ) of $W_p$, we know that the distance $D(2^q,W_p)$, for $q\neq p$, is bounded from below by $2^{|p|}$. Therefore, if $2^{|p|} > R$, we obtains:

In addition, Property (ⅳ) of $W_p$ gives the existence of a constant $C>0$ such that the cardinality of the set of $q$ satisfying $V_q\cap W_p \neq \emptyset$ is bounded by $C$. Estimate (58) therefore implies that

Since $\varepsilon$ can be chosen arbitrarily small, we finally obtain $\lim\limits_{|p| \rightarrow \infty} \int_{A_{p}} |f_p|^2 = 0$, that is

We next prove that $\lim\limits_{|p| \rightarrow \infty} \| \nabla I_{2,p}\|^2_{L^2(V_p)} = 0$. We split $\nabla I_{2,p}$ in two parts such that for every $x \in V_p$:

We want to estimate $\| J_{1,p}\|_{L^2(V_p)}$ and $\| J_{1,p}\|_{L^2(V_p)}$. We proceed exactly in the same way as in the previous proof (see the details of estimate (53)) and, using estimate (58), we obtain the following inequalities:

and

To conclude, we consider $P>0$ such that for every $p \in \mathcal{P}$ satisfying $|p|>P$, we have:

Therefore, for every $|p|>P$, we use (59) and (60) and we obtain:

Since we can choose $\varepsilon$ arbitrarily small, we conclude that $\lim\limits_{|p| \rightarrow \infty} \|\nabla I_{2,p}\|_{L^2(V_p)} = 0$. Finally, by a triangle inequality we have $\lim\limits_{|p| \rightarrow \infty} \|\nabla u_{p}\|_{L^2(V_p)} = 0$, that is $\nabla u \in \mathcal{B}^2(\mathbb{R}^d)^d$.

Remark 4. It is important to note that the essential point of the two above proofs is the convergence of the sums of the form $\sum\limits_{q \in \mathcal{P}} \int_{V_q} \dfrac{1}{|x-y|^d} f(y) dy$ given in estimates (44), (54) and (55). Although we use here the particular distribution of the $2^p$, these convergence results are not specific to the set (9) considered in this study. They are actually ensured by Assumptions (H1), (H2) and (H3), particularly by the logarithmic bound given in Proposition 3 and Corollary 1. Indeed, with the notations of Section 1.2 and given Assumptions (H1)-(H2)-(H3), we could similarly argue to obtain estimates such as in (54)-(55) by splitting the sums over each annulus $A_n: = A_{2^n,2^{n+1}}$ and studying $\sum\limits_{n \in \mathbb{N}} \sum\limits_{x_q \in \mathcal{G}\cap A_n} \int_{V_{x_q}} \dfrac{1}{|x-y|^d} f(y) dy$. The results of existence stated in this section therefore still hold if we consider a generic set $\mathcal{G}$ satisfying our general assumptions.

Remark 5. In the two-dimensional context, the results of Lemmas 4.2, 4.3 and 4.4 remain true since estimates (36), (37) and (36) still hold. However the proof requires some additional technicalities, in particular to prove that the function $u$ defined by (40) makes sense. In this case the series (45) does not actually converge but it is still possible to prove that the series of the gradients (43) converges. Here, the difficulty is to show that the limit of (43), denoted by $T$ here, is the gradient in a distribution sense of a solution to (35). To this end, it is actually sufficient to show that $\partial_i T_j = \partial_j T_i$ for every $i,j \in \{1,...,d\}$. This result is obtained considering the property of the limit of $(43)$ in $\mathcal{D}(\mathbb{R}^d)$.

4.3.   Existence results in the general problem

Our aim is now to generalize the results established in the case of periodic coefficients to our original problem (31). Here, our approach is to prove in Lemma 4.5 the continuity of the linear operator $\nabla\left(-\operatorname{div}a\nabla\right)^{-1}\operatorname{div}$ from $\mathcal{B}^2(\mathbb{R}^d)^d$ to $\mathcal{B}^2(\mathbb{R}^d)^d$ in order to apply a method adapted from [8] and based on the connexity of the set $[0,1]$. This method is used in the proof of existence of Lemma 4.6. Finally, this result allows us to prove the existence of a corrector stated in Theorem 1.1.

Actually, we could have proved Lemmas 4.5 and 4.6 simultaneously but, in the interest of clarity, we first prove a priori estimate (61) and next, we establish the existence result in the general case.

Lemma 4.5. There exists a constant $C>0$ such that for every $f$ in $\mathcal{B}^2(\mathbb{R}^d)^d$ and $u$ solution in $\mathcal{D}'(\mathbb{R}^d)$ to (31) with $\nabla u$ in $\mathcal{B}^2(\mathbb{R}^d)^d$, we have the following estimate:

Proof. We give here a proof by contradiction using a compactness-concentration method. We assume that there exists a sequence $f_n$ in $\mathcal{B}^2(\mathbb{R}^d)^d$ and an associated sequence of solutions $u_n$ such that $\nabla u_n$ is in $\mathcal{B}^2(\mathbb{R}^d)^d$ and:

First of all, a property of the supremum bound ensures that for every $n\in \mathbb{N}$, there exists $x_n \in \mathbb{R}^d$ such that:

Next, in the spirit of the method of concentration-compactness [20], we denote ${\bar{u}}_n = \tau_{x_n}u_n$, $\bar{f}_n = \tau_{x_n}f_n$, $\bar{a}_n = \tau_{x_n}a$ and $\bar{{\tilde a}}_n = \tau_{x_n}{\tilde a}$ and we have for every $n \in \mathbb{N}$:

Next, for every $n\in \mathbb{N}$, ${\bar{u}}_n$ is a solution to:

Since the norm of $L^2_{unif}$ is invariant by translation, (63) and (64) ensure that $\bar{f}_n$ strongly converges to 0 in $L^2_{unif}(\mathbb{R}^d)$ and that the sequence $(\nabla {\bar{u}}_n)_{n\in \mathbb{N}}$ is bounded in $L^2_{unif}(\mathbb{R}^d)$. Therefore, up to an extraction, $\nabla{\bar{u}}_n$ weakly converges to a function $\nabla {\bar{u}}$ in $L^2_{loc}(\mathbb{R}^d)$.

The idea is now to study the limit of $\bar{a}_n$. To start with, we denote

Since $a_{per}$ is periodic, we have $\tau_{x_n}a_{per} = \; \tau_{\mathbf{x}_n}a_{per}$. In addition, the sequence $\mathbf{x}_n$ belongs to the unit cube of $\mathbb{R}^d$ and, therefore, it converges (up to an extraction) to $\mathbf{x} \in \mathbb{R}^d$. Since $a_{per}$ is Holder continuous, $\tau_{\mathbf{x}_n}a_{per}$ converges uniformly to $\tau_{\mathbf{x}}a_{per}$, which also belongs to $\left(L^2_{per}(\mathbb{R}^d) \cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^{d\times d}$.

In order to study the convergence of $\bar{{\tilde a}}_n$, we consider several cases depending on $x_n$:

$1.$ If $x_n$ is bounded, it converges (up to an extraction) to $x_{lim}\in \mathbb{R}^d$. Then, since ${\tilde a}$ is Holder-continuous, $\bar{{\tilde a}}_n$ strongly converges in $L^2_{loc}(\mathbb{R}^d)$ to $\tau_{x_{lim}}{\tilde a} \in\; \mathcal{B}^2(\mathbb{R}^d)^{d\times d}$.

$2.$ If $x_n$ is not bounded, since $\left(V_p\right)_{p\in \mathcal{P}}$ is a partition of $\mathbb{R}^d$, there exists an unbounded sequence $(p_n)_{n}$ in $\mathcal{P}$ such that $x_n = 2^{p_n} + t_n$ with $t_n \in V_{p_n}-2^{p_n}$.

– If $t_n$ is bounded, it converges (up to an extraction) to $t_{lim} \in \mathbb{R}^d$. In this case, for any compact subset $K$ of $\mathbb{R}^d$, we have

First, since $t_n$ is bounded and $p_n$ is unbounded, we have $K+2^{p_n}+t_n$ is included in $V_{p_n}$ for $n$ sufficiently large. Therefore, $\|{\tilde a} - \tau_{-p_n}{\tilde a}_{\infty}\|_{L^2(K+2^{p_n}+t_n)}$ converges to 0 when $n \to \infty$. Second, ${\tilde a}_{\infty}$ is Holder-continuous and $t_n$ converges to $t_{lim}$. Thus, ${\tilde a}_{\infty}(.+t_n)$ converges uniformly to ${\tilde a}_{\infty}(.+t_{lim})$ and $\|{\tilde a}_{\infty}(.+t_n)- {\tilde a}_{\infty}(.+t_{lim})\|_{L^2(K)}$ converges to 0. Finally, $\bar{{\tilde a}}_n$ converges to ${\tilde a}_{\infty}(.+t_{lim})$ in $L^2(K)$ for every compact subset $K$.

– If $t_n$ is unbounded, we can always assume that $|t_n| \to \infty$ up to an extraction. We have for every $K$ compact of $\mathbb{R}^d$,

First, since ${\tilde a}_{\infty}$ belongs to $L^2(\mathbb{R}^d)^{d\times d}$ and $t_n$ is unbounded we have that $\|{\tilde a}_{\infty}\|_{L^2(K+t_n)}$ converges to 0 when $n \to \infty$. Secondly, we introduce the set $W_{2^{p_n}}$ defined as in Proposition 4. For every $R>0$, the properties of $W_{2^{p_n}}$ allow to show that there exists $N\in \mathbb{N}$ such that for all $n>N$, we have $K + 2^{p_n} +t_n \subset W_{2^{p_n}}$ and:

Using Proposition 4, we know that the number of $q$ such that $V_q \cap W_{2^{p_n}}$ is not empty, is uniformly bounded with respect to $n$. Proposition 12 finally ensures that $\|{\tilde a} - \tau_{-p_n}{\tilde a}_{\infty}\|_{L^2(K+2^{p_n}+t_n)} \rightarrow 0$. Therefore, $\bar{{\tilde a}}_n$ strongly converges to 0 in $L^2_{loc}(\mathbb{R}^d)$.

In any case, the sequence $a_{per}+\bar{{\tilde a}}_n$ therefore converges to a coefficient $A = \tau_{\mathbf{x}}a_{per} + \tilde{A}$, where $\tilde{A}$ is of the form

In the three cases, as a consequence of Assumptions (12) and (13), the coefficient $A$ is clearly bounded, elliptic and belongs to $\left(\mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^{d \times d}$. Moreover, as a consequence of the uniform Holder-continuity (with respect to $n$) of $\bar{a}_n - A$, the convergence of $\bar{a}_n$ to $A$ is also valid in $L^{\infty}_{loc}(\mathbb{R}^d)$.

The next step of the proof is to study the limit $\nabla {\bar{u}}$ of $\nabla {\bar{u}}_n$ in these three cases. First, since $\bar{a}_n$ strongly converges to $A$ in $L^{2}_{loc}(\mathbb{R}^d)$, considering the weak limit in (62) when $n\to \infty$, we obtain

We now state that $\nabla {\bar{u}} = 0$. Indeed,

$1.$ if $x_n$ is bounded, assumption (64) ensures that there exists a constant $C>0$ such that for all $n \in \mathbb{N}$ and $p \in \mathcal{P}$, we have:

Therefore, the property of lower semi-continuity satisfied by the norm $\|.\|_{L^2}$ implies

And we obtain $\sup\limits_p \| \nabla {\bar{u}} \|_{L^2(V_p)} < \infty$. Finally, since $A$ is elliptic and bounded and ${\bar{u}}$ is solution to (66), the uniqueness results of Lemma 4.1 gives $\nabla {\bar{u}} = 0$ on $\mathbb{R}^d$.

$2.$ if $x_n$ is not bounded, we know that $x_n = 2^{p_n} + t_n$ where $|p_n| \to \infty$. For every $n \in \mathbb{N}$:

Up to an extraction, the sequence $V_{p_n} - x_n$ is an increasing sequence of sets, and we can show that $\bigcup\limits_{n\in \mathbb{N}} \left(V_{p_n} - x_n\right) = \mathbb{R}^d$ (see the proof of Proposition 2). Consequently, for every $R>0$, there exists $N\in \mathbb{N}$ such that $B_R \subset \left(V_{p_N}-x_N\right)$ and

Using again lower semi-continuity, we have for every $R>0$:

We obtain that $\nabla {\bar{u}}\in L^2(\mathbb{R}^d)$. Since $A$ is bounded and elliptic, a result of uniqueness established in [10,Lemma 1] finally ensures that $\nabla {\bar{u}} = 0$.

We are now able to show that $\nabla {\bar{u}}_n$ strongly converges to 0 in $L^2(B_1)$. To this aim, we note that, for every $n$, the addition of a constant to ${\bar{u}}_n$ does not affect $\nabla {\bar{u}}_n$. Then, without loss of generality, we can always assume that $\int_{B_2} {\bar{u}}_n$ = 0 and the Poincaré-Wirtinger inequality gives the existence of a constant $C>0$ independent of $n$ such that:

${\bar{u}}_n$ is therefore bounded in $H^1(B_2)$ according to Assumption (64). The Rellich theorem ensures that, up to an extraction, ${\bar{u}}_n$ strongly converges to ${\bar{u}}$, that is to 0, in $L^2(B_2)$. Since ${\bar{u}}_n$ is solution to (62), a classical inequality of elliptic regularity gives the following estimate (see for instance [15,Theorem 4.4 p.63]):

where $C$ depends only of $a$ and the ambient dimension $d$. We therefore consider the limit when $n\rightarrow \infty$ to conclude that $\nabla {\bar{u}}_n$ strongly converges to $0$ in $L^2(B_1)$. We next use (65) and the strong convergence of $\nabla {\bar{u}}_n$ to 0 in $L^2(B_1)$ to conclude that

That is, $\nabla u_n$ strongly converges to 0 in $L^2_{unif}(\mathbb{R}^d)$.

In order to conclude this proof, we will show that $\nabla u_n$ actually converges to 0 in $\mathcal{B}^2(\mathbb{R}^d)$ and obtain a contradiction.

First of all, we study the behavior of the sequence $\nabla u_{n,\infty}$. For $p \in \mathcal{P}$, we consider the $2^p$-translation of (62) and we have

Letting $|p|$ go to the infinity, for every $n \in \mathbb{N}$, we obtain that $\nabla u_{n,\infty}$ is a solution to:

An estimate established in [8,Proposition 2.1], gives the existence of a constant $C>0$ independent of $n$ such that:

By assumption, we have $\lim\limits_{n \rightarrow \infty}\| f_{n,\infty}\|_{L^2(\mathbb{R}^d)} = 0$ and we deduce that $\nabla u_{n,\infty}$ strongly converges to 0 in $L^2(\mathbb{R}^d)$, that is:

The last step is to establish that:

Let $\varepsilon>0$. Since ${\tilde a}$ belongs to $\left(\mathcal{B}^2(\mathbb{R}^d)\right)^{d\times d}$ and is uniformly continuous, a direct consequence of Proposition 12 gives the existence of $R>0$ such that:

In addition, since $\nabla u_n$ strongly converges to 0 in $L^2_{unif}$, there exists $N\in \mathbb{N}$ such that:

Using the last two inequalities, we obtain for every $q \in \mathcal{P}$:

Therefore:

We next consider equation (62) and we use Lemma 4.1 to ensure that, up to the addition of a constant, $u_n$ is the unique solution to:

such that $\sup\limits_p \|\nabla u_n\|_{ L^2(V_p)} < \infty$. Then, estimate (41) established in Lemma 4.3 gives the existence of a constant $C>0$ independent of $n$ such that:

Letting $n$ go to the infinity, we deduce that $\lim\limits_{n \rightarrow \infty} \sup\limits_p \|\nabla u_n\|_{ L^2(V_p)} = 0$. We can finally conclude that

and, since $\nabla u_n$ satisfies (64), we have a contradiction.

Lemma 4.6. Let $f \in \mathcal{B}^2(\mathbb{R}^d)^d$, there exists $u\in H^1_{loc}(\mathbb{R}^d)$ solution to (31) such that $\nabla u \in\; \mathcal{B}^2(\mathbb{R}^d)^d$.

Proof. First of all, we remark that it is sufficient to prove this existence result when $f \in \left(\mathcal{B}^2(\mathbb{R}^d)\cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^d$. Indeed, if we denote $\Phi = \nabla\left(-\operatorname{div}a\nabla\right)^{-1}\operatorname{div}$ the reciprocal linear operator from $\left(\mathcal{B}^2(\mathbb{R}^d)\cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^d$ to $\left(\mathcal{B}^2(\mathbb{R}^d)\right)^d$ associated with equation (31) and we assume that $\Phi$ is well defined, Lemma 4.5 ensures it is continuous with respect to the norm of $\mathcal{B}^2(\mathbb{R}^d)$. Then, we are able to conclude in the general case using the density result stated in Proposition 8. In the sequel of this proof, we therefore assume that $f$ belongs to $\mathcal{C}^{0,\alpha}(\mathbb{R}^d)^d$.

To start with, we show a preliminary result of regularity satisfied by the solutions to (31). Assuming $f\in \left(\mathcal{B}^2(\mathbb{R}^d)\cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^d$, we want to prove that a solution $u$ to (31) such that $\nabla u \in \mathcal{B}^2(\mathbb{R}^d)^d$ also satisfies $\nabla u \in \mathcal{C}^{0,\alpha}(\mathbb{R}^d)^d$. Indeed, if $u$ is such a solution to (31), a consequence of a regularity result established in [15,Theorem 5.19 p.87] (see also [14,Theorem 3.2 p.88]) gives the existence of $C>0$ such that for all $x \in \mathbb{R}^d$:

Therefore, $\nabla u$ belongs to $\left(\mathcal{C}^{0,\alpha}(\mathbb{R}^d)\cap \mathcal{B}^2(\mathbb{R}^d)\right)^d$.

In the sequel of the proof, we use an argument of connexity adapted from [8]. Let $\mathbf{P}(a)$ the following assertion: "There exists a solution $u\in \mathcal{D}'(\mathbb{R}^d)$ to:

such that $\nabla u \in \left(\mathcal{B}^2(\mathbb{R}^d)\cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^d$."

For $t\in [0,1]$, we denote $a_t = a_{per} + t{\tilde a}$ and we define the following set:

Our aim is to show that $\mathbf{P}(a_1) = \mathbf{P}(a)$ is true. To this end, we will prove that $\mathcal{I}$ is non empty, closed and open for the topology of $[0,1]$ and conclude that $\mathcal{I} = [0,1]$.

$\mathcal{I}$ is not empty

For $t = 0$, the existence of a solution $u$ such that $\nabla u \in \mathcal{B}^2(\mathbb{R}^d)^d$ is a direct consequence of Lemma 4.4. We just have to use (67) to show the uniform Holder continuity of the gradient of the solution.

$\mathcal{I}$ is open

We assume there exists $t\in \mathcal{I}$ and we will find $\varepsilon>0$ such that $[t,t+\varepsilon] \subset \mathcal{I}$. For $f \in \left(\mathcal{B}^2(\mathbb{R}^d) \cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^d$, we want to solve:

where $\nabla u \in \left(\mathcal{B}^2(\mathbb{R}^d)\cap \mathcal{C}^{0, \alpha}(\mathbb{R}^d)\right)^d$. According to Proposition 9, for such a solution, we have $\varepsilon {\tilde a} \nabla u \in \left(\mathcal{B}^2(\mathbb{R}^d)\cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^d$. Next, we remark that equation (68) is equivalent to:

where $\Phi_t$ is the reciprocal linear operator associated with the equation when $a = a_t$. Lemma 4.5 and estimate (67) imply the continuity of $\Phi_t$ from $\left(\mathcal{B}^2(\mathbb{R}^d) \cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^d$ to $\left(\mathcal{B}^2(\mathbb{R}^d) \cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^d$ for the norm $\|.\|_{\mathcal{B}^2(\mathbb{R}^d)} + \|.\|_{\mathcal{C}^{0,\alpha}(\mathbb{R}^d)}$. We fix $\varepsilon$ such that:

Therefore $g \rightarrow \Phi_t(\varepsilon{\tilde a}g + f) \in \mathcal{L}\left(\left(\mathcal{B}^2(\mathbb{R}^d) \cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^d \right)$ is a contraction in a Banach space. Finally, we can apply the Banach fixed-point theorem to obtain the existence and the uniqueness of a solution to (69) and we deduce that $[t,t+ \varepsilon] \subset \mathcal{I}$.

$\mathcal{I}$ is closed

We assume there exist a sequence $(t_n) \in \mathcal{I}^{\mathbb{N}}$ and $t\in [0,1]$ such that $\lim_{n \rightarrow \infty} t_n = t$ and $t_n<t$. For every $t_n$, there exists $u_n$ solution to:

such that $\nabla u_n \in \mathcal{B}^2(\mathbb{R}^d)^d$. For every $n \in \mathbb{N}$, Lemma 4.5 gives the existence of a constant $C_n$ such that:

We first assume that $C_n$ is bounded independently of $n$ by a constant $C>0$. Therefore, up to an extraction, $\nabla u_n$ weakly converges to a gradient $\nabla u$ in $L^2_{loc}(\mathbb{R}^d)$ and, using the lower semi-continuity of the $L^2$-norm, we have

In addition, for every $n\in \mathbb{N}$, $u_n$ is a solution to the equivalent equation:

Next, since $t_n$ converges to $t$, we directly obtain that $a_{t_n}$ converges to $a_t$ in $\mathcal{B}^2(\mathbb{R}^d)$. In addition, since $\nabla u_n$ is bounded by a constant independent of $n$ in $L^2_{unif}(\mathbb{R}^d)$, the sequence $(a_{t_n} - a_{t})\nabla u_n$ strongly converges to $0$ in $L^2_{loc}(\mathbb{R}^d)$. We can therefore consider the limit in (70) when $n \to \infty$ and deduce that $u$ is a solution to:

We have to prove that $\nabla u \in \mathcal{B}^2(\mathbb{R}^d)$. For every $m, \ n \in \mathbb{N}$, $u_n - u_m$ is a solution to:

and we have the following estimate:

Therefore, $\nabla u_n$ is a Cauchy-sequence in $\mathcal{B}^2(\mathbb{R}^d)^d$ and since this space is a Banach space, we directly obtain that $\nabla u$ belongs to $\mathcal{B}^2(\mathbb{R}^d)^d$ and we have the expected result.

Now, we want to prove that $C_n$ is bounded independently of $n$ using a proof by contradiction. We assume there exist two sequences $f_n$ and $u_n$ such that $\nabla u_n$ belongs to $\mathcal{B}^2(\mathbb{R}^d)^d$ and:

For every $n \in \mathbb{N}$, the above equation is equivalent to:

We can next remark that the boundedness of $\nabla u_n$ in $\mathcal{B}^2(\mathbb{R}^d)$ ensures that the sequence $(a_{t_n} - a_{t})\nabla u_n$ is strongly convergent to 0 in $\mathcal{B}^2(\mathbb{R}^d)$. Finally, we can conclude exactly as in the proof of Lemma 4.5.

Since $[0,1]$ is a connected space, we can finally conclude that $\mathcal{I} = [0,1]$. In addition, if $u \in \mathcal{D}'(\mathbb{R}^d)$ is such that $\nabla u \in \mathcal{B}^2(\mathbb{R}^d)^d\subset{L^2_{loc}(\mathbb{R}^d)^d}$, the result of [12,Corollary 2.1] finally ensures that $u \in L^2_{loc}(\mathbb{R}^d)$.

In the above proof, we have proved the following result:

Corollary 4. Let $f \in \left(\mathcal{B}^2(\mathbb{R}^d) \cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^d$ and $u \in H^1_{loc}(\mathbb{R}^d)$ solution to (31) such that $\nabla u \in \mathcal{B}^2(\mathbb{R}^d)^d$. Then $\nabla u \in \mathcal{C}^{0,\alpha}(\mathbb{R}^d)^d$.

Remark 6. Again, we do not need the restriction that we did on the dimension to prove the existence results stated in this section and we can easily generalize the existence of a solution to (31) in a two-dimensional context.

4.4.   Existence of the corrector

To conclude this section, we finally give a proof of Theorem 1.1 and, therefore, we obtain the existence of a unique corrector solution to (14) such its gradient belongs to $L^2_{per}(\mathbb{R}^d) + \mathcal{B}^2(\mathbb{R}^d)$. To this end, we remark that corrector equation (14) is equivalent to a an equation of the form (31) and we use the preliminary results of uniqueness and existence proved in this section.

Proof of theorem 1.1. $Existence$

Let $p$ be in $\mathbb{R}^d$. We want to find a solution to (14) of the form $w_{per,p} + \tilde{w}_p$ where $w_{per,p}$ is the unique periodic corrector (that is the unique periodic solution to the corrector equation (14) when ${\tilde a} = 0$) and such that $\nabla \tilde{w}_p\in \mathcal{B}^2(\mathbb{R}^d)^d$. First of all, we remark that equation (14) is equivalent to:

It is well known that $\nabla w_{per,p} \in \left(L^2_{per}(\mathbb{R}^d) \cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^d$ and therefore, using the periodicity of $\nabla w_{per,p}$, we can easily show that ${\tilde a}(p + \nabla w_{per,p}) \in \left(\mathcal{B}^2(\mathbb{R}^d)\cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^d$. Then, the existence of $\tilde{w}_p$ such that $\nabla \tilde{w}_p \in \left(\mathcal{B}^2(\mathbb{R}^d)\cap \mathcal{C}^{0,\alpha}(\mathbb{R}^d)\right)^d$ is given by Lemma 4.6 and Corollary 4. Since $\nabla \tilde{w}_p \in \left(\mathcal{B}^2(\mathbb{R}^d)\cap \ L^{\infty}(\mathbb{R}^d)\right)^d$, the sub-linearity at infinity of $\tilde{w}_p$ is a direct consequence of Proposition 11.

$Uniqueness$

We assume there exist two solutions $u_1$ and $u_2$ to (14) such that $\nabla u_1$ and $\nabla u_2$ belong to $\left(L^2_{per}(\mathbb{R}^d) + \mathcal{B}^2(\mathbb{R}^d)\right)^d$. We denote $v = u_1-u_2$ and we have $\nabla v = g_{per} + \tilde{g}$ where $g_{per} \in L^2_{per}(\mathbb{R}^d)^d$ and $\tilde{g}\in\mathcal{B}^2(\mathbb{R}^d)^d$. For every $q\in \mathcal{P}$, we have $\tau_{q}\nabla v = g_{per} + \tau_{q}\tilde{g}$ by periodicity of $g_{per}$. Since $\tilde{g}$ belongs to $\mathcal{B} ^2(\mathbb{R}^d)^d$, there exists $\tilde{g}_{\infty} \in L^2(\mathbb{R}^d)^d$ such that $\tau_q\nabla v$ converges in $\mathcal{D}'(\mathbb{R}^d)$ to $\nabla v_{\infty} = g_{per} + \tilde{ g}_{\infty}$ when $|q| \rightarrow \infty$. In addition, considering the limit in equation (14), we obtain that $v_{\infty}$ is a solution to:

Since $a$ satisfies assumption (12) and (13), the coefficient $a_{per} + {\tilde a}_{\infty}$ is a bounded and elliptic matrix-valued coefficient. Therefore, the result established in [10,Lemma 1] allows us to conclude that $g_{per} = 0$ and finally, that $\nabla v = \tilde{g} \in \mathcal{B}^2(\mathbb{R}^d)^d$. Since $v$ is a solution to:

we use Lemma 4.1 to obtain that $\nabla v = 0$ and the uniqueness is proved.

5.   Homogenization results and convergence rates

In this section we use the corrector, solution to (14) and defined in Theorem 1.1, to establish an homogenization theory similar to that established in [6] for the periodic case with local perturbations. In Proposition 13 we first study the homogenized equation associated with (1) and we conclude showing estimates (15) and (16) stated in Theorem 1.2.

5.1.   Homogenization results

To start with, we determine here the limit of the sequence $u^{\varepsilon}$ of solutions to (1). In Proposition 13 below we show the homogenized equation is actually the diffusion equation (3) where the diffusion coefficient $a^*$ is defined by (5), that is the homogenized coefficient is the same as in the periodic case when $a = a_{per}$. This phenomenon is similar to the results established in [8] in the case of localized defects of $L^p(\mathbb{R}^d)$. It is a direct consequence of Proposition 10 regarding the average of the functions in $\mathcal{B}^2(\mathbb{R}^d)$ which is satisfied by our perturbations. The idea is that, on average, the perturbations belonging to $\mathcal{B}^2(\mathbb{R}^d)$ therefore do not impact the periodic background.

Proposition 13. Assume $\Omega$ is an open bounded set of $\mathbb{R}^d$, let $f \in L^2(\Omega)$ and consider the sequence $u^{\varepsilon}$ of solutions in $H^1_0(\Omega)$ to (1).Then the homogenized (weak-$H^1(\Omega)$ and strong-$L^2(\Omega)$) limit $u^*$ obtained when $\varepsilon \rightarrow 0$ is the solution to (3) where the homogenized coefficient is identical to the periodic homogenized coefficient (5).

Proof. We denote $w = (w_{e_i})_{i \in \{1,...,d\}}$, the correctors given by Theorem 1.1 for $p = e_i$. The general homogenization theory of equations in divergence form (see for instance [21,Chapter 6,Chapter 13]), gives the convergence, up to an extraction, of $u^{\varepsilon}$ to a function $u^*$ solution to an equation in the form (3). In addition, for all $1 \leq i,j \leq d$, the homogenized matrix $a^*$ associated with $a$ is given by:

where the weak limit is taken in $L^{2}(\Omega)^{d \times d}$. By assumption, we have $a = a_{per} + {\tilde a}$ and we know that $\nabla w_{e_i} = \nabla w_{per,e_i} + \nabla \tilde{w}_{e_i}$ where ${\tilde a} \in \left(\mathcal{B}^2(\mathbb{R}^d) \cap L^{\infty}(\mathbb{R}^d)\right)^{d\times d}$ and $\nabla \tilde{w}_{e_i}\; \in \left(\mathcal{B}^2(\mathbb{R}^d) \cap L^{\infty}(\mathbb{R}^d)\right)^{d}$. Therefore, Corollary 2 ensures that $|{\tilde a}(./\varepsilon)|$ and $|\nabla \tilde{w}_{e_i}(./ \varepsilon)|$ converge to 0 in $weak^*-L^{\infty}$ and, since $a_{per}$ and $\nabla w_{per}$ are bounded, we can deduce that:

Consequently, we have

This limit being independent of the extraction, all the sequence $u^{\varepsilon}$ converges to $u^*$ and we have the equality $a^* = a^*_{per}$.

5.2.   Approximation of the homogenized solution and quantitative estimates

The existence of the corrector established in Theorem 1.1 allows to consider a sequence of approximated solutions defined by $u^{\varepsilon,1} = u^* + \varepsilon \sum_{i = 1}^d \partial_{i}u^* w_{i}(./ \varepsilon)$ where for every $i$ in $\{1,...,d\}$, we have denoted $w_i = w_{e_i}$. Our aim here is to estimate the accuracy of this approximation for the topology of $H^1(\Omega)$. In particular, we want to prove the convergence to $0$ of the sequence $R^{\varepsilon}$ defined by:

and specify the convergence rate in $H^1(\Omega)$.

A classical method in homogenization used to obtain some expected quantitative estimates consists in defining a divergence-free matrix (as a consequence of corrector equation (14)) by

and to find a potential $B$ which formally solves $M = \operatorname{curl}(B)$. Knowing that both the coefficient $a$ and $\nabla w$ belong to $L^2_{per} + \mathcal{B}^2(\mathbb{R}^d)$, we can split $M$ in two terms and obtain $M = M_{per} + \tilde{M} \in \left(L^2_{per}(\mathbb{R}^d) + \mathcal{B}^2(\mathbb{R}^d)\right)^{d\times d}$. Therefore, we expect to find a potential of the same form, that is $B = B_{per} + \tilde{B}$. Rigorously, for every $i,j \in \{1,...,d\}$, we want to solve the equation:

The existence of a periodic potential $B_{per}$ solution to $M_{per} = \operatorname{curl}(B_{per})$ is well known since, component by component, $M_{per}$ is divergence-free. Here, the main difficulty is to show the existence of the potential $\tilde{B}$ associated with the $\mathcal{B}^2$- perturbation. This result is given by the following lemma.

Lemma 5.1. Let $\tilde{M} = \big( \tilde{M}^i_k\big)_{1 \leq i,k\leq d} \in \left(\mathcal{B}^2(\mathbb{R}^d)\right)^{d \times d}$ such that $\operatorname{div}(\tilde{M}_k) = 0$ for every $k \in \{1, ...,d\}$. Then, the potential $\tilde{B}^{i,j}_k$ defined by:

where $C(d)>0$ is a constant associated with the unit ball surface of $\mathbb{R}^d$, satisfies $\nabla \tilde{B}^{i,j}_k \in \mathcal{B}^2(\mathbb{R}^d)^d$ and for all $i,j,k \in \{1,...,d\}$:

In addition, there exists a constant $C_1>0$ which only depends of the ambient dimension $d$ such that:

Proof. First, for every $i,j,k\in \{1,...,d\}$, equation (73) is equivalent to an equation of the following form:

where $\mathcal{M}^{i,j}_k$ is a vector function defined by:

Since $\mathcal{M}^{i,j}_k \in \mathcal{B}^2(\mathbb{R}^d)^d$, the existence of $\tilde{B}$ and estimate (76) are given by Lemmas 4.3, 4.4 and 4.5 (here $a_{per} \equiv 1$). Equality (74) is a direct consequence of the definition of $\tilde{B}$. Property (75) can be easily obtained applying the divergence operator to (72).

Corollary 5. The potential $B = B_{per} + \tilde{B}$, where $\tilde{B}$ is given by Lemma 5.1, is the expected potential solution to (71). In addition, the couple $(M,B)$ satisfies the following equalities:

Now that existence of the potential $B$ has been dealt with, we can remark that $R^{\varepsilon}$ is a solution to the following equation:

where:

For a complete proof of equality (77), we refer to [6,Proposition 2.5].

To conclude, we have to study the properties of $H^{\varepsilon}$. In particular, we next prove that both the corrector $\tilde{w}$ and the potential $\tilde{B}$ are bounded. This property is essential for establishing the estimates of Theorem 1.2.

Lemma 5.2. The corrector $w = \left(w_i\right)_{i \in \{1,...,d\}}$ defined by Theorem 1.1 and the potential $B$ solution to (71) are in $L^{\infty}(\mathbb{R}^d)$.

Proof. First, it is well known that both $w_{per}$ and $B_{per}$ belong to $L^{\infty}(\mathbb{R}^d)$. Next, for all $i \in \{1,...,d\}$, $\tilde{w}_i$ solves:

We know the gradient of the corrector defined in Theorem 1.1 is in $\mathcal{C}^{0,\alpha}(\mathbb{R}^d)^d$. A direct consequence of Assumption (13) and Proposition 9 ensures that $f = {\tilde a}\left(e_i + \nabla w_{per,i} + \nabla \tilde{w}_i \right)$ belongs to $\left(L^{\infty}(\mathbb{R}^d) \cap \mathcal{B}^2(\mathbb{R}^d)\right)^d$ and the results of uniqueness and existence established in Lemmas 4.1 and 4.4 imply we have the following representation:

We want to prove that the integral is bounded independently of $x$. We take $x\in \mathbb{R}^d$ and denote $p_x$ the unique element of $\mathcal{P}$ such that $x \in V_{p_x}$. We define $W_{p_{x}} = W_{2^{p_x}}$ such as in Proposition 4 and we split the integral in three parts:

We start by finding a bound for $I_1(x)$. To this end, we use estimate (36) for the Green function and we obtain

Where $C$ denotes a positive constant independent of $x$. Indeed, the value of the integral in the last inequality depends only of the dimension $d$.

Next, using Proposition 4, we know there exists $C_1>0$ and $C_2>0$ independent of $x$ such that $W_{p_x} \subset\; B_{C_12^{p_x}(x)}$ and the number of $q \in \mathcal{P}$ such that $V_q \cap W_{p_x} \neq \emptyset$ is bounded by $C_2$. Therefore, using the Cauchy-Schwarz inequality, we have:

In addition since $d>2$, we have:

and therefore:

Finally, to bound $I_3(x)$ we split the integral on each cell $V_q$ for $q \in \mathcal{P}$. Using the Cauchy-Schwarz inequality, we obtain:

We proceed exactly as in the proof of Lemma 4.3 (see the proof of estimate (53) for details) to obtain:

Finally we have bounded the integral independently of $x$ and we deduce that $\tilde{w}_i \in \; L^{\infty}(\mathbb{R}^d)$. With the same method we obtain the same result for $B = B_{per} + \tilde{B}$ which allows us to conclude.

Remark 7. The assumption $d>2$ is essential in the above proof and the boundedness of $\tilde{w}$ in $L^{\infty}(\mathbb{R}^d)$ may be false if $d = 1$ or $d = 2$. If $d = 1$ we give a counter-example in Remark 9. The case $d = 2$ is a critical case and we are not able to conclude. This phenomenon is closely linked to the critical integrability of the function $|x|^{-1}$ in $L^2(\mathbb{R}^2)$.

Remark 8. As in the proofs of Lemmas 4.3 and 4.4, the above proof strongly uses the specific behavior of the Green function $G_{per}$ and our approach consists in showing the convergence of a sum of the form $\sum\limits_{q \in \mathcal{P}} \int_{V_q} \dfrac{1}{|x-y|^{d-1}}f(y) dy$. Here, we explicitly use the geometric properties satisfied by the $2^p$ but, once again, this convergence is not specific to the set (9) and also holds under the generality of (H1), (H2) and (H3). We refer to Remark 4 for additional details.

We are now able to give a complete proof of Theorem 1.2.

Proof of Theorem 1.2. First, we use the explicit definition of $H^{\varepsilon}$ given by (78) and a triangle inequality to obtain the following estimate:

Applying Lemma 5.2, we obtain the existence of $C>0$ independent of $\varepsilon$ such that

Next, we use the following two estimates satisfied by $R^{\varepsilon}$:

and for every $\Omega_1 \subset \subset \Omega$:

where $C_1>0$ and $C_2>0$ are independent of $\varepsilon$. These estimates are established for instance in [6] where the authors use the elliptic regularity associated with equation (77) and the properties of the Green function associated with the operator $-\operatorname{div}(a^*\nabla.)$ on $\Omega$ with homogeneous Dirichlet boundary condition. The first estimate is established in the proof of [6,Lemma 4.8] and the second estimate is a classical inequality of elliptic regularity proved in [6,Proposition 4.2] and applied to equation (77).

In addition, an application of elliptical regularity to equation (3) provides the existence of $C_3>0$ such that:

To conclude we use Lemma 5.2 to bound $w$ and $B$ and estimates (79), (80), (81) and (82). We finally obtain:

and

where $C$ and $\tilde{C}$ are independent of $\varepsilon$. We have proved Theorem 2.

Remark 9. In the one-dimensional case, that is when $d = 1$, we are not able to conclude in the same way. With an explicit calculation, we obtain:

where:

In this case,

and

and we can show the corrector $w$ is not necessarily bounded. However, the results of Proposition 10, allow us to obtain the following estimate:

As an illustration, we can consider $\Omega = ]0,1[$, $a_{per} = 1$ and ${\tilde a} = \sum\limits_{p \in \mathbb{Z}} \tau_{-2^p}\varphi$, where $\varphi$ is a positive function of $\mathcal{D}(\mathbb{R})$, $\|\varphi\|_{L^{\infty}} = 1$, $\int_{\mathbb{R}} \varphi> 0$ and $Supp(\varphi) \in [0,1/2]$. With these parameters, for every $x\in \Omega$, we have:

And therefore, the corrector is actually not bounded.

Remark 10. The result of Theorem 1.2 ensures that the corrector introduced in Theorem 1.1 allows to precisely describe the behavior of the sequence $u^{\varepsilon}$ in $H^1$ using the approximation defined by $u^{\varepsilon,1} = u^* + \varepsilon \sum\limits_{i = 1}^d \partial_i u^* w_i(./\varepsilon)$. However, since the perturbations of $\mathcal{B}^2(\mathbb{R}^d)$ are "small" at the macroscopic scale (in the sense of average given by (25)), we can naturally expect that it is also possible to approximate $u^{\varepsilon}$ in $H^1$ considering the sequence $u^{\varepsilon,1}_{per} : = u^* + \varepsilon \sum\limits_{i = 1}^d \partial_i u^* w_{per,i}(./\varepsilon)$ which only uses the periodic part $w_{per}$ of our corrector. To this aim, we can show that $u^{\varepsilon} - u^{\varepsilon,1}_{per}$ is solution to

where the right-hand side

strongly converges to 0 in $L^2$ when $\varepsilon \to 0$. A method similar to that used in the proof of Theorem 1.2 therefore allows to show the convergence to 0 of $u^{\varepsilon} - u^{\varepsilon,1}_{per}$ in $H^1$. It follows, at the macroscopic scale, that the choice of our adapted corrector instead of the periodic corrector seems to be not necessarily relevant in order to calculate an approximation of $u^{\varepsilon}$ in $H^1$. However, the choice of the periodic corrector is not adapted if the idea is to approximate the behavior of $u^{\varepsilon}$ at the microscopic scale $\varepsilon$. Indeed, at this scale, the perturbations of the periodic background can be possibly large and it is necessary to consider a corrector that take these perturbations into account. Particularly, if $H^{\varepsilon}_{per}$ is the function defined by (83), we can easily show that $H^{\varepsilon}_{per}(\varepsilon.)$ does not converge to 0 in any ball $B_R$ such that $\varepsilon B_R \subset \Omega$, which formally reflects a poor quality of the approximation of $u^{\varepsilon}$ by $u^{\varepsilon,1}_{per}$ at the scale $\varepsilon$. This fact particularly motivates the choice of our adapted corrector in order to approximate $u^{\varepsilon}$. We refer to [17] for a rigorous formalization of the above argument.

Acknowledgments

The author thanks the anonymous referee for many constructive comments. The author also thanks Claude Le Bris and Xavier Blanc for suggesting this problem, for their support, and for many fruitful discussions.