A nonlinear diffusion equation with reaction localized in the half-line

In memoriam of our friend Ireneo Peral. Master of Mathematics.

1.   Introduction

We consider the following Cauchy problem

We take exponents $m, p > 0$ and the coefficient is the characteristic function of a half-line, $a(x) = \mathbb{1}_{(0, \infty)}(x)$. The initial value $u_0\in L^1(\mathbb{R})\cap L^\infty(\mathbb{R})$ is assumed to be continuous and nonnegative, so that nonnegative solutions $u\ge0$ are considered. We are interested in characterizing and describing the phenomena of blow-up and grow-up for the solutions to (1.1) in terms of the parameters of the problem, the exponents $m$ and $p$ and the initial datum $u_0$. By a solution $u$ having blow-up we mean that there exists a finite time $T$ such that $u$ is well defined and finite for $t < T$ and

When $T = \infty$ we say that $u$ has grow-up.

The problem with global reaction $a(x) = 1$ has been deeply studied in the last years mainly concerning blow-up and $p > 1$, see for instance the survey book ^[14], but also in relation to grow-up, and thus $p\le1$, see ^[1,12]. In fact there can exist blow-up solutions only if $p > 1$, and in that range small initial data produce global solutions if and only if $p > m+2$. The global solutions are unbounded if $p\le1$, i.e., they have grow-up, while they are globally bounded if $p > m+2$. The exponents $p_0 = 1$ and $p_c = m+2$ are called, respectively, global existence exponent and Fujita exponent. For the related case in which the reaction coefficient is $a(x) = \mathbb{1}_{(-L, L)}(x)$, $0 < L < \infty$, the exponents are $p_0 = \max\{1, \frac{m+1}2\}$ and $p_c = m+1$, see ^[2,4,13].

The first result in the paper establishes precisely for which exponents and data we have such phenomena of blow-up or grow-up. We prove that the exponents are the same as for the case $a(x) = 1$.

Theorem 1.1.

1). If $0 < p\le p_0 = 1$ all the solutions to problem (1.1) are globally defined and unbounded.

2). If $1 < p\le p_c = m+2$ all the solutions blow up in finite time.

3). If $p > m+2$ solutions may blow up in finite time or not depending on the initial data. Global solutions are bounded.

The second question to deal with is the speed at which the unbounded solutions tend to infinity, both in the grow-up and in the blow-up cases. For global solutions we show that these rates are not the natural ones given by the corresponding no diffusion ODE (2.1). This in fact gives an upper estimate of the grow-up rate by comparison,

We remark that when $p < 1$ the reaction function is not Lipschitz, and uniqueness does not necessarily hold, neither comparison, see ^[1,12]. In that case we can use for comparison a maximal solution or a minimal solution, ^[12].

In the case of global reaction $a(x) = 1$, it is proved in ^[1,11] that the above is indeed the grow-up rate when $0 < p < 1$, that is

uniformly in compact sets. By $f\sim g$ we mean $0 < c_1\le f/g\le c_2 < \infty$.

However, for $p = 1$ it is well known, through and easy change of variables that eliminates the reaction, that $u(x, t)\sim t^{-1/2}e^{t}$ if $m = 1$ and

when $m\neq1$, for $t$ large uniformly in compact sets of $\mathbb{R}$, see ^[15].

On the other hand, when $a(x) = \mathbb{1}_{(-L, L)}$ it is proved in ^[3] that estimate (1.2) is far from being sharp in most of the cases. In particular

uniformly in compact sets in the first case, only for $|x| < L$ in the last two cases. For $|x| > L$ the rate is different in the case $p > m$, namely

In the limit case of linear diffusion and linear reaction, $m = p = 1$, it holds

where $\omega = \omega(L)\in(0, 1)$, $\lim_{L\to\infty}\omega(L) = 1$.

For our problem (1.1) we show that the rate is the same as for global reaction only if $p\le m$ with $p < 1$ or $p = m = 1$; it is the same as for $a(x) = \mathbb{1}_{(-L, L)}$ if $p > m$, and strictly in between of those two problems if $p = 1 < m$. Again the rate is different for $p > m$ inside or outside the support of the reaction coefficient $a(x)$.

Theorem 1.2. Let $u$ be a solution to problem (1.1) with $p = 1$.

1). If $m > 1$ then $u(x, t)\sim e^{\alpha t}$ uniformly in compact sets of $\mathbb{R}$, where $\alpha\in(1/m, 2/(m+1))$ depends on the behaviour of $u_0$ at infinity.

2). If $m = 1$ then $u(x, t)\sim e^t$ uniformly in compact sets of $\mathbb{R}$.

3). If $m < 1$ then $u(x, t)\sim e^t$ uniformly in compact sets of $\mathbb{R}^+$ and $u(x, t)\sim t^{\frac1{1-m}}$ uniformly in compact sets of $\mathbb{R}^-$, provided $u_0(x)\sim |x|^{\frac{-2}{1-m}}\left(\log|x|\right)^{\frac{1}{1-m}}$ for $x\sim -\infty$.

Theorem 1.3. Let $u$ be a solution to problem (1.1) with $p < 1$.

1). If $m\ge p$ then $u(x, t)\sim t^{\frac1{1-p}}$ uniformly in compact sets of $\mathbb{R}$.

2). If $m < p$ then $u(x, t)\sim t^{\frac1{1-p}}$ uniformly in compact sets of $\mathbb{R}^+$ and $u(x, t)\sim t^{\frac1{1-m}}$ uniformly in compact sets of $\mathbb{R}^-$, provided $u_0(x)\sim |x|^{\frac{-2}{1-m}}$ for $x\sim -\infty$.

We show in Table 1 the different grow-up rates. The exponents are

In the case $p > m$ we have two different rates, inside or outside the support of $a(x)$.

As for blow-up, the rate at which the solutions approach infinity in a finite time has been studied for the case of global reaction under different conditions on the initial datum and exponents, with special care in the multidimensional case, see ^[14] and the references therein. For dimension one, as is our situation, any solution with blow-up at time $t = T$ satisfies, for $t$ close to $T$,

For localized reaction $a(x) = \mathbb{1}_{(-L, L)}$ the rates have been established in ^[2,4], giving a different rate depending on $p$ being bigger or smaller than $m$,

In addition the property $\partial_tu\ge0$ is required in the proof of this result.

We prove here for problem (1.1) that the rate is the same as for global reaction, assuming again monotonicity in time $u_t\ge0$, but this is required only above the Fujita exponent, i.e., for $p > m+2$.

Theorem 1.4. Let $u$ be a solution to problem (1.1) with $p > 1$ such that becomes infinity for $t\to T^-$, and assume further that $u_t\ge0$ if $p > m+2$. Then

We end the description of solutions of problem (1.1) by studying the set where the solution tends to infinity, the blow-up set

In the global reaction case it has been proved the three possibilities according to the reaction exponent: single point blow-up, $B(u)$ is a discrete set, if $p > m$; regional blow-up, $B(u)$ is a compact set of positive measure, if $p = m$; and global blow-up, $B(u) = \mathbb{R}$, if $p < m$. See again ^[14]. The same happens for localized reaction $a(x) = \mathbb{1}_{(-L, L)}$, at least for $m > 1$ and symmetric nondecreasing initial values, see ^[4]. In our case we prove that the same happens, and we additionally show where this blow-up set can lie in the case where the blow-up is not the whole line. To do that we assume in the case $p\ge m$ that there exists some point $x_0$ for which the blow-up rate (1.3) holds, i.e.,

Theorem 1.5. Let $u$ be a blow-up solution to problem (1.1), with compactly supported initial datum. Assume also (1.4). We have for the blow-up set $B(u)$:

1). if $p > m$ then $B(u)\subset\mathbb{R}^+$. Moreover if $m > 1$ it is bounded;

2). if $p = m$ then $B(u)$ is bounded with nontrivial measure;

3). if $p < m$ then $B(u) = \mathbb{R}$.

We remark that due to the lack of symmetry in the problem it is not clear the existence of the point assumed in the statement. In general we can prove that $B(u) = [x_1, \infty)$ for some $-\infty\le x_1 < \infty$ if $p < m$, and $B(u)$ is bounded if $p = m$.

Organization of the paper: We characterize the critical exponents, Theorem 1.1, in Sections 2 and 3. The grow-up rates, Theorems 1.2 and 1.3 are proved in Section 4, while the blow-up rates, Theorem 1.4 is proved in Section 5. Finally we devote Section 6 to describe the blow-up sets, Theorem 1.5.

2.   Blow-up versus global existence

We prove in this section that the global existence exponent is $p_0 = 1$. First it is obvious that if $0 < p\le1$ every solution to problem (1.1) is global. Just use comparison with the flat supersolution

Remark 2.1. Though in the case $p < 1$ there is in general no uniqueness, and therefore no comparison (the reaction is not Lipschitz), we always can compare with a supersolution which is a maximal solution of the equation, like the function $U$ in (2.1) is, see ^[12].

In order to complete the proof of the first item in Theorem 1.1 we observe that all the solutions have grow-up if $p\le1$.

Lemma 2.1. Let $u$ be a solution of (1.1). If $p\le1$ then

uniformly in compact sets.

Proof. We only note that this occurs for the solutions to the problem if the reaction is localized in a bounded interval, $a(x) = \mathbb{1}_{(-L, L)}$, see ^[3], and any solution to that problem (translated) is a subsolution to our problem.

We now show that for $p > 1$ there exist solutions that blow up in finite time provided the initial value is large in some sense.

Lemma 2.2. If $p > \max\{m, 1\}$ problem (1.1) has blow-up solutions.

Proof. We observe that $u$ is a supersolution to the Dirichlet problem

for any interval $(A, B)\subset (0, \infty)$. Use then the results in ^[14].

Lemma 2.3. If $1 < p\le m$ there exist blow-up solutions.

Proof. We construct a self-similar subsolution

satisfying $u(0, t) = 0$. The self-similar exponents are given by

and the self-similar profile satisfies

Using $(f^m)'(0) = \mu$ as shooting parameter we claim that there exists some $\mu_0 > 0$ such that the corresponding profile $f_0$ satisfies

for some $\xi_0 > 0$. This gives the desired blow-up subsolution with profile

Then, if $u_0(x) > \underline u(x, 0)$ the solution of (1.1) blows up.

In order to prove the claim we argue by contradiction, assuming that for every large $\mu$ the corresponding profiles $f_\mu$ are positive in $(0, \infty)$. Given any of such profiles with $\mu > 1$ we take $k = \mu^{\frac{p+m}2}$ and consider the function

It satisfies the initial value problem

We define the energy of the system at a point $\xi$ as

Multiplying the equation by $g_k'$ we get that

since $\beta\le0$. Thus,

Also, calculating the minimum of the potential $V$ we have

Since $p > 1$ this implies that there exists two constants $C_1, C_2$ depending on $m$ and $p$ such that

Hence, letting $k\to\infty$ we have that $g_k$ converges uniformly in compact sets to a non negative function $G$. It is clear that $G$ satisfies

However the solution of the above problem crosses the axis at some finite point with non-zero slope. This is a contradiction and the claim is proved.

3.   Fujita exponent

In this section we prove that the Fujita exponent is $p_c = m+2$, that is, all solutions blow up if $1 < p\le m+2$, and if $p > m+2$ not all solutions do so. In this last range $p > m+2$, it is easy to see that small initial data produce global solutions, by comparison with the global supersolutions corresponding to the case $a(x) = 1$, see for instance the book ^[14]. In fact they tend to zero for $t\to\infty$.

We divide the proof of blow-up below $p_c$ in three cases, $1 < p\le m$, $m < p < m+2$ and $p = m+2$, the most difficult case being the last one.

Lemma 3.1. If $1 < p\le m$ then all solutions blow up in finite time.

Proof. We only have to check that the self-similar subsolution constructed in Lemma 2.3 can be put below any solution if we let pass enough time.

$1).$ It is clear when $p < m$ that we can do it since $\underline u(x, 0)$ is small taking $T$ large, as well as its support is small, due to the fact that $\beta < 0$.

$2).$ For $p = m$ we note that $\underline u(x, 0)$ is still small if $T$ is large but it has a fixed support $[0, \xi_0]$ since $\beta = 0$. Nevertheless, using the penetration property of the solutions of the porous medium equation we obtain that there exists $t_0 > 0$ such that the support of $u(\cdot, t_0)$ contains any interval.

Lemma 3.2. If $m < p < m+2$ then all solutions blow up in finite time.

Proof. The proof is the same as for the global reaction and is an easy consequence of the energy argument of ^[10], also called concavity argument. In fact, defining the energy of a function $v$ as

we have that if for a solution $u$ to (1.1) there exists some $t_0$ such that $E_u(t_0) < 0$ then $u$ blows up in finite time. Now we consider the Barenblatt function

where $k = \frac{m-1}{2m(m+1)}$, $D > 0$. It is a subsolution to our equation and it satisfies, for some constants $c_1, c_2$ depending only on $m$, $p$ and $D$,

which is negative for $t$ large provided $p < m+2$. The final step is a standard comparison argument: we make $B(x, 1;D)$ small by taking $D$ small, so that it can be put below $u_0$; this implies $u(x, t)\ge B(x, t+1;D)$ for $t > 0$; let $t_1$ be such that $E_B(t_1) < 0$; let $v$ be the solution corresponding to the initial value $B(\cdot, t_1;D)$, which by the above energy argument blows up in finite time; since $u\ge v$ so does $u$. In the case $m < 1$ we need the behaviour at infinity of every solution, see ^[9], since the function (3.2) is positive, while for $m = 1$ a Gaussian is used instead of a Barenblatt function.

We observe that the fact that the integral in the reaction term is performed only in $(0, \infty)$ does not affect the original argument. In ^[4] we used the fact that the integral in $(0, L)$ produces a different time power term if $L$ is finite, and so the Fujita exponent is different in that case.

Lemma 3.3. If $p = m+2$ then all solutions blow up in finite time.

Proof. We use the method introduced in ^[7] to prove blow-up for the critical exponent in the case $a(x) = 1$, but here the nonsymmetry of the problem makes things more involved. The argument goes like this: assuming by contradiction that the solution is global, we rescale and pass to the limit in time, thus obtaining a solution to some problem for which we prove nonexistence.

Let $u$ be a global solution, and let $t_0\ge1$ and $D$ be such that $u(x, t_0)\ge B(x, t_0;D)$, where $B$ is given by (3.2) (if $m\ne1$, for $m = 1$ we use instead a Gaussian like in the proof of Lemma 3.2). We define the rescaled function

We have that $v$ is a solution, for $\tau > \tau_0 = \log t_0$, of the equation

If $g$ is the solution to Eq (3.3) with $g(\xi, \tau_0) = B(\xi, 1;D)$, by comparison we have that $v\ge g$ for every $\tau > \tau_0$, and in particular $g$ is globally defined in $\tau$. For the special form of the initial value, it is easy to see that $g$ is nondecreasing in $\tau$, and therefore there exists the limit

We claim the following alternative:

$a)$ $f$ is locally bounded. Thus we can pass to the limit in (3.3), by means of a Lyapunov functional, to get that $f$ is a positive solution of

see ^[7]. Now we observe that the function

satisfies $\mathcal{E}'(\xi) = -\rho(\xi)f^{m+2}(\xi)$, so it is constant for $\xi < 0$ and decreasing for $\xi > 0$. Then, if we assume $\mathcal E(0) = \mathcal E_0 > 0$, we have that

This implies that there exists a point $\xi_1 < 0$ such that $f(\xi_1) = 0$ and $(f^m)'(\xi_1)\ne0$. Therefore $\mathcal E(0)\le 0$, and there exists some $\xi_2 > 0$ such that $\mathcal E(\xi_2) = \mathcal E_2 < 0$. Exactly as before

so there exists a point $\xi_3 > \xi_2$ such that $f(\xi_3) = 0$, $(f^m)'(\xi_3)\ne0$. This gives a contradiction and $f$ cannot exist.

$b)$ There exists $\xi_0$ such that $f(\xi_0) = \infty$. Then $g$ is large in a nontrivial interval and this would imply that it blows up in a finite time. This is again a contradiction, and the theorem would be proved.

We have that $f$ satisfies Eq (3.4) in any interval in which it is bounded. It is clear that $f$ cannot have any minima since at such a point we would have from the equation $(f^m)'' < 0$. This implies

Assume $\xi_0 > 0$. If $f$ is bounded in some interval $(\xi_0-\delta, \xi_0)$, $\delta\le\xi_0$, then $f$ is increasing in that interval with

This is a contradiction and thus $f(\xi) = \infty$ for every $0\le\xi\le\xi_0$. Moreover, if $f$ is bounded in $\xi < 0$, we have

by the above. Thus by (3.5), there is a sequence $\xi_j\to0^-$ such that $|\xi_j|f(\xi_j)\to\infty$. The same argument works from the left to the right, assuming $\xi_0 < 0$. In conclusion $f$ is large in some interval $|\xi|\le\xi_*$, that could be small, but it satisfies that $\xi_*f(\xi_*)$ is large.

Let us now show that in this situation the function $g$ blows up in finite time. By the monotonicity of $g$ in time we have that for any large constant $A_* > 0$ there exists $M > 0$, $\xi_M > 0$ and $\tau_M$ such that $M\xi_M \ge A_*^{3/2}$ and $g(\xi, \tau)\ge M$ for every $|\xi|\le \xi_M$, $\tau\ge\tau_M$. Now we argue as in ^[4]. Let $z(x, t) = e^{-\alpha\tau} g(\xi, \tau)$ be the function $g$ in the original variables, and define $h(x, t+e^{\tau_M})$ the solution of (1.1) with initial datum

where

It is clear that $W(x)\le z(x, e^{\tau_M})$, since

Moreover,

for some $c_1, c_2$ depending only on $m$. This is negative for $A > A_* = A_*(m)$. Thus $h$ blows up in finite time, and by comparison $z$, or which is the same $g$, also blows up. This ends the proof.

4.   Grow-up rates

The aim of this section is to study the speed at which the global unbounded (grow-up) solutions to problem (1.1) tend to infinity. We therefore consider the range $p\le1$. In order to avoid nonuniqueness issues when $p < 1$ we assume in that case that the initial value is positive for $x > 0$, that is where the non-Lipschitz reaction applies.

As we have said in the Introduction, the upper estimate of the grow-up rate is given by comparison with the function in (2.1). In the case of global reaction $a(x) = 1$ this is sharp if $p < 1$ or $m < 1$. In fact we have for $t$ large

see ^[1,11,15].

On the other hand, when $a(x) = \mathbb{1}_{(-L, L)}$ the rates are proved in ^[3]. Though in that situation the global existence exponent is different, $p_0 = \max\{1, \frac{m+1}2\}$, we quote the results proved in ^[3] in our range $p\le1$:

$i)$ if $p\le1 < m$ then

in compact sets.

$ii)$ if $m < p < 1$ then

provided that the initial datum satisfies

$iii)$ if $m < p = 1$ then

provided that the initial datum satisfies

$iv)$ if $p = m = 1$ then

We prove in this paper that for problem (1.1) the rate can be that corresponding to global reaction or to reaction localized in a bounded interval, or none of them, depending on the sign of $p-m$. We can also have a different rate inside or outside the region where the reaction applies when $p > m$, like in the case $a(x) = \mathbb{1}_{(-L, L)}$.

4.1.   $\underline{ Case \ p= 1 }$

Though the reaction is linear this is the more involved case. We consider separately the three cases according to $m$ being larger, equal or smaller than 1.

The proof of the grow-up rate follows by comparison with special selfsimilar subsolutions and supersolutions. We construct such functions in the form

where necessarily

Also, by (4.1) we consider only $\alpha\le 2/(m+1)$.

The profile $f$ will be given by matching two functions,

where $\psi$ and $\phi$ are the truncation by zero of the solutions of the initial value problems, for some $\lambda\in\mathbb{R}$,

We start with $m > 1 = p$.

4.1.1.   $\underline{ \text{Slow } \ \text{diffusion} , m > 1 }$

The existence of solutions with compact support for equations of the above type has been studied in ^[8]. Let us consider, as in that paper, the problem for some $\xi_0 > 0$ given,

It is proved in ^[8],

Theorem 4.1. Let $\beta > 0$. There exists a continuous solution $g$ to problem (4.7) such that $g(0) > 0$ for $2\beta+q > 0$; $g(0) = 0$ for $2\beta+q = 0$; and if $2\beta+q < 0$ there exists a point $\xi_1\in(0, \xi_0)$ with $g(\xi_1) = 0$. Moreover, in the first case, $g'(0) < 0$ if $\beta+q > 0$; $g'(0) = 0$ if $\beta+q = 0$; and $g'(0) > 0$ if $\beta+q > 0$. Finally

Translating this result to our problems (4.5) and (4.6), where $q$ takes the values, respectively, $q = \alpha-1 < 0$ and $q = \alpha > 0$, we obtain the following results.

Corollary 4.2.

1). For each $\alpha > 0$ there exists a unique $\lambda_-(\alpha) > 0$ such that problem (4.6) with $\lambda = \lambda_-(\alpha)$ has a decreasing solution with compact support.

2). Problem (4.5) has solutions with compact support for some $\lambda$ if and only if $\alpha > 1/m$, the solution being unique for each $\alpha$ given, and thus $\lambda = \lambda_+(\alpha)$. Moreover, $\lambda_+(\alpha) > 0$ if $\alpha < 2/(m+1)$; and $\lambda_+(2/(m+1)) = 0$.

If we find some $\alpha\in(1/m, 2/(m+1))$ such that $\lambda_-(\alpha) = \lambda_+(\alpha)$, we will obtain a solution $w$ with frofile $f$ defined in $\mathbb{R}$ which has compact support. But we are also interested in subsolutions, and these are obtained constructing profiles with compact support $[-a, b]$ with a bad behaviour at the interfaces $(f^m)'(-a) > 0$, $(f^m)'(b) < 0$. On the other hand, positive profiles will serve as supersolutions.

Thus, in order to study in more detail the solutions to the equation in (4.7) we introduce the variables

We also fix the value $g(0) = 1$ and consider the different values of $g'(0)$. We obtain the differential system,

defined in the half-plane $Y\ge0$, where $\dot{X} = dX/d\eta$. As we have said only the values $q = \alpha$ and $q = \alpha-1$ are of interest, with $\alpha\in(1/m, 2/(m+1))$. We have two finite critical points

(if $q = \alpha-1$ there exists a third critical point but it lies in the lower half-plane), and three critical points at infinity

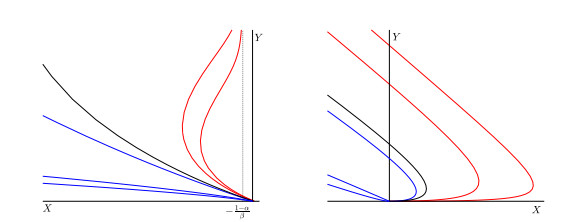
The point $P_1$ is an unstable node: we have a trajectory $\Gamma_0$ escaping this point from $\eta = -\infty$ along the vector $(q, 1)$, and a family of trajectories $\Gamma_\kappa$, $\kappa\neq0$, behaving near the origin like

The first one produces a profile $g$ with $g'(0) = 0$. The profile corresponding to each $\Gamma_\kappa$ satisfies $g'(0) = \kappa/\sqrt m$. The point $P_2$ is a saddle and plays no role at this stage.

Now fix $q = \alpha-1 < 0$. We first observe that defining the energy associated to the problem

it satisfies

Therefore $g$ is bounded, and all the trajectories starting at $P_1$ must go to one of the points at infinity $\Lambda_1$ or $\Lambda_2$. In fact $\Lambda_3$ is unstable.

The profiles satisfying (4.8) correspond to trajectories entering the point $\Lambda_1$ linearly, since they satisfy

Using the equation

we get that the only possible behaviours near $\Lambda_1$ are

Thus from Corollary 4.2 we get that the trajectory $\Gamma_{\kappa^+}$ with $\kappa^+ = \lambda_+(\alpha)\sqrt m$ joins $P_1$ with $\Lambda_1$ satisfying $Y\sim-DX$ near $\Lambda_1$, and it is the unique trajectory with that behaviour at infinity. All the other trajectories joining these two points enter $\Lambda_1$ below $\Gamma_{\kappa^+}$, and above this trajectory near the origin. See Figure 1.

This implies that the corresponding profiles have slopes at the origin $g'(0) < \lambda_+(\alpha)$. Observe that this implies that $g$ vanishes at some point $b < \infty$ with $g(\xi)\sim(b-\xi)^{\frac1m}$, so $(g^m)'(b) < 0$.

On the other hand, the trajectories with $\kappa > \kappa^+$ must go to $\Lambda_2$. The corresponding profiles are positive with

for $\xi$ large.

In summary we have proved the following result.

Lemma 4.3. Let $\psi$ be a solution of (4.5) with some $\alpha\in(1/m, 2/(m+1))$. There exists some $\lambda_+ = \lambda_+(\alpha) > 0$ such that

1). If $\lambda < \lambda_+$ there exists $\xi_0 < \infty$ such that $\psi(\xi_0) = 0 > (\psi^m)'(\xi_0)$.

2). If $\lambda = \lambda_+$ there exists $\xi_0 < \infty$ such that $\psi(\xi_0) = (\psi^m)'(\xi_0) = 0$.

3). If $\lambda > \lambda_+$ the solution $\psi$ is positive and $\psi\sim < /italic > < italic > \xi^{-\frac{1-\alpha}\beta}$ for $\xi$ large.

We comment by passing what is the behaviour in the case $\alpha = 1/m$. If we trace back the unique trajectory entering $\Lambda_1$ linearly, we see that it goes to $P_2$. In fact integrating the equation between $\xi$ and $a$ we get

A second integration gives

Then $\lim_{\xi\to0} Y(\xi) = 0$ trivially, while

Therefore all the trajectories starting at $P_1$ must go to $\Lambda_1$ like $Y\sim |X|^{\frac{m-1}m}$. We obtain profiles with a bad interface behaviour for every value of $\lambda$.

The phase-space (4.9) is studied in the same way in the case $q = \alpha > 0$. Following the same argument for problem (4.6) we obtain:

Lemma 4.4. Let $\phi$ be a solution of (4.6) with some $\alpha\in(0, 2/(m+1))$. There exist $\lambda_- = \lambda_-(\alpha) > 0$ such that:

1). If $\lambda > \lambda_-$ there exists $\xi_1 < \infty$ such that $\phi(\xi_1) = 0 > (\phi^m)'(\xi_1)$.

2). If $\lambda = \lambda_-$ there exists $\xi_1 < \infty$ such that $\phi(\xi_1) = (\phi^m)'(\xi_1) = 0$.

3). If $\lambda < \lambda_-$ the solution $\phi$ is positive and unbounded.

Moreover $\lambda_-(\alpha) = K /\sqrt{\alpha}$.

We now study the matching.

Lemma 4.5. There exists a unique value $\alpha_*\in(1/m, 2/(m+1))$ such that $\lambda_-(\alpha_*) = \lambda_+(\alpha_*)$.

Proof. Define the continuous function $h(\alpha) = \lambda_+(\alpha)-\lambda_-(\alpha)$. It is clear that $h(2/(m+1)) < 0$. On the other hand, taking $\lambda = \lambda_-(1/m)$ in (4.6) and (4.5) we obtain a profile which crosses the axis at some positive point with bad interface behaviour. Therefore by continuous dependence of the profile with respect to the parameter $\alpha$ we have the same behaviour for $\alpha = \varepsilon+1/m$. This implies $h(\varepsilon+1/m) > 0$. Then there exists $\alpha_*\in(1/m, 2/(m+1))$ with $h(\alpha_*) = 0$. The uniqueness follows by comparison. Indeed, if we assume that $h(\alpha_1) = 0 = h(\alpha_2)$ with $\alpha_1 < \alpha_2$ we have that the solutions $w_1$ with profile $f_1, f_2$ given in (4.4) with $\alpha = \alpha_1, \alpha_2$ satisfy

for some $t_1 > 0$. This implies $w_1(x, t)\ge w_2(x, t-t_1)$ for any $t > 0$. In particular at $x = 0$ this means $f_1(0)e^{\alpha_1 t}\ge f_2(0)e^{\alpha_2 (t-t_1)}$, which is impossible if $t$ is large.

Theorem 4.6. Let $u$ be the solution to problem (1.1) with $p = 1 < m$. Let $\alpha_*$ be given in Lemma 4.5 and define $\gamma_* = \frac{2(1-\alpha_*)}{(m-1)\alpha_*}$.

1). If there exists some $1 < \gamma < \gamma_*$ such that $u_0(x) \sim x^{-\gamma}$ as $x\to\infty$, then

where

2). If $\limsup_{x\to\infty}x^{-\gamma_*}u_0(x) < \infty$, then for all $\varepsilon > 0$

3). If $u_0$ has support bounded from the right then

The above estimates are uniform in compact subsets of $\mathbb R$ for $t$ large.

Proof. The proof follows by comparison with the self-similar functions constructed before. We define:

$\bullet$ $w_*$ the self-similar function given in (4.3) with $\alpha = \alpha_*$. It is a solution to (1.1) with compact support

$\bullet$ For $\alpha\in(\alpha_*, 2/(m+1))$, which implies $\lambda_+(\alpha) < \lambda_-(\alpha)$, we consider $w_-$ the self-similar function given in (4.3) with $\lambda = \lambda_-(\alpha)$. It is a solution to (1.1) with support bounded from the left

In this case, we also consider $w_\lambda$ the self-similar function given in (4.3) with $\lambda_+(\alpha) < \lambda < \lambda_-(\alpha)$. It is a positive solution to (1.1) such that

We now consider the different cases in the statement of the theorem.

$1).$ $u_0(x)\sim x^{-\gamma}$ with $1 < \gamma < \gamma_*$. Taking $\alpha = \alpha(\gamma)\in(\alpha_*, 2/(m+1))$ we have $\lambda_+(\alpha) < \lambda_-(\alpha)$, and the functions $u_0$, $w_-$ and $w_\lambda$ have the same behaviour at infinity. Then there exists $t_1$ large enough such that

and by comparison

The grow-up rate follows.

$2).$ $u_0(x)\le x^{-\gamma_*}$. The lower bound follows by comparison with $w_*(x, t-t_1)$. For the upper bound we compare with $w_\lambda(x, t+t_1)$ with $\alpha = \alpha_*+\delta$, $\delta > 0$ small.

$3).$ $u_0$ with compact support. We compare from below as in the previous case, and from above with $w_*(x, t+t_1)$.

We observe that for any initial value $u_0$ the grow-up rate is always exponential, like for global reaction $a(x) = 1$, but with an exponent strictly smaller $\alpha\le\alpha_* < 2/(m+1)$. In the case of a localized reaction, $a(x) = \mathbb{1}_{(-L, L)}$, the grow-up was polynomial.

The second case to consider when $p = 1$ is $m = 1$, where things are more or less explicit.

4.1.2.   $\underline{\text{ Linear diffusion } m=1 }$

Lemma 4.7. Let $u$ be the solution to (1.1) with $p = m = 1$. Then,

uniformly in compact subsets of $\mathbb R$.

Proof. The upper estimate is given by comparison with the function in (2.1). For the lower bound we use again comparison, this time with an exponential selfsimilar function, see (4.5), (4.6). Since here $\beta = 0$, we look for a function in separated variables

where the profile $f$ satisfies

This gives

The matching condition at $x = 0$ means

Notice that for any $x_- < 0$ given we can take

so that $f(x_-) = 0$. Moreover, for

we have $f(x) > 0$ in $(0, x_+)$ and $f(x_+) = 0$. This profile gives us a subsolution by the procedure of truncation by zero. We denote by $f_{x_-}$ this truncated profile.

Let now $u$ be a solution of (1.1). Since the heat equation has infinite speed of propagation, we can assume without loss of generality that $u_0(x) > 0$. Then there exists $t_1 > 0$ such that

By comparison we deduce $u(x, t)\ge w_{x_-}(x, t-t_1)$. We obtain the lower grow-up rate for compact subsets of $(-\infty, \frac{\pi}{2\sqrt{1-\alpha}})$ and for every $\alpha < 1$.

Finally we observe that for $A > 0$ the function $w_{x_-}(x-A, t-t_1)$ is also a subsolution to (1.1), so we obtain the lower grow-up rate for any compact subset of $\mathbb R$.

We end by considering the case $m < 1 = p$.

4.1.3.   $\underline{ \text{Fast diffusion } m < 1 }$

Here the rate is different for $x > 0$ and for $x < 0$, as in the case of a localized reaction, $a(x) = \mathbb{1}_{(-L, L)}$.

We first show that the grow-up rate given in (4.1) is sharp for compact subsets of $\mathbb R^+$ by proving the lower bound. To do that we compare with a subsolution in separated variables with compact support in $\mathbb R^+$,

Notice that since $u$ has global grow-up, see Lemma 2.1, we have that $u(x, t_0)\ge \underline u(x, 0)$ for $t_0$ large enough, so then the comparison of the initial data is granted by a time shift.

Lemma 4.8. Let $u$ be a solution of (1.1) with $m < 1 = p$. Then,

uniformly in compact subsets of $\mathbb R^+$.

Proof. Let $\phi$ be the solution to the problem

Since $\phi^m$ is concave $\phi$ must vanish at some point $\xi_0 < \infty$. Now we consider the rescaled function

which satisfies the same equation and vanishes at $x = \xi_0A^{-\frac{1-m}2}$. This is the spatial part of our subsolution. The time part $g$ is defined as the solution to

We have $u(x, t+t_0)\ge f(x)g(t)$ for any $x > 0$ and $t > 0$. Since $g'\sim g$ as $t\to\infty$, the comparison gives the desired lower bound.

In order to obtain the grow-up rate for $\mathbb R^-$, we note that by (4.1) $u$ is a subsolution of the problem

It is proved in ^[3] that there exists a unique self-similar solution of exponencial type

which is increasing in both variables $x$ and $t$. Moreover, for $|\xi|$ large

Then, if the initial datum satisfies

we can take as a supersolution $\overline w (x, t) = A W(x, t)$. Notice that from the property $W_t\ge0$ we have

provided $A > 1$. Moreover, taking $A$ large enough we get $\overline w(x, 0)\ge u_0(x)$.

On the other hand, by Lemma 4.8 we have that $u$ is a supersolution to the problem

and $\underline w (x, t) = A W(x, t)$ with $A$ small enough to have $\underline w(x, 0)\le u_0(x)$ is a subsolution.

As a conclusion we get the following result.

Lemma 4.9. Let $u$ be a solution of (1.1) with $m < 1 = p$, such that the initial datum $u_0$ satisfies the condition (4.10). Then, for $x < 0$

4.2.   $\underline{ Case \ p < 1 }$

Here we distinguish between $m < p$ and $m\ge p$.

4.2.1.   $\underline{ \text{Case} \ m < p }$

Lemma 4.10. Let $m < p < 1$. If $u_0$ satisfies (4.2) for $x\sim -\infty$, then

uniformly in compact sets.

Proof. The proof follows in the same way as in the case $p = 1$, using here the selfsimilar profile

constructed in ^[3], which is again increasing in both variables $x$ and $t$, and that satisfies, for $|\xi|$ large,

4.2.2.   $\underline{ \text{Case} \ m\ge p }$

Lemma 4.11. Let $p < 1\le m$. then

uniformly in compact sets of $\mathbb R$.

Proof. We consider a subsolution in selfsimilar form

where

and the selfsimilar profile satisfies

We construct the profile gluing four functions. Let $A > 0$ be a constant to be fixed and put $\xi_0 = -\sqrt{2/\alpha} A^{\frac{m-1}{2m}}$.

$1).$ For $\xi\le\xi_0$ we put $f_1(\xi) = 0$.

$2).$ For $\xi_0\le\xi\le0$ we define

Notice that $f^m_2(\xi_0) = (f_2^m)'(\xi_0) = 0$. Moreover since $\beta\le0$ and $f_2$ is non-decreasing

$3).$ For $0\le \xi\le \xi_1 = \sqrt{2\alpha} A^{\frac{1+m-2p}{2m}}$ we define

We have $f_3^m(0) = f_2^m(0) = A$, $(f_3^m)'(0) = (f_2^m)'(0)$, so this function $f_3$ matches well with $f_2$. Also $f_3$ is increasing in $0 < \xi < \xi_1$, with

Since $p < 1$ we get that for $A$ small enough, both $\xi_1$ and $f_3(\xi_1)$ are small. Hence, the function $f_3^p(\xi)-\alpha f_3(\xi)$ is increasing. Then

$4).$ For $\xi > \xi_1$ we consider $f_4 = g_+$, where $g$ is the solution to the initial value problem

It is clear that if $f_3(\xi_1) < (1/\alpha)^\alpha$ then $g$ is nonincresing and positive for $\xi_1\le\xi < \xi_2\le\infty$.

The final function putting together $f_i$, $i = 1, \cdots, 4$ is a subsolution to our problem with zero initial value. This gives the desired lower bound of the grow-up rate.

5.   Blow-up rates

Proof of Theorem 1.4. The lower blow-up rate is obtained easily by (strict) comparison with the supersolution

Indeed, if we assume that there exists $t_0\in(0, T)$ such that

it also holds

for some $\varepsilon > 0$, which is a contradiction with the fact that $u$ blows up at time $T$.

In order to prove the upper blow-up rate we use a rescaling technique inspired in the work ^[6].

Let us define

and consider, for any fixed $t_0\in(0, T)$, the increasing sequence of times

Observe that for this sequence we have $\|u(\cdot, t_j)\|_\infty = M(t_j)$. We also observe that since the reaction only takes place for $x > 0$, we get that near the blow-up time the maximum of $u$ is achieved in $\mathbb R^+$. Therefore we can take $x_j\ge 0$ such that

We consider the sequence

Let us observe that if $z_j$ is bounded, we get that

Performing the sum,

that is, the desired upper blow-up rate. Therefore, in order to arrive at a contradiction, we assume that there exists a subsequence of times, still denoted $t_j$, such that

Now we define the functions

for

where $M_j = M(t_j)$. Notice that $I_j\to \mathbb R$ as $j\to\infty$ and $\varphi_j$ is a solution to the equation

It also satisfies

The uniform bounds for $\varphi_j$ impliy that $\varphi_j$ is Hölder continuous with uniform coefficient. Since $\varphi_j(0, 0) = 1$ we have a uniform nontrivial lower bound for every $\varphi_j$, that is,

for some nontrivial function $g$.

We claim that, under the assumption of Theorem 1.4, each function $\varphi_j$ blows up at a finite time $S_j$ which is uniformly bounded, that is $S_j < S$. This is a contradiction with the fact that $\varphi_j(y, s)\le 2$ for $s\in (0, z_j)$. Indeed, since $z_j\to\infty$ we can take $j$ large such that $z_j > S$. Therefore (5.1) can not be true and the blow-up rate follows.

In order to prove the claim we first observe that since $x_j\ge0$, we have that $\varphi_j$ is a supersolution of the equation

Therefore, for $p\le m+2$, we can apply Theorem 1.1 to get that the solution of the above equation with initial datum $h(y, 0) = g(y)$ blows up at some time $S$. Then, by comparison, $\varphi_j$ blows up at time $S_j < S$.

For the case $p > m+2$ we need the extra hypothesis $u_t\ge0$, which implies $(\varphi_j)_s\ge0$. Therefore $\varphi_j$ is a supersolution of the problem

Since $w\le 1$ we can pass to the limit, by means of a Lyapunov functional, to get that $w(x, s)\to 1$ as $s\to\infty$ uniformly in compact sets of $\mathbb R^+$. Actually in the linear case $m = 1$ the solution to problem (5.3) is explicit, while if $m\neq1$ the solution is the so-called Polubarinova-Kochina solution. Notice that this behaviour is also true if we consider the problem in $\mathbb R^-$. Therefore, by comparison $\varphi_j(y, s)\ge 1/2$ in $|y| < K$ and $s > s_K$, and then

Observe that the energy of $h_0$ given in (3.1) satisfies

for $K$ large. Then, applying the concavity argument the solution of (5.2) with initial datum $h_0$ blows up at finite time $S$ and by comparison $\varphi_j$ also blows up at a time $S_j < S$.

6.   Blow-up sets

We prove here Theorem 1.5. We first consider the case $p\ge m$.

Lemma 6.1. Let $u$ be a blow-up solution to (1.1) with compactly supported initial datum. Then $B(u)$ is bounded from the left. In fact,

1). $B(u)\subset \mathbb R^+$ if $p > m$;

2). $B(u)\subset [-K, \infty)$ if $p = m$.

Proof. Notice that by the upper blow-up rate, $u$ is a subsolution to the problem on the left half-line

provided that $C$ is large. For $m = 1$ we have and explicit formula for $w$, and it is easy to see that $w$ is bounded for $x < 0$, see for instance ^[14]. For $m\ne1$ we use comparison with a selfsimilar solution in the form

where the profile $F$ satisfies the equation

Observe that for $p = m$, i.e., $\beta = 0$, this equation is the same as for problem (4.6), so by Lemma 4.4 there exists a profile $F_1$ with compact support and satisfying $F_1(0) = 1$. By scaling $F(\xi) = CF(C^{\frac{1-m}2}\xi)$ is also a solution, with large support if $C$ is large. We then take $C$ large so as to have that the corresponding solution $W$ satisfies $W(x, 0)\ge u_0(x)$ and by comparison we obtain the bound of $B(u)$.

For the case $p > m$ ($\beta > 0$), we introduce as in Seccion 4.1.1 the variables (4.9),

to obtain the differential system,

It is easy to see that all the orbits in the second quadrant start at the origin and have three posible behaviours: they cross the vertical axis; or the horizontal variable goes to $-\infty$; or $(X, Y)\to (-\alpha/\beta, \infty)$. The existence of a unique orbit joining the origin with $(-\alpha/\beta, \infty)$ is given in ^[5] for $m < 1$, but the argument works as well for $m > 1$. From this orbit we obtain a positive, increasing profile $F_1$ such that $F_1(0) = 1$ and $F_1(\xi)\sim |\xi|^{-\alpha/\beta}$ for $\xi\sim -\infty$. Notice that, for $x < 0$ and $t$ near $T$, we have

that is $W$ is bounded. Rescaling and comparison as before implies the same property for our solution, $u$ is bounded for $x < 0$.

Remark 6.1. Notice that for $p < m$ we have again an equation like (4.6) for the profile. This gives us a family of selfsimilar solutions

with global blow-up.

Lemma 6.2. Let $u$ be a blow-up solution to (1.1) with compactly supported initial datum. Then $B(u)$ is bounded from the right provided $p\ge m > 1$.

Proof. We only have to notice that the support of the blow-up solutions to the equation with global reaction, $a(x) = 1$, are bounded if $p\ge m > 1$, see ^[14]. The proof of this result uses the intersection comparison technique with self-similar profiles in a neighborhood of the free boundary. Then the same result holds for our equation near the right-hand free boundary.

Lemma 6.3. Let $u$ be a blow-up solution to (1.1) with $p < m$ and a compactly supported initial datum. Assume also that there exists $x_0\in\mathbb R$ satisfying (1.4). Then $B(u) = \mathbb R$.

Proof. Thanks to the hypothesis at $x_0$ we have that $u$ is a supersolution to the problem defined on the left of $x_0$,

as well as to the problem on the right, $x > x_0$. Moreover, the self-similar solution given in Remark 6.1 is a subsolution if we choose $C$ small enough such that $W_C(x, 0) < u_0(x)$. Comparison ends the proof.

The same argument allows to prove that if $p = m$ the blow-up set contains some nontrivial interval, thus concluding the proof of Theorem 1.5.

To finish this section we remark that, in the range $p < m$, but without the hypothesis of the existence of $x_0$, it is easy to see that $B(u)$ is unbounded at least from the right. In fact if we assume that at some point $u(x_1, t) < M$, then $u$ is a subsolution to

On the other hand, the stationary solution of the equation with $z(0) = K$ and $z'(0) = 0$, is a supersolution of the problem if $K$ is large enough. Then by comparison $u$ must be bounded in $(-\infty, x_1)$. Therefore if $u$ is bounded in some interval $(x_1, \infty)$ then it cannot blow up.

Acknowledgments

Work supported by the Spanish project MTM2017-87596. The first author was also supported by Grupo de Investigación UCM 920894.

Conflict of interest

The authors declare no conflict of interest.