Fixed point for an $\mathbb{O}g\mathfrak{F}$-c in $\mathbb{O}$-complete $\mathfrak{b}$-metric-like spaces

1.   Introduction

As one of the generalizations of metric space, by introducing the conception of metric-like space in 2000, Hitzler ^[1] gives a valuable contribution to fixed point theory, permitting self-distance to be nonzero, as that can not be possible in metric space. During his studies at the time, he explored the metric-like space under the name "dislocated metric space." Amini-Harandi ^[2] was the one who renamed the dislocated metric space as metric-like space. Several researchers developed the concept of metric space in many types see ^[3,4,5,6,7].

In 2017, Gordji et al. ^[8] introduced the concept of an orthogonality and presented several fixed-point theorems in an orthogonal metric space. Furthermore, Gordji and Habibi extended more results in generalized orthogonal metric space and $\epsilon$-connected orthogonal metric space; see ^[9,10]. Hamid Baghain et al. ^[11] proved fixed-point theorem in orthogonal space via orthogonal $F$-contraction. In 2018, Senapati ^[12] initiated the concept of w-distance and proved fixed point results in orthogonal metric space. In 2018, Yamaod et al. ^[13] came up with the concept of s-orthogonal contraction in b-metric space. In 2019, Gungor et al. ^[14] changed the distance functions to show more results in orthogonal metric space. Sawangsup and Sintunavarat extended this to an orthogonal concept in O-complete metric space, see ^[15,16]. The notion of multivalued orthogonal $(\tau, F_T)$-contraction in O-complete orthogonal metric space was introduced by Sumit Chandok et al. ^[17]. Also, Ismat Beg et al. ^[18] extended the notion of a generalized orthogonal F-Suzuki contraction mapping in O-complete b-metric space. The notion of F-contraction introduced by Wardowski ^[19] who has proved a fixed point theorem in generalized Banach contraction principle.

This article, introduces some new concepts of an $\mathbb{O}$-generalized $\mathfrak{F}$-contraction and proves fixed point theorems such as new $\mathfrak{F}$-contractions in $\mathfrak{b}$-metric-like space. Our results primarily generalize and improve the related results in the literature. Moreover, an example and application to the integral equation are given to exhibit the utility of the obtained results.

Definition 1.1. ^[19] Let $(\mathcal{H}, \wp)$ be a metric space. A self mapping $\mathcal{P}$ on $\mathcal{H}$ is said to be a $\mathfrak{F}$-contraction if $\varrho > 0$ exists such that

where $\mathfrak{F}: [0, \infty) \to \mathbb{R}$ is a map which holds the following axioms:

$(\mathfrak{F}_{1})\; \mathfrak{F}$ is strictly increasing; that is, for all $\xi, \eta \in [0, \infty) \; \text{such that}\; \xi < \eta, \, \, \mathfrak{F}(\xi) < \mathfrak{F}(\eta);$

$(\mathfrak{F}_{2})$ for every sequence $\{ \xi_{n} \}$ of non-negative numbers,

$(\mathfrak{F}_{3})$ there exists $s \in [0, 1]$ such that $\lim\limits_{\xi \to 0^+} {\xi^s}\mathfrak{F}(\xi) = 0$.

Let us remember from ^[2], some facts and definitions about $\mathfrak{b}$-metric-like space.

Definition 1.2. ^[2] A nonempty set $\mathcal{H}$ and a function $\wp :\mathcal{H} \times \mathcal{H} \to [0, \infty)$ satisfies the following conditions holds for all $\mathfrak{h}, \mathfrak{k}, \mathfrak{l} \in \mathcal{H}$ and a constant $\mathfrak{s} > 1$:

($\wp_1)$ If $\wp(\mathfrak{h}, \mathfrak{k}) = 0$ then $\mathfrak{h} = \mathfrak{k}$;

$(\wp_2)\; \wp(\mathfrak{h}, \mathfrak{k}) = \wp(\mathfrak{k}, \mathfrak{h})$;

$(\wp_3)\; \wp(\mathfrak{h}, \mathfrak{l}) \leq \mathfrak{s}(\wp(\mathfrak{h}, \mathfrak{k})+\wp(\mathfrak{k}, \mathfrak{l}))$.

The pair of $(\mathcal{H}, \wp)$ is called a $\mathfrak{b}$-metric-like space.

Example 1.3. ^[7] Let $\mathcal{H} = \mathbb{R}$. Define a mapping $\wp : \mathbb{R} \times \mathbb{R} \rightarrow [0, \infty)$ by

for all $\mathfrak{h}, \mathfrak{k} \in \mathbb{R}$. Then $(\mathbb{R}, \wp)$ is a $\mathfrak{b}$-metric-like space with the coefficient $\mathfrak{s} = 2$.

efinition 1.4. ^[2] Each $\mathfrak{b}$-metric-like $\wp$ on $\mathcal{H}$ generalizes a topology $\varrho_{\wp}$ on $\mathcal{H}$ whose base is the family of open $\wp$-balls $\mathfrak{B}_{\wp}(\mathfrak{h}, \delta) = \{\mathfrak{k} \in \mathcal{H}: |\wp(\mathfrak{h}, \mathfrak{k}) - \wp(\mathfrak{h}, \mathfrak{h})| < \delta\}$ for all $\mathfrak{h} \in \mathcal{H}$ and $\delta > 0$.

efinition 1.5. ^[2] Suppose that $(\mathcal{H}, \wp)$ be a $\mathfrak{b}$-metric-like space. A map $\mathcal{P} :\mathcal{H} \to \mathcal{H}$ is called continuous at $\mathfrak{h} \in \mathcal{H}$, if for every $\epsilon > 0\; \; \; \exists\; \; \delta > 0$ such that $\mathcal{P}(\mathfrak{B}_{\wp}(\mathfrak{h}, \delta))\subseteq \mathfrak{B}_{\wp}(\mathcal{P}\mathfrak{h}, \epsilon).$ We say that $\mathcal{P}$ is continuous on $\mathcal{H}$ if $\mathcal{P}$ is continuous at all $\mathfrak{h} \in \mathcal{H}$.

efinition 1.6. ^[7] Let $(\mathcal{H}, \wp)$ be a $\mathfrak{b}$-metric-like space, $\{ \xi_{n} \}$ be a sequence in $\mathcal{H}$ and $\xi \in \mathcal{H}$. Then a sequence $\{ \xi_{n} \} \subset \mathcal{H}$ is said to be converge to a point $\xi \in \mathcal{H}$ if, for every $\epsilon > 0$ there exists $n_{0} \in \mathbb{N}$ such that $\wp(\xi_{n}, \xi) < \epsilon$ for all $n > n_{0}$. The convergence is also represented as

2.   Orthogonal metric spaces

The concept of an orthogonality was introduced by Gordji, Ramezani, De La Sen and Cho ^[8] as follows:

Definition 2.1. ^[8] Let $\mathcal{H} \neq \phi$ and $\perp \subseteq \mathcal{H}\times \mathcal{H}$ be a binary relation. If $\perp$ satisfies the following condition:

then $(\mathcal{H}, \perp)$ is called an $\mathbb{O}$-set.

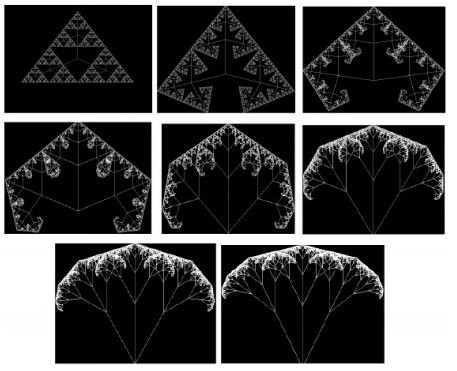
Example 2.2. ^[8] Let us make a famous fractal called the Sierpinski Triangle.

Sierpinski's triangle starts as a shaded triangle of equal lengths in page $\mathbb{R} \times \mathbb{R}$ with vertices (-1, 0), (1, 0) and (0, $\sqrt{3}$). We split the triangle into four same triangles by connecting the centers of each side together and remove this central triangle. We then repeat this process on the $3$ newly created smaller triangles. This process is repeated several times on each newly created smaller triangle to arrive at the displayed picture. A Sierpinski's triangle is created by infinitely repeating this construction process.

Let $\mathcal{H}$ be the set of all (infinite) removed triangles. Define the binary relation $\perp$ on $\mathcal{H}$ by $a\perp b$, for all $a, b \in \mathcal{H}$ if there exists $a \in \mathcal{H}$, $\{\mathfrak{k}: (\mathfrak{h}, \mathfrak{k}) \in a \; \text{for some}\; \mathfrak{h} \in \mathbb{R}\}$ give to $\{\mathfrak{k} : (\mathfrak{h}, \mathfrak{k}) \in b \; \text{for some}\; \mathfrak{h} \in \mathbb{R}\}$. According to Figure 1 if $\{\mathfrak{k}_{0}: (\mathfrak{h}_{0}, \mathfrak{k}_{0}) \in a_{0} \; \text{for some}\; \mathfrak{h}_{0} \in \mathbb{R}\}$, then $a_{0} \perp b$ for all $b \in \mathcal{H}$. Proceeding this way, we get

Then $(\mathcal{H}, \perp)$ is an $\mathbb{O}$-set.

Example 2.3. ^[8] Let $(\mathcal{H}, \wp)$ be a metric space and $\mathcal{P} : \mathcal{H} \to \mathcal{H}$ be a Picard operator, that is, $\mathcal{H}$ has a unique fixed point $\mathfrak{h}^{*} \in \mathcal{H}$ and $\lim\limits_{n \to \infty} \mathcal{P}^{n}(\mathfrak{k}) = \mathfrak{h}^{*}\; \text{for all} \; \mathfrak{k} \in \mathcal{H}$. We define the binary relation $\perp$ on $\mathcal{H}$ by $\mathfrak{h} \perp \mathfrak{k}$ if

Then $(\mathcal{H}, \perp)$ is an $\mathbb{O}$-set.

Definition 2.4. ^[8] Let $(\mathcal{H}, \perp)$ be an $\mathbb{O}$-set. A sequence $\{\mathfrak{h}_n\}_{n\in \mathbb{N}}$ is called an orthogonal sequence (shortly, $\mathbb{O}$-sequence) if

Example 2.5. Let $\mathcal{H} = \mathbb{R}$ and suppose that $\mathfrak{h} \perp \mathfrak{k}$ if

for some $n \in \mathbb{Z}$ or $\mathfrak{h} = 0$.

It is easy to see that $(\mathcal{H}, \perp)$ is an $\mathbb{O}$-set. Define $\mathcal{P} : \mathcal{H} \to \mathcal{H} \; \; by\; \; \mathcal{P}(\mathfrak{h}) = [\mathfrak{h}]$. Then $\mathcal{P}$ is $\perp$ continuous on $\mathcal{H}$. Because if $\{\mathfrak{h}_{n}\}$ is an arbitrary $\mathbb{O}$-sequence in $\mathcal{H}$ such that $\{\mathfrak{h}_{n}\}$ converges to $\mathfrak{h} \in \mathcal{H}$, then the below cases hold:

Case 1: If $\mathfrak{h}_k = 0$ for all $k$, then $\mathfrak{h} = 0$ and $\mathcal{P}(\mathfrak{h}_k) = 0 = \mathcal{P}(\mathfrak{h})$.

Case 2: If $\mathfrak{h}_{k_0} \neq 0$ for some $k_0$, then there exists $m \in \mathbb{Z}$ such that $\mathfrak{h}_k \in (m + \frac{1}{5}, m + \frac{2}{5})$ for all $k \geq k_0$. Thus $\mathfrak{h} \in [m + \frac{1}{5}, m + \frac{2}{5}]$ and $\mathcal{P}(\mathfrak{h}_k) = m = \mathcal{P}(\mathfrak{h})$.

This means that $\mathcal{P}$ is $\perp$-continuous on $\mathcal{H}$ while it is not continuous on $\mathcal{H}$.

Definition 2.6. ^[8] Let $(\mathcal{H}, \perp, \wp)$ be an orthogonal set with the metric $\wp$. Then $\mathcal{H}$ is called an orthogonal complete (shortly, $\mathbb{O}$-complete) if every Cauchy $\mathbb{O}$-sequence is convergent.

Example 2.7. Let $\mathcal{H} = [0, 1)$ and suppose that

Then $(\mathcal{H}, \perp)$ is an O-set. Clearly, $\mathcal{H}$ with the Euclidian metric is not complete metric space, but it is $\mathbb{O}$-complete. In fact, if $\{x_k\}$ is an arbitrary Cauchy $\mathbb{O}$-sequence in $\mathcal{H}$, then there exists a subsequence $\{\mathfrak{h}_{k_n}\}$ of $\{\mathfrak{h}_k\}$ for which $\{\mathfrak{h}_{k_n}\} = 0$ for all $n \geq 1$ or there exists a monotone subsequence $\{\mathfrak{h}_{k_n}\}$ of $\{\mathfrak{h}_k\}$ for which $\{\mathfrak{h}_{k_n}\} \leq \frac{1}{5}$ for all $n \geq 1$. It follows that $\{\mathfrak{h}_{k_n}\}$ converges to a point $\mathfrak{h} \in [0, \frac{1}{5}] \subset \mathcal{H}$.

Definition 2.8. ^[8] Let $(\mathcal{H}, \perp \wp)$ be an orthogonal metric space and $0 < \lambda < 1$. A mapping $\mathcal{P} : \mathcal{H} \to \mathcal{H}$ is called an orthogonal contraction (shortly $\mathbb{O}$-contraction) with Lipschitz constant $\lambda$ if, $\forall\; \; \mathfrak{h}, \mathfrak{k} \in \mathcal{H}$ with $\mathfrak{h} \perp \mathfrak{k}$,

It is verify that every contraction is $\mathbb{O}$-contraction, but the converse is not true. See the following example:

Example 2.9. ^[8] Let $\mathcal{H} = [0, 1)$ and let the metric $\mathbb{H}$ on $\mathcal{H}$ be the euclidian metric. Define $\mathfrak{h} \perp \mathfrak{k}$ if $\mathfrak{h}, \mathfrak{k} \in \{\mathfrak{h}, \mathfrak{k}\}$, for all $\mathfrak{h}, \mathfrak{k} \in \mathcal{H}$. Let $\mathcal{P} : \mathcal{H} \to \mathcal{H}$ be a mapping defined by

Then, it is easy to show that $\mathcal{P}$ is an $\mathbb{O}$-contraction on $\mathcal{H}$, but it is not a contraction.

Definition 2.10. ^[8] Let $(\mathcal{H}, \perp)$ be an orthogonal metric space. A map $\mathcal{P} : \mathcal{H} \to \mathcal{H}$ is said to be $\perp$-preserving if $\mathcal{P}\mathfrak{h} \perp \mathcal{P}\mathfrak{k}$ whenever $\mathfrak{h} \perp \mathfrak{k}$.

3.   Main results

In this section, we present an $\mathbb{O}$-generalized $\mathfrak{F}$-contraction of type-$(1)$ and type-$(2)$ and prove fixed point theorem for an $\mathbb{O}$-generalized $\mathfrak{F}$-contraction of type-$(1)$ and type-$(2)$ maps in an $\mathbb{O}$-$\mathfrak{b}$-metric-like space.

Definition 3.1. Let $(\mathcal{H}, \perp, \wp)$ be an $\mathbb{O}$-$\mathfrak{b}$-metric-like space. A mapping $\mathcal{P} :\mathcal{H} \to \mathcal{H}$ is called an $\mathbb{O}$-generalized $\mathfrak{F}$-contraction of type-$(1)$ if $\exists\; \; \varrho > 0$ and $\mathfrak{F} \in \varLambda$(be a family of function) such that

where $\mathfrak{t}, \mathfrak{a}, \mathfrak{c}, \mathfrak{m}, \Im \in [0, 1]$ such that $\mathfrak{t}+\mathfrak{a}+\mathfrak{c}+\mathfrak{m}+\Im = 1$ and $1 - \mathfrak{m} - \mathfrak{c} > 0.$

Definition 3.2. Let $(\mathcal{H}, \perp, \wp)$ be an $\mathbb{O}$-$\mathfrak{b}$-metric-like space. A self-mapping $\mathcal{P} : \mathcal{H} \to \mathcal{H}$ is called an $\mathbb{O}$-generalized $\mathfrak{F}$-contraction of type-(2) if $\exists\; \; \varrho > 0$ and $\mathfrak{F} \in \varLambda$ such that

where $n \in [0, 1)\; \; \text{and}\; \; \mathfrak{t}, \mathfrak{a}, \mathfrak{m}, \Im \in [0, 1], \, such \; that \, \mathfrak{t}+\mathfrak{a}+\mathfrak{c}+\mathfrak{m}+\Im = 1, \, 1 - \mathfrak{c} - \mathfrak{m} > 0.$

Theorem 3.3. Let $(\mathcal{H}, \perp, \wp)$ be an $\mathbb{O}$-complete $\mathfrak{b}$-metric-like space with an orthogonal element $\mathfrak{h}_{0}$ and a map $\mathcal{P} : \mathcal{H} \to \mathcal{H}$ satisfying the following conditions:

(i) $\mathcal{P}$ is $\perp$ preserving,

(ii) $\mathcal{P}$ is an $\mathbb{O}$-generalized $\mathfrak{F}$-contraction of type-(1).

Then, $\mathcal{P}$ has a unique fixed point.

Proof. Since $(\mathcal{H}, \perp)$ is an $\mathbb{O}$-set,

It follows that $\mathfrak{h}_{0}\perp \mathcal{P}\mathfrak{h}_{0}$ or $\mathcal{P}\mathfrak{h}_{0}\perp \mathfrak{h}_{0}$. Let

for all $n\in \mathbb{N}\cup\{0\}$. If there exists $n_{0} \in \mathbb{N}$ such that $\wp(\mathfrak{h}_{n_{0}}, \mathfrak{h}_{n_{0} +1}) = 0$, then $\mathfrak{h} = \mathfrak{h}_{n_{0}}$ is the desired fixed point of $\mathcal{H}$ which completes the proof. Consequently, we suppose that $0 < \wp(\mathfrak{h}_{n}, \mathfrak{h}_{n +1})$ for all $n \in \mathbb{N}\cup\{0\}$. Since $\mathcal{H}$ is $\perp$-preserving, we have

This implies that $\{\mathfrak{h}_{n}\}$ is an $\mathbb{O}$-sequence. We have

By (3.1), we get

Now, we prove that

Suppose, on the contrary, that there exists $n_{0} \in \mathbb{N}$ such that $\wp(\mathfrak{h}_{n_{0}+1}, \mathcal{P}\mathfrak{h}_{n_{0}+1}) \geq \wp(\mathfrak{h}_{n_0}, \mathcal{P}\mathfrak{h}_{n_0})$, due to (3.6), we have

which yields

which together with $(\mathfrak{F}_{1})$ implies $\wp(\mathcal{P}\mathfrak{h}_{n_0}, \mathcal{P}^{2}\mathfrak{h}_{n_0}) < \wp(\mathcal{P}\mathfrak{h}_{n_0}, \mathfrak{h}_{n_0})$, that is, $\wp(\mathfrak{h}_{n_{0}+1}, \mathcal{P}\mathfrak{h}_{n_{0}+1}) < \wp(\mathcal{P}\mathfrak{h}_{n_0}, \mathfrak{h}_{n_0})$. It is a contradiction to $\wp(\mathfrak{h}_{n_{0}+1}, \mathcal{P}\mathfrak{h}_{n_{0}+1}) \geq \wp(\mathfrak{h}_{n_0}, \mathcal{P}\mathfrak{h}_{n_0})$, so (3.3) holds.

Therefore, $\{ \wp(\mathfrak{h}_{n}, \mathcal{P}\mathfrak{h}_{n})\}$ is a decreasing sequence of real numbers which is boundary below. Suppose that $\exists\; \; \mathbf{A} > 0$ such that

Now, we prove $\mathbf{A} = 0$. Suppose, conversely $\mathbf{A} > 0$. For every $\epsilon > 0$, there exists $\psi \in \mathbb{N}$ such that

By $(\mathfrak{F}_{1})$, we get

From (3.5), we get

Since $\mathcal{P}$ is an $\mathbb{O}$-generalized $\mathfrak{F}$-contraction of type-(1), we get

which implies

Taking

Since

from (3.1), we have

This yields

Continuing the above process and (3.8), we get

Letting $n \to +\infty$ in (3.10), we get $\lim\limits_{n \to +\infty} \mathfrak{F}(\wp(\mathcal{P}^{n}\mathfrak{h}_{\psi}, \mathcal{P}^{n+1}\mathfrak{h}_{\psi})) = -\infty$, which together with $(\mathfrak{F}_2)$ implies $\lim\limits_{n \to +\infty} \wp(\mathcal{P}^{n}\mathfrak{h}_{\psi}, {\mathcal{P}^{n+1}\mathfrak{h}_{\psi}}) = 0.$ So, $\exists \; \; \mathcal{N}_{1}\in\mathbb{N}$ such that $\wp(\mathcal{P}^{n}\mathfrak{h}_{\psi}, \mathcal{P}^{n+1}\mathfrak{h}_{\psi}) < \mathbf{A}, \; \forall\; n > \mathcal{N}_{1}$, that is, $\wp(\mathfrak{h}_{\psi+n}, \mathfrak{h}_{\psi+n}) < \mathbf{A}, \; \forall\; n > \mathcal{N}_{1}$, which is a contradiction of $\mathbf{A}$, therefore,

Now, we prove that

Suppose, conversely, $\exists\; \; \epsilon > 0$ and $\{p(n)\}$ and $\{q(n)\}$ of natural numbers such that

Applying the triangle inequality, we get

which implies that

Owing to (3.11), there exists $\mathcal{N}_{2} \in \mathbb{N}$ such that

which together with (3.14) shows

hence

From (3.13) and (3.15), we get

Using the triangle inequality, we have

Letting $n \to +\infty$ in (3.23), by (3.11), we obtain $\dfrac{\epsilon}{\mathfrak{s}^{2}} \leq\lim\limits_{n \to +\infty}\inf \wp(\mathfrak{h}_{p(n)+1}, \mathfrak{h}_{q(n)+1}),$ hence, there exists $\mathcal{N}_{3} \in \mathbb{N},$ such that $\wp(\mathfrak{h}_{p(n)+1}, \mathfrak{h}_{q(n)+1}) > 0$ for $n > \mathcal{N}_{3}$ that is, $\wp(\mathfrak{h}_{p(n)}, \mathfrak{h}_{q(n)}) > 0$ for $n > \mathcal{N}_{3}.$ By (1.1) and (3.17), we have

for $n > max \{\mathcal{N}_{2}, \mathcal{N}_{3}\}.$

Taking (3.15)–(3.17) into account, (3.20) yields

for $n > \max \{\mathcal{N}_{2}, \mathcal{N}_{3}\}.$

Letting $n \to +\infty$ in (3.21), we obtain

which yields $\lim\limits_{n, \to +\infty}\mathfrak{F}(\wp(\mathcal{P}\mathfrak{h}_{p(n)}, \mathcal{P}\mathfrak{h}_{q(n)}) = 0,$ which together with

shows $\lim\limits_{n, \to +\infty}\wp(\mathfrak{h}_{p(n)}, \mathfrak{h}_{q(n)}) = 0,$ which is contradiction to (3.13), so (3.5) holds, therefore $\{\mathfrak{h}_{n}\}$ is a Cauchy $\mathbb{O}$-sequence in $\mathcal{H}$. Since $(\mathcal{H}, \wp)$ is an $\mathbb{O}$-complete, there exists $\gamma \in \mathcal{H}$ such that

It is easy to prove the fact satisfies,

Suppose, conversely that there exists $\psi_{0} \in \mathbb{N}$ such that

By (3.3) and (3.24), we get

This is a contradiction. Hence (3.23) holds and there exists $\gamma \in \mathcal{H}$ such that

or

Now, we discuss the below cases.

Case 1: Suppose that (3.25) holds. From (3.25), we have

Owing to (3.11) and (3.22), for some $\epsilon_{0} > 0$, there exists $\mathcal{N}_{4} \in \mathbb{N}$ such that

for $\mathcal{N} > \mathcal{N}_{4}.$

With the help of (3.27) and (3.28), we get

for $\mathcal{N} > \mathcal{N}_{4}.$ Taking $n \to +\infty$ in the above equation, we have $\lim\limits_{n \to +\infty}\mathfrak{F}(\wp(\mathcal{P}\mathfrak{h}_{n}, \mathcal{P}\gamma)) = -\infty$ which yields

On the other hand, we have

By letting $n \to +\infty$ in the above inequality, by (3.22) and (3.29), we get $\wp(\gamma, \mathcal{P}\gamma) = 0,$ it means $\gamma = \mathcal{P}\gamma.$ Thus $\gamma$ is a fixed point of $\mathcal{P}$.

Case 2: Let (3.26) hold. From (3.26), we have

From (3.28) and (3.30) yield

for $\mathcal{N} > \mathcal{N}_{4}.$

Taking $n \to +\infty$ in the above equation, we get $\lim\limits_{n, \to +\infty}\mathfrak{F}(\wp(\mathcal{P}^{2}\mathfrak{h}_{n}, \mathcal{P}\gamma)) = -\infty$ which yields

On the other way, we have

By letting $n \to +\infty$ in the above inequality, by (3.22) and (3.31), we get $\wp(\gamma, \mathcal{P}\gamma) = 0,$ it means $\gamma = \mathcal{P}\gamma.$ Thus $\gamma$ is the fixed point of $\mathcal{P}$ and the proof is over.

Let $\mathcal{P}$ have two fixed points are $\mathfrak{h}, \mathfrak{k} \in \mathcal{H}$ and suppose that $\mathcal{P}^n \mathfrak{h} = \mathfrak{h} \neq \mathfrak{k} = \mathcal{P}^n \mathfrak{k}, \, \forall\, n \in \mathbb{N}$. By choice of $\mathfrak{h}_{0}$ we obtain

Since $\mathcal{H}$ is $\perp$- preserving, we have

Now

As $n \to \infty$, we obtain $\wp(\mathfrak{h}, \mathfrak{k}) \leq 0$. Thus $\mathfrak{h} = \mathfrak{k}$. Hence $\mathcal{P}$ has a unique fixed point.

Theorem 3.4. Let $(\mathcal{H}, \wp)$ be an $\mathbb{O}$-complete $\mathfrak{b}$-metric-like space and a map $\mathcal{P} : \mathcal{H} \to \mathcal{H}$ satisfying the following conditions:

(i) $\mathcal{P}$ is $\perp$ preserving,

(ii) $\mathcal{P}$ is an $\mathbb{O}$-generalized $\mathfrak{F}$-contraction of type-(2),

(iii) if $\wp(\mathcal{P}\mathfrak{h}, \mathcal{P}\mathfrak{h}) \leq\wp(\mathfrak{h}, \mathfrak{h})$.

Then $\mathcal{P}$ has a unique fixed point.

Proof. As in the proof of Theorem 3.3, choosing $\mathfrak{h}_{0}\in \mathcal{H}$, we construct sequence $\{\mathfrak{h}_{n}\}$ by $\mathfrak{h}_{n} = \mathcal{P}\mathfrak{h}_{n} = \mathcal{P}^{n}\mathfrak{h}_{0}$ and we can suppose

From (3.31) and (3.2), we have

We claim

Suppose, conversely that $\exists\; \; n_{0} \in \mathbb{N}$ such that $\wp(\mathfrak{h}_{n_{0}}, \mathcal{P}\mathfrak{h}_{n_{0}}) \geq \wp(\mathfrak{h}_{n_{0}-1}, \mathcal{P}\mathfrak{h}_{n_{0}-1}),$ which together with (3.32) yields

By (3.35) which implies that

which shows

Applying (3.36) and $\mathfrak{F}(1)$, we have $\wp(\mathfrak{h}_{n_{0}}, \mathcal{P}\mathfrak{h}_{n_{0}}) < \wp(\mathfrak{h}_{n_{0}-1}, \mathcal{P}\mathfrak{h}_{n_{0}-1})$, this is a contradiction. Hence, (3.34) holds.

Applying(3.2) and (3.34), we obtain

which yields

Continuing this process, we get

Letting $n \to +\infty$, (3.37) shows $\lim\limits_{n \to +\infty}\mathfrak{F}(\wp(\mathfrak{h}_{n}, \mathcal{P}\mathfrak{h}_{n})) = -\infty$, hence

Now, we prove

Suppose, conversely, $\exists\; \; \epsilon > 0$ and sequences $\{p(n)\}$ and $\{q(n)\}$ of natural numbers such that

Applying the triangle inequality, we get

which implies that

Owing to (3.38), there exists $\mathcal{N}_{1} \in \mathbb{N}$ such that

which together with (3.41) shows

hence

From (3.40), we get

which together with (3.2) yields

for all $n > \mathcal{N}_{1}.$

Taking (3.42)–(3.44) into account, (3.45) yields

Taking $n \to +\infty$ in (3.46), we get

which yields $\lim\limits_{n\to+\infty}(\wp(\mathcal{P}\mathfrak{h}_{p(n)-1}, \mathcal{P}\mathfrak{h}_{q(n)-1})) = 0,$ by $\mathfrak{F}(2),$ that is, $\lim\limits_{n\to+\infty}\wp(\mathfrak{h}_{p(n)}, \mathfrak{h}_{q(n)}) = 0$, which is contradiction to (3.40), so (3.39) holds, therefore, $\{\mathfrak{h}_{n}\}$ is a Cauchy $\mathbb{O}$-sequence in $\mathcal{H}$. Since $(\mathcal{H}, \wp)$ is an $\mathbb{O}$-complete, there exists $\gamma \in \mathcal{H}$ such that

Since $\mathcal{P}$ is $\mathbb{O}$-continuous, we have

Due to $\wp(\mathcal{P}\gamma, \mathcal{P}\gamma) \leq\wp(\gamma, \gamma),$ from (3.47) and (3.48), we have

Since $\wp(\gamma, \mathcal{P}\gamma)\leq\wp(\gamma, \mathfrak{h}_{n}) + \wp(\mathfrak{h}_{n}, \mathcal{P}\gamma)$, by (3.49), we get $\wp(\gamma, \mathcal{P}\gamma) = 0$, which gives $\gamma = \mathcal{P}\gamma$, therefore, $\mathcal{P}$ has a fixed point.

Let $\mathfrak{h}, \mathfrak{k} \in \mathcal{H}$ be two fixed point of $\mathcal{P}$ and suppose that $\mathcal{P}^n \mathfrak{h} = \mathfrak{h} \neq \mathfrak{k} = \mathcal{P}^n \mathfrak{k}, \, \forall n \in \mathbb{N}$. By choice of $\mathfrak{h}_{0} \in \mathcal{H}$ we obtain

Since $\mathcal{H}$ is $\perp$- preserving, we have

Now

As $n \to \infty$, we get $\wp(\mathfrak{h}, \mathfrak{k}) \leq 0$. Thus $\mathfrak{h} = \mathfrak{k}$. Hence, $\mathcal{P}$ has a unique fixed point.

4.   Application to an integral equation

Let $\mathcal{H} = [0, \mathtt{D}]$. Let $\mathtt{Q} = C(\mathcal{H}, \mathbb{R})$ be the real valued continuous functions with $\mathcal{H}$. Consider the following equation

where

(a) $\Omega: \mathcal{H}\times\mathbb{R}\rightarrow \mathbb{R}$ is continuous;

(b) $\rightthreetimes:\mathcal{H}\times \mathcal{H}$ is continuous and measurable at $\beta\in\mathcal{H}$, $\forall \; \mathtt{Q}\in \mathcal{H}$;

(c) $\rightthreetimes(\mathtt{Q}, \beta)\geq 0$, $\forall \; \mathtt{Q}, \beta\in \mathcal{H}$ and $\int_{0}^{\mathtt{D}}\rightthreetimes(\mathtt{Q}, \beta) d\beta\leq 1$, $\forall \; \mathtt{Q}\in \mathcal{H}$.

Theorem 4.1. Assume that the conditions $(a)-(c)$ hold. Suppose that there exists $\iota > 0$ such that

for every $\mathtt{Q}\in \mathcal{H}$ and $\forall \, \, \zeta, \xi\in C(\mathcal{H}, \mathbb{R})$. Then (4.1) has a unique solution in $C(\mathcal{H}, \mathbb{R})$.

Proof. Let $\mathtt{Q} = \{w\in C(\mathcal{H}, \mathbb{R}): w(\mathfrak{h}) > 0, \; \forall \; \mathfrak{h}\in \mathcal{H} \}$. Define the orthogonal relation $\perp$ on $\mathtt{Q}$ by

Define a function $\wp: \mathtt{Q}\times \mathtt{Q}\rightarrow [0, \infty)$ by

$\forall\; \zeta, \xi\in\mathtt{Q}$. Thus, $(\mathtt{Q}, \wp)$ is a $\mathbb{O}$-$\mathfrak{b}$-metric-like space and also a $\mathbb{O}$-complete $\mathbb{O}$-$\mathfrak{b}$-metric-like space. Define $D: C(\mathcal{H}, \mathbb{R})\rightarrow C(\mathcal{H}, \mathbb{R})$ by

Now, we show that $\mathtt{Q}$ is $\perp$-preserving. For each $\zeta, \xi\in \mathtt{Q}$ with $\zeta\perp \xi$ and $\mathfrak{h}\in I$, we have

It follows that $[(D\zeta)(\mathfrak{h})][(D\xi)(\mathfrak{h})]\geq (D\xi)(\mathfrak{h})$ and so $(D\zeta)(\mathfrak{h})\perp (D\xi)(\mathfrak{h})$. Then, $\mathtt{Q}$ is $\perp$-preserving.

Now, to show that $\mathtt{Q}$ is $\mathbb{O}$-generalized $\mathfrak{F}$-contraction of type-(1). Let $\zeta, \xi\in \mathtt{Q}$ with $\zeta\perp \xi$. Suppose that $\mathtt{D}(\zeta)\neq \mathtt{D}(\xi)$. For every $\zeta\in [0, \mathtt{D}]$, we have

Therefore,

Letting $\mathfrak{F}(\mathtt{Q}) = \ln(\mathtt{Q})$, we get

for all $\zeta, \xi\in\mathtt{Q}$. Therefore, by Theorem 3.3, $\mathtt{Q}$ has a unique fixed point. Hence, there is a unique solution for (4.1).

5.   Conclusions

In this paper, we proved fixed point theorems for an $\mathbb{O}$-generalized $\mathfrak{F}$-contraction of types in an $\mathbb{O}$-complete $\mathfrak{b}$-metric like space. We also given an example to manifest the authenticity of the obtained results. As application of our main results, we looked into the solution to the integral equation.

Acknowledgements

The authors S. Haque and N. Mlaiki would like to thank Prince Sultan University for paying the publication fees for this work through TAS LAB.

Conflict of interest

The authors declare no conflicts of interest.